On Generalized Class of Bell Polynomials Associated with Geometric Applications
Abstract
1. Introduction
- (i)
- If and have differential realizations, then the polynomials satisfy the differential equation
- (ii)
- Assuming that , then the polynomials can be explicitly constructed aswhich gives the series definition of .
- (iii)
- In view of identity (14), the exponential generating function of can be written in the form
2. Generalized Bell Polynomials
3. Differential and Integral Formulas
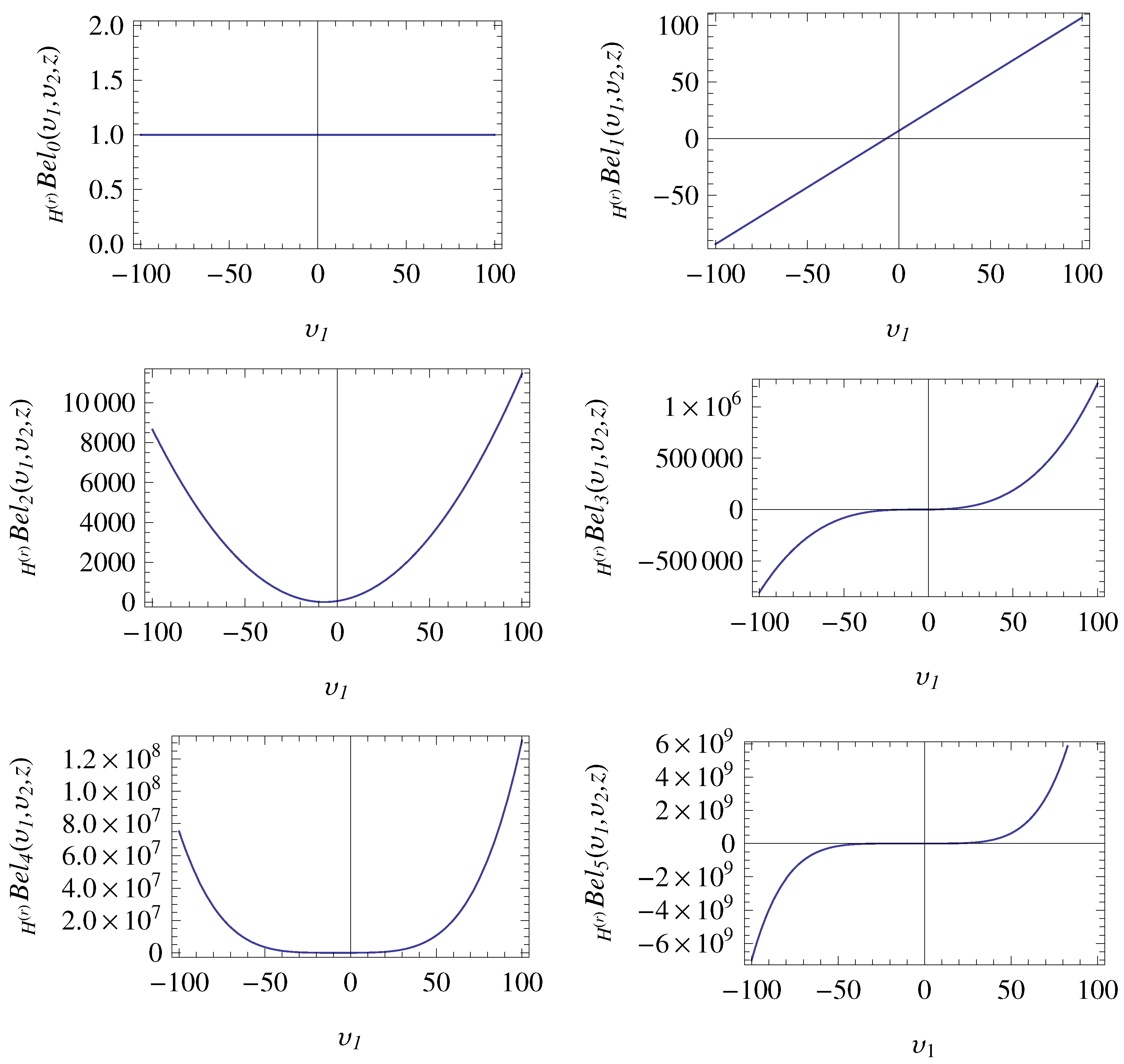
4. Special Members
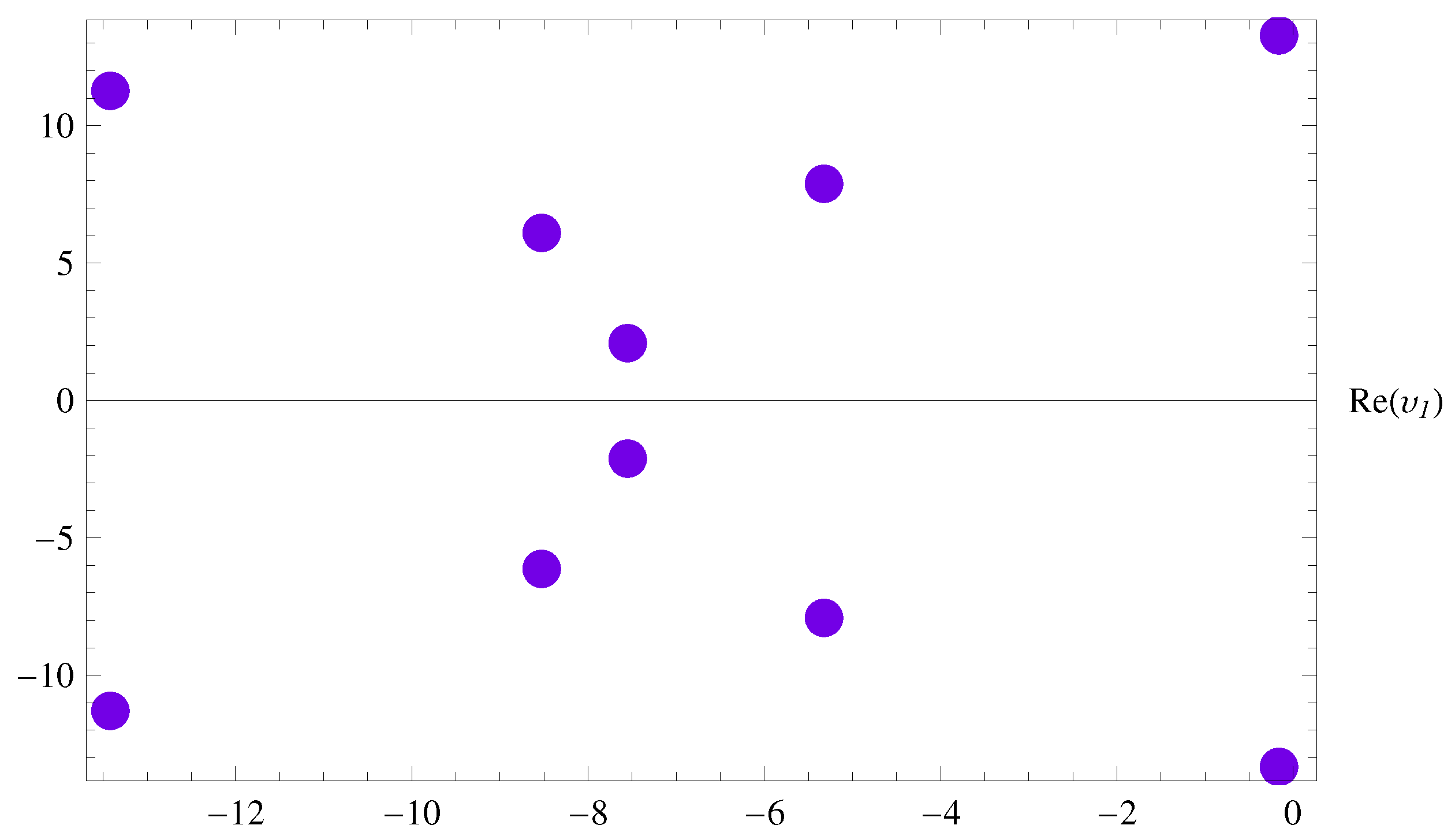
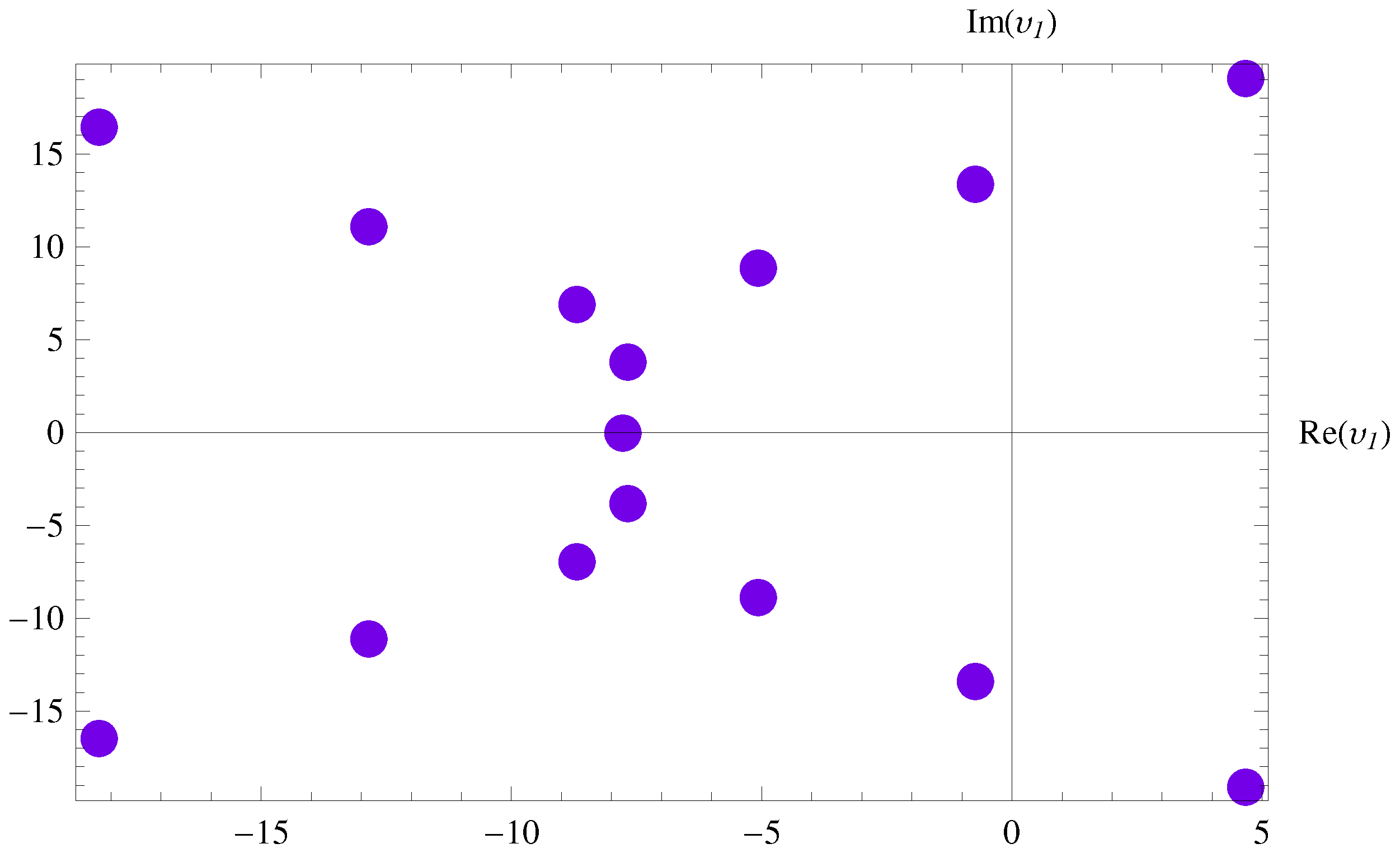
5. Applications in Computer Modeling
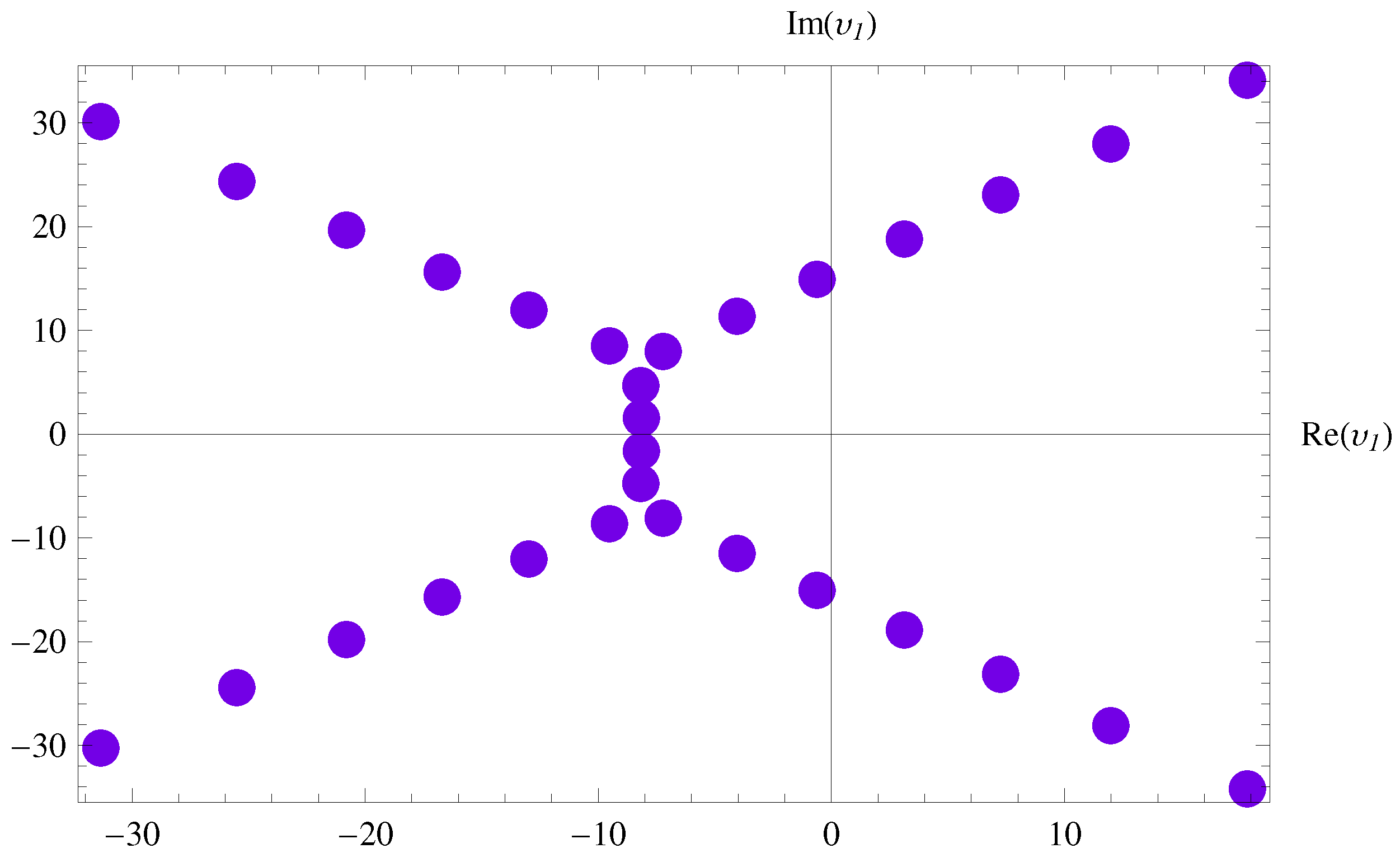
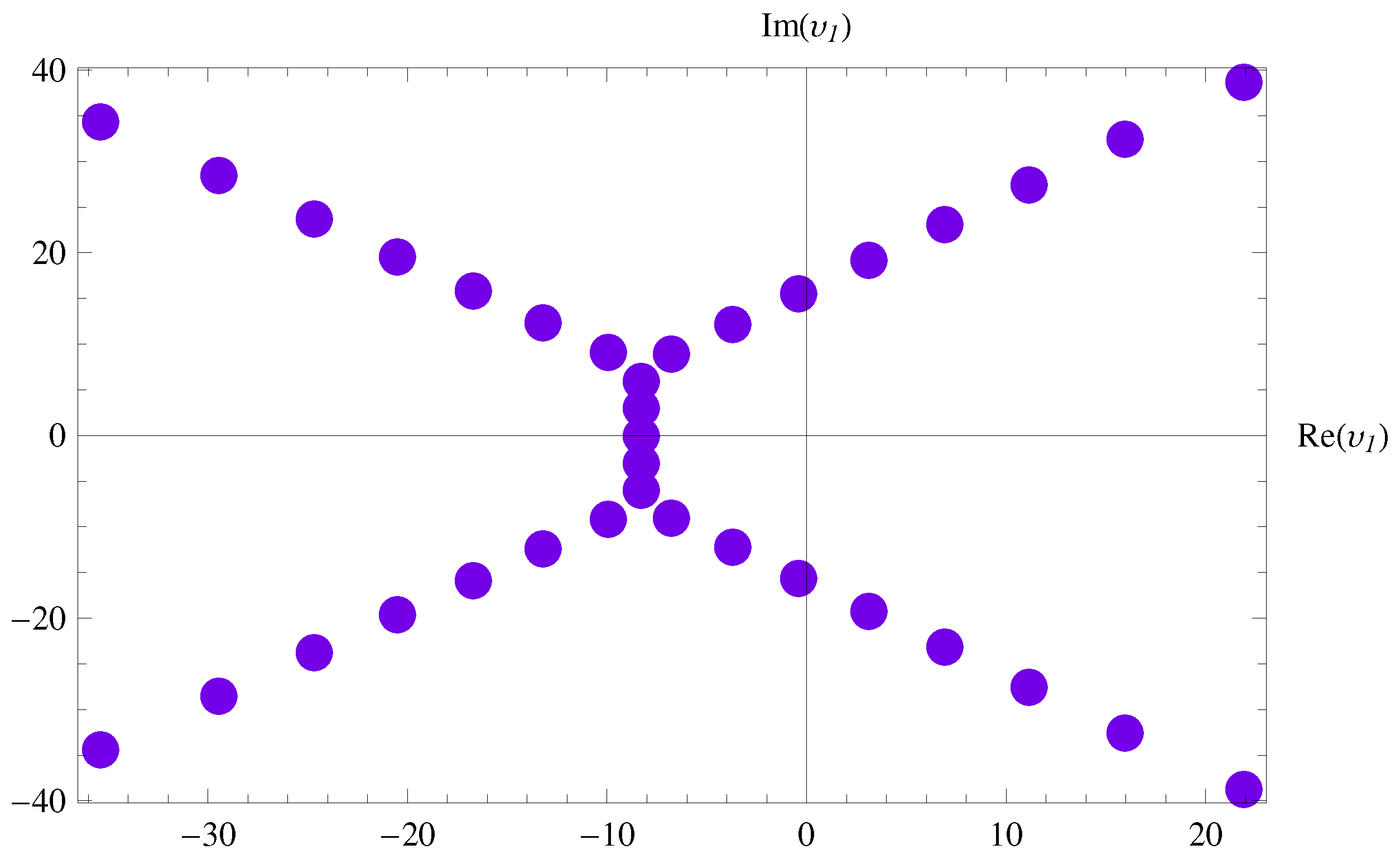
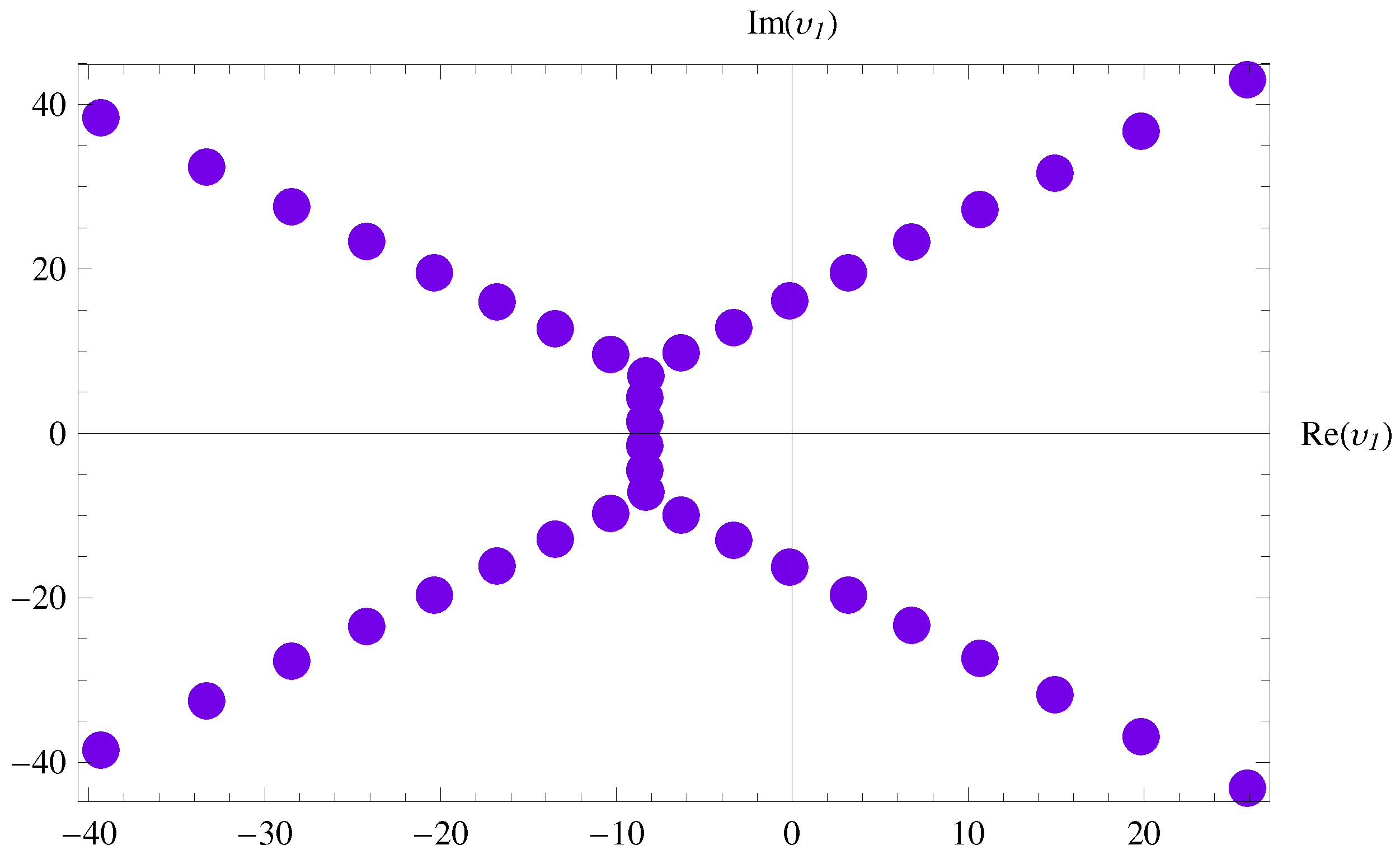
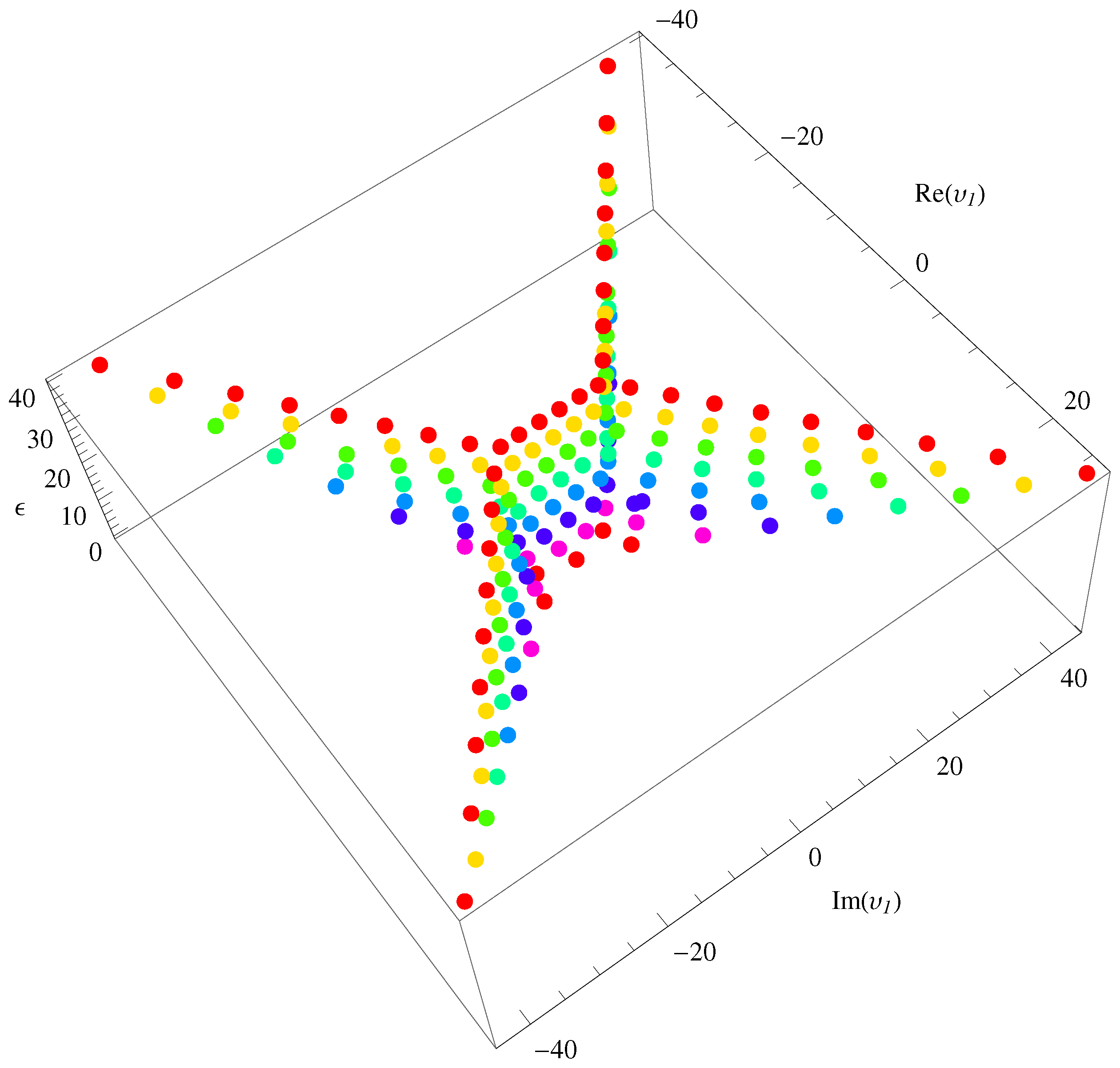
- 1.
- If ε is odd, the GHBelP has one real zero and complex zeros.
- 2.
- If ε is even, the GHBelP has ε complex zeros.
- 3.
- The zeros of the GHBelP are symmetric with respect to the real axis.
6. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bell, E.T. Exponential polynomials. Ann. Math. 1934, 35, 258–277. [Google Scholar] [CrossRef]
- Benbernou, S.; Gala, S.; Ragusa, M.A. On the regularity criteria for the 3D magnetohydrodynamic equations via two components in terms of BMO space. Math. Methods Appl. Sci. 2014, 37, 2320–2325. [Google Scholar] [CrossRef]
- Boas, R.B.; Buck, R.C. Polynomial Expansions of Analytic Functions; Springer: Berlin, Germany, 2013. [Google Scholar]
- Sándor, J.; Crstici, B. Handbook of Number Theory; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2004; Volume II. [Google Scholar]
- Gao, X.-Y.; Guo, Y.-J.; Shan, W.-R. Beholding the shallow water waves near an ocean beach or in a lake via a Boussinesq-Burgers system. Chaos Soliton. Fract. 2021, 147, 110875. [Google Scholar] [CrossRef]
- Gao, X.-Y.; Guo, Y.-J.; Shan, W.-R. Bilinear forms through the binary Bell polynomials, N solitons and Bäcklund transformations of the Boussinesq–Burgers system for the shallow water waves in a lake or near an ocean beach. Commun. Theor. Phys. 2020, 72, 095002. [Google Scholar] [CrossRef]
- Gao, X.-Y.; Guo, Y.-J.; Shan, W.-R. Scaling transformation, hetero-Backlund transformation and similarity reduction on a (2+1)-dimensional generalized variable-coefficient Boiti-Leon-Pempinelli system for water waves. Rom. Rep. Phys. 2021, 73, 111. [Google Scholar]
- Gao, X.-Y.; Guo, Y.-J.; Shan, W.-R. Looking at an open sea via a generalized (2+1)-dimensional dispersive long-wave system for the shallow water: Scaling transformations, hetero-Bäcklund transformations, bilinear forms and N solitons. Eur. Phys. J. Plus 2021, 136, 893. [Google Scholar] [CrossRef]
- Li, J.-C.; Nie, B.-C. A few frontier issues in ocean engineering mechanics. China Ocean Eng. 2021, 35, 1–11. [Google Scholar] [CrossRef]
- Duran, U.; Araci, S.; Acikgoz, M. Bell-based Bernoulli polynomials with applications. Axioms 2021, 10, 29. [Google Scholar] [CrossRef]
- Duran, U.; Acikgoz, M. Bell-based Genocchi polynomials. In Proceedings of the International Conference on Applied Analysis and Mathematical Modeling (ICAAMM21), Istanbul, Turkey, 11–13 June 2021. [Google Scholar]
- Carlitz, L. Some remarks on the Bell numbers. Fibonacci Quart. 1980, 18, 66–73. [Google Scholar]
- Kim, D.S.; Kim, T. Some identities of Bell polynomials. Sci. China Math. 2015, 58, 2095–2104. [Google Scholar] [CrossRef]
- Kim, T.; Kim, D.S.; Dolgy, V.; Kim, H.K.; Lee, H. A new approach to Bell and poly-Bell numbers and polynomials. AIMS Math. 2021, 7, 4004–4016. [Google Scholar] [CrossRef]
- Khan, S.; Raza, N. General-Appell polynomials within the context of monomiality principle. Int. J. Anal. 2013, 3013, 328032. [Google Scholar] [CrossRef]
- Steffensen, J.F. The poweriod, an extension of the mathematical notion of power. Acta Math. 1941, 73, 333–366. [Google Scholar] [CrossRef]
- Dattoli, G. Hermite–Bessel and Laguerre–Bessel functions: A by-product of the monomiality principle. In Advanced Special Functions and Applications, Proceedings of the First Melfi School on Advanced Topics in Mathematics and Physics, Melfi, Italy, 9–12 May 1999; Aracne: Rome, Italy, 2000; Volume 1, pp. 147–164. [Google Scholar]
- Gould, H.W.; Hopper, A.T. Operational formulas connected with two generalizations of Hermite polynomials. Duke. Math. J. 1962, 29, 51–63. [Google Scholar] [CrossRef]
- Cocolicchio, D.; Dattoli, G.; Srivastava, H.M. Advanced Special Functions and Applications. In Proceedings of the First Melfi School on Advanced Topics in Mathematics and Physics, Melfi, Italy, 9–12 May 1999; Aracne Editrice: Rome, Italy, 2000. [Google Scholar]
- Dattoli, G.; Migliorati, M.; Srivastava, H.M. A class of Bessel summation formulas and associated operational methods. Fract. Calc. Appl. Anal. 2004, 7, 169–176. [Google Scholar]
- Khan, S.; Yasmin, G.; Khan, R.; Hassan, N.A.M. Hermite-based Appell polynomials: Properties and applications. J. Math. Anal. Appl. 2009, 351, 756–764. [Google Scholar] [CrossRef]
- Kilar, N.; Simsek, Y. A new family of Fubini type numbers and polynomials associated with Apostol-Bernoulli numbers and polynomials. J. Korean Math. Soc. 2017, 54, 1605–1621. [Google Scholar]
- Srivastava, H.M.; Srivastava, R.; Muhyi, A.; Yasmin, G.; Islahi, H.; Araci, S. Construction of a new family of Fubini-type polynomials and its applications. Adv. Differ. Equ. 2021, 2021, 36. [Google Scholar] [CrossRef]
- Khan, S.; Raza, N.; Ali, M. Finding mixed families of special polynomials associated with Appell sequences. J. Math. Anal. Appl. 2017, 447, 398–418. [Google Scholar] [CrossRef]
- Kim, T.; Kim, D.S.; Kim, H.Y.; Kwon, J. Some identities of degenerate Bell polynomials. Mathematics 2020, 8, 40. [Google Scholar] [CrossRef]
- Muhyi, A. A new class of Gould-Hopper-Eulerian-type polynomials. Appl. Math. Sci. Eng. 2022, 30, 283–306. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Araci, S.; Khan, W.A.; Acikgoz, M. A note on the truncated-exponential based Apostol-type polynomials. Symmetry 2019, 11, 538. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Özarslan, M.A.; Yılmaz, B. Some families of differential equations associated with the Hermite-based Appell polynomials and other classes of Hermite-based polynomials. Filomat 2014, 28, 695–708. [Google Scholar] [CrossRef]
- Yasmin, G.; Muhyi, A. Certain results of hybrid families of special polynomials associated with Appell sequences. Filomat 2019, 33, 3833–3844. [Google Scholar] [CrossRef]
- Yasmin, G. Some properties of Legendre–Gould Hopper polynomials and operational methods. J. Math. Anal. Appl. 2014, 413, 84–99. [Google Scholar] [CrossRef]
- Khan, N.; Husain, S. Analysis of Bell Based Euler Polynomials and Their Application. Int. J. Appl. Comput. Math. 2021, 7, 195. [Google Scholar] [CrossRef]
- Kim, T.; Kim, D.S.; Kwon, H.-I.; Rim, S.-H. Some identities for umbral calculus associated with partially degenerate Bell numbers and polynomials. J. Nonlinear Sci. Appl. 2017, 10, 2966–2975. [Google Scholar] [CrossRef]
- Rainville, E.D. Special Functions; Reprint of 1960 First Edition; Chelsea Publishing Co.: Bronx, NY, USA, 1971. [Google Scholar]

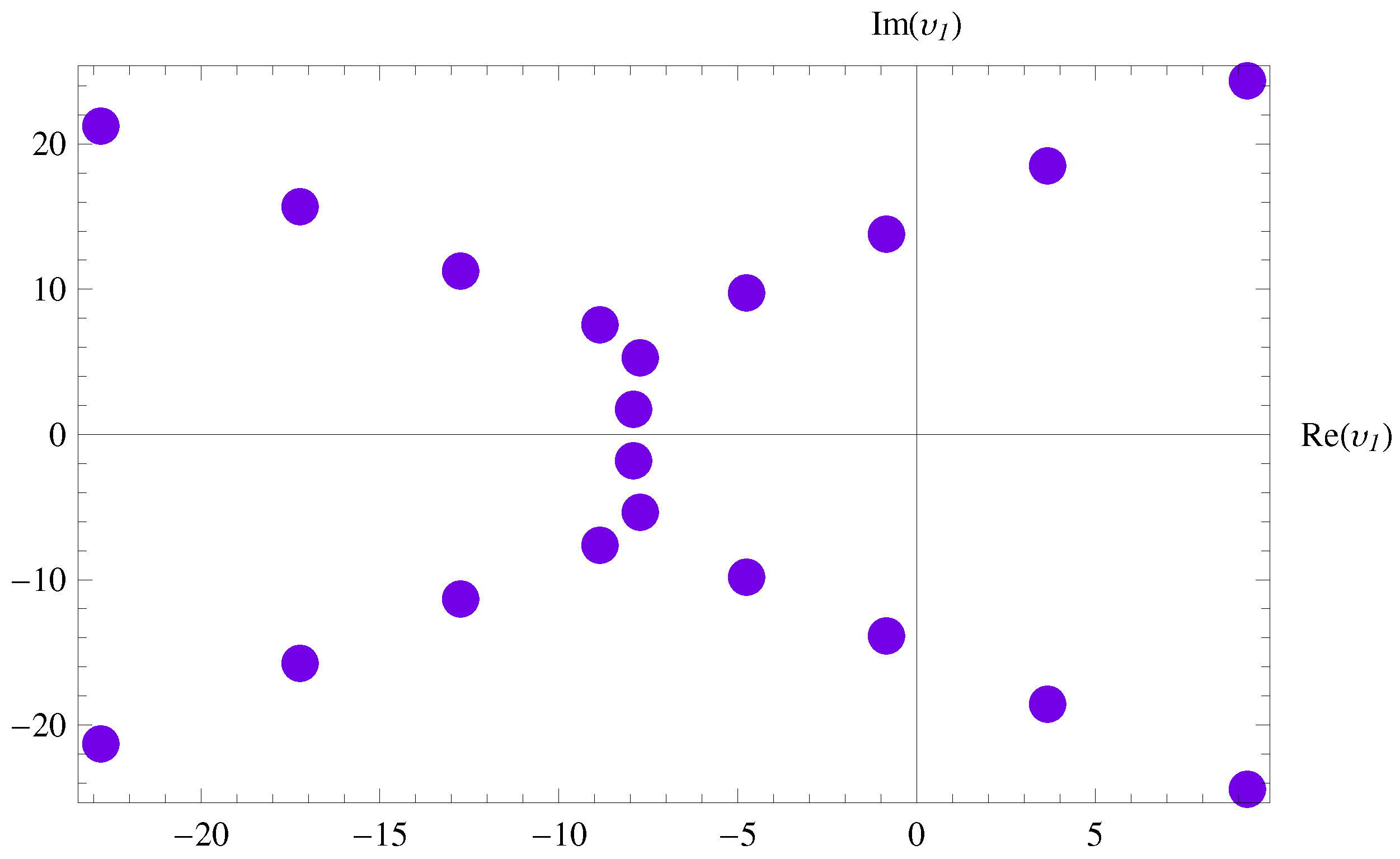

| Multiplicative and | |
| derivative operators | |
| Differential equation | |
| Identities and | |
| relations | |
| Differential and | |
| Integral Formulas | |
| Multiplicative and | |
| derivative operators | |
| Differential equation | |
| Identities and | |
| relations | |
| Differential and | |
| Integral Formulas | |
| Multiplicative and | |
| derivative operators | |
| Differential equation | |
| Identities and | |
| relations | |
| Differential and | |
| Integral Formulas | |
| Multiplicative and | |
| derivative operators | |
| Differential equation | |
| Identities and | |
| relations | |
| Differential and | |
| Integral Formulas | |
| Multiplicative and | |
| derivative operators | |
| Differential equation | |
| Identities and | |
| relations | |
| Differential and | |
| Integral Formulas | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al-Jawfi, R.A.; Muhyi, A.; Al-shameri, W.F.H. On Generalized Class of Bell Polynomials Associated with Geometric Applications. Axioms 2024, 13, 73. https://doi.org/10.3390/axioms13020073
Al-Jawfi RA, Muhyi A, Al-shameri WFH. On Generalized Class of Bell Polynomials Associated with Geometric Applications. Axioms. 2024; 13(2):73. https://doi.org/10.3390/axioms13020073
Chicago/Turabian StyleAl-Jawfi, Rashad A., Abdulghani Muhyi, and Wadia Faid Hassan Al-shameri. 2024. "On Generalized Class of Bell Polynomials Associated with Geometric Applications" Axioms 13, no. 2: 73. https://doi.org/10.3390/axioms13020073
APA StyleAl-Jawfi, R. A., Muhyi, A., & Al-shameri, W. F. H. (2024). On Generalized Class of Bell Polynomials Associated with Geometric Applications. Axioms, 13(2), 73. https://doi.org/10.3390/axioms13020073









