On the Structure of Quantum Markov Chains on Cayley Trees Associated with Open Quantum Random Walks
Abstract
1. Introduction
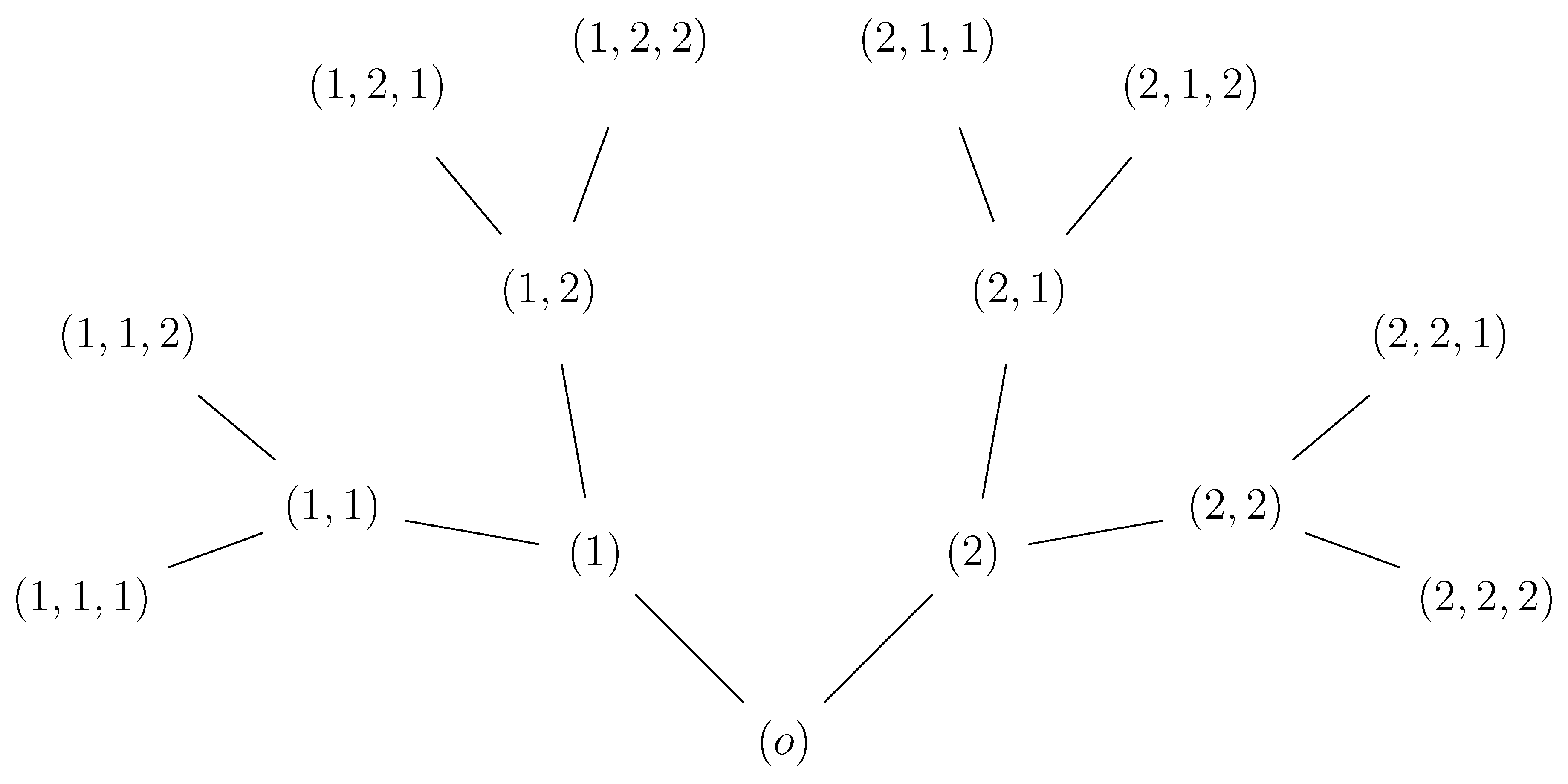
2. Preliminaries on Trees
3. Quantum Markov Chains on Trees
- A quasi-conditional expectation [51] is a linear map that is completely positive and identity-preserving, satisfying the condition for all and .
- A (Markov) transition expectation is a linear map between two unitary -algebras that is completely positive and identity-preserving.
- is the initial state;
- For each n, the map is a transition expectation from into ;
- For each n, is a positive boundary condition.
4. Main Result
5. Classical Probability Associated with OQRW
6. Application to OQRW on
6.1. Example 1
6.2. Example 2
7. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Accardi, L. Noncommutative Markov chains. In Proceedings of the International School of Mathematical Physics, Camerino, Italy, 30 September–12 October 1974; pp. 268–295. [Google Scholar]
- Accardi, L. On noncommutative Markov property. Funct. Anal. Appl. 1975, 8, 1–8. [Google Scholar] [CrossRef]
- Accardi, L.; Frigerio, A. Markovian cocycles. Proc. R. Ir. Acad. 1983, 83A, 251–263. [Google Scholar]
- Accardi, L.; Khrennikov, A.; Ohya, M. Quantum Markov Model for Data from Shafir—Tversky Experiments in Cognitive Psychology. Open Syst. Inf. Dyn. 2009, 16, 371–385. [Google Scholar] [CrossRef]
- Accardi, L.; Souissi, A.; Soueidy, E.G. Quantum Markov chains: A unification approach. Infin. Dimens. Anal. Quantum Probab. Relat. Top. 2020, 23, 2050016. [Google Scholar] [CrossRef]
- Gudder, S. Quantum Markov chains. J. Math. Phys. 2008, 49, 072105. [Google Scholar] [CrossRef]
- Lardizabal, C.F. A quantization procedure based on completely positive maps and Markov operators. Quantum Inf. Process. 2013, 12, 1033–1051. [Google Scholar] [CrossRef]
- Liu, C.; Petulante, N. On Limiting distributions of quantum Markov chains. Int. J. Math. Math. Sci. 2011, 2011, 740816. [Google Scholar] [CrossRef]
- Dhahri, A.; Fagnola, F. Potential theory for quantum Markov states and other quantum Markov chains. Anal. Math. Phys. 2023, 13, 31. [Google Scholar] [CrossRef]
- Fannes, M.; Nachtergaele, B.; Werner, R.F. Finitely correlated states on quantum spin chains. Commun. Math. Phys. 1992, 144, 443–490. [Google Scholar] [CrossRef]
- Feng, Y.; Yu, N.; Ying, M. Model checking quantum Markov chains. J. Comput. Syst. Sci. 2013, 79, 1181–1198. [Google Scholar] [CrossRef]
- Kümmerer, B. Quantum Markov processes and applications in physics. In Quantum Independent Increment Processes II; Lecture Notes in Mathematics; Springer: Berlin, Germany, 2006; Volume 1866, pp. 259–330. [Google Scholar]
- Abundo, M.; Accardi, L.; Rosato, N. A Markovian model for cooperative interactions in proteins. Math. Models Methods Appl. Sci. 1995, 6, 5. [Google Scholar] [CrossRef]
- Abundo, M.; Accardi, L.; Finazzi; Agrò, A.; Mei, G.; Rosato, N. A stochastic model for the sigmoidal behaviour of cooperative biological systems. Biophys. Chem. 1996, 58, 313–323. [Google Scholar] [CrossRef] [PubMed]
- Abundo, M.; Accardi, L.; Stella, L.; Rosato, N. A stochastic model for the cooperative relaxation of proteins, based on a hierarchy of interactions between amino acid residues. Math. Models Methods Appl. Sci. 1998, 8, 327–358. [Google Scholar] [CrossRef]
- Accardi, L.; Abundo, M. A stochastic model for the cooperative behaviour of biological systems. Amino Acids 1999, 17, 120–121. [Google Scholar]
- Accardi, L.; Abundo, M.; Rosato, N.; Stella, L. Analysing protein energy data by a stochastic model for cooperative interactions: Comparison and characterization of cooperativity. J. Math. Biol. 2002, 44, 341–359. [Google Scholar]
- Dobrushin, R.L. The description of a random field by means of conditional probabilities and conditions of its regularity. Theor. Probab. Appl. 1968, 13, 197–224. [Google Scholar] [CrossRef]
- Accardi, L.; Fidaleo, F. Quantum Markov fields. Infin. Dimens. Anal. Quantum Probab. Relat. Top. 2003, 6, 123–138. [Google Scholar] [CrossRef]
- Liebscher, V. Markovianity of quantum random fields. In Quantum Probability and Infinite-Dimensional Analysis, Proceedings of the Burg Conference, Burg, Germany, 15–20 March 2001; Freudenberg, W., Ed.; QP-PQ Series; World Scientific: Singapore, 2003; Volume 15, pp. 151–159. [Google Scholar]
- Accardi, L.; Mukhamedov, F.; Saburov, M. On Quantum Markov Chains on Cayley tree II: Phase transitions for the associated chain with XY -model on the Cayley tree of order three. Ann. Henri Poincare 2011, 12, 1109–1144. [Google Scholar] [CrossRef]
- Mukhamedov, F.; Barhoumi, A.; Souissi, A. Phase transitions for quantum Markov chains associated with Ising type models on a Cayley tree. J. Stat. Phys. 2016, 163, 544–567. [Google Scholar] [CrossRef]
- Mukhamedov, F.; Souissi, A. Quantum Markov States on Cayley trees. J. Math. Anal. Appl. 2019, 473, 313–333. [Google Scholar] [CrossRef]
- Kempe, J. Quantum random walks: An introductory overview. Contemp. Phys. 2003, 44, 307–327. [Google Scholar] [CrossRef]
- Kitagawa, T. Topological phenomena in quantum walks: Elementary introduction to the physics of topological phases. Quantum Inf. Process. 2012, 11, 1107–1148. [Google Scholar] [CrossRef]
- Konno, N.; Yoo, H.J. Limit theorems for open quantum random walks. J. Stat. Phys. 2013, 150, 299–319. [Google Scholar] [CrossRef]
- Konno, N. A new type of limit theorems for one-dimensional quantum random walks. J. Math. Soc. Jpn. 2005, 57, 1179–1195. [Google Scholar] [CrossRef]
- Ohno, H. Unitary equivalent classes of one-dimensional quantum walks. Quantum Inf. Process. 2016, 15, 3599–3617. [Google Scholar] [CrossRef]
- Portugal, R. Quantum Walks and Search Algorithms; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Venegas-Andraca, S.E. Quantum walks: A comprehensive review. Quantum Inf. Process. 2012, 11, 1015–1106. [Google Scholar] [CrossRef]
- Accardi, L.; Watson, G.S. Quantum random walks. In Quantum Probability and Applications IV, Proceedings of the Year of Quantum Probability, Rome, Italy, 1987; Accardi, L., von Waldenfels, W., Eds.; LNM; 1989; Springer: Berlin/Heidelberg, Germany; Volume 1396, pp. 73–88.
- Lardizabal, C.F.; Souza, R.R. On a class of quantum channels, open random walks and recurrence. J. Stat. Phys. 2015, 159, 772–796. [Google Scholar] [CrossRef][Green Version]
- Attal, S.; Petruccione, F.; Sabot, C.; Sinayskiy, I. Open Quantum Random Walks. J. Stat. Phys. 2012, 147, 832–852. [Google Scholar] [CrossRef]
- Carbone, R.; Pautrat, Y. Homogeneous open quantum random walks on a lattice. J. Stat. Phys. 2015, 160, 1125–1152. [Google Scholar] [CrossRef]
- Carbone, R.; Pautrat, Y. Open quantum random walks: Reducibility, period, ergodic properties. Ann. Henri Poincaré 2016, 17, 99–135. [Google Scholar] [CrossRef]
- Goolam Hossen, Y.H.; Sinayskiy, I.; Petruccione, F. Non-reversal Open Quantum Walks. Open Syst. Inf. Dyn. 2018, 25, 1850017. [Google Scholar] [CrossRef]
- Machida, T. Phase transition of an open quantum walk. Int. J. Quantum Inf. 2021, 19, 2150028. [Google Scholar] [CrossRef]
- Dhahri, A.; Ko, C.K.; Yoo, H.J. Quantum Markov chains associated with open quantum random walks. J. Stat. Phys. 2019, 176, 1272–1295. [Google Scholar] [CrossRef]
- Dhahri, A.; Mukhamedov, F. Open quantum random walks, quantum Markov chains and recurrence. Rev. Math. Phys. 2019, 31, 1950020. [Google Scholar] [CrossRef]
- Ko, C.K.; Yoo, H.J. Quantum Markov chains associated with unitary quantum walks. J. Stoch. Anal. 2020, 1, 4. [Google Scholar] [CrossRef]
- Mukhamedov, F.; Souissi, A.; Hamdi, T. Open quantum random walks and quantum Markov chains on trees I: Phase transitions. Open Syst. Inf. Dyn. 2022, 29, 2250003. [Google Scholar] [CrossRef]
- Accardi, L.; Ohya, M.; Watanabe, N. Dynamical entropy through quantum Markov chains. Open Syst. Inf. Dyn. 1997, 4, 71–87. [Google Scholar] [CrossRef]
- Ohmura, K.; Watanabe, N. Quantum dynamical mutual entropy based on AOW entropy. Open Syst. Inf. Dyn. 2019, 26, 1950009. [Google Scholar] [CrossRef]
- Accardi, L.; Koroluk, D. Stopping times for quantum Markov chains. J. Theor. Probab. 1992, 5, 521–535. [Google Scholar] [CrossRef]
- Souissi, A. On Stopping Rules for Tree-indexed Quantum Markov chains. Infin. Dimens. Anal. Quantum Probab. Relat. Top. 2023, 2250030. [Google Scholar] [CrossRef]
- Barhoumi, A.; Souissi, A. Recurrence of a class of quantum Markov chains on trees. Chaos Solitons Fractals 2022, 164, 112644. [Google Scholar] [CrossRef]
- Mukhamedov, F.; Souissi, A.; Hamdi, T.; Andolsi, A. Open quantum random walks and quantum Markov Chains on trees II: The recurrence. Quantum Inf. Process. 2023, 22, 232. [Google Scholar] [CrossRef]
- Souissi, A.; Mukhamedov, F.; Barhoumi, A. Tree-Homogeneous Quantum Markov Chains. Int. J. Theor. Phys. 2023, 62, 19. [Google Scholar] [CrossRef]
- McKay, B.D.; Piperno, A. Practical graph isomorphism, II. J. Symb. Comput. 2014, 60, 94–112. [Google Scholar] [CrossRef]
- Bratteli, O.; Robinson, D.W. Operator Algebras and Quantum Statistical Mechanics I; Springer: New York, NY, USA, 1987. [Google Scholar]
- Accardi, L.; Cecchini, C. Conditional expectations in von Neumann algebras and a Theorem of Takesaki. J. Funct. Anal. 1982, 45, 245–273. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Souissi, A.; Hamdi, T.; Mukhamedov, F.; Andolsi, A. On the Structure of Quantum Markov Chains on Cayley Trees Associated with Open Quantum Random Walks. Axioms 2023, 12, 864. https://doi.org/10.3390/axioms12090864
Souissi A, Hamdi T, Mukhamedov F, Andolsi A. On the Structure of Quantum Markov Chains on Cayley Trees Associated with Open Quantum Random Walks. Axioms. 2023; 12(9):864. https://doi.org/10.3390/axioms12090864
Chicago/Turabian StyleSouissi, Abdessatar, Tarek Hamdi, Farrukh Mukhamedov, and Amenallah Andolsi. 2023. "On the Structure of Quantum Markov Chains on Cayley Trees Associated with Open Quantum Random Walks" Axioms 12, no. 9: 864. https://doi.org/10.3390/axioms12090864
APA StyleSouissi, A., Hamdi, T., Mukhamedov, F., & Andolsi, A. (2023). On the Structure of Quantum Markov Chains on Cayley Trees Associated with Open Quantum Random Walks. Axioms, 12(9), 864. https://doi.org/10.3390/axioms12090864





