Embedding Spanning Disjoint Cycles in Hypercube Networks with Prescribed Edges in Each Cycle
Abstract
1. Introduction
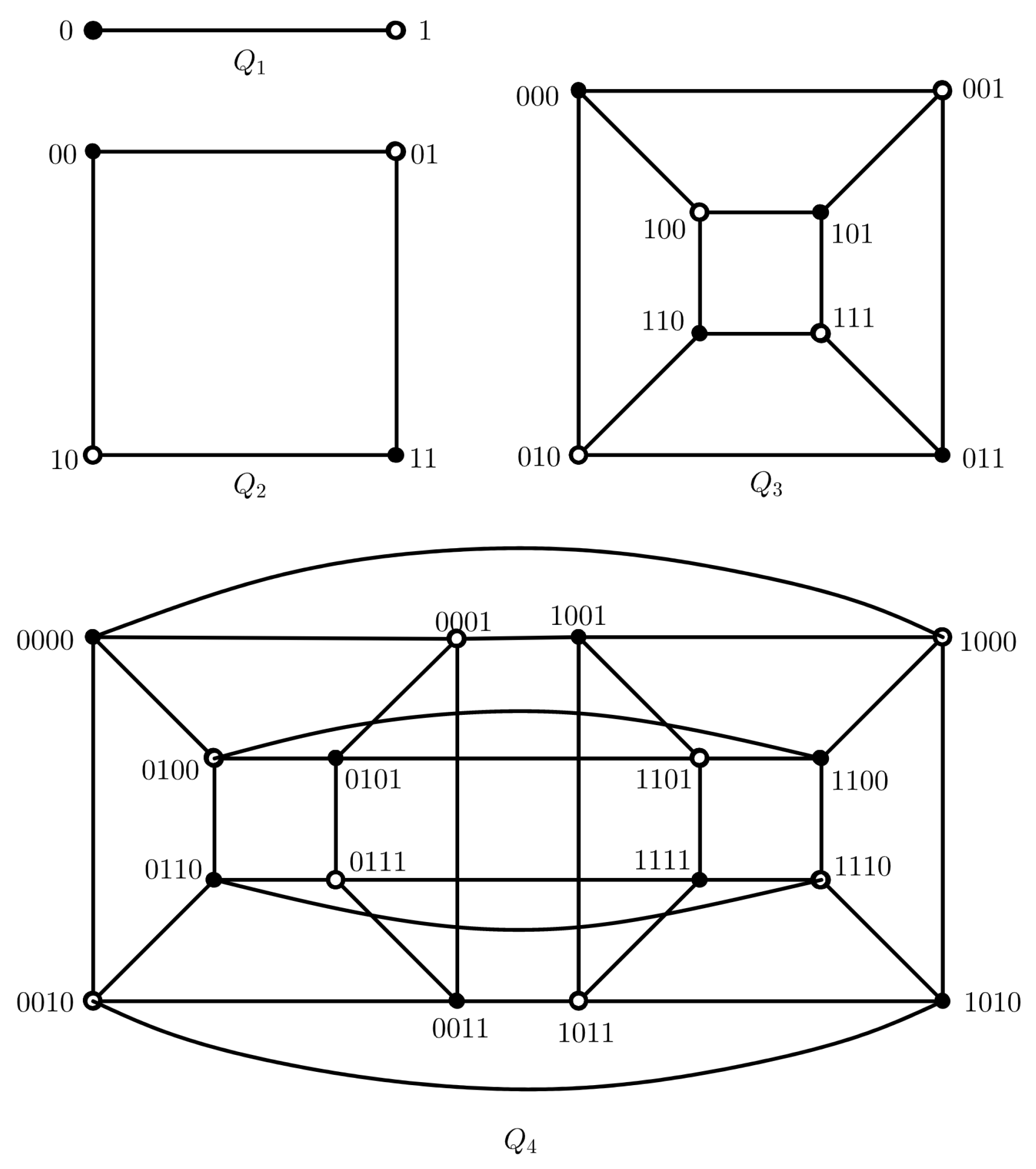
2. Preliminaries
- (1)
- , and
- (2)
- for all .
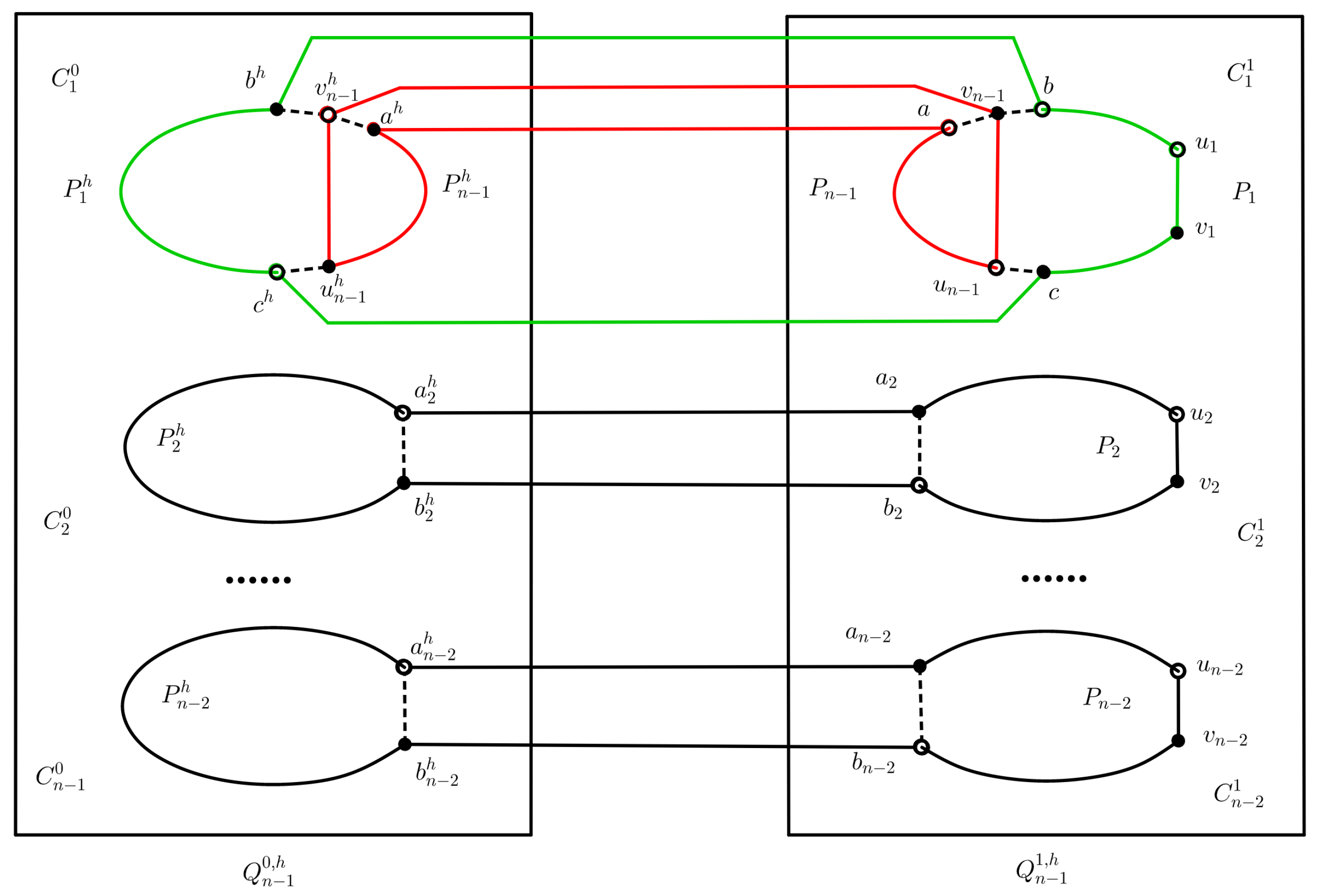
3. Proof of the Main Result
- (1)
- and are in different ’s for and .
- (2)
- and are in the same for and .
- (1)
- Three prescribed edges , and are not in the same for .
- (2)
- Three prescribed edges , and are in the same with .
- (1)
- with .
- (2)
- with .
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lu, J.; Zhu, L.; Gao, W. Remarks on bipolar cubic fuzzy graphs and its chemical applications. Int. J. Math. Comput. Eng. 2023, 1, 1–10. [Google Scholar] [CrossRef]
- Wang, Y.; Guo, F.; Rong, B.; Zhang, H.; Alsultan, J. The Control Relationship between the Enterprise’s Electrical Equipment and Mechanical Equipment Based on Graph Theory. Appl. Math. Nonlinear Sci. 2022, 8, 399–408. [Google Scholar] [CrossRef]
- Li, Q.; Wan, J.; Zhang, W.; Kweh, Q. Spatial-temporal graph neural network based on node attention. Appl. Math. Nonlinear Sci. 2022, 7, 703–712. [Google Scholar] [CrossRef]
- Wang, X.; Ma, L.; Yang, Z. Research on industrial Internet of Things and power grid technology application based on knowledge graph and data asset relationship model. Appl. Math. Nonlinear Sci. 2022, 8, 2717–2728. [Google Scholar] [CrossRef]
- Sun, X.; Hao, T.; Li, X. Knowledge graph construction and Internet of Things optimisation for power grid data knowledge extraction. Appl. Math. Nonlinear Sci. 2022, 8, 2729–2738. [Google Scholar] [CrossRef]
- Lv, Y.; Lin, C.-K.; Fan, J.; Jia, X. Hamiltonian cycle and path embeddings in 3-ary n-cubes based on K1,3-structure faults. J. Parallel Distr. Comput. 2018, 120, 148–158. [Google Scholar] [CrossRef]
- Wang, X.; Erickson, A.; Fan, J.; Jia, X. Hamiltonian Properties of DCell Networks. Comput. J. 2015, 58, 2944–2955. [Google Scholar] [CrossRef]
- Leighton, F.T. Introduction to Parallel Algorithms and Architecture: Arrays, Trees, Hypercubes; Morgan Kaufmann Publishers Inc.: Burlington, MA, USA, 1991. [Google Scholar]
- Qiao, H.; Meng, J.; Sabir, E. Embedding spanning disjoint cycles in enhanced hypercube networks with prescribed vertices in each cycle. Appl. Math. Comput. 2022, 435, 127481. [Google Scholar] [CrossRef]
- Hsu, L.-H.; Tan, J.J.M.; Cheng, E.; Lipták, L.; Lin, C.-K.; Tsai, M. Solution to an open problem on 4-ordered Hamiltonian graphs. Discrete Math. 2012, 312, 2356–2370. [Google Scholar] [CrossRef][Green Version]
- Liu, J. Hamiltonian decompositions of cayley graphs on abelian groups of even order. J. Comb. Theory B 2003, 88, 305–321. [Google Scholar] [CrossRef]
- Hsieh, S.-Y.; Shiu, J.-Y. Cycle embedding of augmented cubes. Appl. Math. Comput. 2007, 191, 314–319. [Google Scholar] [CrossRef]
- Hsu, L.-H.; Lin, C.-K. Graph Theory and Interconnection Networks; CRC Press: Boca Raton, FL, USA, 2008. [Google Scholar]
- Lin, C.-K.; Tan, J.J.M.; Hsu, L.-H.; Kung, T.-L. Disjoint cycles in hypercubes with prescribed vertices in each cycle. Discrete Appl. Math. 2013, 161, 2992–3004. [Google Scholar] [CrossRef]
- Wang, H. Covering a graph with cycles passing through given edges. J. Graph Theory 1997, 26, 105–109. [Google Scholar] [CrossRef]
- Egawa, Y.; Faudree, R.J.; Györi, E.; Ishigami, Y.; Schelp, R.H.; Wang, H. Vertex-disjoint cycles containing specified edges. Graphs Comb. 2000, 16, 81–92. [Google Scholar] [CrossRef]
- Wang, H. Covering a bipartite graph with cycles passing through given edges. J. Graph Theory 1999, 19, 115–121. [Google Scholar]
- Egawa, Y.; Enomoto, H.; Faudree, R.; Li, H.; Schiermeyer, I. Two-factors each component of which contains a specified vertex. J. Graph Theory 2003, 43, 188–198. [Google Scholar] [CrossRef]
- Ishigami, Y.; Jiang, T. Vertex-disjoint cycles containing prescribed vertices. J. Graph Theory 2003, 42, 276–296. [Google Scholar] [CrossRef]
- Chiba, S.; Yamashita, T. Degree conditions for the existence of vertex-disjoint cycles and paths: A Survey. Graphs Comb. 2018, 4, 1–83. [Google Scholar] [CrossRef]
- Gould, R. A look at cycles containing specified elements of a graph. Discrete Math. 2009, 309, 6299–6311. [Google Scholar] [CrossRef][Green Version]
- Kung, T.-L.; Hung, C.-N.; Lin, C.-K.; Chen, H.-C.; Lin, C.-H.; Hsu, L.-H. A framework of cycle-based clustering on the crossed cube architecture. In Proceedings of the International Conference on Innovation Mobile and Internet Services in Ubiquitous Computing, Fukuoka, Japan, 6–8 July 2016; Volume 10, pp. 430–434. [Google Scholar]
- Shinde, A.; Borse, Y.M. Disjoint cycles through prescribed vertices in multidimensional tori. J. Ramanujan Math. 2021, 4, 283–290. [Google Scholar]
- Yang, M.-C.; Hsu, L.-H.; Hung, C.-N.; Cheng, E. 2-spanning cyclability problems of some generalized Petersen graphs. Discuss. Math. Graph Theory 2020, 40, 713–731. [Google Scholar]
- Qiao, H.; Sabir, E.; Meng, J. The spanning cyclability of Cayley graphs generated by transposition trees. Discrete Appl. Math. 2023, 328, 60–69. [Google Scholar] [CrossRef]
- Tsai, C.-H.; Jiang, S.-Y. Path bipancyclicity of hypercubes. Inf. Process. Lett. 2007, 101, 93–97. [Google Scholar] [CrossRef]
- Chen, X.-B. Hamiltonian of hypercubes with faulty vertices. Inf. Process. Lett. 2016, 116, 343–346. [Google Scholar] [CrossRef]
- Fu, J.-S. Fault-tolerant cycle embedding in the hypercube. Parallel Comput. 2003, 29, 821–832. [Google Scholar] [CrossRef]
- Li, T.K.; Tsai, C.H.; Tan, J.J.M.; Hsu, L.H. Bipannectivity and edge-fault-tolerant bipancyclicity of hypercubes. Inf. Process. Lett. 2003, 87, 107–110. [Google Scholar] [CrossRef]
- Saad, Y.; Schultz, M.H. Topological properties of hypercubes. IEEE Trans. Comput. 1988, 37, 867–872. [Google Scholar] [CrossRef]
- Xu, J.-M.; Ma, M. Survey on path and cycle embedding in some networks. Front. Math. China 2009, 4, 217–252. [Google Scholar] [CrossRef]
- Sun, C.-M.; Hung, C.-N.; Huang, H.-M.; Hsu, L.-H.; Jou, Y.-D. Hamiltonian laceability of faulty hypercubes. J. Interconnect. Netw. 2007, 8, 133–145. [Google Scholar] [CrossRef]
| Prescribed Edges | Spanning Disjoint Cycles | |
|---|---|---|
| Prescribed Edges | Spanning Disjoint Cycles | |
|---|---|---|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, W.; Sabir, E. Embedding Spanning Disjoint Cycles in Hypercube Networks with Prescribed Edges in Each Cycle. Axioms 2023, 12, 861. https://doi.org/10.3390/axioms12090861
Wu W, Sabir E. Embedding Spanning Disjoint Cycles in Hypercube Networks with Prescribed Edges in Each Cycle. Axioms. 2023; 12(9):861. https://doi.org/10.3390/axioms12090861
Chicago/Turabian StyleWu, Weiyan, and Eminjan Sabir. 2023. "Embedding Spanning Disjoint Cycles in Hypercube Networks with Prescribed Edges in Each Cycle" Axioms 12, no. 9: 861. https://doi.org/10.3390/axioms12090861
APA StyleWu, W., & Sabir, E. (2023). Embedding Spanning Disjoint Cycles in Hypercube Networks with Prescribed Edges in Each Cycle. Axioms, 12(9), 861. https://doi.org/10.3390/axioms12090861





