Extropy Based on Concomitants of Order Statistics in Farlie-Gumbel-Morgenstern Family for Random Variables Representing Past Life
Abstract
1. Introduction
2. Past Extropy for Concomitants of Order Statistics in FGM Family
3. Cumulative Past Extropy of Concomitants of Order Statistics in FGM Family
4. Dynamic Survival Past Extropy for Concomitants of Order Statistics in FGM Family
5. Estimation of CPE for Concomitant of rth Order Statistic
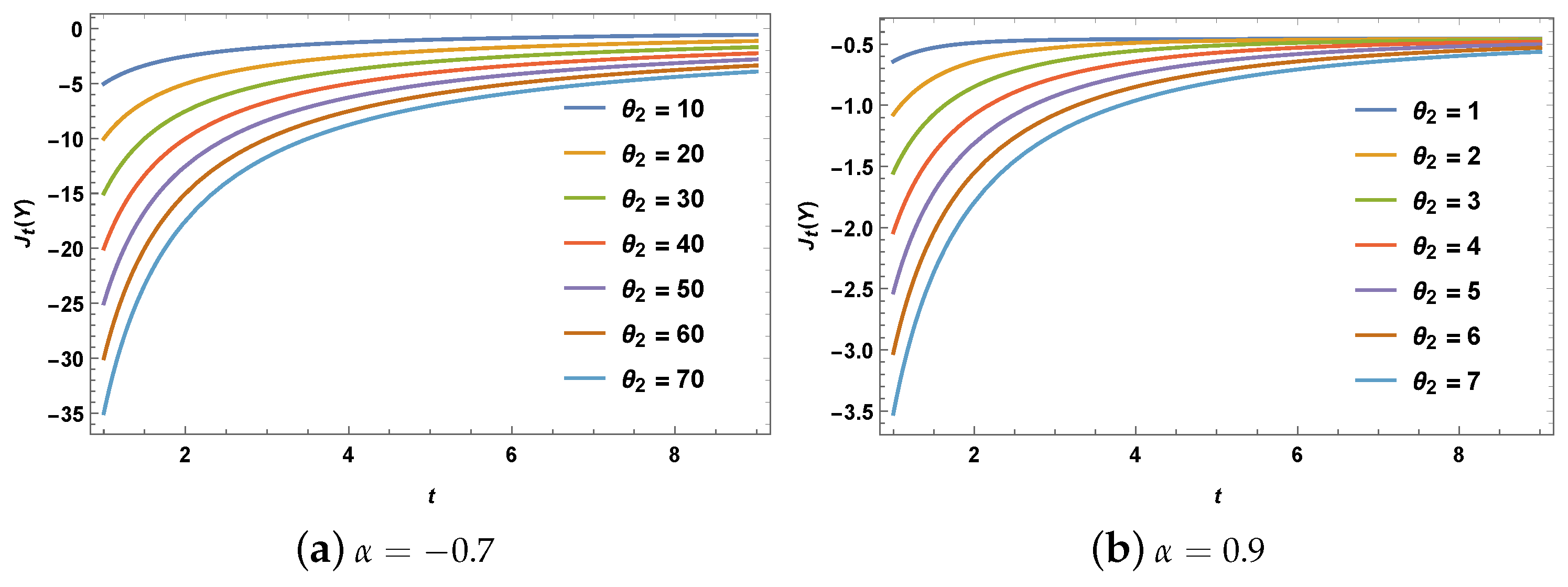
- For a fixed value of n, the values of are decreasing as the values of increases, whereas the values of are increasing with .
- For a fixed , both the values of and are decreasing with the increasing value of n.
- As n tends to infinity, the value of tends to zero.
6. Simulation
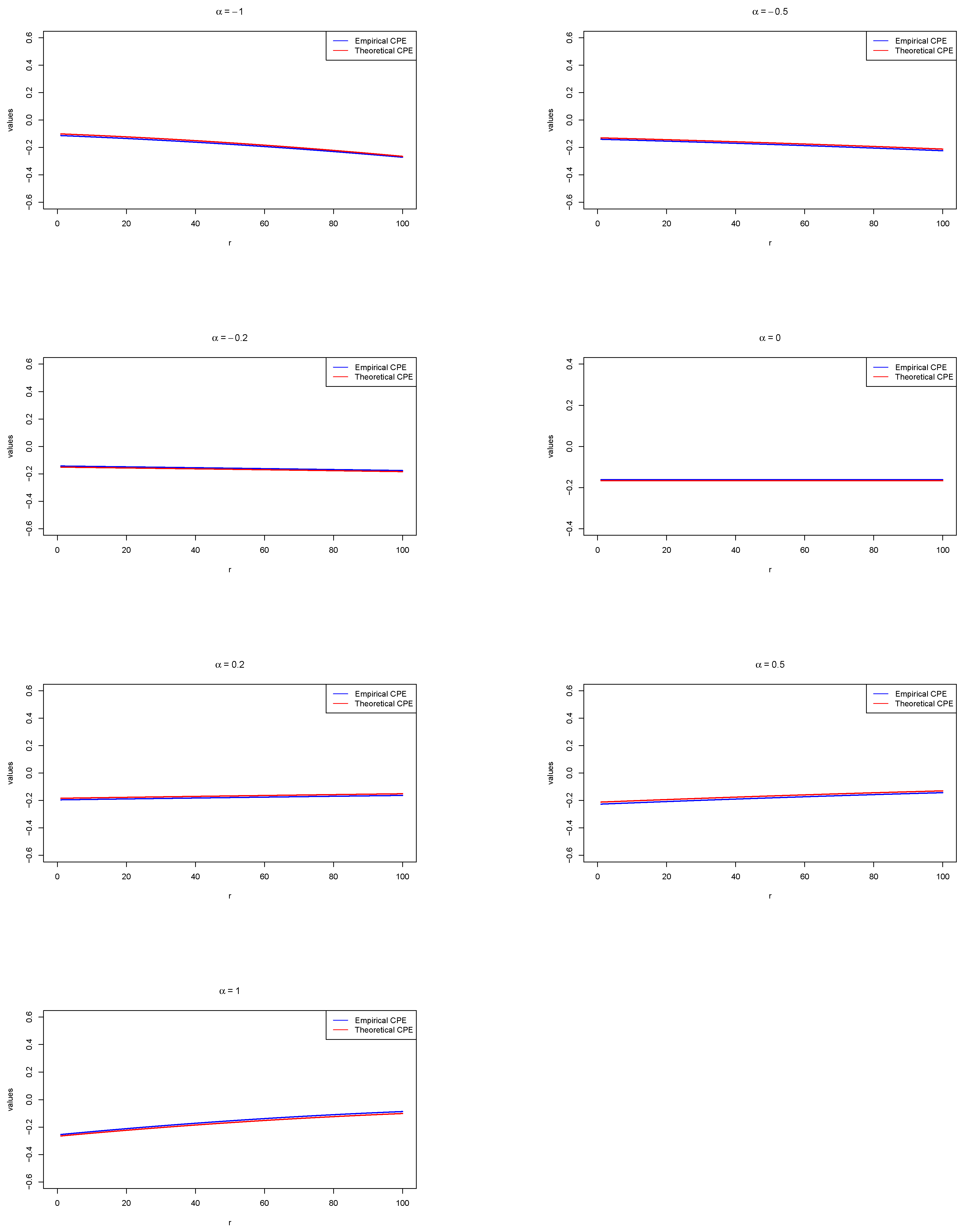
- In all the cases discussed in the figure, both and behaves alike. Both the values are almost similar for various values of OS.
- For , both the theoretical and empirical CPE of show a decreasing trend as the value of r increases.
- On the other hand, for , both the functions and exhibit an increasing pattern as r falls within this range.
7. Conclusions and Future Works
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- David, H.A. Concomitants of order statistics. Bull. Int. Stat. Inst. 1973, 45, 295–300. [Google Scholar]
- David, H.A.; Nagaraja, H.N. Concomitants of order statistics. In Handbook of Statistics; Balakrishnan, N., Rao, C.R., Eds.; Elsevier: Amsterdam, The Netherlands, 1998; pp. 487–513. [Google Scholar]
- Morgenstern, D. Einfache beispiele zweidimensionaler verteilungen. Mitteilungsblatt Math. Stat. 1956, 8, 234–235. [Google Scholar]
- Bairamov, I.; Kotz, S.; Bekci, M. New generalized Farlie-Gumbel-Morgenstern distributions and concomitants of order statistics. J. Appl. Stat. 2001, 28, 521–536. [Google Scholar] [CrossRef]
- Susam, S.O. A multi-parameter Generalized Farlie-Gumbel-Morgenstern bivariate copula family via Bernstein polynomial. Hacet. J. Math. Stat. 2022, 51, 618–631. [Google Scholar] [CrossRef]
- Shannon, C.E. A mathematical theory of communication. Bell Syst. Tech. J. 1948, 27, 379–432. [Google Scholar] [CrossRef]
- Baratpour, S.; Ahmadi, J.; Arghami, N.R. Some characterizations based on entropy of order statistics and record values. Commun. Stat.-Theory Methods 2007, 36, 47–57. [Google Scholar] [CrossRef]
- Al-Omari, A.I. A new measure of sample entropy of continuous random variable. J. Stat. Theory Pract. 2015, 10, 721–735. [Google Scholar] [CrossRef]
- Al-Omari, A.I. Estimation of entropy using random sampling. J. Comput. Appl. Math. 2014, 261, 95–102. [Google Scholar] [CrossRef]
- Song, K.S. A mathematical theory of communication. J. Stat. Plan. Inference 2001, 93, 51–69. [Google Scholar] [CrossRef]
- Frank, L.; Sanfilippo, G.; Agro, G. Extropy: Complementary dual of entropy. Stat. Sci. 2015, 30, 40–58. [Google Scholar]
- Martinas, K.; Frankowicz, M. Extropy-reformulation of the Entropy Principle. Period. Polytech. Chem. Eng. 2000, 44, 29–38. [Google Scholar]
- Becerra, A.; de la Rosa, J.I.; Gonzalez, E.; Pedroza, A.D.; Escalante, N.I. Training deep neural networks with non-uniform frame-level cost function for automatic speech recognition. Multimed. Tools Appl. 2018, 77, 27231–27267. [Google Scholar] [CrossRef]
- Qiu, G. Extropy of order statistics and record values. Stat. Probab. Lett. 2017, 120, 52–60. [Google Scholar] [CrossRef]
- Qiu, G.; Wang, L.; Wang, X. On extropy properties of mixed systems. Probab. Eng. Inf. Sci. 2019, 33, 471–486. [Google Scholar] [CrossRef]
- Gupta, N.; Chaudhary, S.K. On general weighted extropy of ranked set sampling. Commun. Stat.-Theory Methods 2023. [Google Scholar] [CrossRef]
- Di Crescenzo, A.; Longobardi, M. Entropy-based measure of uncertainity in past lifetime distributions. J. Appl. Probab. 2002, 39, 434–440. [Google Scholar] [CrossRef]
- Krishnan, A.S.; Sunoj, S.M.; Nair, N.U. Some reliability properties of extropy for residual and past lifetime random variables. J. Korean Stat. Soc. 2020, 49, 457–474. [Google Scholar] [CrossRef]
- Kamari, O.; Buono, F. On extropy of past lifetime distribution. Ric. Mat. 2021, 70, 505–515. [Google Scholar] [CrossRef]
- Vaselabadi, N.M.; Tahmasebi, S.; Kazemi, M.R.; Buono, F. Results on varextropy measure of random variables. Entropy 2021, 23, 356. [Google Scholar] [CrossRef]
- Irshad, M.R.; Maya, R.; Archana, K.; Tahmasebi, S. On Past Extropy and Negative Cumulative Extropy Properties of Ranked Set Sampling and Maximum Ranked Set Sampling with Unequal Samples. Stat. Optim. Inf. Comput. 2023, 11, 740–754. [Google Scholar]
- Jose, J.; Abdul Sathar, E.I. Extropy for past life based on classical records. Extropy Past Life Based Class. Rec. 2021, 22, 27–46. [Google Scholar] [CrossRef]
- Sathar, E.A.; Nair, R.D. On dynamic weighted extropy. J. Comput. Appl. Math. 2021, 393, 113507. [Google Scholar] [CrossRef]
- Abdul Sathar, E.I.; Nair, R.D. On dynamic survival extropy. Commun. Stat.-Theory Methods 2020, 50, 1295–1313. [Google Scholar] [CrossRef]
- Pakdaman, Z.; Hashempour, M. On dynamic survival past extropy properties. J. Stat. Res. Iran 2019, 16, 229–244. [Google Scholar]
- Abo-Eleneen, Z.A.; Nagaraja, H.N. Fisher information in an order statistic and its concomitant. Ann. Inst. Stat. Math. 2002, 54, 667–680. [Google Scholar] [CrossRef]
- Tahmasebi, S.; Behboodian, J. Information properties for concomitants of order statistics in Farlie–Gumbel–Morgenstern (FGM) Family. Commun. Stat.-Theory Methods 2012, 41, 1954–1968. [Google Scholar] [CrossRef]
- Almaspoor, Z.; Jafari, A.A.; Tahmasebi, S. Measures of extropy for concomitants of generalized order statistics in Morgenstern Family. J. Stat. Theory Appl. 2022, 21, 1–20. [Google Scholar] [CrossRef]
- Mohamed, M.S.; Abdulrahman, A.T.; Almaspoor, Z.; Yusuf, M. Ordered variables and their concomitants under extropy via COVID-19 data application. Complexity 2021, 2021, 6491817. [Google Scholar] [CrossRef]
- Hussiney, I.A.; Shyam, A.H. The extropy of concomitants of generalized order statistics from Huang–Kotz–Morgenstern bivariate distribution. Hindawi J. Math. 2022, 2022, 6385998. [Google Scholar] [CrossRef]
- Mohie EL-Din, M.M.; Amein, M.M.; Ali, N.S.A.; Mohamed, M.S. Residual and past entropy for concomitants of ordered random variables of Morgenstern Family. Hindawi J. Math. 2015, 2015, 159710. [Google Scholar] [CrossRef][Green Version]
- Johnson, N.L.; Kotz, S. On some generalized Farlie–Gumbel–Morgenstern distributions. Commun. Stat.-Theory Methods 1975, 4, 415–427. [Google Scholar]
- Scaria, J.; Nair, U. On concomitants of order statistics from Morgenstern family. Biom. J. 1999, 41, 483–489. [Google Scholar] [CrossRef]



| n | ||||
|---|---|---|---|---|
| 5 | −0.0793 | −0.0892 | −0.1114 | −0.1237 |
| 15 | −0.0914 | −0.1140 | −0.1549 | −0.2080 |
| 25 | −0.0945 | −0.1197 | −0.1723 | −0.2299 |
| 50 | −0.0971 | −0.1243 | −0.1816 | −0.2476 |
| n | ||||
|---|---|---|---|---|
| 5 | 0.0019 | 0.0023 | 0.0033 | 0.0040 |
| 15 | 0.0012 | 0.0016 | 0.0029 | 0.0039 |
| 25 | 0.0008 | 0.0011 | 0.0021 | 0.0029 |
| 50 | 0.0004 | 0.0006 | 0.0012 | 0.0017 |
| r | n | Bias | MSE | |||
|---|---|---|---|---|---|---|
| 2 | 0.2 | 50 | −0.1825 | −0.1747 | 0.0078 | 0.0005 |
| 0.2 | 100 | −0.1832 | −0.1782 | 0.0050 | 0.0002 | |
| 0.2 | 200 | −0.1836 | −0.1815 | 0.0020 | 0.0001 | |
| 0.5 | 50 | −0.2086 | −0.1991 | 0.0094 | 0.0005 | |
| 0.5 | 100 | −0.2105 | −0.2064 | 0.0040 | 0.0002 | |
| 0.5 | 200 | −0.2115 | −0.2092 | 0.0022 | 0.0001 | |
| 0.8 | 50 | −0.2371 | −0.2280 | 0.0091 | 0.0005 | |
| 0.8 | 100 | −0.2405 | −0.2360 | 0.0044 | 0.0003 | |
| 0.8 | 200 | −0.2422 | −0.2399 | 0.0023 | 0.0001 | |
| 4 | 0.2 | 50 | −0.1811 | −0.1718 | 0.0093 | 0.0005 |
| 0.2 | 100 | −0.1825 | −0.1788 | 0.0037 | 0.0002 | |
| 0.2 | 200 | −0.1832 | −0.1807 | 0.0025 | 0.0001 | |
| 0.5 | 50 | −0.2047 | −0.1958 | 0.0089 | 0.0005 | |
| 0.5 | 100 | −0.2085 | −0.2046 | 0.0039 | 0.0002 | |
| 0.5 | 200 | −0.2105 | −0.2081 | 0.0023 | 0.0001 | |
| 0.8 | 50 | −0.2304 | −0.2209 | 0.0095 | 0.0005 | |
| 0.8 | 100 | −0.2370 | −0.2321 | 0.0049 | 0.0003 | |
| 0.8 | 200 | −0.2405 | −0.2378 | 0.0026 | 0.0001 | |
| 10 | 0.2 | 50 | −0.1770 | −0.1678 | 0.0092 | 0.0004 |
| 0.2 | 100 | −0.1804 | −0.1763 | 0.0041 | 0.0002 | |
| 0.2 | 200 | −0.1822 | −0.1801 | 0.0020 | 0.0001 | |
| 0.5 | 50 | −0.1935 | −0.1851 | 0.0083 | 0.0005 | |
| 0.5 | 100 | −0.2027 | −0.1982 | 0.0045 | 0.0002 | |
| 0.5 | 200 | −0.2075 | −0.2051 | 0.0024 | 0.0001 | |
| 0.8 | 50 | −0.2111 | −0.2034 | 0.0076 | 0.0005 | |
| 0.8 | 100 | −0.2269 | −0.2227 | 0.0042 | 0.0002 | |
| 0.8 | 200 | −0.2353 | −0.2334 | 0.0018 | 0.0001 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Irshad, M.R.; Archana, K.; Al-Omari, A.I.; Maya, R.; Alomani, G. Extropy Based on Concomitants of Order Statistics in Farlie-Gumbel-Morgenstern Family for Random Variables Representing Past Life. Axioms 2023, 12, 792. https://doi.org/10.3390/axioms12080792
Irshad MR, Archana K, Al-Omari AI, Maya R, Alomani G. Extropy Based on Concomitants of Order Statistics in Farlie-Gumbel-Morgenstern Family for Random Variables Representing Past Life. Axioms. 2023; 12(8):792. https://doi.org/10.3390/axioms12080792
Chicago/Turabian StyleIrshad, Muhammed Rasheed, Krishnakumar Archana, Amer Ibrahim Al-Omari, Radhakumari Maya, and Ghadah Alomani. 2023. "Extropy Based on Concomitants of Order Statistics in Farlie-Gumbel-Morgenstern Family for Random Variables Representing Past Life" Axioms 12, no. 8: 792. https://doi.org/10.3390/axioms12080792
APA StyleIrshad, M. R., Archana, K., Al-Omari, A. I., Maya, R., & Alomani, G. (2023). Extropy Based on Concomitants of Order Statistics in Farlie-Gumbel-Morgenstern Family for Random Variables Representing Past Life. Axioms, 12(8), 792. https://doi.org/10.3390/axioms12080792









