Stabilization Control for a Class of Fractional-Order HIV-1 Infection Model with Time Delays
Abstract
1. Introduction
2. Preliminaries
3. Main Results
3.1. Stabilization Control of the Fractional-Order HIV-1 Infection Model
3.2. Stabilization Control of the Fractional-Order HIV-1 Infection Model with a Time Delay
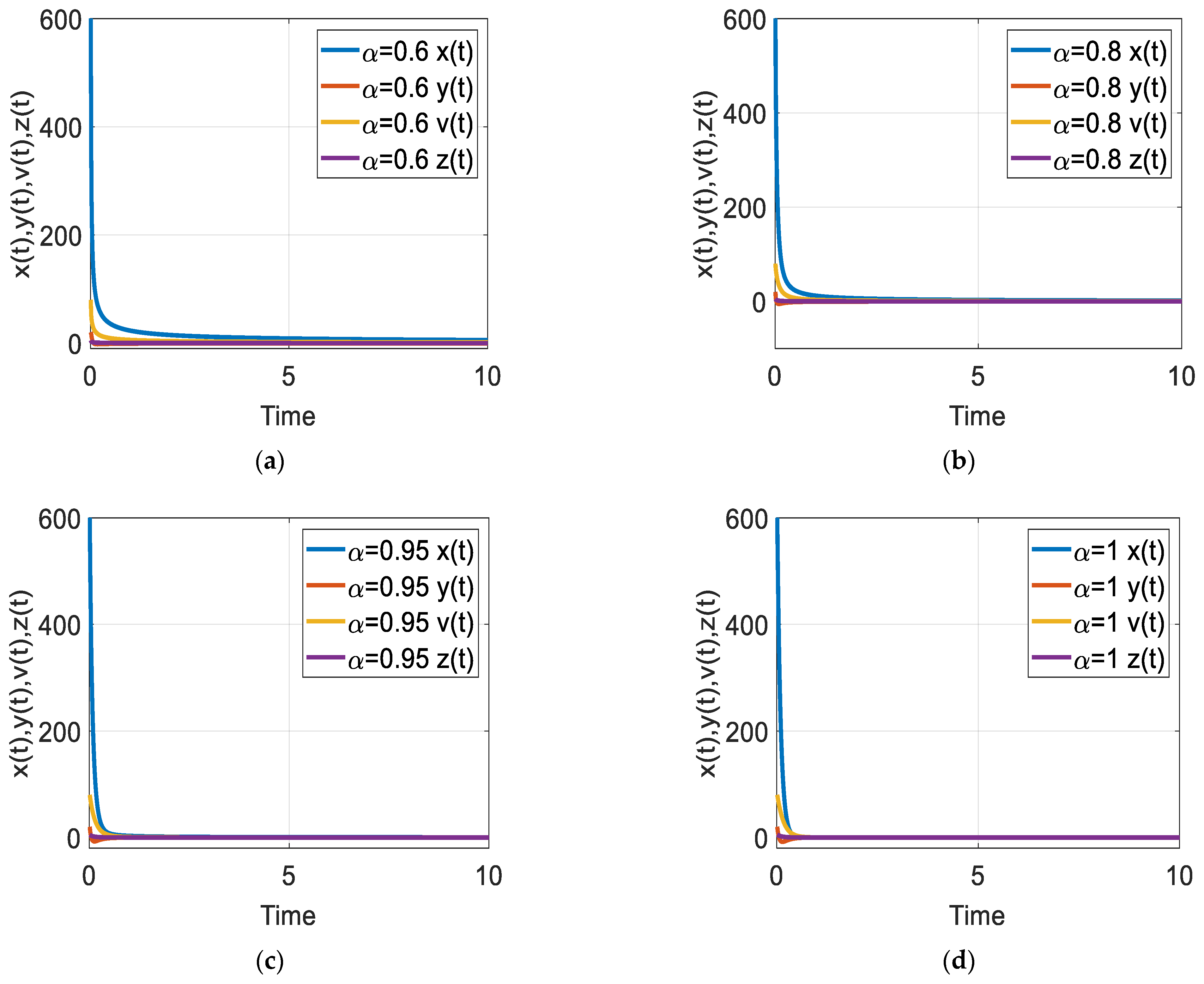
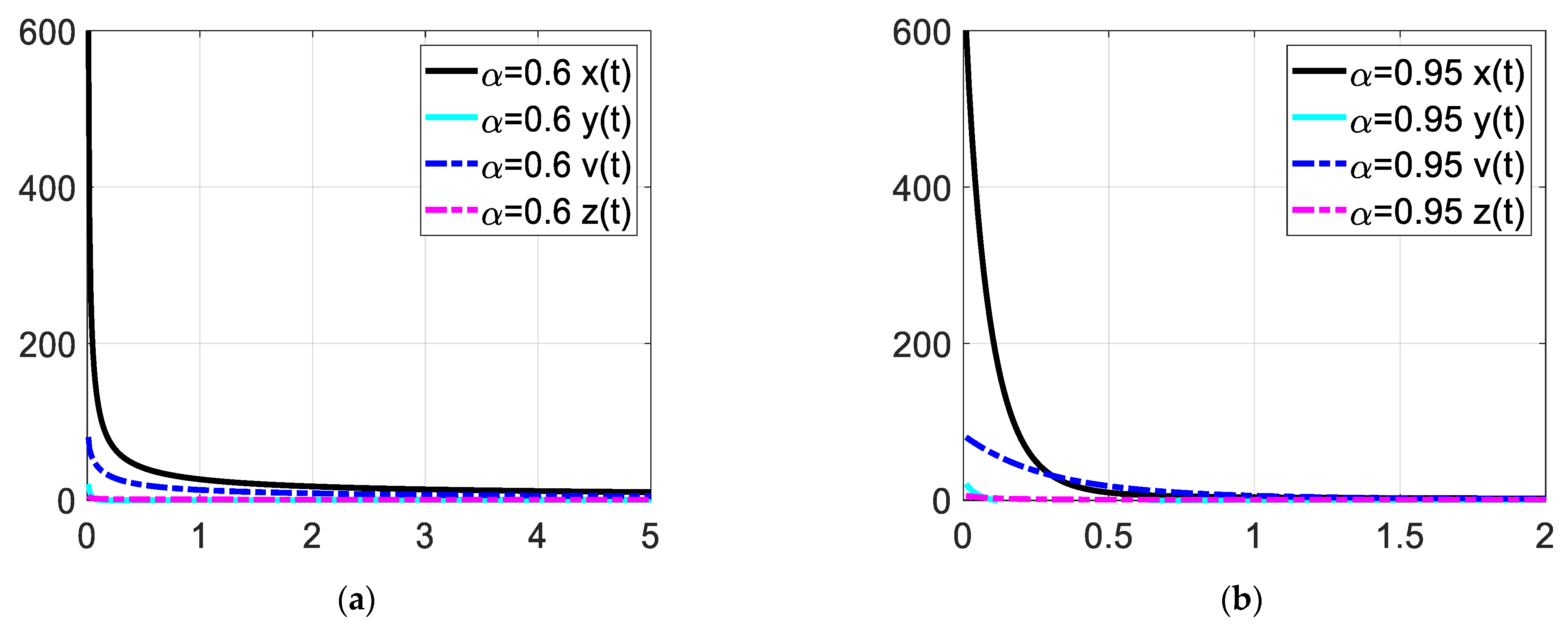
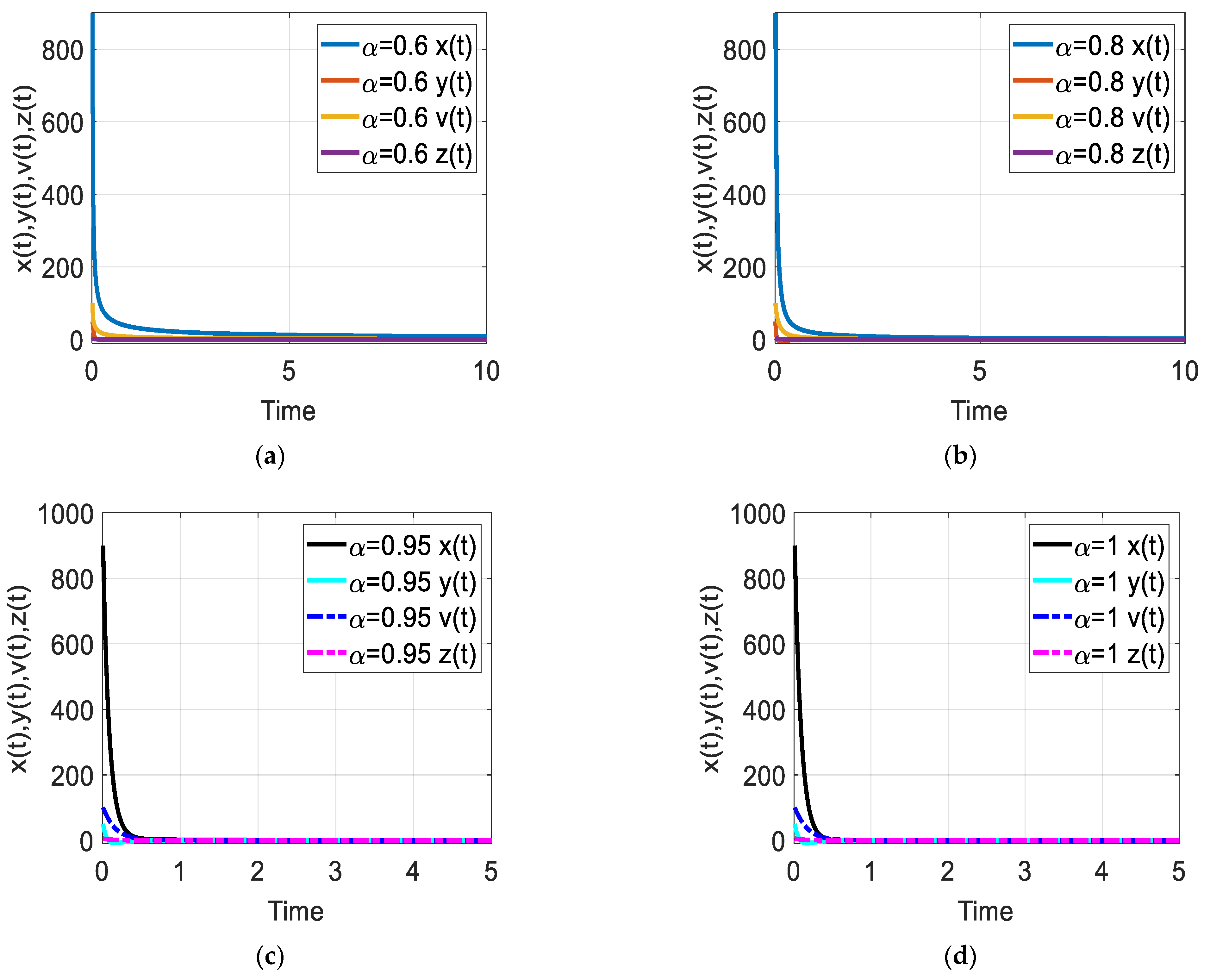
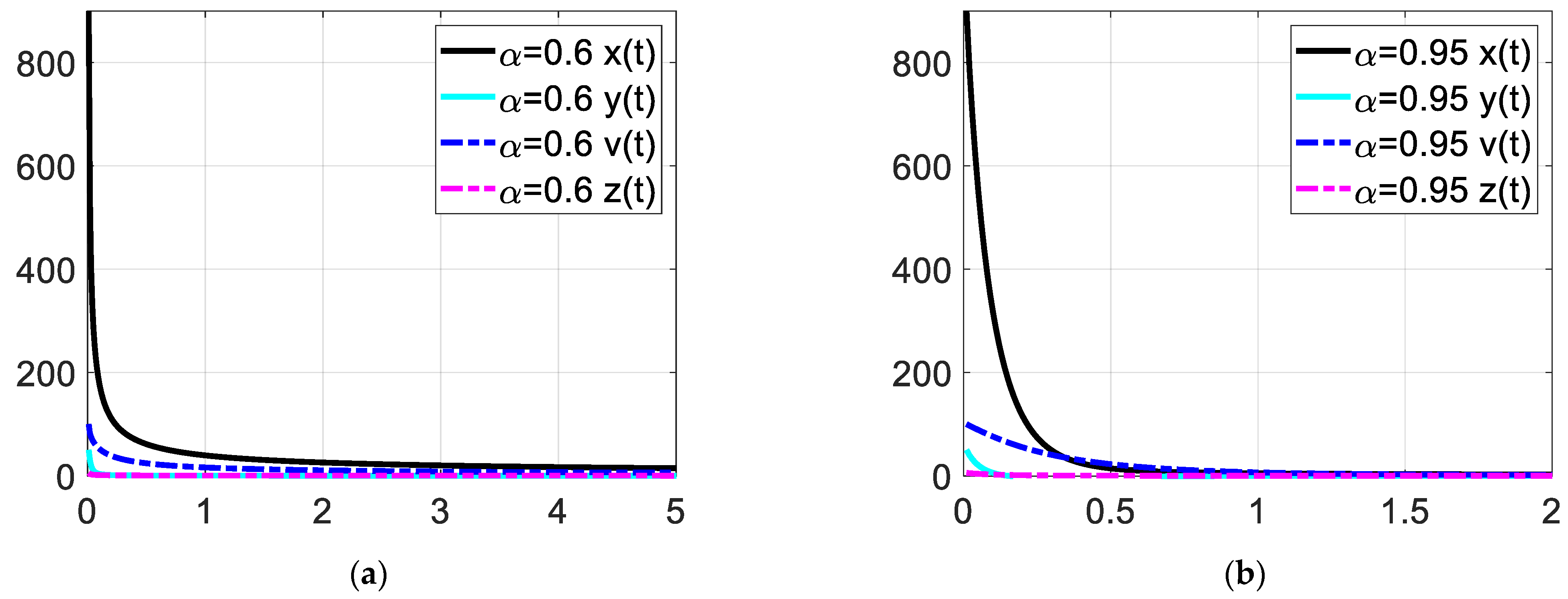
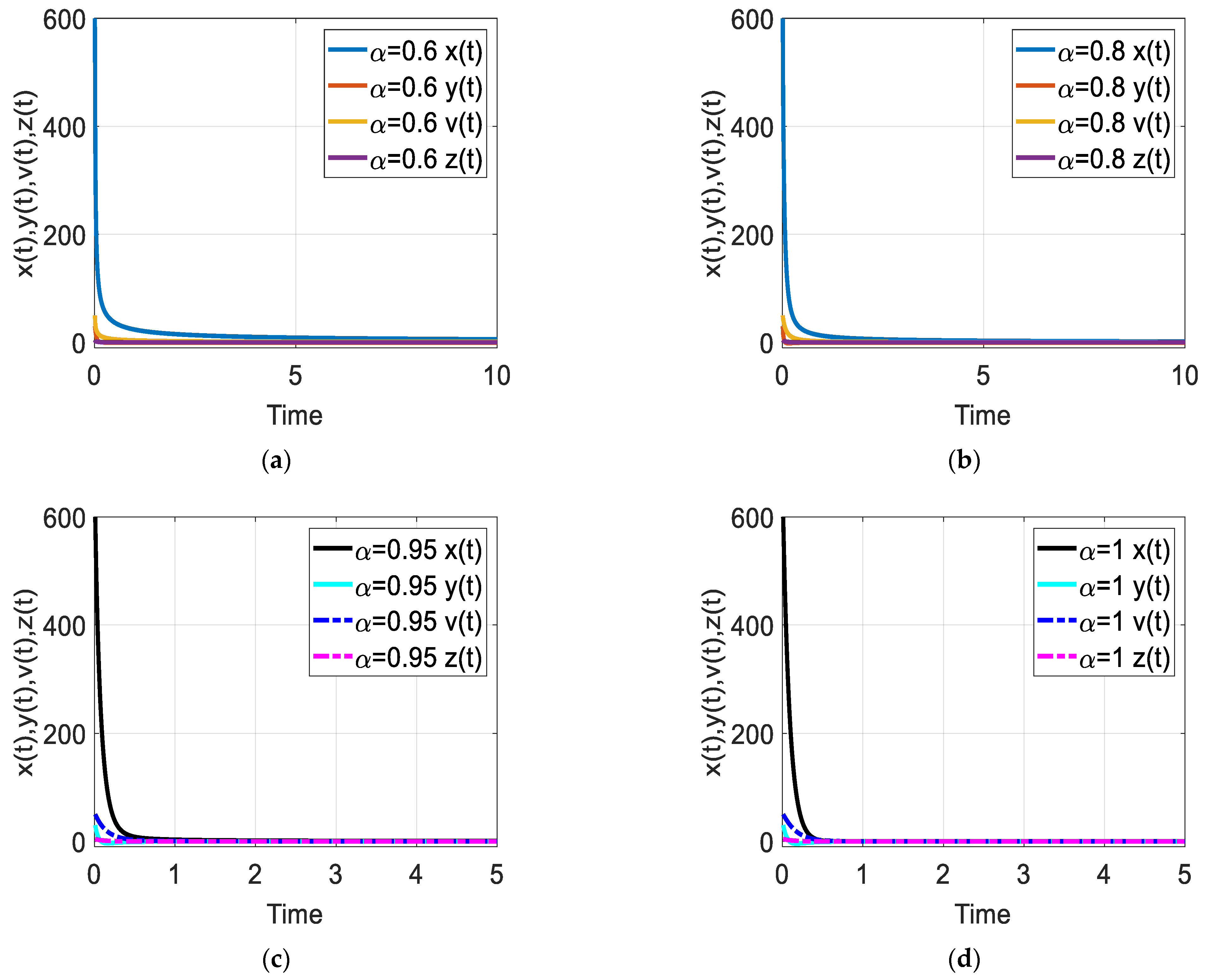
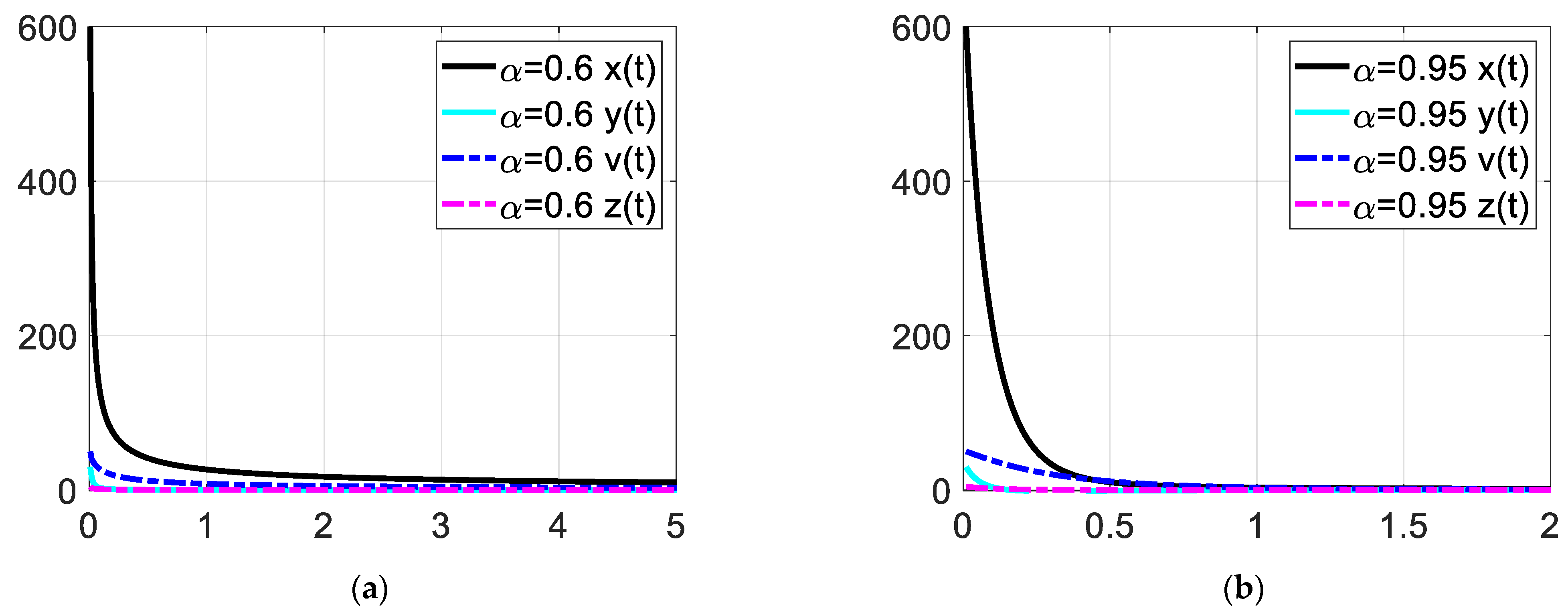
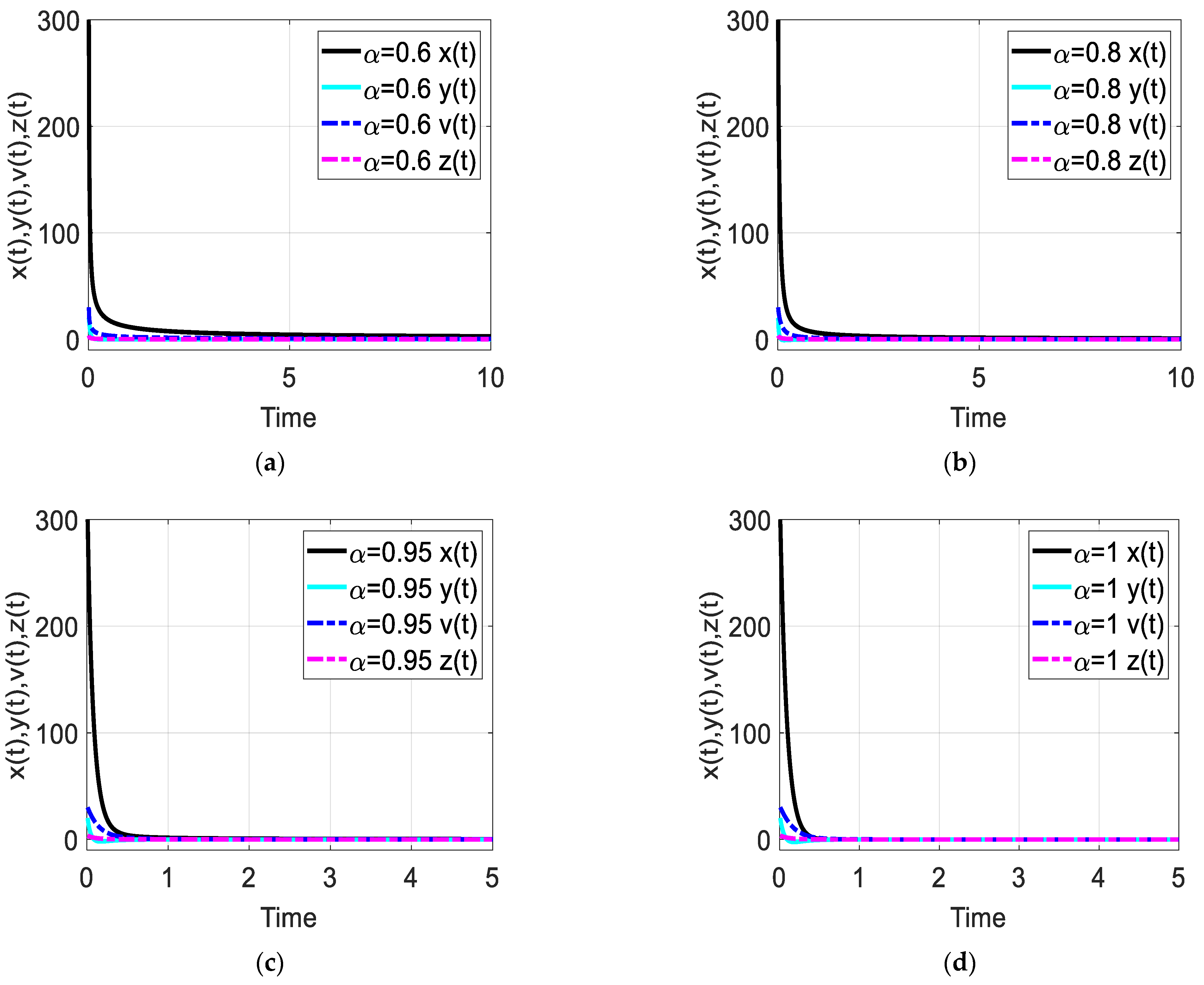
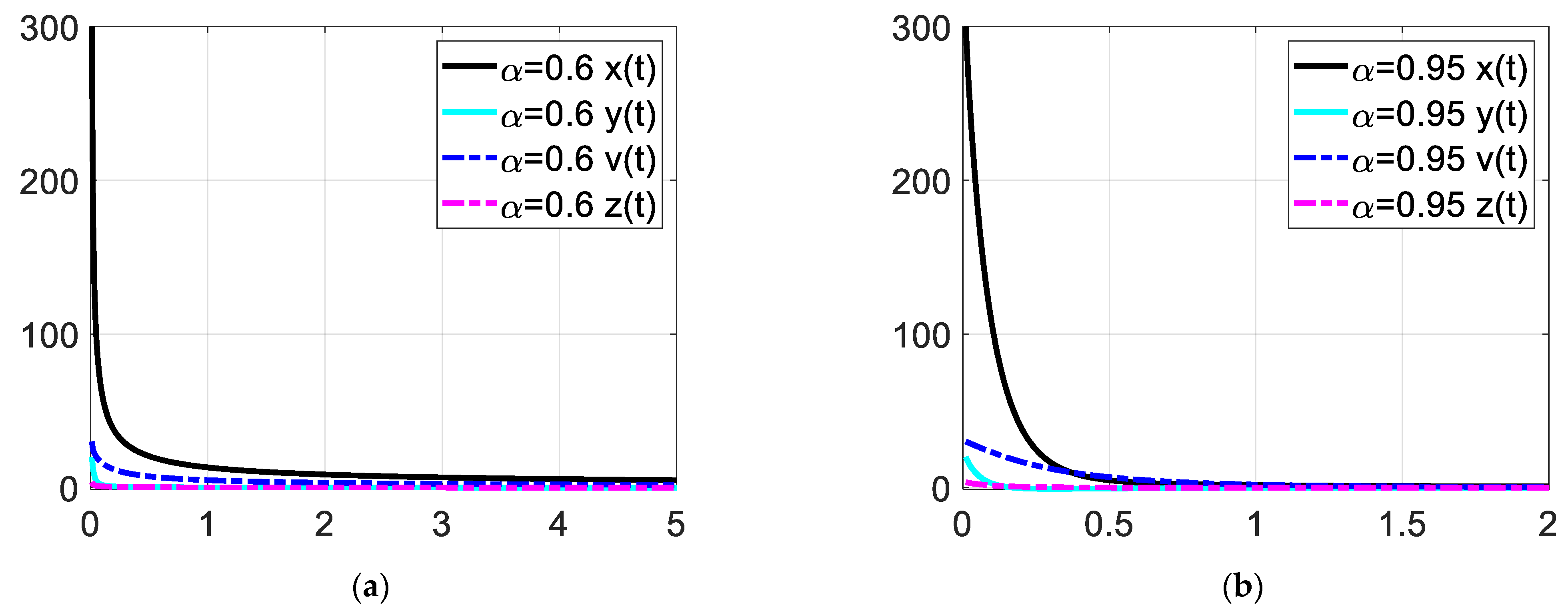
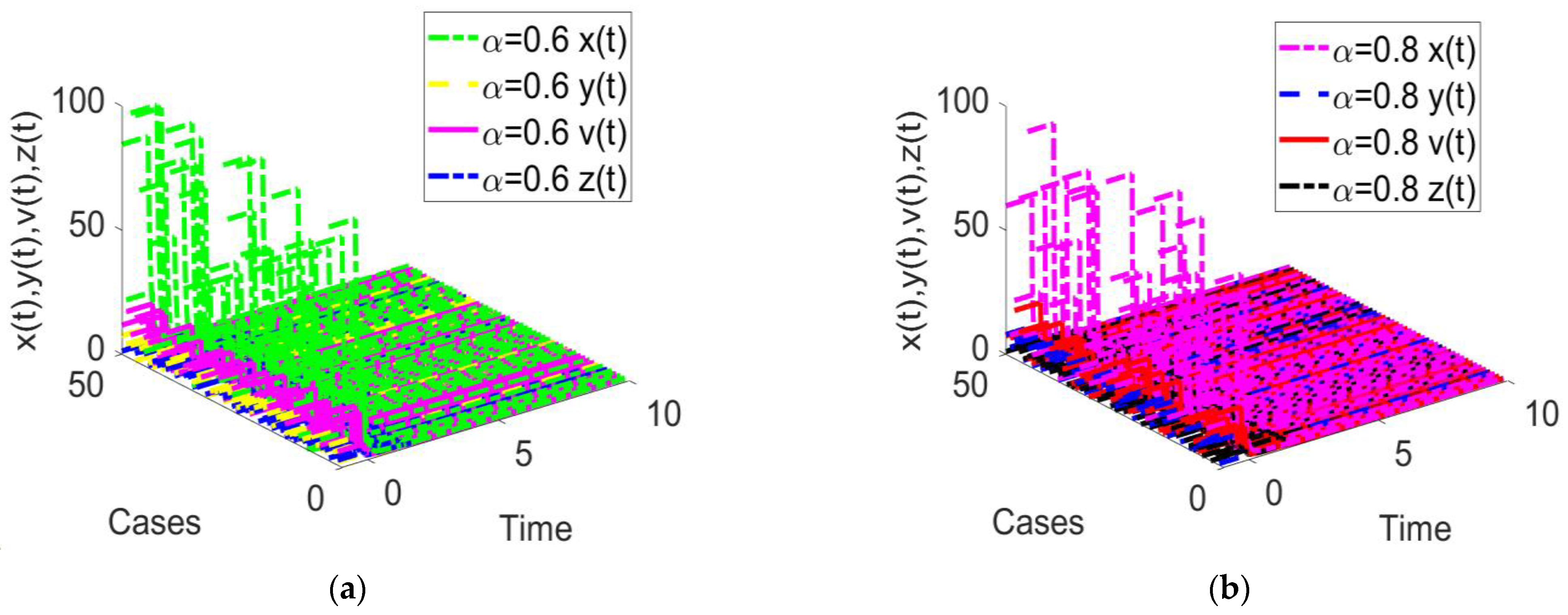
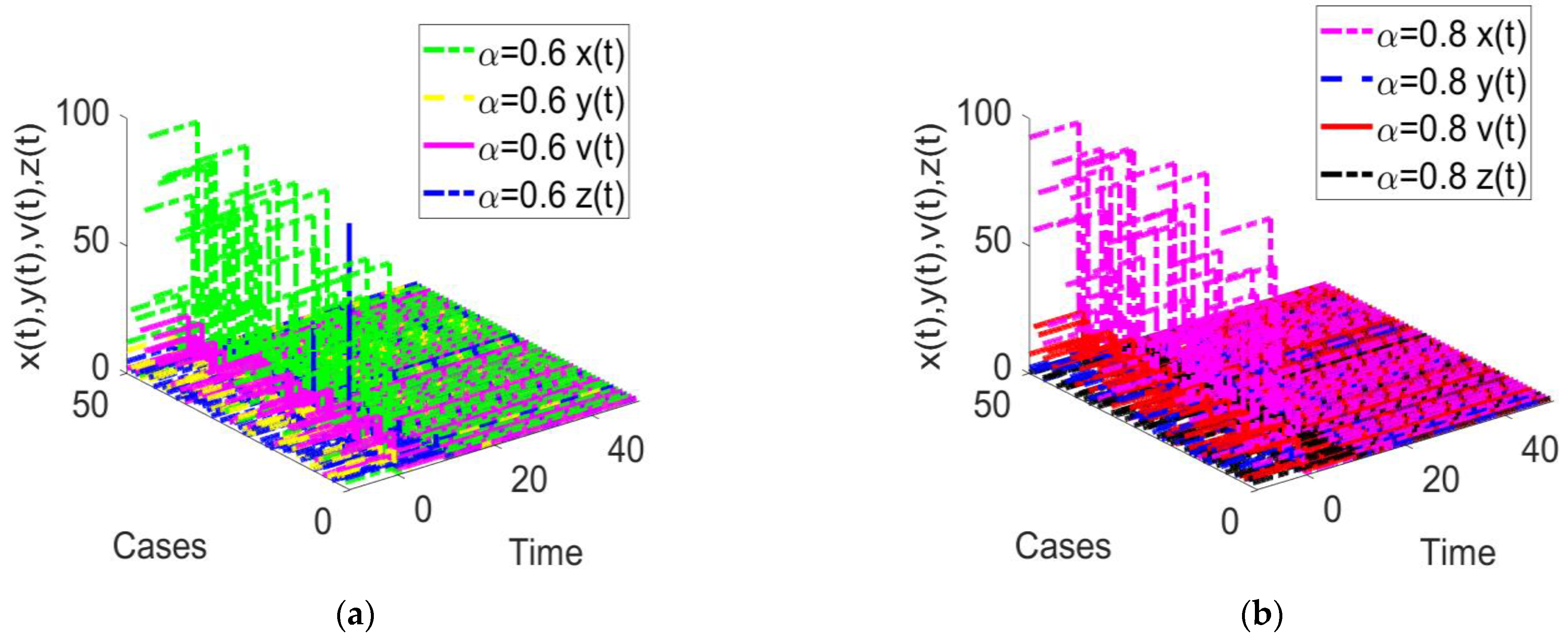
4. Numerical Simulation
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- González, S.F.; Martín, G.J. The neuropathogenesis of AIDS. Nat. Rev. Immunol. 2005, 5, 6981. [Google Scholar]
- Cantara, W.A.; Pathirage, C.; Hatterschide, J.; Olson, E.D.; Musier-Forsyth, K. Phosphomimetic S207D Lysyl–tRNA synthetase binds HIV-1 5′ UTR in an open conformation and increases RNA dynamics. Viruses 2022, 14, 1556. [Google Scholar] [CrossRef] [PubMed]
- AlShamrani, N.H.; Alshaikh, M.A.; Elaiw, A.M.; Hattaf, K. Dynamics of HIV-1/HTLV-I Co-Infection Model with Humoral Immunity and Cellular Infection. Viruses 2022, 14, 1719. [Google Scholar] [CrossRef]
- Ramya, R.; Maheswari, M.C.; Krishnan, K. Modified HIV-1 infection model with delay in saturated CTL immune response. Commun. Math. Biol. Neurosci. 2022, 2022, 77. [Google Scholar]
- Gumbs, S.B.; Berdenis van Berlekom, A.; Kübler, R.; Schipper, P.J.; Gharu, L.; Boks, M.P.; Ormel, P.R.; Wensing, A.M.; de Witte, L.D.; Nijhuis, M. Characterization of HIV-1 infection in microglia-containing human cerebral organoids. Viruses 2022, 14, 829. [Google Scholar] [CrossRef] [PubMed]
- Lu, F.; Zankharia, U.; Vladimirova, O.; Yi, Y.; Collman, R.G.; Lieberman, P.M. Epigenetic Landscape of HIV-1 Infection in Primary Human Macrophage. J. Virol. 2022, 96, e00162-22. [Google Scholar] [CrossRef]
- Wang, J.; Qin, C.; Chen, Y.; Wang, X. Hopf bifurcation in a CTL-inclusive HIV-1 infection model with two time delays. Math. Biosci. Eng. 2019, 16, 2587–2612. [Google Scholar]
- Ahmad, S.; Ullah, A.; Partohaghighi, M.; Saifullah, S.; Akgül, A.; Jarad, F. Oscillatory and complex behaviour of Caputo-Fabrizio fractional order HIV-1 infection model. AIMS Math. 2021, 7, 4778–4792. [Google Scholar] [CrossRef]
- Shrivastava, S.; Ray, R.M.; Holguin, L.; Echavarria, L.; Grepo, N.; Scott, T.A.; Burnett, J.; Morris, K.V. Exosome-mediated stable epigenetic repression of HIV-1. Nat. Commun. 2021, 12, 5541. [Google Scholar] [CrossRef]
- Elaiw, A.M.; Aljahdali, A.K.; Hobiny, A.D. Dynamical Properties of Discrete-Time HTLV-I and HIV-1 within-Host Coinfection Model. Axioms 2023, 12, 201. [Google Scholar] [CrossRef]
- Pradeesh, M.; Manivannan, A.; Lakshmanan, S.; Rihan, F.A.; Mani, P. Dynamical Analysis of Posttreatment HIV-1 Infection Model. Complexity 2022, 2022, 9752628. [Google Scholar] [CrossRef]
- Liu, X.L.; Zhu, C.C. A Non-Standard Finite Difference Scheme for a Diffusive HIV-1 Infection Model with Immune Response and Intracellular Delay. Axioms 2022, 11, 129. [Google Scholar] [CrossRef]
- Dubey, P.; Dubey, U.S.; Dubey, B. Modeling the role of acquired immune responseand antiretroviral therapy in the dynamics of HIV infection. Math. Comput. Simul. 2018, 144, 120–137. [Google Scholar] [CrossRef]
- Brociek, R.; Wajda, A.; Słota, D. Comparison of heuristic algorithms in identification of parameters of anomalous diffusion model based on measurements from sensors. Sensors 2023, 23, 1722. [Google Scholar] [CrossRef]
- Brociek, R.; Słota, D.; Król, M.; Matula, G.; Kwaśny, W. Modeling of heat distribution in porous aluminum using fractional differential equation. Fractal Fract. 2017, 1, 17. [Google Scholar] [CrossRef]
- Abbas, S.; Tyagi, S.; Kumar, P.; Ertürk, V.S.; Momani, S. Stability and bifurcation analysis of a fractional-order model of cell-to-cell spread of HIV-1 with a discrete time delay. Math. Methods Appl. Sci. 2022, 45, 7081–7095. [Google Scholar] [CrossRef]
- Naik, P.A.; Owolabi, K.M.; Yavuz, M.; Zu, J. Chaotic dynamics of a fractional order HIV-1 model involving AIDS-related cancer cells. Chaos Solitons Fractals 2020, 140, 110272. [Google Scholar] [CrossRef]
- Wu, Y.; Ahmad, S.; Ullah, A.; Shah, K. Study of the fractional-order HIV-1 infection model with uncertainty in initial data. Math. Probl. Eng. 2022, 2022, 7286460. [Google Scholar] [CrossRef]
- Arafa AA, M.; Rida, S.Z.; Khalil, M. A fractional-order model of HIV infection: Numerical solution and comparisons with data of patients. Int. J. Biomath. 2014, 7, 1450036. [Google Scholar] [CrossRef]
- Virgin, H.W.; Walker, B.D. Immunology and the elusive AIDS vaccine. Nature 2010, 464, 224–231. [Google Scholar] [CrossRef]
- Rong, L.B.; Gilchrist, M.A.; Feng, Z.L.; Perelson, A.S. Modeling within-host HIV-1 dynamics and the evolution of drug resistance: Trade-offs between viral enzyme function and drug susceptibility. J. Theor. Biol. 2007, 247, 804–818. [Google Scholar] [CrossRef]
- Shu, H.Y.; Wang, L.; Watmough, J. Global stability of a nonlinear viral infection model with infinitely distributed intracellular delays and CTL immune responses. SIAM J. Appl. Math. 2013, 73, 1280–1302. [Google Scholar] [CrossRef]
- Lv, C.F.; Huang, L.H.; Yuan, Z.H. Global stability for an HIV-1 infection model with Beddington-De Angelis incidence rate and CTL immune response. Commun. Nonlinear Sci. Numer. Simul. 2014, 19, 121–127. [Google Scholar] [CrossRef]
- Li, M.Y.; Shu, H.Y. Multiple Stable Periodic Oscillations in a Mathematical Model of CTL Response to HTLV-I Infection. Bull. Math. Biol. 2011, 73, 1774–1793. [Google Scholar] [CrossRef] [PubMed]
- Zhe, Z.; Ushio, T.; Jing, Z.; Wang, Y. A novel asymptotic stability condition for a delayed distributed order nonlinear composite system with uncertain fractional order. J. Frankl. Inst. 2022, 359, 10986–11006. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhang, J.; Cheng, F.; Liu, F.; Ding, C. Dynamic analysis of a novel time-lag four-dimensional fractional-order financial system. Asian J. Control. 2021, 23, 536–547. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, Y.; Zhang, J.; Zhang, H.; Ai, Z.; Liu, K.; Liu, F. Asymptotic stabilization control of fractional-order memristor-based neural networks system via combining vector Lyapunov function with M-matrix. IEEE Trans. Syst. Man Cybern. Syst. 2022, 53, 1734–1747. [Google Scholar] [CrossRef]
- Zhe, Z.; Yaonan, W.; Jing, Z.; Ai, Z.; Cheng, F.; Liu, F. Novel fractional-order decentralized control for nonlinear fractional-order composite systems with time delays. ISA Trans. 2022, 128, 230–242. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhang, J.; Ai, Z.; Cheng, F.; Liu, F. A novel general stability criterion of time-delay fractional-order nonlinear systems based on WILL Deduction Method. Math. Comput. Simul. 2020, 178, 328–344. [Google Scholar] [CrossRef]
- Zhang, Z.; Ai, Z.; Zhang, J.; Cheng, F.; Liu, F.; Ding, C. A general stability criterion for multidimensional fractional-order network systems based on whole oscillation principle for small fractional-order operators. Chaos Solitons Fractals 2020, 131, 109506. [Google Scholar] [CrossRef]
- Chefnaj, N.; Taqbibt, A.; Hilal, K.; Melliani, S.; Kajouni, A. Boundary Value Problems for Differential Equations Involving the Generalized Caputo-Fabrizio Fractional Derivative in λ-Metric Space. Turk. J. Sci. 2023, 8, 24–36. [Google Scholar]
- Singh, J.; Kumar, D.; Hammouch, Z.; Atangana, A. fractional epidemiological model for computer viruses pertaining to a new fractional derivative. Appl. Math. Comput. 2018, 316, 504–515. [Google Scholar] [CrossRef]
- Bayrak, M.A.; Demir, A. On the challenge of identifying space dependent coefficient in spacetime fractional diffusion equations by fractional scaling transformations method. Turk. J. Sci. 2022, 7, 132–145. [Google Scholar]
- Dokuyucu, M.A. Analysis of a novel finance chaotic model via ABC fractional derivative. Numer. Methods Partial. Differ. Equ. 2021, 37, 1583–1590. [Google Scholar] [CrossRef]
- İlhan, E. Analysis of the spread of Hookworm infection with Caputo-Fabrizio fractional derivative. Turk. J. Sci. 2022, 7, 43–52. [Google Scholar]
- Liu, D.; Li, T.; Wang, Y. Adaptive Dual Synchronization of Fractional-Order Chaotic System with Uncertain Parameters. Mathematics 2022, 10, 470. [Google Scholar] [CrossRef]
- Nuez-Perez, J.C.; Adeyemi, V.A.; Sandoval-Ibarra, Y.; Perez-Pinal, F.-J.; Tlelo-Cuautle, E. Maximizing the Chaotic Behavior of Fractional Order Chen System by Evolutionary Algorithms. Mathematics 2021, 9, 1194. [Google Scholar] [CrossRef]
- Alesemi, M.; Iqbal, N.; Botmart, T. Novel Analysis of the Fractional-Order System of Non-Linear Partial Differential Equations with the Exponential-Decay Kernel. Mathematics 2022, 10, 615. [Google Scholar] [CrossRef]
- Motorga, R.; Murean, V.; Ungurean, M.L.; Abrudean, M.; Vălean, H.; Clitan, I. Artificial Intelligence in Fractional-Order Systems Approximation with High Performances: Application in Modelling of an Isotopic Separation Process. Mathematics 2022, 10, 1459. [Google Scholar] [CrossRef]
- Ding, Q.; Abba, O.A.; Jahanshahi, H.; Alassafi, M.O.; Huang, W.H. Dynamical Investigation, Electronic Circuit Realization and Emulation of a Fractional-Order Chaotic Three-Echelon Supply Chain System. Mathematics 2022, 10, 625. [Google Scholar] [CrossRef]
- Culshaw, R.V.; Ruan, S. A delay-di erential equation model of HIV infection of CD4 T-cells. Math. Biosci. 2000, 165, 27–39. [Google Scholar] [CrossRef] [PubMed]
- Ding, Y.; Ye, H. A fractional-order differential equation model of HIV infection of CD4+ T-cells. Math. Comput. Model. 2009, 50, 386–392. [Google Scholar] [CrossRef]
- Yan, Y.; Kou, C. Stability analysis for a fractional differential model of HIV infection of CD4+ T-cells with time delay. Math. Comput. Simul. 2012, 82, 1572–1585. [Google Scholar] [CrossRef]
- Jajarmi, A.; Baleanu, D. A new fractional analysis on the interaction of HIV with CD4+ T-cells. Chaos Solitons Fractals 2018, 113, 221–229. [Google Scholar] [CrossRef]
- Cole, K.S. Electric conductance of biological systems//Cold Spring Harbor symposia on quantitative biology. Cold Spring Harb. Lab. Press 1933, 1, 107–116. [Google Scholar] [CrossRef]
- Anastasio, T.J. The fractional-order dynamics of brainstem vestibulo-oculomotor neurons. Biol. Cybern. 1994, 72, 69–79. [Google Scholar] [CrossRef] [PubMed]
- Wen, Y.; Zhou, X.F.; Zhang, Z.; Liu, S. Lyapunov method for nonlinear fractional differential systems with delay. Nonlinear Dyn. 2015, 82, 1015–1025. [Google Scholar] [CrossRef]
- Li, Y.; Chen, Y.Q.; Podlubny, I. Stability of fractional-order nonlinear dynamic systems: Lyapunov direct method and generalized Mittag–Leffler stability. Comput. Math. Appl. 2010, 59, 1810–1821. [Google Scholar] [CrossRef]
- Li, Y.; Chen, Y.Q.; Podlubny, I. Mittag–Leffler stability of fractional order nonlinear dynamic systems. Automatica 2009, 45, 1965–1969. [Google Scholar] [CrossRef]
- Siljak, D.D. Decentralized Control of Complex Systems; Courier Corporation: North Chelmsford, MA, USA, 2011. [Google Scholar]
- Azoz, S.; Hussien, F.; Abdelsamea, H. Analysis of a fractional order HIV-1 infection model with saturated immune response. Assiut Univ. J. Multidiscip. Sci. Res. 2023, 52, 23–47. [Google Scholar] [CrossRef]

| Rate of the uninfected cells | 260 | |
| Rate parameter of uninfected cell death | 0.2 | |
| Infection rate of uninfected cells by the virus | 0.001 | |
| Infected rate parameter of uninfected cells | 0.0008 | |
| Rate parameter of CTL perishing | 2.5 | |
| Rate parameter of CTL killing infected cells | 0.04 | |
| Rate of each reproducing HIV-1 particle | 1.5 | |
| Per capita rate | 3.2 | |
| Rate numerator parameter of CTL cells multiplying | 0.03 | |
| Rate denominator parameter of CTL cells multiplying | p | 0.8 |
| Rate parameter of CTL cell death | 2.7 |
| Rate of the uninfected cells | 260 | |
| Rate parameter of uninfected cell death | 6.2 | |
| Infection rate of uninfected cells by the virus | 0.001 | |
| Infected rate parameter of uninfected cells | 0.0008 | |
| Rate parameter of CTL perishing | 0.5 | |
| Rate parameter of CTL killing infected cells | 0.04 | |
| Rate of each reproducing HIV-1 particle | 1.5 | |
| Per capita rate | 3.2 | |
| Rate numerator parameter of CTL cells multiplying | 0.03 | |
| Rate denominator parameter of CTL cells multiplying | 0.8 | |
| Rate parameter of CTL cell death | 2.7 | |
| Time delay of the process of interaction of different variables | 0.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Z.; Zhang, Z. Stabilization Control for a Class of Fractional-Order HIV-1 Infection Model with Time Delays. Axioms 2023, 12, 695. https://doi.org/10.3390/axioms12070695
Li Z, Zhang Z. Stabilization Control for a Class of Fractional-Order HIV-1 Infection Model with Time Delays. Axioms. 2023; 12(7):695. https://doi.org/10.3390/axioms12070695
Chicago/Turabian StyleLi, Zitong, and Zhe Zhang. 2023. "Stabilization Control for a Class of Fractional-Order HIV-1 Infection Model with Time Delays" Axioms 12, no. 7: 695. https://doi.org/10.3390/axioms12070695
APA StyleLi, Z., & Zhang, Z. (2023). Stabilization Control for a Class of Fractional-Order HIV-1 Infection Model with Time Delays. Axioms, 12(7), 695. https://doi.org/10.3390/axioms12070695







