Study of a Random Warranty Model Maintaining Fairness and a Random Replacement Next Model Sustaining Post-Warranty Reliability
Abstract
1. Introduction
2. Random Warranty Model to Maintain Fairness
2.1. Warranty Definition
- The warranty service including the former stage warranty and the latter stage warranty sustains the reliability of the product, under which each failure is minimally repaired;
- The former stage warranty is confined to a coverage range formed by the warranty period or the random mission cycle completion, whichever occurs first;
- If the first stage warranty expires at , then the reliability of the related product will be sustained by the second stage warranty whose coverage range is confined to a region formed by the warranty period or the random mission cycle completion, whichever occurs first;
- If the former stage warranty expires at the random mission cycle completion, then the reliability of the related product will still be sustained by the latter stage warranty, whose coverage range is confined to a region formed by the warranty period or the random mission cycle completion, whichever occurs last.
2.2. The Cost Measure Modeling for the Two-Stage 2DFRW
2.2.1. The Cost Measure of the Former Stage Warranty
2.2.2. The Cost Measure of the Latter Stage Warranty
2.2.3. The Cost Measure of the Two-Stage 2DFRW
2.2.4. Derivative Models of the Two-Stage 2DFRW
3. Random Replacement Next Model Sustaining the Post-Warranty Reliability
3.1. The Design of the Random Replacement Next Model
- The product through the two-stage 2DFRW is minimally repaired at each failure before replacement.
- If the random mission cycle is completed before the working time is reached, then the product through the two-stage 2DFRW will be replaced at next random mission cycle completion, i.e., the random mission cycle completion; otherwise, it will be replaced at the working time .
3.2. The Expected Cost Rate
3.2.1. The Length of Renewable Cycle
3.2.2. The Total Cost during the Renewable Cycle
3.2.3. The Expected Cost Rate
3.2.4. Other Expected Cost Rates
4. Numerical Examples
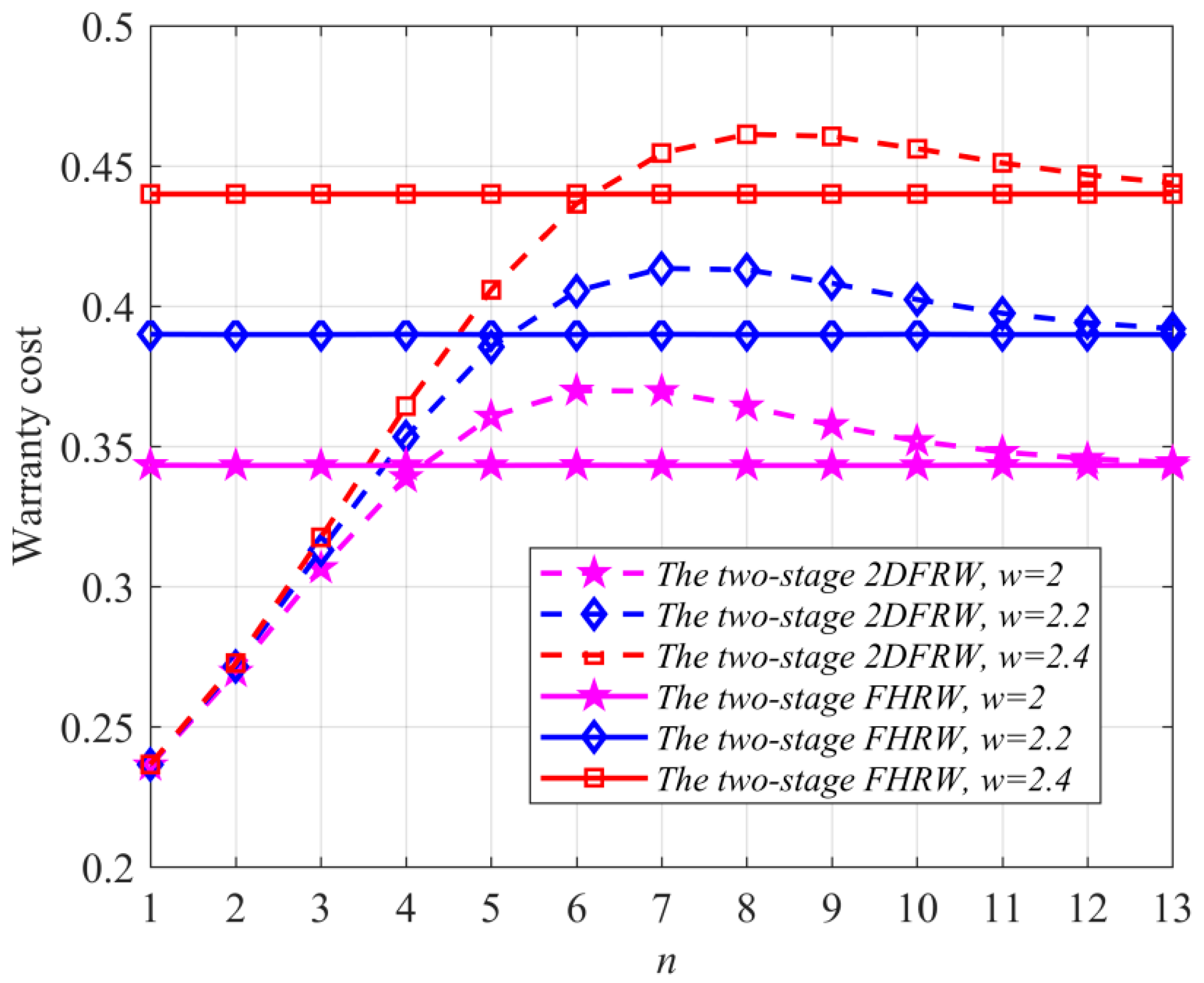
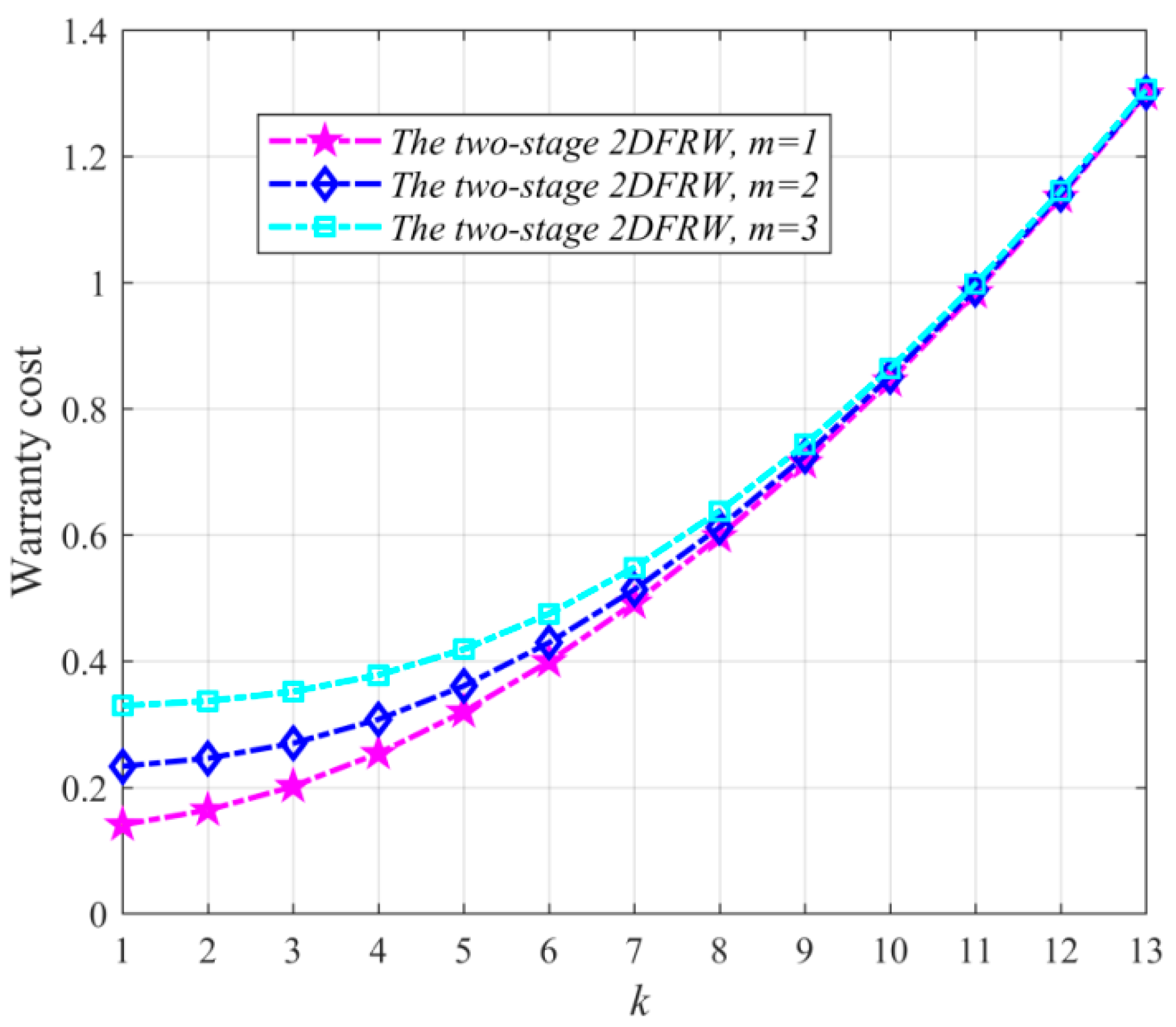
4.1. Exploration of the Characteristics of the Designed Warranty
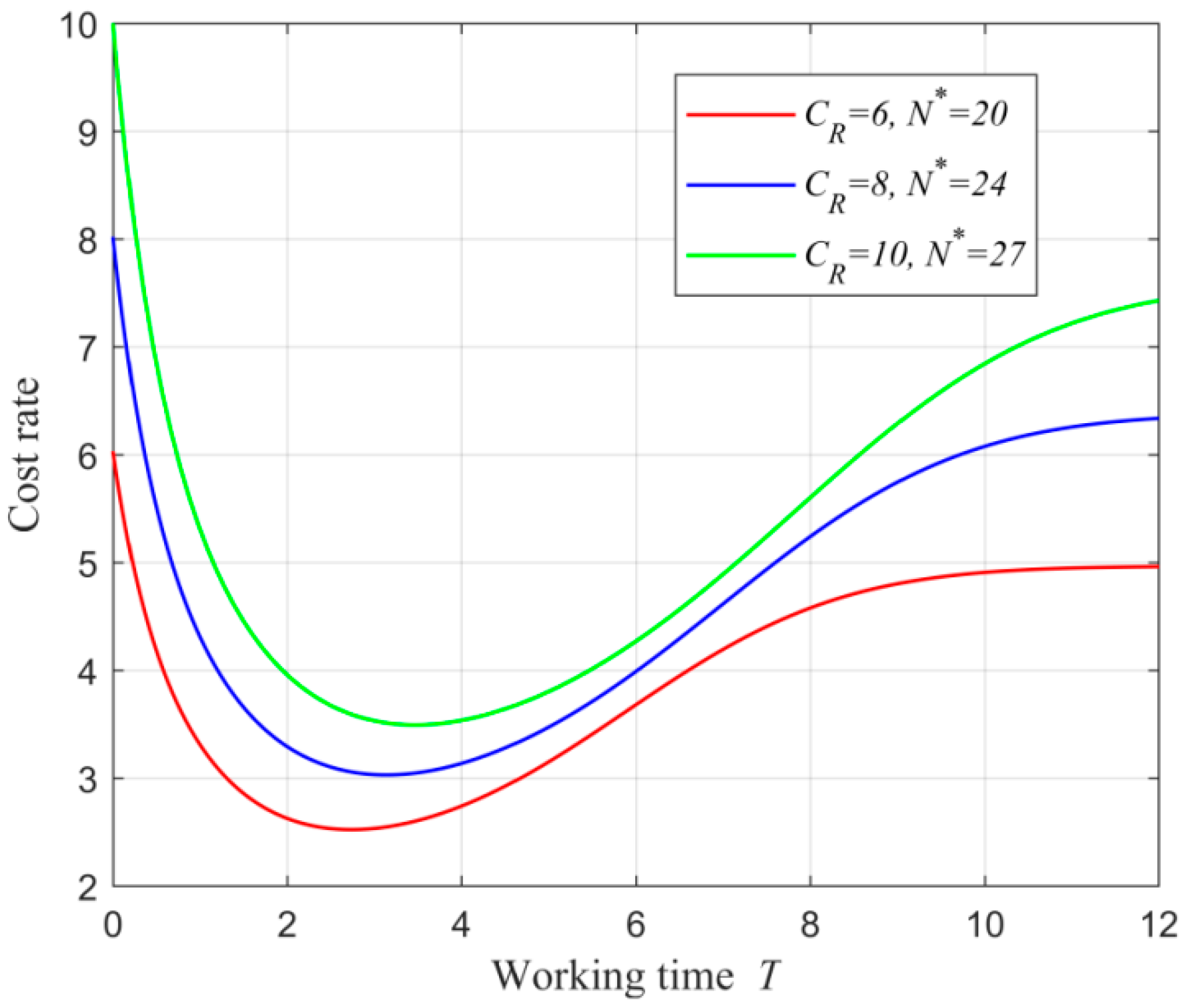
4.2. Exploration of the Characteristics of RNNs
5. Conclusions
- ◆
- Flexible warranty models under the case of the multi-failure mode;
- ◆
- Customized maintenance models to sustain the different post-warranty reliabilities.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Liu, B.; Wu, J.; Xie, M. Cost analysis for multi-component system with failure interaction under renewing free-replacement warranty. Eur. J. Oper. Res. 2015, 243, 874–882. [Google Scholar] [CrossRef]
- Qiao, P.; Shen, J.; Zhang, F.; Ma, Y. Optimal warranty policy for repairable products with a three-dimensional renewable combination warranty. Comput. Ind. Eng. 2022, 168, 108056. [Google Scholar] [CrossRef]
- Chen, C.-K.; Lo, C.-C.; Weng, T.-C. Optimal production run length and warranty period for an imperfect production system under selling price dependent on warranty period. Eur. J. Oper. Res. 2017, 259, 401–412. [Google Scholar] [CrossRef]
- Wang, L.; Pei, Z.; Zhu, H.; Liu, B. Optimising extended warranty policies following the two-dimensional warranty with repair time threshold. Eksploat. Niezawodn. Maint. Reliab. 2018, 20, 523–530. [Google Scholar] [CrossRef]
- Wang, X.; Ye, Z.-S. Design of customized two-dimensional extended warranties considering use rate and heterogeneity. IISE Trans. 2020, 53, 341–351. [Google Scholar] [CrossRef]
- Wang, X.-L. Design and pricing of usage-driven customized two-dimensional extended warranty menus. IISE Trans. 2022, 1–33. [Google Scholar] [CrossRef]
- Ye, Z.; Murthy, D.N.P.; Xie, M.; Tang, L. Optimal burn-in for repairable systems sold with a two-dimensional warranty. IIE Trans. 2013, 45, 164–176. [Google Scholar] [CrossRef]
- Gavish, B.; Sobol, M. Warranty Policy Impact On Net Revenues Due To Optional Purchases. Int. J. Inf. Technol. Decis. Mak. 2010, 9, 507–523. [Google Scholar] [CrossRef]
- Wu, S.; Longhurst, P. Optimising age-replacement and extended non-renewing warranty policies in lifecycle costing. Int. J. Prod. Econ. 2011, 130, 262–267. [Google Scholar] [CrossRef]
- Su, C.; Wang, X. A two-stage preventive maintenance optimization model incorporating two-dimensional extended warranty. Reliab. Eng. Syst. Saf. 2016, 155, 169–178. [Google Scholar] [CrossRef]
- Wang, X.; Li, L.; Xie, M. An unpunctual preventive maintenance policy under two-dimensional warranty. Eur. J. Oper. Res. 2019, 282, 304–318. [Google Scholar] [CrossRef]
- Peng, S.; Jiang, W.; Wei, L.; Wang, X.-L. A new cost-sharing preventive maintenance program under two-dimensional warranty. Int. J. Prod. Econ. 2022, 254, 108580. [Google Scholar] [CrossRef]
- Liu, B.; Pandey, M.D.; Wang, X.; Zhao, X. A finite-horizon condition-based maintenance policy for a two-unit system with dependent degradation processes. Eur. J. Oper. Res. 2021, 295, 705–717. [Google Scholar] [CrossRef]
- Li, H.; Zhu, W.; Dieulle, L.; Deloux, E. Condition-based maintenance strategies for stochastically dependent systems using Nested Lévy copulas. Reliab. Eng. Syst. Saf. 2021, 217, 108038. [Google Scholar] [CrossRef]
- Wang, J.; Qiu, Q.; Wang, H. Joint optimization of condition-based and age-based replacement policy and inventory policy for a two-unit series system. Reliab. Eng. Syst. Saf. 2020, 205, 107251. [Google Scholar] [CrossRef]
- Zhu, W.; Fouladirad, M.; Berenguer, C. Condition-based maintenance policies for a combined wear and shock deterioration model with covariates. Comput. Ind. Eng. 2015, 85, 268–283. [Google Scholar] [CrossRef]
- Qiu, Q.; Maillart, L.M.; Prokopyev, O.A.; Cui, L. Optimal Condition-Based Mission Abort Decisions. IEEE Trans. Reliab. 2022, 1–18. [Google Scholar] [CrossRef]
- Wang, J.; Qiu, Q.; Wang, H.; Lin, C. Optimal condition-based preventive maintenance policy for balanced systems. Reliab. Eng. Syst. Saf. 2021, 211, 107606. [Google Scholar] [CrossRef]
- Zhao, X.; Sun, J.; Qiu, Q.; Chen, K. Optimal inspection and mission abort policies for systems subject to degradation. Eur. J. Oper. Res. 2020, 292, 610–621. [Google Scholar] [CrossRef]
- Zhang, N.; Tian, S.; Cai, K.; Zhang, J. Condition-based maintenance assessment for a deteriorating system considering stochastic failure dependence. IISE Trans. 2022, 1–11. [Google Scholar] [CrossRef]
- Zhang, N.; Fouladirad, M.; Barros, A.; Zhang, J. Condition-based maintenance for a K-out-of-N deteriorating system under periodic inspection with failure dependence. Eur. J. Oper. Res. 2020, 287, 159–167. [Google Scholar] [CrossRef]
- Chen, Y.; Qiu, Q.; Zhao, X. Condition-based opportunistic maintenance policies with two-phase inspections for continuous-state systems. Reliab. Eng. Syst. Saf. 2022, 228, 108767. [Google Scholar] [CrossRef]
- Shang, L.; Si, S.; Sun, S.; Jin, T. Optimal warranty design and post-warranty maintenance for products subject to stochastic degradation. IISE Trans. 2018, 50, 913–927. [Google Scholar] [CrossRef]
- Shang, L.; Qiu, Q.; Wang, X. Random periodic replacement models after the expiry of 2D-warranty. Comput. Ind. Eng. 2021, 164, 107885. [Google Scholar] [CrossRef]
- Afsahi, M.; Kashan, A.H.; Ostadi, B. A Bi-Objective Simulation-Based Optimization Approach for Optimizing Price, Warranty, and Spare Part Production Decisions Under Imperfect Repair. Int. J. Inf. Technol. Decis. Mak. 2021, 20, 903–932. [Google Scholar] [CrossRef]
- Park, M.; Jung, K.M.; Park, D.H. A Generalized Age Replacement Policy for Systems Under Renewing Repair-Replacement Warranty. IEEE Trans. Reliab. 2015, 65, 604–612. [Google Scholar] [CrossRef]
- Park, M.; Pham, H. Cost models for age replacement policies and block replacement policies under warranty. Appl. Math. Model. 2016, 40, 5689–5702. [Google Scholar] [CrossRef]
- Zhao, X.; Fan, Y.; Qiu, Q.; Chen, K. Multi-criteria mission abort policy for systems subject to two-stage degradation process. Eur. J. Oper. Res. 2021, 295, 233–245. [Google Scholar] [CrossRef]
- Ye, S.Z.; Xie, M. Stochastic modelling and analysis of degradation for highly reliable products. Appl. Stoch. Model. Bus. Ind. 2015, 31, 16–32. [Google Scholar] [CrossRef]
- Qiu, Q.; Cui, L. Gamma process based optimal mission abort policy. Reliab. Eng. Syst. Saf. 2019, 190, 106496. [Google Scholar] [CrossRef]
- Yang, L.; Chen, Y.; Qiu, Q.; Wang, J. Risk Control of Mission-Critical Systems: Abort Decision-Makings Integrating Health and Age Conditions. IEEE Trans. Ind. Inform. 2022, 18, 6887–6894. [Google Scholar] [CrossRef]
- Zhao, X.; Chai, X.; Sun, J.; Qiu, Q. Joint optimization of mission abort and protective device selection policies for multistate systems. Risk Anal. 2022, 42, 2823–2834. [Google Scholar] [CrossRef] [PubMed]
- Qiu, Q.; Cui, L.; Wu, B. Dynamic mission abort policy for systems operating in a controllable environment with self-healing mechanism. Reliab. Eng. Syst. Saf. 2020, 203, 107069. [Google Scholar] [CrossRef]
- Qiu, Q.; Kou, M.; Chen, K.; Deng, Q.; Kang, F.; Lin, C. Optimal stopping problems for mission oriented systems considering time redundancy. Reliab. Eng. Syst. Saf. 2020, 205, 107226. [Google Scholar] [CrossRef]
- Qiu, Q.; Cui, L. Optimal mission abort policy for systems subject to random shocks based on virtual age process. Reliab. Eng. Syst. Saf. 2019, 189, 11–20. [Google Scholar] [CrossRef]
- Qiu, Q.; Cui, L.; Dong, Q. Preventive maintenance policy of single-unit systems based on shot-noise process. Qual. Reliab. Eng. Int. 2019, 35, 550–560. [Google Scholar] [CrossRef]
- Shang, L.; Qiu, Q.; Wu, C.; Du, Y. Random replacement policies to sustain the post-warranty reliability. J. Qual. Maint. Eng. 2022; ahead-of-print. [Google Scholar] [CrossRef]
- Shang, L.; Yu, X.; Wang, X.; Qiu, Q. Study of A Two-stage Random Warranty to Maintain Fairness. Procedia Comput. Sci. 2022, 214, 437–440. [Google Scholar] [CrossRef]
- Shang, L.; Liu, B.; Cai, Z.; Wu, C. Random maintenance policies for sustaining the reliability of the product through 2D-warranty. Appl. Math. Model. 2022, 111, 363–383. [Google Scholar] [CrossRef]
- Nakagawa, T. Maintenance Theory of Reliability; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Zhao, X.; Qian, C.; Nakagawa, T. Comparisons of replacement policies with periodic times and repair numbers. Reliab. Eng. Syst. Saf. 2017, 168, 161–170. [Google Scholar] [CrossRef]
- Qiu, Q.; Cui, L.; Gao, H. Availability and maintenance modelling for systems subject to multiple failure modes. Comput. & Ind. Eng. 2017, 108, 192–198. [Google Scholar]
- Barlow, R.E.; Proschan, F. Mathematical Theory of Reliability; John Wiley & Sons: New York, NY, USA, 1965. [Google Scholar]
- Sheu, S.-H.; Liu, T.-H.; Zhang, Z.-G. Extended optimal preventive replacement policies with random working cycle. Reliab. Eng. Syst. Saf. 2019, 188, 398–415. [Google Scholar] [CrossRef]
- Zhang, Q.; Yao, W.; Xu, P.; Fang, Z. Optimal age replacement policies of mission-oriented systems with discounting. Comput. Ind. Eng. 2023, 177, 109027. [Google Scholar] [CrossRef]
| 2 | 26 | 3.1342 | 3.0287 | 25 | 3.1279 | 3.0495 | 24 | 3.1270 | 3.0513 |
| 3 | 24 | 2.8049 | 2.9686 | 24 | 2.7775 | 3.0329 | 24 | 2.7748 | 3.0411 |
| 4 | 23 | 2.5050 | 2.8612 | 23 | 2.4250 | 2.9931 | 22 | 2.4142 | 3.0174 |
| 2 | 16 | 1.9893 | 3.0398 | 24 | 2.7775 | 3.0329 | 30 | 3.1705 | 2.9773 |
| 3 | 15 | 1.7025 | 3.2991 | 23 | 2.6090 | 3.2694 | 30 | 3.1575 | 3.0477 |
| 4 | 14 | 1.4184 | 3.5938 | 22 | 2.4474 | 3.5239 | 30 | 2.9298 | 3.3493 |
| The Optimal RNN | The Optimal Random Periodic Replacement | |||
|---|---|---|---|---|
| 4 | 4.1707 | 2.9773 | 4.0592 | 3.1639 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shang, L.; Zhang, N.; Yang, L.; Shang, L. Study of a Random Warranty Model Maintaining Fairness and a Random Replacement Next Model Sustaining Post-Warranty Reliability. Axioms 2023, 12, 258. https://doi.org/10.3390/axioms12030258
Shang L, Zhang N, Yang L, Shang L. Study of a Random Warranty Model Maintaining Fairness and a Random Replacement Next Model Sustaining Post-Warranty Reliability. Axioms. 2023; 12(3):258. https://doi.org/10.3390/axioms12030258
Chicago/Turabian StyleShang, Lifeng, Nan Zhang, Li Yang, and Lijun Shang. 2023. "Study of a Random Warranty Model Maintaining Fairness and a Random Replacement Next Model Sustaining Post-Warranty Reliability" Axioms 12, no. 3: 258. https://doi.org/10.3390/axioms12030258
APA StyleShang, L., Zhang, N., Yang, L., & Shang, L. (2023). Study of a Random Warranty Model Maintaining Fairness and a Random Replacement Next Model Sustaining Post-Warranty Reliability. Axioms, 12(3), 258. https://doi.org/10.3390/axioms12030258







