Abstract
In this paper we generalize the allocation rule (point solution or value) known as the mixed value by introducing the weighted mixed value.The proposed solution assigns value in graph games where players, and/or links, have weights representing asymmetries of the players, and different flows, lengths, emotional intensities, trust in the transmission of the information, etc. in the links. We present several characterizations of this value using properties, such as mixed component efficiency, weighted mixed fairness, weighted balanced contributions and weighted balanced link contributions. These properties were inspired by the classical properties used to characterize the Myerson value and the position value.
MSC:
05C20; 91A12; 91A43
1. Introduction
A cooperative game with transferable utility or a TU-game is a model for a situation in which a set of actors can obtain benefits by cooperating. Any coalition (subset of players) is feasible and gets a payoff (a real number interpreted as the maximum gains they can obtain regardless of the strategies of the players outside the coalition) if it is formed. Coalition payoffs are assumed to be transferable between players. One of the main issues in cooperative game theory is how to obtain a reasonable distribution of the value of the grand coalition among all the players. The Shapley value [] is one of the most prominent allocation rules (point solution or value) for players in a TU-game. It assigns each player a convex linear combination of his or her marginal contributions to different coalitions (the value of the coalition when he incorporates minus the value of the coalition without him).
In this paper we deal with TU-games with cooperation restricted by a graph, also called communication situations or graph games.
The first attempt in this setting was due to []. He defined the graph-restricted game in which the value of a coalition is the sum of the values of its maximally connected (in the graph) subcoalitions. Given the prominence of the Shapley value as the allocation rule for players in a TU-game, Myerson applied it to this graph restricted game obtaining an allocation rule, which is now known as the Myerson value [,].
The position value is another allocation rule for TU-games with cooperation restricted by a graph, introduced by [,]. They used a different approach from Myerson, defining another graph-restricted game, the so called link game, a new TU-game in which the players are the links of the graph and the coalitions are its subgraphs. The outcome of a subgraph coincides with the payoff given to the grand coalition by the restricted game of Myerson to that subgraph. Then, the position value assigns to each player half of the Shapley value (in the link game) of all the links he or she is involved in.
Since their introduction, the Myerson value and the position value have received incessant attention. Most recent works have obtained characterizations in different settings [,,,,,].
Another value, the mixed value, that allocates worth not only to players, but also to links in a communication situation, was introduced and characterized in []. They defined the corresponding restricted TU-game. In it, the actors are both the players in the original game and the links in the graph. The value of a coalition (of players and links) coincides with the sum of the values (in the original game) of the maximally connected subcoalitions of the players in the graph given by the links. The mixed value is the Shapley value of the previous restricted game. They characterized this allocation rule using efficiency, additivity in the game, the superfluous link property and anonymity.
Mixed value has received less attention in the literature, and one of the aims of this paper was to further analyze it. Recently, reference [] used the mixed value to analyze the impact of intermediaries in a negotiation.
In this paper we present a generalization of this mixed value to take into account that players and/or links can have weights representing a priori differences between them. As an example, these link weights can represent different flows, lengths, latencies in the information transmission, emotional intensities, trust in the transmission of information or even probabilities of relation. Similarly, players can have asymmetries in bargaining or cooperation abilities.
In all the three previously referred to allocations rules, the Shapley value [] of the corresponding newly defined game (the graph restricted game, the link game or the pseudogame of players and links) was always used.
In this paper we propose the use of the weighted Shapley value [] to allocate worth in the pseudogame, when players and links have associated weights. The obtained allocation rule is named weighted mixed value. As mentioned above, a crucial difference between the mixed value and the Myerson and position values is that the former allows us to know how much of the value of the grand coalition should be allocated to the links. This can be interpreted as the costs for the players of maintaining the communication channels, paying the intermediaries who own the links, the importance of a partner’s relationships in a social network, etc. In this paper we intend to go deeper into the measurement of the value, not only of the players, but also of these connections, to characterize the value and to address situations in which the different communications present symmetries due to length, capacity, emotional intensity, probability, etc. The obtained results could be useful in distributing maintenance costs between different towns and different road sections in a terrestrial communications network, distributing income among the owners of suburban buses, commuter trains and the metro, and, also, to strategically decide on the need to build a new pipeline (see Example 1) (at the time of writing this paper, the European Union was addressing the strategic problem of building a new gas pipeline to reduce dependence on external countries), distributing benefits between telephone companies from different countries who provide communications, and measuring centrality of individuals and their relationships in a social network, etc.
We present several characterizations of this value using the following properties: mixed component efficiency, weighted mixed fairness, weighted balanced contributions and weighted balanced link contributions. They were inspired by well-known properties, such as fairness and balanced contributions used to characterize the Myerson value [,] and the balanced link contributions used to characterize the position value [].
The obtained characterizations are also useful in the particular case in which all the players and links have no weights. Then, in fact, in this paper we also obtain new characterizations for the mixed value.
The remainder of the paper is organized as follows. In Section 2 we include some preliminaries and notation on cooperative TU-games, graphs and communication situations. Section 3 is devoted to obtaining the dividends of the pseudogame, or mixed game, and to introducing the weighted mixed value. In Section 4 we present several characterizations of this value. The paper ends with a section of conclusions, final remarks and references.
2. Preliminaries
2.1. Cooperative TU-Games
An n-person TU-game (cooperative game with transferable utility) is an ordered pair where is the set of players and v, the characteristic function, is a map assigning to each coalition the payoff, , of the members in S if they cooperate.
We denote the vector space of all n-person TU-games by . The family with the characteristic functions , , defined as
is a basis of , known as the unanimity games basis. Then, every characteristic function v can be uniquely written as
These coordinates of , in such a basis, , are known as the Harsanyi dividends [], and can be calculated from the value of the coalitions in the following way (the cardinality of the subsets of N is denoted by ),
An allocation rule on assigns a specific payoff to each player in the game. The Shapley value [], , is a well-known allocation rule whereby the allocation of each player i is obtained as a convex linear combination of the marginal contributions of the player to different coalitions
Alternatively, it can be obtained as
i.e., as the sum of the proportional part (to the size of the coalition) of the dividend of different coalitions to which the player belongs.
In [] the weighted Shapley value is defined, , in which for a vector of positive weights ,
i.e., the weighted Shapley value of a player is the sum of the dividends of the coalitions to which the player belongs multiplied by the proportional part of the weight of the player with respect to the sum of the weights of the members of the coalition. Simplifying, while the dividends in the Shapley value are distributed proportionally to the size of the coalition, in the case of the weighted Shapley value they are distributed proportionally to the weights.
2.2. Graphs
A () is a pair in which is the set of nodes and . Each is a link representing a direct communication between i and j.
We say that i and j are in if there exists with and such that , for . A set is connected in if it is a singleton or if every pair of nodes in S is connected in the graph with .
A maximal connected subset in is a connected component. For denotes the partition of S in connected components in the graph . For , denotes the partition of N in connected components in the graph .
Given a graph , the following subgraphs of are relevant in this paper: the subgraph in which the link has disappeared, ; the subgraph of the links incident on , , with ; and the subgraph in which has broken all links, so becoming isolated, , with .
2.3. Communication Situations
A communication situation, a graph game or a game with communication restricted by a graph can be modeled by means of a triple where is a TU-game and is a network. The set of all communication situations with N as the set of nodes/players is denoted by .
For communication situations, refs. [,] introduced a graph-restricted game, , as follows:
Later, also for a communication situation , with a zero-normalized game, references [,] introduced the link game, in which the characteristic function was given by:
In [] another game was introduced, a pseudogame, or mixed game , in which the pseudo-players were either players in the game or links in the network. It is defined as:
where given and with and , we denote by the order given by and . If but or (or both), then we will write .
The Myerson value, [,] and the position value, [,] are two well known allocation rules for communication situations that assign value to players.
They are defined as
and
with
where the cardinality of the sets of links and is denoted by and .
A mixed allocation rule, the mixed value, , assigning payoff to players and links is introduced in []. It is given by:
Myerson characterized his value in terms of component efficiency and fairness in []. Later, reference [] obtained another characterization substituting fairness with balanced contributions.
An allocation rule satisfies component efficiency if it distributes the value of each component among the members of that component and, therefore, there is no transfer of utility between components. It satisfies fairness if, when two players break their link, the payoff of both is equally modified. It satisfies balanced contributions if the effect on the payoff that one player isolating makes to another is the same as the other player’s isolating makes to him or her.
Formally, an allocation rule on , i.e., a map , representing the outcome for a player i in the communication situation , satisfies:
-Component efficiency [] if, and all .
-Fairness [] if, for all and every ,
-Balanced contributions [] if, for all and all .
Recent and interesting papers on the fairness property are [,], and on the balanced contributions property are [,,,].
Slikker characterized the position value in terms of component efficiency and balanced link contributions in [].
An allocation rule satisfies balanced link contributions if the sum of the effects generated by removing, one by one, the links of a player on another player is equal to what this other player would generate in he or she acting the same.
Formally, an allocation rule , on satisfies the balanced link contributions property, if, given , and , it holds that .
Extensions of the balanced link contributions property have been used to characterize different generalizations of the position value, as in [,,,,].
The mixed value is characterized using efficiency, additivity in the game, the superfluous link property and anonymity in [].
A rule (for players and links) satisfies additivity if the allocation in a communication situation, in which the game is the sum of TU-games (ceteris paribus), coincides with the sum of the allocations in the respective communication situations. Formally, on is additive if for , , , .
An allocation rule (for players and links) satisfies the superfluous link property if the allocation does not change when eliminating links that are null players in the pseudogame. Finally, a rule on for players and links satisfies anonymity if the allocation only depends on the number of non-isolated players and links.
One of the aims of this paper is to obtain new characterizations of the mixed value, based on properties close to fairness, balanced contributions and balanced link contributions. As mentioned, these properties are prominent in the literature on communication situations. Furthermore, these new characterizations permit us to relate the three aforementioned values. Moreover, additivity is not an appealing property, especially in economy contexts, where the sum of TU-games may not have interpretation. Thus, new characterizations avoiding additivity can be useful in such contexts.
3. The Weighted Mixed Value
In this section we introduce the weighted mixed value as an allocation rule for communication situations in which players are possibly not symmetric, having different weights and also where the links can have weights representing different capacities, flows, distances, etc. The defined rule allocates the weighted Shapley value [] of the mixed game defined in [].
To motivate this rule let us consider the following example.
Example 1.
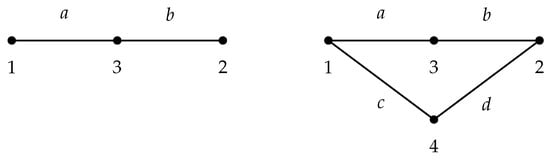
Suppose country 1 produces gas and country 2 uses it in its industry. If 1 and 2 agree, they can make a joint unit profit when 1 sells the gas to 2. However, in order to move the gas from 1 to 2, a pipeline is needed that passes through country 3, which does not need gas, because it uses other types of energy in its industry. This situation can be modeled by with , and .
The high cost for players 1 and 2 to pay 3 and maintain the pipeline makes 1 and 2 consider building another pipeline through country 4, which also does not need gas. Moreover, this new pipeline would have twice the capacity of the existing one. Then, this new situation can be modeled by with , and .
A representation of and is given in Figure 1.

Figure 1.
Graphs and .
The pseudogames are given by:
We will suppose that the weights of the links a and b are, respectively, , and the weights for c and d are . We assume equal unitary weight for the countries. It is not easy, in general, to assign weights to the links. In this case we suppose that the weight is the pipeline capacity, i.e., the amount of gas that moves per unit of time, flow rate, between the entry and exit points of the pipeline, considering given the entry and exit pressures.
For the communication situation we have an allocation, the weighted Shapley value of , of
i.e., players 1 and 2 must reward with to the intermediation of country 3 in the connection of the pipeline and with to the owners of the pipeline. This quantity can also be seen as the cost of the maintenance of the pipeline.
For the communication situation we have an allocation, the weighted Shapley value of , of
In this way (excluding the cost of creating the pipeline) 1 and 2 must face a cost of to reward players 3 and 4, and a cost of a pipeline of Thus, to have alternatives reduces the cost for players and weakens the intermediaries.
Before characterizing the value, let us obtain the pseudogame for communication situations in which the game is a unanimity one. The obtained result is useful to calculate the weighted mixed value and also to characterize it. To do this, first we introduce the definition of minimal connection set-graph for a coalition in a graph.
Definition 1.
Given and , we say that with is a connection set-graph of S in , if is a connected graph. We say that , a connection set-graph of S in , is minimal if, for all with , is not a connection set-graph of S in .
Given , we denote the family, occasionally empty, of the minimal connection set-graphs of S in .
Example 2.
Consider the communication situations and as in the Example 1.
For , the unique minimal connection set-graph of S in is .
For , the minimal connection set-graphs of S in are , .
Proposition 1.
For , with the unanimity game of S in , we have:
if , and (the null game), otherwise.
We denote if for the games of the unanimity basis in .
Proof.
Suppose . The other case is trivial.
For , we have that
On the other hand, using the right hand side of (3)
Thus, the result is proved. □
Corollary 1.
For , with the unanimity game of S in , and , we have
Example 3.
In the following we define the concept of mixed allocation rule in and we introduce the weighted mixed value, which assigns to each player in a communication situation the weighted Shapley value of the pseudogame .
Definition 2.
A mixed allocation rule φ on is a map that assigns to every communication situation a vector containing the payoffs of the players (nodes), , , and the value of the links, , , in the communication situation.
Definition 3.
Given a communication situation , suppose there is a vector such that its components are the positive weights of the players of the game and the links of the graph. Then, the weighted mixed value of , is defined as,
Remark 1.
In the special case in which all the weights are equal to one (in fact, it suffices if they are all equal), the weighted mixed value coincides with the mixed value of [].
4. Characterizing the Weighted Mixed Value
In this section we obtain three characterizations of the weighted mixed value (that also apply in the particular case of the mixed value), using the following five properties: mixed component efficiency, weighted mixed fairness, weighted balanced contributions, weighted mixed balanced contributions and weighted balanced link contributions.
The mixed component efficiency property states that the value of a connected component should be shared among the members and the links of the component.
Definition 4.
A mixed allocation rule, φ, defined on satisfies mixed component efficiency if, for each ,
The weighted mixed fairness property is, of course, inspired by the Myerson’s fairness. It states that when two players break their link, the ratio between the variation in their payoffs coincides with the ratio of their weights. Moreover, for each one of these two players, the ratio between the variation of his or her payoff and the value of the link also coincides with the ratio of the respective weights.
Definition 5.
A mixed allocation rule on , φ, satisfies weighted mixed fairness, if for every , every vector of weights λ, and every directly connected pair of players with ,
and
Remark 2.
In the special case in which we obtain the mixed fairness property that, for each pair of directly connected players , can be written as:
In the following definition we introduce the weighted balanced contributions property that generalizes the property introduced in [].
Definition 6.
A mixed allocation rule, φ, on satisfies the weighted balanced contributions property, if for each and every vector of weights λ, it holds that
Remark 3.
In the special case in which the previous property becomes the classical balanced contributions property of [].
The weighted mixed balanced contributions property establishes that the effect in a link of the isolation of a player equals the effect in the same player if that link is broken.
Definition 7.
A mixed allocation rule, φ, on satisfies the weighted mixed balanced contributions property, if for and and a vector of weights, λ, it holds that
The weighted balanced link contributions property adapts the property of [] to this weighted setting.
Definition 8.
An allocation rule, φ, on satisfies the weighted balanced link contributions property, if, given , a vector of weights λ, and , it holds that
Remark 4.
In the special case in which the previous property becomes the classical balanced link contributions property of [].
Proposition 2.
The weighted mixed value, , satisfies mixed component efficiency, weighted mixed fairness, weighted balanced contributions, weighted mixed balanced contributions and weighted balanced link contributions.
Proof.
- (a)
- Let us prove that satisfies mixed component efficiency. Given , a vector of weights and , by definitionAs the weighted Shapley value is efficient:
- (b)
- As the weighted mixed value is clearly linear (in the game), to prove that satisfies weighted mixed fairness, it is sufficient to see that the property holds for communication situations of the form with the unanimity game of the coalition . Suppose is the vector of weights. Then,where is the pseudogame in whichThus, for and ,is zero, or is the weighted Shapley value of a linear combination of unanimity games, such that l belongs to in all of them. If the difference is zero, the result is trivial. In another case, taking into account that the weighted Shapley value of each player or link is proportional to his, her or its weight, we haveandand, thus, the result is proved.
- (c)
- Let us prove that satisfies weighted balanced contributions. For , andandare both zero, or the weighted Shapley value of a linear combination of unanimity games . The property trivially holds if both quantities vanish. In another case, as the weighted Shapley value assigns to each player a quantity proportional to his weight, we have thatand thus the result.
- (d)
- The proof of satisfies weighted mixed balanced contributions follows immediately from the previous one, considering two players and a link and reproduces the argument.
- (e)
- To prove that satisfies weighted balanced link contributions we use the linearity (in the game) of the mixed weighted value and, thus, it is sufficient to prove that the property holds for with, the characteristic function of the unanimity game of . Suppose and is a vector of weights. Aswe have, for that is a linear combination of unanimity games such that in all of them. Thusis the value of i in all unanimity games in which is present. However, in these unanimity games j is also present as it is incident on the different arcs . By symmetry, the variation of value of jcorresponds to the same unanimity games and, thus, both values are proportional to the weights, which completes the proof.
□
Theorem 1.
The weighted mixed value, , is the unique mixed allocation rule on satisfying mixed component efficiency and weighted mixed fairness.
Proof.
It has already been proved that the weighted mixed value satisfies these two properties. Reciprocally, consider a mixed allocation rule, , on satisfying both properties. Suppose , , and is a vector of weights. The proof uses induction on , the cardinally of . If , then C is necessarily a singleton, the value of which is uniquely determined using the mixed component efficiency property. Suppose the result is proved for and consider . If C is a singleton the value is again uniquely determined. Then, suppose a sequence of nodes , , …, exist, such that
Then,
which, using the induction hypothesis, is equal to
As satisfies the weighted mixed fairness property,
and, thus,
or
Using this argument iteratively, we have
for all . As we can label the nodes in C, , without loss of generality, we have
for . Moreover, for each and one of the nodes on which l is incident, we have
or
the last equality holds because of the induction hypothesis.
The linear equations in (4), the equations in (5) and the mixed component efficiency equation form a linear system in independent equations with variables, which has a unique solution, . The proof of the independence of the equations is given in the Appendix A. □
A trivial consequence of previous theorem is the following corollary that introduces a new characterization for the mixed value defined in [].
Corollary 2.
The mixed value is the unique mixed allocation rule on satisfying mixed component efficiency and mixed fairness.
Theorem 2.
The weighted mixed value is the unique mixed allocation rule on satisfying mixed component efficiency, weighted balanced contributions and weighted mixed balanced contributions.
Proof.
It has already been proved that the weighted mixed value satisfies these three properties. Reciprocally, let us suppose is a mixed allocation rule satisfying them, , is a vector of weights and . We prove that , by induction on , the cardinality of .
If , the result is trivial using the mixed efficiency in the (singleton) connected component C. Suppose the result is true for and consider with . If C is a singleton, again the mixed efficiency determines the value of the node-player. Otherwise, for , as satisfies the weighted balanced contributions property:
or equivalently
Using the induction hypothesis (recall that and as , a connected component),
and using that also satisfies the weighted balanced contributions property,
and, thus,
for all . Moreover, as satisfies the weighted mixed balanced contributions property, given and
Then,
the last equality holding by the induction hypothesis. If and, without loss of generality, , from (6), (7) and the mixed component efficiency we have the following linear system with in the variables , and :
All these equations are linearly independent (the proof mimics the corresponding Theorem 1 and then it is omitted) and thus the system has a unique solution that necessarily coincides with . □
Corollary 3.
The mixed value defined in [] is the unique mixed allocation rule on satisfying mixed component efficiency, balanced contributions and mixed balanced contributions.
The following theorem introduces a third characterization of the weighted mixed value. The proof is straightforward from the proof of the previous theorem and is omitted.
Theorem 3.
The weighted mixed value is the unique allocation rule on satisfying mixed component efficiency, weighted balanced link contributions and weighted mixed balanced contributions.
Corollary 4.
The mixed value defined in [] is the unique allocation rule on satisfying mixed component efficiency, balanced link contributions and mixed balanced contributions.
5. Conclusions and Final Remarks
In this paper we introduced the weighted mixed value to allocate value for players and links in communication situations in which players and/or links have different importance. In this way, the defined value is useful to assign an outcome in situations in which players and their relations have asymmetrical importance, such as the following: social networks, in which players and the intensity of the relations have different importance, wireless networks with different information trust, network settings with variable flows, peer-to-peer data transfer, etc.
We characterized the value using variations of the classical properties for graph games, such as component efficiency, fairness, balanced contributions and balanced link contributions. The different characterizations obtained also hold for the mixed value (when it is assumed that players and links have no different weights). In Table 1 we present comparison of the properties satisfied by this value and the mixed value, the Myerson value and the position value.

Table 1.
Comparison of the properties of the values.
The obtained results can be generalized in several ways by using something other than the weighted Shapley value to allocate in the pseudogame (see for example [,]). Moreover, it seems interesting to us to analyze the relationship between the value introduced in this paper and the results for multigraph games obtained in [].
Author Contributions
Conceptualization, E.C.G., C.M. and D.M.; methodology, E.C.G., C.M. and D.M; software, E.C.G., C.M. and D.M.; validation, E.C.G., C.M. and D.M.; formal analysis, E.C.G., C.M. and D.M.; investigation, E.C.G., C.M. and D.M.; resources, E.C.G., C.M. and D.M.; data curation, E.C.G., C.M. and D.M.; writing—original draft preparation, E.C.G., C.M. and D.M.; writing—review and editing, E.C.G., C.M. and D.M.; visualization, E.C.G., C.M. and D.M.; supervision, E.C.G., C.M. and D.M.; project administration, E.C.G., C.M. and D.M.; funding acquisition, E.C.G., C.M. and D.M. All authors have read and agreed to the published version of the manuscript.
Funding
This research was partially supported by the “Plan Nacional de I+D+i” of the Spanish Government under the project PID2020-116884GB-I00.
Data Availability Statement
Not applicable.
Acknowledgments
The authors would like to thank the editor and two anonymous reviewers for their meticulous work that greatly helped us improve the paper. EC. Gavilán wants to thank University Complutense of Madrid and Bank of Santander for his pre-doctoral contract.
Conflicts of Interest
The authors declare no conflict of interest.
Appendix A
The matrix of coefficients of the system, M, is defined by blocks, and given by
where A is a -matrix given by
B is a -matrix given by
and D is the diagonal matrix:
where is the weight of one of the nodes on which the link is incident, for . Finnaly C is a matrix in which the kth row and is placed in the position corresponding to .
Then, coincides with if H is obtained adding to the cth row, the row divided by , the row divided by and so on. And thus,
in which , , …, are greater or equal than 1. It is easy to see that
Then which completes the proof.
References
- Shapley, L.S. A value for n-person games. Ann. Math. Stud. 1953, 28. [Google Scholar]
- Myerson, R.B. Graphs and cooperation in games. Math. Oper. Res. 1977, 2, 225–229. [Google Scholar] [CrossRef]
- Myerson, R.B. Conference structures and fair allocation rules. Int. J. Game Theory 1980, 9, 169–182. [Google Scholar] [CrossRef]
- Meessen, R. Communication Games. Master’s Thesis, University of Nijmegen, Nijmegen, The Netherlands, 1988. [Google Scholar]
- Borm, P.; Owen, G.; Tijs, S. On the position value for communication situations. SIAM J. Discret. Math. 1992, 5, 305–320. [Google Scholar] [CrossRef]
- González-Arangüena, E.; Manuel, C.; Owen, G.; del Pozo, M. The within groups and the between groups Myerson values. Eur. J. Oper. Res. 2017, 257, 586–600. [Google Scholar] [CrossRef]
- Li, D.L.; Shan, E. The position value and the structures of graphs. Appl. Math. Comput. 2019, 356, 190–197. [Google Scholar] [CrossRef]
- Li, D.L.; Shan, E.F. The Myerson value on local structures of coalitions. J. Oper. Res. Soc. China 2019, 7, 461–473. [Google Scholar] [CrossRef]
- Manuel, C.; Ortega, E.; del Pozo, M. Marginality and Myerson values. Eur. J. Oper. Res. 2020, 284, 301–312. [Google Scholar] [CrossRef]
- Shan, E.; Shi, J.; Lv, W. A note on the position value for hypergraph communication situations. In Complexity and Approximation; Springer: Berlin/Heidelberg, Germany, 2020; pp. 230–237. [Google Scholar]
- Wang, G.; Cai, L.; Shan, E. The efficient proportional Myerson values for hypergraph games. Math. Probl. Eng. 2021, 2021, 1468979. [Google Scholar] [CrossRef]
- Feltkamp, V.; Nouweland, A. Controlled Communication Networks. In Operations Research’92; Springer: Berlin/Heidelberg, Germany, 1993; pp. 413–415. [Google Scholar]
- López, S.; Owen, G.; Saboya, M. The impact of intermediaries on a negotiation: An approach from game theory. Cent. Eur. J. Oper. Res. 2022, 30, 837–859. [Google Scholar] [CrossRef]
- Shapley, L.S. Additive and Non-Additive Set Functions; Princeton University: Princeton, NJ, USA, 1953. [Google Scholar]
- Slikker, M. A characterization of the position value. Int. J. Game Theory 2005, 33, 505–514. [Google Scholar] [CrossRef]
- Harsanyi, J.C. A Bargaining Model for the Cooperative n-Person Game; Stanford University: Stanford, CA, USA, 1959. [Google Scholar]
- Navarro, F. Necessary players, Myerson fairness and the equal treatment of equals. Ann. Oper. Res. 2019, 280, 111–119. [Google Scholar] [CrossRef]
- Béal, S.; Rémila, E.; Solal, P. Fairness and fairness for neighbors: The difference between the Myerson value and component-wise egalitarian solutions. Econ. Lett. 2012, 117, 263–267. [Google Scholar] [CrossRef]
- Yokote, K.; Kongo, T.; Funaki, Y. The balanced contributions property for equal contributors. Games Econ. Behav. 2018, 108, 113–124. [Google Scholar] [CrossRef]
- Yokote, K.; Kongo, T. The balanced contributions property for symmetric players. Oper. Res. Lett. 2017, 45, 227–231. [Google Scholar] [CrossRef]
- Yokote, K.; Kongo, T.; Funaki, Y. Relationally equal treatment of equals and affine combinations of values for TU games. Soc. Choice Welf. 2019, 53, 197–212. [Google Scholar] [CrossRef]
- Casajus, A. Weakly balanced contributions and solutions for cooperative games. Oper. Res. Lett. 2017, 45, 616–619. [Google Scholar] [CrossRef]
- Ghintran, A.; González-Arangüena, E.; Manuel, C. A probabilistic position value. Ann. Oper. Res. 2012, 201, 183–196. [Google Scholar] [CrossRef]
- Shan, E.; Zhang, G.; Shan, X. The degree value for games with communication structure. Int. J. Game Theory 2018, 47, 857–871. [Google Scholar] [CrossRef]
- Manuel, C.; Ortega, E.; Pozo, M.D. Marginality and the position value. In TOP; Springer: Berlin/Heidelberg, Germany, 2022; pp. 1–16. [Google Scholar]
- Zhang, G.; Shan, E.; Qu, S. Characterizations of the Position Value for Hypergraph Communication Situations. In Proceedings of the East Asia Game Theory International Conference, Fuzhou, China, 7–9 March 2019; pp. 27–42. [Google Scholar]
- Manuel, C.M.; Martín, D. A monotonic weighted Shapley value. Group Decis. Negot. 2020, 29, 627–654. [Google Scholar] [CrossRef]
- Haeringer, G. A new weight scheme for the Shapley value. Math. Soc. Sci. 2006, 52, 88–98. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).