Existence Results for a Class of Fractional Differential Beam Type Equations
Abstract
:1. Introduction
2. Preliminary Results
- (i)
- and where
- (ii)
- if and only ifwhere is the ceiling function and
- (iii)
- If thenwhere is the ceiling function and
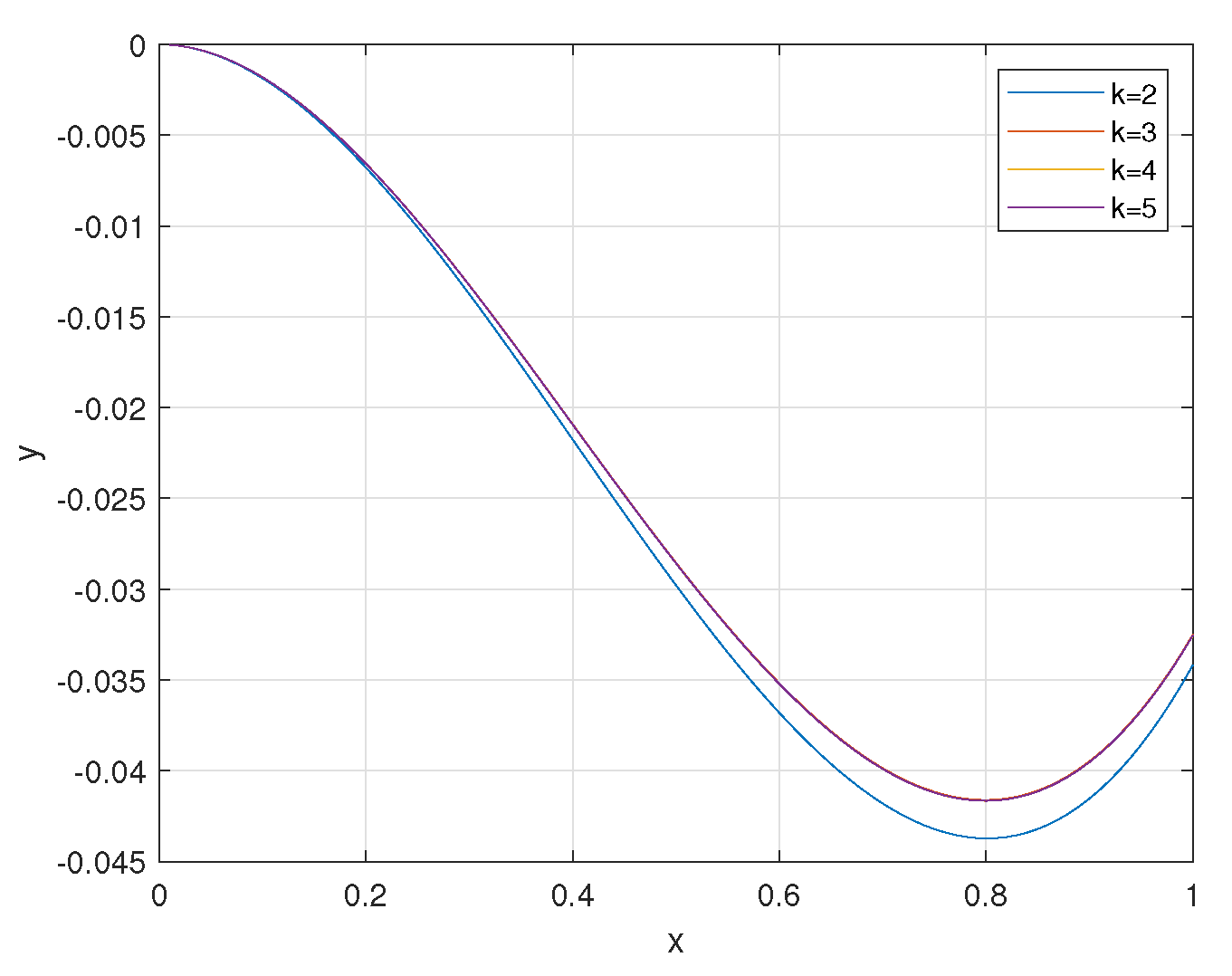
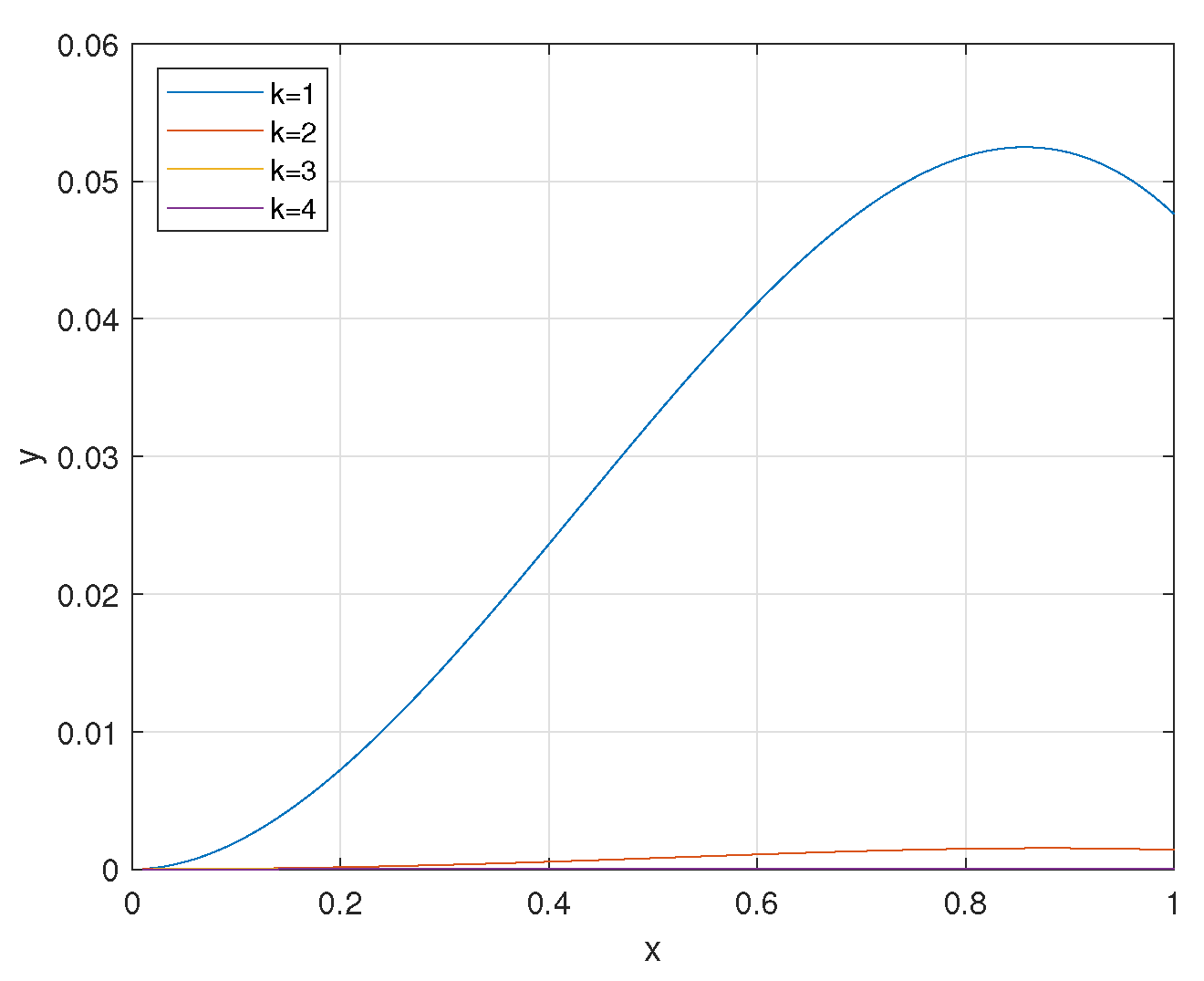
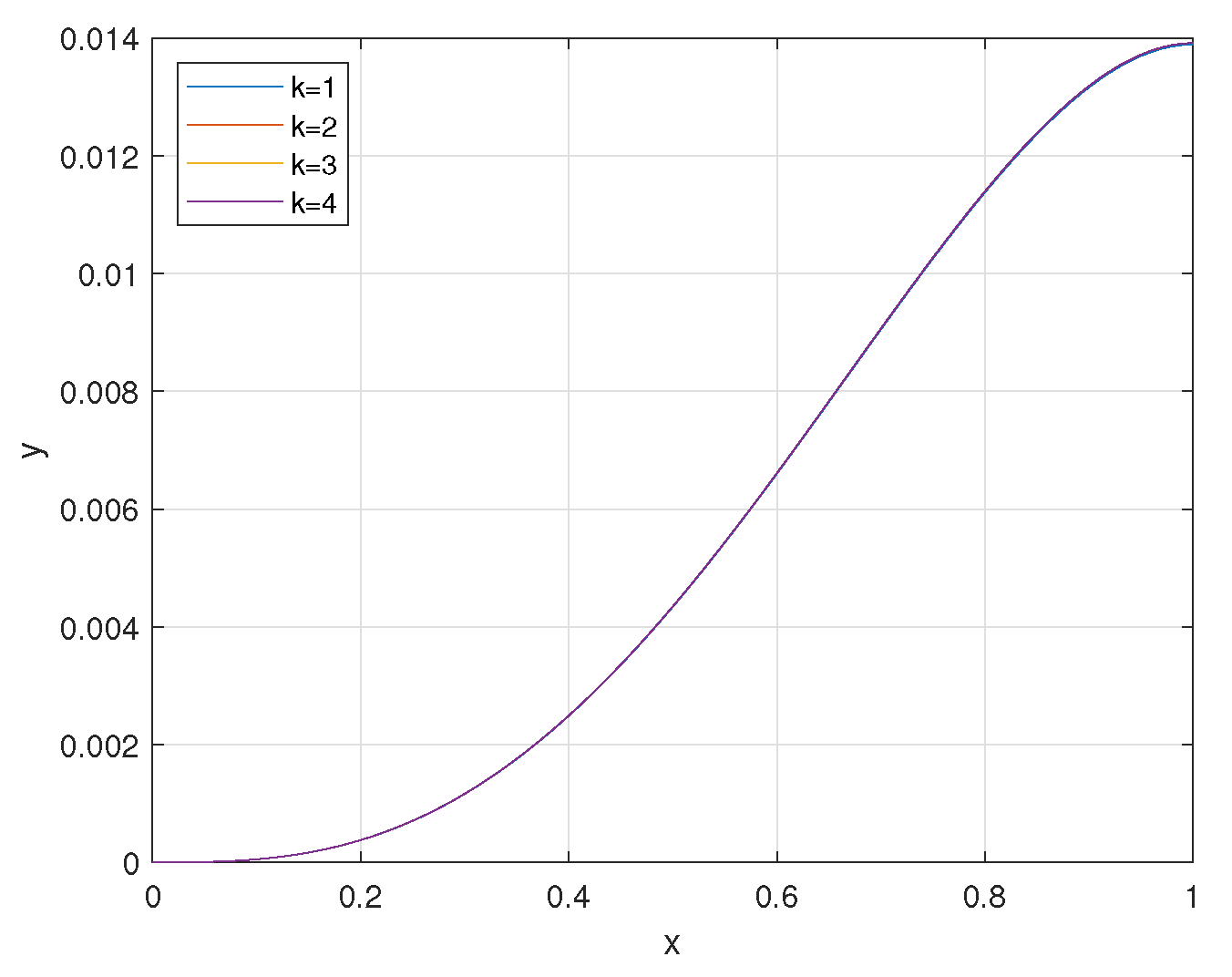
3. Main Results
- (i)
- for any
- (ii)
- for any
- (iii)
- (i)
- for any
- (ii)
- for any
- (iii)
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Reiss, E.L.; Callegari, A.J.; Ahluwalia, D.S. Ordinary Differential Equations with Applications; Holt, Rinehart and Winston: New York, NY, USA, 1976. [Google Scholar]
- Aftabizadeh, A.R. Existence and uniqueness theorems for fourth-order boundary value problems. J. Math. Anal. Appl. 1986, 116, 415–426. [Google Scholar] [CrossRef]
- Bai, Z. The upper and lower solution method for some fourth-order boundary value problems. Nonlinear Anal. 2007, 67, 1704–1709. [Google Scholar] [CrossRef]
- Dang, Q.A. Iterative method for solving the Neumann boundary value problem for biharmonic type equation. J. Comput. Appl. Math. 2006, 196, 634–643. [Google Scholar] [CrossRef]
- Dang, Q.A.; Dang, Q.L.; Ngo, T.K.Q. A novel efficient method for nonlinear boundary value problems. Numer. Algor. 2017, 76, 427–439. [Google Scholar]
- Gupta, C.P. Existence and uniqueness theorems for the bending of an elastic beam equation. Appl. Anal. 1988, 264, 289–304. [Google Scholar] [CrossRef]
- Gupta, C.P. Existence and uniqueness theorems for a fourth order boundary value problem of Sturm-Liouville type. Differ. Integral Equ. 1991, 4, 397–410. [Google Scholar] [CrossRef]
- Li, Y. A monotone iterative technique for solving the bending elastic beam equations. Appl. Math. Comput. 2010, 217, 2200–2208. [Google Scholar] [CrossRef]
- Li, Y. Existence of positive solutions for the cantilever beam equations with fully nonlinear terms. Nonlinear Anal. 2016, 27, 221–237. [Google Scholar] [CrossRef]
- Li, Y.; Liang, Q. Existence results for a fully fourth-order boundary value problem. J. Funct. Spaces Appl. 2013, 2013, 641617. [Google Scholar] [CrossRef]
- Wei, Y.; Song, Q.; Bai, Z. Existence and iterative method for some fourth order nonlinear boundary value problems. Appl. Math. Lett. 2019, 87, 101–107. [Google Scholar] [CrossRef]
- Minhós, F.; Gyulov, T.; Santos, A.I. Lower and upper solutions for a fully nonlinear beam equation. Nonlinear Anal. 2009, 71, 281–292. [Google Scholar] [CrossRef]
- Dang, Q.A.; Ngo, T.K.Q. Existence results and iterative method for solving the cantilever beam equation with fully nonlinear term. Nonlinear Anal. Real World Appl. 2017, 36, 56–68. [Google Scholar] [CrossRef]
- Hilfer, R. Applications of Fractional Calculus in Physics; World Scientific: Singapore, 2000. [Google Scholar]
- Kilbas, A.; Srivastava, H.; Trujillo, J. Theory and Applications of Fractional Differential Equations. In North-Holland Mathematics Studies; Elsevier: Amsterdam, The Netherlands, 2006; Volume 204. [Google Scholar]
- Miller, K.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations; Wiley: New York, NY, USA, 1993. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Academic Press: New York, NY, USA, 1999. [Google Scholar]
- Samko, S.; Kilbas, A.; Marichev, O. Fractional Integrals and Derivative. Theory and Applications; Gordon and Breach: Yverdon, Switzerland, 1993. [Google Scholar]
- Liang, S.; Zhang, J. Positive solutions for boundary value problems of nonlinear fractional differential equation. Nonlinear Anal. 2009, 71, 5545–5550. [Google Scholar] [CrossRef]
- Liu, Y. Existence and non-existence of positive solutions of BVPs for fractional order elastic beam equations with a non-Caratheodory nonlinearity. Appl. Math. Model. 2014, 38, 620–640. [Google Scholar] [CrossRef]
- Mâagli, H.; Dhifli, A. Existence and asymptotic behavior of positive solutions for semilinear fractional Navier boundary-value problems. Electron. J. Differ. Equ. 2017, 141, 1–13. [Google Scholar]
- Agarwal, R.P.; O’Regan, D.; Staněk, S. Positive solutions for Dirichlet problems of singular nonlinear fractional differential equations. J. Math. Anal. Appl. 2010, 371, 57–68. [Google Scholar] [CrossRef]
- Bachar, I.; Mâagli, H.; Eltayeb, H. Existence and Iterative Method for Some Riemann Fractional Nonlinear Boundary Value Problems. Mathematics 2019, 7, 961. [Google Scholar] [CrossRef]
- Bai, Z.; Lü, H. Positive solutions for boundary value problem of nonlinear fractional differential equation. J. Math. Anal. Appl. 2005, 311, 495–505. [Google Scholar] [CrossRef]
- Graef, J.R.; Kong, L.; Kong, Q.; Wang, M. Existence and uniqueness of solutions for a fractional boundary value problem with Dirichlet boundary condition. Electron. J. Qual. Theory Differ. Equ. 2013, 55, 1–11. [Google Scholar] [CrossRef]
- Jassim, H.K.; Hussein, M.A. A New Approach for Solving Nonlinear Fractional Ordinary Differential Equations. Mathematics 2023, 11, 1565. [Google Scholar] [CrossRef]
- Sina, E.; Azhar, H.; Atika, I.; Jehad, A.; Shahram, R.; George Maria, S.A. On a fractional cantilever beam model in the q-difference inclusion settings via special multi-valued operators. J. Inequal. Appl. 2021, 174, 20. [Google Scholar]
- Zhang, X.; Liu, L.; Wu, Y. Multiple positive solutions of a singular fractional differential equation with negatively perturbed term. Math. Comput. Model. 2012, 55, 1263–1274. [Google Scholar] [CrossRef]
- Agarwal, R.P.; Jleli, M.; Samet, B. Fixed Point Theory in Metric Spaces. Recent Advances and Applications; Springer: Singapore, 2018. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bachar, I.; Eltayeb, H.; Mesloub, S. Existence Results for a Class of Fractional Differential Beam Type Equations. Axioms 2023, 12, 939. https://doi.org/10.3390/axioms12100939
Bachar I, Eltayeb H, Mesloub S. Existence Results for a Class of Fractional Differential Beam Type Equations. Axioms. 2023; 12(10):939. https://doi.org/10.3390/axioms12100939
Chicago/Turabian StyleBachar, Imed, Hassan Eltayeb, and Said Mesloub. 2023. "Existence Results for a Class of Fractional Differential Beam Type Equations" Axioms 12, no. 10: 939. https://doi.org/10.3390/axioms12100939
APA StyleBachar, I., Eltayeb, H., & Mesloub, S. (2023). Existence Results for a Class of Fractional Differential Beam Type Equations. Axioms, 12(10), 939. https://doi.org/10.3390/axioms12100939






