How Micelles Influence the Optical Limiting Properties of Zinc Porphyrins and J-Aggregates for Picosecond Pulse Trains
Abstract
:1. Introduction
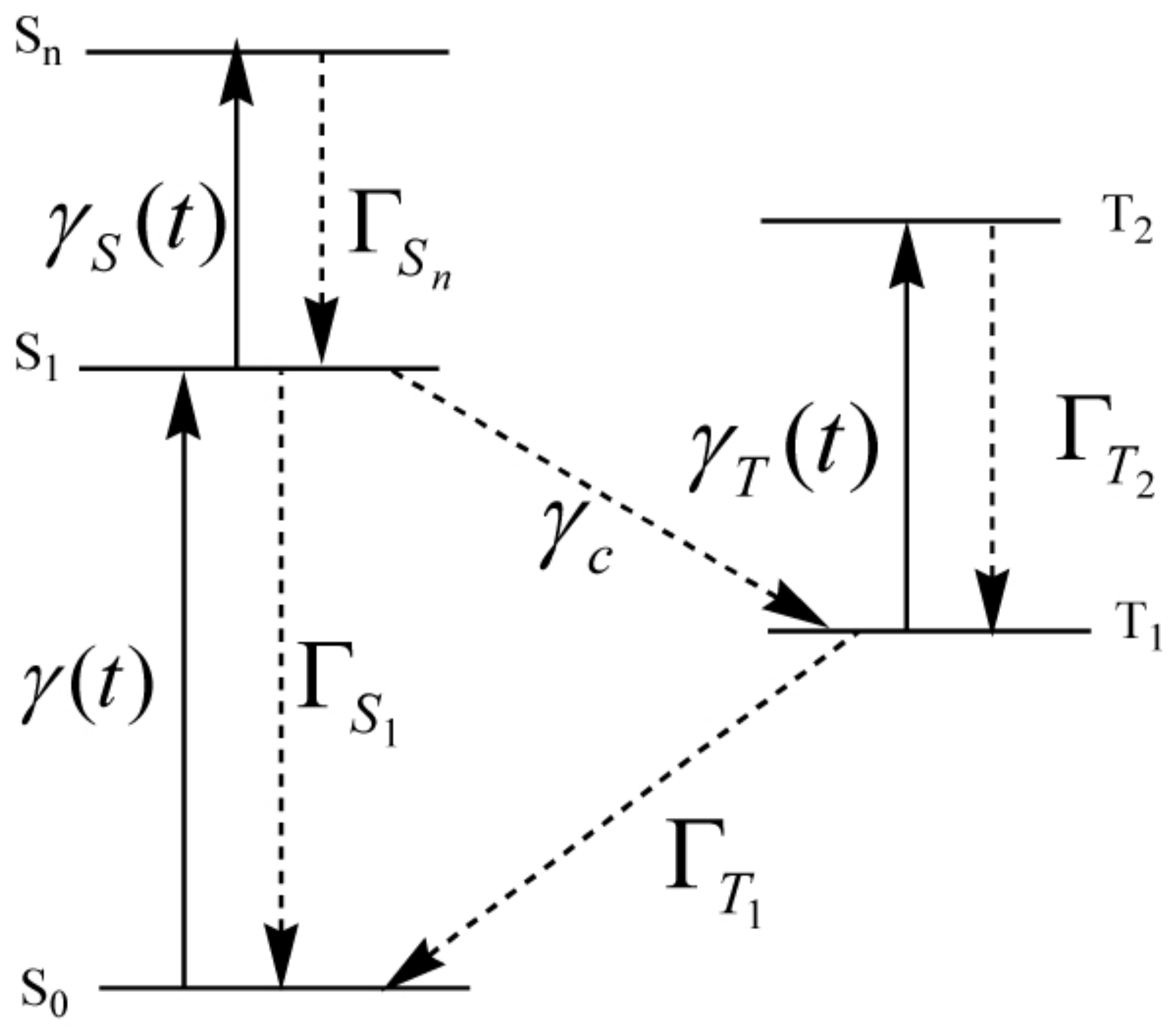
2. Method
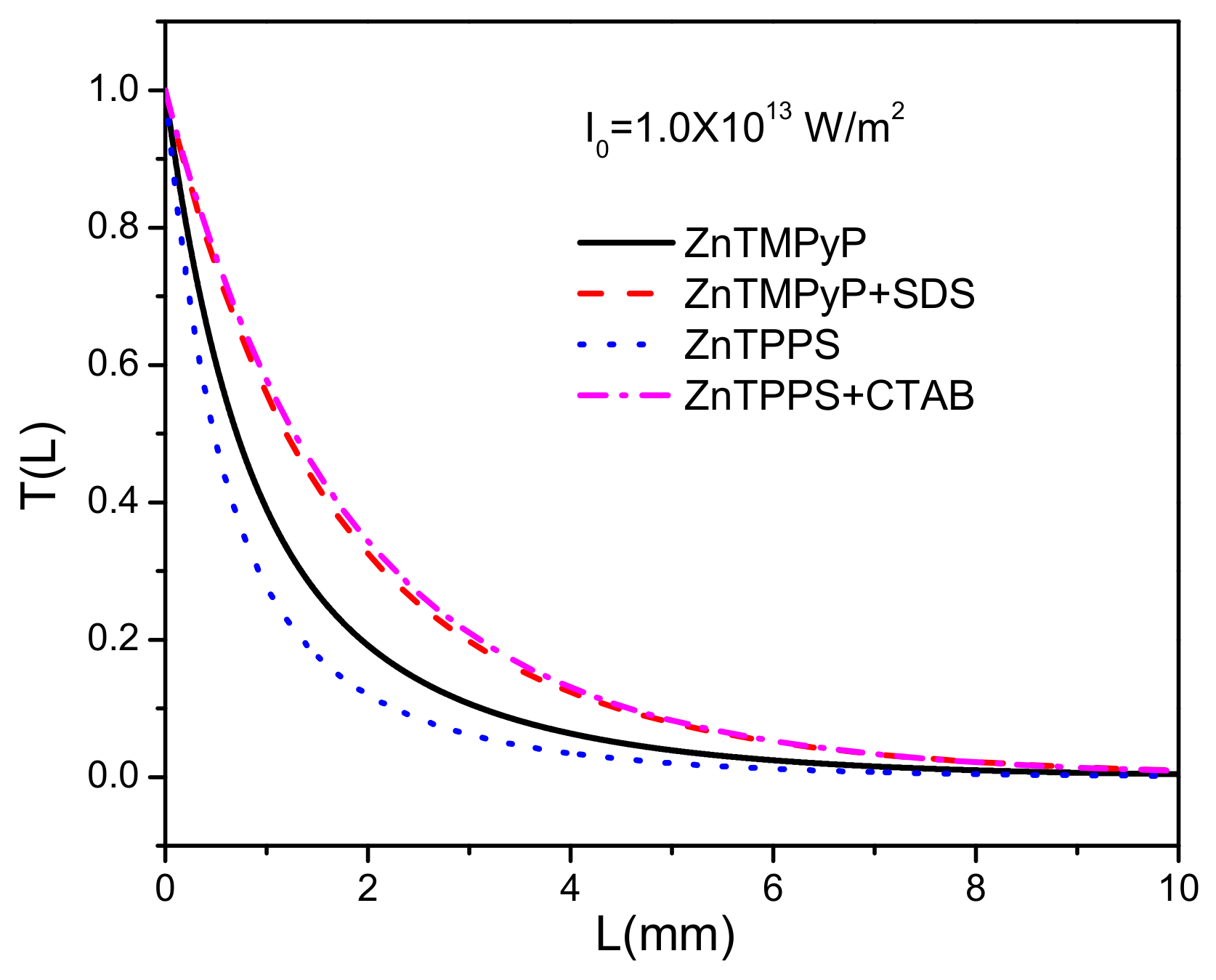
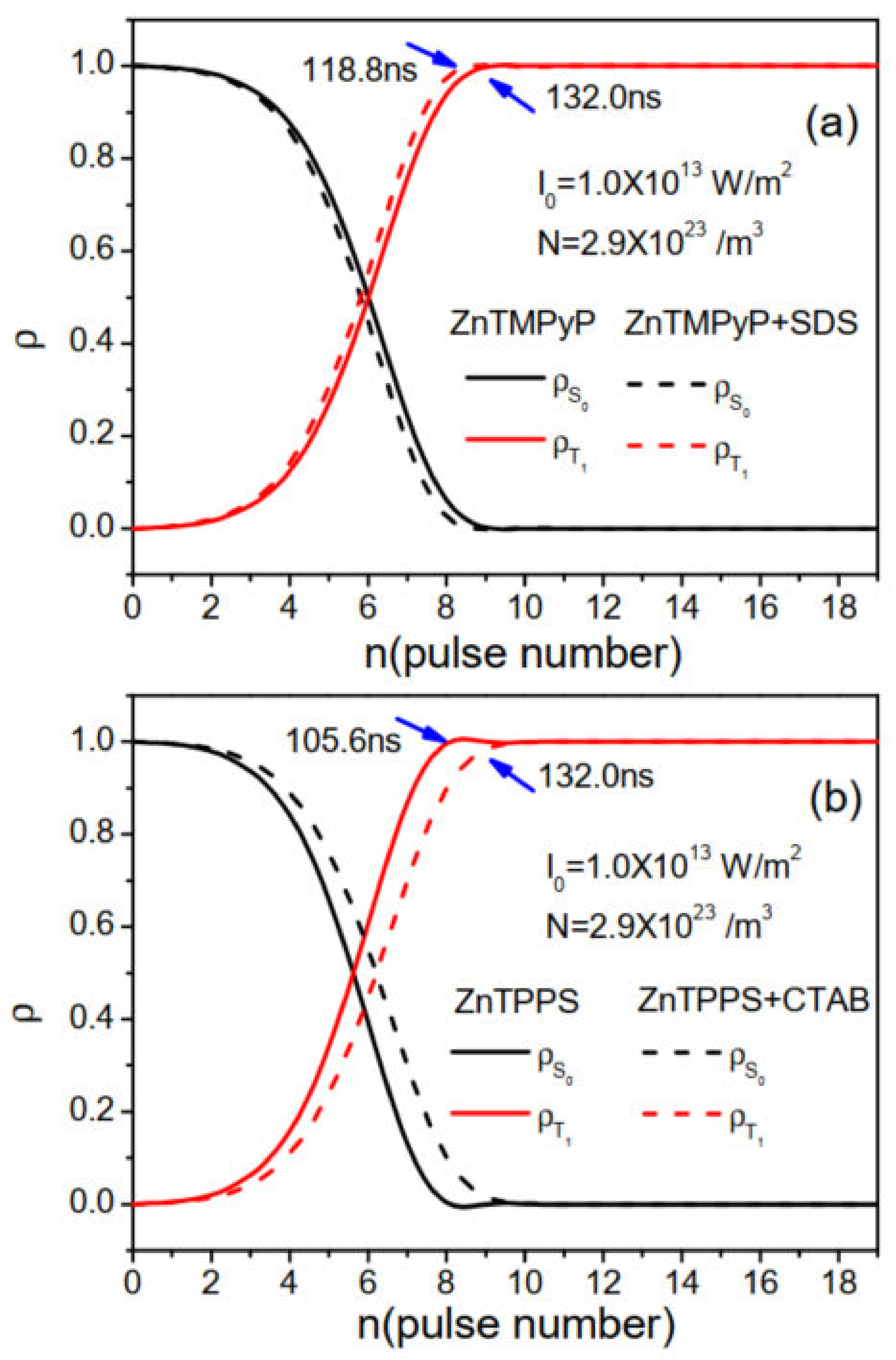
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Singh, C.P.; Bindra, K.S.; Jain, B.; Oak, S.M. All-optical switching characteristics of metalloporphyrins. Opt. Commun. 2005, 245, 407. [Google Scholar] [CrossRef]
- Liu, M.O.; Tai, C.H.; Hu, A.T.; Wei, T.H. Reverse saturable absorption of lanthanide bisphthalocyanines and their application for optical switches. J. Organomet. Chem. 2004, 689, 2138. [Google Scholar] [CrossRef]
- Dini, D.; Calvete, M.J.F.; Hanack, M. Nonlinear optical materials for the smart filtering of optical radiation. Chem. Rev. 2016, 116, 13043. [Google Scholar] [CrossRef] [PubMed]
- Calvete, M.; Yang, G.Y.; Hanack, M. Porphyrins and phthalocyanines as materials for optical limiting. Synth. Met. 2004, 141, 231. [Google Scholar] [CrossRef]
- Thanopulos, I.; Paspalakis, E.; Yannopapas, V. Optical switching of electric charge transfer pathways in porphyrin: A light-controlled nanoscale current router. Nanotechnology 2008, 19, 445202. [Google Scholar] [CrossRef]
- Barbosa, N.M.; Oliveira, S.L.; Misoguti, L.; Mendonca, C.R.; Goncalves, P.J.; Borissevitch, I.E.; Dinelli, L.R.; Romualdo, L.L.; Batista, A.A.; Zilio, S.C. Singlet excited state absorption of porphyrin molecules for pico- and femtosecond optical limiting application. J. Appl. Phys. 2006, 99, 123103. [Google Scholar] [CrossRef]
- Xu, Y.F.; Liu, Z.B.; Zhang, X.L.; Wang, Y.; Tian, J.G.; Huang, Y.; Ma, Y.F.; Zhang, X.Y.; Chen, Y.S. A Graphene Hybrid Material Covalently Functionalized with Porphyrin: Synthesis and Optical Limiting Property. Adv. Mater. 2009, 21, 1275. [Google Scholar] [CrossRef]
- Senge, M.O.; Fazekas, M.; Notaras, E.G.A.; Blau, W.J.; Zawadzka, M.; Locos, O.B.; Mhuircheartaigh, E.M.N. Nonlinear Optical Properties of Porphyrins. Adv. Mater. 2007, 19, 2737. [Google Scholar] [CrossRef]
- de la Torre, G.; Vaquez, P.; Agullo-Lopez, F.; Torres, T. Role of Structural Factors in the Nonlinear Optical Properties of Phthalocyanines and Related Compounds. Chem. Rev. 2004, 104, 3723. [Google Scholar] [CrossRef]
- Horiuchi, H.; Kameya, T.; Hosaka, M.; Yoshimura, K.; Kyushin, S.; Matsumoto, H.; Okutsu, T.; Takeuchi, T.; Hiratsuka, H. Silylation enhancement of photodynamic activity of tetraphenylporphyrin derivative. J. Photochem. Photobiol. 2001, A 221, 98. [Google Scholar] [CrossRef]
- Kubat, P.; Mosinger, J. Photophysical properties of metal complexes of meso-tetrakis(4-sulphonatophenyl)porphyrin. J. Photochem. Photobiol. A Chem. 1996, 96, 93. [Google Scholar] [CrossRef]
- Monteiro, C.J.P.; Serpa, C.; Pereira, M.M.; Azenha, M.E.; Burrows, H.D.; Arnaut, L.G.; Tapia, M.J.; Sarakha, M.; Navaratnam, S. A comparative study of water soluble 5,10,15,20-tetrakis(2,6-dichloro-3-sulfophenyl)porphyrin and its metal complexes as efficient sensitizers for photodegradation of phenols. Photochem. Photobiol. Sci. 2005, 4, 617. [Google Scholar] [CrossRef] [Green Version]
- Dudkowiak, A.; Teslak, E.; Habdas, J. Photophysical studies of tetratolylporphyrin photosensitizers for potential medical applications. J. Mol. Struct. 2006, 792–793, 93. [Google Scholar] [CrossRef]
- Bonnett, R. Photosensitizers of the porphyrin and phthalocyanine series for photodynamic therapy. Chem. Soc. Rev. 1995, 24, 19. [Google Scholar] [CrossRef]
- Ochsner, M. Photophysical and photobiological processes in the photodynamic therapy of tumours. J. Photochem. Photobiol. 1997, 39, 1. [Google Scholar] [CrossRef]
- Jasuja, R.; Jameson, D.M.; Nishijo, C.K.; Larsen, R.W. Singlet Excited State Dynamics of Tetrakis(4-N-methylpyridyl)porphine Associated with DNA Nucleotides. J. Phys. Chem. B 1997, 101, 1444. [Google Scholar] [CrossRef]
- Uno, T.; Hamasaki, K.; Tanigawa, M.; Shimabayashi, S. Binding of meso-Tetrakis(N-methylpyridinium-4-yl)porphyrin to Double Helical RNA and DNA·RNA Hybrids. Inorg. Chem. 1997, 36, 1676. [Google Scholar] [CrossRef]
- Josefsen, L.B.; Boyle, R.W. Unique diagnostic and therapeutic roles of porphyrins and phthalocyanines in photodynamic therapy, imaging and theranostics. Theranostics 2012, 9, 916. [Google Scholar] [CrossRef] [Green Version]
- Taratula, O.; Schumann, C.; Naleway, M.A.; Pang, A.J.; Chon, K.J.; Taratula, O. A multifunctional theranostic platform based on phthalocyanine-loaded dendrimer for image-guided drug delivery and photodynamic therapy. Mol. Pharm. 2013, 10, 3946. [Google Scholar] [CrossRef]
- Pucelik, B.; Paczynski, R.; Dubin, G.; Pereira, M.M.; Arnaut, L.G.; Dabrowski, J.M. Properties of halogenated and sulfonated porphyrins relevant for the selection of photosensitizers in anticancer and antimicrobial therapies. PLoS ONE 2017, 12, e0185984. [Google Scholar] [CrossRef]
- Goncalves, P.J.; De Boni, L.; Barbosa, N.M.; Rodrigues, J.J., Jr.; Zilio, S.C.; Borissevitch, I.E. Effect of protonation on the photophysical properties of meso-tetra(sulfonatophenyl) porphyrin. Chem. Phys. Lett. 2005, 407, 236. [Google Scholar] [CrossRef]
- Goncalves, P.J.; Borissevitch, I.E.; Zilio, S.C. Effect of protonation on the singlet–singlet excited-state absorption of meso-tetrakis(p-sulphonatophenyl) porphyrin. Chem. Phys. Lett. 2009, 469, 270. [Google Scholar] [CrossRef]
- Sampaio, R.N.; Gomes, W.R.; Araujo, D.M.S.; Machado, A.E.H.; Silva, R.A.; Marletta, A.; Borissevitch, I.E.; Ito, A.S.; Dinelli, L.R.; Batista, A.A.; et al. Investigation of Ground- and Excited-State Photophysical Properties of 5,10,15,20-Tetra(4-pyridyl)-21H,23H-porphyrin with Ruthenium Outlying Complexes. J. Phys. Chem. A 2012, 116, 18. [Google Scholar] [CrossRef] [PubMed]
- Goncalves, P.J.; De Boni, L.; Borissevitch, I.E.; Zilio, S.C. Excited State Dynamics of meso-Tetra(sulphonatophenyl) Metalloporphyrins. J. Phys. Chem. A 2008, 112, 6522. [Google Scholar] [CrossRef] [PubMed]
- Pavani, C.; Uchoa, A.F.; Oliveira, C.S.; Iamamoto, Y.; Baptista, M.S. Effect of zinc insertion and hydrophobicity on the membrane interactions and PDT activity of porphyrin photosensitizers. Photochem. Photobiol. Sci. 2009, 8, 233. [Google Scholar] [CrossRef]
- Goncalvesa, P.J.; Franzen, P.L.; Correa, D.S.; Almeida, L.M.; Takara, M.; Ito, A.S.; Zilio, S.C.; Borissevitch, I.E. Effects of environment on the photophysical characteristics of mesotetrakis methylpyridiniumyl porphyrin (TMPyP). Spectrochim. Acta Part A 2011, 79, 1532. [Google Scholar] [CrossRef]
- Reddi, E.; Ceccon, M.; Valduga, G.; Jori, G.; Bommer, J.C.; Elisei, F.; Latterini, L.; Mazzucato, U. Photophysical Properties and Antibacterial Activity of Meso-substituted Cationic Porphyrins. Photochem. Photobiol. 2002, 75, 462. [Google Scholar] [CrossRef]
- Snyder, J.W.; Lambert, J.D.C.; Ogilby, P.R. 5,10,15,20-Tetrakis(N-Methyl-4-Pyridyl)-21 H,23H-Porphine (TMPyP) as a Sensitizer for Singlet Oxygen Imaging in Cells: Characterizing the Irradiation-dependent Behavior of TMPyP in a Single Cell. Photochem. Photobiol. 2006, 82, 177. [Google Scholar] [CrossRef]
- Jimenez-Banzo, A.; Sagrista, M.L.; Mora, M.; Nonell, S. Kinetics of singlet oxygen photosensitization in human skin fibroblasts. Free Radic. Biol. Med. 2008, 44, 1926. [Google Scholar] [CrossRef]
- Goncalves, P.J.; Bezerra, F.C.; Almeida, L.M.; Alonso, L.; Souza, G.R.L.; Alonso, A.; Zilio, S.C.; Borissevitch, I.E. Effects of bovine serum albumin (BSA) on the excited-state properties of meso-tetrakis(sulfonatophenyl) porphyrin (TPPS4). Eur. Biophys. J. 2019, 48, 721. [Google Scholar] [CrossRef]
- Borissevitch, I.E.; Tominaga, T.T.; Schmitt, C.C. Photophysical studies on the interaction of two water soluble porphyrins with bowine serum albumin. Effects upon the porphyrin triplet state characteristics. J. Photochem. Phototobiol. A 1998, 114, 201. [Google Scholar] [CrossRef]
- Andrade, S.M.; Costa, S.M.B. Spectroscopic studies on the interaction of a water soluble porphyrin and two drug carrier proteins. Biophys. J. 2002, 82, 1607. [Google Scholar] [CrossRef] [Green Version]
- Dubbelman, T.M.A.R. Porphyrin-protein interaction. In Photosensitisation; Moreno, G., Pottier, R.H., Truscott, T.G., Eds.; NATO ASI Series (Series H: Cell Biology); Springer: Berlin, Germany, 1988; Volume 15. [Google Scholar]
- Morgan, W.T.; Smith, A.; Koskelo, P. The interaction of human serum albumin and hemopexin with porphyrins. BBA Protein Struct. 1980, 624, 271. [Google Scholar] [CrossRef]
- Kadish, K.M.; Maiya, G.B.; Araullo, C.; Guillard, R. Micellar effects on the aggregation of tetraanionic porphyrins. Spectroscopic characterization of free-base meso-tetrakis(4-sulfonatophenyl)porphyrin, (TPPS)H2, and (TPPS)M (M = zinc(II), copper(II), and vanadyl) in aqueous micellar media. Inorg. Chem. 1989, 28, 2725. [Google Scholar] [CrossRef]
- Kadish, K.M.; Maiya, B.G.; Araullo-McAdams, C. Spectroscopic characterization of meso-tetrakis(1-methylpyridinium-4-yl)porphyrins, [(TMpyP)H2]4+ and [(TMpyP)M]4+, in aqueous micellar media, where M = VO2+, Cu(II), and Zn(II). J. Phys. Chem. 1991, 95, 427. [Google Scholar] [CrossRef]
- Gandini, S.C.; Yushmanov, V.E.; Tabak, M. Interaction of Fe(III)- and Zn(II)-tetra(4-sulfonatophenyl) porphyrins with ionic and nonionic surfactants: Aggregation and binding. J. Inorg. Biochem. 2001, 85, 263. [Google Scholar] [CrossRef]
- Wang, X.; Zhao, L.; Ma, R.; An, Y.; Shi, Y. Stability enhancement of ZnTPPS in acidic aqueous solutions by polymeric micelles. Chem. Commun. 2010, 46, 6560. [Google Scholar] [CrossRef]
- Szmytkowski, J.; Brunet, S.M.K.; Tripathy, U.; O’Brien, J.A.; Paige, M.P.; Steer, R.P. Photophysics and halide quenching of Soret-excited ZnTPPS4− in aqueous media. Chem. Phys. Lett. 2011, 501, 278. [Google Scholar] [CrossRef]
- Lebold, T.P.; Yeow, K.L.; Steer, R.P. Fluorescence quenching of the S1 and S2 states of zinc meso-tetrakis(4-sulfonatophenyl)porphyrin by halide ions. Photochem. Photobiol. Sci. 2004, 3, 160. [Google Scholar] [CrossRef]
- Zhao, B.; Li, Y.; Zhao, Y.; Ma, Y.; Li, F.; Han, H.; Wang, N.; Wang, X. A sensing platform based on zinc-porphyrin derinative in hexadecyl trimethyl ammonium bromide (CTAB) microemulsion for highly sensitive detection of theophylline. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2022, 281, 121592. [Google Scholar] [CrossRef]
- Mamardashvili, G.M.; Kaigorodova, E.Y.; Lebedev, I.S.; Khodov, I.A.; Mamardashvili, N.Z. Supramolecular assembly of hydrophilic Co(III)-porphyrin with bidentate ligands in aqueous buffer medi. Inorganica Chim. Acta 2022, 538, 120972. [Google Scholar] [CrossRef]
- Wang, X.; Zhang, S.; Zhao, B. Determination of ultra trace amounts of metronidazole by 3-phenyl-N-[4-(10,15,20-triphenyl-porphyrin-5-yl)-phenyl]-acrylamide as the fluorescence spectral probe in CTAB microemulsion. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2020, 227, 117699. [Google Scholar] [CrossRef]
- Mamardashvili, G.M.; Kaigorodova, E.Y.; Khodov, I.A.; Sheblykin, I.; Nugzar, Z.; Mamardashvili, N.Z.; Koifman, O.I. Micelles encapsulated Co(III)-tetra(4-sulfophenyl)porphyrin in aqueous CTAB solutions: Micelle formation, imidazole binding and redox Co(III)/Co(II) processes. J. Mol. Liq. 2019, 293, 111471. [Google Scholar] [CrossRef]
- Guo, L. Side-chain-controlled H- and J-aggregation of amphiphilic porphyrins in CTAB micelles. J. Colloid Interface Sci. 2008, 322, 281–286. [Google Scholar] [CrossRef] [PubMed]
- Goncalves, P.J.; Aggarwal, L.P.F.; Marquezin, C.A.; Ito, A.S.; Boni, L.D.; Neto, N.M.B.; Rodrigues, J.J., Jr.; Zilio, S.C.; Borissevitch, I.E. Effects of interaction with CTAB micelles on photophysical characteristics of meso-tetrakis(sulfonatophenyl) porphyrin. J. Photochem. Photobiol. A Chem. 2006, 181, 378–384. [Google Scholar] [CrossRef]
- Amao, Y.; Tomonou, Y.; Okura, I. Highly efficient photochemical hydrogen production systemusing zinc porphyrin and hydrogenase in CTAB micellar system. Sol. Energy Mater. Sol. Cells 2003, 79, 103–111. [Google Scholar] [CrossRef]
- Sun, Y.P.; Gavrilyuk, S.; Liu, J.C.; Wang, C.K.; Ågren, H.; Gel’mukhanov, F. Optical limiting and pulse reshaping of picosecond pulse trains by fullerene C60. J. Electron. Spectrosc. Relat. Phenom. 2009, 174, 125. [Google Scholar] [CrossRef]
- Gel’mukhanov, F.; Ågren, H. Resonant X-ray Raman scattering. Phys. Rep. 1999, 312, 87. [Google Scholar] [CrossRef]
- Goncalves, P.J.; Correa, D.S.; Franzen, P.L.; De Boni, L.; Almeida, L.M.; Mendonca, C.R.; Borissevitch, I.E.; Zilio, S.C. Effect of interaction with micelles on the excited-state optical properties of zinc porphyrins and J-aggregates formation. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2013, 112, 309. [Google Scholar] [CrossRef]
- Gavrilyuk, S.; Liu, J.C.; Kamada, K.; Ågren, H.; Gel’mukhanov, F. Optical limiting for microsecond pulses. J. Chem. Phys. 2009, 130, 054114. [Google Scholar] [CrossRef]

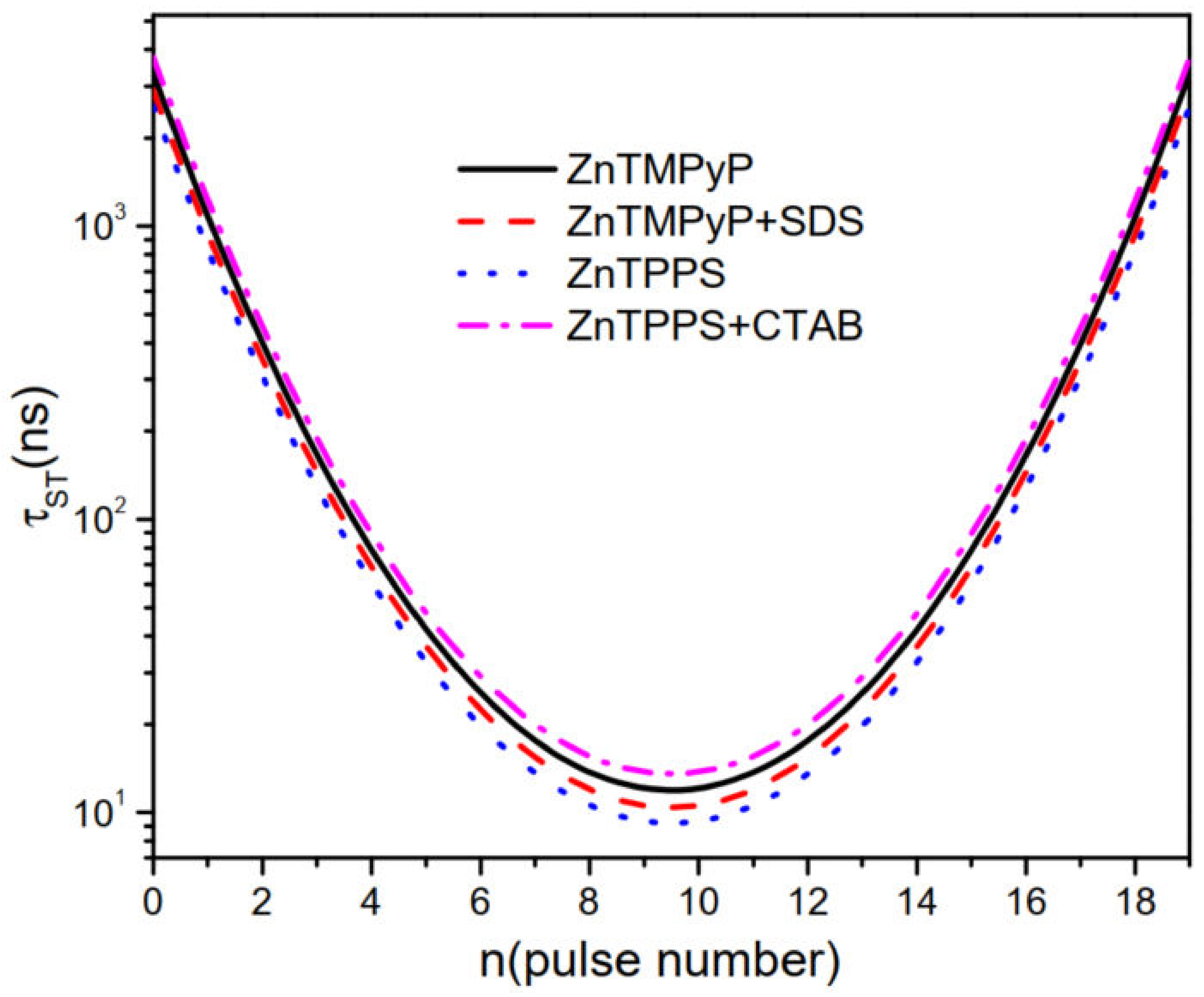
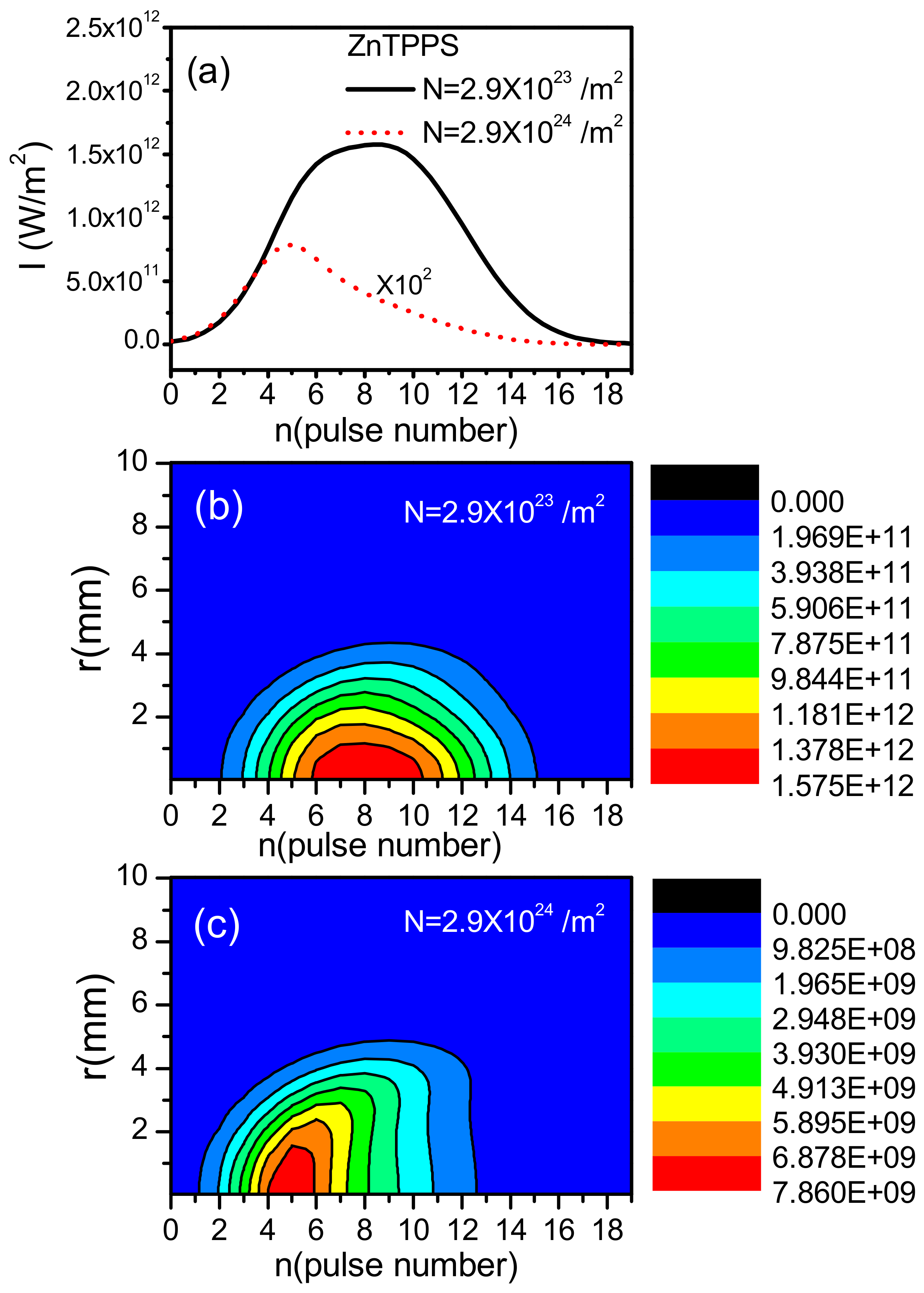
| Compounds | |||||
|---|---|---|---|---|---|
| ZnTMPyP | |||||
| ZnTMPyP + SDS | |||||
| ZnTPPS | |||||
| ZnTPPS + CTAB |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Miao, Q.; Sun, E.; Xu, Y. How Micelles Influence the Optical Limiting Properties of Zinc Porphyrins and J-Aggregates for Picosecond Pulse Trains. Axioms 2023, 12, 23. https://doi.org/10.3390/axioms12010023
Miao Q, Sun E, Xu Y. How Micelles Influence the Optical Limiting Properties of Zinc Porphyrins and J-Aggregates for Picosecond Pulse Trains. Axioms. 2023; 12(1):23. https://doi.org/10.3390/axioms12010023
Chicago/Turabian StyleMiao, Quan, Erping Sun, and Yan Xu. 2023. "How Micelles Influence the Optical Limiting Properties of Zinc Porphyrins and J-Aggregates for Picosecond Pulse Trains" Axioms 12, no. 1: 23. https://doi.org/10.3390/axioms12010023
APA StyleMiao, Q., Sun, E., & Xu, Y. (2023). How Micelles Influence the Optical Limiting Properties of Zinc Porphyrins and J-Aggregates for Picosecond Pulse Trains. Axioms, 12(1), 23. https://doi.org/10.3390/axioms12010023







