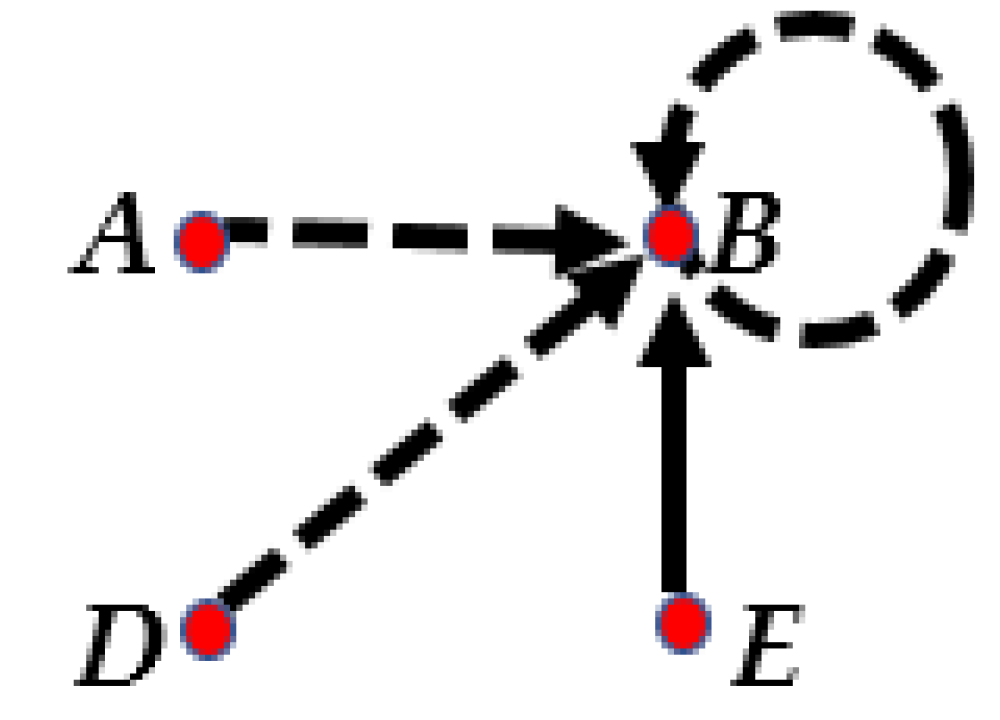
Puzzles are known from ancient ages for entertaining, recreation and also for philosophical and reasoning points of view. Nowadays, puzzles could be related to different disciplines, since they motivate logical thinking and knowledge reasoning. Knight and knave puzzles are some of the popular puzzles that were investigated by Raymond Smullyan in depth [
1,
2,
3,
4,
5,
6]. Several papers have discussed the solvability of Smullyan’s puzzles [
7,
8,
9,
10], their applications [
11,
12,
13] and the way of finding automatic solutions using different algorithms [
14,
15,
16,
17,
18,
19]. Basically, knights are truthtellers who (can) say only true statements and knaves are liars, who (can) tell only false statements. In some puzzles only short, so-called atomic statements are allowed, and they should be about the type of a person in the puzzle. These types are named as strong truthtellers and strong liars, respectively, to highlight that each (short) statement a person told is reflecting his or her type [
20,
21,
22,
23,
24,
25]. In the simplest class of puzzles, in the SS puzzles, only these two types of people may appear (the acronym comes from the word strong for both the truthtellers and liars). In general, a puzzle is good if it has a unique solution. On the other hand, it is known [
20,
23] that there is no good SS puzzle because of the symmetric roles of the two abovementioned types of people. Thus, we may need other types of people to appear in the puzzles, and, therefore, later on, more kinds of people were introduced in puzzles (not only knights and knaves). In [
26,
27,
28,
29], normal is a type of person who can say both true and false statements. KNK puzzles [
29], i.e., puzzles with knights, knaves and normal persons, have always a trivial solution such that everybody is normal. Weak truthtellers and weak liars were also introduced and studied by denoting those persons by these terms who must have at least one true or at least one false (atomic) statement, respectively, if she or he says anything in the puzzle. This means that, e.g., if a weak truthteller says anything in the puzzle, then at least one of her or his atomic statements must be true. Thus, in SW puzzles [
20,
23], there are strong truthtellers and weak liars, i.e., it can also be understood as the conjunctive interpretation of the short statements: all must be true for a truthteller and at least one must be false for a liar. The dual puzzles, the WS type with weak truthtellers and strong liars, were also studied [
30] and these puzzles are connected to the disjunctive interpretation of the statements. In this paper, however, we work with the simplest type of truthtellers and liars, the strong ones. Since there are no good SS puzzles, we may need to have types which can be used in the solutions as fixed points. Actually, since the usage of normal type leads to puzzles with a higher number of solutions, we are interested in expanding the possible types to the other direction. As we have mentioned the normal type may be used for people who can say both true and false statements. To complement this, there are two known ways to introduce a type for people who cannot tell true and cannot tell false statements. The first way we recall here is our recently introduced direction, which allows these people to say self-contradictory statements based on the ancient liar paradox. These people may say paradoxical statements that neither knights nor knaves can say in the puzzle. This type of statement defines a new type of puzzle, where the strong crazy type was initiated [
21]. Strong crazy people are persons who can say only paradoxical statements. Various types of paradoxical statements and issues with self-reference in puzzles were also presented in [
31,
32,
33,
34,
35,
36,
37,
38,
39]. Rather than strong types of crazy persons, we may also have the weak crazy type, similar to the already mentioned weak truthtellers and liars [
22]. Generally, in the weak types, the person with the weak type should say at least one statement that reflects his or her type (if the person speaks anything). In contrast, in the strong types of persons, all statements said by those persons should reflect their types. The other, earlier used option for those who could not tell truth, nor could lie, is to introduce the type of Mutes [
26]. In puzzles with Mutes, those who did not say anything in the puzzle, could be Mutes. However, it could also happen that some other people, e.g., truthtellers or liars remained silent. To resolve this ambiguity and provide some fix point for the solution, we also investigate puzzles, where only Mutes can remain silent, i.e., in the solution everybody is a Mute if and only if he or she is silent. Thus, in this paper, we compare five different types of puzzles regarding their solvability: SS, SSS, SSW, SSM
W and SSM
S puzzles. The first two letters, SS, representing that the truthtellers and liars are of the strong types in all of these puzzles. The third letter S or W stands for the possible type of crazy person who may appear. Letter M in the third position means that the introduced third type of person is a Mute. Since Mutes do not say any statement, there is no way to differentiate strong and weak Mutes. However, a somewhat similar thing gives us a way to refine the situation in SSM puzzles: we differentiate two types of puzzles with Mutes, thus expanding the horizons of these types of puzzles. Letter M with index W shows that in the puzzles the types are weak in the sense that anybody can be silent (i.e., mute), not only Mutes (the latter are defined to be mute and have to be mute), but also some truthtellers or liars can be accidentally mute in the puzzle if they do not say anything. (We refer to the type of Mute by writing it with capital letter ‘M’). On the other hand, by index S, we show that the types are also strong in the sense that each person can be identified by his or her statement, even if he or she does not say anything, i.e., by the lack of his or her statements. In this comparison, we are trying to figure out the possibility to have a good puzzle in a specific puzzle type, which puzzle type has the greatest number of solvable puzzles, what the maximum and the minimum number of statements are that can be said in a good puzzle and other brainstorming questions regarding different types of puzzles. Some statistics about SS puzzles were presented in [
25], while some statistical comparisons between SS and SSS puzzles and between SS and SSW puzzles were presented in [
21] and [
22], respectively. In our work here, we do a more comprehensive comparison of five different types of puzzles in various aspects. One of our aims is to show how the symmetry of the truthtellers and liars can be broken by introducing a third type into the scope, and thus to have good puzzles. Another aim is to make more solvable and, if possible, good puzzles by shifting the interpretation, i.e., the possible types of the people appearing in it. We statistically compare the puzzles to see which way can be considered as the most efficient from these points of view. Actually, we introduce here two types of SSM puzzles (where the people can be strong truthtellers, strong liars and Mutes) by differentiating the muteness based on two different points of view as we have already mentioned, this way also complementing the earlier studies. Graph representations and diagrams are used to facilitate the interpretation of logical puzzles somewhat similarly as in [
19,
20,
21,
22,
23,
29]. Our puzzles can be linked easily to other scenarios, the persons are not necessarily always persons. Persons of our puzzles can simulate various peripherals or sensors in various types of networks which helps to define their identity, i.e., whether that device is a noisy device (crazy person), a defected or passive hacker device (Mute person) or an active hacking device (liar person). A similar scenario was introduced in [
40] about satellites and messages between them about their status. Thus, some parts of our study may have applications beyond recreational mathematics and logic.