Fractional Dynamical Behavior of an Elastic Magneto Piezo Oscillator Including Non-Ideal Motor Excitation
Abstract
1. Introduction
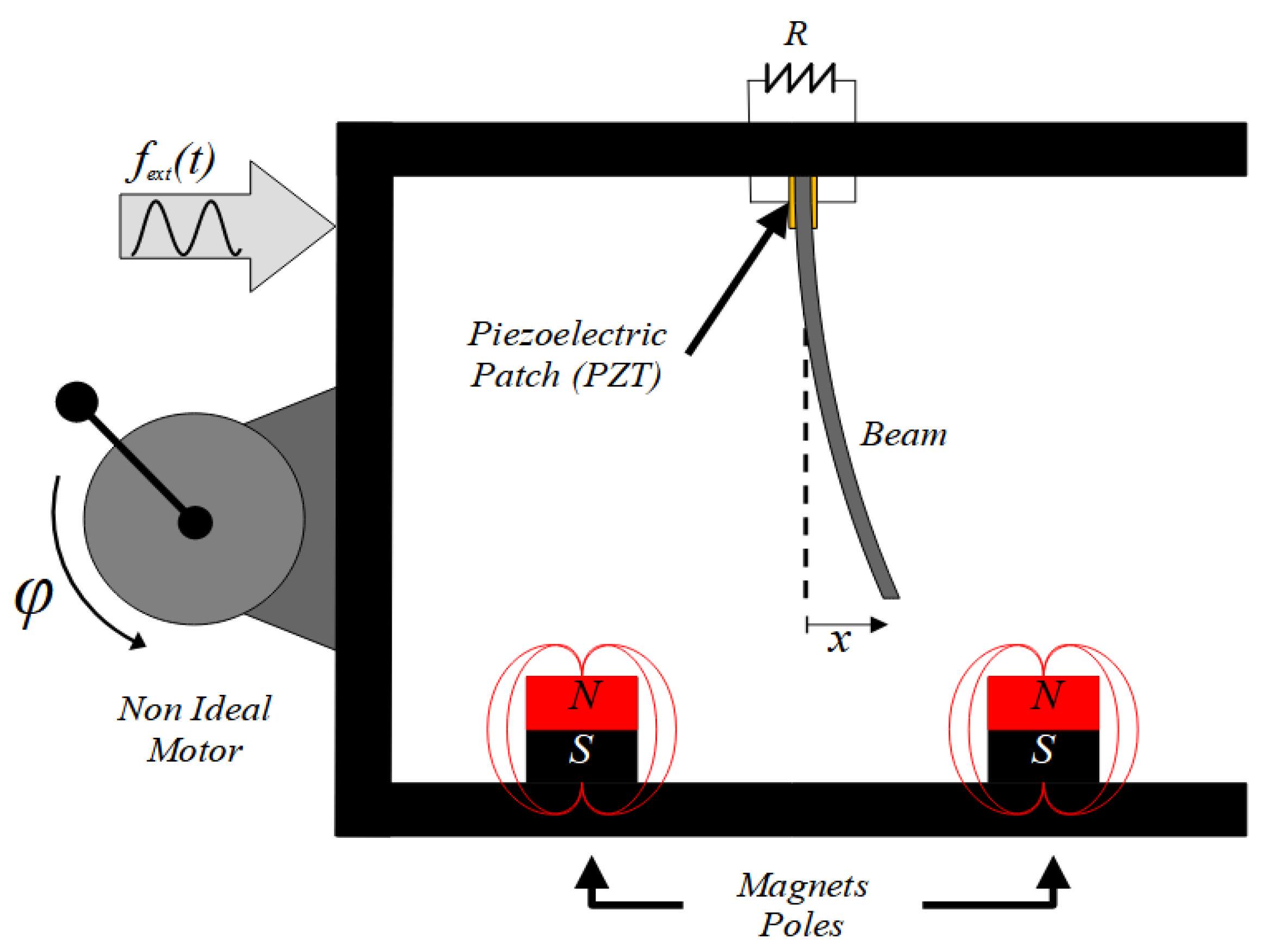
2. Magneto Piezo Oscillator Device
3. Mathematical Modeling
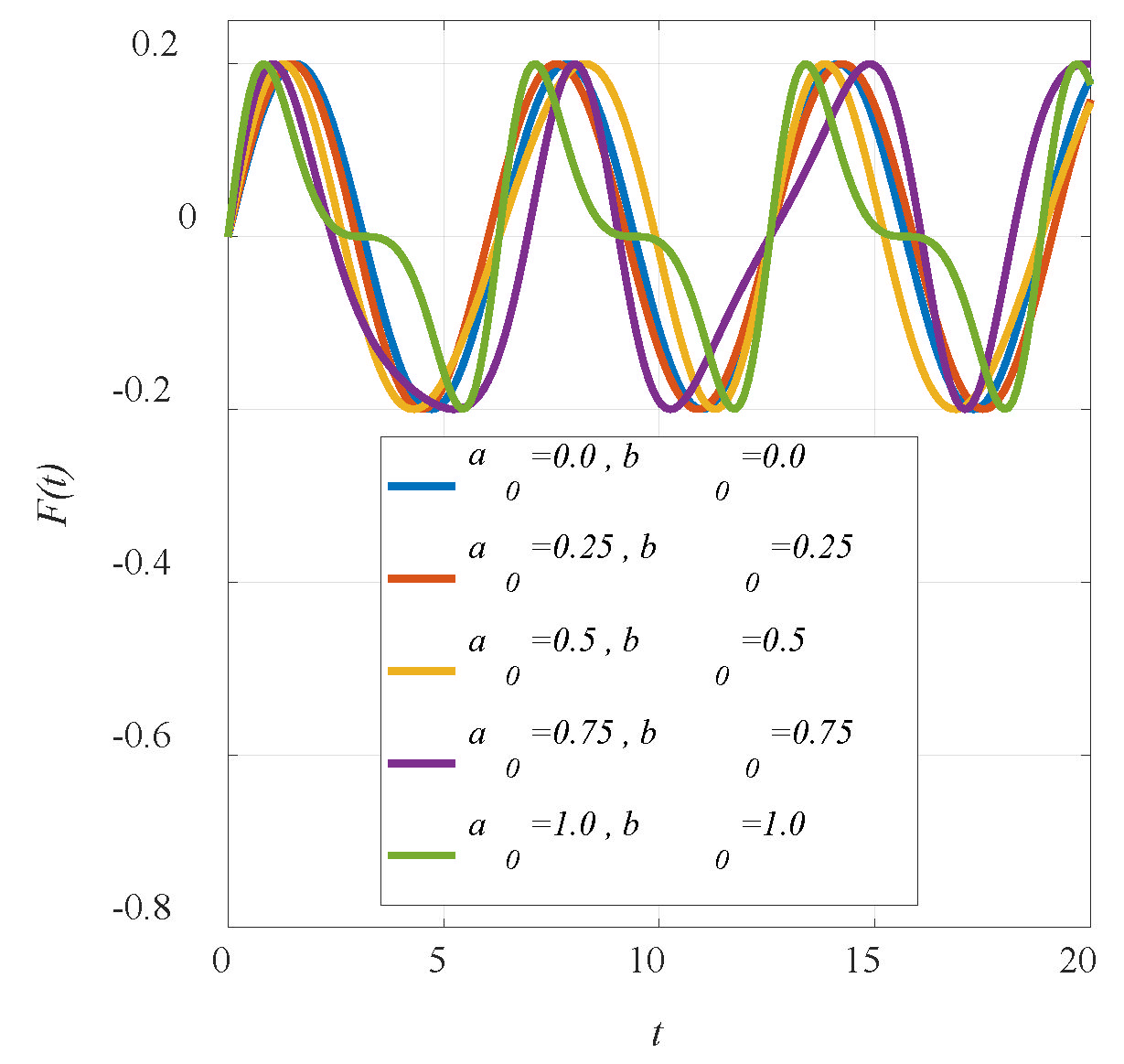
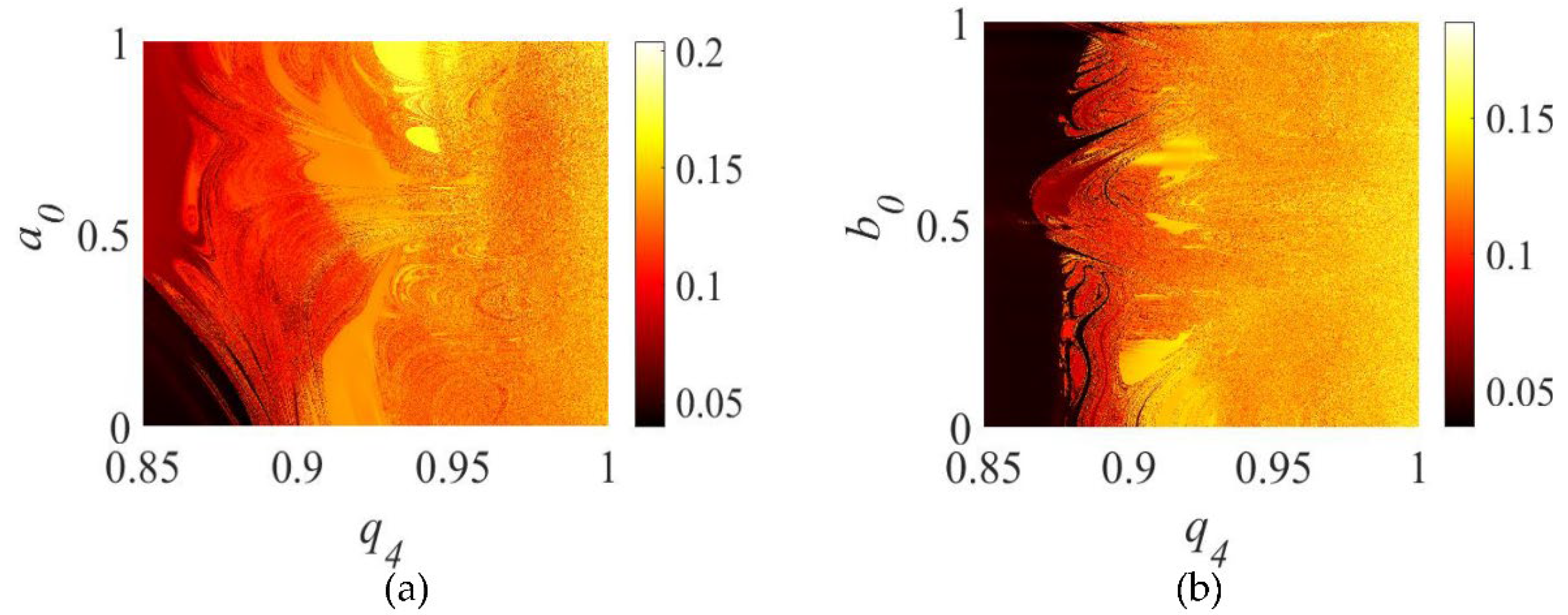
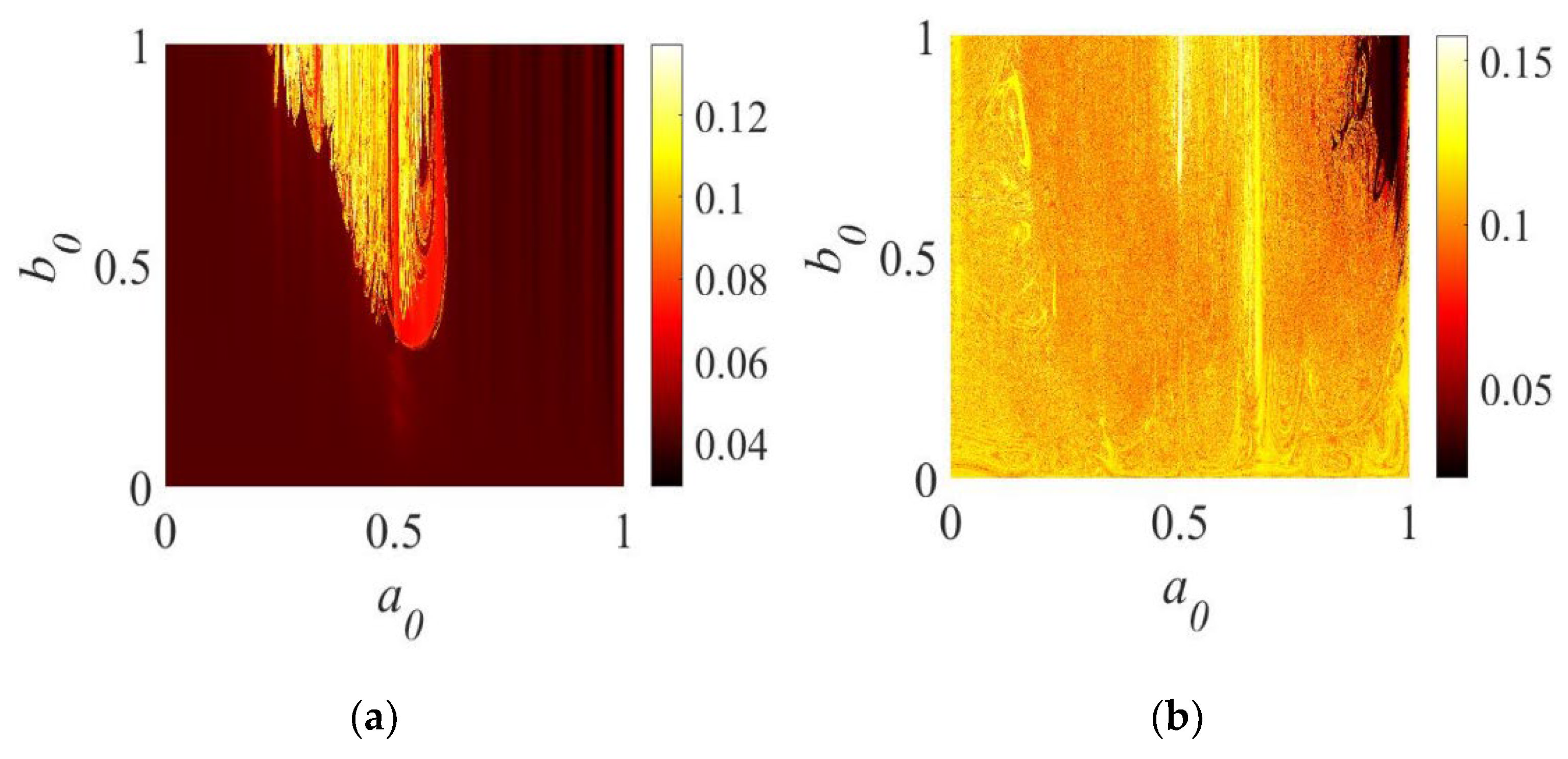
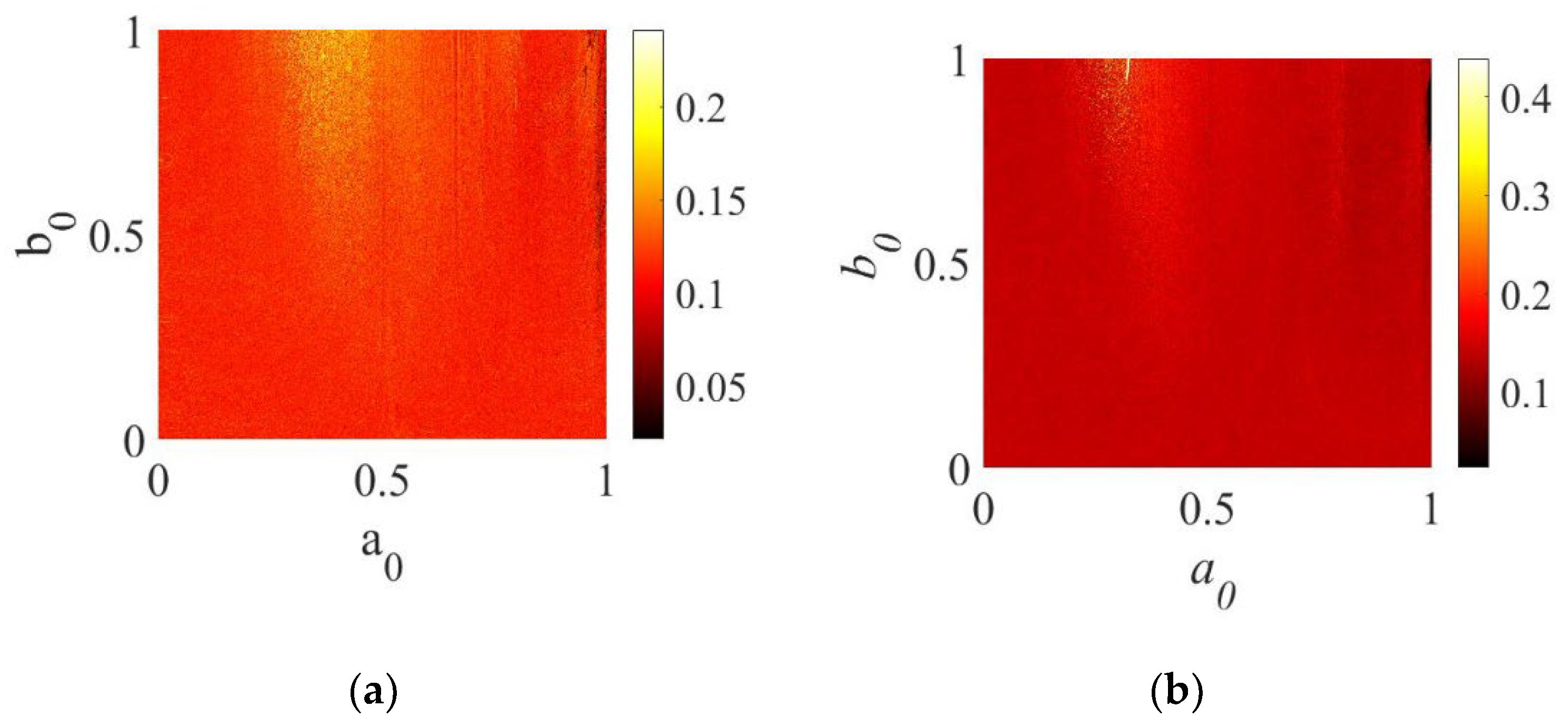
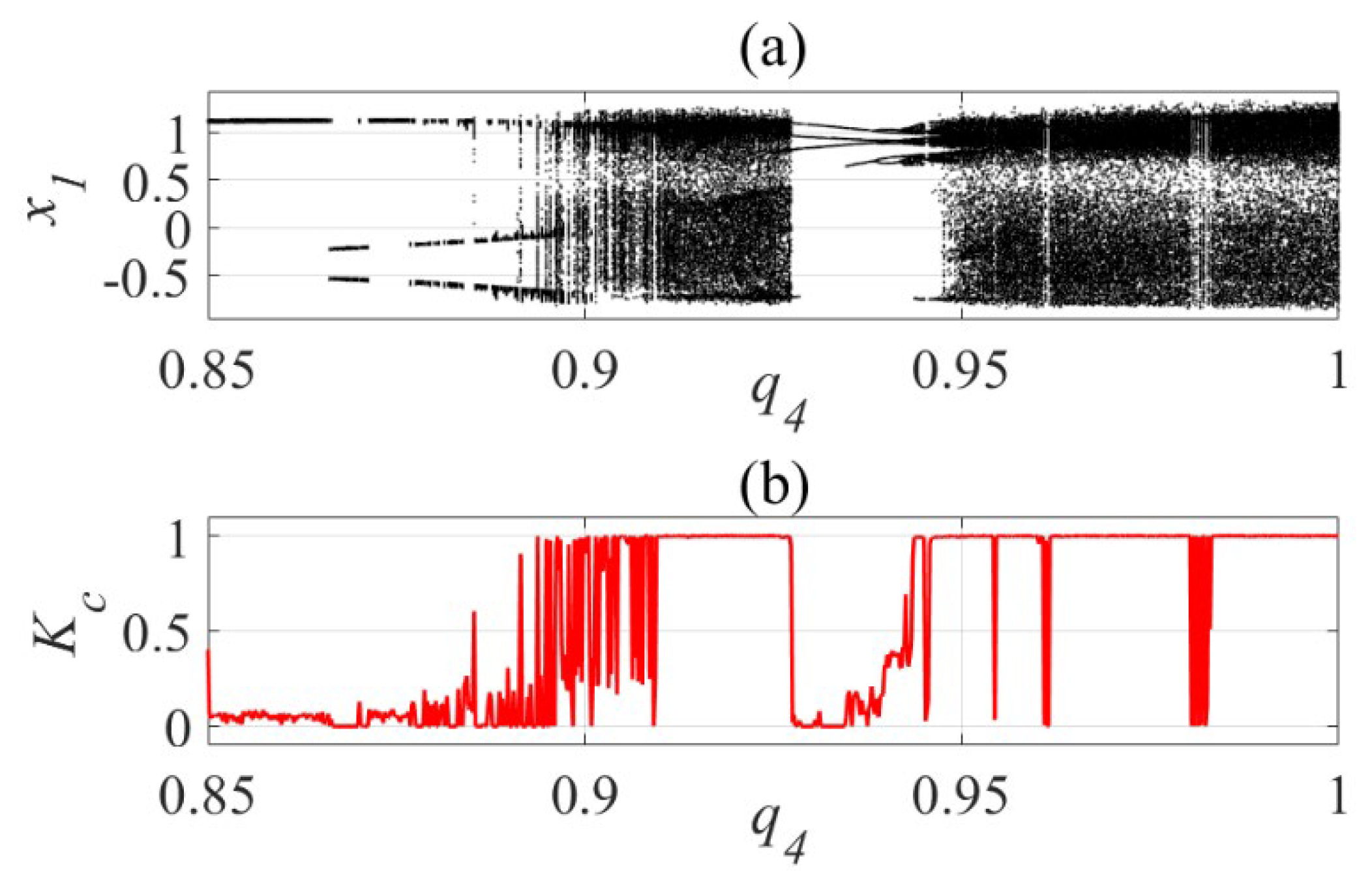
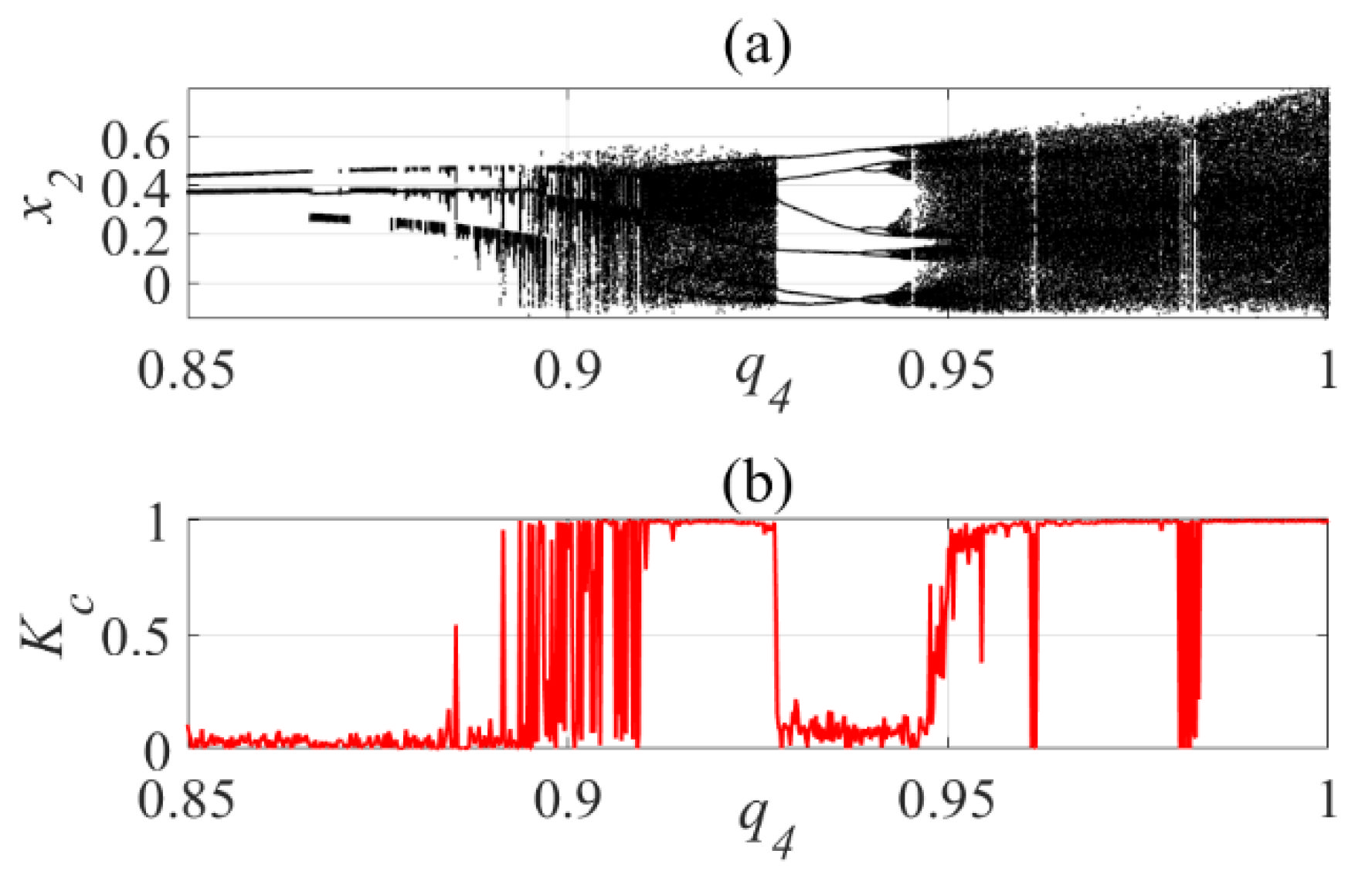
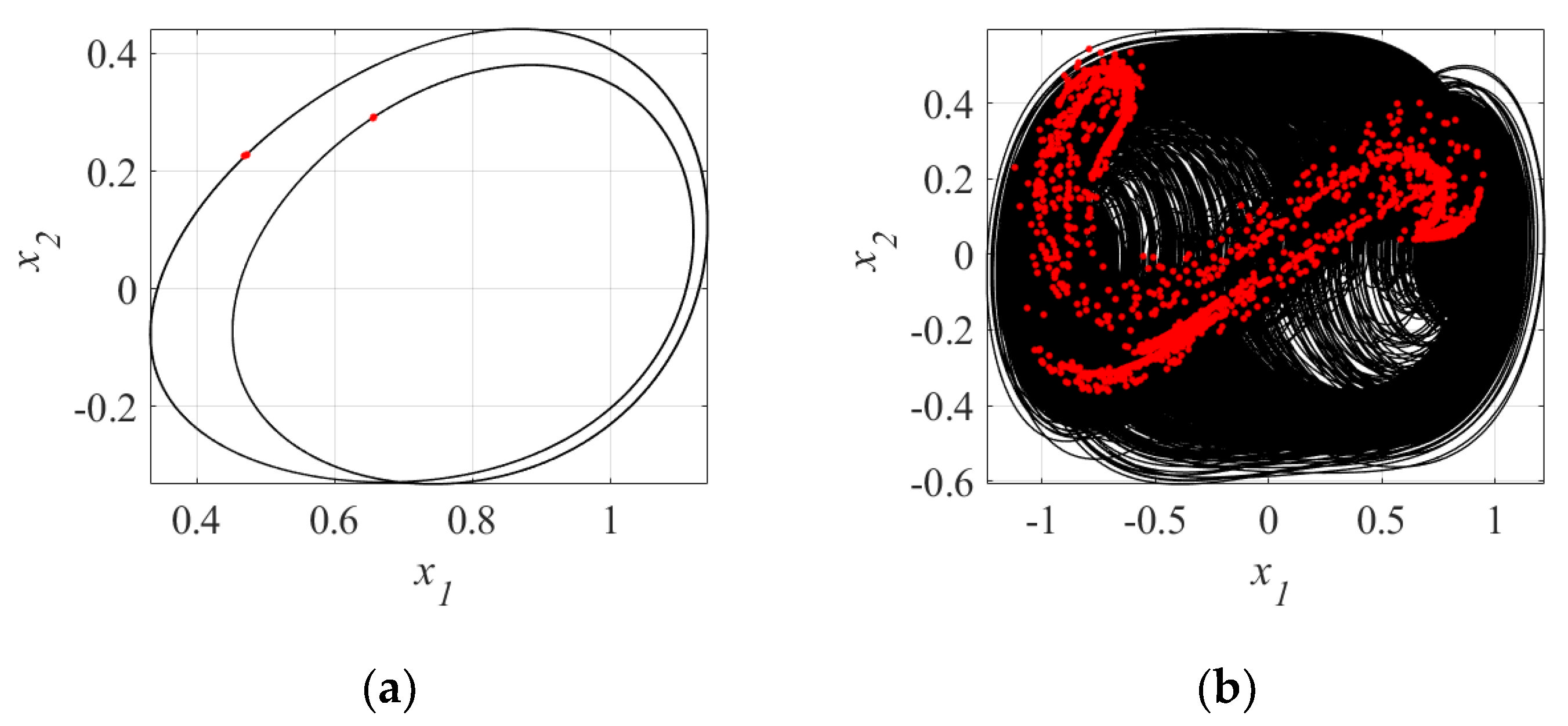
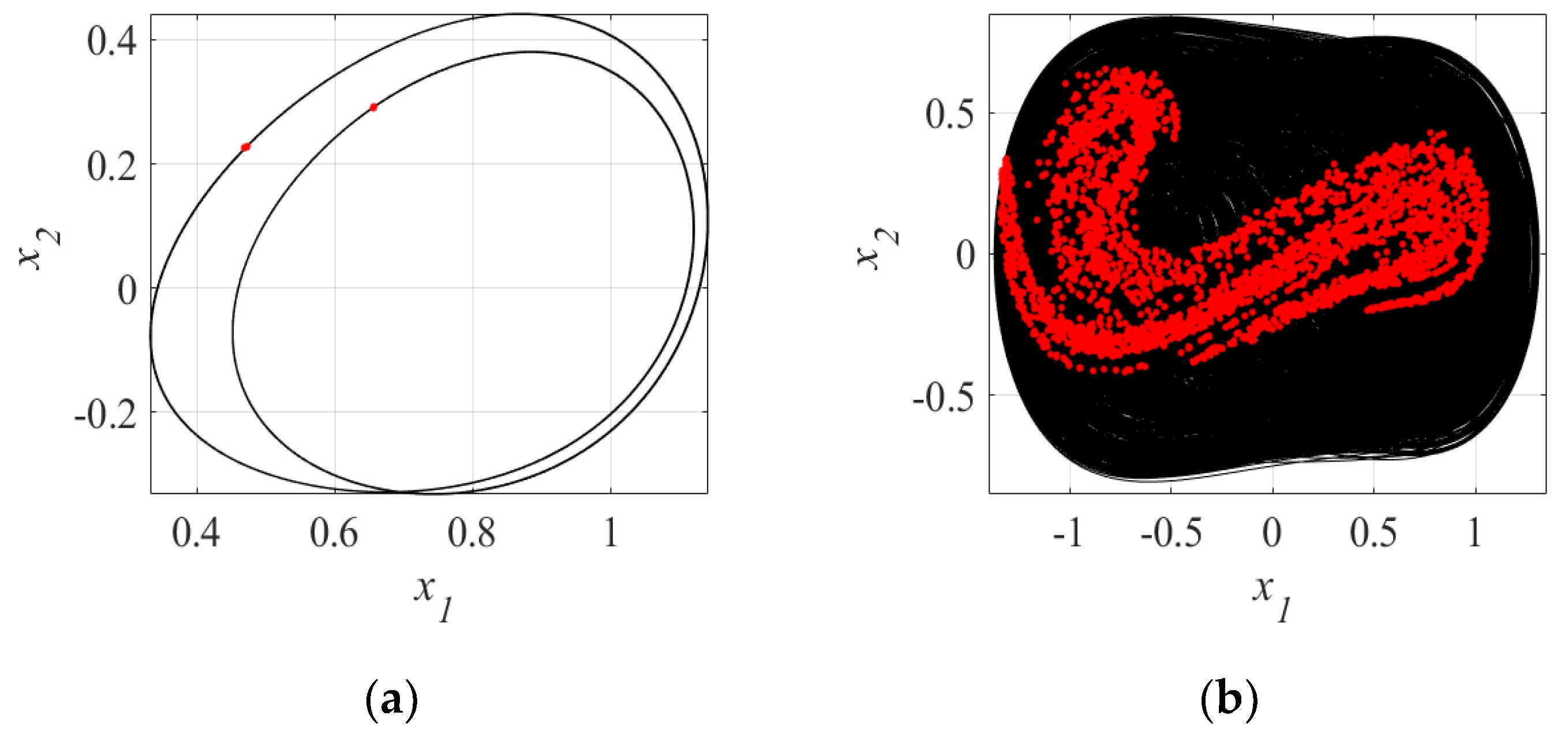
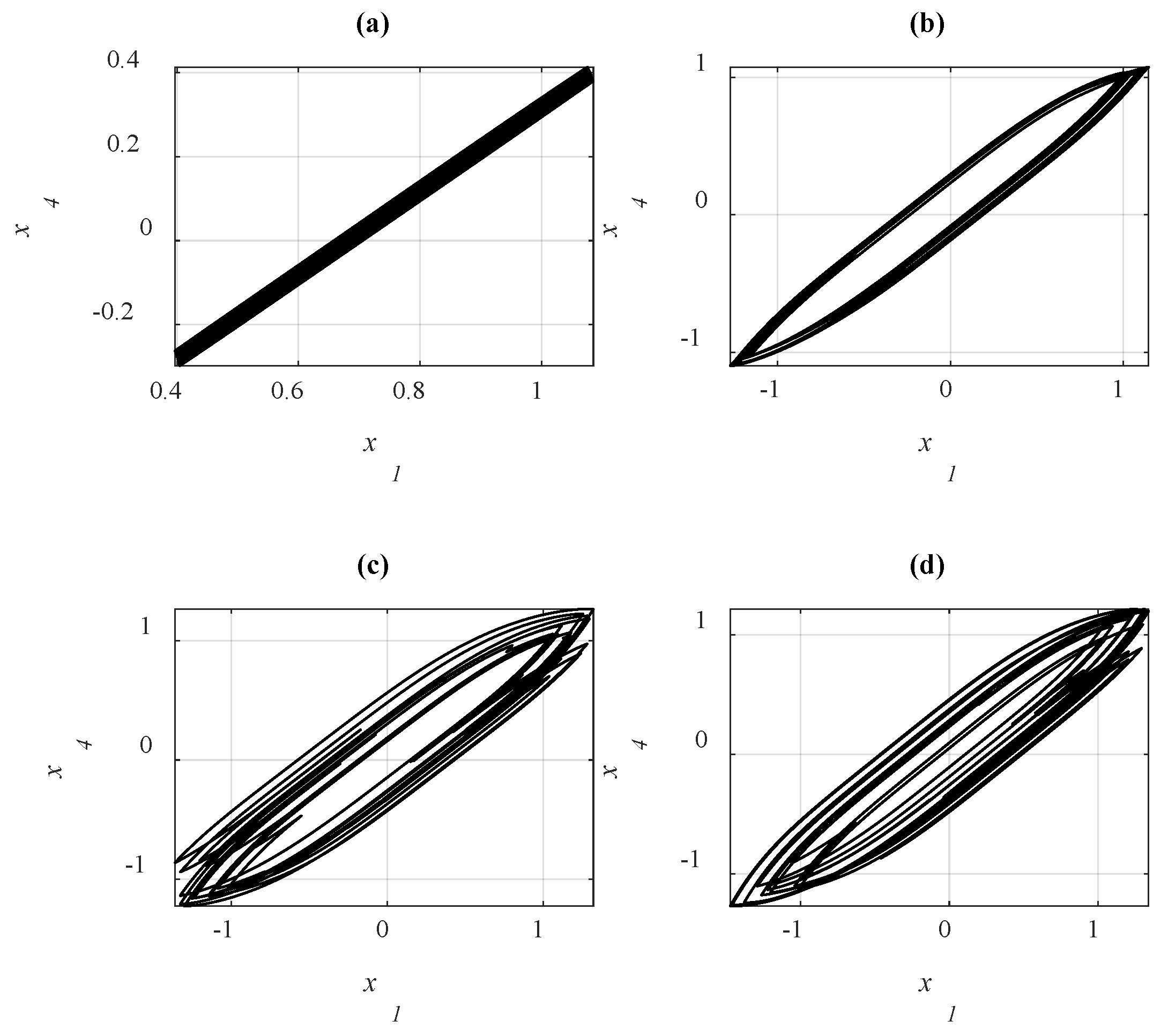
4. Numerical Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Ribeiro, M.A.; Tusset, A.M.; Lenz, W.B.; Felix, J.L.P.; Litak, G.; Balthazar, J.M. On non-ideal and fractional dynamics of a magneto piezo elastic oscillator with Bouc-Wen damping to harvesting energy. In DSTA-2021 Conference Books—Abstracts, Proceedings of the 16th International Conference of Dynamical Systems—Theory and Applications, Łódź, Poland, 6–9 December 2021; Awrejcewicz, J., Kaźmierczak, M., Mrozowski, J., Olejnik, P., Eds.; Marek Kaźmierczak Editor: Łódź, Poland; pp. 103–104. [CrossRef]
- Rocha, R.T.; Tusset, A.M.; Ribeiro, M.A.; Lenz, W.B.; Junior, R.H.; Jarzebowska, E.; Balthazar, J.M. On the positioning of a piezoelectric material in the energy harvesting from a nonideally excited portal frame. J. Comput. Nonlinear Dyn. 2020, 15, 121002-12. [Google Scholar] [CrossRef]
- Wang, J.; Gu, S.; Zhang, C.; Hu, G.; Chen, G.; Yang, K.; Li, H.; Lai, Y.; Litak, G.; Yurchenko, D. Hybrid wind energy scavenging by coupling vortex-induced vibrations and galloping. Energy Convers. Manag. 2020, 213, 112835. [Google Scholar] [CrossRef]
- Holmes, P. A nonlinear oscillator with a strange attractor. Philos. Trans. R. Soc. London. Ser. A Math. Phys. Sci. 1979, 292, 419–448. [Google Scholar]
- Wu, Z.; Harne, R.L.; Wang, K.W. Excitation-induced stability in a bistable Duffing oscillator: Analysis and experiments. J. Comput. Nonlinear Dyn. 2015, 10, 011016-7. [Google Scholar] [CrossRef]
- Erturk, A.; Inman, D.J. Piezoelectric Energy Harvesting; John Wiley & Sons: New York, NY, USA, 2011. [Google Scholar]
- Erturk, A.; Hoffmann, J.; Inman, D.J. A piezomagnetoelastic structure for broadband vibration energy harvesting. Appl. Phys. Lett. 2009, 94, 254102. [Google Scholar] [CrossRef]
- Litak, G.; Friswell, M.I.; Adhikari, S. Magnetopiezoelastic energy harvesting driven by random excitations. Appl. Phys. Lett. 2010, 96, 214103-3. [Google Scholar] [CrossRef]
- Kononenko, V.O. Vibrating Systems with A Limited Power Supply; Iliffe: Cambridge, London, UK, 1969. [Google Scholar]
- Felix, J.L.P.; Bianchin, R.P.; Almeida, A.; Balthazar, J.M.; Rocha, R.T.; Brasil, R.M. On energy transfer between vibration modes under frequency-varying excitations for energy harvesting. Appl. Mech. Mater. 2016, 849, 65–75. [Google Scholar] [CrossRef]
- Wang, L.X.; Willatzen, M. Nonlinear dynamical model for hysteresis based on nonconvex potential energy. J. Eng. Mech. 2007, 133, 506–513. [Google Scholar] [CrossRef]
- Noll, M.U.; Lentz, L.; Wagner, U.V. Comparison of the dynamics of a Duffing equation model and experimental results for a bistable beam beam in magnetoelastic energy harvesting. Tech. Mech. 2020, 40, 111–119. [Google Scholar]
- Wang, L.; Lu, Z.R. Identification of Bouc-Wen hysteretic parameters based on enhanced response sensitivity approach. J. Phys. 2017, 842, 012021. [Google Scholar] [CrossRef]
- Kumar, J.A.; Sundar, D.S. A numerical study on vibration control of a nonlinear Jeffcott rotor via Bouc-Wen model. FME Trans. 2019, 47, 190–194. [Google Scholar] [CrossRef]
- Kang, S.; Wu, H.; Li, Y.; Yang, X.; Yao, J. A Fractional-Order Normalized Bouc–Wen Model for Piezoelectric Hysteresis Nonlinearity. IEEE/ASME Trans. Mechatron. 2022, 27, 126–136. [Google Scholar] [CrossRef]
- Lu, Y.; Shan, J.; Gabbert, U.; Qi, N. Hysteresis and creep modeling and compensation for a piezoelectric actuator using a fractional-order Maxwell resistive capacitor approach. Smart Mater. Struct. 2013, 22, 115020. [Google Scholar] [CrossRef]
- Barba-Franco, J.J.; Gallegos, A.; Jaimes-Reátegui, R.; Pisarchik, A.N. Dynamics of a ring of three fractional-order Duffing oscillators. Chaos Solitons Fractals 2022, 155, 111747. [Google Scholar] [CrossRef]
- Erturk, A.; Inman, D.J. Broadband piezoelectric power generation on high-energy orbits of the bistable Duffing oscillator with electromechanical coupling. J. Sound Vib. 2011, 330, 2339–2353. [Google Scholar] [CrossRef]
- Elvin, N.; Erturk, A. Advances in Energy Harvesting Methods; Springer Science: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Wang, W.; Cao, J.; Bowen, C.R.; Inman, D.J.; Lin, J. Performance enhancement of nonlinear asymmetric bistable energy harvesting from harmonic, random and human motion excitations. Appl. Phys. Lett. 2018, 112, 213903. [Google Scholar] [CrossRef]
- Triplett, A.; Quinn, D.D. The effect of non-linear piezoelectric coupling on vibration-based energy harvesting. J. Intell. Mater. Syst. Struct. 2009, 20, 1959–1967. [Google Scholar] [CrossRef]
- Norenberg, J.P.; Cunha, A.; da Silva, S.; Varoto, P.S. Global sensitivity analysis of asymmetric energy harvesters. Nonlinear Dyn. 2022, 109, 443–458. [Google Scholar] [CrossRef]
- Litak, G.; Friswell, M.I.; Kwuimy, C.A.K.; Adhikari, S.; Borowiec, M. Energy harvesting by two magnetopiezoelastic oscillators with mistuning. Theor. Appl. Mech. Lett. 2012, 2, 043009. [Google Scholar] [CrossRef]
- Amin Karami, M.; Inman, D.J. Powering pacemakers from heartbeat vibrations using linear and nonlinear energy harvesters. Appl. Phys. Lett. 2012, 100, 042901. [Google Scholar] [CrossRef]
- Abouelregal, A.E.; Alanazi, R.; Sedighi, H.M. Thermal plane waves in unbounded non-local medium exposed to a moving heat source with a non-singular kernel and higher order time derivatives. Eng. Anal. Bound. Elem. 2022, 140, 464–475. [Google Scholar] [CrossRef]
- Hilal, M.I. Thermomechanical interactions of rotating thermoelastic magneto-microelongated medium heated by laser and initially stressed via non-local elasticity and GN III. Acta Mech. 2022, 233, 5183–5197. [Google Scholar] [CrossRef]
- Zhou, S.; Cao, J.; Inman, D.J.; Lin, J.; Li, D. Harmonic balance analysis of nonlinear tristable energy harvesters for performance enhancement. J. Sound Vib. 2016, 373, 223–235. [Google Scholar] [CrossRef]
- Lee, A.J.; Inman, D.J. Electromechanical modelling of a bistable plate with macro fiber composites under nonlinear vibrations. J. Sound Vib. 2019, 446, 326–342. [Google Scholar] [CrossRef]
- Fang, S.; Zhou, S.; Yurchenko, D.; Yang, T.; Liao, W.H. Multistability phenomenon in signal processing, energy harvesting, composite structures, and metamaterials: A review. Mech. Syst. Signal Process. 2022, 166, 108419. [Google Scholar] [CrossRef]
- Shahsavar, M.; Ashory, M.R.; Khatibi, M.M. Increasing the efficiency of a bistable beam beam energy harvester exploiting vibro-impact effects. J. Intell. Mater. Syst. Struct. 2022, 1045389X221115703. [Google Scholar] [CrossRef]
- Abedini, A.; Wang, F. Energy harvesting of a frequency up-conversion piezoelectric harvester with controlled impact. Eur. Phys. J. Spec. Top. 2019, 228, 1459–1474. [Google Scholar] [CrossRef]
- Ghouli, Z.; Litak, G. Effect of High-Frequency Excitation on a Bistable Energy Harvesting System. J. Vib. Eng. Technol. 2022, 1–8. [Google Scholar] [CrossRef]
- Harne, R.L.; Wang, K.W. A review of the recent research on vibration energy harvesting via bistable systems. Smart Mater. Struct. 2013, 22, 023001. [Google Scholar] [CrossRef]
- Syta, A.; Bowen, C.R.; Kim, H.A.; Rysak, A.; Litak, G. Experimental analysis of the dynamical response of energy harvesting devices based on bistable laminated plates. Meccanica 2015, 50, 1961–1970. [Google Scholar] [CrossRef]
- Wang, K.W.; Harne, R.L. Harnessing Bistable Structural Dynamics: For Vibration Control, Energy Harvesting and Sensing; John Wiley & Sons: Hoboken, NJ, USA, 2017. [Google Scholar]
- Safaei, M.; Sodano, H.A.; Anton, S.R. A review of energy harvesting using piezoelectric materials: State-of-the-art a decade later (2008–2018). Smart Mater. Struct. 2019, 28, 113001. [Google Scholar] [CrossRef]
- Daqaq, M.F.; Masana, R.; Erturk, A.; Quinn, D.D. On the role of nonlinearities in vibratory energy harvesting: A critical review and discussion. Appl. Mech. Rev. 2014, 66, 40801. [Google Scholar] [CrossRef]
- Huang, D.; Zhou, S.; Litak, G. Theoretical analysis of multistable energy harvesters with high-order stiffness terms. Commun. Nonlinear Sci. Numer. Simul. 2019, 69, 270–286. [Google Scholar] [CrossRef]
- Osinaga, S.; Febbo, M.; Machado, S. Effect of elastic restraints in the modeling of prestressed piezoelectric energy harvesters. Appl. Math. Model. 2022, 101, 573–585. [Google Scholar] [CrossRef]
- Kang, S.; Wu, H.; Yu, S.; Li, Y.; Yang, X.; Yao, J. Modeling and control of a six-axis parallel piezo-flexural micropositioning stage with cross-coupling hysteresis nonlinearities. In Proceedings of the 2020 IEEE/ASME International Conference on Advanced Intelligent Mechatronics (AIM), Boston, MA, USA, 6–9 July 2020; pp. 1350–1355. [Google Scholar]
- Spanos, P.D.; Di Matteo, A.; Pirrotta, A. Steady-state dynamic response of various hysteretic systems endowed with fractional derivative elements. Nonlinear Dyn. 2019, 98, 3113–3124. [Google Scholar] [CrossRef]
- Solovyov, A.M.; Semenov, M.E.; Meleshenko, P.A.; Barsukov, A.I. Bouc-Wen model of hysteretic damping. Procedia Eng. 2017, 201, 549–555. [Google Scholar] [CrossRef]
- Ismail, M.; Ikhouane, F.; Rodellar, J. The hysteresis Bouc-Wen model, a survey. Arch. Comput. Methods Eng. 2009, 16, 161–188. [Google Scholar] [CrossRef]
- Ikhouane, F.; Rodellar, J. On the hysteretic Bouc–Wen model. Nonlinear Dyn. 2005, 42, 63–78. [Google Scholar] [CrossRef]
- Petrás, I. Fractional Derivatives, Fractional Integrals, and Fractional Differential Equations in Matlab. In Engineering Education and Research Using MATLAB; Ali, H.A., Ed.; INTECH Open Access Publisher: Rijeka, Croatia, 2011; pp. 239–264. [Google Scholar]
- Petrás, I. Fractional-Order Nonlinear Systems: Modeling, Analysis and Simulation; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Baleanu, D.; Avkar, T. Lagrangians with linear velocities within riemann-liouville fractional derivatives. Il Nuovo Cim. B 2004, 119, 73–79. [Google Scholar]
- Atangana, A.; Gómez-Aguilar, J.F. Numerical approximation of riemann-liouville definition of fractional derivative: From riemann-liouville to atangana-baleanu. Numer. Methods Partial. Differ. Equ. 2018, 34, 1502–1523. [Google Scholar] [CrossRef]
- Hilfer, R. Applications of Fractional Calculus in Physics; World Scientific Singapore: Singapore, 2000; Volume 35. [Google Scholar]
- Iliuk, I.; Balthazar, J.M.; Tusset, A.M.; Piqueira, J.R.; de Pontes, B.R.; Felix, J.L.; Bueno, A.M. Application of passive control to energy harvester efficiency using a nonideal portal frame structural support system. J. Intell. Mater. Syst. Struct. 2014, 25, 417–429. [Google Scholar] [CrossRef]
- Priya, S.; Inman, D.J. (Eds.) Energy Harvesting Technologies; Springer: New York, NY, USA, 2009; Volume 21, p. 2. [Google Scholar]
- Stoleriu, L.; Ciomaga, C.; Fochi, F.; Ochoa, P.; Fernández, J.F.; Galassi, C.; Buscaglia, V.; Mitoseriu, L. Mechanically clamped PZT ceramics investigated by First-order reversal curves diagram. Process. Appl. Ceram. 2010, 4, 209–214. [Google Scholar] [CrossRef]
- Silva, L.L.; Savi, M.A.; Monteiro, P.C., Jr.; Netto, T.A. Effect of the piezoelectric hysteretic behavior on the vibration-based energy harvesting. J. Intell. Mater. Syst. Struct. 2013, 24, 1278–1285. [Google Scholar] [CrossRef]
- Gottwald, G.A.; Melbourne, I. A new test for chaos in deterministic systems. Proc. R. Soc. Lond. A Math. Phys. Eng. Sci. 2004, 460, 603–611. [Google Scholar] [CrossRef]
- Gottwald, G.; Melbourne, I. Testing for chaos in deterministic systems with noise. Phys. D Nonlinear Phenom. 2005, 212, 100–110. [Google Scholar] [CrossRef]
- Litak, G.; Syta, A.; Wiercigroch, M. Identification of chaos in a cutting process by the 0–1 test. Chaos Solitons Fractals 2009, 40, 2095–2101. [Google Scholar] [CrossRef]
- Fractional Order Chaotic Systems. Available online: https://www.mathworks.com/matlabcentral/fileexchange/27336-fractional-order-chaotic-systems (accessed on 21 November 2022).
| Parameter | Values | Parameter | Values |
|---|---|---|---|
| 0.01 | 0.01 | ||
| 0.25 | 0.5 | ||
| 0.05 | 1.0 | ||
| 1.0 | 0.55 | ||
| 1.1 | 0.45 | ||
| 3 | 0.2 | ||
| a | 0.5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ribeiro, M.A.; Balthazar, J.M.; Lenz, W.B.; Felix, J.L.P.; Litak, G.; Tusset, A.M. Fractional Dynamical Behavior of an Elastic Magneto Piezo Oscillator Including Non-Ideal Motor Excitation. Axioms 2022, 11, 667. https://doi.org/10.3390/axioms11120667
Ribeiro MA, Balthazar JM, Lenz WB, Felix JLP, Litak G, Tusset AM. Fractional Dynamical Behavior of an Elastic Magneto Piezo Oscillator Including Non-Ideal Motor Excitation. Axioms. 2022; 11(12):667. https://doi.org/10.3390/axioms11120667
Chicago/Turabian StyleRibeiro, Mauricio A., Jose M. Balthazar, Wagner B. Lenz, Jorge L. P. Felix, Grzegorz Litak, and Angelo M. Tusset. 2022. "Fractional Dynamical Behavior of an Elastic Magneto Piezo Oscillator Including Non-Ideal Motor Excitation" Axioms 11, no. 12: 667. https://doi.org/10.3390/axioms11120667
APA StyleRibeiro, M. A., Balthazar, J. M., Lenz, W. B., Felix, J. L. P., Litak, G., & Tusset, A. M. (2022). Fractional Dynamical Behavior of an Elastic Magneto Piezo Oscillator Including Non-Ideal Motor Excitation. Axioms, 11(12), 667. https://doi.org/10.3390/axioms11120667









