Hypercomplex Systems and Non-Gaussian Stochastic Solutions with Some Numerical Simulation of χ-Wick-Type (2 + 1)-D C-KdV Equations
Abstract
:1. Introduction
2. Solitary TWS in Equation (2)
- Case I.
- Case II.
- Case III. At and , then . So, we findwith
3. NG-Stochastic Solutions of Equation (2)
- (I) NG-Stochastic Solutions of JEF Type:
- (II) NG-Stochastic Solutions of the Trigonometric Type
- (III) NG-Stochastic Solutions of the Hyperbolic Type
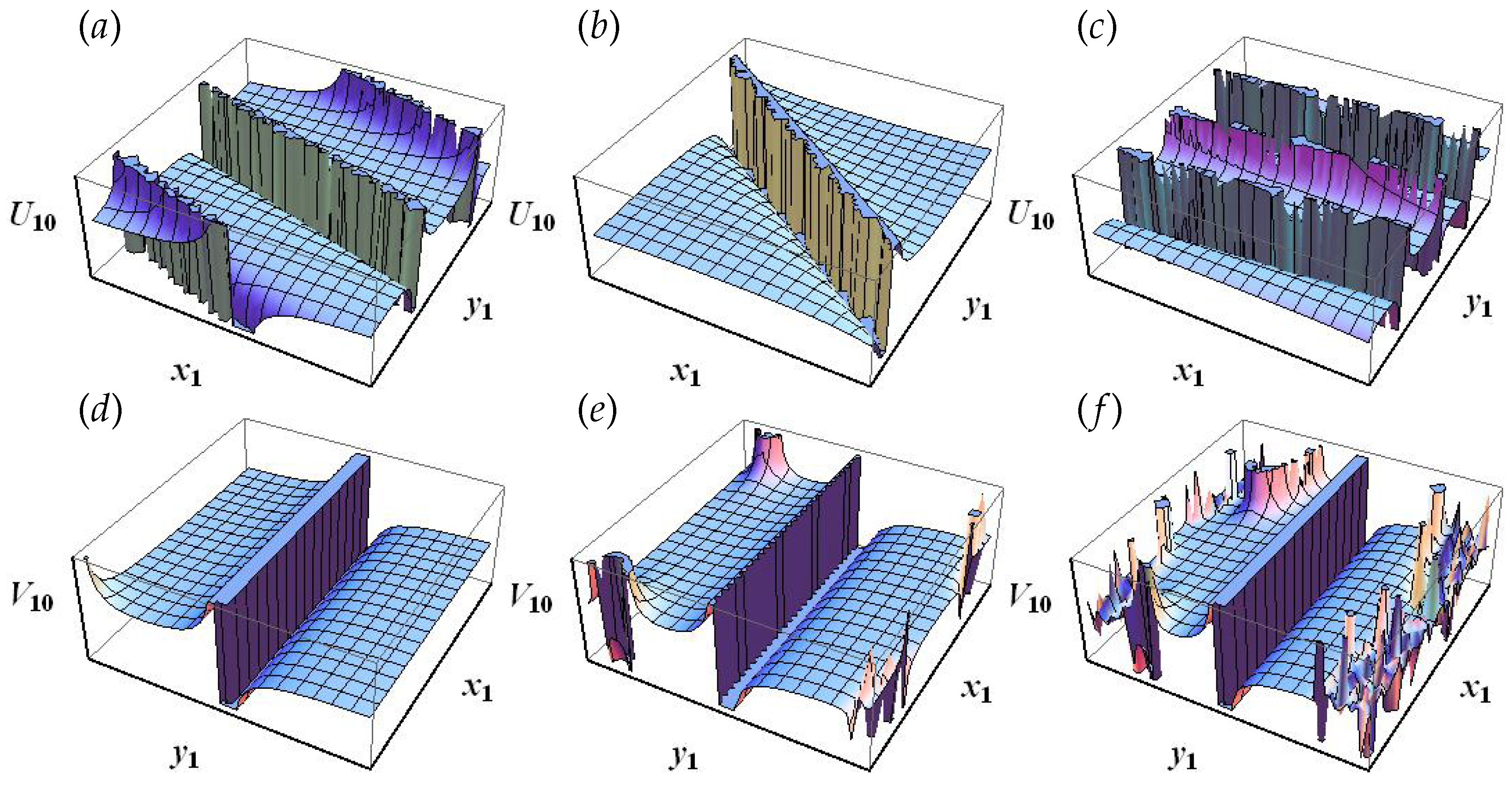
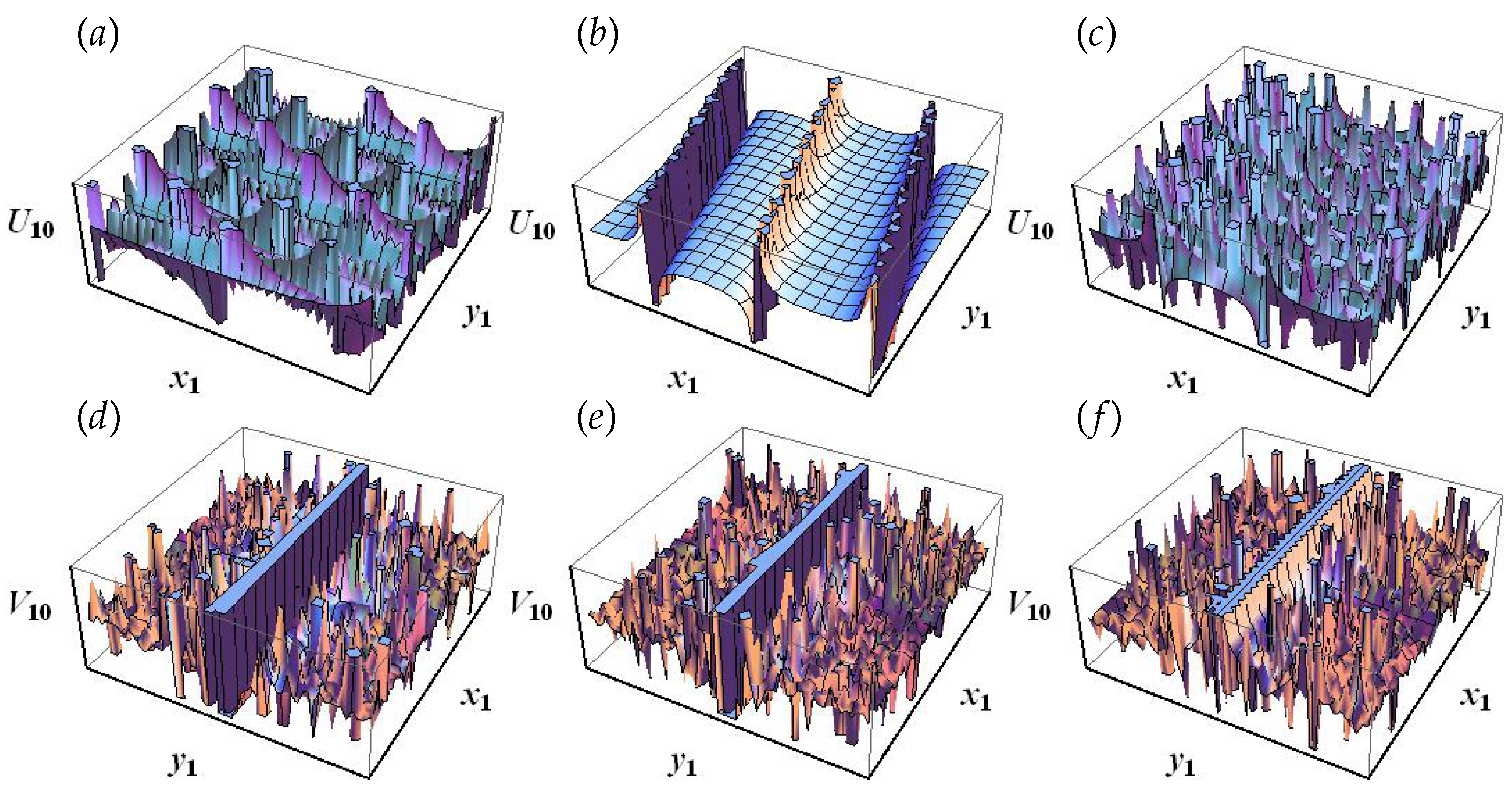
4. Example
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. ODE and Jacobi Elliptic Functions
| 1 | |||
| 1 | |||
| 1 | |||
| 1 | |||
| 1 | |||
Appendix B
Appendix C
References
- El Bab, A.S.O.; Ghany, H.A.; Zakarya, M. A construction of non-Gaussian white noise analysis using the theory of hypercomplex systems. Glob. J. Sci. Front. Res. F Math. Decis. Sci. 2016, 16, 11–25. [Google Scholar]
- Zakarya, M. Hypercomplex Systems with Some Applications of White Noise Analysis; LAP LAMBERT Academic Publishing: Port Louis, Mauritius, 2017; ISBN-13: 978-620-2-07650-0. [Google Scholar]
- Borhanifar, A.; Reza, A. Exact solutions for non-linear Schrodinger equations by differential transformation method. J. Appl. Math. Comput. 2011, 35, 37–51. [Google Scholar] [CrossRef]
- Tari, A.; Rahimi, M.Y.; Shahmorad, S.; Talati, F. Solving a class of two dimensional linear and nonlinear Volterra integral equations by the differential transform method. J. Comput. Appl. Math. 2009, 228, 70–76. [Google Scholar] [CrossRef]
- Ghany, H.A.; Zakarya, M. Generalized solutions of Wick-type stochastic KdV-Burgers equations using Exp-function method. Int. Rev. Phys. 2014, 18, 38–46. [Google Scholar]
- Ghany, H.A.; El Bab, A.O.; Zabel, A.M.; Hyder, A.A. The fractional coupled KdV equations: Exact solutions and white noise functional approach. Chin. Phys. B 2013, 22, 080501. [Google Scholar] [CrossRef]
- Ghany, H.A.; Elagan, S.K.; Hyder, A. Exact travelling wave solutions for stochastic fractional Hirota-Satsuma coupled KdV equations. Chin. J. Phys. 2015, 53, 153–166. [Google Scholar]
- Drazin, P.G.; Johnson, R.S. Soliton: An Introduction; Cambridge Texts in Applied Mathematics; Cambridge University Press: Cambridge, UK, 1989. [Google Scholar] [CrossRef]
- Abbasbandy, S. The application of homotopy analysis method to solve a generalized Hirota-Satsuma coupled KdV equation. Phys. Lett. A 2007, 361, 478–483. [Google Scholar] [CrossRef]
- Ghanbari, B.; Nisar, K.S. Determining new soliton solutions for a generalized nonlinear evolution equation using an effective analytical method. Alex. Eng. J. 2020, 59, 3171–3179. [Google Scholar] [CrossRef]
- Munusamy, K.; Ravichandran, C.; Nisar, K.S.; Ghanbari, B. Existence of solutions for some functional integrodifferential equations with nonlocal conditions. Math. Meth. Appl. Sci. 2020, 43, 10319–10331. [Google Scholar] [CrossRef]
- Ghanbari, B.; Nisar, K.S.; Aldhaifallah, M. Abundant solitary wave solutions to an extended nonlinear Schrödinger’s equation with conformable derivative using an efficient integration method. Adv. Differ. Equ. 2020, 328, 1–25. [Google Scholar] [CrossRef]
- Akbar, M.; Nawaz, R.; Ahsan, S.; Nisar, K.S.; Abdel-Aty, A.H.; Eleuch, H. New Approach to Approximate the Solution of System of Fractional order Volterra Integro-differential Equations. Results Phys. 2020, 19, 103453. [Google Scholar] [CrossRef]
- Raza, A.; Rafiq, M.; Ahmed, N.; Khan, I.; Nisar, K.S.; Iqbal, Z. A Structure Preserving Numerical Method for Solution of Stochastic Epidemic Model of Smoking Dynamics. Comput. Mater. Contin. 2020, 65, 263–278. [Google Scholar] [CrossRef]
- Ghaffar, A.; Ali, A.; Ahmed, S.; Akram, S.; Junjua, M.U.; Baleanu, D.; Nisar, K.S. A novel analytical technique to obtain the solitary solutions for nonlinear evolution equation of fractional order. Adv. Differ. Equ. 2020, 2020, 1–15. [Google Scholar] [CrossRef]
- Akram, S.; Nawaz, A.; Yasmin, N.; Ghaffar, A.; Baleanu, D.; Nisar, K.S. Periodic Solutions of Some Classes of One Dimensional Non-autonomous Equation. Front. Phys. 2020, 8, 264. [Google Scholar] [CrossRef]
- Baleanu, D.; Ghanbari, B.; Asad, J.H.; Jajarmi, A.; Pirouz, H.M. Planar System-Masses in an Equilateral Triangle: Numerical Study within Fractional Calculus. Comput. Model. Eng. Sci. 2020, 124, 953–968. [Google Scholar] [CrossRef]
- Jajarmi, A.; Baleanu, D. A New Iterative Method for the Numerical Solution of High-Order Non-linear Fractional Boundary Value Problems. Front. Phys. 2020, 8, 220. [Google Scholar] [CrossRef]
- Baleanu, D.; Jajarmi, A.; Sajjadi, S.S.; Asad, J.H. The fractional features of a harmonic oscillator with position-dependent mass. Commun. Theor. Phys. 2020, 72, 055002. [Google Scholar] [CrossRef]
- Hajipour, M.; Jajarmi, A.; Baleanu, D.; Sun, H. On an accurate discretization of a variable-order fractional reaction-diffusion equation. Commun. Nonlinear Sci. Numer. Simul. 2019, 69, 119–133. [Google Scholar] [CrossRef]
- Hajipour, M.; Jajarmi, A.; Malek, A.; Baleanu, D. Positivity-preserving sixth-order implicit finite difference weighted essentially non-oscillatory scheme for the nonlinear heat equation. Appl. Math. Comput. 2018, 325, 146–158. [Google Scholar] [CrossRef]
- Ghany, H.A.; Hyder, A. White noise functional solutions for the Wick-type two dimensional stochastic Zakharov-Kuznetsov equations. Int. Rev. Phys. 2012, 6, 153–157. [Google Scholar]
- Ghany, H.A.; Hyder, A. Exact solutions for the Wick-type stochastic time-fractional KdV equations. Kuwait J. Sci. 2014, 22, 75–84. [Google Scholar]
- Ghany, H.A.; Hyder, A. Abundant solutions of Wick-type stochastic fractional 2D KdV equations. Chin. Phys. B 2014, 23, 75–84. [Google Scholar] [CrossRef]
- Ghany, H.A.; Zakarya, M. Exact solutions for Wick-type stochastic coupled KdV equations. Glob. J. Sci. Front. Res. F Math. Decis. Sci. 2014, 14, 57–71. [Google Scholar]
- Ghany, H.A.; Zakarya, M. Exact travelling wave solutions for Wick-type stochastic Schamel-KdV equations using F-expansion method. Phys. Res. Int. 2014, 1, 1–9. [Google Scholar] [CrossRef]
- Ghany, H.A.; Hyder, A. Local and global well-posedness of stochastic Zakharov-Kuznetsov equation. J. Comput. Anal. Appl. 2013, 15, 1332–1343. [Google Scholar]
- Ghany, H.A. Exact solutions for stochastic generalized Hirota-Satsuma coupled KdV equations. Chin. J. Phys. 2011, 49, 926–940. [Google Scholar]
- Ghany, H.A. Exact solutions for stochastic fractional Zakharov-Kuznetsov equations. Chin. J. Phys. 2013, 51, 875–881. [Google Scholar]
- Agarwal, P.; Hyder, A.A.; Zakarya, M.; AlNemer, G.; Cesarano, C.; Assante, D. Exact Solutions for a Class of Wick-Type Stochastic (3+1)-Dimensional Modified Benjamin-Bona-Mahony Equations. Axioms 2019, 8, 134. [Google Scholar] [CrossRef]
- Zhou, Y.; Wang, M.; Wang, Y. Periodic wave solutions to a coupled KdV equations with variable coefficients. Phys. Lett. A 2003, 308, 31–36. [Google Scholar] [CrossRef]
- Hyder, A.; Zakarya, M. The Well-Posedness of Stochastic Kawahara Equation: Fixed Point Argument and Fourier Restriction Method. J. Egypt. Math. Soc. 2019, 27, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Berezansky, Y.M. A connection between the theory of hypergroups and white noise analysis. Rep. Math. Phys. 1995, 36, 215–234. [Google Scholar] [CrossRef]
- Holden, H.; Øksendal, B.; Ubøe, J.; Zhang, T. Stochastic Partial Differential Equations; Springer: New York, NY, USA, 2010. [Google Scholar]
- Lindstrøm, T.; Øksendal, B.; Ubøe, J. Stochastic differential equations involving positive noise. In Stochastic Analysis; Barlow, M., Bingham, N., Eds.; Cambridge University Press: Cambridge, MA, USA, 1991; pp. 261–303. [Google Scholar]
- Zakarya, M. Hypercomplex Systems and Non-Gaussian Stochastic Solutions of χ-ick-Type (3 + 1) - Dimensional Modified BBM Equations Using the Generalized Modified Tanh-Coth Method. Therm. Sci. 2020, Accepted. [Google Scholar]
- Ma, W.X. Soliton solutions by means of Hirota bilinear forms. Partial Differ. Equations Appl. Math. 2022, 5, 100220. [Google Scholar] [CrossRef]
- Ma, W.X. N-soliton solution and the Hirota condition of a (2 + 1)-dimensional combined equation. Math. Comput. Simul. 2021, 190, 270–279. [Google Scholar] [CrossRef]
- Ma, W.X. Reduced nonlocal integrable mKdV equations of type (-lambda, lambda) and their exact soliton solutions. Commun. Theor. Phys. 2022, 74, 065002. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zakarya, M.; Abd-Rabo, M.A.; AlNemer, G. Hypercomplex Systems and Non-Gaussian Stochastic Solutions with Some Numerical Simulation of χ-Wick-Type (2 + 1)-D C-KdV Equations. Axioms 2022, 11, 658. https://doi.org/10.3390/axioms11110658
Zakarya M, Abd-Rabo MA, AlNemer G. Hypercomplex Systems and Non-Gaussian Stochastic Solutions with Some Numerical Simulation of χ-Wick-Type (2 + 1)-D C-KdV Equations. Axioms. 2022; 11(11):658. https://doi.org/10.3390/axioms11110658
Chicago/Turabian StyleZakarya, Mohammed, Mahmoud A. Abd-Rabo, and Ghada AlNemer. 2022. "Hypercomplex Systems and Non-Gaussian Stochastic Solutions with Some Numerical Simulation of χ-Wick-Type (2 + 1)-D C-KdV Equations" Axioms 11, no. 11: 658. https://doi.org/10.3390/axioms11110658
APA StyleZakarya, M., Abd-Rabo, M. A., & AlNemer, G. (2022). Hypercomplex Systems and Non-Gaussian Stochastic Solutions with Some Numerical Simulation of χ-Wick-Type (2 + 1)-D C-KdV Equations. Axioms, 11(11), 658. https://doi.org/10.3390/axioms11110658







