The Algebraic Surfaces of the Enneper Family of Maximal Surfaces in Three Dimensional Minkowski Space

Abstract

We consider the Enneper family of real maximal surfaces via Weierstrass data $(1,{\zeta }^m)$ for $\zeta \in \mathbb{C}$, $m\in {\mathbb{Z}}_{\ge 1}$. We obtain the irreducible surfaces of the family in the three dimensional Minkowski space ${\mathbb{E}}^{2,1}$. Moreover, we propose that the family has degree ${(2m+1)}^2$ (resp., class $2m(2m+1)$) in the cartesian coordinates $x,y,z$ (resp., in the inhomogeneous tangential coordinates $a,b,c$).

1. Introduction

A minimal surface is a surface of vanishing mean curvature in three dimensional Euclidean space ${\mathbb{E}}^3$. There are many classical and modern minimal surfaces in the literature. See Darboux [1,2], Dierkes [3], Fomenko and Tuzhilin [4], Gray, Salamon, and Abbena [5], Nitsche [6], Osserman [7], Spivak [8] for some books, Lie [9], Schwarz [10], Small [11,12], and Weierstrass [13,14] for some papers related to minimal surfaces in Euclidean geometry.

Lie [9] studied the algebraic minimal surfaces and gave a table classifying these surfaces. See also Enneper [15], Güler [16], Nitsche [6], and Ribaucour [17] for details.

Weierstrass [13] revealed a representation for minimal surfaces in three dimensional Euclidean space ${\mathbb{E}}^3.$ Almost one hundred years later, Kobayashi [18] gave an analogous Weierstrass-type representation for conformal spacelike surfaces with mean curvature identically 0, called maximal surfaces, in three dimensional Minkowski space ${\mathbb{E}}^{2,1}$.

In this paper, we consider the Enneper family of maximal surfaces ${\mathcal{E}}_m$ for positive integers $m\ge 1$ by using Weierstrass data $(1,{\zeta }^m)$ for $\zeta \in \mathbb{C}$, and then show that these surfaces are algebraic in ${\mathbb{E}}^{2,1}$. See Güler [16] for a Euclidean case of Enneper’s algebraic minimal surfaces family.

In Section 2, we give this family of real maximal surfaces in $\left(r,\theta \right)$ and $(u,v)$ coordinates by using Weierstrass representation in ${\mathbb{E}}^{2,1}$. In Section 3, we find irreducible algebraic equations defining surfaces ${\mathcal{E}}_m(u,v)$ in terms of running coordinates $x,y,z,$ and $a,b,c,$ and also compute degrees and classes of ${\mathcal{E}}_m(u,v)$. Finally, we summarize all findings in tables in the last section, then give some open problems.

2. Family of Enneper Maximal Surfaces

Let ${\mathbb{E}}^{n,1}:=\left(\{x={(x_1,\cdots ,x_n,x_0)}^t|x_i\in \mathbb{R}\},⟨·,·⟩\right)$ be the $(n+1)$-dimensional Lorentz–Minkowski (for short, Minkowski) space with Lorentzian metric $⟨x,y⟩=x_1{y}_1+\cdots +x_n{y}_n-x_0{y}_0$.

A vector $x\in {\mathbb{E}}^{n,1}$ is called space-like if $⟨x,x⟩>0$, time-like if $⟨x,x⟩<0,$ and light-like if $x\ne 0$ and $⟨x,x⟩=0.$ A surface in ${\mathbb{E}}^{n,1}$ is called space-like (resp. time-like, light-like) if the induced metric on the tangent planes is a Riemannian (resp. Lorentzian, degenerate) metric.

Now, let ${\mathbb{E}}^{2,1}$ be three dimensional Minkowski space with Lorentzian metric $⟨.,.⟩=x_1{y}_1+x_2{y}_2-x_3{y}_3.$ We identify $\overrightarrow{x}$ and $\overrightarrow{x^t}$ without further comment.

Let $\mathcal{U}$ be an open subset of $\mathbb{C}$. A maximal curve is an analytic function $\vartheta :\mathcal{U}\to {\mathbb{C}}^n$ such that $⟨{\vartheta }^{\prime }\left(\zeta \right),{\vartheta }^{\prime }\left(\zeta \right)⟩=0,$ where $\zeta \in \mathcal{U}$, and ${\vartheta }^{\prime }:=\frac{\partial \vartheta }{\partial \zeta }.$ In addition, if $⟨{\vartheta }^{\prime },\overline{{\vartheta }^{\prime }}⟩={\left|{\vartheta }^{\prime }\right|}^2\ne 0,$ then $\vartheta$ is a regular maximal curve. We then have maximal surfaces in the associated family of a maximal curve, given by the following Weierstrass representation theorem for ZMC (zero mean curvature) surfaces, or maximal surfaces.

Kobayashi [18] found a Weierstrass type representation for space-like conformal maximal surfaces in ${\mathbb{E}}^{2,1}$:

Theorem 1.

Let $g\left(\omega \right)$ be a meromorphic function and let $f\left(\omega \right)$ be a holomorphic function, $f{g}^2$ is analytic, defined on a simply connected open subset $U\subset C$ such that $f\left(\omega \right)$ does not vanish on U except at the poles of $g\left(\omega \right)$. Then,

$$\mathbf{x}(u,v)=\mathrm{Re}{\int }^{\zeta }\left(f\left(1+g^2\right),if\left(1-g^2\right),-2fg\right)d\omega ,\left(\zeta =u+iv\right)$$

(1)

is a space-like conformal immersion with mean curvature identically 0 (i.e., space-like conformal maximal surface). Conversely, any spacelike conformal maximal surface can be described in this manner.

Next, we give some facts about Weierstrass data, and a maximal curve to construct some maximal surfaces.

Definition 1.

A pair of a meromorphic function g and a holomorphic function $f,$$(f,g)$ is called Weierstrass data for a maximal surface.

Lemma 1.

The curve of Enneper of order m:

$${\epsilon }_m\left(\zeta \right)=\left(\zeta +\frac{{\zeta }^{2m+1}}{2m+1},i\left(\zeta -\frac{{\zeta }^{2m+1}}{2m+1}\right),-\frac{2{\zeta }^{m+1}}{m+1}\right)$$

(2)

is a maximal curve, $\zeta \in \mathbb{C}-\left\{0\right\}$, $i=\sqrt{-1}$, $m\ne -1,-1/2.$

Therefore, we have $⟨{\epsilon }_m,{\epsilon }_m⟩=0$ by using (2). Hence, in ${\mathbb{E}}^{2,1}$, the Enneper maximal surface is given by

$${\mathcal{E}}_m\left(u,v\right)=\mathit{Re}\int {\epsilon }_m\left(\zeta \right)d\zeta ,$$

(3)

where $\zeta =u+iv.$$\mathit{Im}\int {\epsilon }_m\left(\zeta \right)d\zeta$ gives the adjoint minimal surface ${\mathcal{E}}_m^{*}\left(u,v\right)$ of the surface ${\mathcal{E}}_m\left(u,v\right)$ in (3). Then, we get the following:

Corollary 1.

The Weierstrass data $\left(1,{\zeta }^m\right)$ of (3) is a representation of the Enneper maximal surface, where integer $m\ge 1.$

Considering the findings above with $\zeta =r{e}^{i\theta }$, we get the following Enneper family of maximal surfaces:

$${\mathcal{E}}_m\left(r,\theta \right)=\left(\begin{array}{c}rcos\left(\theta \right)+\frac{1}{2m+1}{r}^{2m+1}cos\left[\left(2m+1\right)\theta \right]\\ -rsin\left(\theta \right)+\frac{1}{2m+1}{r}^{2m+1}sin\left[\left(2m+1\right)\theta \right]\\ -\frac{2}{m+1}{r}^{m+1}cos\left[\left(m+1\right)\theta \right]\end{array}\right)$$

(4)

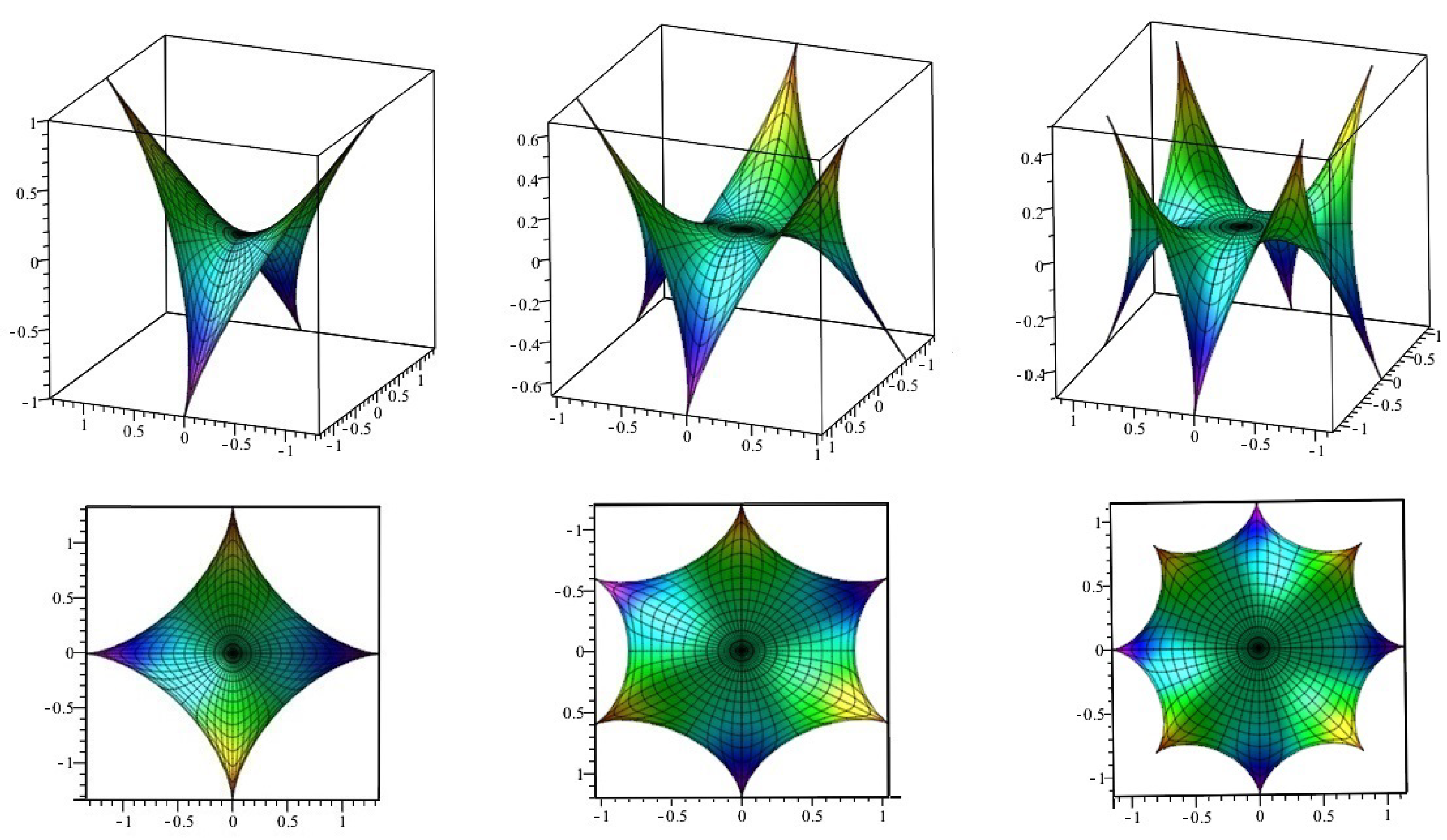
where $m\ne -1,-1/2.$ See Figure 1 for Enneper maximal surfaces ${\mathcal{E}}_1,{\mathcal{E}}_2,{\mathcal{E}}_3$ in $\left(r,\theta \right)$ coordinates.

Figure 1. Enneper maximal surfaces, and its top views (Left): ${\mathcal{E}}_1\left(r,\theta \right)$, (Middle): ${\mathcal{E}}_2\left(r,\theta \right)$, (Right): ${\mathcal{E}}_3\left(r,\theta \right)$.

Hence, using the binomial formula, we obtain more clear representation of ${\mathcal{E}}_m\left(u,v\right)$ in (3):

$$\begin{array}{ccc}x\left(u,v\right)\hfill & =\hfill & \mathit{Re}\left\{u+iv+\frac{1}{2m+1}{\displaystyle \sum _{k=0}^{2m+1}}\left(\begin{array}{c}2m+1\\ k\end{array}\right)u^{2m+1-k}{\left(iv\right)}^k\right\},\hfill \\ \hfill & \hfill & \\ y\left(u,v\right)\hfill & =\hfill & \mathit{Re}\left\{iu-v+\frac{i}{2m+1}{\displaystyle \sum _{k=0}^{2m+1}}\left(\begin{array}{c}2m+1\\ k\end{array}\right)u^{2m+1-k}{\left(iv\right)}^k\right\},\hfill \\ \hfill & \hfill & \\ z\left(u,v\right)\hfill & =\hfill & \mathit{Re}\left\{-\frac{2}{m+1}{\displaystyle \sum _{k=0}^{m+1}}\left(\begin{array}{c}m+1\\ k\end{array}\right)u^{m+1-k}{\left(iv\right)}^k\right\}.\hfill \end{array}$$

(5)

We study surface ${\mathcal{E}}_m\left(u,v\right)$ in $(u,v)$ coordinates for $m=1,2,\dots ,5,$ taking $\zeta =u+iv$ at Cartesian coordinates $x,y,z$, and also in inhomogeneous tangential coordinates $a,b,c,$ by using Weierstrass representation equation.

Next, we give a theorem about maximality of surface ${\mathcal{E}}_1\left(u,v\right)$ (see Figure 1, Left):

Theorem 2.

The surface

$${\mathcal{E}}_1\left(u,v\right)=\left(\begin{array}{c}\frac{1}{3}{u}^3-u{v}^2+u\\ -\frac{1}{3}{v}^3+u^{2}v-v\\ -u^2+v^2\end{array}\right)=\left(\begin{array}{c}x(u,v)\\ y(u,v)\\ z(u,v)\end{array}\right)$$

(6)

which has Weierstrass data $\left(1,\zeta \right)$, is an Enneper maximal surface in ${\mathbb{E}}^{2,1}$.

Proof. 

The coefficients of the first fundamental form of the surface ${\mathcal{E}}_1\left(u,v\right)$ (${\mathcal{E}}_1$, for short) are given by

$$\begin{array}{ccc}\hfill E& =& {\left(\lambda -1\right)}^2=G,\hfill \\ \hfill F& =& 0,\hfill \end{array}$$

where $\lambda =u^2+v^2.$ That is, conformality holds. Then, the Gauss map $e_1(u,v)$ of ${\mathcal{E}}_1$ is as follows

$$e_1=\left(-\frac{2u}{\lambda -1},-\frac{2v}{\lambda -1},\frac{{\lambda }^2+1}{\lambda -1}\right),$$

(7)

where $\lambda \ne 1.$ The coefficients of the second fundamental form of ${\mathcal{E}}_1$ are given by

$$\begin{array}{ccc}\hfill L& =& -\frac{2\left(3\lambda +1\right)}{\lambda -1}=-N,\hfill \\ \hfill M& =& 0.\hfill \end{array}$$

Then, we obtain the following mean curvature and the Gaussian curvature of the surface ${\mathcal{E}}_1$:

$$\begin{array}{ccc}\hfill H& =& 0,\hfill \\ \hfill K& =& \frac{4{\left(3\lambda +1\right)}^2}{{\left(\lambda -1\right)}^6},\hfill \end{array}$$

respectively. Here, $H=⟨\sigma ,\sigma ⟩\frac{EN+GL-2FM}{2\left(EG-F^2\right)},$$K=⟨\sigma ,\sigma ⟩\frac{LN-M}{EG-F^2},$ where $⟨\sigma ,\sigma ⟩=-1.$ Hence, the Enneper surface is maximal surface with positive Gaussian curvature. □

Therefore, we obtain the following parametric equations of the higher order maximal Enneper surfaces ${\mathcal{E}}_m\left(u,v\right)=\left(x(u,v),y(u,v),z(u,v)\right)$ (see Figure 2 Middle for ${\mathcal{E}}_2,$ and Figure 2 Right for ${\mathcal{E}}_3.$):

$$\begin{array}{ccc}\hfill {\mathcal{E}}_2\left(u,v\right)& =& \left(\begin{array}{c}\frac{1}{5}{u}^5-2u^3{v}^2+u{v}^4+u\\ \frac{1}{5}{v}^5-2u^2{v}^3+u^{4}v-v\\ -\frac{2}{3}{u}^3+2u{v}^2\end{array}\right),\hfill \end{array}$$

(8)

$$\begin{array}{ccc}\hfill {\mathcal{E}}_3\left(u,v\right)& =& \left(\begin{array}{c}\frac{1}{7}{u}^7-3u^5{v}^2+5u^3{v}^4-u{v}^6+u\\ -\frac{1}{7}{v}^7+3u^2{v}^5-5u^4{v}^3+u^{6}v-v\\ -\frac{1}{2}{u}^4+3u^2{v}^2-\frac{1}{2}{v}^4\end{array}\right),\hfill \end{array}$$

(9)

$$\begin{array}{ccc}\hfill {\mathcal{E}}_4\left(u,v\right)& =& \left(\begin{array}{c}\frac{1}{9}{u}^9-4u^7{v}^2+14u^5{v}^4-\frac{23}{3}{u}^3{v}^6+u{v}^8+u\\ \frac{1}{9}{v}^9-4u^2{v}^7+14u^4{v}^5-\frac{23}{3}{u}^6{v}^3+u^{8}v-v\\ -\frac{2}{5}{u}^5+4u^3{v}^2-2u{v}^4\end{array}\right),\hfill \end{array}$$

(10)

$$\begin{array}{ccc}\hfill {\mathcal{E}}_5\left(u,v\right)& =& \left(\begin{array}{c}\frac{1}{11}{u}^{11}-5u^9{v}^2+30u^7{v}^4-42u^5{v}^6+15u^3{v}^8+u{v}^{10}+u\\ -\frac{1}{11}{v}^{11}+5u^2{v}^9-30u^4{v}^7+42u^6{v}^5-15u^8{v}^3+u^{10}v-v\\ -\frac{1}{3}{u}^6+5u^4{v}^2-5u^2{v}^4+\frac{1}{3}{v}^6\end{array}\right).\hfill \end{array}$$

(11)

Figure 2. Enneper maximal surfaces (Left): ${\mathcal{E}}_1\left(u,v\right)$, (Middle): ${\mathcal{E}}_2\left(u,v\right)$, (Right): ${\mathcal{E}}_3\left(u,v\right)$.

3. Degree and Class of Enneper Maximal Surfaces

In this section, using some elimination techniques, we derive the irreducible algebraic surface equation, degree and class of Enneper maximal surfaces family ${\mathcal{E}}_m\left(u,v\right)$ for integers $1\le m\le 5$ in three dimensional Minkowski space ${\mathbb{E}}^{2,1}.$

Let us see some basic notions of the surfaces.

Definition 2.

The set of roots of a polynomial $Q(x,y,z)=0$ gives an algebraic surface equation. An algebraic surface $\mathbf{s}$ is said to be of degree $\mathbf{d}$ when $\mathbf{d}=deg\left(\mathbf{s}\right).$

Definition 3.

At a point $(u,v)$ on a surface $\mathbf{s}\left(u,v\right)=(x(u,v),y(u,v),z(u,v)),$ the tangent plane is given by

$$Xx+Yy-Zz+P=0,$$

(12)

where $e=\left(X\right(u,v),Y(u,v),Z(u,v\left)\right)$ is the Gauss map, and $P=P(u,v).$ Then, in inhomogeneous tangential coordinates $a,b,c,$ we have the following surface:

$$\widehat{\mathbf{s}}\left(u,v\right)=\left(a,b,c\right)=\left(X/P,Y/P,Z/P\right).$$

(13)

Therefore, we can obtain an algebraic equation $\widehat{Q}(a,b,c)=0$ of $\widehat{\mathbf{s}}\left(u,v\right)$ in inhomogeneous tangential coordinates.

Definition 4.

The maximum degree of the algebraic equation $\widehat{Q}(a,b,c)=0$ of $\widehat{\mathbf{s}}\left(u,v\right)$ in inhomogeneous tangential coordinates gives the $class$ of $\widehat{\mathbf{s}}\left(u,v\right).$

See [6], for details of a Euclidean case. Hence, we obtain the following findings for degrees and classes of Enneper maximal surfaces that we use:

3.1. Degree

We compute the irreducible algebraic surface equation $Q_1(x,y,z)=0$ (see Figure 3, Left) of Enneper’s maximal surface ${\mathcal{E}}_1\left(u,v\right)$ in (6) by using some elimination techniques. We find the following algebraic equation:

$$\begin{array}{ccc}\hfill Q_1(x,y,z)& =& 64z^9+432x^2{z}^6-432y^2{z}^6-1215x^4{z}^3-6318x^2{y}^2{z}^3+3888x^2{z}^5\hfill \\ & & -1215y^4{z}^3+3888y^2{z}^5-1152z^7+729x^6-2187x^4{y}^2-4374x^4{z}^2\hfill \\ & & +2187x^2{y}^4+6480x^2{z}^4-729y^6+4374y^4{z}^2-6480y^2{z}^4+729x^{4}z\hfill \\ & & -1458x^2{y}^{2}z-3888x^2{z}^3+729y^{4}z-3888y^2{z}^3+5184z^5.\hfill \end{array}$$

(14)

Figure 3. Enneper algebraic maximal surfaces (Left): $Q_1(x,y,z)=0$, (Middle): $Q_2(x,y,z)=0$, (Right): $Q_3(x,y,z)=0$.

Then, its degree number is 9.

Next, we continue our computations to find $Q_m(x,y,z)=0$ for integers $m=2,3.$ We compute the following irreducible algebraic surface equations $Q_2(x,y,z)=0$ (see Figure 3, Middle) and $Q_3(x,y,z)=0$ (see Figure 3, Right) of the surfaces ${\mathcal{E}}_2\left(u,v\right)$ and ${\mathcal{E}}_3\left(u,v\right),$ respectively,

$$\begin{array}{ccc}\hfill Q_2(x,y,z)& =& 847288609443z^{25}-4358480501250x^3{z}^{20}\hfill \\ & & +13075441503750x{y}^2{z}^{20}-131157978046875x^6{z}^{15}\hfill \\ & & -474186536015625x^4{y}^2{z}^{15}+107\mathrm{other}\mathrm{lower}\mathrm{degree}\mathrm{terms},\hfill \end{array}$$

$$\begin{array}{ccc}\hfill Q_3(x,y,z)& =& 2475880078570760549798248448z^{49}\hfill \\ & & +5079604062565768134821675008x^4{z}^{42}\hfill \\ & & -30477624375394608808930050048x^2{y}^2{z}^{42}\hfill \\ & & +5079604062565768134821675008y^4{z}^{42}\hfill \\ & & -633850350654216217766624493568x^8{z}^{35}\hfill \\ & & +446\mathrm{other}\mathrm{lower}\mathrm{degree}\mathrm{terms}.\hfill \end{array}$$

Therefore, $Q_m(x,y,z)=0$ are the algebraic maximal surfaces of the surfaces ${\mathcal{E}}_m\left(u,v\right),$ where $m=2,3,$ and they have degree numbers 25 and $49,$ respectively.

3.2. Class

Now, we introduce the class of the surfaces ${\mathcal{E}}_m\left(u,v\right)$ for integers $1\le m\le 4.$ The case $m=5$, marked with “*” presented in tables of Section 4. Computing the irreducible algebraic surface equations ${\widehat{Q}}_m(a,b,c)=0,$ we obtain the Gauss maps $e_m\left(u,v\right)$ (see Figure 4 for $e_1,e_2,e_3$) for integers $1\le m\le 5$ of the surfaces ${\mathcal{E}}_m\left(u,v\right),$ and we also generalize them as follows:

$$\begin{array}{ccc}\hfill e_1& =& \left(-2\frac{u}{\lambda -1},-2\frac{v}{\lambda -1},\frac{\lambda +1}{\lambda -1}\right),\hfill \end{array}$$

$$\begin{array}{ccc}\hfill e_2& =& \left(-2\frac{u^2-v^2}{{\lambda }^2-1},-2\frac{2uv}{{\lambda }^2-1},\frac{{\lambda }^2+1}{{\lambda }^2-1}\right),\hfill \end{array}$$

(15)

$$\begin{array}{ccc}\hfill e_3& =& \left(-2\frac{u^3-3u{v}^2}{{\lambda }^3-1},-2\frac{3u^{2}v-v^3}{{\lambda }^3-1},\frac{{\lambda }^3+1}{{\lambda }^3-1}\right),\hfill \end{array}$$

(16)

$$\begin{array}{ccc}\hfill e_4& =& \left(-2\frac{u^4-6u^2{v}^2+v^4}{{\lambda }^4-1},-2\frac{4u^{3}v-4u{v}^3}{{\lambda }^4-1},\frac{{\lambda }^4+1}{{\lambda }^4-1}\right),\hfill \end{array}$$

(17)

$$\begin{array}{ccc}\hfill e_5& =& \left(-2\frac{u^5-10u^3{v}^2+5u{v}^4}{{\lambda }^5-1},-2\frac{5u^{4}v-10u^2{v}^3+v^5}{{\lambda }^5-1},\frac{{\lambda }^5+1}{{\lambda }^5-1}\right),\hfill \\ & & ⋮\hfill \end{array}$$

(18)

$$\begin{array}{ccc}\hfill e_m& =& \left(-2\frac{\mathit{Re}\left({\zeta }^m\right)}{{\left|\zeta \right|}^m-1},-2\frac{\mathit{Im}\left({\zeta }^m\right)}{{\left|\zeta \right|}^m-1},\frac{{\left|\zeta \right|}^m+1}{{\left|\zeta \right|}^m-1}\right),\left(\zeta =u+iv,\left|\zeta \right|=\lambda \right).\hfill \end{array}$$

(19)

Figure 4. Top views of the Gauss maps of the surfaces ${\mathcal{E}}_{m=1,2,3}\left(u,v\right)$ (Left): $e_1\left(u,v\right)$, (Middle): $e_2\left(u,v\right)$, (Right): $e_3\left(u,v\right)$.

Using (6), (7), (12) and (13), with $P_1(u,v)=\frac{(\lambda -3)\left(-u^2+v^2\right)}{3\left(\lambda -1\right)},$ we get the following surface ${\widehat{\mathcal{E}}}_1\left(u,v\right)$ (see Figure 5, Left) in inhomogeneous tangential coordinates:

$$a=\frac{6u}{\left(-u^2+v^2\right)(\lambda -3)},b=\frac{6v}{\left(-u^2+v^2\right)(\lambda -3)},c=-\frac{3\left(\lambda +1\right)}{\left(-u^2+v^2\right)(\lambda -3)}.$$

where $\lambda =u^2+v^2,$$\lambda \ne 3,$$u,v\ne 0.$ Therefore, we compute Enneper’s irreducible algebraic maximal surface equation ${\widehat{Q}}_1(a,b,c)=0$ (see Figure 6, Left) of the surface ${\widehat{\mathcal{E}}}_1\left(u,v\right)$:

$$\begin{array}{ccc}\hfill {\widehat{Q}}_1(a,b,c)& =& 4a^6-4a^4{b}^2-3a^4{c}^2-4a^2{b}^4+6a^2{b}^2{c}^2+4b^6-3b^4{c}^2\hfill \\ & & -18a^{4}c+12a^2{c}^3+18b^{4}c-12b^2{c}^3+9a^4+18a^2{b}^2+9b^4.\hfill \end{array}$$

Figure 5. Enneper maximal surfaces in inhomogeneous tangential coordinates (Left): ${\widehat{\mathcal{E}}}_1\left(u,v\right)$, (Middle): ${\widehat{\mathcal{E}}}_2\left(u,v\right)$, (Right): ${\widehat{\mathcal{E}}}_3\left(u,v\right)$.

Figure 6. Enneper’s algebraic maximal surfaces in inhomogeneous tangential coordinates (Left): ${\widehat{Q}}_1(a,b,c)=0$, (Middle): ${\widehat{Q}}_2(a,b,c)=0$, (Right): ${\widehat{Q}}_3(a,b,c)=0$.

So, Enneper’s maximal surface ${\mathcal{E}}_1\left(u,v\right)$ in (6) has class number 6.

Next, we continue our computations to find ${\widehat{Q}}_m$ for integers $2,3,4.$ To find the class of surface ${\mathcal{E}}_2\left(u,v\right)$ (see Figure 5, Middle), we use (9), (12), (13) and (16). Calculating $P_2(u,v)=-\frac{4\left(u^3-3u{v}^2\right)({\lambda }^2-5)}{15\left({\lambda }^2-1\right)},$ we get the following surface ${\widehat{\mathcal{E}}}_2$ inhomogeneous tangential coordinates:

$$a=\frac{15\left(u^2-v^2\right)}{2\left(u^3-3u{v}^2\right)({\lambda }^2-5)},b=\frac{15uv}{\left(u^3-3u{v}^2\right)({\lambda }^2-5)},c=-\frac{15\left({\lambda }^2+1\right)}{4\left(u^3-3u{v}^2\right)({\lambda }^2-5)},$$

where $\lambda =u^2+v^2,$${\lambda }^2\ne 5,$$u,v\ne 0.$ In the inhomogeneous tangential coordinates $a,b,c,$ we find the following irreducible algebraic surface equation ${\widehat{Q}}_2(a,b,c)=0$ (see Figure 6, Middle) of the surface ${\widehat{\mathcal{E}}}_2(u,v)$:

$$\begin{array}{ccc}\hfill {\widehat{Q}}_2(a,b,c)& =& 2176782336a^{16}{b}^4+5804752896a^{14}{b}^6-4837294080a^{14}{b}^4{c}^2\hfill \\ & & +2902376448a^{12}{b}^8-8062156800a^{12}{b}^6{c}^2+120\mathrm{other}\mathrm{lower}\mathrm{degree}\mathrm{terms}.\hfill \end{array}$$

Hence, ${\widehat{Q}}_2(a,b,c)=0$ is the algebraic surface of the surface ${\widehat{\mathcal{E}}}_2(u,v),$ and Enneper’s maximal surface ${\mathcal{E}}_2\left(u,v\right)$ in (8) has class number 20.

Using similar ways, we compute the irreducible algebraic surface equation ${\widehat{Q}}_3(a,b,c)=0$ (see Figure 6, Right) of surface ${\widehat{\mathcal{E}}}_3(u,v)$ (see Figure 5, Right) as follows:

$$\begin{array}{ccc}\hfill {\widehat{Q}}_3(a,b,c)& =& 26623333280885243904a^{42}-718829998583901585408a^{40}{b}^2\hfill \\ & & -104829374793485647872a^{40}{c}^2+6868819986468392927232a^{38}{b}^4\hfill \\ & & +2935222494217598140416a^{38}{b}^2{c}^2+774\mathrm{other}\mathrm{lower}\mathrm{degree}\mathrm{terms}.\hfill \end{array}$$

${\widehat{Q}}_3(a,b,c)=0$ is the algebraic surface of the surface ${\widehat{\mathcal{E}}}_3(u,v),$ and Enneper’s maximal surface ${\mathcal{E}}_3\left(u,v\right)$ in (9) has class number 42. We also compute the following irreducible algebraic surface equation ${\widehat{Q}}_4(a,b,c)=0$ of the surface ${\widehat{\mathcal{E}}}_4(u,v)$:

$$\begin{array}{ccc}\hfill {\widehat{Q}}_4(a,b,c)& =& 42949672960000000000000000000000000000000000000000a^{64}{b}^8\hfill \\ & & -247390116249600000000000000000000000000000000000000a^{62}{b}^8{c}^2\hfill \\ & & -962072674304000000000000000000000000000000000000000a^{60}{b}^{12}\hfill \\ & & +247390116249600000000000000000000000000000000000000a^{60}{b}^{10}{c}^2\hfill \\ & & +667953313873920000000000000000000000000000000000000a^{60}{b}^8{c}^4\hfill \\ & & +2604\mathrm{other}\mathrm{lower}\mathrm{degree}\mathrm{terms}.\hfill \end{array}$$

${\widehat{Q}}_4(a,b,c)=0$ is an algebraic surface of ${\widehat{\mathcal{E}}}_4(u,v),$ and Enneper’s maximal surface ${\mathcal{E}}_4\left(u,v\right)$ in (10) has class number 72.

We obtain the following functions $P_i(u,v),$ where $1\le i\le 6,$

$$\begin{array}{ccc}{P}_1(u,v)=-\frac{\left(u^2-v^2\right)(\lambda -3)}{3\left(\lambda -1\right)},\hfill & \hfill & P_2(u,v)=-\frac{4\left(u^3-3u{v}^2\right)({\lambda }^2-5)}{15\left({\lambda }^2-1\right)},\hfill \\ \hfill & \hfill & \\ P_3(u,v)=-\frac{3\left(u^4-6u^2{v}^2+v^4\right)({\lambda }^3-7)}{14\left({\lambda }^3-1\right)},\hfill & \hfill & P_4(u,v)=-\frac{8\left(u^5-10u^3{v}^2+5u{v}^4\right)\left({\lambda }^4-9\right)}{45\left({\lambda }^4-1\right)},\hfill \\ \hfill & \hfill & \\ P_5(u,v)=-\frac{5\left(u^6-15u^4{v}^2+15u^2{v}^4-v^6\right)({\lambda }^5-11)}{33\left({\lambda }^5-1\right)},\hfill & \hfill & P_6(u,v)=-\frac{12\left(u^7-21u^5{v}^2+35u^3{v}^4-7u{v}^6\right)\left({\lambda }^6-13\right)}{91\left({\lambda }^6-1\right)}.\hfill \\ \hfill & \hfill \end{array}$$

We generalize the above functions, and give the following results:

Corollary 2.

The functions $P_{m\ge 1}$ for integers $m,$ are given by

$$\begin{array}{ccc}\hfill P_{2k-1}& =& -\frac{2\left(2k-1\right)\left[{\lambda }^{2k-1}-\left(2k+1\right)\right]}{(k+1)\left(2k+1\right)\left({\lambda }^{2k-1}-1\right)}\mathit{Re}\left({\zeta }^{2k}\right),\hfill \\ & & \\ \hfill P_{2k}& =& -\frac{4k\left[{\lambda }^{2k}-\left(2k+1\right)\right]}{\left(2k+1\right)\left(4k+1\right)\left({\lambda }^{2k}-1\right)}\mathit{Re}\left({\zeta }^{2k+1}\right),\hfill \end{array}$$

where integers $k\ge 1,$$\zeta =u+iv$ and $\left|\zeta \right|=\lambda .$

So far, we find surfaces ${\widehat{\mathcal{E}}}_1$ and ${\widehat{\mathcal{E}}}_2$. By using ${\mathcal{E}}_3-{\mathcal{E}}_5$, $e_3-e_5$, and also (12), (13), we obtain the following surfaces: ${\widehat{\mathcal{E}}}_m(u,v)=\left(a,b,c\right)$:

$$\begin{array}{ccc}\hfill {\widehat{\mathcal{E}}}_1& =& -\frac{3}{\left(u^2-v^2\right)(\lambda -3)}\left(\begin{array}{c}-2u\\ -2v\\ \lambda +1\end{array}\right),\hfill \\ \hfill {\widehat{\mathcal{E}}}_2& =& -\frac{15}{4\left(u^3-3u{v}^2\right)({\lambda }^2-5)}\left(\begin{array}{c}-2\left(u^2-v^2\right)\\ -4uv\\ {\lambda }^2+1\end{array}\right),\hfill \\ \hfill {\widehat{\mathcal{E}}}_3& =& -\frac{14}{3\left(u^4-6u^2{v}^2+v^4\right)({\lambda }^3-7)}\left(\begin{array}{c}-2\left(u^3-3u{v}^2\right)\\ -2\left(u^{2}v-v^3\right)\\ {\lambda }^3+1\end{array}\right),\hfill \\ \hfill {\widehat{\mathcal{E}}}_4& =& -\frac{45}{8\left(u^5-10u^3{v}^2+5u{v}^4\right)({\lambda }^4-9)}\left(\begin{array}{c}-2\left(u^4-6u^2{v}^2+v^4\right)\\ -2\left(4u^{3}v-4u{v}^3\right)\\ {\lambda }^4+1\end{array}\right),\hfill \\ \hfill {\widehat{\mathcal{E}}}_5& =& -\frac{33}{5\left(u^6-15u^4{v}^2+15u^2{v}^4-v^6\right)({\lambda }^5-11)}\left(\begin{array}{c}-2\left(u^5-10u^3{v}^2+5u{v}^4\right)\\ -2\left(5u^{4}v-10u^2{v}^3+v^5\right)\\ {\lambda }^5+1\end{array}\right).\hfill \end{array}$$

We also generalize the above functions, and find the following results:

Corollary 3.

The surfaces ${\widehat{\mathfrak{S}}}_{m\ge 1}(u,v)$ for integers $m,$ are given by

$$\begin{array}{ccc}\hfill {\widehat{\mathcal{E}}}_{2k-1}(u,v)& =& -\frac{k\left(4k-1\right)}{\left(2k-1\right)\left[{\lambda }^{2k-1}-\left(2k+1\right)\right]\mathit{Re}\left({\zeta }^{2k}\right)}\left(\begin{array}{c}-2\mathit{Re}\left({\zeta }^{2k-1}\right)\\ -2\mathit{Im}\left({\zeta }^{2k-1}\right)\\ {\left|\zeta \right|}^{2k-1}+1\end{array}\right)=\left(\begin{array}{c}a\\ b\\ c\end{array}\right),\hfill \\ & & \\ \hfill {\widehat{\mathcal{E}}}_{2k}(u,v)& =& -\frac{\left(2k+1\right)\left(4k+1\right)}{4k\left[{\lambda }^{2k}-\left(4k+1\right)\right]\mathit{Re}\left({\zeta }^{2k+1}\right)}\left(\begin{array}{c}-2\mathit{Re}\left({\zeta }^{2k}\right)\\ -2\mathit{Im}\left({\zeta }^{2k}\right)\\ {\left|\zeta \right|}^{2k}+1\end{array}\right)=\left(\begin{array}{c}a\\ b\\ c\end{array}\right),\hfill \end{array}$$

where integers $k\ge 1,$$\zeta =u+iv$ and $\left|\zeta \right|=\lambda .$

Corollary 4.

In ${\mathbb{E}}^{2,1},$ the relations between the Enneper maximal surface ${\widehat{\mathcal{E}}}_{m\ge 1}(u,v)$ in the inhomogeneous tangential coordinates and the Gauss map $e_{m\ge 1}(u,v)$ of the Enneper maximal surface ${\mathcal{E}}_{m\ge 1}(u,v)$ in the cartesian coordinates are given by

$$\begin{array}{ccc}\hfill {\widehat{\mathcal{E}}}_{2k-1}(u,v)& =& -\frac{k\left(4k-1\right)\left({\lambda }^{2k-1}-1\right)}{\left(2k-1\right)\left[{\lambda }^{2k-1}-\left(2k+1\right)\right]\mathit{Re}\left({\zeta }^{2k}\right)}{e}_{2k-1}(u,v),\hfill \\ & & \\ \hfill {\widehat{\mathcal{E}}}_{2k}(u,v)& =& -\frac{\left(2k+1\right)\left(4k+1\right)\left({\lambda }^{2k}-1\right)}{4k\left[{\lambda }^{2k}-\left(2k+1\right)\right]\mathit{Re}\left({\zeta }^{2k+1}\right)}{e}_{2k}(u,v),\hfill \\ & \end{array}$$

where integers $k\ge 1,$$\zeta =u+iv$ and $\left|\zeta \right|=\lambda .$

4. Conclusions

To reveal the irreducible algebraic surface equations of the Enneper maximal surfaces ${\mathcal{E}}_m\left(u,v\right)$ in ${\mathbb{E}}^{2,1},$ we have tried a series of standard techniques in elimination theory: only Sylvester by hand for $Q_1(x,y,z)=0$, and then projective (Macaulay) and sparse multivariate resultants implemented in the Maple software [19] package multires for $Q_m(x,y,z)=0$ and ${\widehat{Q}}_m(a,b,c)=0$.

Maple’s native implicitization command Implicitize, and implicitization based on Maple’s native implementation of the Groebner Basis. For the latter, we implemented in Maple the method in [20] (Chapter 3, p. 128). Under reasonable time, we only succeed for $m=1,2$ in all above methods.

For $m=3$, the successful method we have tried was to compute the equation defining the elimination ideal using the Groebner Basis package FGb of Faugère in [21].

The time required to output the irreducible algebraic surface equations $Q_m(x,y,z)=0$ (resp. ${\widehat{Q}}_m(a,b,c)=0$) for integers $1\le m\le 3$ and polynomials defining the elimination ideal was under reasonable seconds determined by the following Table 1 (resp. Table 2).

Table 1. Results for the Enneper algebraic maximal surfaces $Q_m(x,y,z)=0$.

Algebraic	Degree	Number	Gröbner	FGb
Surface	of Surface	of Terms	Time (s)	Time (s)
$Q_1$	9	23	0.266	0.041
$Q_2$	25	112	321.953	0.835
$Q_3$	49	451	∗	266.854
$Q_4$	81	∗	∗	∗
⋮	⋮	⋮	⋮	⋮
$Q_m$	${\left(2m+1\right)}^2$	∗	∗	∗

Table 2. Results for the Enneper algebraic maximal surfaces ${\widehat{Q}}_m(a,b,c)=0$.

Algebraic	Class	Number	Gröbner	FGb
Surface	of Surface	of Terms	Time (s)	Time (s)
${\widehat{Q}}_1$	6	14	0.94	0.030
${\widehat{Q}}_2$	20	125	61.152	0.114
${\widehat{Q}}_3$	42	779	∗	125.904
${\widehat{Q}}_4$	72	2609	∗	1306.718
${\widehat{Q}}_5$	110	∗	∗	∗
⋮	⋮	⋮	⋮	⋮
${\widehat{Q}}_m$	$2\mathit{m}\left(2m+1\right)$	∗	∗	∗

For the degree (resp. class) of the irreducible algebraic surface equation $Q_4(x,y,z)=0$ (resp. ${\widehat{Q}}_5(a,b,c)=0$) of the surface ${\mathcal{E}}_4\left(u,v\right)$ (resp. ${\widehat{\mathcal{E}}}_5(u,v)$), marked with “∗” in Table 1 (resp. Table 2), was rejected (i.e., “out of memory”) by Maple 17 on a laptop Pentium Core i5-4310M 2.00 GHz, 4 GB RAM, with the time given in CPU seconds.

Hence, we propose the following:

Proposition 1.

For integers $m\ge 1$, degree number of the irreducible algebraic surfaces $Q_m(x,y,z)=0$ in the Cartesian coordinates is of ${\left(2m+1\right)}^2,$ and class number of irreducible algebraic surfaces ${\widehat{Q}}_m(a,b,c)=0$ in inhomogeneous tangential coordinates is of $2m\left(2m+1\right)$ of the $(1,{\zeta }^m)$-type real Enneper maximal surfaces ${\mathcal{E}}_m\left(u,v\right)$.

Open Problems

Here, we give some problems that we could not find the answers in this paper:

Problem 1.

Find the irreducible Enneper algebraic maximal surface eq. $Q_{m\ge 4}(x,y,z)=0$ in the cartesian coordinates by using the parametric equation of the Enneper maximal surface ${\mathcal{E}}_{m\ge 4}\left(u,v\right)$.

Problem 2.

Find the irreducible Enneper algebraic maximal surface eq. ${\widehat{Q}}_{m\ge 5}(a,b,c)=0$ in the inhomogeneous tangential coordinates by using the parametric equation of the Enneper maximal surface ${\widehat{\mathcal{E}}}_{m\ge 5}(u,v)$.

Finally, we give all findings in Table 1 and Table 2.