A Simple Frequency Formulation for the Tangent Oscillator
Abstract
:1. Introduction
2. The Frequency Formulation
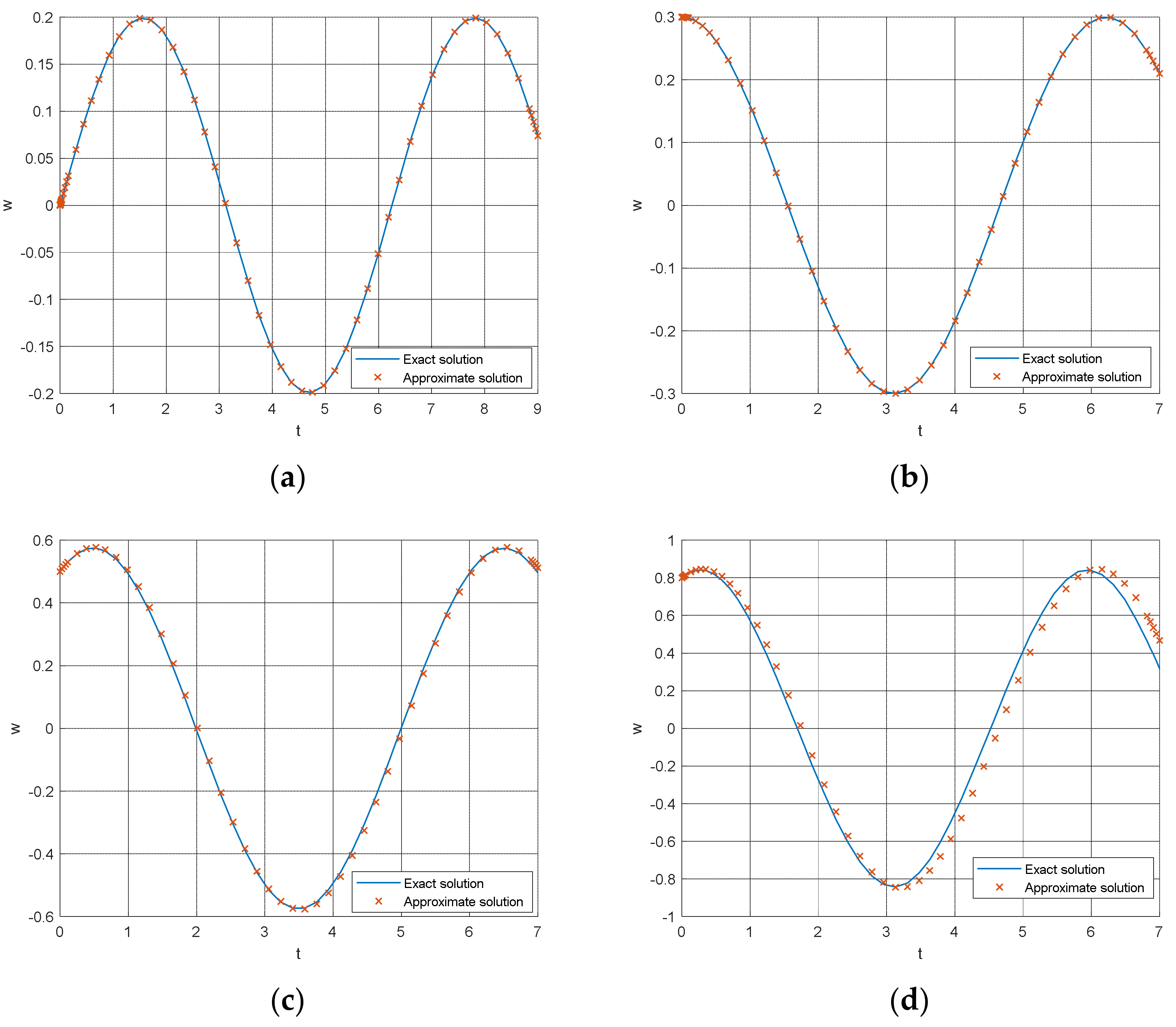
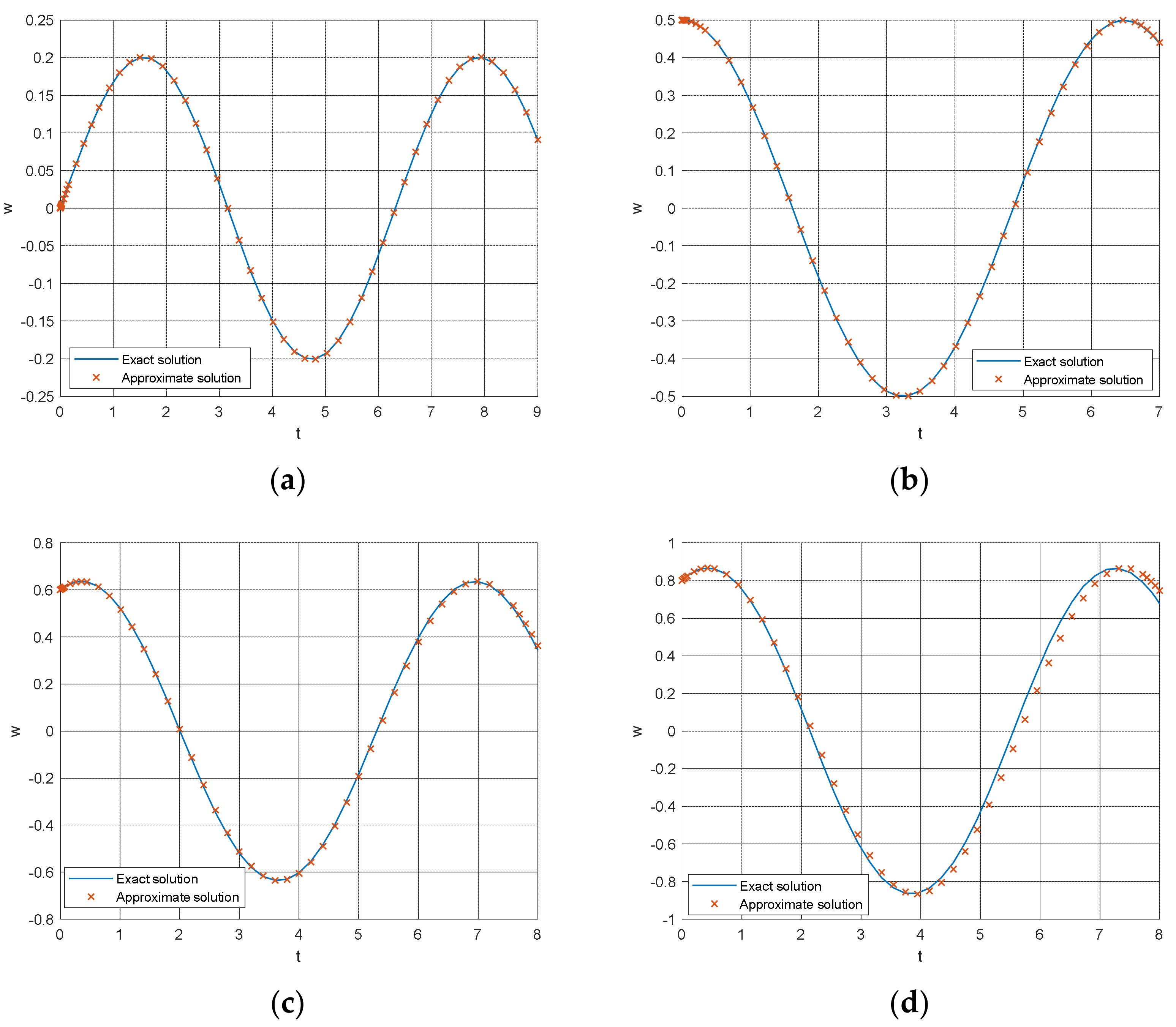
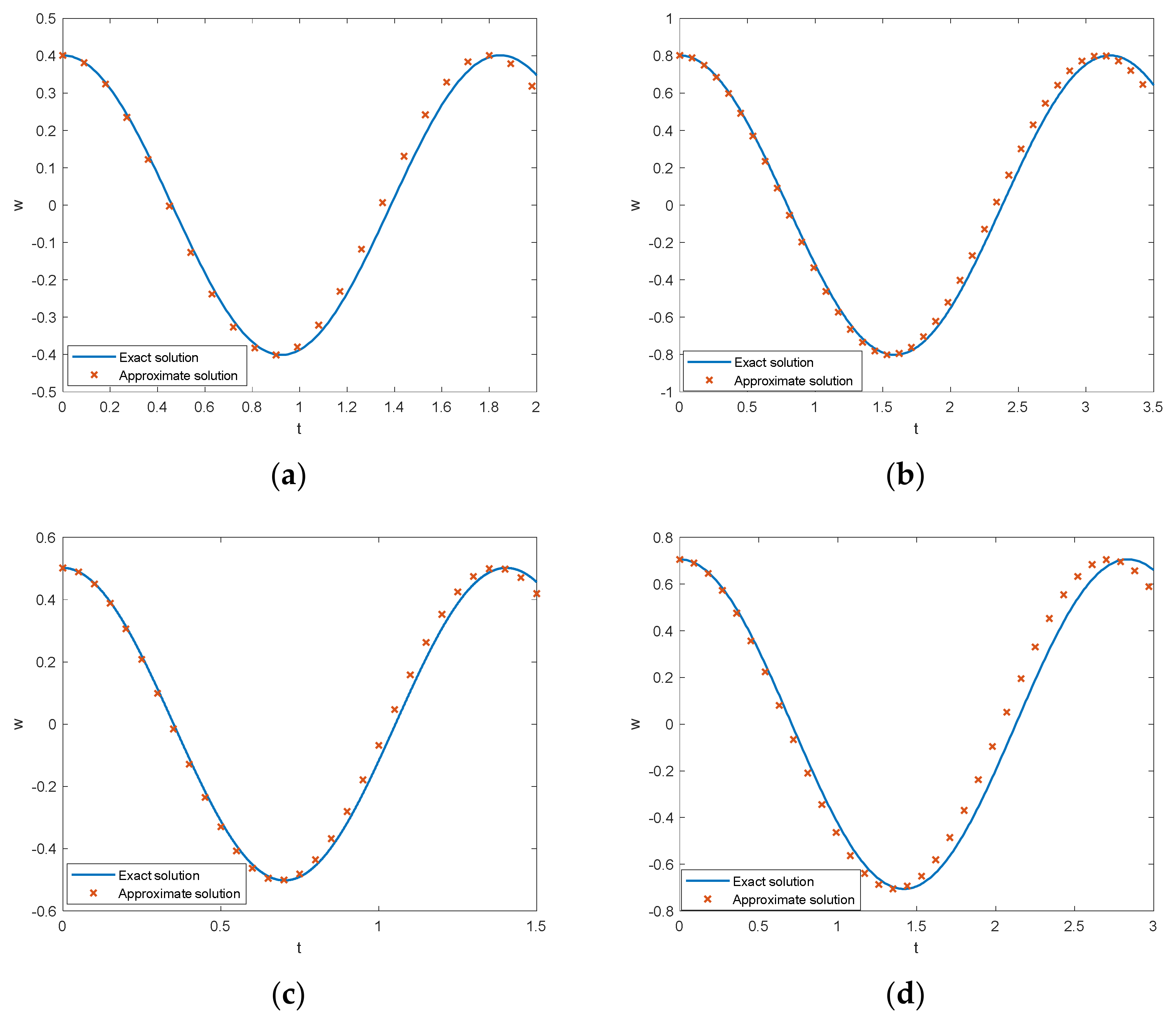
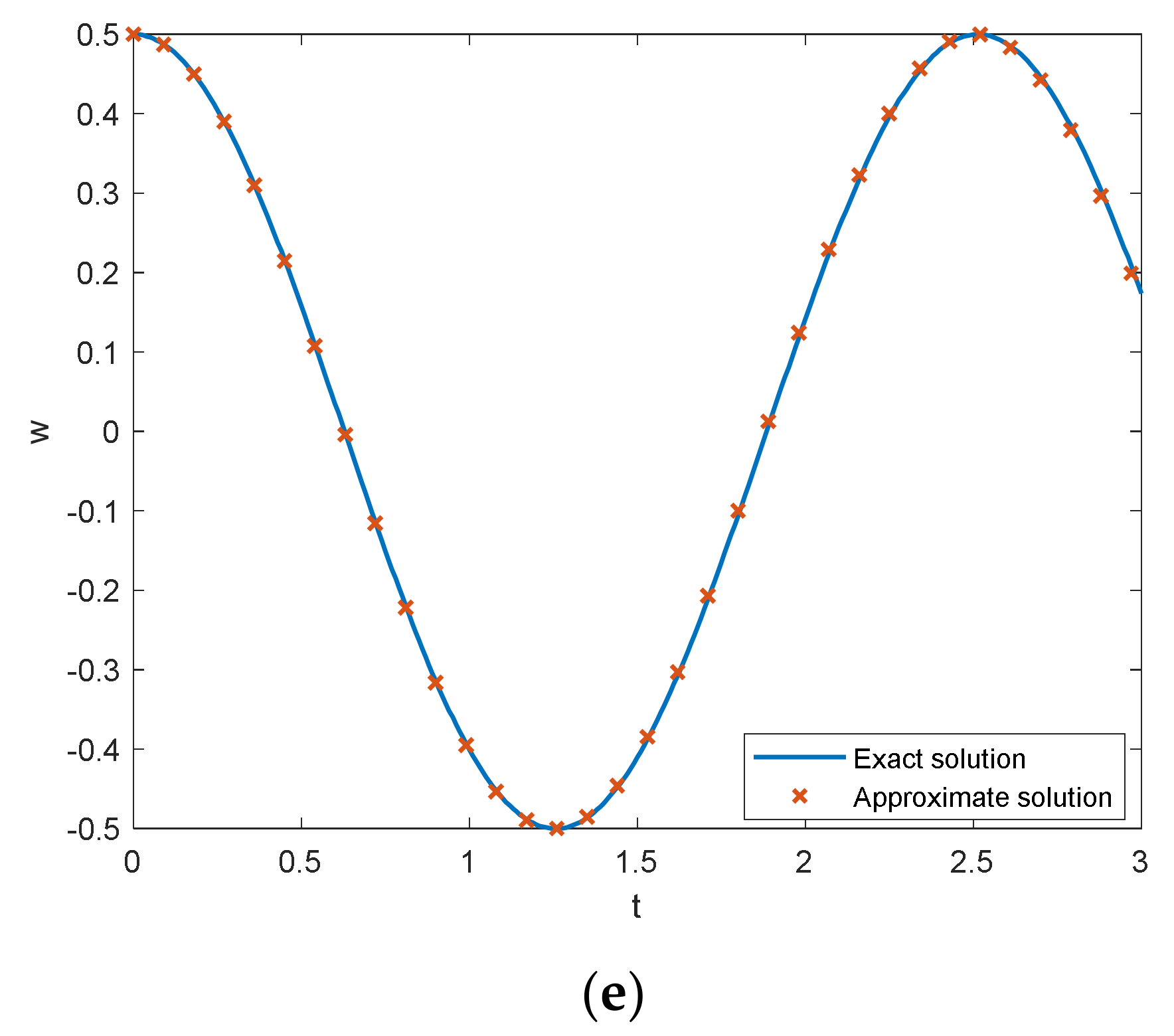
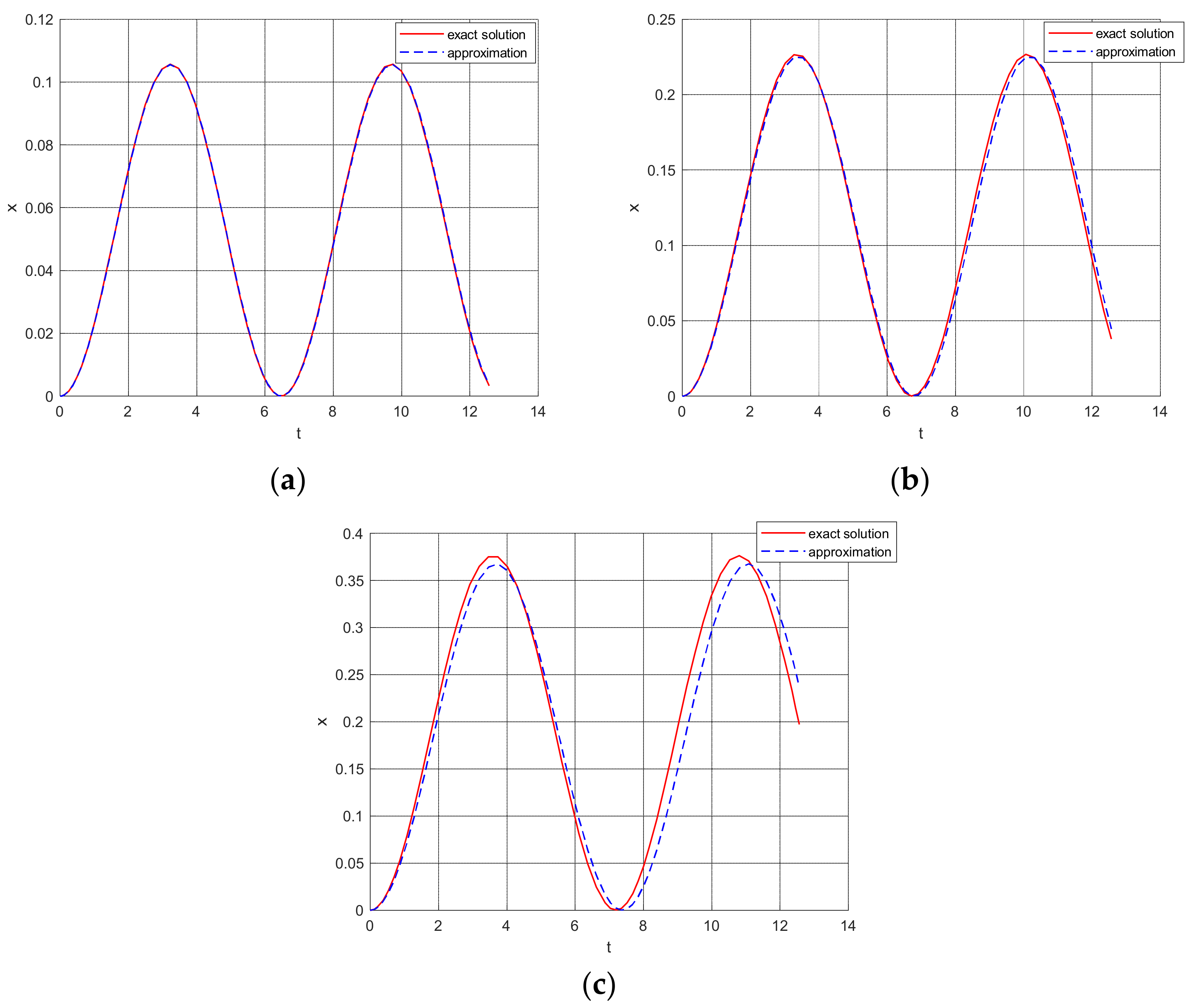
3. Tangent Oscillator
4. Hyperbolic Tangent Oscillator
5. Singular Oscillator
6. MEMS Oscillator
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Song, H.Y. A modification of homotopy perturbation method for a hyperbolic tangent oscillator arising in nonlinear packaging system. J. Low Freq. Noise Vib. Active Control 2019, 38, 914–917. [Google Scholar] [CrossRef] [Green Version]
- Song, H.Y. A thermodynamics model for a packing dynamical system. Therm. Sci. 2020, 24, 2331–2335. [Google Scholar] [CrossRef]
- Kuang, W.; Wang, J.; Huang, C.; Lu, L.; Gao, D.; Wang, Z.; Ge, C. Homotopy perturbation method with an auxiliary term for the optimal design of a tangent nonlinear packaging system. J. Low Freq. Noise Vib. Active Control 2019, 38, 1075–1080. [Google Scholar] [CrossRef]
- Ji, Q.P.; Wang, J.; Lu, L.X.; Ge, C.F. Li-He’s modified homotopy perturbation method coupled with the energy method for the dropping shock response of a tangent nonlinear packaging system. J. Low Freq. Noise Vib. Active Control 2021, 40, 675–682. [Google Scholar] [CrossRef]
- Clementi, F.; Gazzani, V.; Poiani, M.; Lenci, S. Assessment of seismic behaviour of heritage masonry buildings using numerical modelling. J. Build. Eng. 2016, 8, 29–47. [Google Scholar] [CrossRef]
- Dertimanis, V.K.; Chatzi, E.N.; Masri, S.F. On the Active Vibration Control of Nonlinear Uncertain Structures. J. Appl. Comp. Mech. 2021, 7, 1183–1197. [Google Scholar]
- El-Dib, Y.O.; Matoog, R.T. The Rank Upgrading Technique for a Harmonic Restoring Force of Nonlinear Oscillators. J. Appl. Comp. Mech. 2021, 7, 782–789. [Google Scholar]
- Anjum, N.; He, J.H.; Ain, Q.T.; Tian, D. Li-He’s modified homotopy perturbation method for doubly-clamped electrically actuated microbeams-based microelectromechanical system. Facta Univ. Mech. Eng. 2021. [Google Scholar] [CrossRef]
- Nawaz, Y.; Arif, M.S.; Bibi, M.; Naz, M.; Fayyaz, R. An effective modification of He’s variational approach to a nonlinear oscillator. J. Low Freq. Noise Vib. Active Control 2019, 38, 1013–1022. [Google Scholar] [CrossRef]
- Ganji, D.D.; Gorji, M.; Soleimani, S.; Esmaeilpour, M. Solution of nonlinear cubic-quintic Duffing oscillators using He’s Energy Balance Method. J. Zhejiang Univ.-Sci. A 2009, 10, 1263–1268. [Google Scholar] [CrossRef]
- Ganji, S.S.; Barari, A.; Karimpour, S.; Domairry, G. Motion of a rigid rod rocking back and forth and cubic-quitic Duffing oscillators. J. Theor. Appl. Mech. 2012, 50, 215–229. [Google Scholar]
- Beléndez, A.; Beléndez, T.; Martínez, F.J.; Pascual, C.; Alvarez, M.L.; Arribas, E. Exact solution for the unforced Duffing oscillator with cubic and quintic nonlinearities. Nonlinear Dyn. 2016, 86, 1687–1700. [Google Scholar] [CrossRef] [Green Version]
- Suleman, M.; Wu, Q.B. Comparative Solution of Nonlinear Quintic Cubic Oscillator Using Modified Homotopy Perturbation Method. Adv. Math. Phys. 2015, 932905. [Google Scholar] [CrossRef]
- Razzak, M.A. An analytical approximate technique for solving cubic-quintic Duffing oscillator. Alex. Eng. J. 2016, 55, 2959–2965. [Google Scholar] [CrossRef] [Green Version]
- Wang, K.J.; Wang, G.D. Gamma function method for the nonlinear cubic-quintic Duffing oscillators. J. Low Freq. Noise Vib. Active Control 2021. [Google Scholar] [CrossRef]
- Wang, K.J. On new abundant exact traveling wave solutions to the local fractional Gardner equation defined on Cantor sets. Math. Methods Appl. Sci. 2021. [Google Scholar] [CrossRef]
- Wang, K.J. Generalized variational principle and periodic wave solution to the modified equal width-Burgers equation in nonlinear dispersion media. Phys. Lett. A 2021, 419, 127723. [Google Scholar] [CrossRef]
- Wang, K.J.; Zhang, P.L. Investigation of the periodic solution of the time-space fractional Sasa-Satsuma equation arising in the monomode optical fibers. EPL 2021. [Google Scholar] [CrossRef]
- He, J.H. The simpler, the better: Analytical methods for nonlinear oscillators and fractional oscillators. J. Low Freq. Noise Vib. Active Control 2019, 38, 1252–1260. [Google Scholar] [CrossRef] [Green Version]
- Qie, N.; Hou, W.F.; He, J.H. The fastest insight into the large amplitude vibration of a string. Rep. Mechan. Eng. 2020, 2, 1–5. [Google Scholar] [CrossRef]
- Feng, G.Q. He’s frequency formula to fractal undamped Duffing equation. J. Low Freq. Noise Vib. Active Control 2021. [Google Scholar] [CrossRef]
- Liu, C.X. A short remark on He’s frequency formulation. J. Low Freq. Noise Vib. Active Control 2021, 40, 672–674. [Google Scholar] [CrossRef]
- Liu, C.X. Periodic solution of fractal Phi-4 equation. Therm. Sci. 2021, 25, 1345–1350. [Google Scholar] [CrossRef]
- Elías-Zúñiga, A.; Palacios-Pineda, L.M.; Jiménez-Cedeño, I.H.; Martínez-Romero, O.; Trejo, D.O. He’s frequency-amplitude formulation for nonlinear oscillators using Jacobi elliptic functions. J. Low Freq. Noise Vib. Active Control 2020, 39, 1216–1223. [Google Scholar] [CrossRef]
- Elías-Zúñiga, A.; Palacios-Pineda, L.M.; Jiménez-Cedeño, I.H.; Martínez-Romero, O.; Olvera-Trejo, D. Enhanced He’s frequency-amplitude formulation for nonlinear oscillators. Results Phys. 2020, 19, 103626. [Google Scholar] [CrossRef]
- Wu, Y.; Liu, Y.P. Residual calculation in He’s frequency-amplitude formulation. J. Low Freq. Noise Vib. Active Control 2021, 40, 1040–1047. [Google Scholar] [CrossRef] [Green Version]
- Zuo, Y.T. A gecko-like fractal receptor of a three-dimensional printing technology: A fractal oscillator. J. Math. Chem. 2021, 59, 735–744. [Google Scholar] [CrossRef]
- He, C.H. An introduction to an ancient Chinese algorithm and its modification. Int. J. Numer. Methods Heat Fluid Flow 2016, 26, 2486–2491. [Google Scholar] [CrossRef]
- Khan, W.A. Numerical simulation of Chun-Hui He’s iteration method with applications in engineering. Int. J. Numer. Methods Heat Fluid Flow 2021. [Google Scholar] [CrossRef]
- Sedighi, H.M.; Shirazi, K.H.; Attarzadeh, M.A. A study on the quintic nonlinear beam vibrations using asymptotic approximate approaches. Acta Astronaut. 2013, 91, 245–250. [Google Scholar] [CrossRef]
- Sedighi, H.M. Size-dependent dynamic pull-in instability of vibrating electrically actuated microbeams based on the strain gradient elasticity theory. Acta Astronaut. 2014, 95, 111–123. [Google Scholar] [CrossRef]
- Sedighi, H.M.; Keivani, M.; Abadyan, M. Modified continuum model for stability analysis of asymmetric FGM double-sided NEMS: Corrections due to finite conductivity, surface energy and nonlocal effect. Comp. Part B-Eng. 2015, 83, 117–133. [Google Scholar] [CrossRef]
- Zhang, Y.N.; Tian, D.; Pang, J. A fast estimation of the frequency property of the microelectromechanical system oscillator. J. Low Freq. Noise Vib. Active Control 2021. [Google Scholar] [CrossRef]
- Wang, K.L.; Wei, C.F. A powerful and simple frequency formula to nonlinear fractal oscillators. J. Low Freq. Noise Vib. Active Control. 2021, 40, 1373–1379. [Google Scholar] [CrossRef]
- El-Dib, Y.O. The frequency estimation for non-conservative nonlinear oscillation. ZAMM-Z. Angew. Math. Mech. 2021. [Google Scholar] [CrossRef]
- Wang, K.J. A fast insight into the nonlinear oscillation of nano-electro mechanical resonators considering the size effect and the van der Waals force. EPL Lett. J. Explor. Front. Phys. 2021. [Google Scholar] [CrossRef]
- Popov, M. Friction under Large-Amplitude Normal Oscillations. Facta Univ. Ser. Mech. Eng. 2021, 19, 105–113. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, J.-H.; Yang, Q.; He, C.-H.; Khan, Y. A Simple Frequency Formulation for the Tangent Oscillator. Axioms 2021, 10, 320. https://doi.org/10.3390/axioms10040320
He J-H, Yang Q, He C-H, Khan Y. A Simple Frequency Formulation for the Tangent Oscillator. Axioms. 2021; 10(4):320. https://doi.org/10.3390/axioms10040320
Chicago/Turabian StyleHe, Ji-Huan, Qian Yang, Chun-Hui He, and Yasir Khan. 2021. "A Simple Frequency Formulation for the Tangent Oscillator" Axioms 10, no. 4: 320. https://doi.org/10.3390/axioms10040320
APA StyleHe, J.-H., Yang, Q., He, C.-H., & Khan, Y. (2021). A Simple Frequency Formulation for the Tangent Oscillator. Axioms, 10(4), 320. https://doi.org/10.3390/axioms10040320






