Generalized Hypergeometric Function 3F2 Ratios and Branched Continued Fraction Expansions
Abstract
:1. Introduction
2. Main Results
2.1. Recurrence Relations
2.2. Expansions
2.3. Convergence
- (a)
- and replaced by ;
- (b)
- (or ) and (or ), replaced by (or ), respectively;
- (c)
- (or ) and (or ), replaced by (or ), respectively.
3. Numerical Experiments
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Abd-Rabo, M.A.; Zakarya, M.; Cesarano, C.; Aly, S. Bifurcation analysis of time-delay model of consumer with the advertising effect. Symmetry 2021, 13, 417. [Google Scholar] [CrossRef]
- AlNemer, G.; Kenawy, M.; Zakarya, M.; Cesarano, C.; Rezk, H.M. Generalizations of Hardy’s type inequalities via conformable calculus. Symmetry 2021, 13, 242. [Google Scholar] [CrossRef]
- Duan, S.; Song, W.; Zio, E.; Cattani, C.; Li, M. Product technical life prediction based on multi-modes and fractional Levy stable motion. Mech. Syst. Signal Process. 2021, 161, 107984. [Google Scholar] [CrossRef]
- Elayaraja, R.; Ganesan, V.; Bazighifan, O.; Cesarano, C. Oscillation and asymptotic properties of differential equations of third-order. Axioms 2021, 10, 192. [Google Scholar] [CrossRef]
- Mohammad, M.; Trounev, A.; Cattani, C. The dynamics of COVID-19 in the UAE based on fractional derivative modeling using Riesz wavelets simulation. Adv. Differ. Equ. 2021, 2021, 115. [Google Scholar] [CrossRef]
- Wojtowicz, M.; Bodnar, D.; Shevchuk, R.; Bodnar, O.; Bilanyk, I. The Monte Carlo type method of attack on the RSA cryptosystem. In Proceedings of the 10th International Conference on Advanced Computer Information Technologies, Institute of Applied Informatics of Deggendorf Institute of Technology, Deggendorf, Germany, 13–15 May 2020; Institute of Electrical and Electronics Engineers Inc.: Deggendorf, Germany, 2020; pp. 755–758. [Google Scholar]
- Baranetskij, Y.O.; Demkiv, I.I.; Kopach, M.I.; Solomko, A.V. Interpolational (L,M)-rational integral fraction on a continual set of nodes. Carpathian Math. Publ. 2021, 13, 587–591. [Google Scholar]
- Cuyt, A.A.M.; Petersen, V.; Verdonk, B.; Waadeland, H.; Jones, W.B. Handbook of Continued Fractions for Special Functions; Springer: Dordrecht, The Netherlands, 2008. [Google Scholar]
- Lascu, D.; Sebe, G.I. A Gauss–Kuzmin–Lévy theorem for Rényi-type continued fractions. Acta Arith. 2020, 193, 283–292. [Google Scholar] [CrossRef]
- Lascu, D.; Sebe, G.I. A Lochs-type approach via entropy in comparing the efficiency of different continued fraction algorithms. Mathematics 2021, 9, 255. [Google Scholar] [CrossRef]
- Lima, H.; Loureiro, A. Multiple orthogonal polynomials associated with confluent hypergeometric functions. J. Approx. Theory 2020, 260, 105484. [Google Scholar] [CrossRef]
- Jones, W.B.; Thron, W.J. Continued Fractions: Analytic Theory and Applications; Addison-Wesley Pub. Co.: Reading, MA, USA, 1980. [Google Scholar]
- Khrushchev, S. Orthogonal Polynomials and Continued Fractions; Cambridge University Press: New York, NY, USA, 2008. [Google Scholar]
- Lorentzen, L.; Waadeland, H. Continued Fractions—Volume 1: Convergence Theory, 2nd ed.; Atlantis Press: Amsterdam, The Netherlands, 2008. [Google Scholar]
- Sebe, G.I.; Lascu, D. Convergence rate for Rényi-type continued fraction expansions. Period. Math. Hung. 2020, 81, 239–249. [Google Scholar] [CrossRef] [Green Version]
- Wall, H.S. Analytic Theory of Continued Fractions; D. Van Nostrand Co.: New York, NY, USA, 1948. [Google Scholar]
- Zou, L.; Song, L.; Wang, X.; Chen, Y.; Zhang, C.; Tang, C. Bivariate Thiele-like rational interpolation continued fractions with parameters based on virtual points. Mathematics 2020, 8, 71. [Google Scholar] [CrossRef] [Green Version]
- Bodnar, D.I. Branched Continued Fractions; Naukova Dumka: Kyiv, Ukraine, 1986. (In Russian) [Google Scholar]
- Bodnar, D.I.; Zators’kyi, R.A. Generalization of continued fractions. I. J. Math. Sci. 2012, 183, 54–64. [Google Scholar] [CrossRef]
- Bodnar, D.I.; Zators’kyi, R.A. Generalization of continued fractions. II. J. Math. Sci. 2012, 184, 45–55. [Google Scholar] [CrossRef]
- Bodnarchuk, P.I.; Skorobogatko, V.Y. Branched Continued Fractions and Their Applications; Naukova Dumka: Kyiv, Ukraine, 1974. (In Ukrainian) [Google Scholar]
- Cuyt, A. A review of multivariate Padé approximation theory. J. Comput. Appl. Math. 1985, 12–13, 221–232. [Google Scholar] [CrossRef] [Green Version]
- Cuyt, A.; Verdonk, B. A review of branched continued fraction theory for the construction of multivariate rational approximants. Appl. Numer. Math. 1988, 4, 263–271. [Google Scholar] [CrossRef]
- Murphy, J.A.; O’Donohoe, M.R. A two-variable generalization of the Stieltjes-type continued fraction. J. Comput. Appl. Math. 1978, 4, 181–190. [Google Scholar] [CrossRef] [Green Version]
- Petreolle, M.; Sokal, A.D. Lattice paths and branched continued fractions II. Multivariate Lah polynomials and Lah symmetric functions. Eur. J. Combin. 2021, 92, 103235. [Google Scholar] [CrossRef]
- Petreolle, M.; Sokal, A.D.; Zhu, B.X. Lattice paths and branched continued fractions: An infinite sequence of generalizations of the Stieltjes-Rogers and Thron-Rogers polynomials, with coefficientwise Hankel-total positivity. arXiv 2020, arXiv:1807.03271v2. [Google Scholar]
- Siemaszko, W. Branched continued fractions for double power series. J. Comput. Appl. Math. 1980, 6, 121–125. [Google Scholar] [CrossRef] [Green Version]
- Skorobogatko, V.Y. Theory of Branched Continued Fractions and Its Applications in Computational Mathematics; Nauka: Moscow, Russia, 1983. (In Russian) [Google Scholar]
- Gauss, C.F. Disquisitiones generales circa seriem infinitam etc. In Commentationes Societatis Regiae Scientiarum Gottingensis Recentiores; Classis Mathematicae, 1812; H. Dieterich: Gottingae, Germany, 1813; Volume 2, pp. 3–46. [Google Scholar]
- Bodnar, D.I. Expansion of a ratio of hypergeometric functions of two variables in branching continued fractions. J. Math. Sci. 1993, 64, 1155–1158. [Google Scholar] [CrossRef]
- Bodnar, D.I.; Manzii, O.S. Expansion of the ratio of Appel hypergeometric functions F3 into a branching continued fraction and its limit behavior. J. Math. Sci. 2001, 107, 3550–3554. [Google Scholar] [CrossRef]
- Bodnar, D.I. Multidimensional C-Fractions. J. Math. Sci. 1998, 90, 2352–2359. [Google Scholar] [CrossRef]
- Bodnar, D.I.; Hoyenko, N.P. Approximation of the ratio of Lauricella functions by a branched continued fraction. Mat. Studii 2003, 20, 210–214. [Google Scholar]
- Hoyenko, N.; Hladun, V.; Manzij, O. On the infinite remains of the Nórlund branched continued fraction for Appell hypergeometric functions. Carpathian Math. Publ. 2014, 6, 11–25. (In Ukrainian) [Google Scholar] [CrossRef] [Green Version]
- Hoyenko, N.; Antonova, T.; Rakintsev, S. Approximation for ratios of Lauricella–Saran fuctions FS with real parameters by a branched continued fractions. Math. Bul. Shevchenko Sci. Soc. 2011, 8, 28–42. (In Ukrainian) [Google Scholar]
- Antonova, T.; Dmytryshyn, R.; Kravtsiv, V. Branched continued fraction expansions of Horn’s hypergeometric function H3 ratios. Mathematics 2021, 9, 148. [Google Scholar] [CrossRef]
- Bailey, W.N. Generalised Hypergeometric Series; Cambridge University Press: Cambridge, UK, 1935. [Google Scholar]
- Herschel, J.F.W. A Collection of Examples of the Applications of the Calculus of Finite Differences; Printed by J. Smith and sold by J. Deighton & Sons: Cambridge, UK, 1820. [Google Scholar]
- Antonova, T.M. On convergence criteria for branched continued fraction. Carpathian Math. Publ. 2020, 12, 157–164. [Google Scholar] [CrossRef]
- Bodnar, D.I.; Bilanyk, I.B. On the convergence of branched continued fractions of a special form in angular domains. J. Math. Sci. 2020, 246, 188–200. [Google Scholar] [CrossRef]
- Bodnar, D.I.; Bilanyk, I.B. Parabolic convergence regions of branched continued fractions of the special form. Carpathian Math. Publ. 2021, 13, 619–630. [Google Scholar]
- Bodnar, D.I.; Dmytryshyn, R.I. Multidimensional associated fractions with independent variables and multiple power series. Ukr. Math. J. 2019, 71, 370–386. [Google Scholar] [CrossRef]
- Bilanyk, I.B.; Bodnar, D.I.; Buyak, L.M. Representation of a quotient of solutions of a four-term linear recurrence relation in the form of a branched continued fraction. Carpathian Math. Publ. 2019, 11, 33–41. [Google Scholar] [CrossRef]
- Dmytryshyn, R.I. Convergence of multidimensional A- and J-fractions with independent variables. Comput. Methods Funct. Theory 2021. [Google Scholar] [CrossRef]
- Dmytryshyn, R.I. On some of convergence domains of multidimensional S-fractions with independent variables. Carpathian Math. Publ. 2019, 11, 54–58. [Google Scholar] [CrossRef]
- Antonova, T.M.; Dmytryshyn, R.I. Truncation error bounds for branched continued fraction . Ukr. Math. J. 2020, 72, 1018–1029. [Google Scholar] [CrossRef]
- Antonova, T.M.; Dmytryshyn, R.I. Truncation error bounds for branched continued fraction whose partial denominators are equal to unity. Mat. Stud. 2020, 54, 3–14. [Google Scholar]
- Bodnar, O.S.; Dmytryshyn, R.I.; Sharyn, S.V. On the convergence of multidimensional S-fractions with independent variables. Carpathian Math. Publ. 2020, 12, 353–359. [Google Scholar] [CrossRef]
- Bodnar, D.I.; Bilanyk, I.B. Estimates of the rate of pointwise and uniform convergence for branched continued fractions with nonequivalent variables. Mat. Method. Fiz. Mech. Polya 2019, 6, 72–82. (In Ukrainian) [Google Scholar]
- Bilanyk, I.B. A truncation error bound for some branched continued fractions of the special form. Mat. Stud. 2019, 52, 115–123. [Google Scholar] [CrossRef]
- Antonova, T.M. Multidimensional generalization of the theorem on parabolic domains of convergence of continued fractions. Mat. Met. Fiz.-Mekh. Polya. 1999, 42, 7–12. (In Ukrainian) [Google Scholar]
- Dmytryshyn, R.I. On the expansion of some functions in a two-dimensional g-fraction independent variables. J. Math. Sci. 2012, 181, 320–327. [Google Scholar] [CrossRef]
- Dmytryshyn, R.I.; Sharyn, S.V. Approximation of functions of several variables by multidimensional S-fractions with independent variables. Carpathian Math. Publ. 2021, 13, 592–607. [Google Scholar]
- Dmytryshyn, R.I. Two-dimensional generalization of the Rutishauser qd-algorithm. J. Math. Sci. 2015, 208, 301–309. [Google Scholar] [CrossRef]
- Zagier, D. The dilogarithm function. In Frontiers in Number Theory, Physics, and Geometry II; Cartier, P., Moussa, P., Julia, B., Vanhove, P., Eds.; Springer: Berlin, Germany, 2007; pp. 3–65. [Google Scholar]
- Cvijović, D.; Klinowsky, J. Continued-fraction expansions for the Riemann zeta function and polylogarithms. Proc. Amer. Math. Soc. 1997, 125, 2543–2550. [Google Scholar] [CrossRef]
- Andrews, G.E.; Askey, R.; Roy, R. Special Functions; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Krupnikov, E.D.; Kölbig, K.S. Some special cases of the generalized hypergeometric function q+lFq. J. Comput. Appl. Math. 1997, 78, 75–95. [Google Scholar] [CrossRef] [Green Version]
- Ho, L.S.T.; Xu, J.; Crawford, F.W.; Minin, V.N.; Suchard, M.A. Birth/birth-death processes and their computable transition probabilities with biological applications. J. Math. Biol. 2018, 76, 911–944. [Google Scholar] [CrossRef]
- Jones, W.B.; Magnus, A. Application of Stieltjes fractions to birth-death processes. In Padé and Rational Approximation; Saff, E.B., Varga, R.S., Eds.; Academic Press: New York, NY, USA, 1977; pp. 173–179. [Google Scholar]
- Murphy, J.A.; O’Donohoe, M.R. Some properties of continued fractions with applications in Markov processes. J. Inst.Math. Appl. 1975, 16, 57–71. [Google Scholar] [CrossRef]
- Komatsu, T. Asymmetric circular graph with Hosoya index and negative continued fractions. Carpathian Math. Publ. 2021, 13, 608–618. [Google Scholar]
- Komatsu, T. Branched continued fractions associated with Hosoya index of the tree graph. MATCH Commun. Math. Comput. Chem. 2020, 84, 399–428. [Google Scholar]
- Komatsu, T. Continued fraction expansions of the generating functions of Bernoulli and related numbers. Indag. Math. 2020, 31, 695–713. [Google Scholar] [CrossRef]


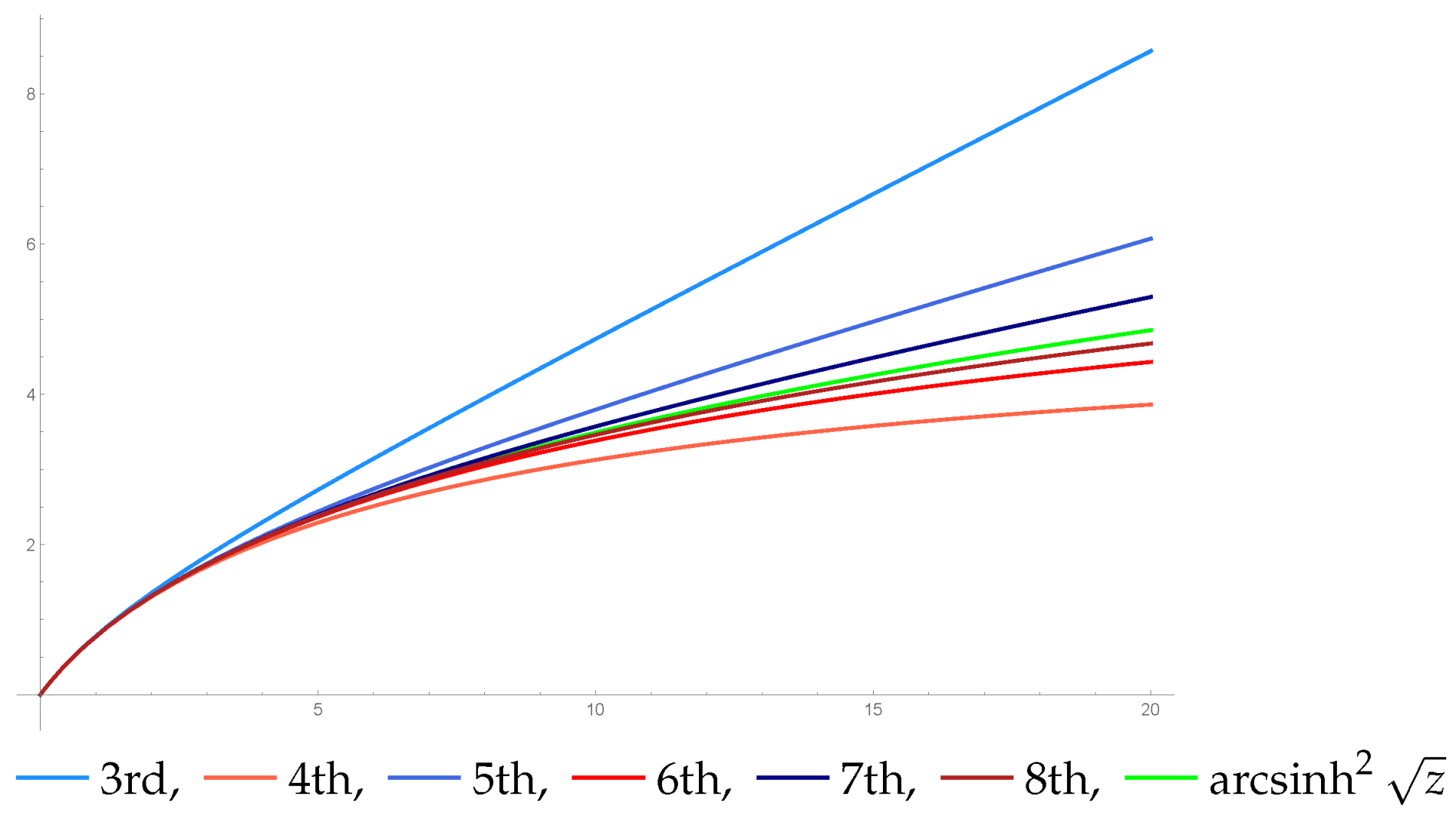
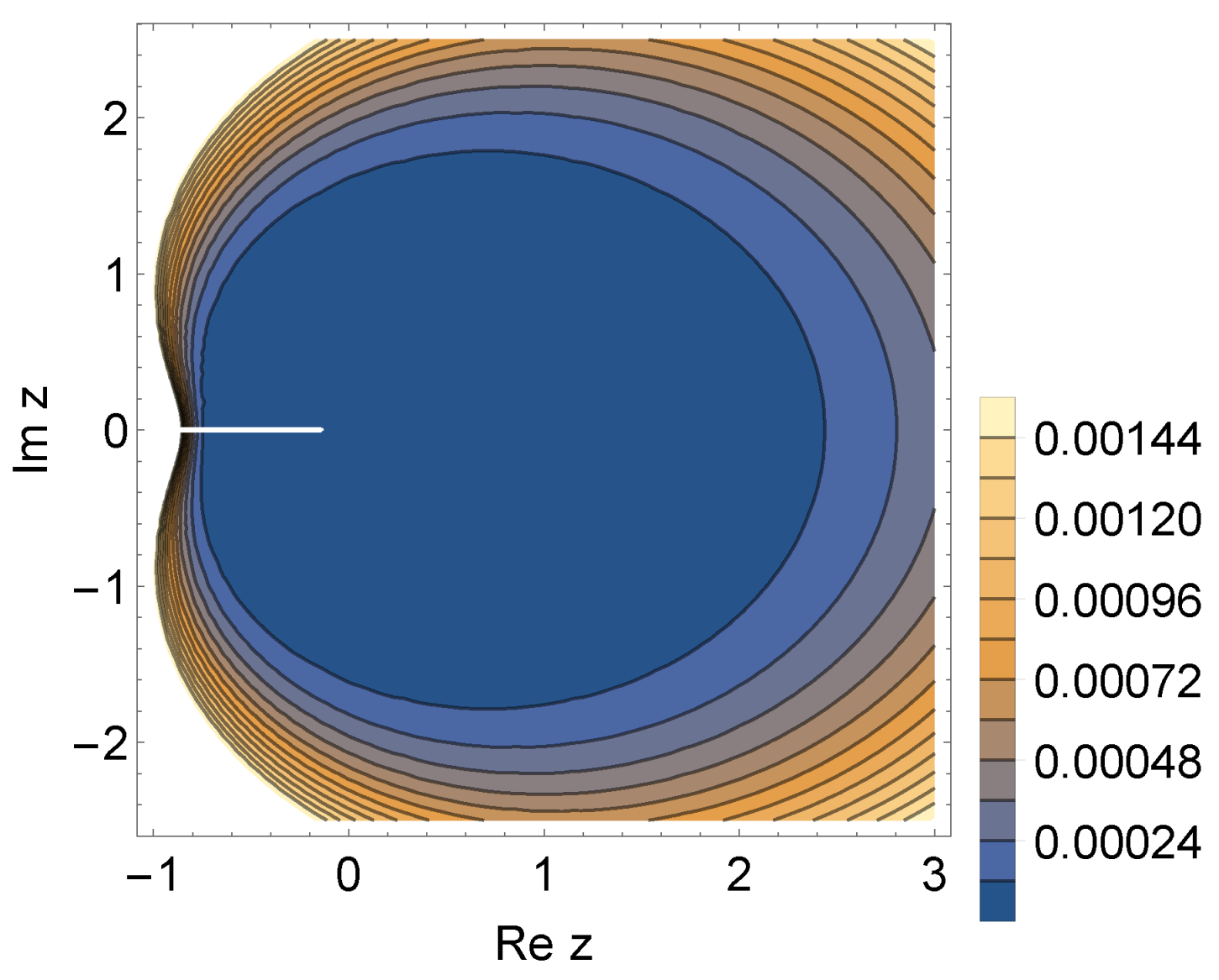
| n | ||||||
|---|---|---|---|---|---|---|
| 1 | 1.000000000000 | 1.000000000000 | 1.000000000000 | 1.000000000000 | 1.000000000000 | 1.000000000000 |
| 2 | 1.004184100418 | 1.004166666667 | 0.960000000000 | 0.958333333333 | 0.857142857143 | 0.833333333333 |
| 3 | 1.004227053140 | 1.004226190476 | 0.963541666667 | 0.964285714290 | 0.893939393940 | 0.928571428570 |
| 4 | 1.004227621300 | 1.004227585565 | 0.963169003257 | 0.962890625000 | 0.883767535070 | 0.839285714286 |
| 5 | 1.004227630503 | 1.004227628968 | 0.963214851933 | 0.963324652778 | 0.886929122814 | 0.950396825397 |
| 6 | 1.004227630666 | 1.004227630596 | 0.963208892004 | 0.963161892361 | 0.885923553202 | 0.783730158730 |
| 7 | 1.004227630669 | 1.004227630666 | 0.963209728694 | 0.963231646825 | 0.886262535450 | 1.069444444444 |
| 8 | 1.004227630669 | 1.004227630669 | 0.963209609365 | 0.963198586116 | 0.886148086455 | 0.527777777778 |
| 9 | 1.004227630669 | 1.004227630669 | 0.963209627248 | 0.963215540326 | 0.886188283668 | 1.638888888889 |
| 10 | 1.004227630669 | 1.004227630669 | 0.963209624564 | 0.963206276061 | 0.886174256708 | −0.789682539683 |
| 11 | 1.004227630669 | 1.004227630669 | 0.963209624981 | 0.963211610032 | 0.886179300167 | 4.803391053391 |
| 12 | 1.004227630669 | 1.004227630669 | 0.963209624917 | 0.963208401315 | 0.886177503402 | −8.654942279943 |
| 13 | 1.004227630669 | 1.004227630669 | 0.963209624927 | 0.963210404948 | 0.886178158992 | 24.960442335442 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Antonova, T.; Dmytryshyn, R.; Sharyn, S. Generalized Hypergeometric Function 3F2 Ratios and Branched Continued Fraction Expansions. Axioms 2021, 10, 310. https://doi.org/10.3390/axioms10040310
Antonova T, Dmytryshyn R, Sharyn S. Generalized Hypergeometric Function 3F2 Ratios and Branched Continued Fraction Expansions. Axioms. 2021; 10(4):310. https://doi.org/10.3390/axioms10040310
Chicago/Turabian StyleAntonova, Tamara, Roman Dmytryshyn, and Serhii Sharyn. 2021. "Generalized Hypergeometric Function 3F2 Ratios and Branched Continued Fraction Expansions" Axioms 10, no. 4: 310. https://doi.org/10.3390/axioms10040310
APA StyleAntonova, T., Dmytryshyn, R., & Sharyn, S. (2021). Generalized Hypergeometric Function 3F2 Ratios and Branched Continued Fraction Expansions. Axioms, 10(4), 310. https://doi.org/10.3390/axioms10040310








