Determining the Paleostress Regime during the Mineralization Period in the Dayingezhuang Orogenic Gold Deposit, Jiaodong Peninsula, Eastern China: Insights from 3D Numerical Modeling
Abstract
:1. Introduction
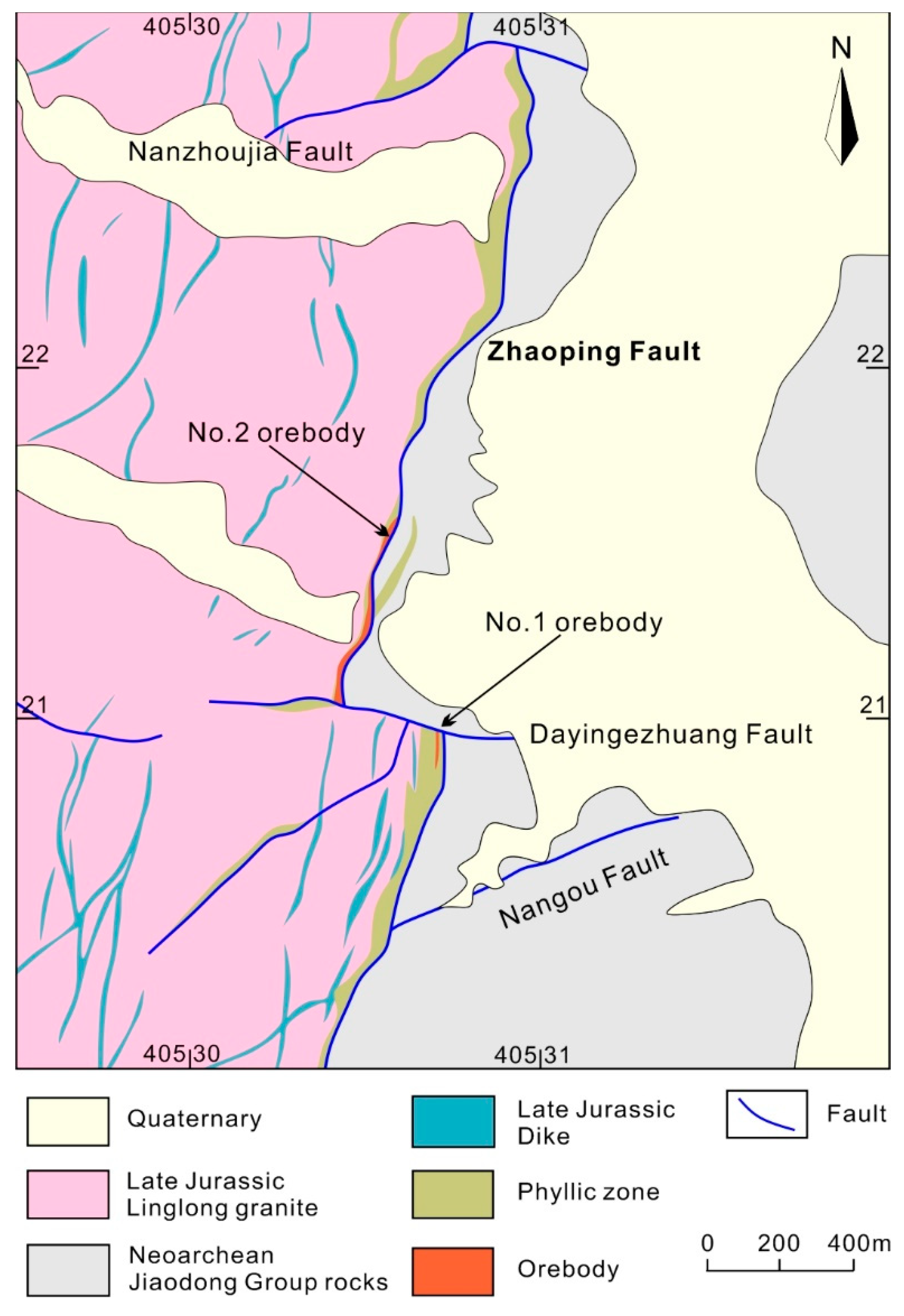
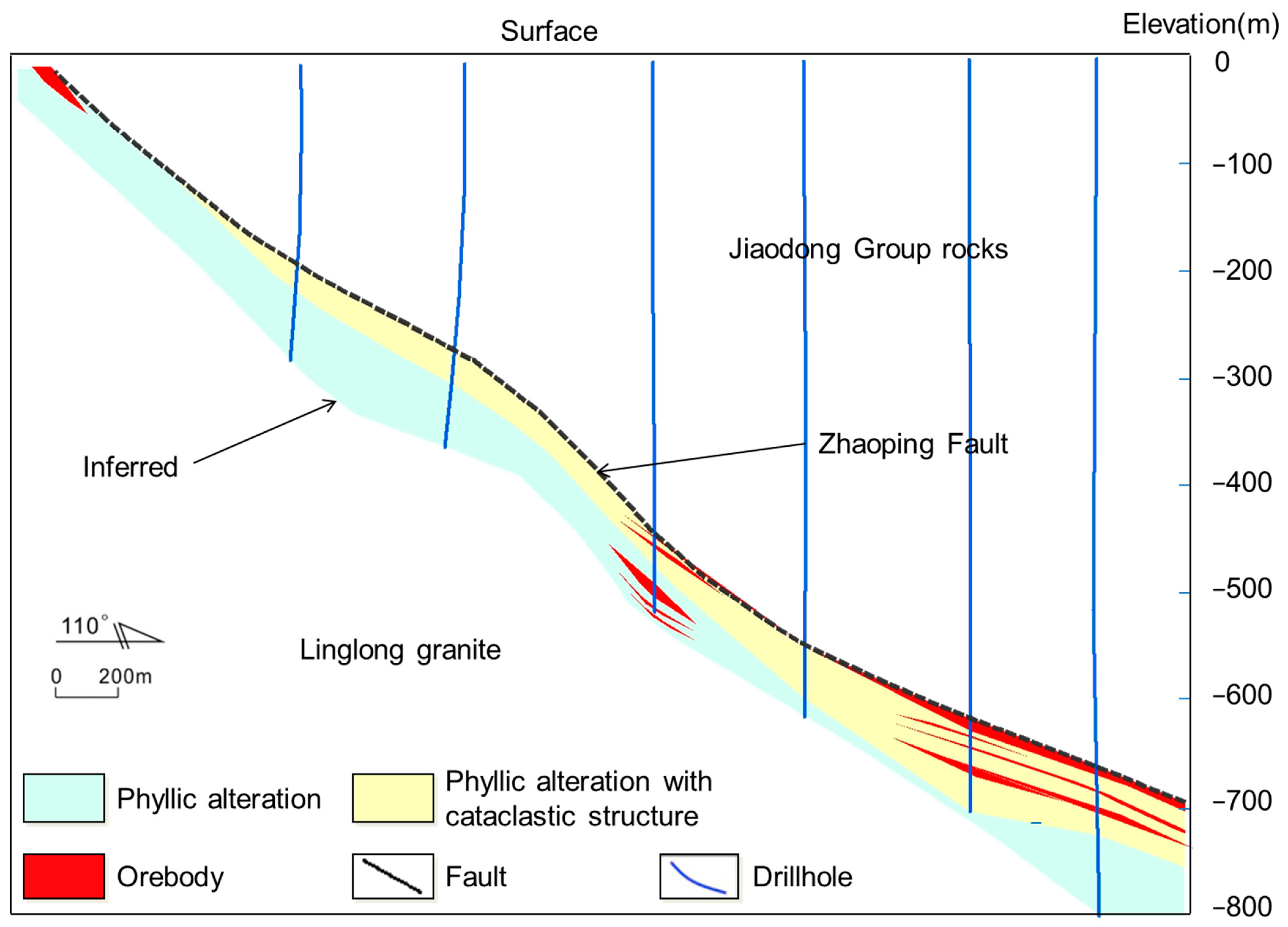
2. Geological Background
3. Method
3.1. Numerical Simulation Methods
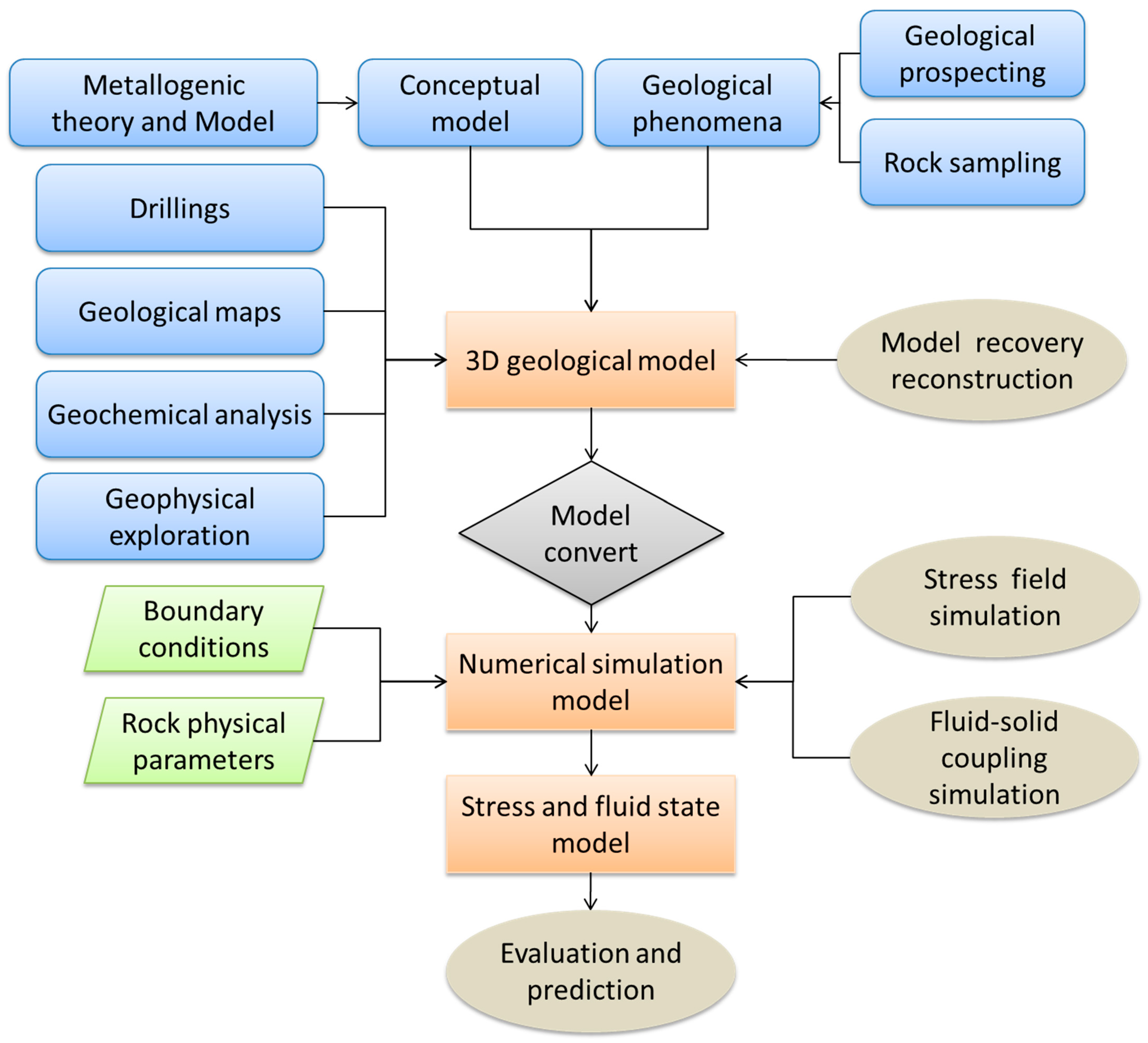
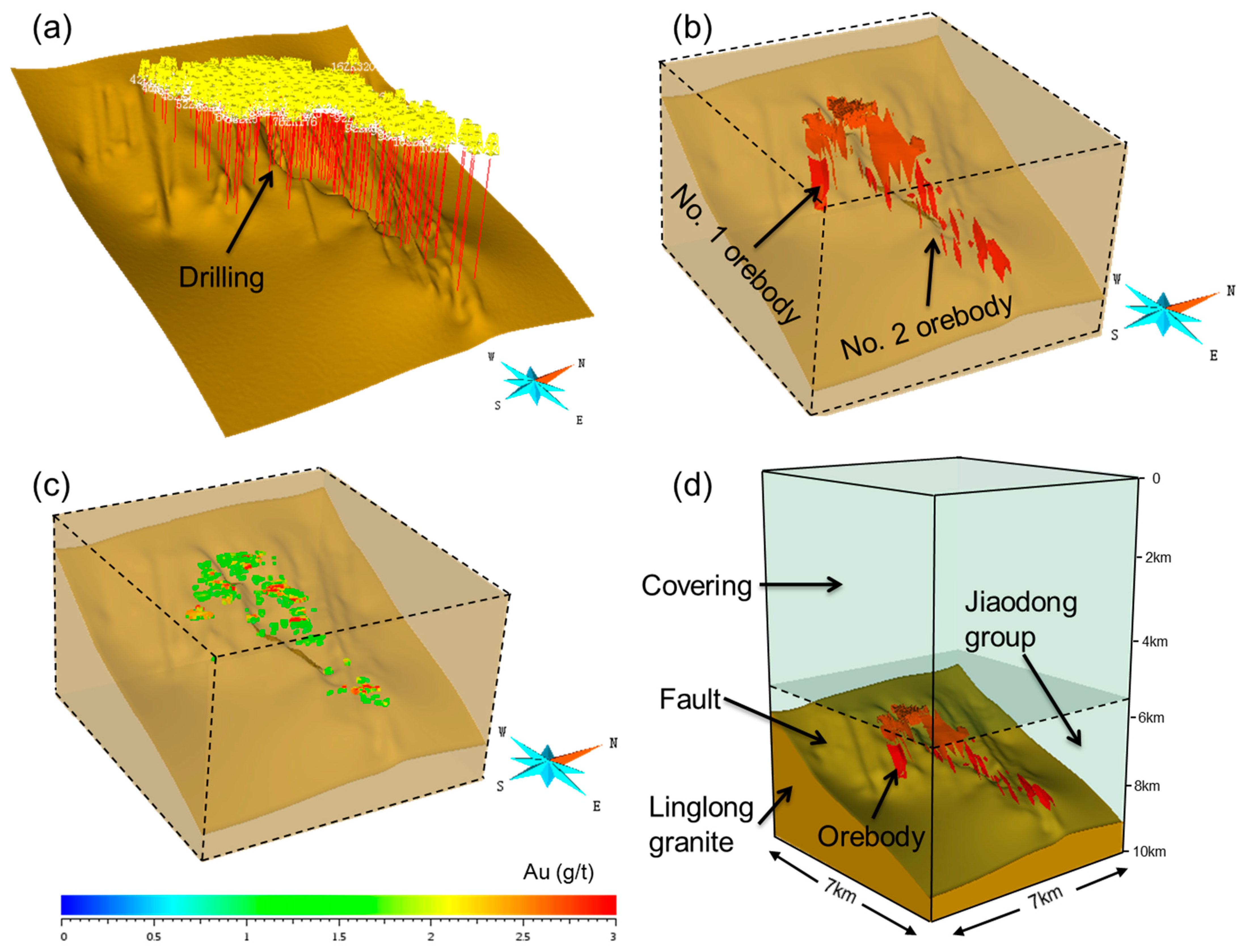
3.2. The Model Setup
3.3. Experiment Settings and Modeling Parameters
- (1)
- The base of the model was fixed vertically but moved freely horizontally. The top deformed freely in both the vertical and horizontal directions during the tectonic deformation. Most geological strain rates ranged from 10−11 to 10−17 s−1 [69]; thus, the strain rate range from 1 × 10−13 s−1 to 3 × 10−13 s−1 was assigned at the boundaries in our model [15]. To avoid geometric errors caused by the distortion of the meshes, all models in our study were compressed to a maximum strain of 1%.
- (2)
- The initial temperature distribution in the model was determined purely by heat conduction, with the temperature at the top parts of the model kept at 20 °C, while the temperature at the bottom of the model was assigned and fixed according to the geothermal gradient of 30 °C/km [65,70]. The side boundaries were treated as insulated from heat transport.
- (3)
4. Results
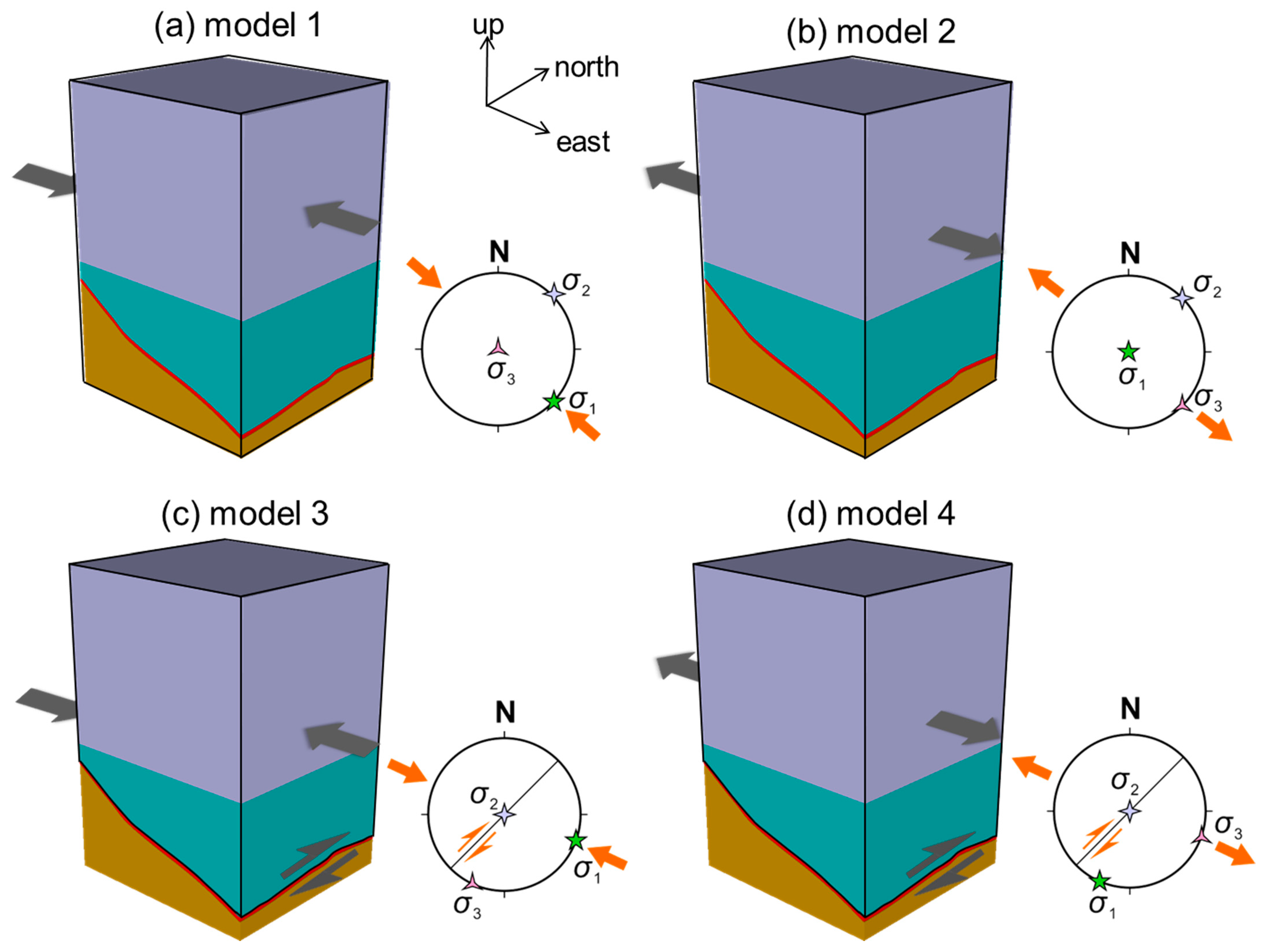
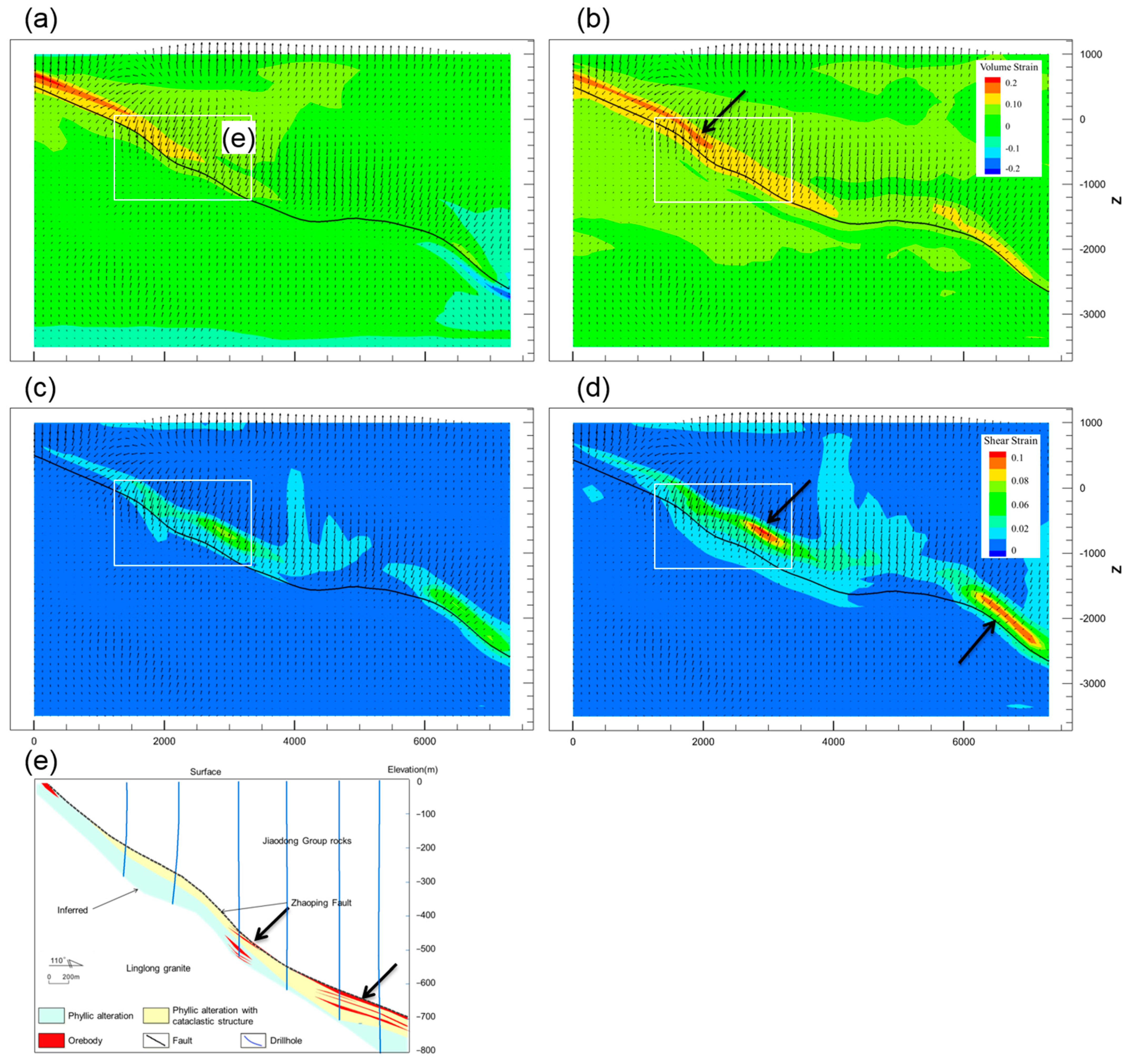
4.1. Model 1: Compression in NW–SE Direction
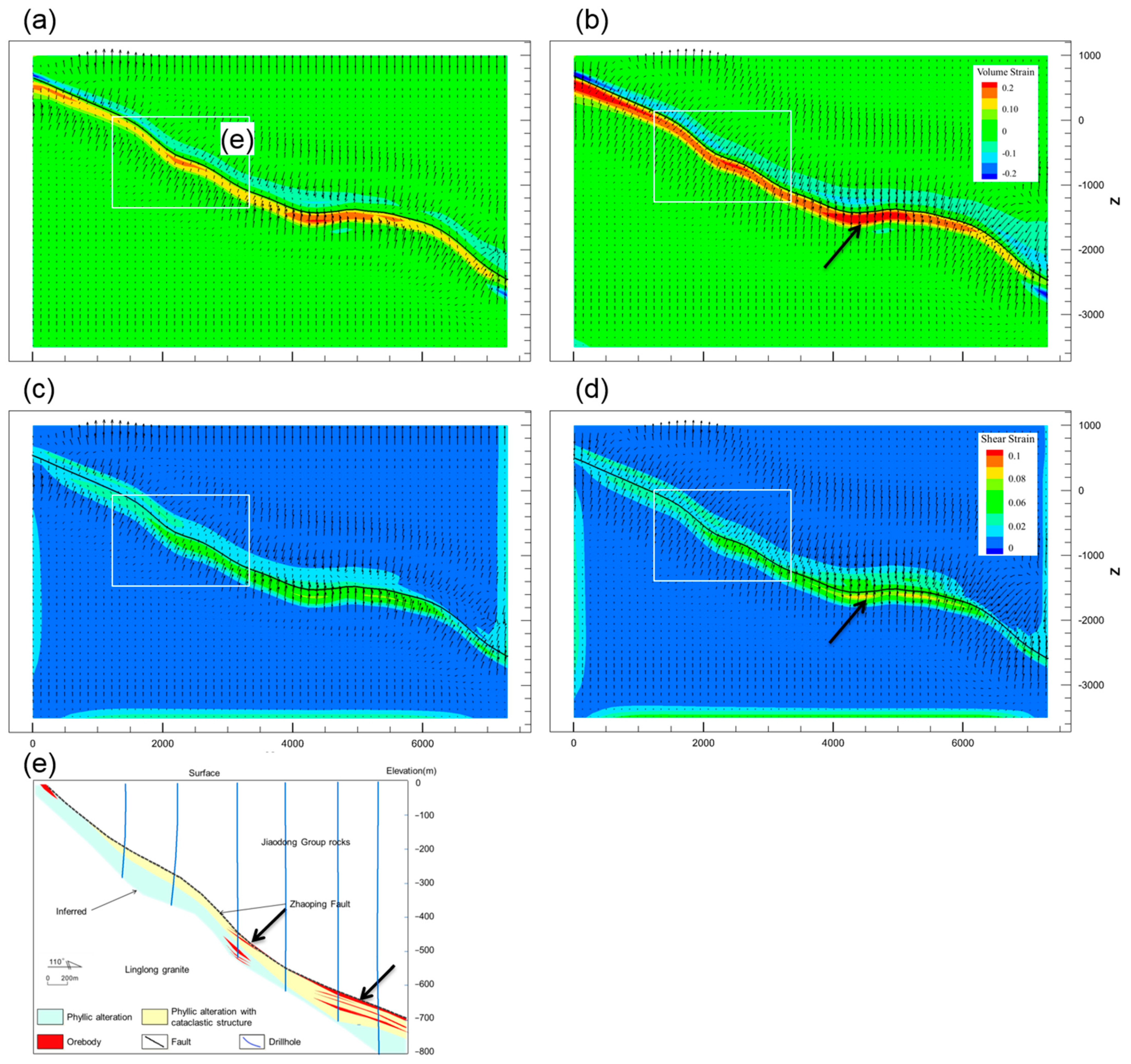
4.2. Model 2: Extension in NW–SE Direction
4.3. Model 3: Compression in NW–SE Direction with Dextral Strike-Slip
4.4. Model 4: Extension in NW–SE Direction with Dextral Strike-Slip
5. Discussion
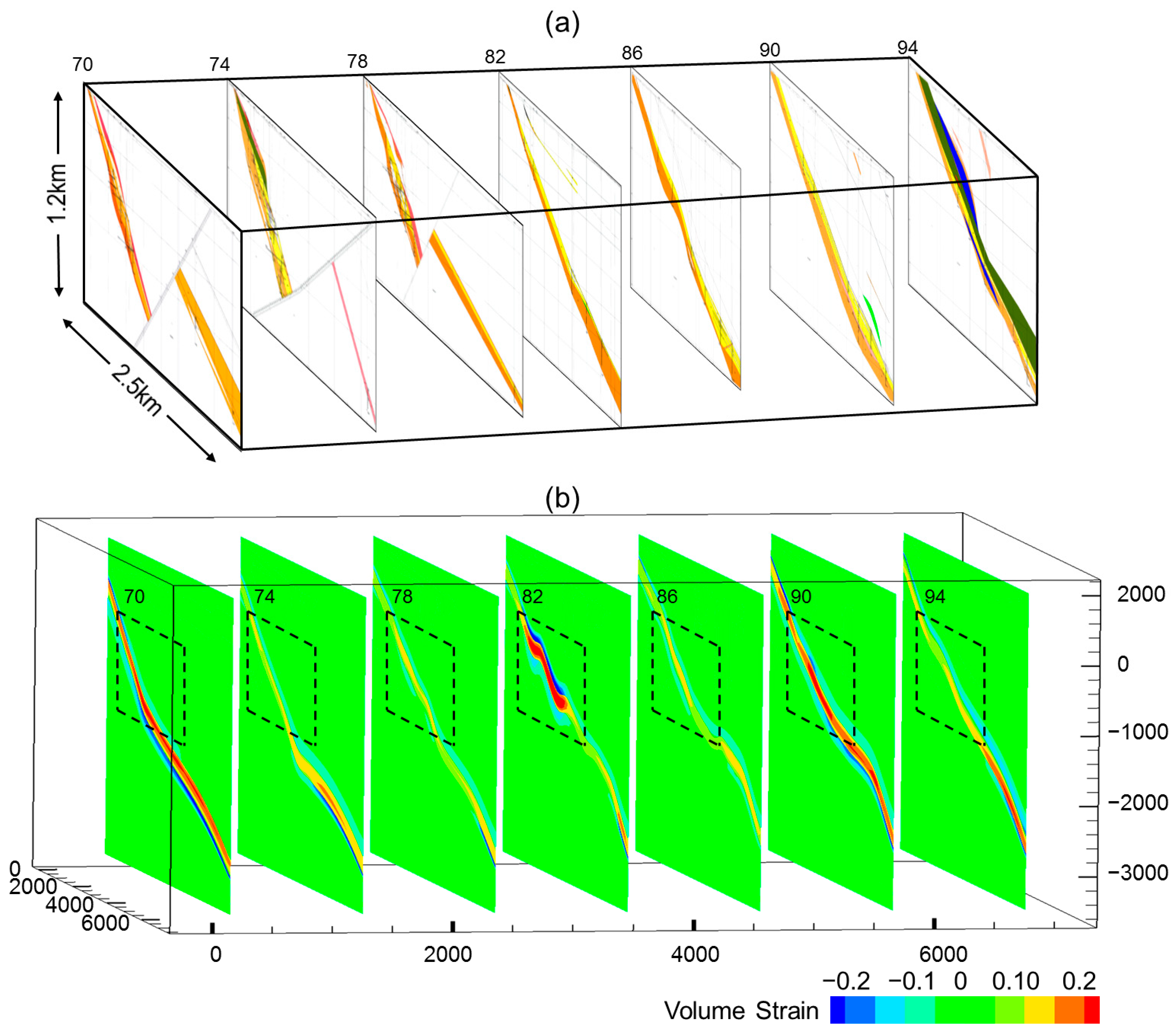
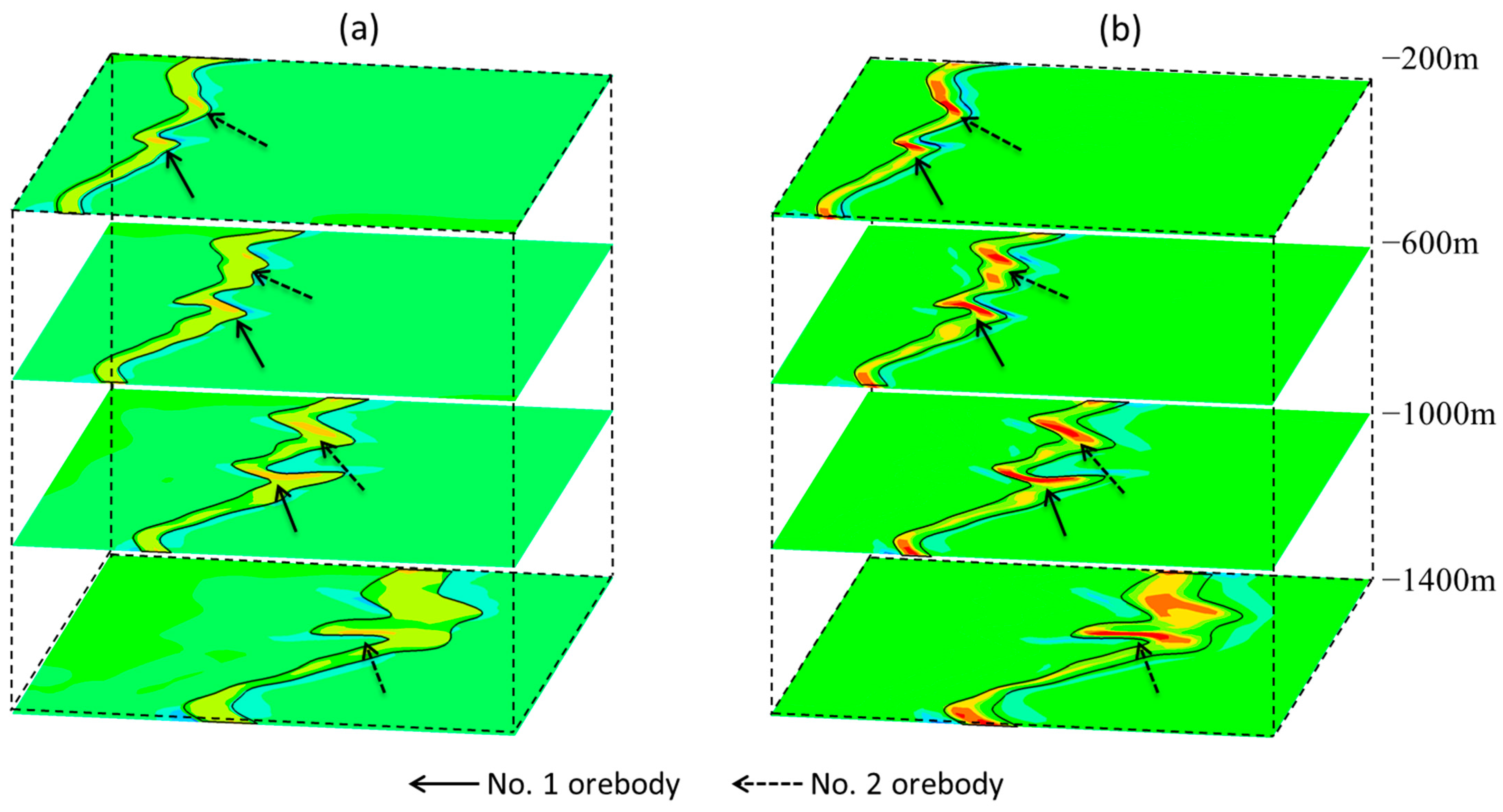
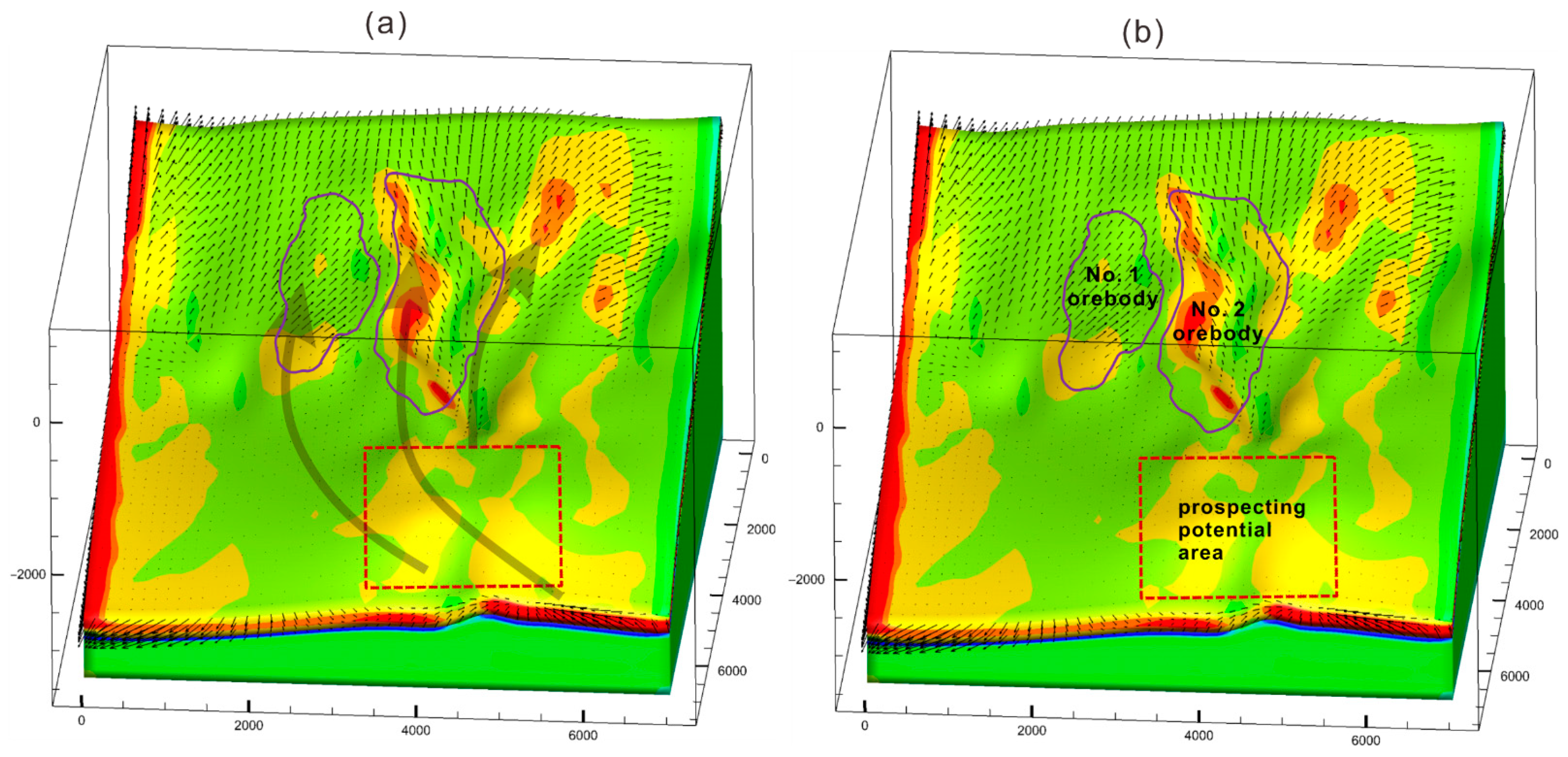
5.1. Paleostress Regimes in the Dayingezhuang District
5.2. Metallogenic and Exploration Implications
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Goldfarb, R.J.; Santosh, M. The dilemma of the Jiaodong gold deposits: Are they unique? Geosci. Front. 2014, 5, 139–153. [Google Scholar] [CrossRef] [Green Version]
- Deng, J.; Wang, Q.F.; Wan, L.; Yang, L.Q.; Gong, Q.J.; Zhao, J.; Liu, H. Self-similar fractal analysis of gold mineralization of Dayingezhuang disseminated-veinlet deposit in Jiaodong gold province, China. J. Geochem. Explor. 2009, 102, 95–102. [Google Scholar] [CrossRef]
- Li, L.; Santosh, M.; Li, S.R. The ‘Jiaodong type’ gold deposits: Characteristics, origin and prospecting. Ore Geol. Rev. 2015, 65, 589–611. [Google Scholar] [CrossRef]
- Song, M.C.; Li, S.Z.; Santosh, M.; Zhao, S.J.; Yu, S.; Yi, P.H.; Cui, S.X.; Lv, G.X.; Xu, J.X.; Song, Y.X.; et al. Types, characteristics and metallogenesis of gold deposits in the Jiaodong Peninsula, Eastern North China Craton. Ore Geol. Rev. 2015, 65, 612–625. [Google Scholar] [CrossRef]
- Yang, L.; Zhao, R.; Wang, Q.F.; Liu, X.F.; Carranza, E.J.M. Fault geometry and fluid-rock reaction: Combined controls on mineralization in the Xinli gold deposit, Jiaodong Peninsula, China. J. Struct. Geol. 2018, 111, 14–26. [Google Scholar] [CrossRef]
- Fan, H.R.; Feng, K.; Li, X.H.; Hu, F.F.; Yang, K.F. Mesozoic gold mineralization in the Jiaodong and Korean peninsulas. Acta Petrol. Sin. 2016, 32, 3225–3238. [Google Scholar]
- Liu, Z.; Mao, X.; Jedemann, A.; Bayless, R.C.; Deng, H.; Chen, J.; Xiao, K. Evolution of Pyrite Compositions at the Sizhuang Gold Deposit, Jiaodong Peninsula, Eastern China: Implications for the Genesis of Jiaodong-Type Orogenic Gold Mineralization. Minerals 2021, 11, 344. [Google Scholar] [CrossRef]
- Liu, S. On the model of space location of ore bodies and its mechanism of LingLong-type gold ore deposit. Earth Sci. J. Wuhan Coll. Geol. 1984, 27, 47–56. [Google Scholar]
- Hu, S.; Ye, Y.; Liu, H. The significance of terrane tectonics related to metallogeny of gold deposits. Gold Geol. 2001, 7, 1–8. [Google Scholar]
- Song, M.; Yi, P.; Cui, S.; Xu, J.; Zhou, M.; Jiang, H.; Huang, T. Thermal Uplifting-Extension Ore-forming Theory and Its Prospecting Significance in Jiaodong Gold Deposit. Shandong Land Resour. 2013, 29, 1–12. [Google Scholar]
- Liu, Z.-K.; Mao, X.-C.; Wang, F.-Y.; Tang, L.; Chen, G.-H.; Chen, J.; Deng, H. Deciphering anomalous Ag enrichment recorded by galena in Dayingezhuang Au(-Ag) deposit, Jiaodong Peninsula, Eastern China. Trans. Nonferrous Met. Soc. China 2021, 31, 3831–3846. [Google Scholar] [CrossRef]
- Wang, J.; Wang, G.; Tang, J. New realization on metallotectonic reging of the gold deposits in Linglong-Jiaojia district of Shandong. J. Guilin Univ. Technol. 2002, 22, 1–4. [Google Scholar]
- Yang, L.; Deng, J.; Goldfarb, R.J.; Zhang, J.; Gao, B.; Wang, Z. Ar-40/Ar-39 geochronological constraints on the formation of the Dayingezhuang gold deposit: New implications for timing and duration of hydrothermal activity in the Jiaodong gold province, China. Gondwana Res. 2014, 25, 1469–1483. [Google Scholar] [CrossRef] [Green Version]
- Li, H.; Zhou, C.; Geng, K. Intra-continental extensional tectonics of the Tan-Lu fault zone: An example from the appearance characteristics of the Yishu fault zone. Earth Sci. Front. 2017, 24, 073–084. [Google Scholar] [CrossRef]
- Li, Y.; Li, H.; Chen, G. On the Compression-extensional Tectonic Environment and Gold Mineralization in the Jiaodong Area, Shandong Province. Geotecton. Metallog. 2019, 43, 1117–1132. [Google Scholar] [CrossRef]
- Deng, J.; Yang, L.Q.; Li, R.H.; Groves, D.I.; Santosh, M.; Wang, Z.L.; Sai, S.X.; Wang, S.R. Regional structural control on the distribution of world-class gold deposits: An overview from the Giant Jiaodong Gold Province, China. Geol. J. 2019, 54, 378–391. [Google Scholar] [CrossRef] [Green Version]
- Yang, L.; Li, R.; Gao, X.; Qiu, K.; Zhang, L. A preliminary study of extreme enrichment of critical elements in the Jiaodong gold deposits, China. Acta Petrol. Sin. 2020, 36, 1285–1314. [Google Scholar] [CrossRef]
- Oliver, N.H.S.; McLellan, J.G.; Hobbs, B.E.; Cleverley, J.S.; Ord, A.; Feltrin, L. Numerical models of extensional deformation, heat transfer, and fluid flow across basement-cover interfaces during basin-related mineralization. Econ. Geol. 2006, 101, 1–31. [Google Scholar] [CrossRef]
- Hobbs, B.E.; Zhang, Y.; Ord, A.; Zhao, C. Application of coupled deformation, fluid flow, thermal and chemical modelling to predictive mineral exploration. J. Geochem. Explor. 2000, 69–70, 505–509. [Google Scholar] [CrossRef]
- Zhao, C.; Hobbs, B.; Ord, A. Fundamentals of Computational Geoscience: Numerical Methods and Algorithms; Lecture Notes in Earth Sciences; Springer; Berlin/Heidelberg, Germany, 2009; Volume 122, pp. 1–257. [Google Scholar] [CrossRef]
- Liu, L.; Wan, C.; Zhao, C.; Zhao, Y. Geodynamic constraints on orebody localization in the Anqing orefield, China: Computational modeling and facilitating predictive exploration of deep deposits. Ore Geol. Rev. 2011, 43, 249–263. [Google Scholar] [CrossRef]
- Liu, L.; Zhao, Y.; Sun, T. 3D computational shape- and cooling process-modeling of magmatic intrusion and its implication for genesis and exploration of intrusion-related ore deposits: An example from the Yueshan intrusion in Anqing, China. Tectonophysics 2012, 526, 110–123. [Google Scholar] [CrossRef]
- Beniest, A.; Koptev, A.; Burov, E. Numerical models for continental break-up: Implications for the South Atlantic. Earth Planet. Sci. Lett. 2017, 461, 176–189. [Google Scholar] [CrossRef] [Green Version]
- Cui, T.; Yang, J.; Samson, I.M. Tectonic Deformation and Fluid Flow: Implications for the Formation of Unconformity-Related Uranium Deposits. Econ. Geol. 2012, 107, 147–163. [Google Scholar] [CrossRef]
- Chi, G.; Bosman, S.; Card, C. Numerical modeling of fluid pressure regime in the Athabasca baSin. and implications for fluid flow models related to the unconformity-type uranium mineralization. J. Geochem. Explor. 2013, 125, 8–19. [Google Scholar] [CrossRef]
- Freeburn, R.; Bouilhol, P.; Maunder, B.; Magni, V.; van Hunen, J. Numerical models of the magmatic processes induced by slab breakoff. Earth Planet. Sci. Lett. 2017, 478, 203–213. [Google Scholar] [CrossRef] [Green Version]
- Sun, T.; Liu, L. Delineating the complexity of Cu–Mo mineralization in a porphyry intrusion by computational and fractal modeling: A case study of the Chehugou deposit in the Chifeng district, Inner Mongolia, China. J. Geochem. Explor. 2014, 144, 128–143. [Google Scholar] [CrossRef]
- Li, Z.; Chi, G.; Bethune, K.M.; Eldursi, K.; Thomas, D.; Quirt, D.; Ledru, P. Synchronous egress and ingress fluid flow related to compressional reactivation of basement faults: The Phoenix and Gryphon uranium deposits, southeastern Athabasca Basin, Saskatchewan, Canada. Miner. Depos. 2017, 53, 277–292. [Google Scholar] [CrossRef]
- Li, Z.; Chi, G.; Bethune, K.M.; Thomas, D.; Zaluski, G. Structural Controls on Fluid Flow During Compressional Reactivation of Basement Faults: Insights from Numerical Modeling for the Formation of Unconformity-Related Uranium Deposits in the Athabasca Basin, Canada. Econ. Geol. 2017, 112, 451–466. [Google Scholar] [CrossRef]
- Zhang, Y.; Robinson, J.; Schaubs, P. Numerical modelling of structural controls on fluid flow and mineralization. Geosci. Front. 2011, 2, 449–461. [Google Scholar] [CrossRef] [Green Version]
- Liu, X.; Xiao, C.; Zhang, S.; Chen, B. Numerical Modeling of Deformation at the Baiyun Gold Deposit, Northeastern China: Insights into the Structural Controls on Mineralization. J. Earth Sci.-China 2021, 32, 174–184. [Google Scholar] [CrossRef]
- Hu, X.; Li, X.; Yuan, F.; Ord, A.; Jowitt, S.M.; Li, Y.; Dai, W.; Zhou, T. Numerical modeling of ore-forming processes within the Chating Cu-Au porphyry-type deposit, China: Implications for the longevity of hydrothermal systems and potential uses in mineral exploration. Ore Geol. Rev. 2020, 116, 103230. [Google Scholar] [CrossRef]
- Liu, L.; Li, J.; Zhou, R.; Sun, T. 3D modeling of the porphyry-related Dawangding gold deposit in south China: Implications for ore genesis and resources evaluation. J. Geochem. Explor. 2016, 164, 164–185. [Google Scholar] [CrossRef]
- Zhou, T.; Lü, G. Tectonics, granitoids and mesozoic gold deposits in East Shandong, China. Ore Geol. Rev. 2000, 16, 71–90. [Google Scholar] [CrossRef]
- Deng, J.; Wang, Q.F.; Wan, L.; Liu, H.; Yang, L.Q.; Zhang, J. A multifractal analysis of mineralization characteristics of the Dayingezhuang disseminated-veinlet gold deposit in the Jiaodong gold province of China. Ore Geol. Rev. 2011, 40, 54–64. [Google Scholar] [CrossRef]
- Liu, Z.; Chen, J.; Mao, X.; Tang, L.; Shuyan, Y.; Deng, H.; Wang, J.; Liu, Y.; Li, S.; Bayless, R. Spatial Association Between Orogenic Gold Mineralization and Structures Revealed by 3D Prospectivity Modeling: A Case Study of the Xiadian Gold Deposit, Jiaodong Peninsula, China. Nat. Resour. Res. 2021, 30, 3987–4007. [Google Scholar] [CrossRef]
- Mao, X.; Ren, J.; Liu, Z.; Chen, J.; Tang, L.; Deng, H.; Bayless, R.C.; Yang, B.; Wang, M.; Liu, C. Three-dimensional prospectivity modeling of the Jiaojia-type gold deposit, Jiaodong Peninsula, Eastern China: A case study of the Dayingezhuang deposit. J. Geochem. Explor. 2019, 203, 27–44. [Google Scholar] [CrossRef]
- Zhang, R.Z.; Gao, B.F.; Guo, C.Y.; Song, J.J.; Fu, J.L. Orebody locating and mineral prospecting in Dayingezhuang gold deposit, Shandong province. Gold 2008, 29, 9–13. [Google Scholar]
- Qiu, Y.; Groves, D.I.; McNaughton, N.J.; Wang, L.-g.; Zhou, T. Nature, age, and tectonic setting of granitoid-hosted, orogenic gold deposits of the Jiaodong Peninsula, eastern North China craton, China. Miner. Depos. 2002, 37, 283–305. [Google Scholar] [CrossRef]
- Yang, L.; Deng, J.; Wang, Q.; Zhou, Y. Coupling Effects on Gold Mineralization of Deep and Shallow Structures in the Northwestern Jiaodong Peninsula, Eastern China. Acta Geol. Sin. Engl. Ed. 2006, 80, 400–411. [Google Scholar] [CrossRef]
- Yang, L.Q.; Deng, J.; Guo, R.P.; Guo, L.N.; Wang, Z.L.; Chen, B.H.; Wang, X.D. World-class Xincheng gold deposit: An example from the giant Jiaodong gold province. Geosci. Front. 2016, 7, 419–430. [Google Scholar] [CrossRef] [Green Version]
- Shen, K.; Hu, S.X.; Sun, J.G.; Ling, H.F.; Zhao, Y.Y.; Sun, M.Z. Characteristics of ore-forming fluids of the Dayingezhuang gold deposit in Eastern Shandong, China. Acta Petrol. Sin. 2000, 16, 542–550. [Google Scholar]
- Fan, H.; Zhai, M.; Xie, Y.; Yang, J.-H. Ore-forming fluids associated with granite-hosted gold mineralization at the Sanshandao deposit, Jiaodong gold province, China. Miner. Depos. 2003, 38, 739–750. [Google Scholar] [CrossRef]
- Wallace, R.E. Geometry of Shearing Stress and Relation to Faulting. J. Geol. 1951, 59, 118–130. [Google Scholar] [CrossRef]
- Bott, M.H.P. The Mechanics of Oblique Slip Faulting. Geol. Mag. 1959, 96, 109. [Google Scholar] [CrossRef]
- Dupin, J.M.; Sassi, W.; Angelier, J. Homogeneous stress hypothesis and actual fault slip: A distinct element analysis. J. Struct. Geol. 1993, 15, 1033–1043. [Google Scholar] [CrossRef]
- Pollard, D.D.; Saltzer, S.D.; Rubin, A.M. Stress inversion methods: Are they based on faulty assumptions? J. Struct. Geol. 1993, 15, 1045–1054. [Google Scholar] [CrossRef]
- Pascal, C.; Angelier, J.; Seland, R.; Lepvrier, C. A simplified model of stress-slip relationships: Application to the Frøy field, northern North Sea. Tectonophysics 2002, 357, 103–118. [Google Scholar] [CrossRef]
- Pascal, C. Interaction of faults and perturbation of slip: Influence of anisotropic stress states in the presence of fault friction and comparison between Wallace–Bott and 3D Distinct Element models. Tectonophysics 2002, 356, 307–322. [Google Scholar] [CrossRef]
- Maerten, L. Variation in slip on intersecting normal faults: Implications for paleostress inversion. J. Geophys. Res. 2000, 105, 197–206. [Google Scholar] [CrossRef] [Green Version]
- Maerten, L.; Maerten, F.; Mostfa, L.; Gillespie, P. Geomechanical paleostress inversion using fracture data. J. Struct. Geol. 2016, 89, 197–213. [Google Scholar] [CrossRef]
- Lejri, M.; Maerten, F.; Maerten, L.; Soliva, R. Paleostress inversion: A multi-parametric geomechanical evaluation of the Wallace–Bott assumptions. Tectonophysics 2015, 657, 129–143. [Google Scholar] [CrossRef]
- Maerten, F.; Maerten, L.; Pollard, D.D. iBem3D, a three-dimensional iterative boundary element method using angular dislocations for modeling geologic structures. Comput. Geosci.-UK 2014, 72, 1–17. [Google Scholar] [CrossRef]
- Maerten, F. Geomechanics to Solve Geological Structure Issues: Forward, Inverse and Restoration Modeling. Ph.D. Thesis, Universite Montpellier II Sciences et Techniques du Languedoc, Montpellier, France, 2010. [Google Scholar]
- Faleide, J.I.; Pascal, C.; Gabrielsen, R.; Miraj, M. Numerical Modeling of Cenozoic BaSin Inversion of the Western Barents Shelf. In Proceedings of the AAPG 2018 Annual Convention & Exhibition, Salt Lake City, UT, USA, 20–23 May 2018. [Google Scholar] [CrossRef]
- Xu, X.; Tang, S.; Lin, S. Paleostress inversion of fault-slip data from the Jurassic to Cretaceous Huangshan BaSin. and implications for the tectonic evolution of southeastern China. J. Geodyn. 2016, 98, 31–52. [Google Scholar] [CrossRef]
- Pascal, C. Paleostress Inversion Techniques: Methods and Applications for Tectonics; Elsevier: Amsterdam, the Netherlands, 2021. [Google Scholar]
- Itasca. FLAC3D User’s Guide; Group Inc.: Minneapolis, MN, USA, 2012. [Google Scholar]
- Garven, G.; Freeze, R.A. Theoretical analysis of the role of groundwater flow in the genesis of stratabound ore deposits; 1, Mathematical and numerical model. Am. J. Sci. 1984, 284, 1085–1124. [Google Scholar] [CrossRef]
- Mao, X.; Zhao, Y.; Deng, H.; Zhang, B.; Liu, Z.; Chen, J.; Zou, Y.; Lai, J. Quantitative analysis of intrusive body morphology and its relationship with skarn mineralization—A case study of Fenghuangshan copper deposit, Tongling, Anhui, China. Trans. Nonferrous Met. Soc. 2018, 28, 151–162. [Google Scholar] [CrossRef]
- Li, X.; Yuan, F.; Zhang, M.; Jowitt, S.M.; Ord, A.; Zhou, T.; Dai, W. 3D computational simulation-based mineral prospectivity modeling for exploration for concealed Fe–Cu skarn-type mineralization within the Yueshan orefield, Anqing district, Anhui Province, China. Ore Geol. Rev. 2019, 105, 1–17. [Google Scholar] [CrossRef]
- Hu, X.; Jowitt, S.; Yuan, F.; Liu, G.; Luo, J.; Chen, Y.; Yang, H.; Ren, K.; Yang, Y. Numerical modeling of mineralizing processes during the formation of the Yangzhuang Kiruna-type iron deposit, Middle and Lower Yangtze River Metallogenic Belt, China: Implications for the genesis and longevity of Kiruna-type iron oxide-apatite systems. Solid Earth Sci. 2022, 7, 23–37. [Google Scholar] [CrossRef]
- Hu, X.; Chen, Y.; Liu, G.; Yang, H.; Luo, J.; Ren, K.; Yang, Y. Numerical modeling of formation of the Maoping Pb-Zn deposit within the Sichuan-Yunnan-Guizhou Metallogenic Province, Southwestern China: Implications for the spatial distribution of concealed Pb mineralization and its controlling factors. Ore Geol. Rev. 2022, 140, 104573. [Google Scholar] [CrossRef]
- Ding, Z.J.; Sun, F.Y.; Liu, F.L.; Liu, J.H.; Peng, Q.M.; Ji, P.; Li, B.L.; Zhang, P.J. Mesozoic geodynamic evolution and metallogenic series of major metal deposits in Jiaodong Peninsula, China. Acta Petrol. Sin. 2015, 31, 3045–3080. [Google Scholar]
- Yang, L.Q.; Deng, J.; Guo, C.Y.; Zhang, J.; Jiang, S.Q.; Gao, B.F.; Gong, Q.J.; Wang, Q.F. Ore-Forming Fluid Characteristics of the Dayingezhuang Gold Deposit, Jiaodong Gold Province, China. Resour. Geol. 2009, 59, 181–193. [Google Scholar] [CrossRef]
- Fridleifsson, I.; Bertani, R.; Huenges, E.; Lund, J.; Ragnarsson, Á.; Rybach, L. The possible role and contribution of geothermal energy to the mitigation of climate change. In Proceedings of the IPCC Scoping Meeting on Renewable Energy Sources, Lübeck, Germany, 20–25 January 2008; pp. 59–80. [Google Scholar]
- Yang, L.Q.; Deng, J.; Wang, Q.F.; Gao, B.F.; Wang, L.; Guo, C. Numerical modeling of coupling metallogenic dynamics of fluid flow and thermal transporta-tion in jiaodong gold ore cluster area, China. In Proceedings of the Iamg ’07: Geomathematics and Gis Analysis of Resources, Environment and Hazards, Beijing, China, 26–31 August 2007; pp. 39–43. [Google Scholar]
- Caine, J.; Evans, J.; Forster, C. Fault zone architecture and permeability structure. Geology 1996, 24, 1025–1028. [Google Scholar] [CrossRef]
- Pfiffner, O.A.; Ramsay, J.G. Constraints on geological strain rates: Arguments from finite strain states of naturally deformed rocks. J. Geophys. Res. Solid Earth 1982, 87, 311–321. [Google Scholar] [CrossRef] [Green Version]
- Tang, J. Study on palaeogeothermal gradient and hydrocarbon generation in north China. Henan Pet. 1998, 12, 1–3. [Google Scholar]
- Oliver, N.H.S.; Pearson, P.J.; Holcombe, R.J.; Ord, A. Mary Kathleen metamorphic-hydrothermal uranium—Rare-earth element deposit: Ore genesis and numerical model of coupled deformation and fluid flow. Aust. J. Earth Sci. 1999, 46, 467–483. [Google Scholar] [CrossRef]
- Li, Z.; Chi, G.; Bethune, K.M.; Eldursi, K.; Quirt, D.; Ledru, P.; Gudmundson, G. Numerical simulation of strain localization and its relationship to formation of the Sue unconformity-related uranium deposits, eastern Athabasca Basin, Canada. Ore Geol. Rev. 2018, 101, 17–31. [Google Scholar] [CrossRef]
- Hronsky, J.M.A. Deposit-scale structural controls on orogenic gold deposits: An integrated, physical process–based hypothesis and practical targeting implications. Miner. Depos. 2020, 55, 197–216. [Google Scholar] [CrossRef]
- Wilson, C.J.L.; Osborne, D.J.; Robinson, J.A.; Miller, J.M. Structural Constraints and Localization of Gold Mineralization in Leather Jacket Lodes, Ballarat, Victoria, Australia. Econ. Geol. 2016, 111, 1073–1098. [Google Scholar] [CrossRef] [Green Version]
- Vermeer, P.A.; De Borst, R. Non-Associated Plasticity for Soils, Concrete and Rock. Mater. Sci. 1984, 29, 163–196. [Google Scholar]
- Bongiolo, E.; Bongiolo, D.; Sardini, P.; Mexias, A.; Siitari-Kauppi, M.; Boscato Gomes, M.; Formoso, M. Quantification of porosity evolution from unaltered to propylitic-altered granites: The14C-PMMA method applied on the hydrothermal system of Lavras do Sul, Brazil. An. Acad. Bras. Cienc. 2007, 79, 503–517. [Google Scholar] [CrossRef]
- Cox, S.F. Coupling between Deformation, Fluid Pressures, and Fluid Flow in Ore-Producing Hydrothermal Systems at Depth in the Crust; Society of Economic Geologists, Inc.: Littleton, CO, USA, 2005; pp. 39–75. [Google Scholar]
- Sibson, R. Controls on maximum fluid overpressure defining conditions for mesozonal mineralisation. J. Struct. Geol. 2004, 26, 1127–1136. [Google Scholar] [CrossRef]
- Li, X.; Yuan, F.; Zhang, M.; Jia, C.; Jowitt, S.; Ord, A.; Zheng, T.; Hu, X.; Li, Y. Three-dimensional mineral prospectivity modeling for targeting of concealed mineralization within the Zhonggu iron orefield, Ningwu Basin, China. Ore Geol. Rev. 2015, 71, 633–654. [Google Scholar] [CrossRef]
- Henry, A.D.; McInnes, P.; Tosdal, R.M. Structural Evolution of Auriferous Veins at the Endeavour 42 Gold Deposit, Cowal Mining District, NSW, Australia. Econ. Geol. 2014, 109, 1051–1077. [Google Scholar] [CrossRef]
- Song, M.; Song, Y.; Ding, Z. The Discovery of The Jiaojia and the Sanshandao Giant Gold Deposits in Jiaodong Peninsula and Discussion on the Relevant Issues. Geotecton. Metallog. 2019, 43, 092–110. [Google Scholar] [CrossRef]



| Symbol | Meaning |
|---|---|
| fluid-specific discharge | |
| coefficient of fluid mobility | |
| P | pressure of the pore fluid |
| fluid density | |
| gravitational acceleration | |
| heat flux | |
| effective thermal conductivity | |
| T | temperature |
| ζ | variation of fluid content |
| volumetric fluid source | |
| vector of fluid-specific discharge in the direction | |
| effective specific heat | |
| heat flux | |
| reference density of the fluid | |
| specific heat of the fluid | |
| thermal fluid source | |
| bulk density of the porous medium | |
| stress tensor of the solid | |
| velocity component in the xi direction | |
| component of gravitational acceleration in the direction | |
| thermal strain tensor | |
| coefficient of linear thermal expansion | |
| Kronecker delta | |
| variation in fluid content | |
| M | Biot modulus |
| Biot coefficient | |
| volumetric strain | |
| volumetric thermal expansion of the porous matrix |
| Density of Rock Unit and Lithology | Denudation Layer | Jiaodong Group | Fault | Linglong Granite |
|---|---|---|---|---|
| Density (kg m−3) | 2.10 | 3.80 | 2.50 | 2.67 |
| Bulk modulus (1010 Pa) | 5.70 | 2.94 | 8.60 | 4.16 |
| Shear modulus (1010 Pa) | 4.10 | 2.20 | 7.20 | 3.38 |
| Cohesive strength (106 Pa) | 2.6 | 3.8 | 4.0 | 4.2 |
| Tensile strength (106 Pa) | 1.3 | 1.8 | 2.2 | 2.3 |
| Friction angle | 16 | 30 | 15 | 32 |
| Dilatancy angle | 4 | 3 | 4 | 3 |
| Permeability (10−12 m2) | 1.6 | 1.6 | 3.0 | 1.5 |
| Porosity | 0.30 | 0.30 | 0.40 | 0.25 |
| Thermal conductivity(W·m−1·K−1) | 2.3 | 2.3 | 4.0 | 2.4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, S.; Mao, X.; Liu, Z.; Deng, H.; Chen, J.; Xiao, K. Determining the Paleostress Regime during the Mineralization Period in the Dayingezhuang Orogenic Gold Deposit, Jiaodong Peninsula, Eastern China: Insights from 3D Numerical Modeling. Minerals 2022, 12, 505. https://doi.org/10.3390/min12050505
Xie S, Mao X, Liu Z, Deng H, Chen J, Xiao K. Determining the Paleostress Regime during the Mineralization Period in the Dayingezhuang Orogenic Gold Deposit, Jiaodong Peninsula, Eastern China: Insights from 3D Numerical Modeling. Minerals. 2022; 12(5):505. https://doi.org/10.3390/min12050505
Chicago/Turabian StyleXie, Shaofeng, Xiancheng Mao, Zhankun Liu, Hao Deng, Jin Chen, and Keyan Xiao. 2022. "Determining the Paleostress Regime during the Mineralization Period in the Dayingezhuang Orogenic Gold Deposit, Jiaodong Peninsula, Eastern China: Insights from 3D Numerical Modeling" Minerals 12, no. 5: 505. https://doi.org/10.3390/min12050505
APA StyleXie, S., Mao, X., Liu, Z., Deng, H., Chen, J., & Xiao, K. (2022). Determining the Paleostress Regime during the Mineralization Period in the Dayingezhuang Orogenic Gold Deposit, Jiaodong Peninsula, Eastern China: Insights from 3D Numerical Modeling. Minerals, 12(5), 505. https://doi.org/10.3390/min12050505









