An Experimental Study of Hydraulic Jump in a Gradually Expanding Rectangular Stilling Basin with Roughened Bed
Abstract
1. Introduction
2. Materials and Methods
2.1. Experimental Set-Up and Instrumentation
2.2. Dimensional Analysis
3. Results and Discussion
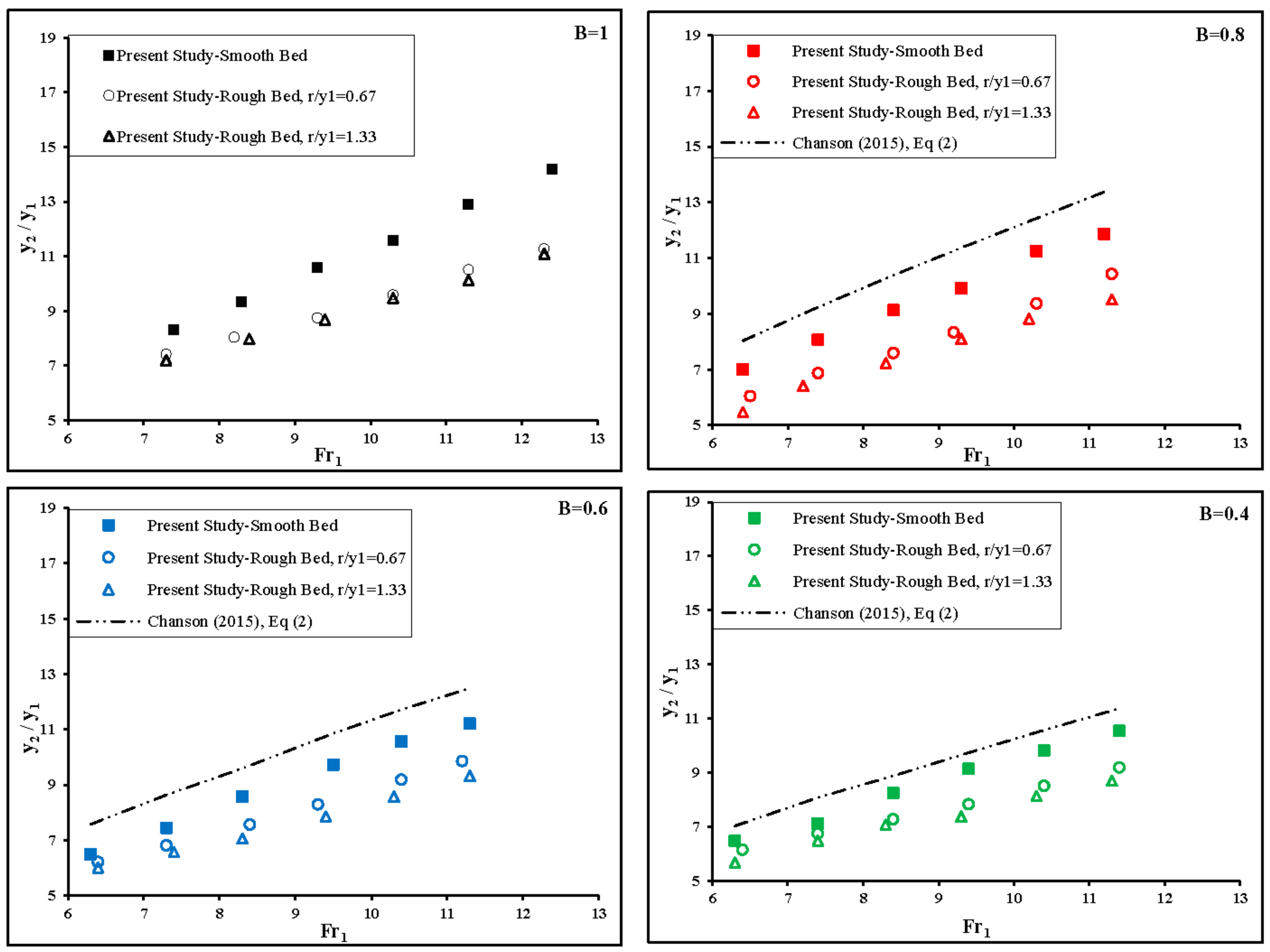
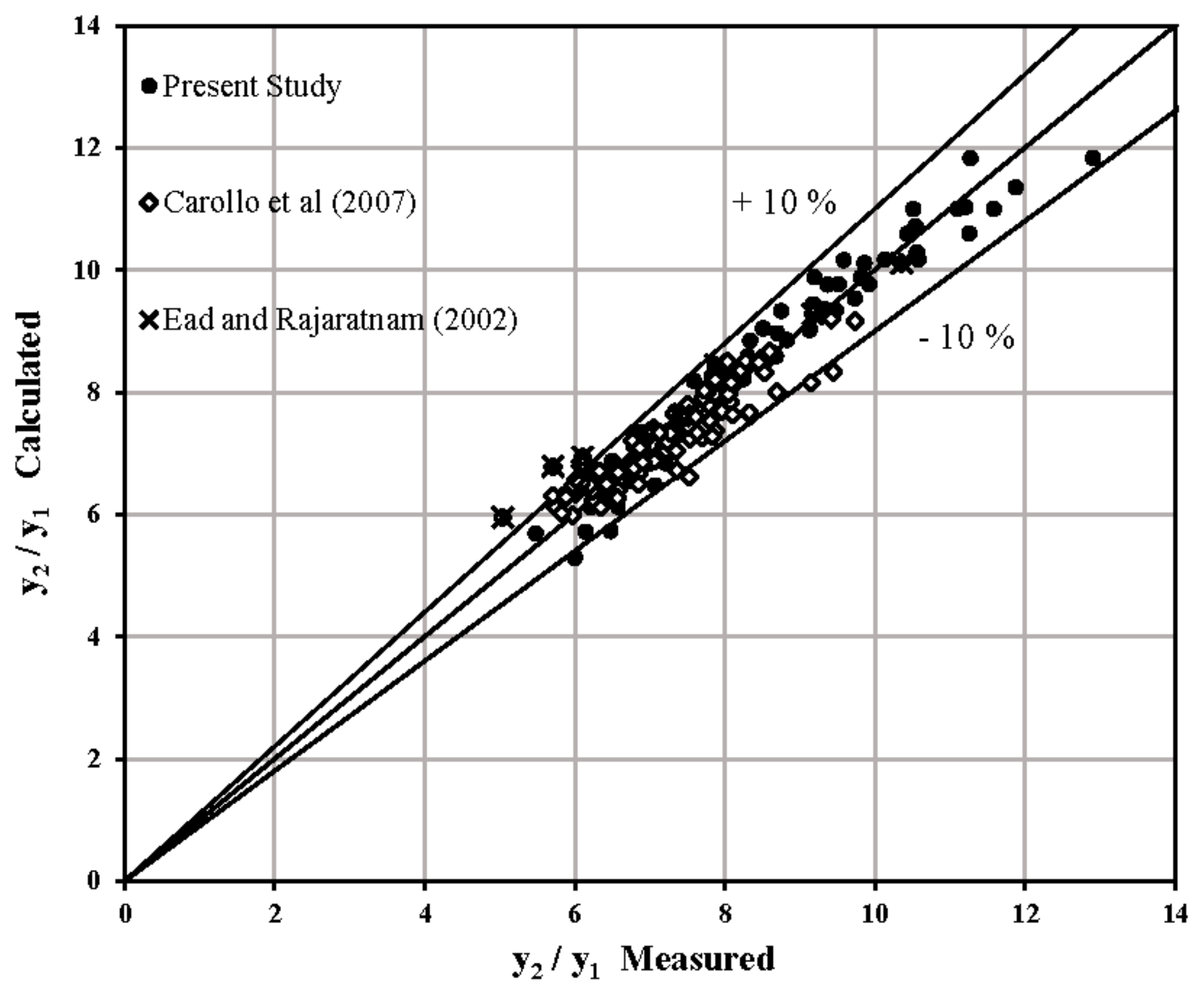
3.1. Sequent Depth Ratio
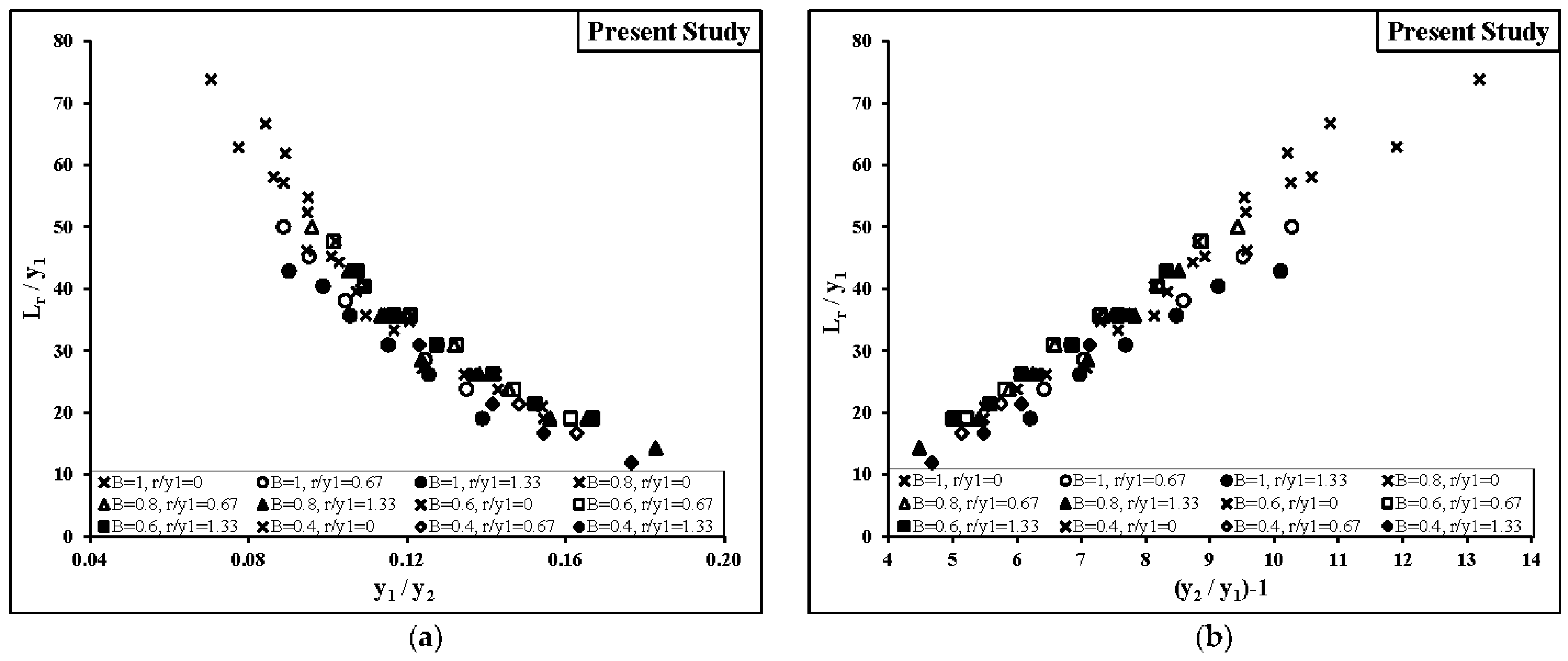
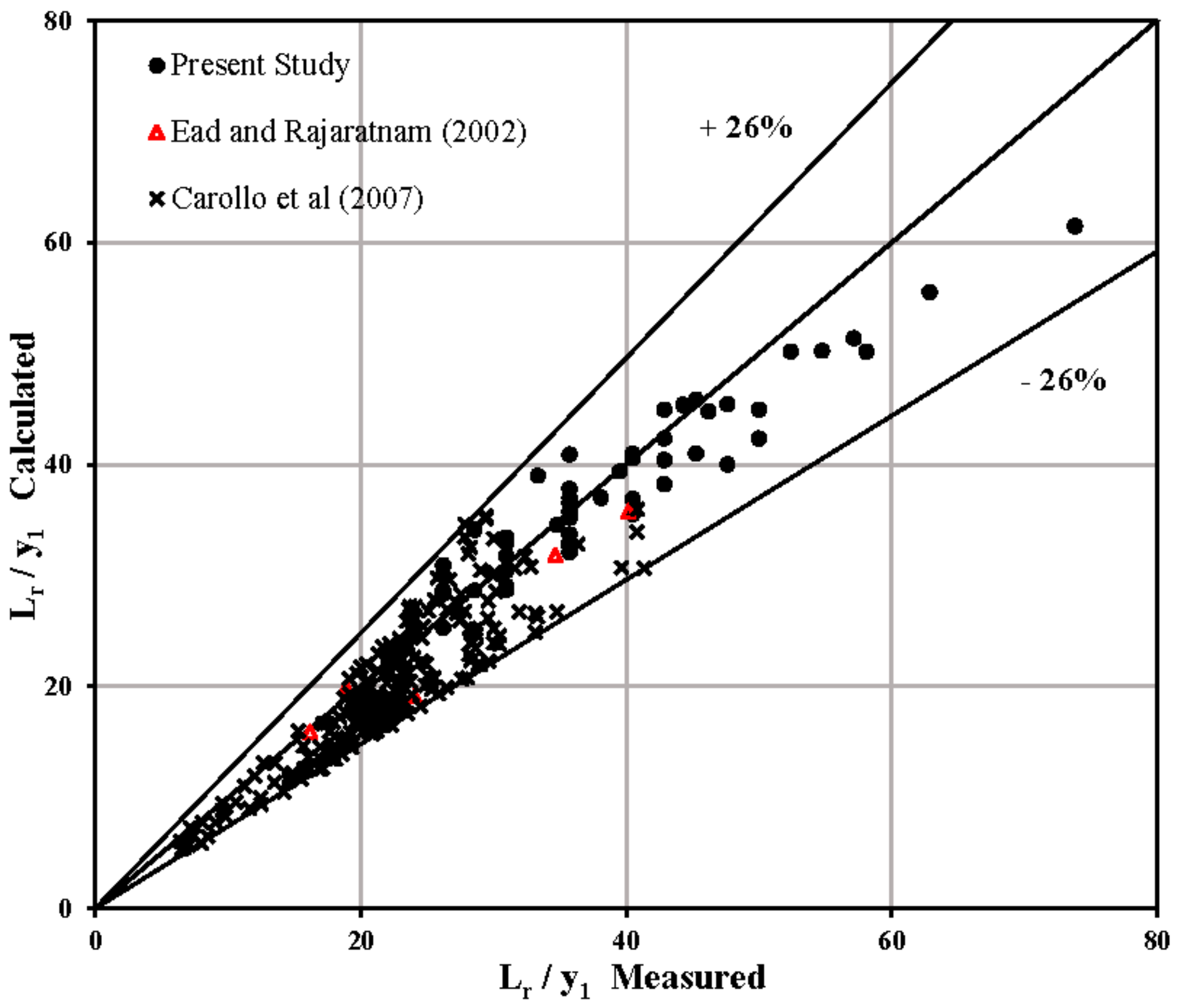
3.2. Relative Roller Length of the Hydraulic Jump
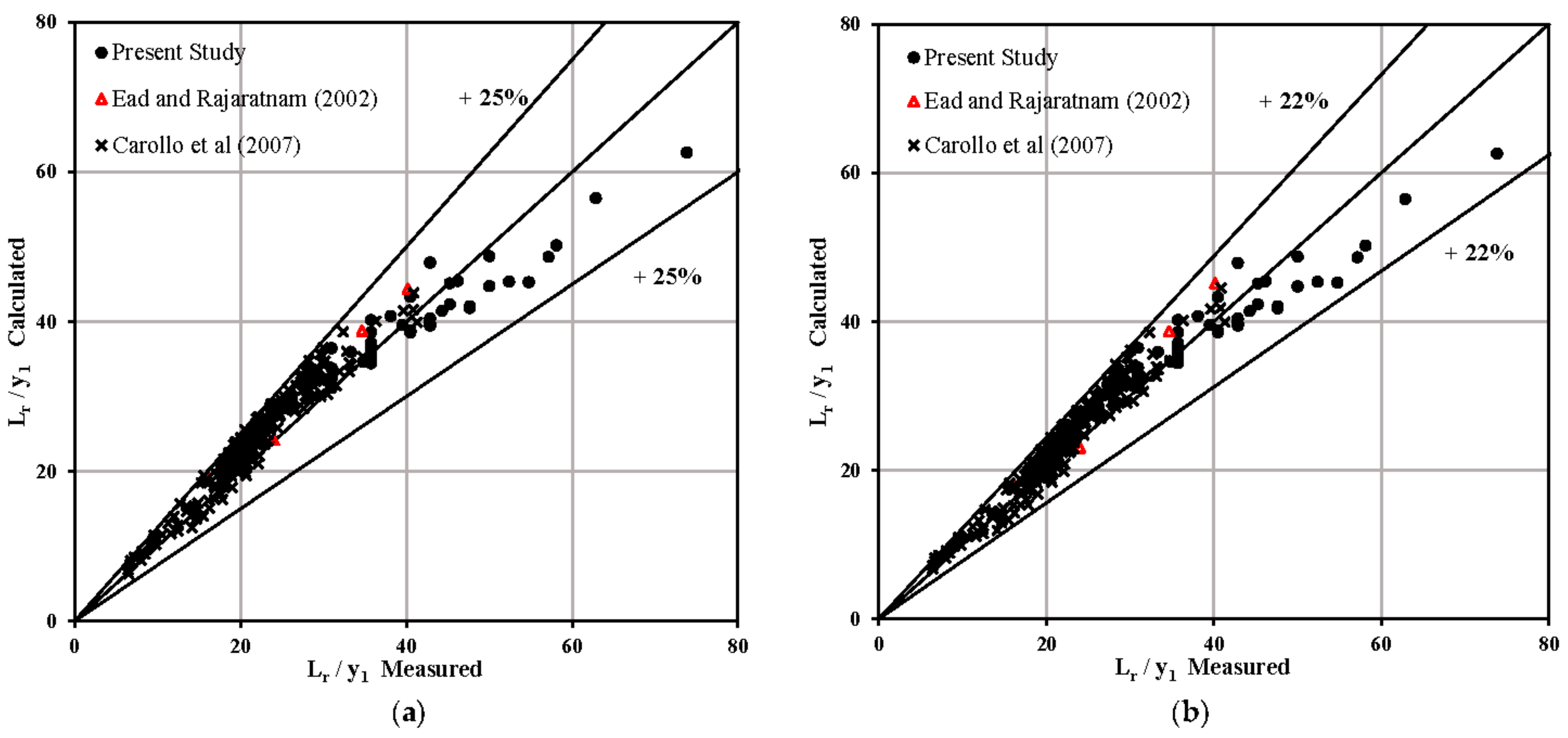
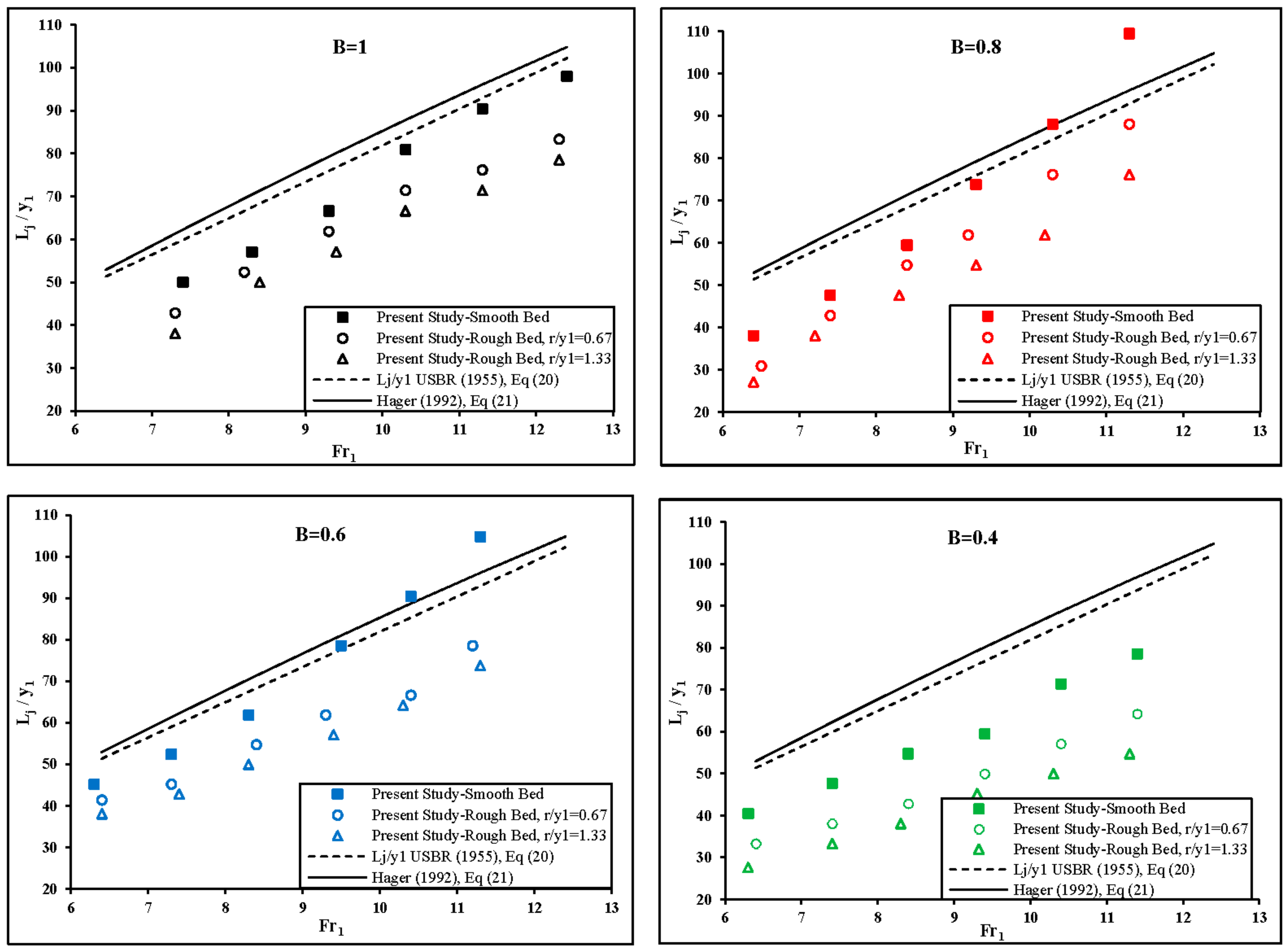
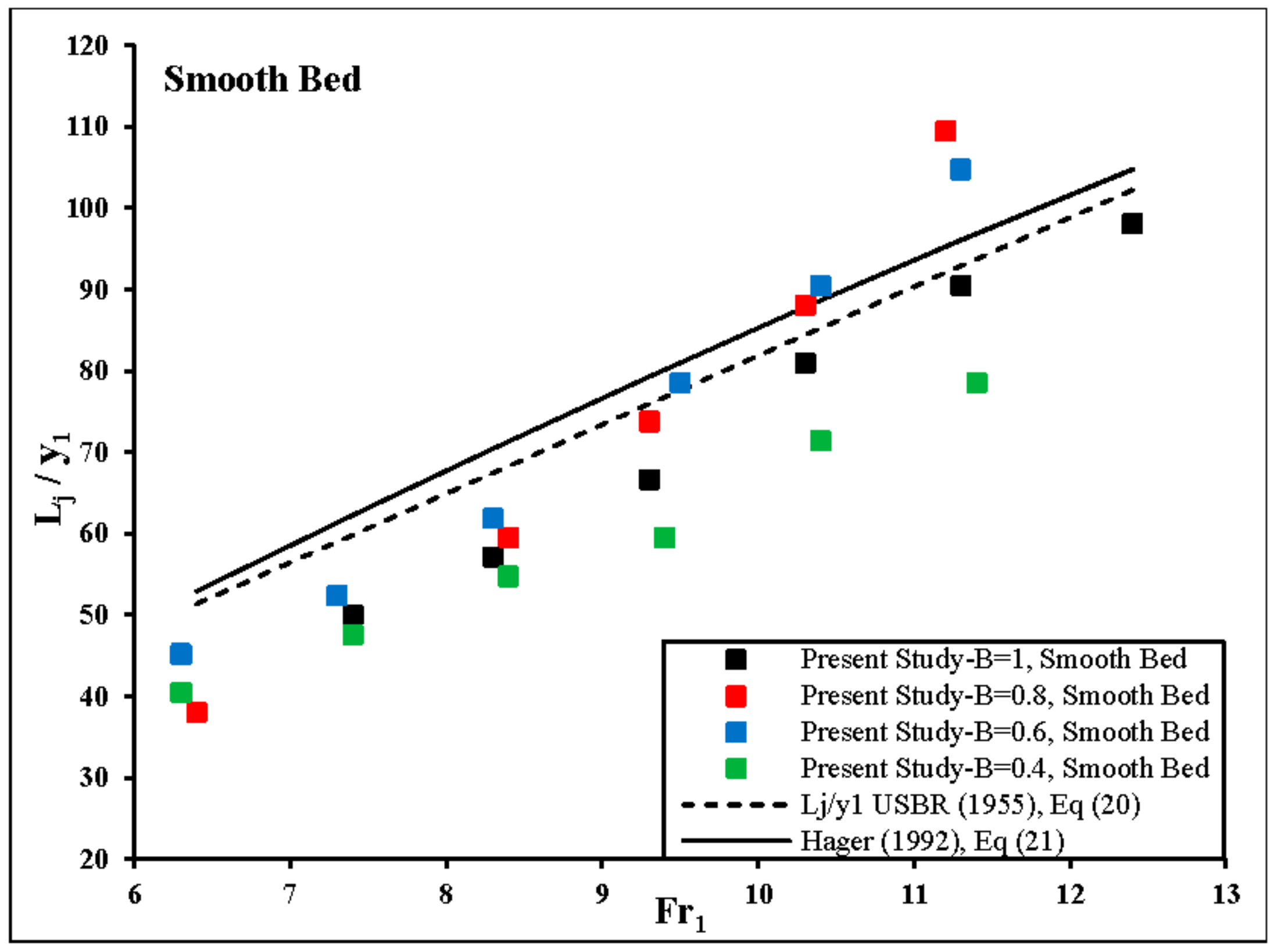
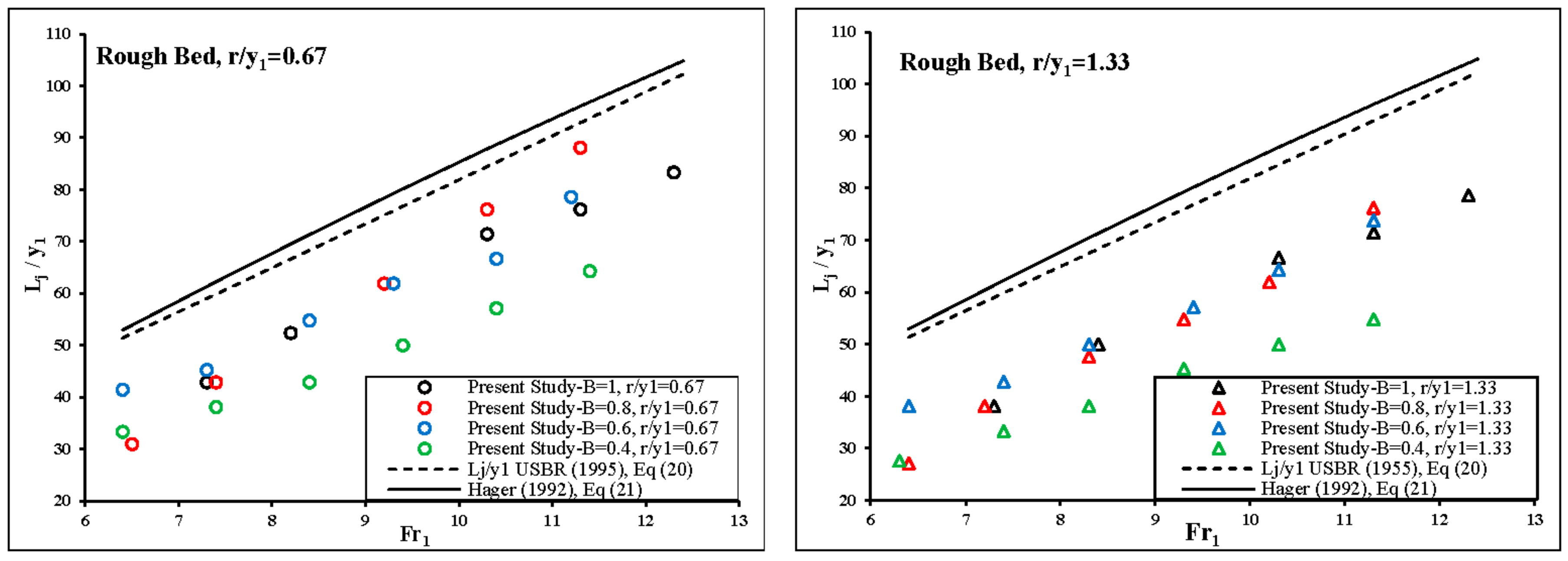
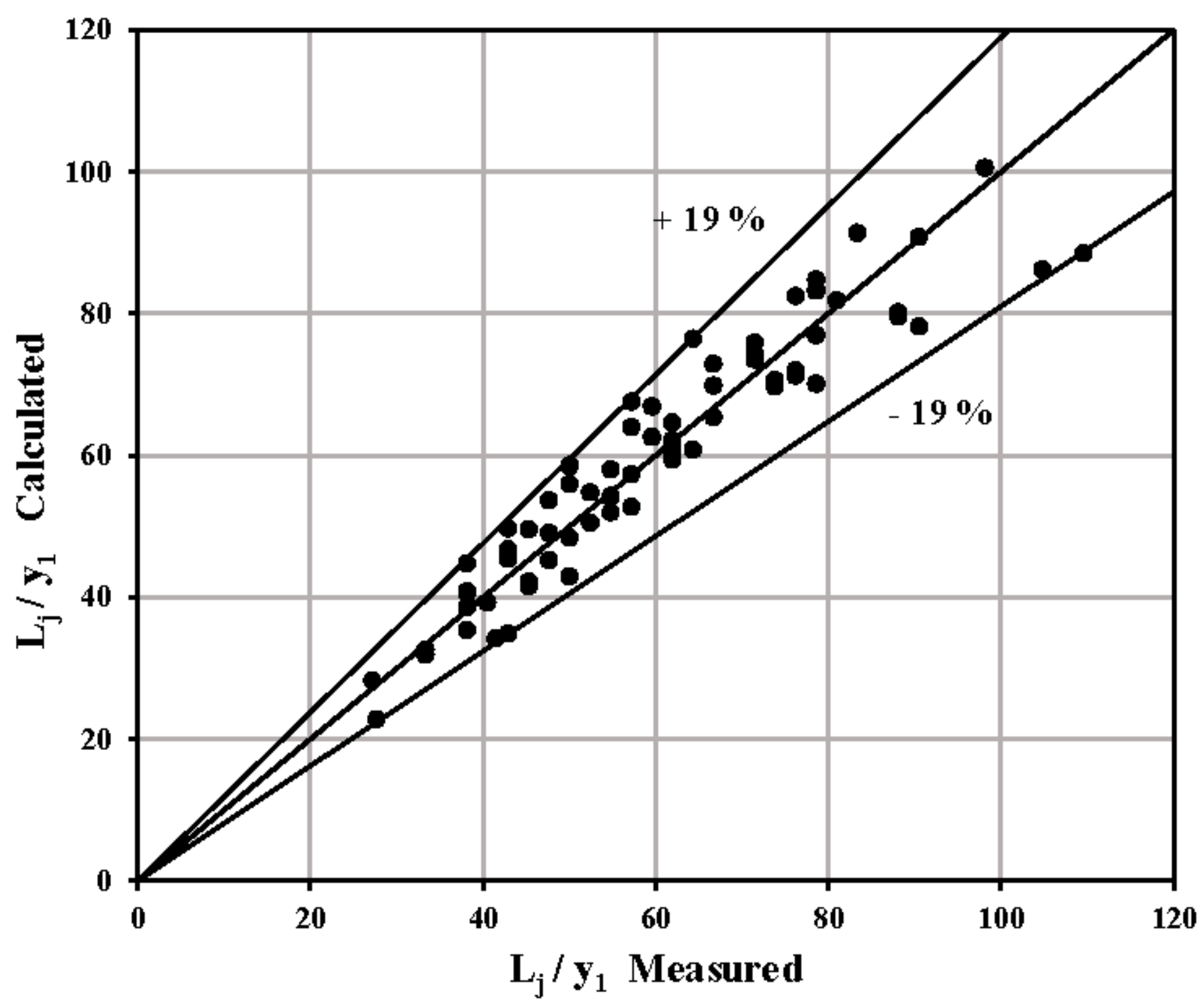
3.3. Relative Length of the Hydraulic Jump
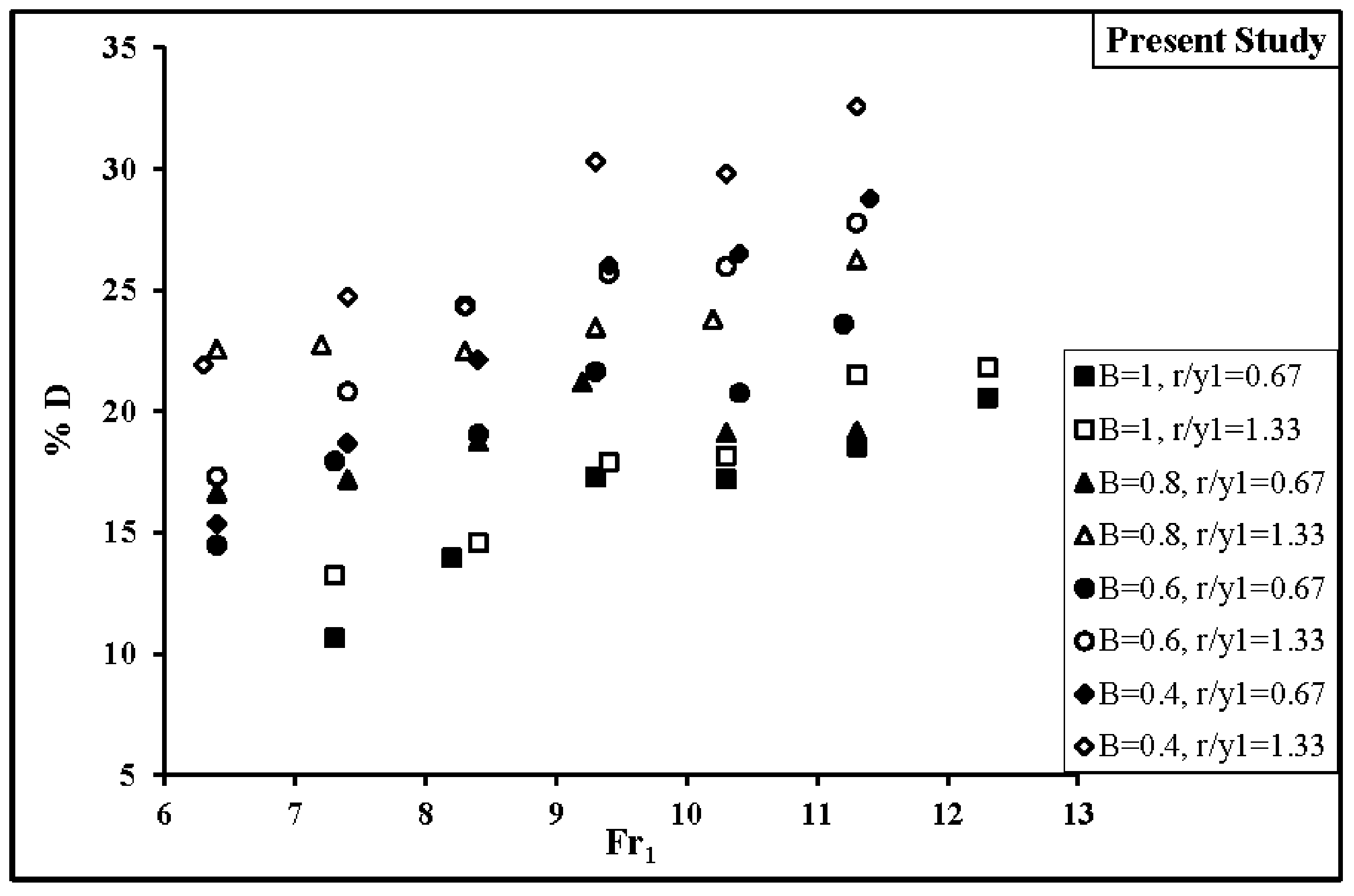
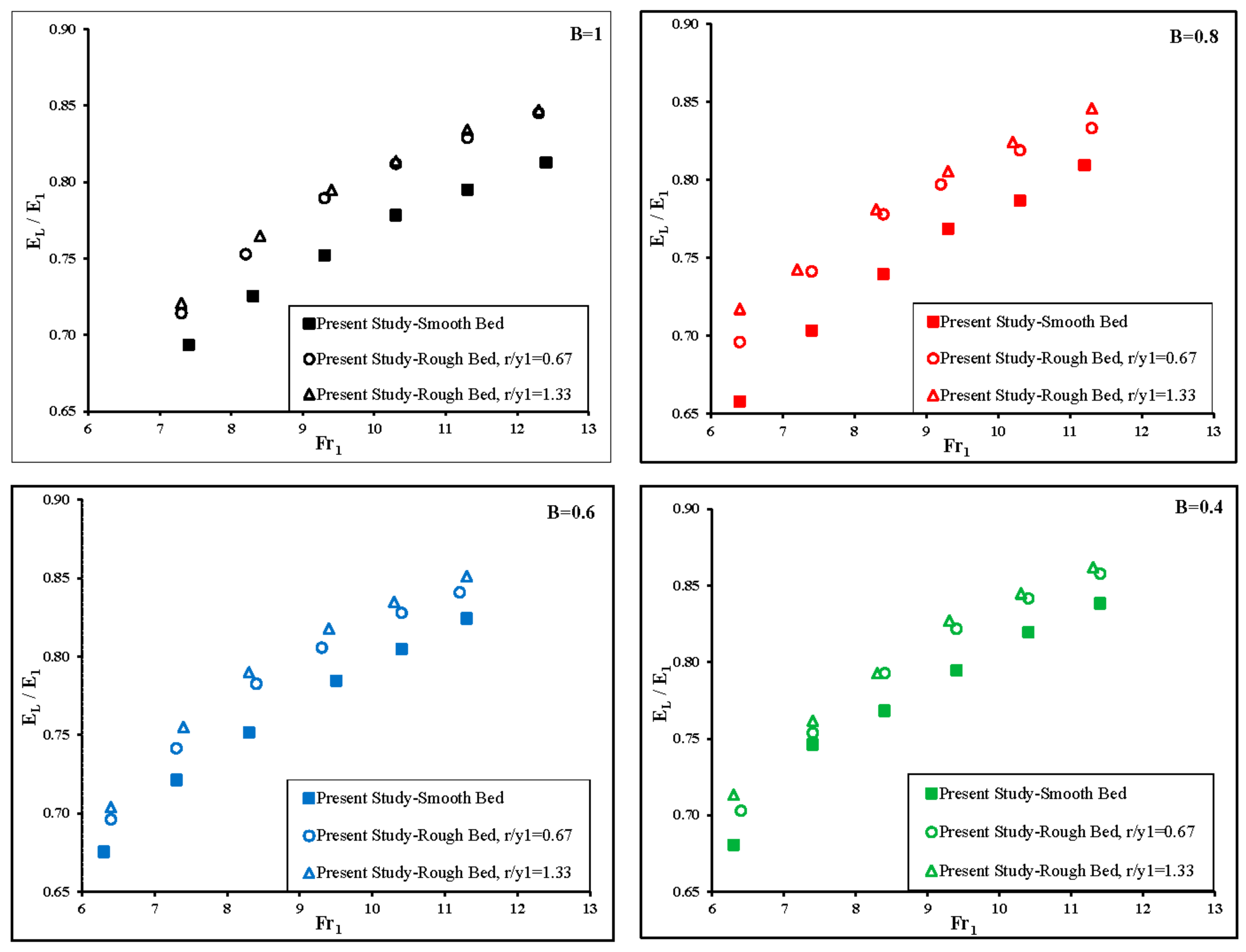
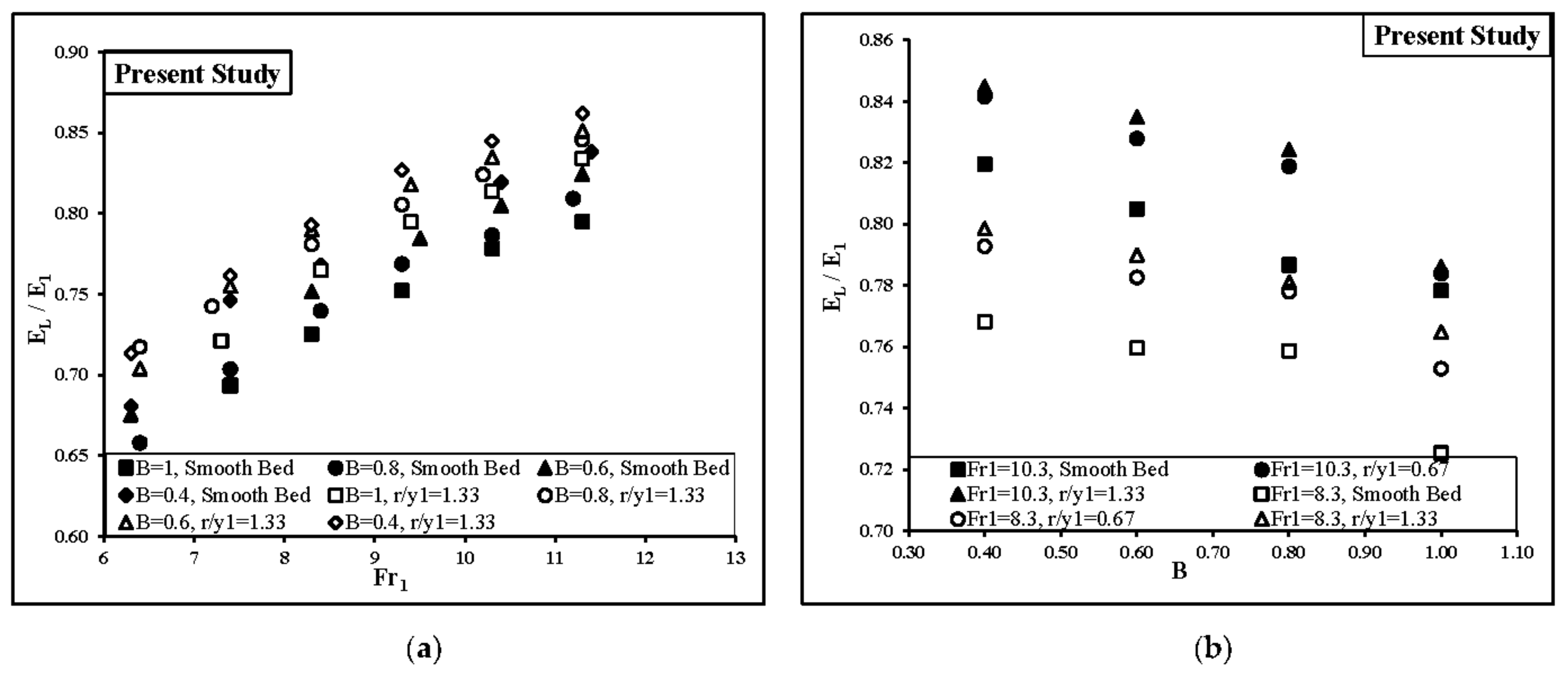
3.4. Energy Dissipation
3.5. Bed Shear Stresses
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Notation
| a | The coefficient of Equation (13) |
| a0 | The coefficient of Equation (14) |
| B | Divergence ratio |
| b0 | The coefficient of Equation (15) |
| b1 | Width of the stilling basin in upstream |
| b2 | Width of the stilling basin in downstream |
| d1 | Inflow depth of the hydraulic jump |
| d2 | Flow depth in downstream of the hydraulic jump |
| E1 | Specific energy upstream the hydraulic jump |
| E2 | Specific energy downstream the hydraulic jump |
| EL | Energy loss in the hydraulic jump |
| Fr1 | Inflow Froude number, where |
| g | Gravitational acceleration |
| Re1 | Inflow Reynolds number |
| r | Height of roughness elements |
| Lj | Length of the hydraulic jump |
| Lr | Roller length of the hydraulic jump |
| Lj/y1 | Relative length of the hydraulic jump |
| Lr/y1 | Relative roller length of the hydraulic jump |
| q | Discharge per unit width |
| EL/E1 | Relative energy loss |
| y1 | Inflow depth of the hydraulic jump |
| y2 | Sequent depth of the hydraulic jump |
| y2* | Sequent depth of the classic hydraulic jump |
| M1 | Momentum flux at the beginning of the hydraulic jump |
| M2 | Momentum flux at the end of the hydraulic jump |
| P1 | Hydrostatic force at the section upstream of the hydraulic jump |
| P2 | Hydrostatic force at the section downstream of the hydraulic jump |
| R2 | Coefficient of determination |
| Fp1 | Pressure force upstream the hydraulic jump |
| Fp2 | Pressure force downstream the hydraulic jump |
| Fe | Pressure force on the expanding wall |
| Integrated bed shear stress over the hydraulic jump length | |
| Shear force coefficient | |
| Mass density of water | |
| Kinematic viscosity of water |
References
- Chanson, H.; Carvalho, R.F. Hydraulic jumps and stilling basins. In Energy Dissipation in Hydraulic Structures; CRC Press: Leiden, The Netherlands, 2015; p. 168. [Google Scholar]
- Chanson, H. Development of the Bélanger equation and backwater equation by Jean-Baptiste Bélanger (1828). J. Hydraul. Eng. 2009, 135, 159–163. [Google Scholar] [CrossRef]
- Carollo, F.G.; Ferro, V.; Pampalone, V. New solution of classical hydraulic jump. J. Hydraul. Eng. 2009, 135, 527–531. [Google Scholar] [CrossRef]
- Gill, M. Effect of boundary roughness on hydraulic jump. Water Power Dam Constr. 1980, 32, 22–24. [Google Scholar]
- Peterka, A.J. Hydraulic Design of Stilling Basins and Energy Dissipators; United States Department of the Interior: Washington, DC, USA, 1958.
- Rajaratnam, N. The Hydraulic Jump as a Well Jet. J. Hydraul. Div. 1965, 91, 107–132. [Google Scholar]
- Leutheusser, H.J.; Kartha, V.C. Effects of inflow conditions on hydraulic jump. J. Hydraul. Div. 1972, 98, 1367–1385. [Google Scholar]
- McCorquodale, J.A.; Khalifa, A. Internal flow in hydraulic jumps. J. Hydraul. Eng. 1983, 109, 684–701. [Google Scholar] [CrossRef]
- Hager, W.H.; Bremen, R. Classical hydraulic jump: Sequent depths. J. Hydraul. Res. 1989, 27, 565–585. [Google Scholar] [CrossRef]
- Hager, W.H. Energy Dissipators and Hydraulic Jump; Springer Science and Business Media: Berlin, Germany, 1992; Volume 8. [Google Scholar]
- Wu, S.; Rajaratnam, N. Free jumps, submerged jumps and wall jets. J. Hydraul. Res. 1995, 33, 197–212. [Google Scholar] [CrossRef]
- Mossa, M.; Tolve, U. Flow visualization in bubbly two-phase hydraulic jump. Trans.-Am. Soc. Mech. Eng. J. Fluids Eng. 1998, 120, 160–165. [Google Scholar] [CrossRef]
- Gualtieri, C.; Chanson, H. Experimental analysis of Froude number effect on air entrainment in the hydraulic jump. Environ. Fluid Mech. 2007, 7, 217–238. [Google Scholar] [CrossRef]
- Chanson, H.; Gualtieri, C. Similitude and scale effects of air entrainment in hydraulic jumps. J. Hydraul. Res. 2008, 46, 35–44. [Google Scholar] [CrossRef]
- Gualtieri, C.; Chanson, H. Effect of Froude number on bubble clustering in a hydraulic jump. J. Hydraul. Res. 2010, 48, 504–508. [Google Scholar] [CrossRef]
- Gualtieri, C.; Chanson, H. Interparticle arrival time analysis of bubble distributions in a dropshaft and hydraulic jump. J. Hydraul. Res. 2013, 51, 253–264. [Google Scholar] [CrossRef]
- Rajaratnam, N. Hydraulic jumps on rough beds. Trans. Eng. Inst. Can. 1968, 11, 1–8. [Google Scholar]
- Ead, S.; Rajaratnam, N. Hydraulic jumps on corrugated beds. J. Hydraul. Eng. 2002, 128, 656–663. [Google Scholar] [CrossRef]
- Hughes, W.C.; Flack, J.E. Hydraulic jump properties over a rough bed. J. Hydraul. Eng. 1984, 110, 1755–1771. [Google Scholar] [CrossRef]
- Mossa, M.; Petrillo, A.; Chanson, H. Tailwater level effects on flow conditions at an abrupt drop. J. Hydraul. Res. 2003, 41, 39–51. [Google Scholar] [CrossRef]
- Pagliara, S.; Lotti, I.; Palermo, M. Hydraulic jump on rough bed of stream rehabilitation structures. J. Hydro-Environ. Res. 2008, 2, 29–38. [Google Scholar] [CrossRef]
- Mossa, M. On the oscillating characteristics of hydraulic jumps. J. Hydraul. Res. 1999, 37, 541–558. [Google Scholar] [CrossRef]
- Wanoschek, R.; Hager, W.H. Hydraulic jump in trapezoidal channel. J. Hydraul. Res. 1989, 27, 429–446. [Google Scholar] [CrossRef]
- Hager, W. Hydraulic jump in non-prismatic rectangular channels. J. Hydraul. Res. 1985, 23, 21–35. [Google Scholar] [CrossRef]
- Lawson, J.D.; Phillips, B.C. Circular hydraulic jump. J. Hydraul. Eng. 1983, 109, 505–518. [Google Scholar] [CrossRef]
- Arbhabhirama, A.; Abella, A.U. Hydraulic jump within gradually expanding channel. J. Hydraul. Div. 1971, 97, 31–42. [Google Scholar]
- Diskin, M. Hydraulic jump in trapezoidal channels. Water Power 1961, 13, 12–17. [Google Scholar]
- Omid, M.; Esmaeeli Varaki, M.; Narayanan, R. Gradually expanding hydraulic jump in a trapezoidal channel. J. Hydraul. Res. 2007, 45, 512–518. [Google Scholar] [CrossRef]
- Herbrand, K. The spatial hydraulic jump. J. Hydraul. Res. 1973, 11, 205–218. [Google Scholar] [CrossRef]
- Agarwal, V. Graphical solution to the problem of sequent depth and energy loss in spatial hydraulic jump. In Proceedings of the Institution of Civil Engineers-Water and Maritime Engineering; Thomas Telford Ltd.: London, UK, 2001. [Google Scholar]
- Carollo, F.G.; Ferro, V.; Pampalone, V. Hydraulic jumps on rough beds. J. Hydraul. Eng. 2007, 133, 989–999. [Google Scholar] [CrossRef]
- Izadjoo, F.; Shafai Bejestan, M. Corrugated bed hydraulic jump stilling basin. Appl. Sci. 2007, 7, 1164–1169. [Google Scholar]
- Bejestan, M.S.; Neisi, K. A new roughened bed hydraulic jump stilling basin. Asian J. Appl. Sci. 2009, 2, 436–445. [Google Scholar] [CrossRef]
- Wang, H.; Chanson, H. Turbulent fluctuations in hydraulic jumps: A physical study. In Proceeding of the 5th International Symposium on Hydraulic Structures: Hydraulic Structures and Society-Engineering Challenges and Extremes, Brisbane, Australia, 25–27 June 2014. [Google Scholar]
- Carollo, F.G.; Ferro, V.; Pampalone, V. New Expression of the Hydraulic Jump Roller Length. J. Hydraul. Eng. 2012, 138, 995–999. [Google Scholar] [CrossRef]
- Hager, W.H.; Bremen, R.; Kawagoshi, N. Classical hydraulic jump: Length of roller. J. Hydraul. Res. 1990, 28, 591–608. [Google Scholar] [CrossRef]
- USBR. Research Studies on Stilling Basins, Energy Dissipators and Associated Appurtenances; Hydraulic Laboratory Report (USBR- United States); USBR: Washington, DC, USA, 1955; Volume 399, pp. 393–438.
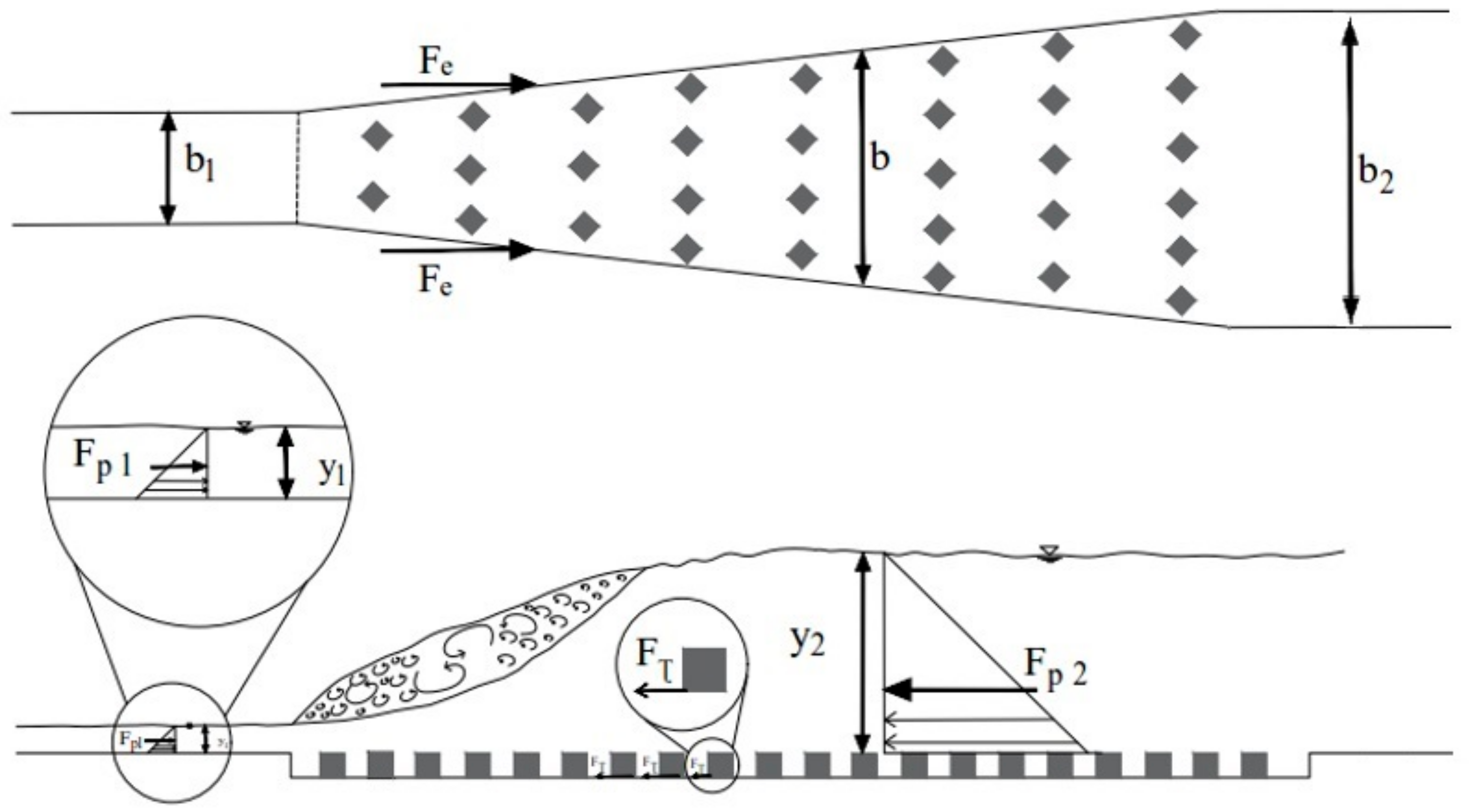
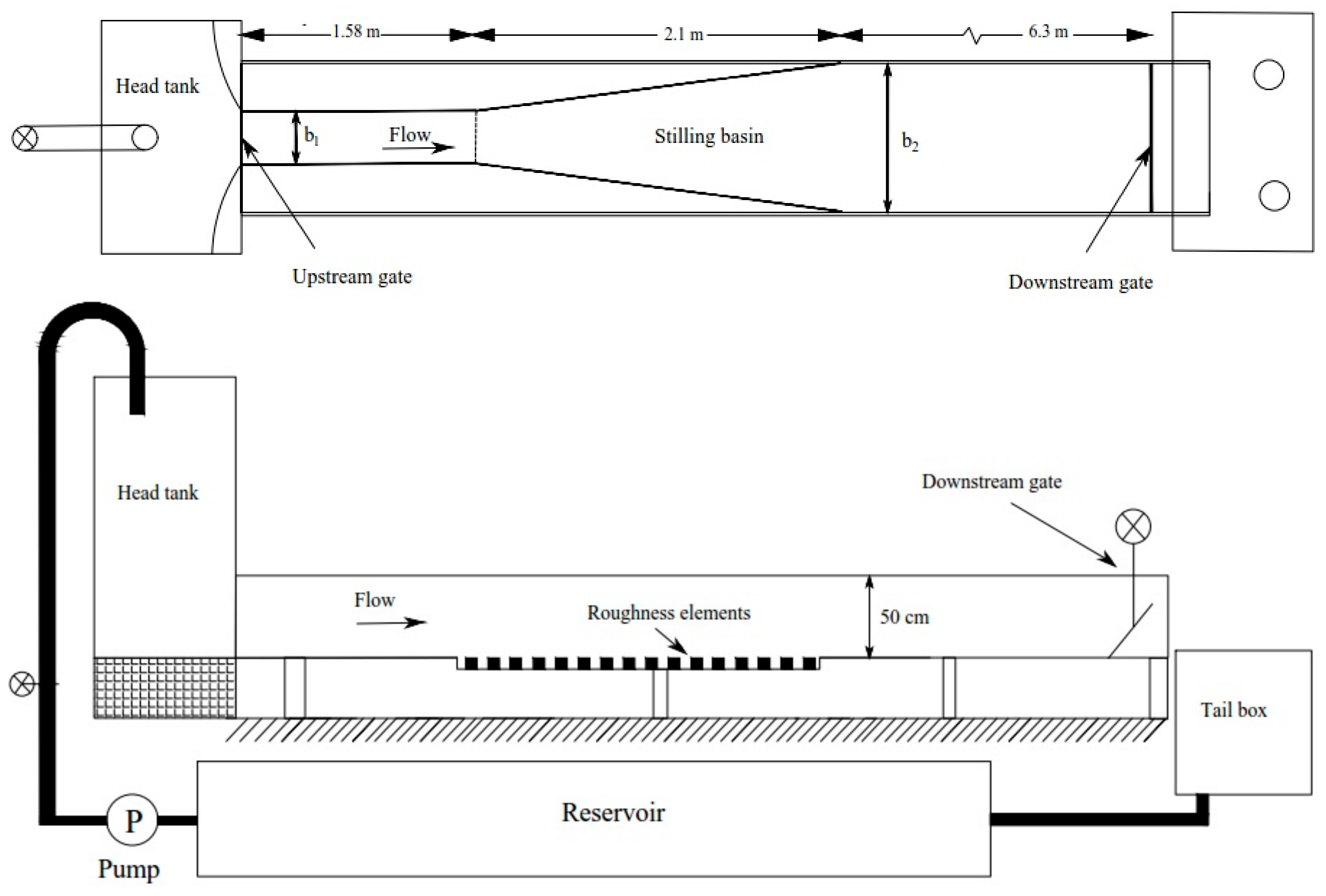



| Reference | Cross Section and Change Bed | Flume Dimension (m) | Roughness (mm) | Range of Inflow Froude Number | Investigated Flow Properties |
|---|---|---|---|---|---|
| Hughes and Flack [19] | - Horizontal bed - Rectangular channel - Smooth and rough bed | FL = 2.13 FW = 0.3 | - Two striproughness beds (RH: 3.2 and 6.4) - Three densely packed gravel beds (RH: 4.3–11.3) | 3.44–8.04 2.34–10.5 | - Discharge - Upstream depth - Tailwater depth - Jump length - Roller length - Velocity |
| Wanoschek and Hager [23] | - Horizontal Bed - Prismatic symmetrical trapezoidal - Side slope: 45° (m = 1) | FL = 8 FW = 0.22–1.60 FH = 0.7 | - Smooth Bed | 5.45–13 | - Discharge - Upstream depth - Tailwater depth - Jump length - Velocity |
| Ead and Rajaratnam [18] | - Horizontal bed - Rectangular channel - Smooth and rough bed | FL = 7.6 FW = 0.44 FH = 0.6 | - Corrugated aluminum Sheets (RH = 13 and 22) | 4–10 | - Discharge - Upstream depth - Tailwater depth - Jump length - Roller length - Velocity - Water surface profile |
| Carollo et al. [31] | - Horizontal bed - Rectangular channel - Smooth and rough bed | FL = 1.4 FW = 0.6 FH = 0.6 | - Crashed gravel particles (d50: 4.6, 8.2, 14.6, 23.9, 32) | 1.1–9.9 | - Upstream depth - Tailwater depth - Jump length - Roller length - Velocity |
| Izadjoo and Shafai Bejestan [32] | - Horizontal bed - Two rectangular channel - Smooth and rough bed | FL = 1.2, 9 FW = 0.25, 0.5 FH = 0.4 | - Wooden baffle with trapezoidal cross section (RH: 13, 26) | 6–12 | - Upstream depth - Tailwater depth - Jump length - Velocity |
| Omid Esmaeeli Varaki [28] | - Horizontal bed - Gradually expanding channel - Trapezoidal cross section - Side slope (m): 0.5:1, 1:1, 1.5:1 - Divergence angle (ϴ): 3°, 5°, 7°, 9° | FL = 10 FW = 0.5 | - Smooth Bed | 2.99–9.83 | - Upstream depth - Tailwater depth - Jump length |
| Bejestan and Neisi [33] | - Horizontal bed - Rectangular channel - Smooth and rough bed | FL = 7.5 FW = 0.35 FH = 0.5 | - Lozenge shape rough element (RH: 16) | 4.5–12 | - Discharge - Upstream depth - Tailwater depth - Jump length - Roller length - Velocity |
| Carollo Ferro [3] | - Horizontal bed - Rectangular channel - Smooth and rough bed | FL = 4.9 FW = 0.3 FH = 0.24 | - Crushed gravel particles cemented (d50: 5.6, 9.9, 15.3, 19) | 1.7–6.9 | - Discharge - Upstream depth - Tailwater depth |
| Pagliara Lotti [21] | - Horizontal bed - Rectangular channel - Smooth and rough bed | FL = 6 FW = 0.35 FH = 0.5 | - Homogeneous and non-homogeneous sediments, gravel (d50: 6.26–45.6) - Rough bed withboulders, metallic | 2.2–12.2 | - Discharge - Upstream depth - Tailwater depth - Jump length - Roller length - Velocity - Water surface profile |
| Wang and Chanson [34] | - Horizontal bed - Rectangular channel | FL = 3.2 FW = 0.5 FH = 0.41 | - Smooth Bed | 3.8–7.5 | - Discharge - Upstream depth - Tailwater depth - Jump length - Roller length - Velocity - Air concentrations - Bubbles frequency - interfacial velocity - turbulence intensity |
| Experiments | b1 (m) | b (m) | b2 (m) | q (m2/s) | r (m) | Fr1 | Re1*106 | y1 (m) | V1 (m/s) | y2 (m) | Lj (m) |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 0.5 | 0.5 | 0.5 | 0.0700–0.1169 | Smooth bed | 7.4–12.4 | 0.065–0.109 | 0.021 | 3.368–5.622 | 0.174–0.298 | 1.05–2.06 |
| 2 | 0.5 | 0.5 | 0.5 | 0.0701–0.1174 | 0.014 | 7.4–12.4 | 0.065–0.108 | 0.021 | 3.338–5.592 | 0.156–0.237 | 0.9–1.75 |
| 3 | 0.5 | 0.5 | 0.5 | 0.0705–0.1173 | 0.028 | 7.3–12.3 | 0.064–0.108 | 0.021 | 3.322–5.584 | 0.151–0.233 | 0.8–1.65 |
| 4 | 0.4 | 0.44–0.50 | 0.5 | 0.0619–0.1071 | Smooth bed | 6.4–11.3 | 0.056–0.097 | 0.021 | 2.946–5.098 | 0.147–0.249 | 0.8–2.3 |
| 5 | 0.4 | 0.433–0.493 | 0.5 | 0.0619–0.1071 | 0.014 | 6.4–11.3 | 0.056–0.097 | 0.021 | 2.948–5.101 | 0.127–0.219 | 0.65–1.85 |
| 6 | 0.4 | 0.428-0.48 | 0.5 | 0.0607–0.1076 | 0.028 | 6.4–11.3 | 0.055–0.097 | 0.021 | 2.889–5.125 | 0.115–0.2 | 0.57–1.6 |
| 7 | 0.3 | 0.393–0.50 | 0.5 | 0.0601–0.1076 | Smooth bed | 6.3–11.3 | 0.053–0.094 | 0.021 | 2.864–5.123 | 0.136–0.235 | 0.95–2.2 |
| 8 | 0.3 | 0.385–0.461 | 0.5 | 0.0615–0.1070 | 0.014 | 6.4–11.2 | 0.054–0.094 | 0.021 | 2.928–5.097 | 0.13–0.207 | 0.87–1.65 |
| 9 | 0.3 | 0.378–0.451 | 0.5 | 0.0610–0.1074 | 0.028 | 6.4–11.3 | 0.053–0.094 | 0.021 | 2.906–5.115 | 0.126–0.196 | 0.8–1.55 |
| 10 | 0.2 | 0.324–0.432 | 0.5 | 0.0612–0.1090 | Smooth bed | 6.3–11.3 | 0.050–0.090 | 0.021 | 2.916–5.192 | 0.136–0.221 | 0.85–1.6 |
| 11 | 0.2 | 0.302–0.396 | 0.5 | 0.0611–0.1084 | 0.014 | 6.4–11.2 | 0.050–0.089 | 0.021 | 2.910–5.160 | 0.129–0.193 | 0.7–1.35 |
| 12 | 0.2 | 0.284–0.367 | 0.5 | 0.0603–0.1070 | 0.028 | 6.4–11.3 | 0.050–0.088 | 0.021 | 2.872–5.097 | 0.119–0.183 | 0.58–1.15 |
| Experiments | D% |
|---|---|
| B = 1, r/y1 = 0.67 | 16.38 |
| B = 1, r/y1 = 1.33 | 17.88 |
| B = 0.8, r/y1 = 0.67 | 18.69 |
| B = 0.8, r/y1 = 1.33 | 23.56 |
| B = 0.6, r/y1 = 0.67 | 19.59 |
| B = 0.6, r/y1 = 1.33 | 23.67 |
| B = 0.4, r/y1 = 0.67 | 22.92 |
| B = 0.4, r/y1 = 1.33 | 27.28 |
| Experimental Investigation | r (cm) | B | a | a0 | b0 |
|---|---|---|---|---|---|
| Present Study | 0 | 1 | 5.24 | 2.44 | 6.02 |
| CarolloFerro [31] | 0 | 1 | 4.12 | 2.04 | 5.73 |
| Hughes and Flack [19] | 0 | 1 | 5.06 | 2.42 | 6.58 |
| Present Study | 0 | 0.8 | 5.14 | 2.48 | 5.59 |
| Present Study | 0 | 0.6 | 5.10 | 2.48 | 5.2 |
| Present Study | 0 | 0.4 | 5.07 | 2.48 | 4.84 |
| Hughes and Flack [19] | 0.32 | 1 | 4.53 | 2.25 | 5.9 |
| CarolloFerro [31] | 0.46 | 1 | 4.26 | 2.15 | 5.02 |
| Hughes and Flack [19] | 0.49 | 1 | 4.66 | 2.33 | 6.00 |
| Hughes and Flack [19] | 0.61 | 1 | 4.06 | 2.00 | 4.92 |
| Hughes and Flack [19] | 0.64 | 1 | 4.43 | 2.22 | 5.44 |
| CarolloFerro [31] | 0.82 | 1 | 3.92 | 1.98 | 4.67 |
| Hughes and Flack [19] | 1.04 | 1 | 4.07 | 2.01 | 4.79 |
| Present Study | 1.4 | 1 | 4.49 | 2.17 | 4.21 |
| Present Study | 1.4 | 0.8 | 4.85 | 2.39 | 4.39 |
| Present Study | 1.4 | 0.6 | 4.89 | 2.41 | 4.34 |
| Present Study | 1.4 | 0.4 | 4.40 | 2.20 | 3.67 |
| CarolloFerro [31] | 1.46 | 1 | 3.86 | 1.94 | 4.16 |
| Present Study | 2.8 | 1 | 4.08 | 1.98 | 3.724 |
| Present Study | 2.8 | 0.8 | 4.33 | 2.15 | 3.662 |
| Present Study | 2.8 | 0.6 | 4.55 | 2.27 | 3.789 |
| Present Study | 2.8 | 0.4 | 3.93 | 2.00 | 3.13 |
| Number of Equation | Equation Form | R2 | Note |
|---|---|---|---|
| 12 | 0.95 | This equation can be used for calculating sequent depth ratio in the gradually roughened stilling basin. | |
| 16 | 0.84 | This equation can be used for calculating relative roller length in the gradually roughened stilling basin. | |
| 17 | 0.86 | This equation can be used for calculating relative roller length in the gradually roughened stilling basin. | |
| 18 | 0.89 | This equation can be used for calculating the coefficient of b0 in Equation (12). | |
| 19 | 0.89 | This equation can be used for calculating relative roller length in the gradually roughened stilling basin. | |
| 22 | 0.89 | This equation can be used for calculating the relative length of the hydraulic jump in the gradually roughened stilling basin. | |
| 26 | 0.97 | This equation can be used for calculating the relative loss of energy in the gradually roughened stilling basin. |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hassanpour, N.; Hosseinzadeh Dalir, A.; Farsadizadeh, D.; Gualtieri, C. An Experimental Study of Hydraulic Jump in a Gradually Expanding Rectangular Stilling Basin with Roughened Bed. Water 2017, 9, 945. https://doi.org/10.3390/w9120945
Hassanpour N, Hosseinzadeh Dalir A, Farsadizadeh D, Gualtieri C. An Experimental Study of Hydraulic Jump in a Gradually Expanding Rectangular Stilling Basin with Roughened Bed. Water. 2017; 9(12):945. https://doi.org/10.3390/w9120945
Chicago/Turabian StyleHassanpour, Nasrin, Ali Hosseinzadeh Dalir, Davod Farsadizadeh, and Carlo Gualtieri. 2017. "An Experimental Study of Hydraulic Jump in a Gradually Expanding Rectangular Stilling Basin with Roughened Bed" Water 9, no. 12: 945. https://doi.org/10.3390/w9120945
APA StyleHassanpour, N., Hosseinzadeh Dalir, A., Farsadizadeh, D., & Gualtieri, C. (2017). An Experimental Study of Hydraulic Jump in a Gradually Expanding Rectangular Stilling Basin with Roughened Bed. Water, 9(12), 945. https://doi.org/10.3390/w9120945







