The Influence of Statistical Uncertainty in the Hydraulic Boundary Conditions on the Probabilistically Computed High Water Level Frequency Curve in the Rhine Delta
Abstract
:1. Introduction
2. Methods
2.1. Statistical Uncertainty in a Distribution
2.2. Uncertainty-Incorporated Distribution
2.3. Impact of the Uncertainty on the High Water Level Frequency in the Rhine Delta
3. Results
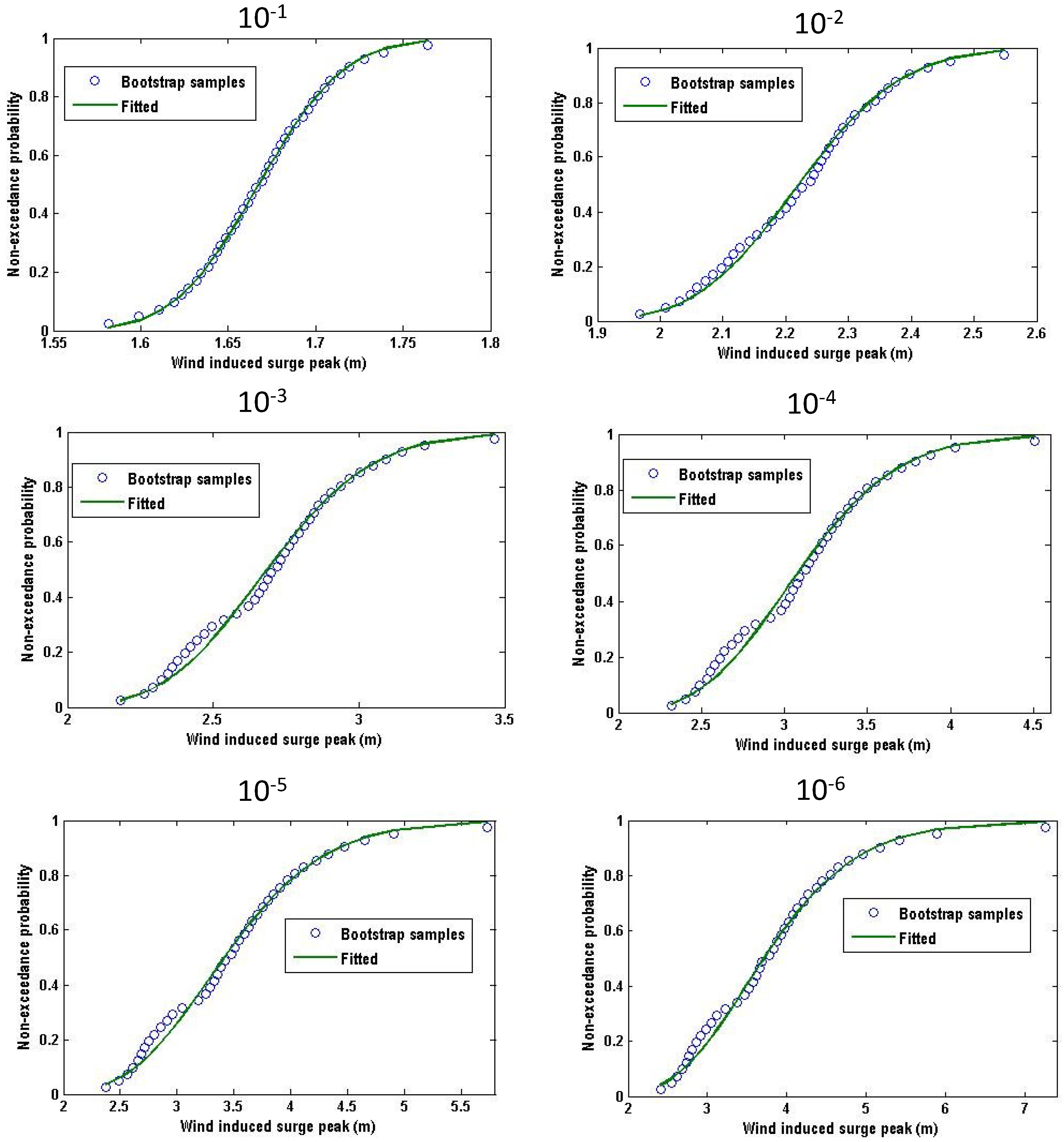
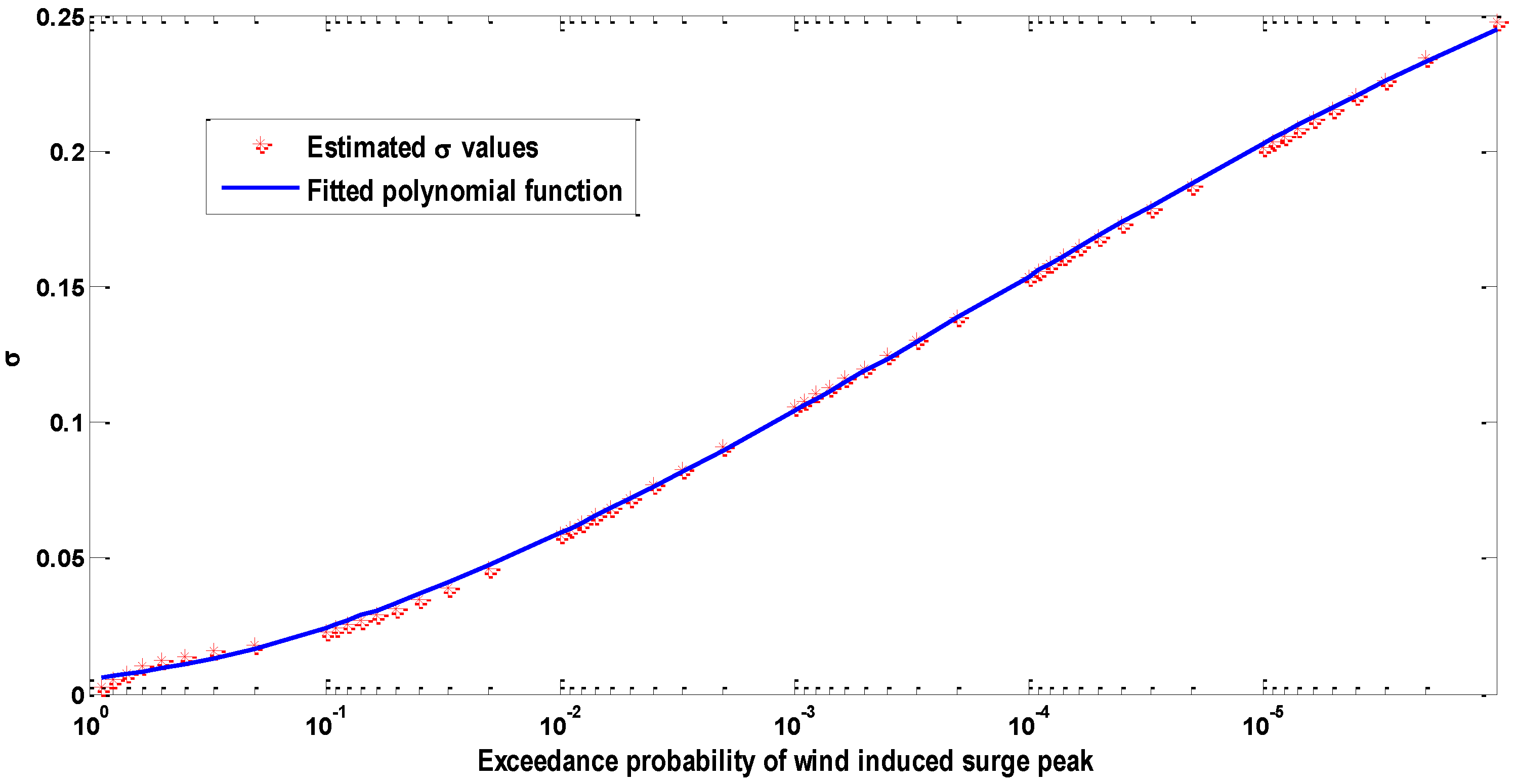
3.1. Statistical Uncertainty
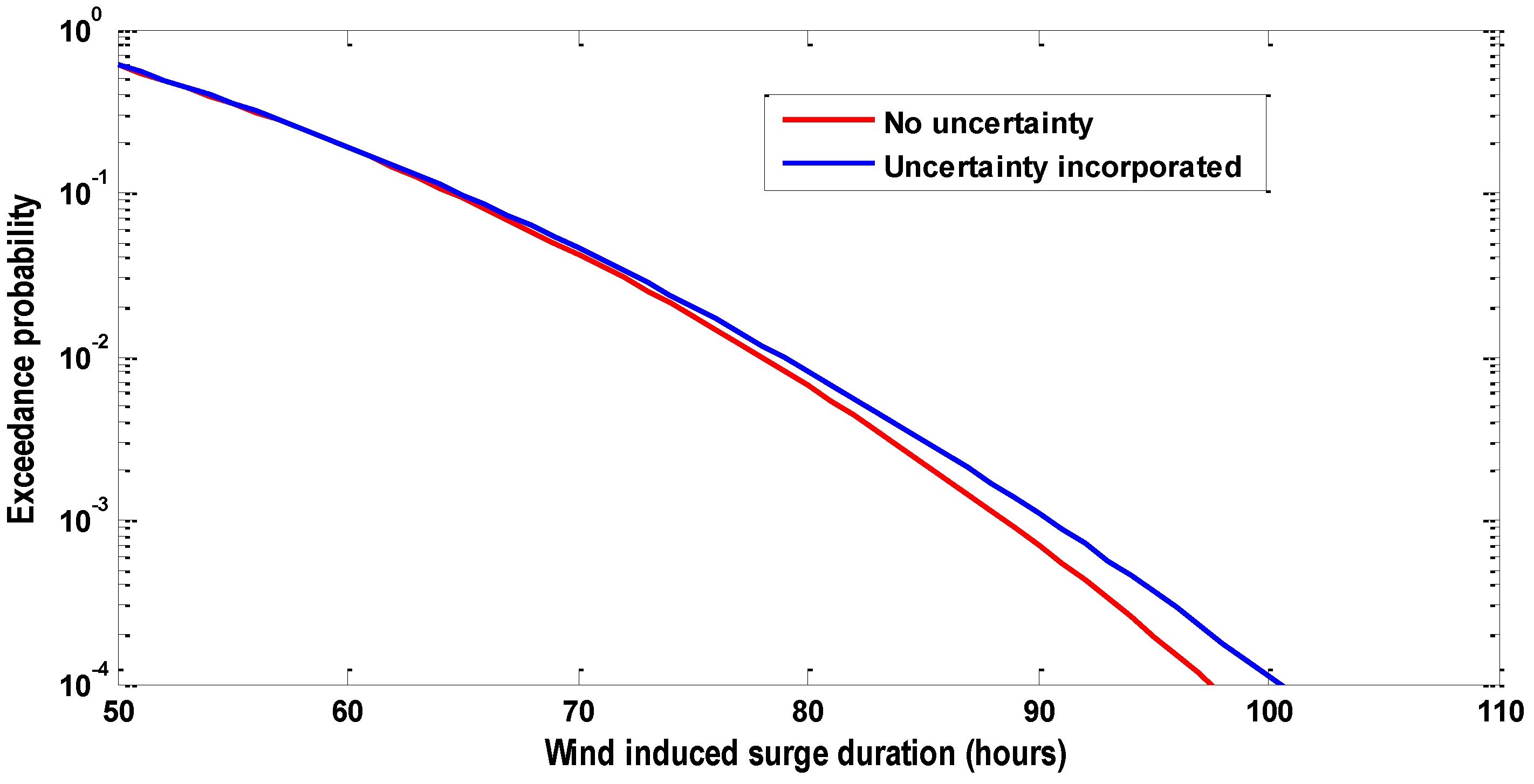
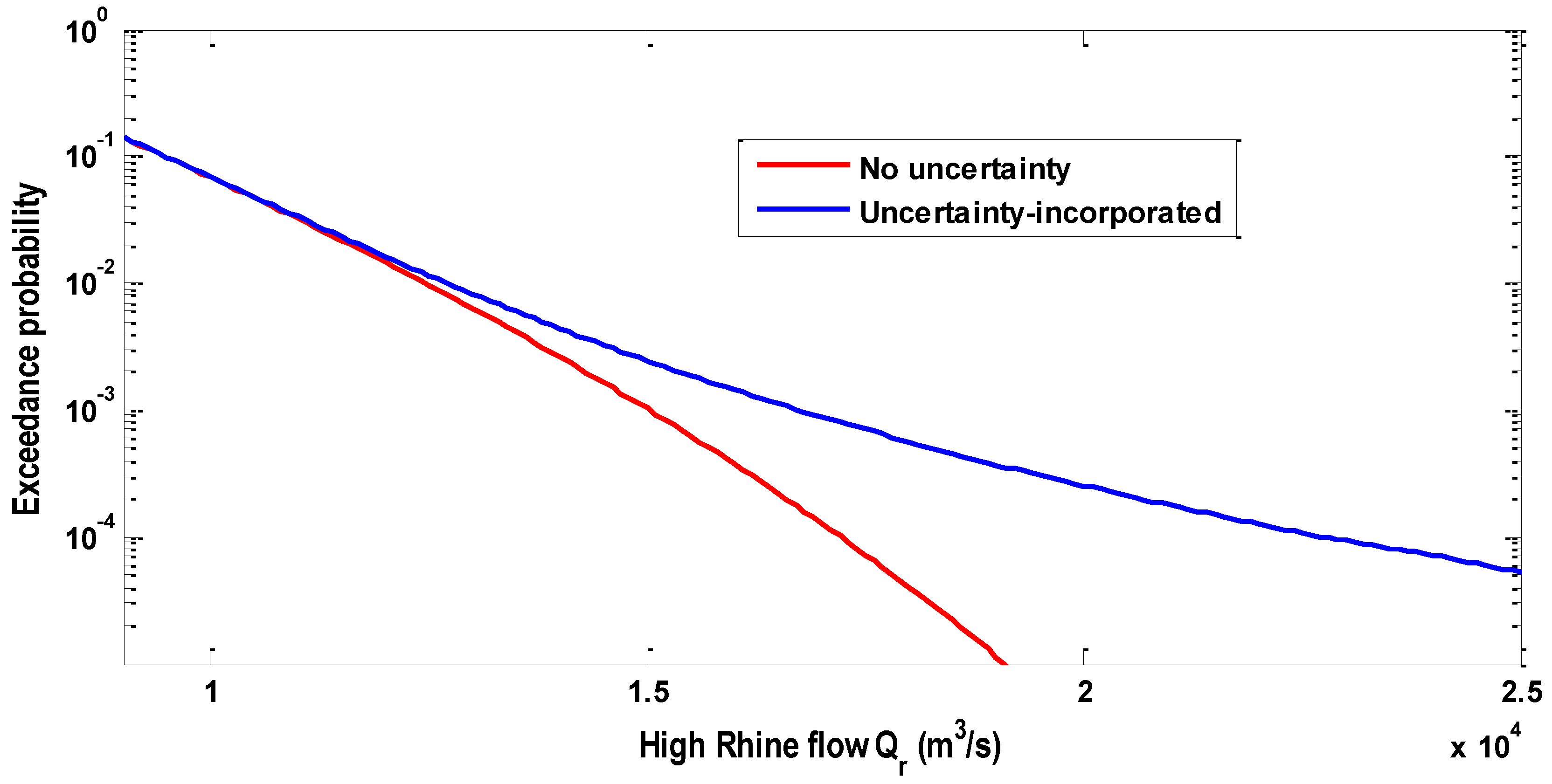
3.2. Uncertainty-Incorporated Marginal Distributions
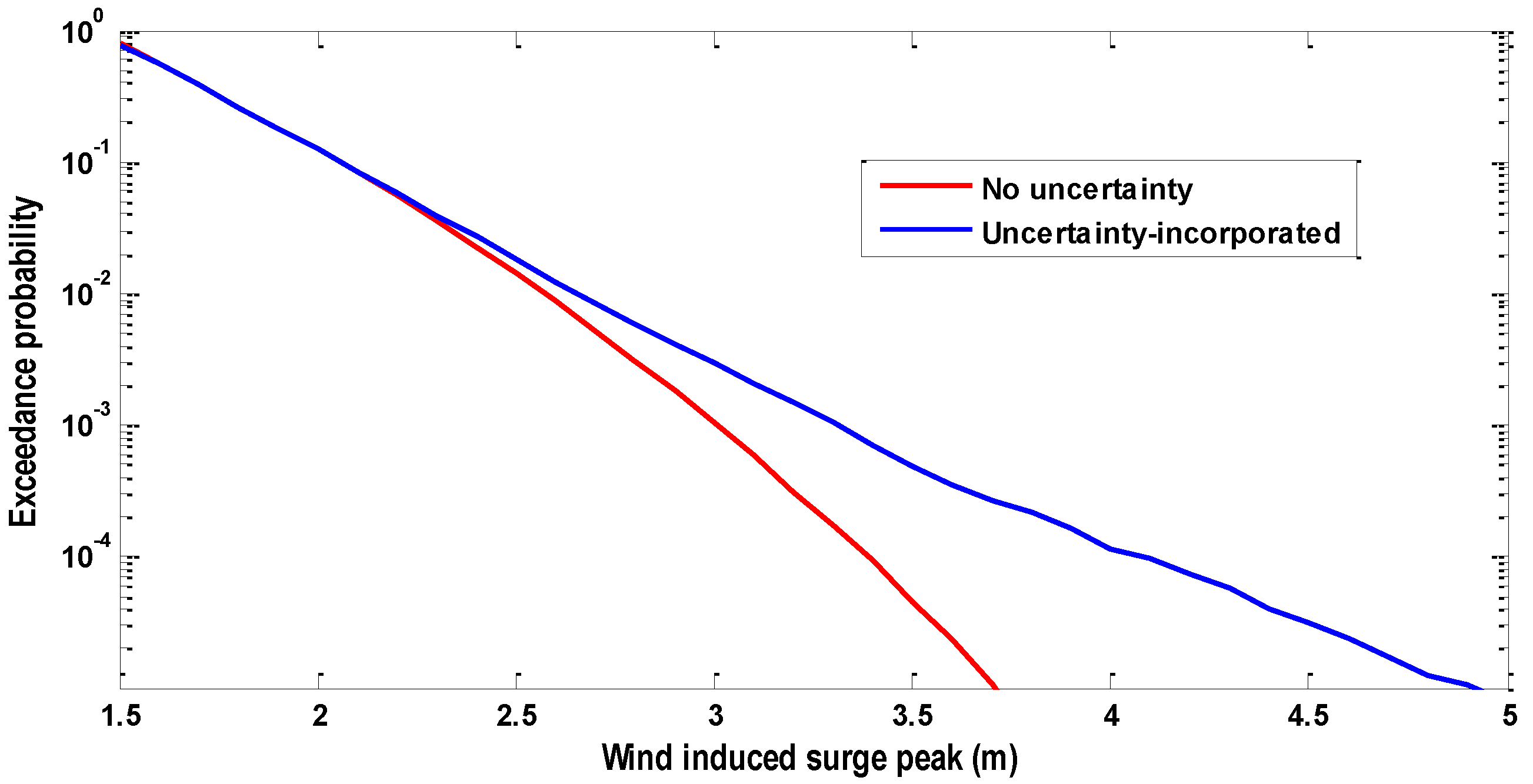
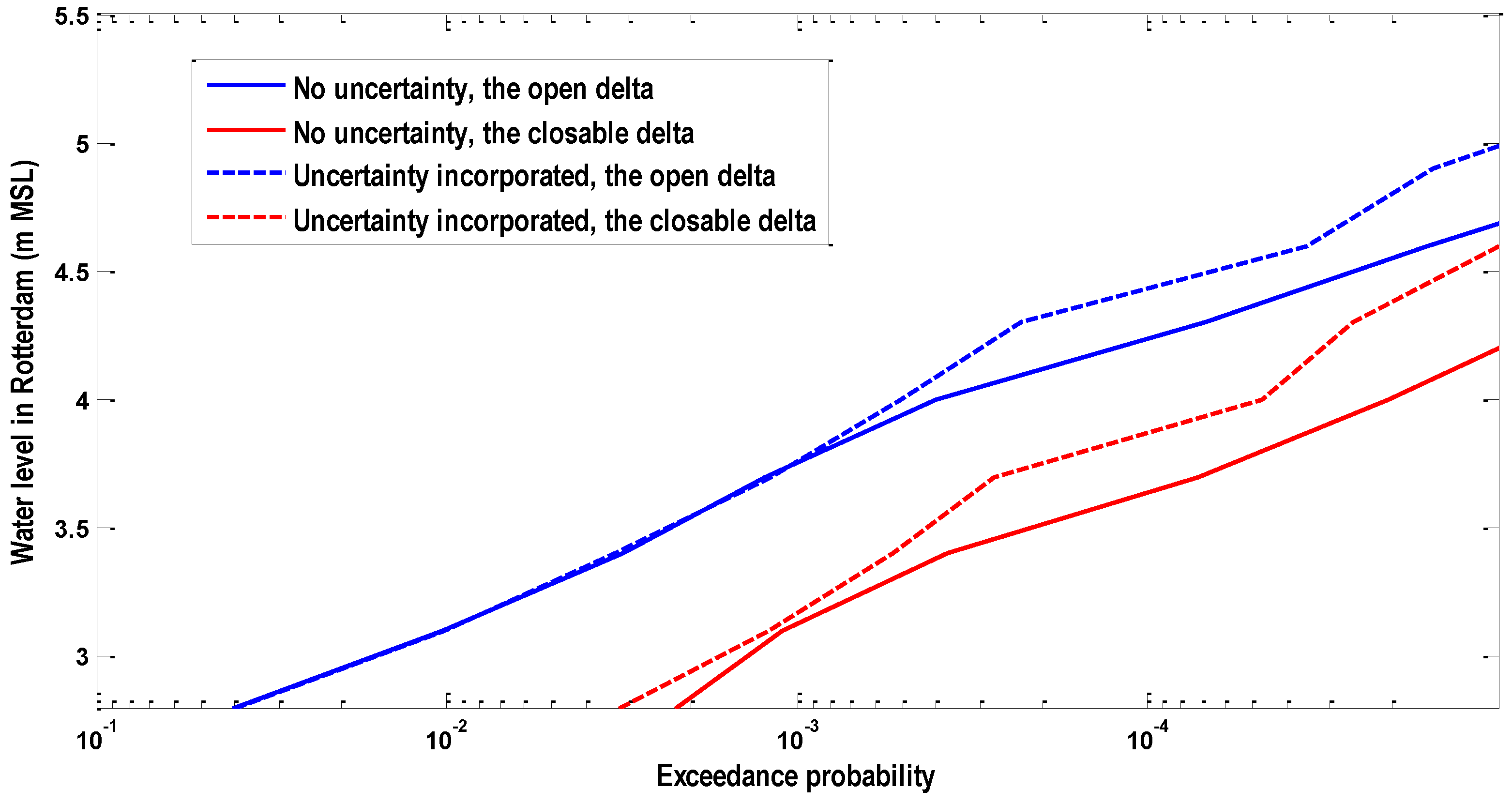
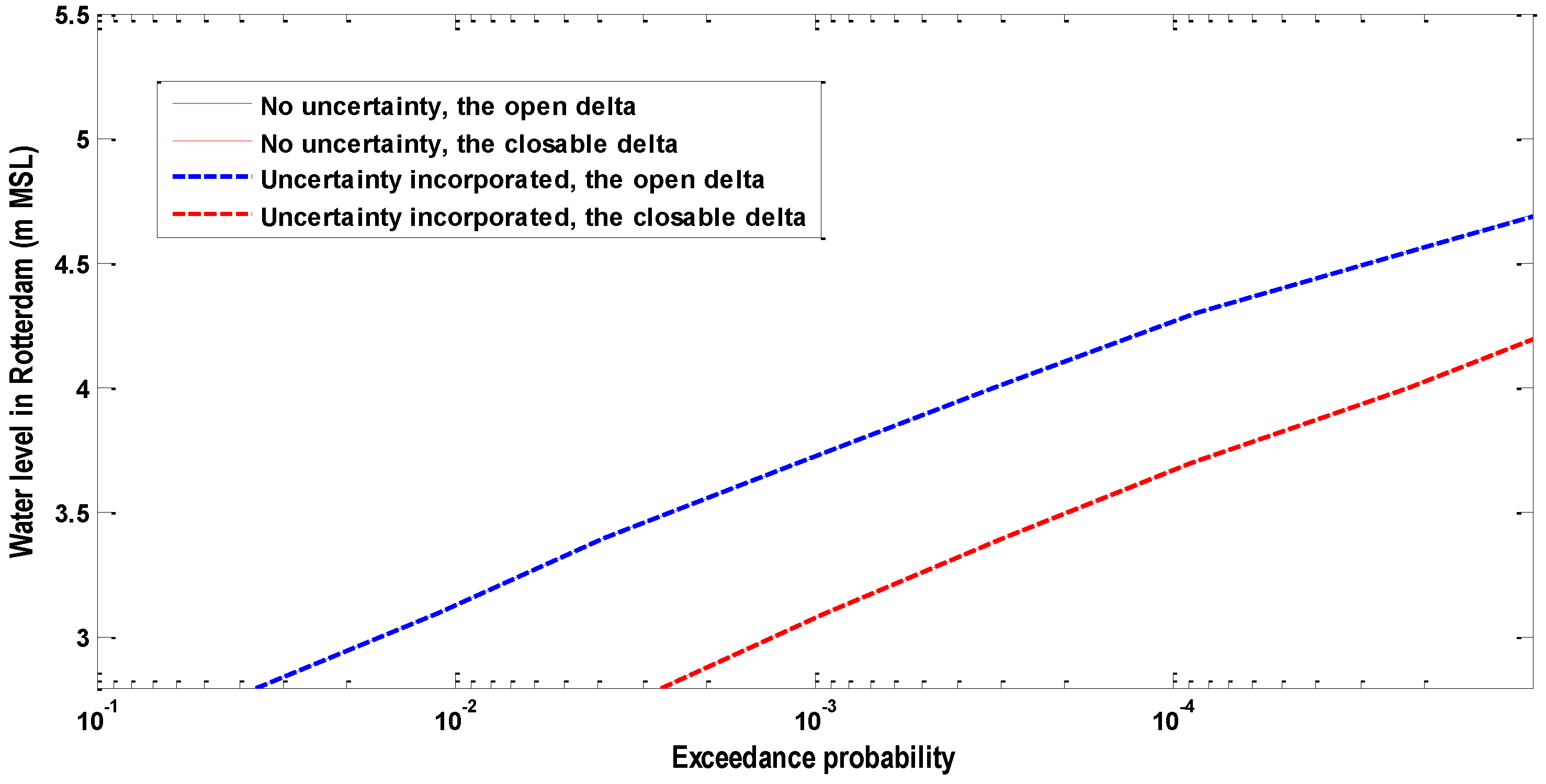
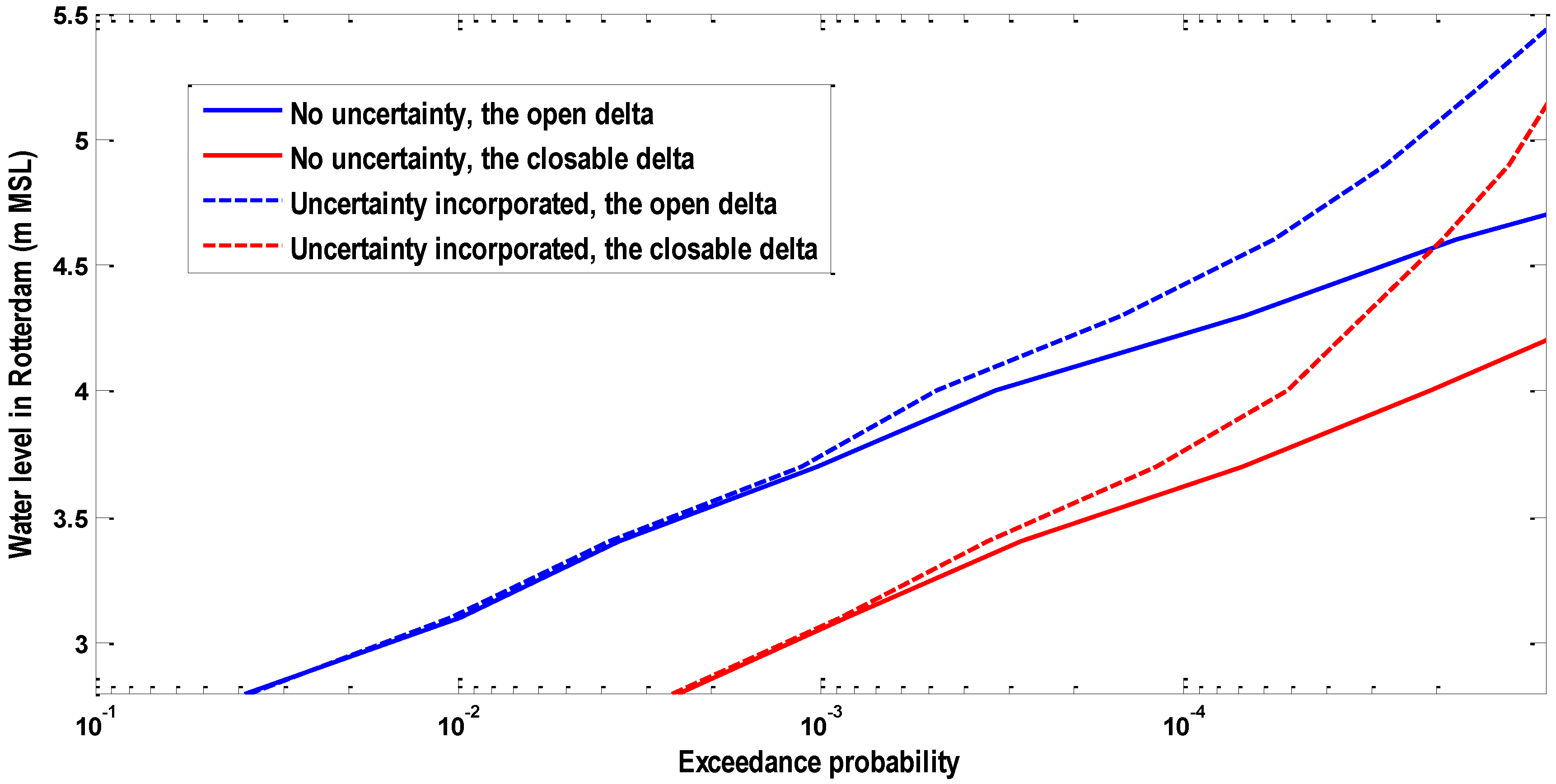
3.3. Impact on the High Water Level Frequency
4. Conclusions and Recommendations
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A
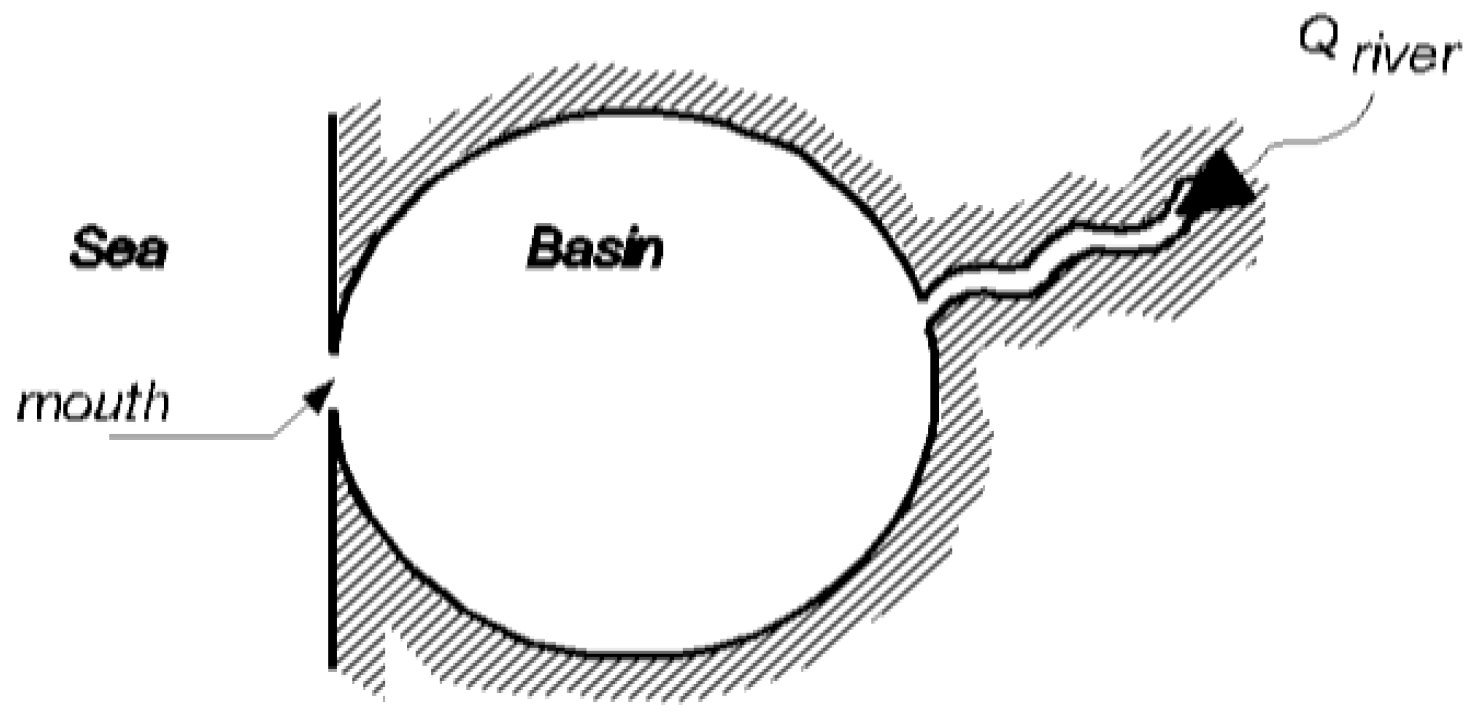
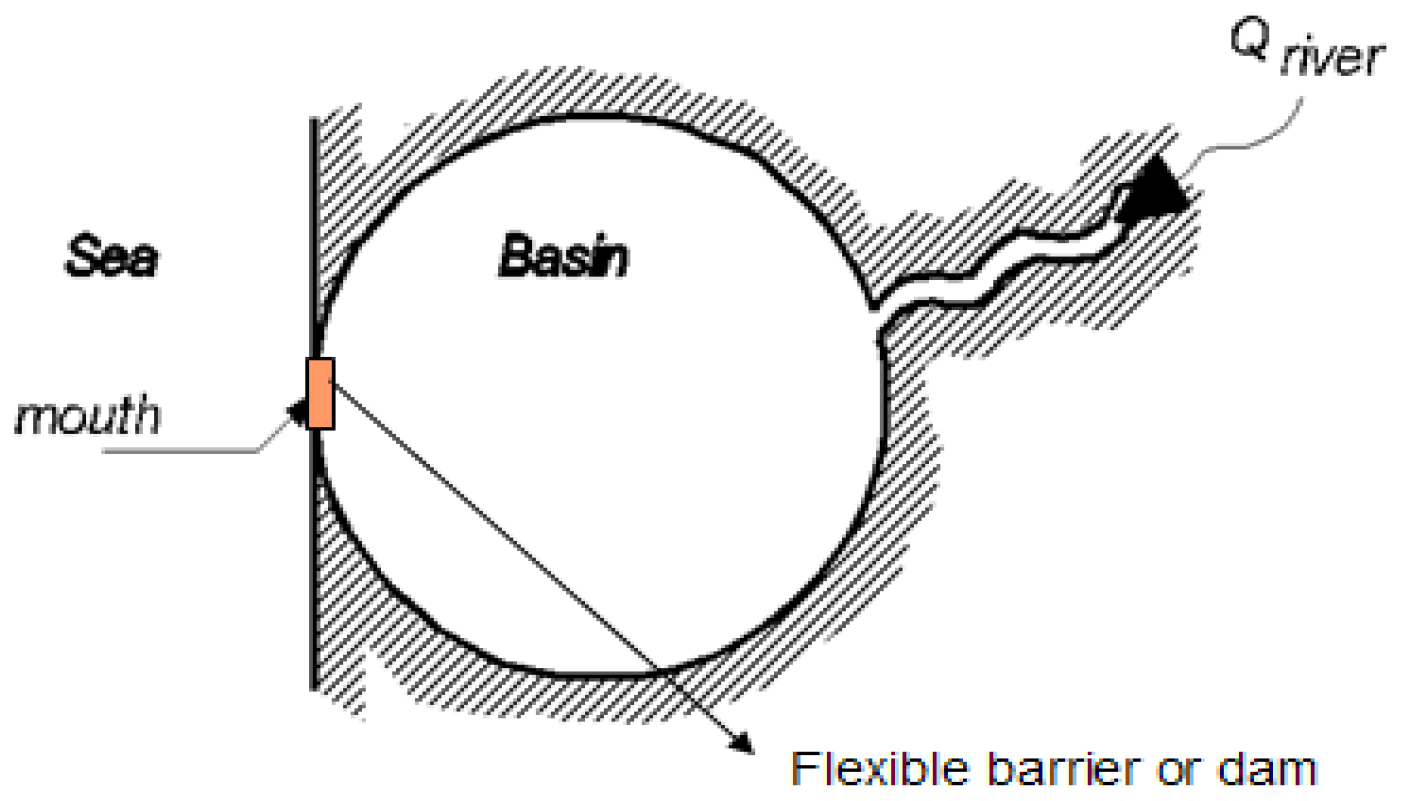
The Conceptual Model of the Rhine Delta
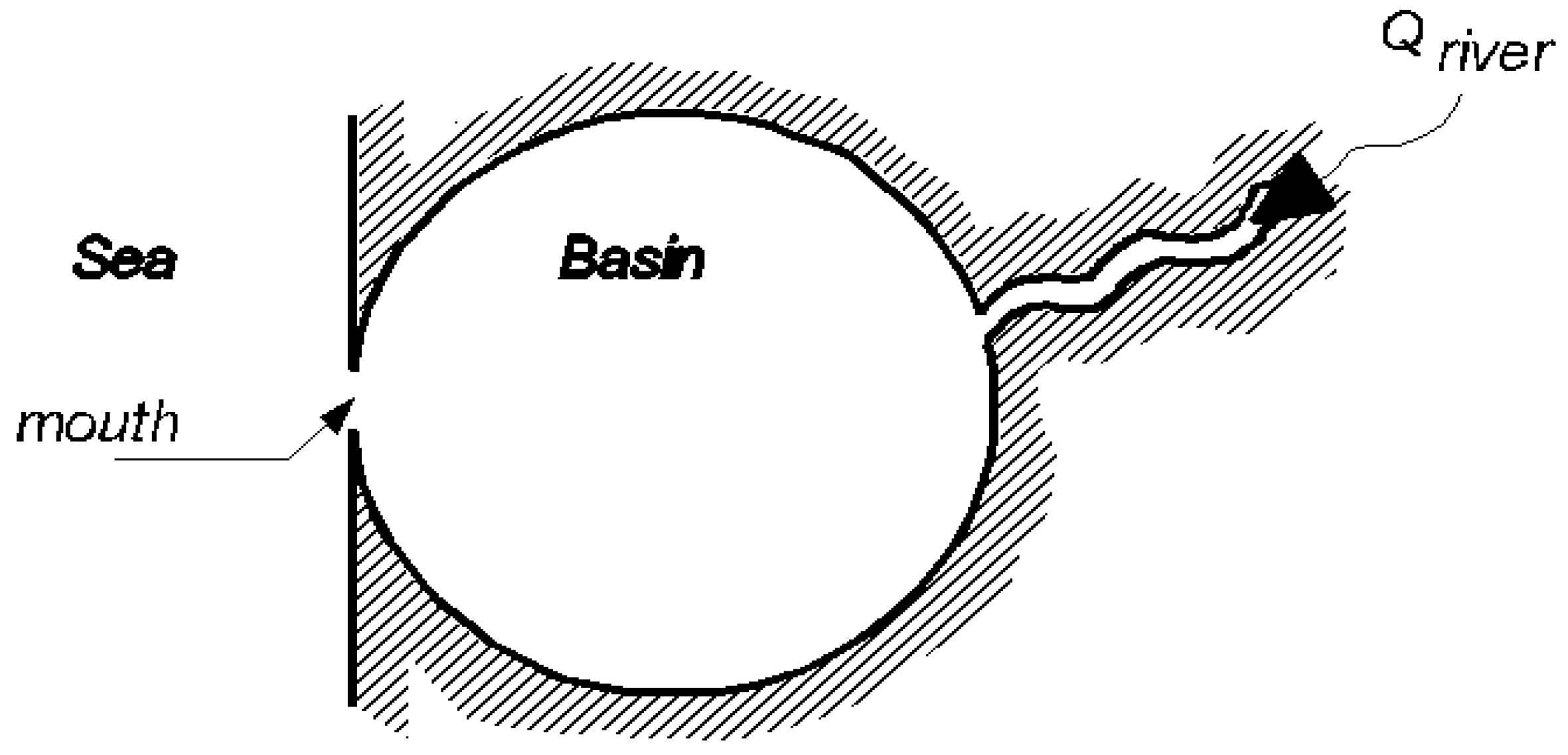
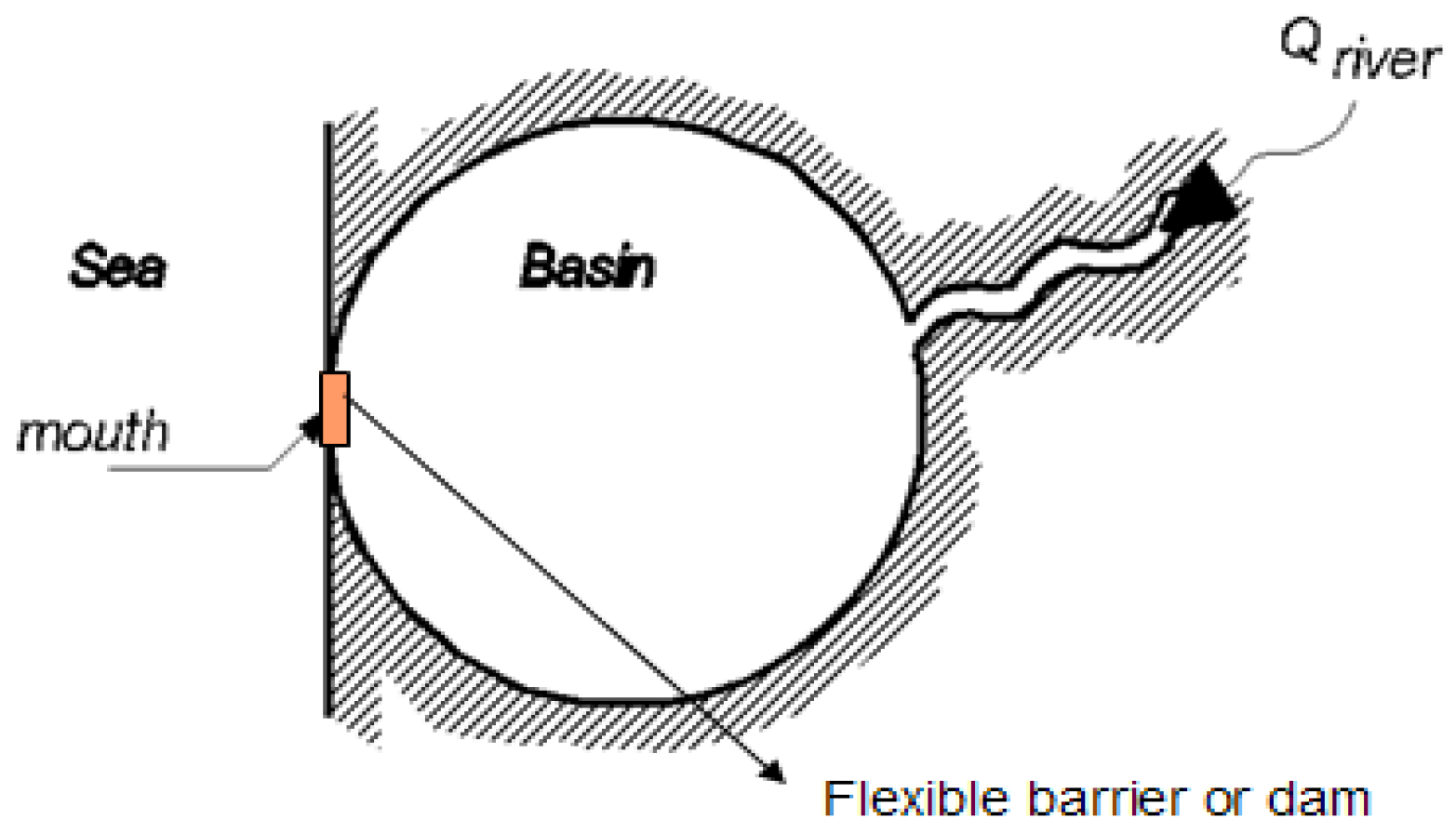
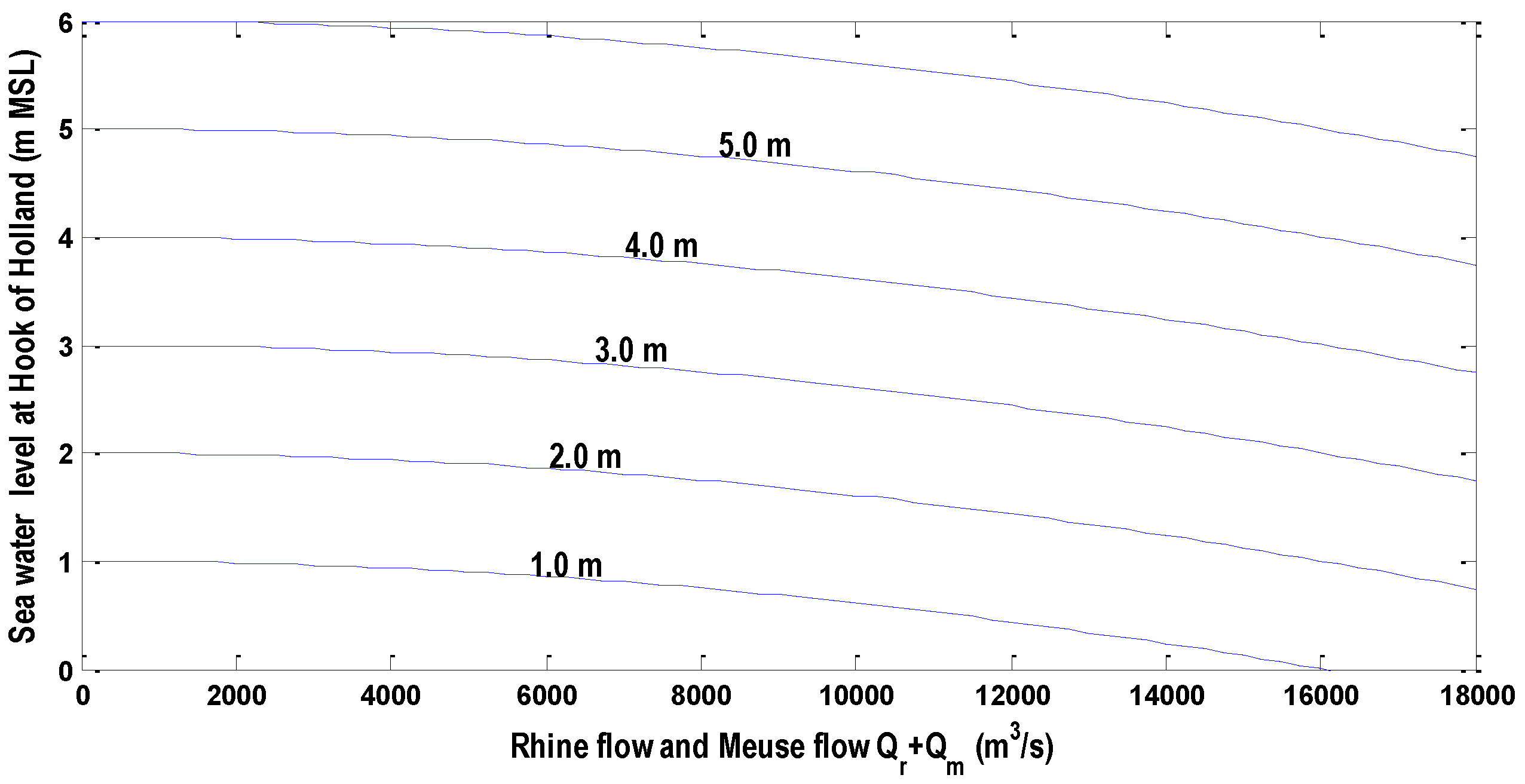
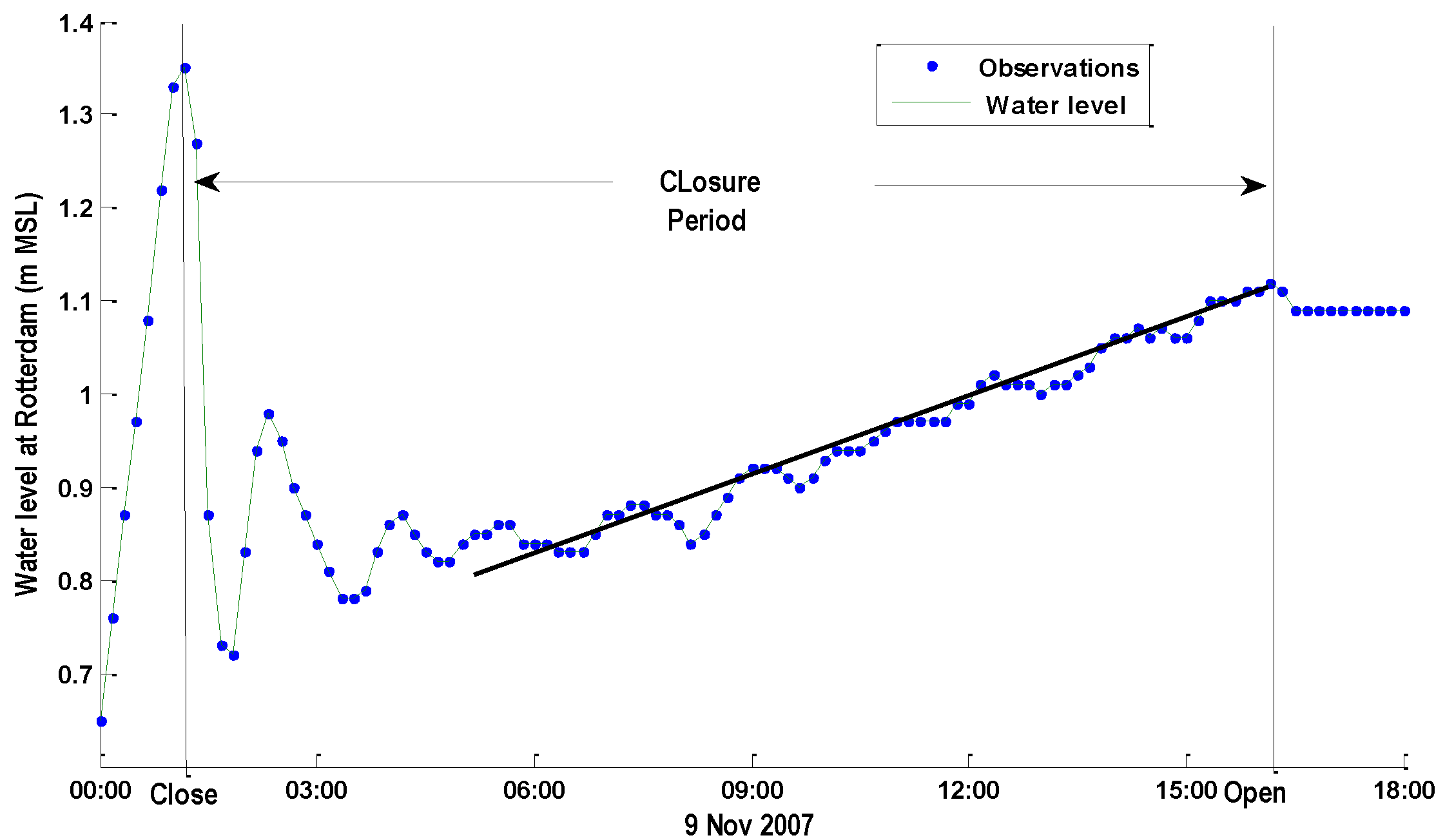
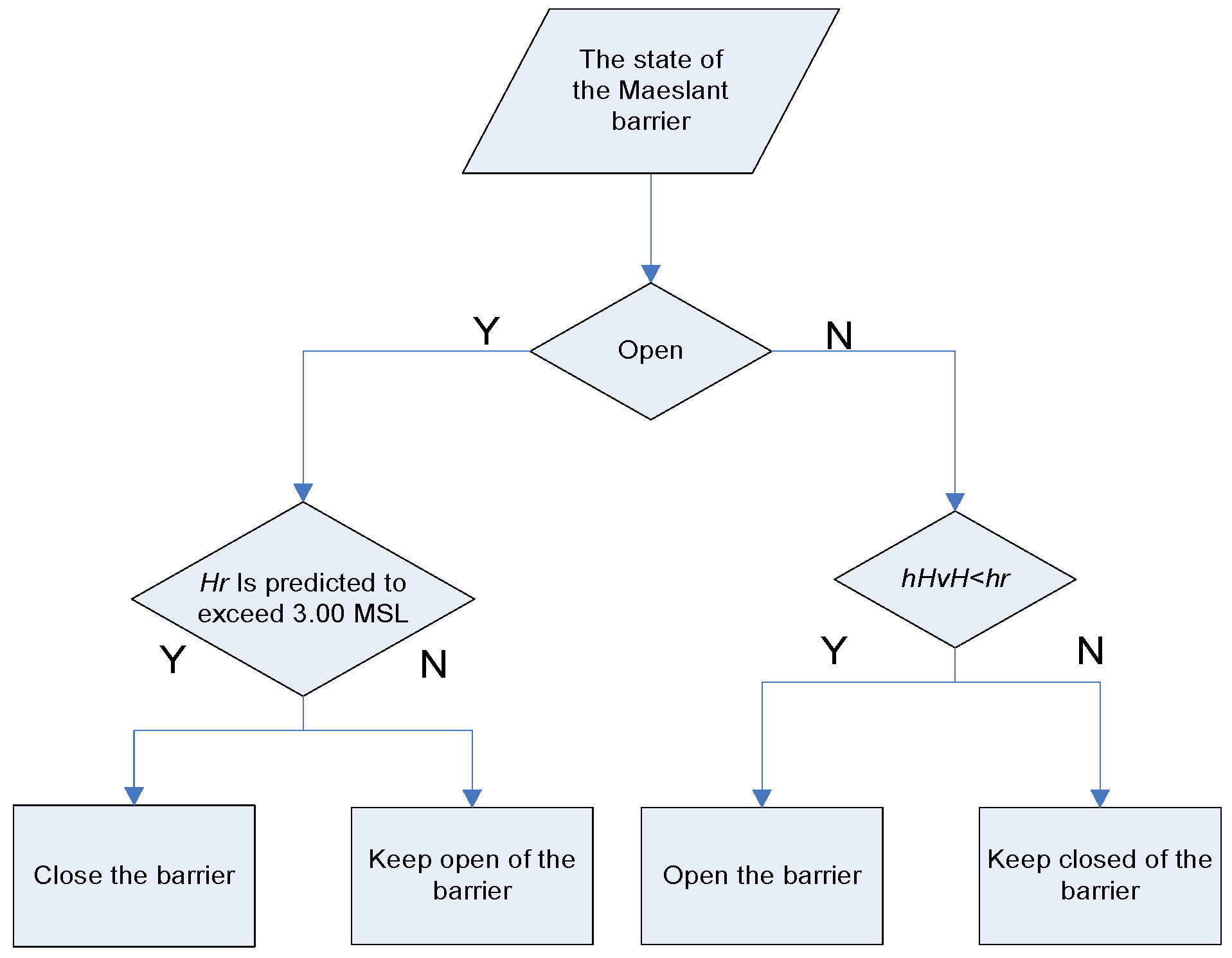
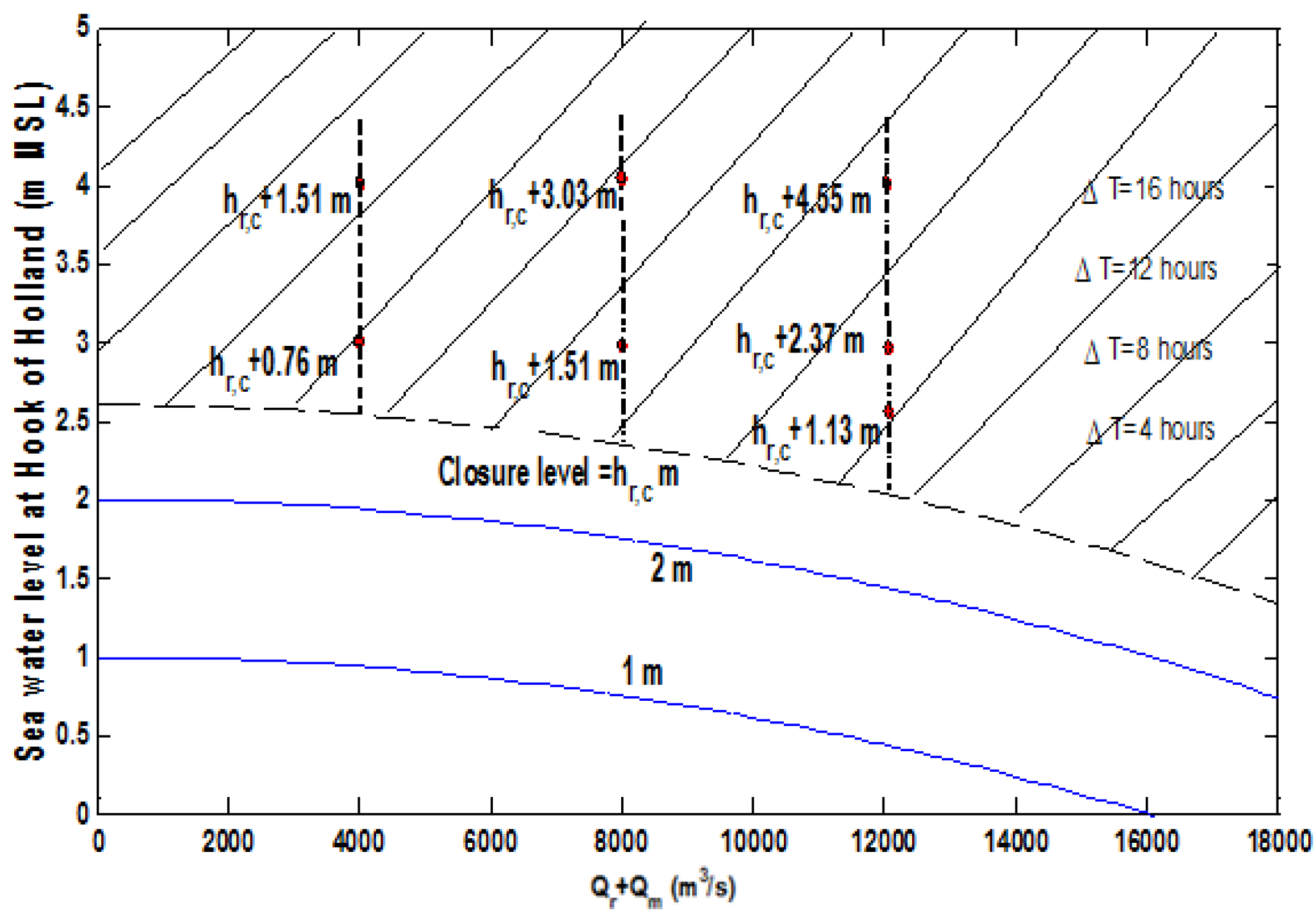
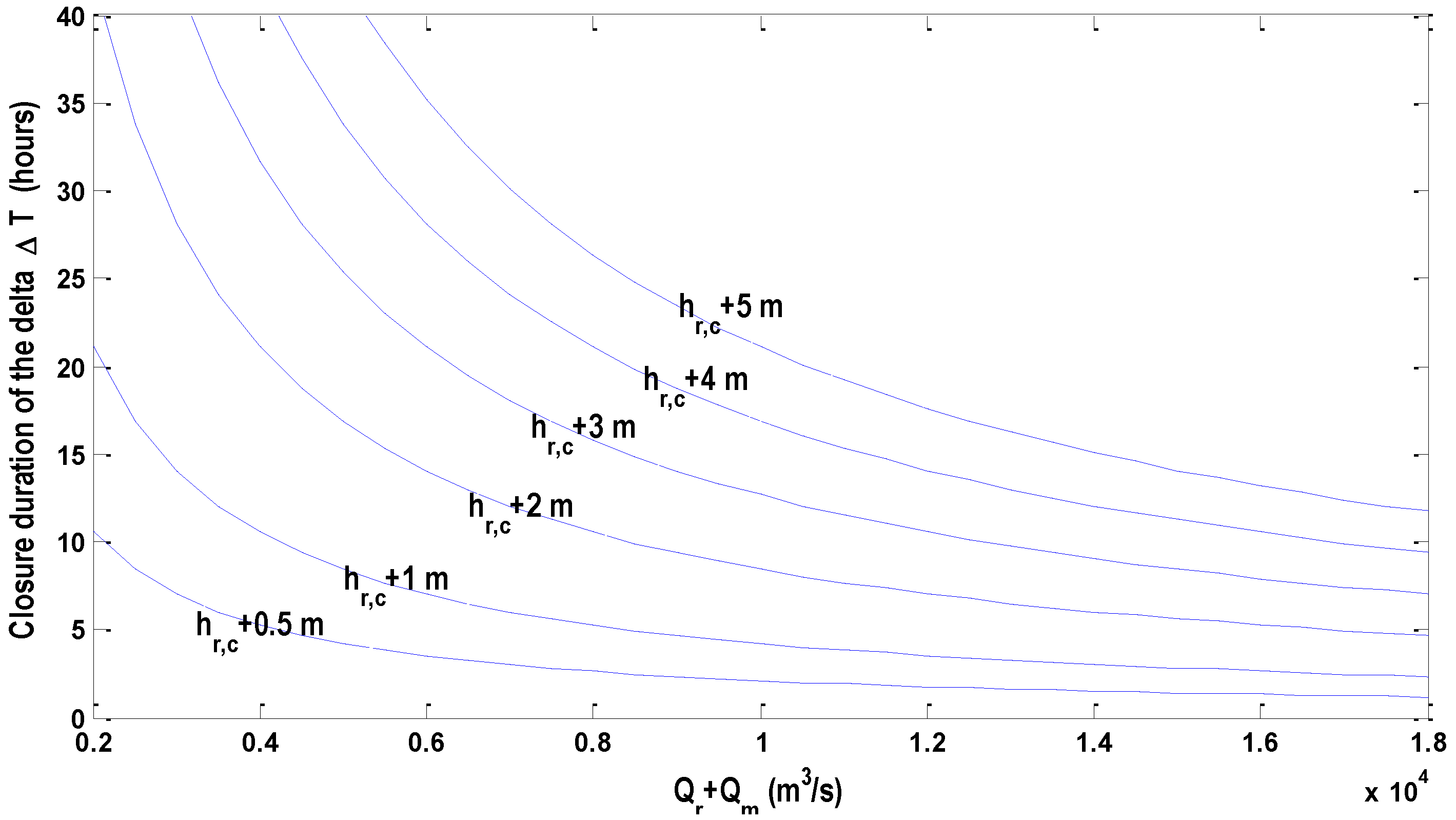
Appendix B
Probability Distributions
References
- Mantz, P.A.; Wakeling, H.L. Forecasting flood levels for jointevents of rainfall and tidal surge flooding using extreme valuestatistics. Proc. Institut. Civil Eng. Res. Theor. 1979, 67, 31–50. [Google Scholar] [CrossRef]
- Acreman, M.C. Assessing the joint probability of fluvial and tidalfloods in the River Roding. J. Inst. Water Environ. Manage. 1994, 8, 490–496. [Google Scholar] [CrossRef]
- Gorji-Bandpy, M. The joint probability method of determining theflood return period of a tidally affected pond, Iran. J. Sci. Technol. 2001, 25, 599–610. [Google Scholar]
- Samuels, P.G.; Burt, N. A new joint probability appraisal offlood risk. Proc. Institut. Civil Eng. Water Maritime Eng. 2002, 154, 109–115. [Google Scholar] [CrossRef]
- Adib, A.; Vaghefi, M.; Labibzadeh, M.; Rezaeian, A.; Tagavifar, A.; Foladfar, H. Predicting extreme water surface elevation intidal river reaches by different joint probability methods. J. FoodAgric. Environ. 2010, 8, 988–991. [Google Scholar]
- Lian, J.J.; Xu, K.; Ma, C. Joint impact of rainfall and tidal level on flood risk in a coastal city with a complex river network: A case study for Fuzhou city, China. Hydrol. Earth Syst. Sci. Discuss. 2012, 9, 7475–7505. [Google Scholar] [CrossRef]
- Zhong, H.; van Overloop, P.J.; van Gelder, P. A joint probability approach using a 1-d hydrodynamic model for estimating high water level frequencies in the lower Rhine delta. Nat. Hazard. Earth Syst. Sci. 2013, 13, 1841–1852. [Google Scholar] [CrossRef]
- Bruno, M.; Annegret, H.T. Separating natural and epistemic uncertainty in flood frequency analysis. J. Hydrol. 2005, 309, 114–132. [Google Scholar]
- Efron, B. Bootstrap methods: Another look at the jackknife. Ann. Statist. 1979, 7, 1–26. [Google Scholar] [CrossRef]
- Efron, B.; Tibschirani, R.J. An Introduction to the Bootstrap; Chapman&Hall: New York, NY, USA, 1993. [Google Scholar]
- Davison, A.C.; Hinkley, D.V. Bootstrap Methods and Their Application; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Dunn, P.K. Bootstrap confidence intervals for predicted rainfall quantiles. Int. J. Climatol. 2001, 21, 89–94. [Google Scholar] [CrossRef]
- Kysely, J. Coverage probability of bootstrap confidence intervals in heavy-tailed frequency models, with application to precipitation data. Theor. Appl. Climatol. 2010, 101, 345–361. [Google Scholar] [CrossRef]
- Roscoe, K.L.; Diermanse, F. Effect of surge uncertainty on probabilistically computed dune erosion. Coast. Eng. 2011, 58, 1023–1033. [Google Scholar] [CrossRef]
- Vrijling, J.K.; van Gelder, P.H.A.J.M. Probabilistic Design in Hydraulic Engineering; Delft University of Technology: Delft, The Netherlands, 1996. [Google Scholar]
- Zhong, H.; van Overloop, P.J.; van Gelder, P.; Rijcken, T. Influence of a storm surge barrier’s operation on the high water level frequency in the Rhine delta area. Water 2012, 4, 474–493. [Google Scholar] [CrossRef]
- van der Made, J. Design levels in the transition zone between the tidal reach and the river regime reach, hydrology of deltas. In Proceedings of the Bucharest Symosium, Bucharest, Rumania, 6–14 May 1969; pp. 246–257.
- Bol, R. Operation of the “Maeslant Barrier”: Storm surge barrier in the Rotterdam new waterway. In Flooding and Environmental Challenges for Venice and Its Lagoon: State of Knowledge; Cambridge University Press: Cambridge, UK, 2005; pp. 311–316. [Google Scholar]
- van Overloop, P.J. Operational Water Management of the Main Waters in the Netherlands; Delft Unversity of Technology: Delft, The Netherlands, 2009. [Google Scholar]
- van Overloop, P.J. Optimization of “Everything”, Prediction and Control of the entire Delta and River System of The Netherlands; Technical Report for Water INNovation (WINN); Delft University of Technology: Delft, The Netherlands, 2011. [Google Scholar]
- Dantzig, D.; Hemelrijk, J.; Kriens, J.; Lauwerier, H. Rapport Deltacommissie; Staatsdrukkerij En Uitgeverijbedrijf: 's-Gravenhage, The Netherlands, 1960. (In Dutch) [Google Scholar]
- Jorigny, M.; Diermanse, F.; Hassan, R.; van Gelder, P. Correlation analysis of water levels along dike-ring areas. Develop. Water Sci. 2002, 47, 1677–1684. [Google Scholar]
- Klerk, W.J.; Winsemius, H.C.; van Verseveld, W.J.; Bakker, A.M.R.; Diermanse, F.D. The co-incidence of storm surges and extreme discharges within the Rhine-Meuse Delta. Environ. Res. Lett. 2015, 10, 035005. [Google Scholar] [CrossRef]
- De Bruijn, K.M.; Klijn, F. Risky places in the netherlands: A first approximation for floods. J. Flood Risk Manag. 2009, 2, 58–67. [Google Scholar] [CrossRef]
- Ministerie van Verkeer en Waterstaat. Hydraulische Randvoorwaarden Primaire Waterkeringen, Voor de Derde Toetsronde 2006–2011 (hr 2006); Ministerie van Verkeer en Waterstaat: Den Haag, The Netherlands, 2007. (In Dutch) [Google Scholar]


| σ | a1 | a2 | a3 | a4 |
|---|---|---|---|---|
| σ(P(hsmax)) | 0.0010 | 0.011 | −0.0078 | 0.0065 |
| σ(P(Ts)) | −0.0001 | −0.0021 | −0.0141 | 0.0189 |
| σ(P(Qr)) | 0.0017 | 0.0247 | 0.0069 | 0.0189 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhong, H.; Van Gelder, P.; Wang, W.; Wang, G.; Liu, Y.; Niu, S. The Influence of Statistical Uncertainty in the Hydraulic Boundary Conditions on the Probabilistically Computed High Water Level Frequency Curve in the Rhine Delta. Water 2016, 8, 147. https://doi.org/10.3390/w8040147
Zhong H, Van Gelder P, Wang W, Wang G, Liu Y, Niu S. The Influence of Statistical Uncertainty in the Hydraulic Boundary Conditions on the Probabilistically Computed High Water Level Frequency Curve in the Rhine Delta. Water. 2016; 8(4):147. https://doi.org/10.3390/w8040147
Chicago/Turabian StyleZhong, Hua, Pieter Van Gelder, Wen Wang, Gaoxu Wang, Yongzhi Liu, and Shuai Niu. 2016. "The Influence of Statistical Uncertainty in the Hydraulic Boundary Conditions on the Probabilistically Computed High Water Level Frequency Curve in the Rhine Delta" Water 8, no. 4: 147. https://doi.org/10.3390/w8040147
APA StyleZhong, H., Van Gelder, P., Wang, W., Wang, G., Liu, Y., & Niu, S. (2016). The Influence of Statistical Uncertainty in the Hydraulic Boundary Conditions on the Probabilistically Computed High Water Level Frequency Curve in the Rhine Delta. Water, 8(4), 147. https://doi.org/10.3390/w8040147









