Analysis of Dry Spells in Southern Italy (Calabria)
Abstract
:1. Introduction
- (i)
- the spatial analysis of the frequency distributions of the sequences of dry days;
- (ii)
- the evaluation, at annual and seasonal scale, of the regional areas most affected by dry events; and
- (iii)
- the estimation, at annual and seasonal scale, of the long-term trend of the dry spells.
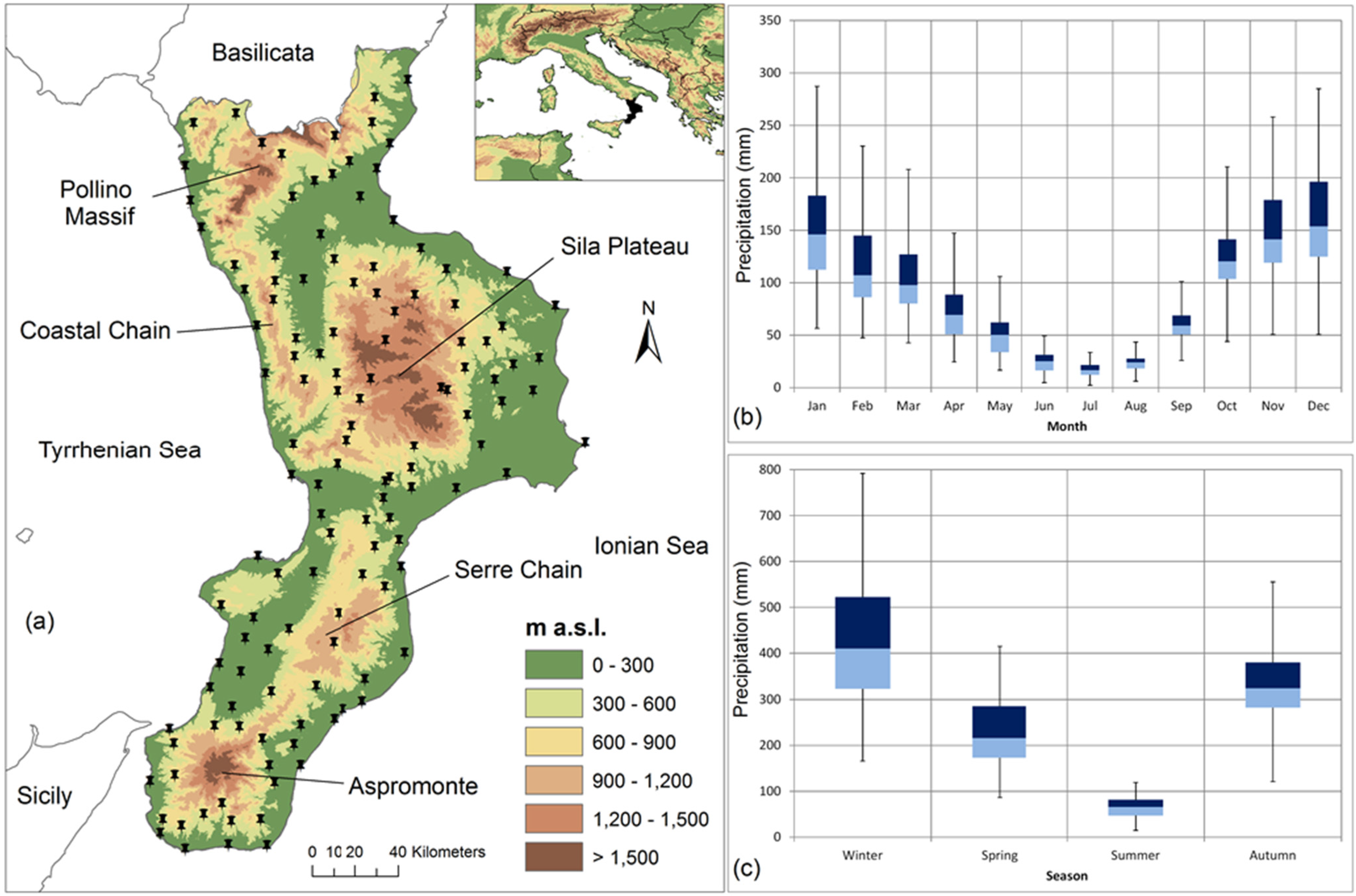
2. Materials and Methods
3. Results and Discussion
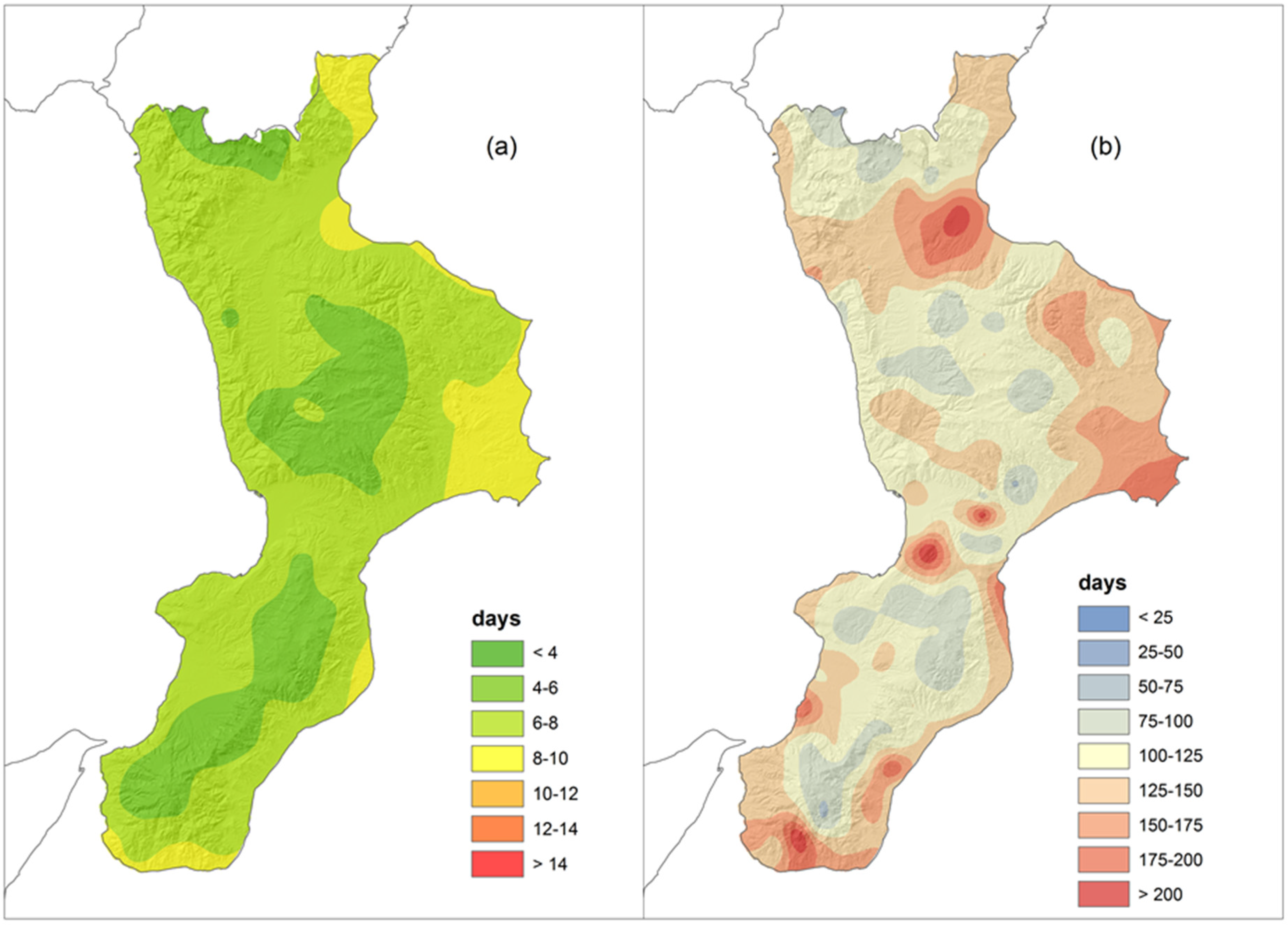
3.1. Characteristics of Dry Spell

3.2. Average and Maximum Lengths of Dry Spells
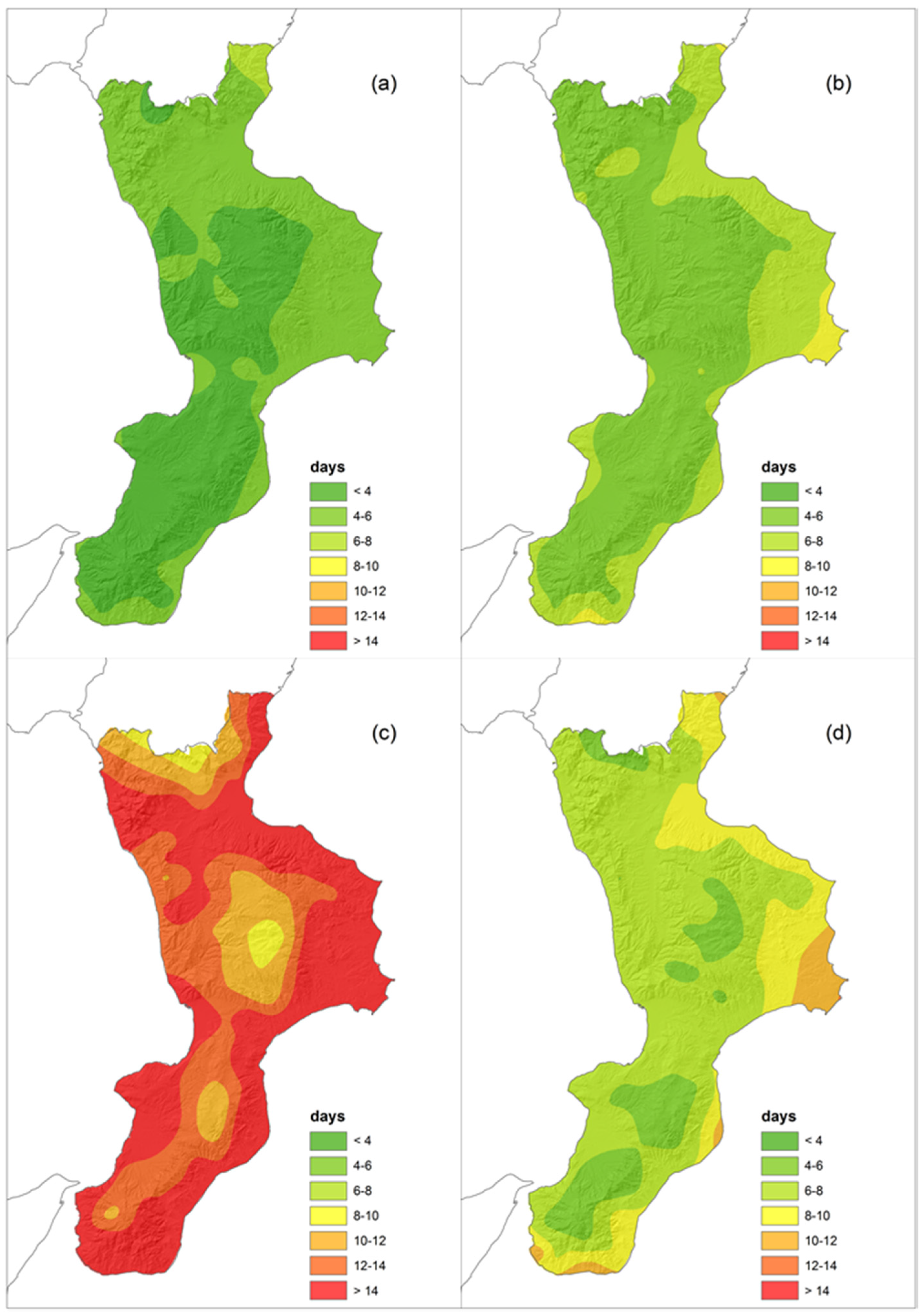
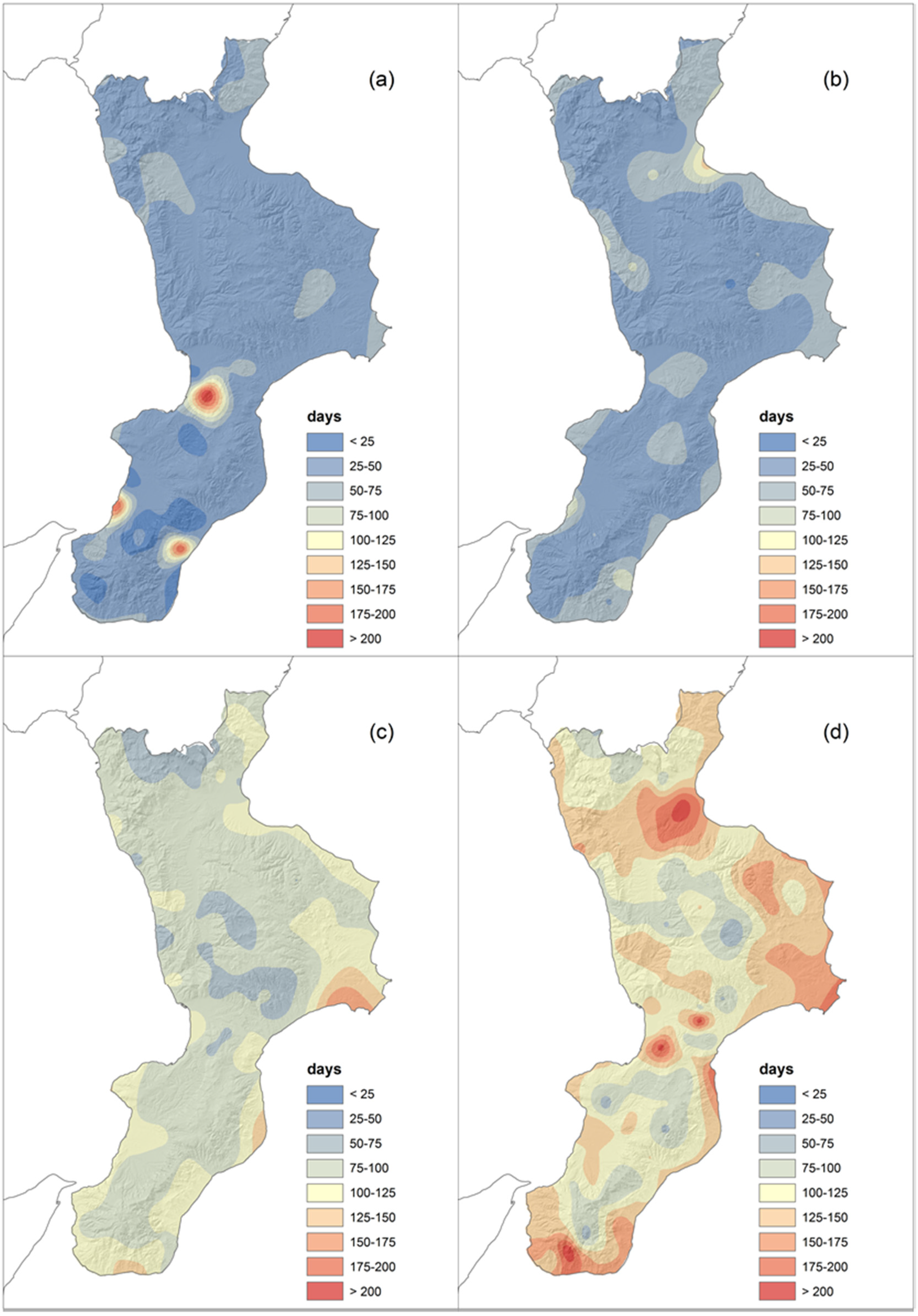
3.3. Temporal Variability of the Dry Spell Lengths
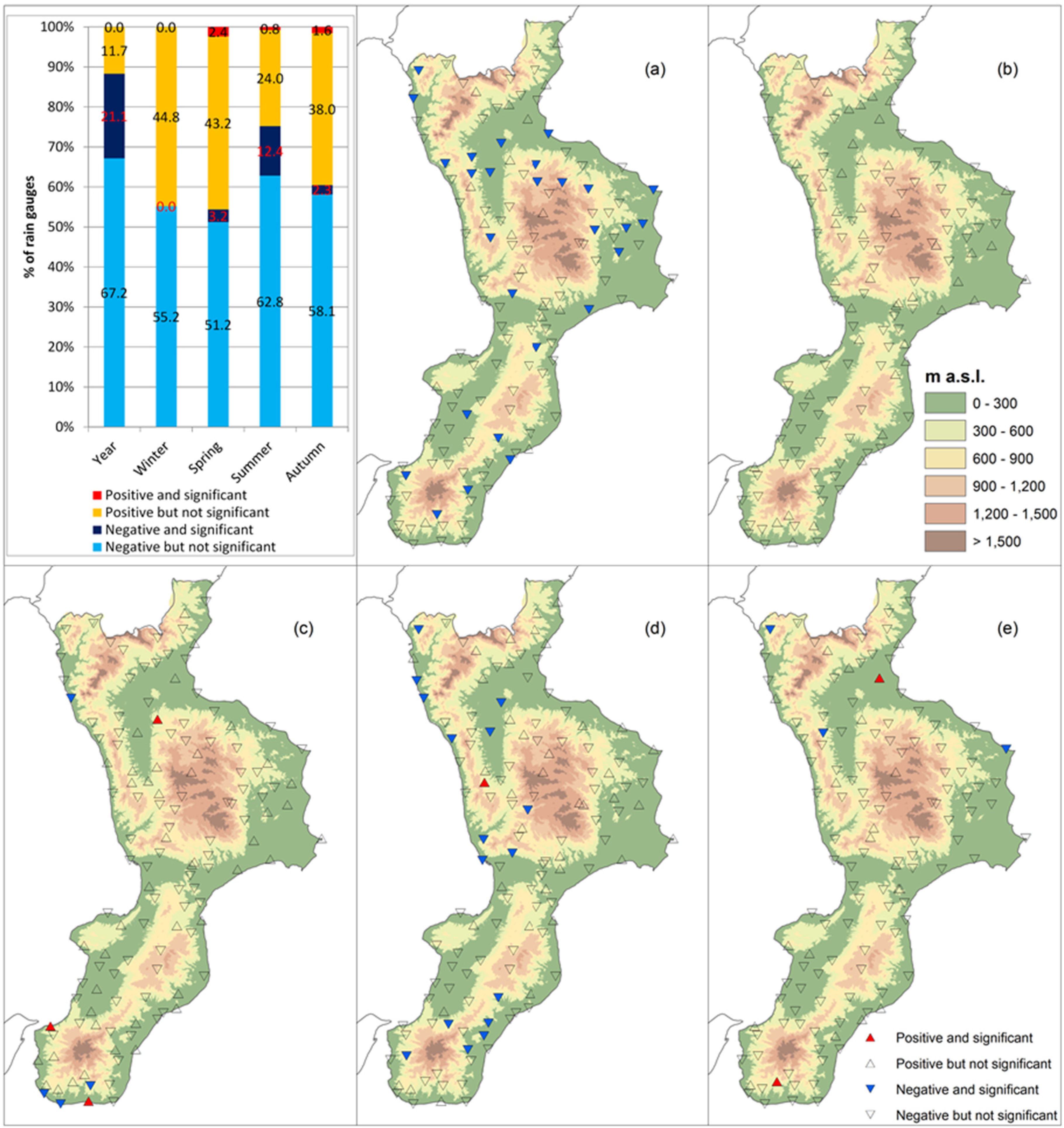
4. Conclusions
Author Contributions
Conflicts of Interest
References
- Solomon, S.; Qin, D.; Manning, M.; Chen, Z.; Marquis, M.; Averyt, K.B.; Tignor, M.; Miller, H.L. Contribution of Working Group I to the Fourth Assessment Report of the Intergovernmental Panel on Climate Change, 2007; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Zhang, X.; Zwiers, F.W.; Hegerl, G.C.; Lambert, F.H.; Gillet, N.P.; Solomon, S.; Stott, P.A.; Nozawa, T. Detection of human influence on twentieth century precipitation trends. Nature 2007, 448, 461–465. [Google Scholar] [CrossRef] [PubMed]
- Min, S.K.; Zhang, X.; Zwiers, F.W.; Hegerl, G.C. Human contribution to more-intense precipitation extremes. Nature 2011, 470, 378–381. [Google Scholar] [CrossRef] [PubMed]
- Pinto, J.G.; Ulbrich, U.; Leckebusch, G.C.; Spangehl, T.; Reyers, M.; Zacharias, S. Changes in storm track and cyclone activity in three SRES ensemble experiments with the ECHAM5/MPI-OM1 GCM. Clim. Dyn. 2007, 29, 195–210. [Google Scholar] [CrossRef]
- Ulbrich, U.; Leckebusch, G.C.; Pinto, J.G. Extra-tropical cyclones in the present and future climate: A review. Theor. Appl. Climatol. 2009, 96, 117–131. [Google Scholar] [CrossRef]
- Ren, L.; Arkin, P.; Smith, T.M.; Shen, S.S.P. Global precipitation trends in 1900–2005 from a reconstruction and coupled model simulations. J. Geophys. Res. Atmos. 2013, 118, 1679–1689. [Google Scholar] [CrossRef]
- Westra, S.; Alexander, L.V.; Zwiers, F.W. Global Increasing trends in annual maximum daily precipitation. J. Clim. 2013, 26, 3904–3918. [Google Scholar] [CrossRef]
- Choi, G.; Collins, D.; Ren, G.; Trewin, B.; Baldi, M.; Fukuda, Y.; Afzaal, M.; Pianmana, T.; Gomboluudev, P.; Huong, P.T.; et al. Changes in means and extreme events of temperature and precipitation in the Asia-Pacific Network region, 1955–2007. Int. J. Clim. 2009, 29, 1906–1925. [Google Scholar] [CrossRef]
- Kelley, C.P.; Ting, M.; Seager, R.; Kushnir, Y. Mediterranean precipitation climatology, seasonal cycle, and trend as simulated by CMIP5. Geophys. Res. Lett. 2012, 39, L21703. [Google Scholar] [CrossRef]
- Partal, T.; Kahya, E. Trend analysis in Turkish precipitation data. Hydrol. Process. 2006, 20, 2011–2026. [Google Scholar] [CrossRef]
- Caloiero, T. Analysis of rainfall trend in New Zealand. Environ. Earth Sci. 2015, 73, 6297–6310. [Google Scholar] [CrossRef]
- Sayemuzzaman, M.; Jha, M.K. Seasonal and annual precipitation time series trend analysis in North Carolina, United States. Atmos. Res. 2014, 137, 183–194. [Google Scholar] [CrossRef]
- Barros, V.; Doyle, M.E.; Camilloni, I.A. Precipitation trends in South Eastern South America: Relationship with ENSO phases and with low-level circulation. Theor. Appl. Climatol. 2008, 93, 19–33. [Google Scholar] [CrossRef]
- Bhend, J.; Von, S.H. Consistency of observed winter precipitation trends in northern Europe with regional climate change projections. Clim. Dyn. 2008, 31, 17–28. [Google Scholar] [CrossRef]
- Huang, J.; Sun, S.L.; Xue, Y.; Zhang, J.C. Spatial and temporal variability of precipitation indices during 1961–2010 in Hunan Province, central South China. Theor. Appl. Climatol. 2014, 118, 581–595. [Google Scholar] [CrossRef]
- Xoplaki, E.; González-Rouco, J.F.; Luterbacher, J.; Wanner, H. Wet season Mediterranean precipitation variability: Influence of large-scale dynamics and trends. Clim. Dyn. 2004, 23, 63–78. [Google Scholar] [CrossRef]
- Brunetti, M.; Maugeri, M.; Monti, F.; Nanni, T. Temperature and precipitation variability in Italy in the last two centuries from homogenised instrumental time series. Int. J. Clim. 2006, 26, 345–381. [Google Scholar] [CrossRef]
- Longobardi, A.; Villani, P. Trend analysis of annual and seasonal rainfall time series in the Mediterranean area. Int. J. Clim. 2010, 30, 1538–1546. [Google Scholar] [CrossRef]
- Gonzalez-Hidalgo, J.C.; Brunetti, M.; de Luis, M. A new tool for monthly precipitation analysis in Spain: MOPREDAS database (Monthly precipitation trends December 1945–November 2005). Int. J. Clim. 2011, 31, 715–731. [Google Scholar] [CrossRef]
- Philandras, C.M.; Nastos, P.T.; Kapsomenakis, J.; Douvis, K.C.; Tselioudis, G.; Zerefos, C.S. Long term precipitation trends and variability within the Mediterranean region. Nat. Hazards Earth Sys. Sci. 2011, 11, 3235–3250. [Google Scholar] [CrossRef]
- Caloiero, T.; Coscarelli, R.; Ferrari, E.; Mancini, M. Precipitation change in southern Italy linked to global scale oscillation indexes. Nat. Hazards Earth Sys. Sci. 2011, 11, 1683–1694. [Google Scholar] [CrossRef]
- Buttafuoco, G.; Caloiero, T.; Coscarelli, R. Spatial and temporal patterns of the mean annual precipitation at decadal time scale in southern Italy (Calabria region). Theor. Appl. Climatol. 2011, 105, 431–444. [Google Scholar] [CrossRef]
- Caloiero, T.; Coscarelli, R.; Ferrari, E.; Mancini, M. Trend detection of annual and seasonal rainfall in Calabria (southern Italy). Int. J. Clim. 2011, 31, 44–56. [Google Scholar] [CrossRef]
- Brunetti, M.; Caloiero, T.; Coscarelli, R.; Gullà, G.; Nanni, T.; Simolo, C. Precipitation variability and change in the Calabria region (Italy) from a high resolution daily dataset. Int. J. Clim. 2012, 32, 57–73. [Google Scholar] [CrossRef]
- Capra, A.; Consoli, S.; Scicolone, B. Long-Term climatic variability in Calabria and effects on drought and agrometeorological parameters. Water Resour. Manag. 2013, 27, 601–617. [Google Scholar] [CrossRef]
- Ferrari, E.; Caloiero, T.; Coscarelli, R. Influence of the North Atlantic oscillation on winter rainfall in Calabria (southern Italy). Theor. Appl. Climatol. 2013, 114, 479–494. [Google Scholar] [CrossRef]
- Caloiero, T.; Buttafuoco, G.; Coscarelli, R.; Ferrari, E. Spatial and temporal characterization of climate at regional scale using homogeneous monthly precipitation and air temperature data: An application in Calabria (southern Italy). Hydrol. Res. 2014, in press. [Google Scholar] [CrossRef]
- Seidel, D.J.; Fu, Q.; Randel, W.J.; Reichler, T.J. Widening of the tropical belt in a changing climate. Nat. Geosci. 2008, 1, 21–24. [Google Scholar]
- Jin, F.; Kitoh, A.; Alpert, P. Water cycle changes over the Mediterranean: A comparison study of a super-high-resolution global model with CMIP3. Philos. Trans. R. Soc. A: Math. Phys. Eng. Sci. 2010, 368, 5137–5149. [Google Scholar] [CrossRef] [PubMed]
- Raible, C.C.; Ziv, B.; Saaroni, H.; Wild, M. Winter synoptic-scale variability over the Mediterranean Basin under future climate conditions as simulated by the ECHAM5. Clim. Dyn. 2010, 35, 473–488. [Google Scholar] [CrossRef]
- Summary for Policymakers. Available online: https://www.ipcc.ch/pdf/assessment-report/ar4/wg1/ar4-wg1-spm.pdf (accessed on 1 April 2015).
- Austin, R.B.; Cantero-Martínez, C.; Arrúe, J.L.; Playán, E.; Cano-Marcellán, P. Yield rainfall relationships in cereal cropping systems in the Ebro river valley of Spain. Eur. J. Agron. 1998, 8, 239–248. [Google Scholar] [CrossRef]
- Quiring, S.M.; Papakryiakou, T.N. An evaluation of agricultural drought indices for the Canadian prairies. Agric. For. Meteorol. 2003, 118, 49–62. [Google Scholar] [CrossRef]
- Kogan, F. Droughts of the late 1980s in the United States as derived from NOAA Polar-Orbiting Satellite data. Bull. Am. Meteorol. Soc. 1995, 76, 655–668. [Google Scholar] [CrossRef]
- Kogan, F. Global drought watch from space. Bull. Am. Meteorol. Soc. 1997, 78, 621–636. [Google Scholar] [CrossRef]
- Abrams, M.D.; Ruffuer, M.C.; Morgan, T.A. Tree-Ring responses to drought across species and contrasting sites in the ridge and valley of central Pennsylvania. For. Sci. 1998, 44, 550–558. [Google Scholar]
- Orwing, D.A.; Abrams, M.D. Variation in radial growth responses to drought among species, site and canopy strata. Trees 1997, 11, 474–484. [Google Scholar] [CrossRef]
- Nicholson, S.E.; Tucker, C.J.; Ba, M.B. Desertification, drought and surface vegetation: An example from the west African Sahel. Bull. Am. Meteorol. Soc. 1998, 79, 815–829. [Google Scholar] [CrossRef]
- Pickup, G. Desertification and climate change—The Australian perspective. Clim. Res. 1998, 11, 51–63. [Google Scholar] [CrossRef]
- Morales, A.; Olcina, J.; Rico, A.M. Diferentes persepciones de la sequía en España: Adaptación, catastrofismo e intentos de corrección. Investig. Geogr. 2000, 23, 5–46. (In Spanish) [Google Scholar] [CrossRef]
- García, R.V.; Smagorinsky, J.; Ellman, M. Nature Pleads Not Guilty; Pergamon Press: Oxford, UK, 1981. [Google Scholar]
- Kanti, B. Coping mechanisms practised by drought victims (1994/1995) in north Bengal, Bangladesh. Appl. Geogr. 1998, 18, 355–373. [Google Scholar]
- Buttafuoco, G.; Caloiero, T. Drought events at different timescales in southern Italy (Calabria). J. Maps 2014, 10, 529–537. [Google Scholar] [CrossRef]
- Buttafuoco, G.; Caloiero, T.; Coscarelli, R. Analyses of drought events in Calabria (Southern Italy) using standardized precipitation index. Water Resour. Manag. 2015, 29, 557–573. [Google Scholar] [CrossRef]
- Kutiel, H. The multimodality of the rainfall course in Israel as reflected by the distribution of dry spells. Arch. Meteorol. Geophys. Bioclimatol. Ser. B 1985, 36, 15–27. [Google Scholar] [CrossRef]
- Bougila, B.; Sushama, L. On the current and future dry spell characteristics over Africa. Atmosphere 2013, 4, 272–298. [Google Scholar] [CrossRef]
- Groisman, P.Y.; Knight, R.W. Prolonged dry episodes over the conterminous United States: New tendencies emerging during the last 40 years. J. Clim. 2008, 21, 1850–1862. [Google Scholar] [CrossRef]
- Llano, M.P.; Penalba, O.C. A climatic analysis of dry sequences in Argentina. Int. J. Clim. 2011, 31, 504–513. [Google Scholar] [CrossRef]
- Carvalho, J.R.P.; Assad, E.D.; Evangelista, S.R.M.; Pinto, H.S. Estimation of dry spells in three Brazilian regions—Analysis of extremes. Atmos. Res. 2013, 132, 12–21. [Google Scholar] [CrossRef]
- Gong, D.Y.; Wang, J.A.; Han, H. Trends of summer dry spells in China during the late twentieth century. Meteorol. Atmos. Phys. 2005, 88, 203–214. [Google Scholar] [CrossRef]
- She, D.; Xia, J.; Song, J.; Du, H.; Chen, J.; Wan, L. Spatio-Temporal variation and statistical characteristic of extreme dry spell in Yellow River Basin, China. Theor. Appl. Climatol. 2013, 112, 201–213. [Google Scholar] [CrossRef]
- Sarhadi, A.; Heydarizadeh, M. Regional frequency analysis and spatial pattern characterization of dry spells in Iran. Int. J. Climatol. 2014, 34, 835–848. [Google Scholar] [CrossRef]
- Heinrich, G.; Gobiet, A. The future of dry and wet spells in Europe: A comprehensive study based on the ENSEMBLES regional climate models. Int. J. Climatol. 2012, 32, 1951–1970. [Google Scholar] [CrossRef]
- Serra, C.; Martínez, M.D.; Lana, X.; Burgueño, A. European dry spells distributions, years 1951–2000. Theor. Appl. Climatol. 2013, 114, 531–551. [Google Scholar] [CrossRef]
- Serra, C.; Martínez, M.D.; Lana, X.; Burgueño, A. European dry spell regimes (1951–2000): Clustering process and time trends. Atmos. Res. 2014, 144, 151–174. [Google Scholar] [CrossRef]
- Zolina, O.; Simmer, C.; Belyaev, K.; Sergey, K.; Gulev, S.; Koltermann, P. Changes in the duration of European wet and dry spells during the last 60 years. J. Clim. 2013, 26, 2022–2047. [Google Scholar] [CrossRef]
- Sánchez, E.; Domínguez, M.; Romera, R.; López de la Franca, N.; Gaertner, M.A.; Gallardo, C.; Castro, M. Regional modeling of dry spells over the Iberian Peninsula for present climate and climate change conditions. Clim. Chang. 2011, 107, 625–634. [Google Scholar] [CrossRef]
- Nastos, P.T.; Zerefos, C.S. Spatial and temporal variability of consecutive dry and wet days in Greece. Atmos. Res. 2009, 94, 616–628. [Google Scholar] [CrossRef]
- Cindrić, K.; Pasarić, Z.; Gajić-Čapka, M. Spatial and temporal analysis of dry spells in Croatia. Theor. Appl. Climatol. 2010, 102, 171–184. [Google Scholar] [CrossRef]
- Brunetti, M.; Maugeri, M.; Nanni, T.; Navarra, A. Droughts and extreme events in regional daily Italian precipitation series. Int. J. Climatol. 2002, 22, 543–558. [Google Scholar] [CrossRef]
- Sirangelo, B.; Caloiero, T.; Coscarelli, R.; Ferrari, E. A stochastic model for the analysis of the temporal change of dry spells. Stoch. Environ. Res. Risk Assess. 2015, 29, 143–155. [Google Scholar] [CrossRef]
- Caloiero, T.; Pasqua, A.A.; Petrucci, O. Damaging hydrogeological events: A procedure for the assessment of severity levels and an application to Calabria (Southern Italy). Water 2014, 6, 3652–3670. [Google Scholar] [CrossRef]
- Craddock, J.M. Methods of comparing annual rainfall records for climatic purposes. Weather 1979, 34, 332–346. [Google Scholar] [CrossRef]
- Simolo, C.; Brunetti, M.; Maugeri, M.; Nanni, T. Improving estimation of missing values in daily precipitation series by a probability density function preserving approach. Int. J. Climatol. 2010, 30, 1564–1576. [Google Scholar] [CrossRef]
- Mathugama, S.C.; Peiris, T.S.G. Critical evaluation of dry spell research. Int. J. Basic Appl. Sci. 2011, 6, 153–160. [Google Scholar]
- Anagnostopoulou, C.H.R.; Maheras, P.; Karacostas, T.; Vafiadis, M. Spatial and temporal analysis of dry spells in Greece. Theor. Appl. Climatol. 2003, 74, 77–91. [Google Scholar] [CrossRef]
- Martín-Vide, J.; Gómez, L. Regionalisation of peninsular Spain based on the length of dry spells. Int. J. Climatol. 1999, 19, 537–555. [Google Scholar] [CrossRef]
- Serra, C.; Burgueño, A.; Martínez, M.D.; Lana, X. Trends in dry spells across Catalonia (NE Spain) during the second half of the 20th century. Theor. Appl. Climatol. 2006, 85, 165–183. [Google Scholar] [CrossRef]
- Lana, X.; Martínez, M.D.; Burgueño, A.; Serra, C.; Martín-Vide, J.; Gomez, L. Spatial and temporal patterns of dry spell lengths in the Iberian Peninsula for the second half of the twentieth century. Theor. Appl. Climatol. 2008, 91, 99–116. [Google Scholar] [CrossRef]
- Mann, H.B. Nonparametric tests against trend. Econometrica 1945, 13, 245–259. [Google Scholar] [CrossRef]
- Kendall, M.G. Rank Correlation Methods; Charles Griffin & Company Limited: London, UK, 1962. [Google Scholar]
- Yue, S.; Wang, C.Y. The Mann-Kendall test modified by effective sample size to detect trend in serially correlated hydrological series. Water Resour. Manag. 2004, 18, 201–218. [Google Scholar] [CrossRef]
- Yue, S.; Wang, C.Y. The applicability of prewhitening to eliminate the influence of serial correlation on the Mann–Kendall test. Water Resour. Res. 2002, 38, 4-1–4-7. [Google Scholar] [CrossRef]
- Von, S.H.; Navarra, A. Analysis of Climate Variability: Applications of Statistical Techniques; Springer Verlag: Berlin, Germany, 1999. [Google Scholar]
- Colacino, M.; Conte, M.; Piervitali, E. Elementi di Climatologia Della Calabria; IFA-CNR: Roma, Italy, 1997. (In Italian) [Google Scholar]
- Coscarelli, R.; Caloiero, T. Analysis of daily and monthly rainfall concentration in Southern Italy (Calabria region). J. Hydrol. 2012, 416, 145–156. [Google Scholar] [CrossRef]
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Caloiero, T.; Coscarelli, R.; Ferrari, E.; Sirangelo, B. Analysis of Dry Spells in Southern Italy (Calabria). Water 2015, 7, 3009-3023. https://doi.org/10.3390/w7063009
Caloiero T, Coscarelli R, Ferrari E, Sirangelo B. Analysis of Dry Spells in Southern Italy (Calabria). Water. 2015; 7(6):3009-3023. https://doi.org/10.3390/w7063009
Chicago/Turabian StyleCaloiero, Tommaso, Roberto Coscarelli, Ennio Ferrari, and Beniamino Sirangelo. 2015. "Analysis of Dry Spells in Southern Italy (Calabria)" Water 7, no. 6: 3009-3023. https://doi.org/10.3390/w7063009
APA StyleCaloiero, T., Coscarelli, R., Ferrari, E., & Sirangelo, B. (2015). Analysis of Dry Spells in Southern Italy (Calabria). Water, 7(6), 3009-3023. https://doi.org/10.3390/w7063009





