1. Introduction
Traditionally, cross-shore sediment transport models have been developed based on energetic-type models (e.g., [
1]). Bowen [
2] and Bailard [
3] extended cross-shore sediment transport models under wave conditions by replacing the oscillating component of waves to the velocity term. The sediment transport rate in the model is proportional to
u3 (bed load transport) and
u4 (suspended load transport), where
u is the cross-shore horizontal velocity. However, in the surf zone, wave breaking turbulence can have a significant effect on the nearshore hydrodynamics and the resulting sediment suspension and transport (e.g., [
4]). Wave breaking turbulence generated near the water surface level penetrates downward and can act to destabilize the sea bed, resulting in large clouds of suspended sediments (e.g., [
5,
6,
7]). The turbulence due to wave breaking also maintains the sediment in suspension and modifies the mean flow field that transports sediments seaward or shoreward [
8].
The improvement of models for cross-shore sediment transport in the surf zone requires the accurate vertical distribution of the sediment concentration across the cross-shore locations to obtain the depth-integrated horizontal sediment flux and corresponding morphodynamics. The vertical distribution of the sediment concentration can be calculated by balancing the settling velocity of sediment particles and convection and/or diffusion processes [
9]. However, because strong turbulent flow conditions in the surf zone enhance sediment suspension in the water column, the vertical distribution of the sediment concentration is influenced by wave breaking turbulence. There is a consensus that turbulence effects should be incorporated into cross-shore sediment transport modeling (e.g
., [
10,
11,
12,
13,
14,
15]).
A number of studies have been conducted on the effect of wave breaking turbulence on sediment suspension. Nadaoka
et al. [
5] observed that “obliquely descending eddies” generated by wave breaking reached the bottom of a water column and caused sediment suspension in a laboratory experiment. The sediment pickup rate was found to be influenced by external turbulence transported from the upper layer. This mechanism has also been observed in the field by Voulgaris and Collins [
16], who noted that sediment suspension is highly dependent on wave breaking rather than bottom boundary layer turbulence. They showed that the reference sediment concentration and vertical sediment concentration are controlled by vortices induced by breaking waves. Ogston and Sternberg [
17] demonstrated the difference of the vertical distribution of the sediment concentration and the sediment diffusivity between the cases of unbroken and broken waves. Because broken waves increase eddy viscosity and sediment diffusion, the vertical distribution of the sediment concentration for broken waves exhibits a uniform tendency. Aagaard and Hughes [
7] also conducted field measurements to investigate the role of coherent vortices generated by wave breaking on the suspended sediment concentration. Aagaard and Jensen [
18] investigated the vertical distribution of sediment diffusivity according to the wave breaking types (e.g., spilling surf bores and plunging waves). They suggested that a different mixing mechanism was predominant for each wave breaking type: diffusion for spilling surf bores and convection for plunging waves.
Many researchers have tried to incorporate the effect of wave breaking turbulence on the sediment suspension into cross-shore sediment transport modeling. Roelvink and Stive [
10] examined the effect of breaking-induced turbulent flow (
i.e., the stirring effect) on the energetic-based bar-generating model by Bowen [
2] and Bailard [
3]. With the stirring effect, they demonstrated improved beach profile prediction by comparison of their results with laboratory data. Kobayashi and Johnson [
11] developed a time-averaged cross-shore sediment transport model that includes a sediment suspension effect due to wave breaking turbulence. Butt
et al. [
12] modified the energetic-type cross-shore sediment transport model by including turbulent kinetic energy (TKE) terms for the swash and inner surf zones. They showed that the model capability was improved by 55% when bore events (
i.e., TKE) were included, suggesting that TKE significantly affects sediment transport. Kobayashi
et al. [
19] also included a term for the wave energy dissipation rate due to wave breaking in their suspended sediment transport model.
Despite the gains made in the studies mentioned above, there is no clear consensus on how to quantitatively express the effect of wave breaking turbulence on sediment concentration or sediment transport modeling for the surf zone. To quantitatively express the effect of turbulence on sediment suspension, this paper proposes a simple relationship between the time-averaged SSC and TKE based on the results of moveable beach observations from a large-scale laboratory experiment. The relationship between time-averaged SSC and TKE was examined with respect to three representative cross-shore locations: the bar crest, the bar trough, and the inner surf zone. These three locations possess different hydrodynamic and morphodynamic characteristics, so different parameterizations should be adopted to improve cross-shore sediment transport modeling for all three. Using data from a large-scale laboratory experiment, we were able to obtain realistic turbulence and sediment concentration data, and the controlled experimental conditions provided us an opportunity to eliminate other factors such as longshore currents or non-stationary forcing conditions.
The remainder of this paper is organized as follows. We briefly describe the large-scale laboratory experiment in
Section 2, and we present the spatial distributions (vertical profiles at three representative cross-shore locations) of TKE and SSC in
Section 3. In
Section 4, we explore the relationship between the spatial distributions of TKE and SSC and propose a simple equation for this relationship. We summarize the findings of the study and the conclusions drawn in
Section 5.
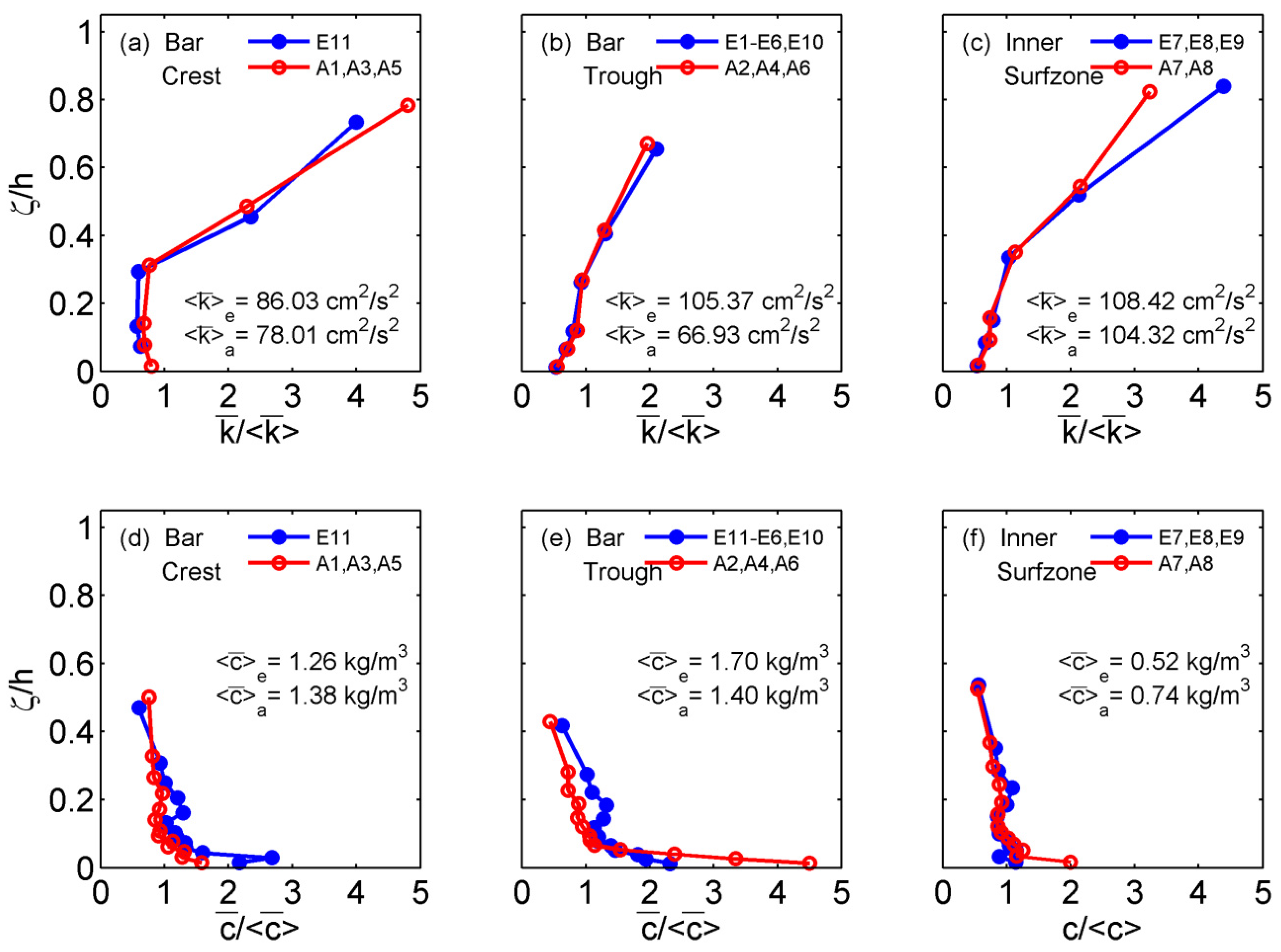
3. Vertical Variation of Turbulent Kinetic Energy and Sediment Suspended Concentration
Figure 4 shows the vertical variation of the time-averaged TKE per unit mass (
) and the time-averaged SSC (
) across the surf zone, at the bar crest (E11 for erosive; A1, A3, and A5 for accretive), the bar trough (E1–E6 and E11 for erosive, A2, A4, and A6 for accretive), and the inner surf zone (E7–E9 for erosive; A7 and A8 for accretive). TKE and SSC were time-averaged from 100 s to 900 s. The upper panels (
Figure 4a–c) show
for the erosive and accretive cases, normalized by the depth-averaged
(indicated by <
>) for each case. The vertical axis represents the relative elevation, where
is the elevation of a sensor from the bed and
h is the water depth. Overall, it is somewhat striking that there is very little difference in the vertical profile of
between erosive and accretive conditions at each of the three location when normalized by <
> at each location. The value of <
> itself was consistent between erosive and accretive cases at the bar crest and increased by approximately 30% at the inner surf zone. Interestingly, at the bar trough, the value of <
> for the erosive is larger than that for accretive cases by 60%, compared to 10% variation over the bar and 4% in the inner surf zone. This large difference is difficult to explain in terms of
or
variations shown in
Figure 2 and
Figure 3, and highlights the complexity of shore-shore sediment transport in the surf zone.
Figure 4.
Vertical variation of time-averaged turbulent kinetic energy per unit mass () and suspended sediment concentration () at bar crest (a,d); bar trough (b,e); and inner surf zone (c) and (f) for erosive (red) and accretive (blue) conditions. Values are normalized by the depth-averaged value from 0 < < 0.5 and are indicated in angle brackets on each panel.
Figure 4.
Vertical variation of time-averaged turbulent kinetic energy per unit mass () and suspended sediment concentration () at bar crest (a,d); bar trough (b,e); and inner surf zone (c) and (f) for erosive (red) and accretive (blue) conditions. Values are normalized by the depth-averaged value from 0 < < 0.5 and are indicated in angle brackets on each panel.
The vertical distribution of
at the bar crest (
Figure 4a) shows the largest variation over the depth. As most waves broke intensively at this location and impinged on the water surface,
was observed to be large near the water surface level but quickly decreased in the middle of the water column at approximately
= 0.3 and then was nearly uniform until the wave bottom boundary layer near the vicinity of the bed
= 0.1. One thing to note was that
increased slightly with depth in the vicinity of the bed (
<0.1) for both erosive and accretive conditions. Strong wave motions over shallow water depths at the bar crest may have generated bottom boundary turbulence and a shear motion, in addition to the wave breaking turbulence from the upper water column. At the bar trough (
Figure 4b),
monotonically decreased downward without the reverse trend near the bottom that was observed in the bar crest. At the inner surf zone (
Figure 4c), the vertical profile of
seems to fall, qualitatively, between the first two. That is,
near the upper is approximately 4 to 5 times larger than the bottom value, as was the case in
Figure 4a at the bar crest. There is a distinct change in the vertical gradient of
at approximately
= 0.3, similar to
Figure 4a. However, unlike
Figure 4a, where the variation of
is almost uniform over depth with a slight increase in the vicinity of the bed, the variation of
decreases downward, consistent with the observations at the bar trough in
Figure 4b.
The lower panels of
Figure 4d–f illustrate the time-averaged SSC (
). As with
, the depth-averaged
is indicated as <
>. The magnitude of <
> is largest at the bar crest and trough, where the most intense and unsteady wave breaking occurs, and significantly decreases in the inner surf zone by almost a half. As expected,
Figure 4d–f shows an increase in the
near the bed, but there are interesting similarities and differences between erosive and accretive concentration profiles at each of the regions. For example,
Figure 4f in the inner surf zone shows that the normalized concentration profiles of both the erosive and accretive conditions shows vertically uniform profiles, except for the very close to the bottom. On the other hand,
Figure 4e showed a strong difference in the profiles of
/<
> between the erosive and accretive cases, despite of the nearly same profiles of
/<
> in
Figure 4b. In particular, the value of the normalized concentration near the bottom is about twice as large for the erosive case (red) compared to the accretive case (blue). This can be partly attributed to the 60% difference in <
>, but also be due to another sediment suspension mechanism such as adverse pressure gradient in the bar trough. Furthermore,
Figure 4d,f show that the concentration profiles are nearly uniform over depth for
> 0.3 owing to the strong mixing by wave breaking. The data seem to suggest that the concentration profile is less uniform over the bar trough (
Figure 4e) for the erosive case. Overall, there is an interesting similarity in the normalized turbulence and concentration profiles over the bar crest (
Figure 4a,d) and the inner surf zone (
Figure 4c,f) even though the values for normalization had opposite trends: <
> increased by 30% from bar to inner surf zone, but <
> decreased by a factor 2.
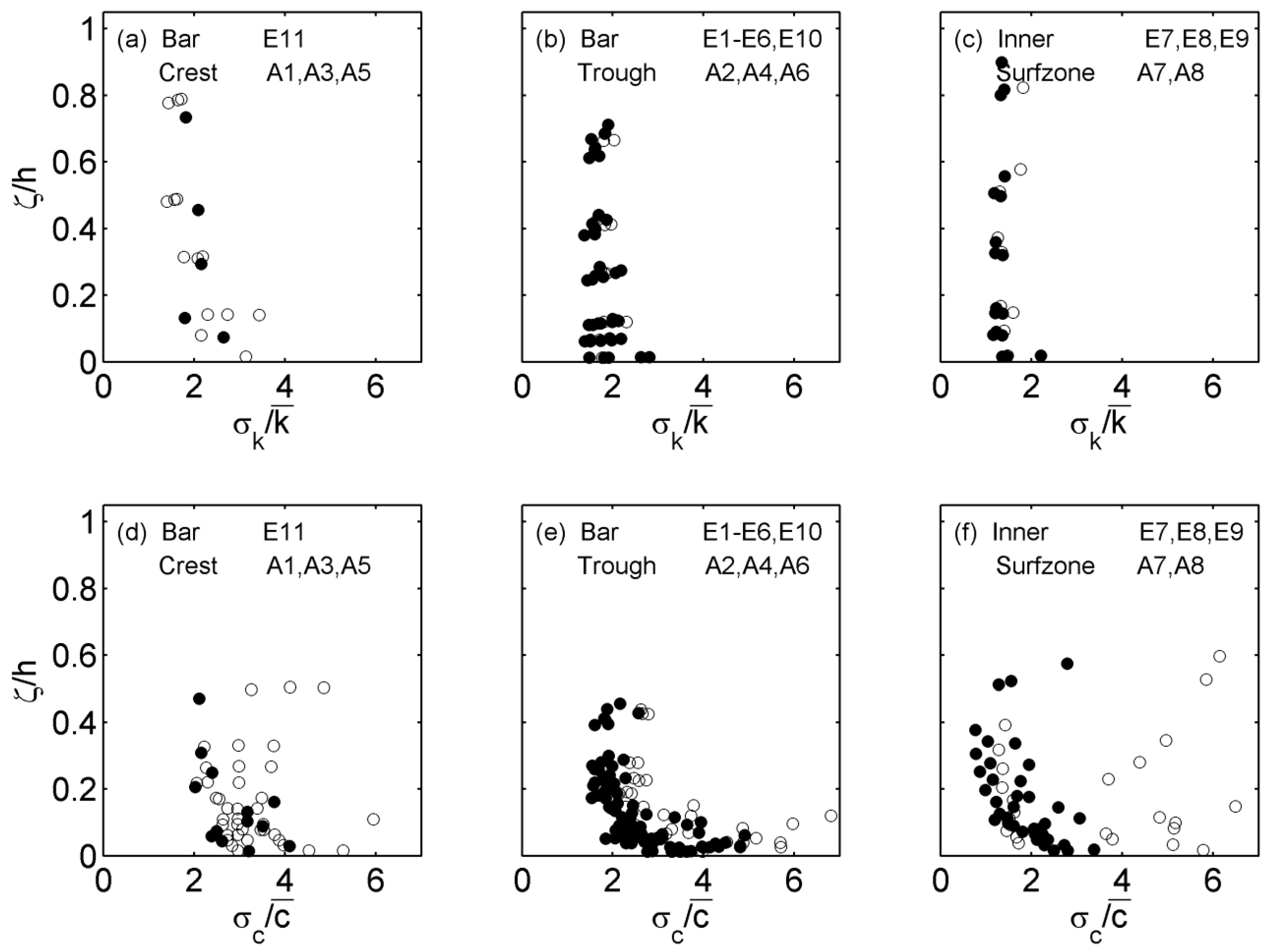
The intermittency of turbulence and sediment suspension can be examined using thresholds based on the mean and standard deviation of the observed
and
at each elevation (e.g., [
15,
25,
26]). Yoon and Cox [
15] observed that intermittent events of turbulent and suspension occurred for a small portion of the time series but contained a significant amount of motions in these events. The intermittent events were defined where the value of turbulent or sediment suspension exceed a certain threshold, such as a mean plus one standard deviation. Therefore the statistical parameters, such as mean and standard deviation, are important for understanding intermittent features of turbulent and sediment suspension.
Figure 5 shows the statistical results such as the standard deviations of
(
) and
(
), normalized by
and
, respectively, at each elevation. In some cases,
/
shows nearly uniform over the vertical as seen in
Figure 5a–c for both erosive and accretive cases and is somewhat surprising given the vertical variation in
seen in
Figure 4a–c. Overall, the value of
/
is approximately 2, although it appears to be slightly smaller for the inner surf zone. On the other hand, there seems to be a larger scatter in
/
as one might expect considering the characteristics of sediment concentration time series measured in the surf zone. Moreover, the vertical variation is no longer uniform over the vertical with
/
increasing near the bottom. For example,
Figure 5e shows that
/
is approximately 2 for
> 0.1 and then increase downward by a factor of 2 to 3. One possible explanation for the larger
/
is the longer time scale for sediment suspension than for turbulence. Due to the shorter time scale, turbulence cannot be advected in the same way as suspended sediments are advected. Once sediments are suspended, they remain suspended for some time even after turbulence has dissipated. Also, some values of
/
for the accretive case were larger than that for the erosive case in the inner surf zone, and this is probably due to longer wave period, keeping sediments suspended longer.
Figure 5.
Vertical variation of the ratio of the standard deviation () to time-averaged turbulent kinetic energy per unit mass () at the bar crest (a); bar trough (b) and inner surf zone (c) for erosive (closed dot) and accretive (open dot) conditions. Vertical variation of the same ratio for the suspended sediment concentration (/) at the same cross-shore locations (d–f).
Figure 5.
Vertical variation of the ratio of the standard deviation () to time-averaged turbulent kinetic energy per unit mass () at the bar crest (a); bar trough (b) and inner surf zone (c) for erosive (closed dot) and accretive (open dot) conditions. Vertical variation of the same ratio for the suspended sediment concentration (/) at the same cross-shore locations (d–f).
4. Relation between Turbulent Kinetic Energy and Suspended Sediment Concentration
The understanding of the relationship between TKE and SSC is important for estimation and modeling of sediment transport in the surf zone. Based on the measurements obtained as described in the previous section, we propose the following simple relationship between
and
at the bar trough, bar crest, and inner surf zone.
where
B is a dimensional coefficient (with units of
). The right-hand term in Equation (4) is analogous to the dissipation rate (
) in the typical
equation, in which
(e.g., [
27]), where
is an empirical constant (typically ~0.09) and
l is the turbulent length scale. Traditionally, the length scale of turbulence in the surf zone is expressed as a function of the water depth,
h, for example,
l 0.2
h to 0.3
h ([
28]),
l 0.25
h ([
29]), and
l 0.1
h to 0.2
h ([
30]), and is considered to be vertically uniform. Pedersen
et al. [
31] argued that the turbulent length scale is not uniform but rather decreases linearly in the vicinity of the bed, varying in the range of
~0.3
h below
< 0.15.
Especially, Cox
et al. [
32] noted that
l also varied from 0.04
h (outside surf zone) to 0.18
h (inner surf zone) according to the cross-shore locations. In the present study, it is assumed that
is proportional to the turbulent dissipation rate at a specific elevation. The turbulent length scale is also assumed to be dependent on the elevation above the bed and to be expressed in a dimensionless form as
. According to these assumptions, the length scale decreases downward because of the effect of the bottom and the contribution of turbulent dissipation to
increases downward. In the following, we investigated the relationship between
and
experimentally by examining the coefficient
B in Equation (4) and the
R2 (coefficient of determination) values at the characteristic locations of the barred beach,
i.e., the bar crest, trough, and inner surf zone.
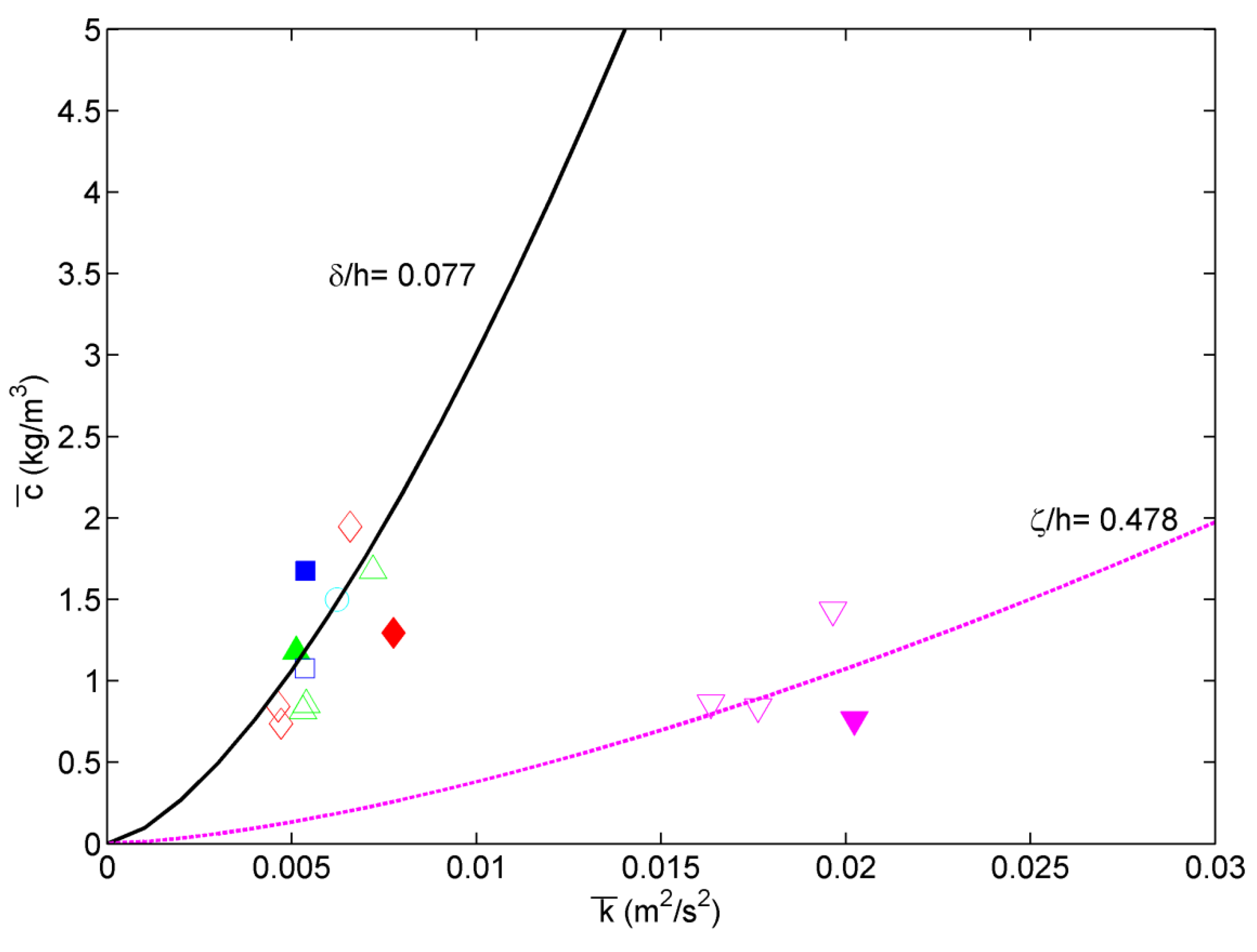
At the bar crest, the relationship between
and
was weak at elevations within ~20 cm above the bed (
~ 0.3). This is shown in
Figure 6 in which the relation between
and
measured at the bar crest for erosive conditions E11 (solid markers) and accretive conditions A1, A3, and A5 (open markers). For the cases in the range of 1 cm ≤
≤ 20 cm (0.016 ≤
≤ 0.309),
was correlated to neither
nor elevation, exhibiting a cluster of data points. Also, there was no clear difference in the turbulence magnitude between the erosive and accretive cases. The bar crest is the region in which depth-limited wave breaking occurs primarily and most strongly over shallow water depths. Thus, sediment could be strongly suspended and mixed farther away from the bed. In addition, strong wave-induced motion in shallow water depths (see
Figure 2 and
Figure 3) can enhance sediment suspension and mixing with current shear. Therefore, for the bar crest case, we modified Equation (4) slightly as follows:
where
is the wave bottom boundary layer thickness (~5 cm), which is taken as a reasonable first estimate for the mixing length near the bottom [
33]. The black solid line in
Figure 6 represents the fitted curve (Equation (5)) for elevations of 1 cm ≤
≤ 20 cm (0.016 ≤
≤ 0.309) and showed reasonable results with
R2 = 0.66 considering the complexity of sediment suspension mechanism in the surf zone. When we applied Equation (4) to the case of
= 0.478, which is in the middle of water column, the least-square fit with Equation (4) yields
B = 180 with scattered data (
R2 = 0.04), as shown in
Table 1.
Figure 6.
Relation between time-averaged turbulent kinetic energy () and suspended sediment concentration () over the bar crest for erosive condition E11 (solid markers) and accretive conditions A1, A3, and A5 (open markers), classified by depth: = 0.016 (cyan circles), 0.079 (blue squares), 0.139 (red diamonds), 0.309 (green triangles), and 0.478 (magenta triangles pointing downward). The solid black line indicates the least-square best fit to Equation (5) for B = 230 (R2 = 0.66) and = 0.077, where is the bottom boundary layer thickness (~5 cm). The dashed line indicates the best fit to Equation (4) for = 0.478.
Figure 6.
Relation between time-averaged turbulent kinetic energy () and suspended sediment concentration () over the bar crest for erosive condition E11 (solid markers) and accretive conditions A1, A3, and A5 (open markers), classified by depth: = 0.016 (cyan circles), 0.079 (blue squares), 0.139 (red diamonds), 0.309 (green triangles), and 0.478 (magenta triangles pointing downward). The solid black line indicates the least-square best fit to Equation (5) for B = 230 (R2 = 0.66) and = 0.077, where is the bottom boundary layer thickness (~5 cm). The dashed line indicates the best fit to Equation (4) for = 0.478.
Table 1.
Coefficient B in Equation (5) and R2 at bar crest.
Table 1.
Coefficient B in Equation (5) and R2 at bar crest.
| ζ (cm) or δ (cm) | or | B | R2 |
|---|
| 5.0 (δ) | 0.077 | 230 | 0.66 |
| 31.0 (ζ) | 0.478 | 180 | 0.04 |
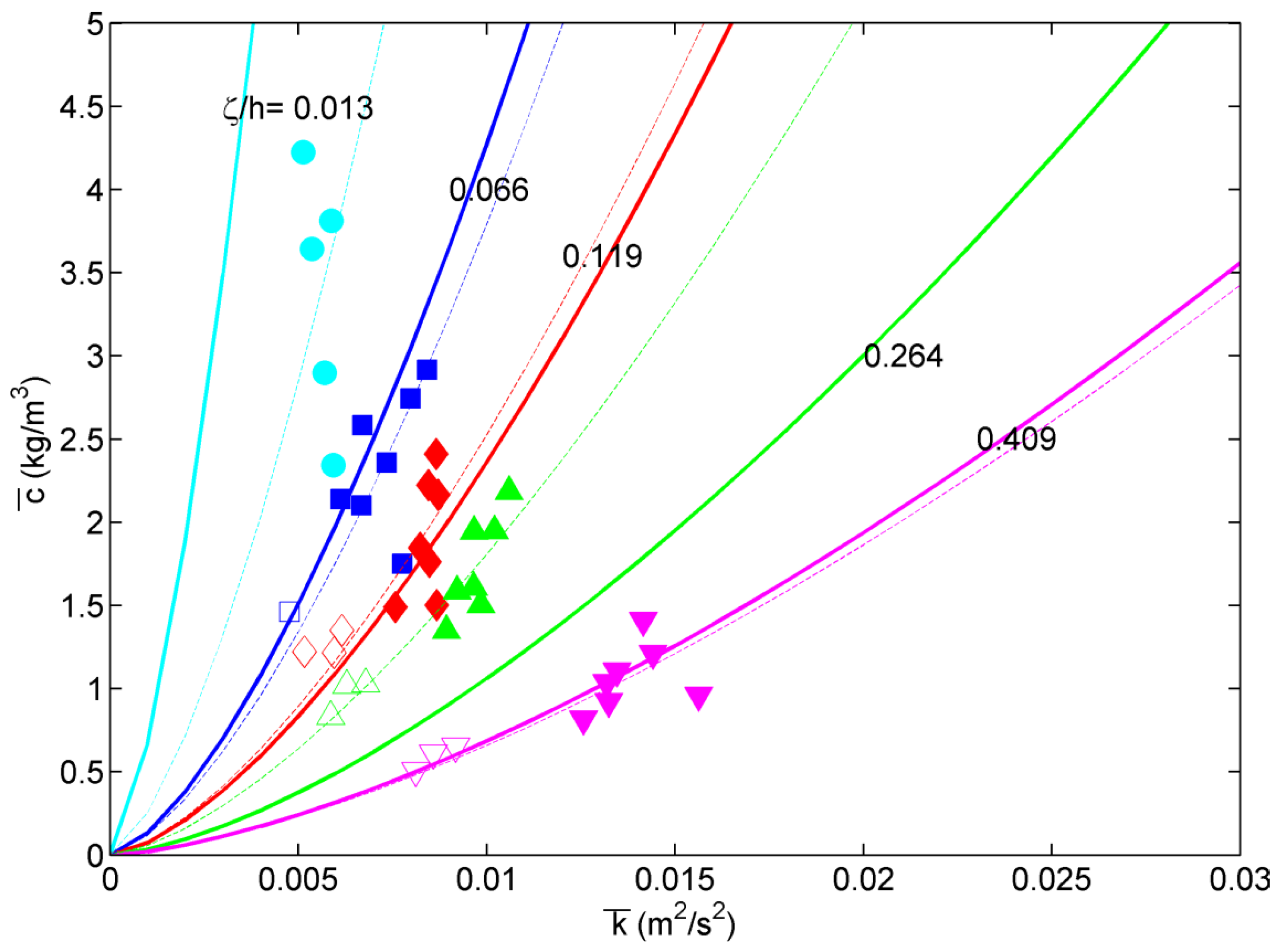
Next, we examined the case at the bar trough where the transient motion is predominant after the initial wave breaking over the bar crest as noted in Yoon and Cox [
15].
Figure 7 shows the relation between
and
measured at the bar trough for erosive conditions E1–E6 and E10 (solid markers) and accretive conditions A2, A4, and A6 (open markers). The turbulent intensity (
) for the erosive case is larger than that for accretive case because of stronger wave breaking conditions. The solid lines in
Figure 7 indicate the “global” best fit to Equation (4) for
B = 280 (
R2 = 0.72), for all of the data at the elevations of
= 0.013, 0.066, 0.119, 0.264 and 0.409 (see
Table 2). The tuning parameter
B, which accounts for the physics of the sediment suspension mechanism besides
and
, appears to be suitable for use in determining the relationship. The fitting results suggest that
could be simply parameterized by the turbulent flow using Equation (4) which is similar to the equation for the turbulent dissipation rate. However, there were some discrepancies at
= 0.013 and 0.264 when we applied the “global” fit to all of the elevations.
Figure 7.
Relation between time-averaged turbulent kinetic energy () and suspended sediment concentration () over the bar trough for erosive conditions E1–E6 and E10 (solid markers) and accretive conditions A2, A4, and A6 (open markers) classified by depth: = 0.013 (cyan circles), 0.066 (blue squares), 0.119 (red diamonds), 0.264 (green triangles), and 0.409 (magenta triangles pointing downward). Solid lines indicate the least-square best fit to Equation (4) for B = 280 (R2 = 0.72) for elevations = 0.066, 0.119, 0.264, and 0.409, except for = 0.013. Dashed lines indicates the best fit to Equation (4) for B determined for each elevation.
Figure 7.
Relation between time-averaged turbulent kinetic energy () and suspended sediment concentration () over the bar trough for erosive conditions E1–E6 and E10 (solid markers) and accretive conditions A2, A4, and A6 (open markers) classified by depth: = 0.013 (cyan circles), 0.066 (blue squares), 0.119 (red diamonds), 0.264 (green triangles), and 0.409 (magenta triangles pointing downward). Solid lines indicate the least-square best fit to Equation (4) for B = 280 (R2 = 0.72) for elevations = 0.066, 0.119, 0.264, and 0.409, except for = 0.013. Dashed lines indicates the best fit to Equation (4) for B determined for each elevation.
Table 2.
Coefficient B in Equation (4) and R2 at bar trough.
Table 2.
Coefficient B in Equation (4) and R2 at bar trough.
| ζ (cm) | | B | R2 |
|---|
| 1.0 | 0.013 | 530 | 0.23 |
| 5.0 | 0.066 | 250 | 0.69 |
| 9.0 | 0.119 | 300 | 0.72 |
| 20.0 | 0.264 | 480 | 0.90 |
| 31.0 | 0.409 | 270 | 0.77 |
| Global (ζ = 5.0–31.0) | 280 | 0.72 |
When we applied Equation (4) to each elevation separately, we were able to obtain better agreement between
and
. The dashed lines in
Figure 7 indicate the “local” best fit to Equation (4) for
B determined for each elevation. The “local” best fit yielded better agreement than the “global” fit above the bottom boundary layer (
≥ 9 cm or
≥ 0.119), as shown in
Table 2. The relationship between
and
had
R2 ≥ 0.72 with
B = 270–480 (average: 350) for the elevations
≥ 0.119. This confirms that SSC is closely related to turbulent flow and can be parameterized using Equation (4) if the intensity of breaking wave (or turbulent intensity) is enough large such as at the bar trough. However, even the “local” fit achieved is not good close to the bottom, as seen in the case of
= 0.013 where
R2 = 0.23. The proposed relationship yielded good agreement only above the wave bottom boundary layer, which may be because another suspension mechanism (e.g., sheet flow) is predominant close to the bottom except near the bar trough.
At the inner surf zone, the relationship between and is not as strong. and for the erosive and accretive cases exhibited no clear difference, as was the case of the bar crest. Here, Equation (4) fits reasonably well near the bottom (within 5 cm above the bed), with R2 values in the range of 0.44 to 0.49. Note that the coefficient of B in Equation (4) is smaller than at the other locations by almost an order of magnitude, because the suspended sediment concentration is lower in the inner surf zone than at the other locations. At elevations greater than 5 cm above the bed, the agreement rapidly decreases and R2 approaches zero.