Integrated Water Resource Management and Energy Requirements for Water Supply in the Copiapó River Basin, Chile
Abstract
:1. Introduction
2. Methods
2.1. Study Area
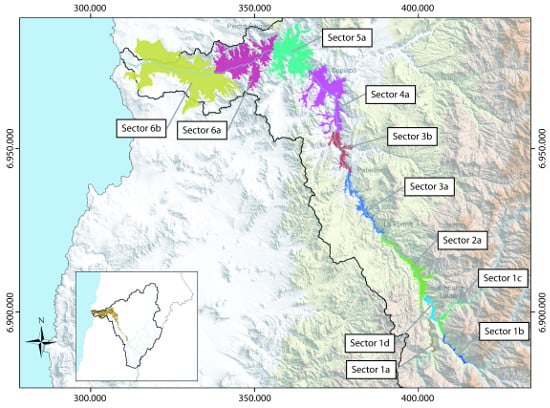
2.1.1. The Copiapó River Basin
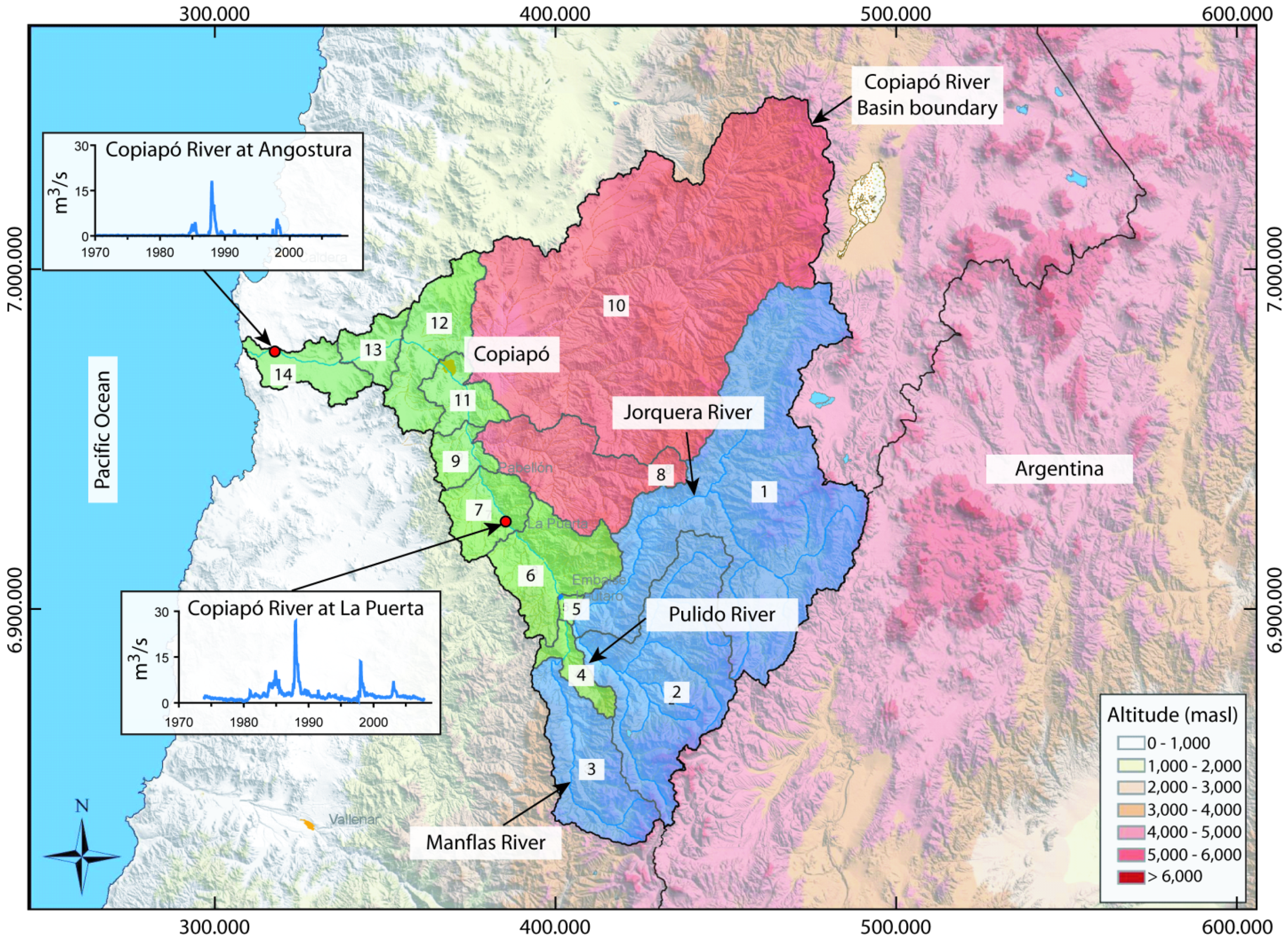
2.1.2. Data Collection
2.1.3. Hydrogeological Characterization
| Meteorological stations | Monthly mean precipitation (mm) | Annual values | ||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Name | Altitude (m) | Jan | Feb | Mar | Apr | May | Jun | Jul | Aug | Sep | Oct | Nov | Dec | Precip. (mm) | Mean temp. (°C) | Evaporation (mm) |
| Caldera | 15 | 0.0 | 0.0 | 0.8 | 0.4 | 1.3 | 5.3 | 4.9 | 5.1 | 0.5 | 0.0 | 0.0 | 0.0 | 18.3 | N/A | N/A |
| Canto de Agua | 330 | 0.2 | 0.0 | 0.4 | 1.2 | 3.1 | 9.7 | 8.8 | 6.6 | 0.4 | 0.4 | 0.0 | 0.2 | 31.0 | 16.9 | 1494.7 |
| Conay Albaricoque | 1600 | 0.1 | 1.8 | 2.6 | 3.1 | 8.5 | 26.1 | 19.8 | 14.0 | 0.5 | 0.0 | 0.8 | 1.0 | 78.3 | 17.2 | 3126.5 |
| Copiapó | 385 | 0.0 | 0.0 | 1.1 | 0.2 | 1.4 | 6.0 | 4.3 | 3.8 | 0.2 | 0.0 | 0.0 | 0.1 | 17.1 | 16.7 | 1680.2 |
| El Totoral | 150 | 0.2 | 0.0 | 0.7 | 0.9 | 3.0 | 10.1 | 7.6 | 5.0 | 0.3 | 0.3 | 0.0 | 0.0 | 28.1 | N/A | N/A |
| Elibor Campanento | 750 | 0.0 | 0.0 | 1.0 | 0.6 | 2.6 | 9.1 | 7.5 | 5.9 | 0.4 | 0.1 | 0.0 | 0.0 | 27.2 | N/A | N/A |
| Hacienda Manflas | 1410 | 0.2 | 0.2 | 1.9 | 2.9 | 4.8 | 13.3 | 10.2 | 10.3 | 1.0 | 0.6 | 0.0 | 0.1 | 45.5 | N/A | N/A |
| Iglesia Colorada | 1550 | 0.2 | 0.2 | 1.5 | 4.0 | 6.0 | 12.2 | 10.9 | 9.5 | 1.4 | 0.8 | 0.0 | 0.3 | 47.0 | 18.6 | 3961.6 |
| Jorquera en la Guardia | 2000 | 0.2 | 1.4 | 3.2 | 3.5 | 6.4 | 13.3 | 7.3 | 7.6 | 2.8 | 1.3 | 0.1 | 0.3 | 47.4 | N/A | N/A |
| Las Vegas | 2250 | 0.0 | 1.0 | 2.9 | 0.8 | 5.7 | 6.6 | 26.4 | 4.5 | 0.8 | 0.2 | 0.0 | 0.0 | 48.9 | N/A | N/A |
| Lautaro embalse | 1110 | 0.3 | 0.4 | 2.7 | 2.4 | 4.2 | 8.9 | 8.1 | 8.8 | 1.5 | 0.3 | 0.2 | 0.2 | 38.0 | 19.6 | 2873.3 |
| Los Loros | 940 | 0.0 | 0.0 | 1.2 | 1.4 | 2.8 | 10.5 | 9.3 | 8.1 | 0.9 | 0.1 | 0.0 | 0.1 | 34.4 | 18.1 | 2908.7 |
| Pastos Grandes | 2260 | 0.0 | 1.6 | 3.2 | 1.8 | 3.4 | 8.9 | 9.8 | 4.8 | 2.3 | 0.7 | 0.0 | 0.2 | 36.7 | N/A | N/A |
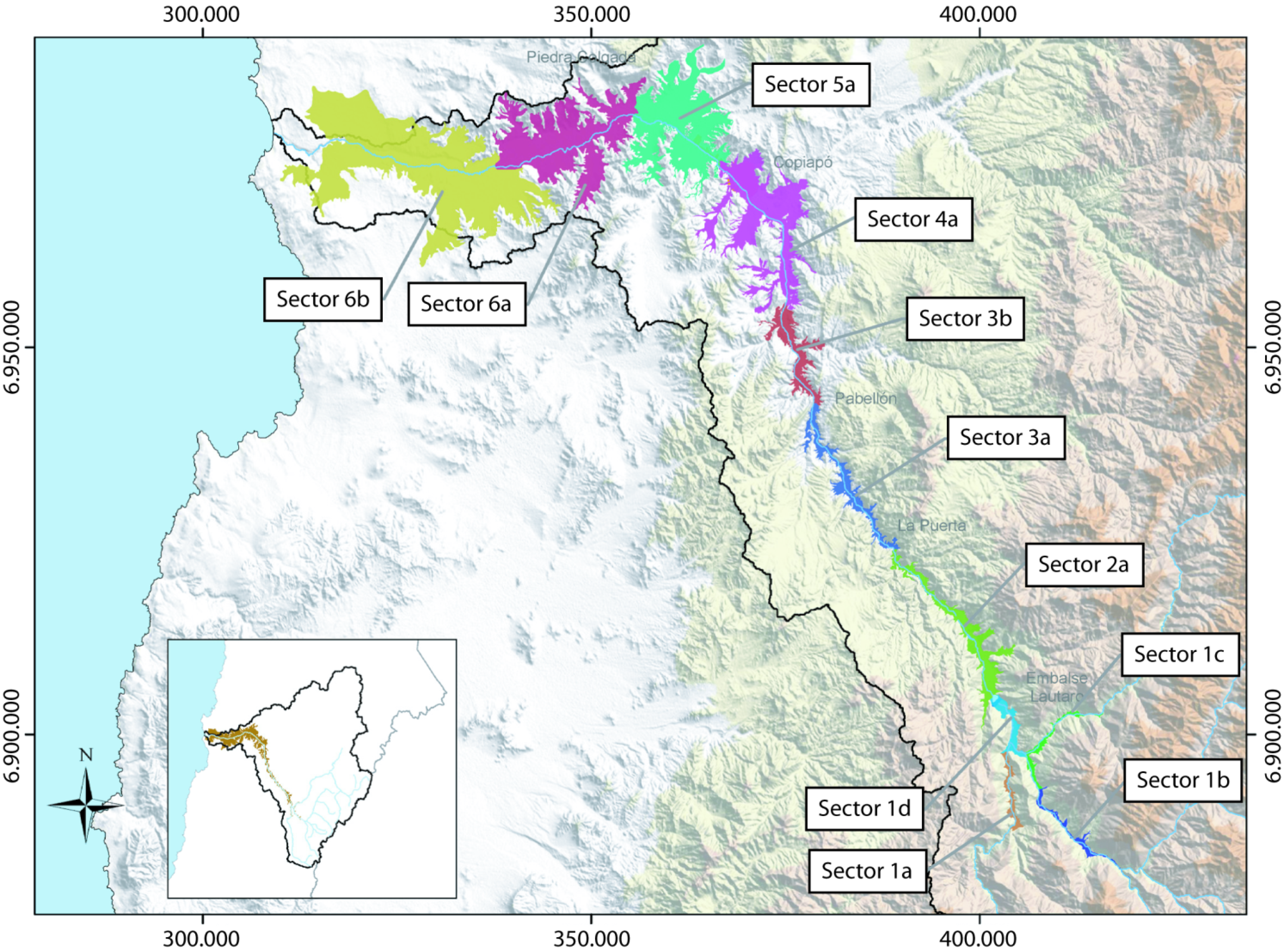
2.2. Water Resources Management Model
2.3. Integrated Water Resource Management Scenarios
| Fluvial stations | Mean annual water flow (m3/s) | |||||
|---|---|---|---|---|---|---|
| Name | Mean | Min | Max | N | σ | γ |
| Río Copiapó en Angostura | 0.44 | 0.10 | 5.29 | 41 | 0.98 | 3.99 |
| Río Copiapó en Mal Paso aguas arriba Canal | 1.06 | 0.23 | 4.88 | 37 | 0.88 | 2.78 |
| Río Copiapó en Mal Paso aguas abajo Canal | 0.99 | 0.69 | 1.28 | 2 | 0.42 | - |
| Río Copiapó en ciudad de Copiapó | 1.93 | 0.33 | 7.19 | 11 | 2.09 | 1.92 |
| Río Copiapó en Lautaro | 1.29 | 0.37 | 4.53 | 53 | 0.96 | 2.01 |
| Río Copiapó en Pastillo | 1.79 | 0.72 | 4.83 | 35 | 0.94 | 1.41 |
| Río Copiapó en La Puerta | 2.41 | 0.79 | 9.92 | 52 | 1.60 | 2.75 |
| Río Copiapó en San Antonio | 1.50 | 0.33 | 5.99 | 17 | 1.44 | 2.18 |
| Río Jorquera en Vertedero | 0.72 | 0.17 | 2.96 | 41 | 0.51 | 2.57 |
| Canal Mal Paso después de Bocatoma | 0.72 | 0.19 | 1.33 | 17 | 0.28 | 0.16 |
| Río Manflas en Vertedero | 0.46 | 0.09 | 2.58 | 33 | 0.49 | 2.98 |
| Río Pulido | 1.43 | 0.42 | 3.76 | 41 | 0.88 | 1.33 |
| Scenario | Simulation | Simulation description |
|---|---|---|
| 1. Current status. | 1.1 | Water demand maintained in the future. |
| 2. Uniform reduction of water demand. | 2.1 | 20% reduction in water demand. |
| 2.2 | 30% reduction in water demand. | |
| 2.3 | 50% reduction in water demand. | |
| 3. Segmented reduction of water demand. | 3.1 | Reduction in agricultural irrigation, mining industry and potable water demand. 20% reduction in Sectors 1 and 2; 35% reduction in Sector 3; 50% reduction in Sector 4. |
| 3.2 | Reduction in agricultural irrigation and mining industry. 20% reduction in Sectors 1 and 2; 35% reduction in Sector 3; 50% reduction in Sector 4. | |
| 4. Water resource management with uniform reduction of water demand and translocation of water between aquifer zones. | 4.1 | 30% reduction in all water uses except for potable water. 50% of potable water demand is transferred from aquifer Sector 4a to 5a. |
| 4.2 | 30% reduction in all water uses except for potable water. 50% of potable water demand is transferred from aquifer Sector 4a to 6a. | |
| 4.3 | 30% reduction in all water uses except potable water. 30% of potable water demand is transferred from aquifer Sector 4a to 5a, and 20% of potable water demand is transferred from aquifer Sector 4a to 6a. | |
| 5. Water resource management with segmented reduction of water demand and translocation of water between aquifer zones. | 5.1 | Segmented reduction in all water uses except potable water: 20% reduction in Sectors 1 and 2; 35% reduction in Sector 3; 50% reduction in Sector 4a; 50% reduction in Sector 5a. 50% of potable water is transferred from aquifer Sector 4a to 5a. |
| 5.2 | Segmented reduction in all water uses except potable water: 20% reduction in Sectors 1 and 2; 35% reduction in Sector 3; 50% reduction in Sector 4a; 50% reduction in Sector 5a. 50% of potable water is transferred from aquifer Sector 4a to 6a. | |
| 5.3 | Segmented reduction in all water uses except potable water: 20% reduction in Sectors 1 and 2; 35% reduction in Sector 3; 50% reduction in Sector 4a; 50% reduction in Sector 5a. 30% of potable water is transferred from aquifer Sector 4a to 5a, and 20% of potable water is transferred from aquifer Sector 4a to 6a. |
2.4. Impacts of Water Resource Management on Energy Requirements for Water Supply
3. Results and Discussion
3.1. Data Collection–Water Use
3.2. Hydrogeological Characterization
| Number of ground water rights granted in the aquifer of the Copiapó River Basin | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Sector | Irrigation | No. | Human consumption | No. | Mining | No. | Other uses | No. | Total | No. |
| 1 | 3% | 9 | 0% | 0 | 0% | 0 | 1% | 1 | 2% | 10 |
| 2 | 18% | 56 | 7% | 2 | 7% | 5 | 6% | 5 | 14% | 68 |
| 3 | 14% | 43 | 0% | 0 | 7% | 5 | 17% | 14 | 13% | 62 |
| 4 | 6% | 19 | 69% | 20 | 46% | 31 | 10% | 8 | 16% | 78 |
| 5 | 30% | 91 | 17% | 5 | 27% | 18 | 35% | 29 | 30% | 143 |
| 6 | 28% | 86 | 7% | 2 | 12% | 8 | 31% | 26 | 25% | 122 |
| Total | 100% | 304 | 100% | 29 | 100% | 67 | 100% | 83 | 100% | 483 |
| Water flow granted (ground water) in the aquifer of the Copiapó River Basin | ||||||||||
| Sector | Irrigation | L/s | Human consumption | L/s | Mining | L/s | Other uses | L/s | Total | L/s |
| 1 | 6% | 619 | 0% | 0 | 0% | 0 | 3% | 100 | 4% | 719 |
| 2 | 25% | 2,482 | 2% | 38 | 13% | 399 | 14% | 447 | 19% | 3,366 |
| 3 | 23% | 2,276 | 0% | 0 | 5% | 159 | 25% | 777 | 18% | 3,211 |
| 4 | 4% | 393 | 79% | 1,234 | 66% | 2,002 | 11% | 342 | 22% | 3,971 |
| 5 | 22% | 2,172 | 16% | 256 | 14% | 418 | 33% | 1,022 | 22% | 3,868 |
| 6 | 21% | 2,066 | 2% | 32 | 2% | 69 | 14% | 453 | 15% | 2,620 |
| Total | 100% | 10,008 | 100% | 1,560 | 100% | 3,047 | 100% | 3,141 | 100% | 17,756 |

3.3. Water Resources Management Model Calibration
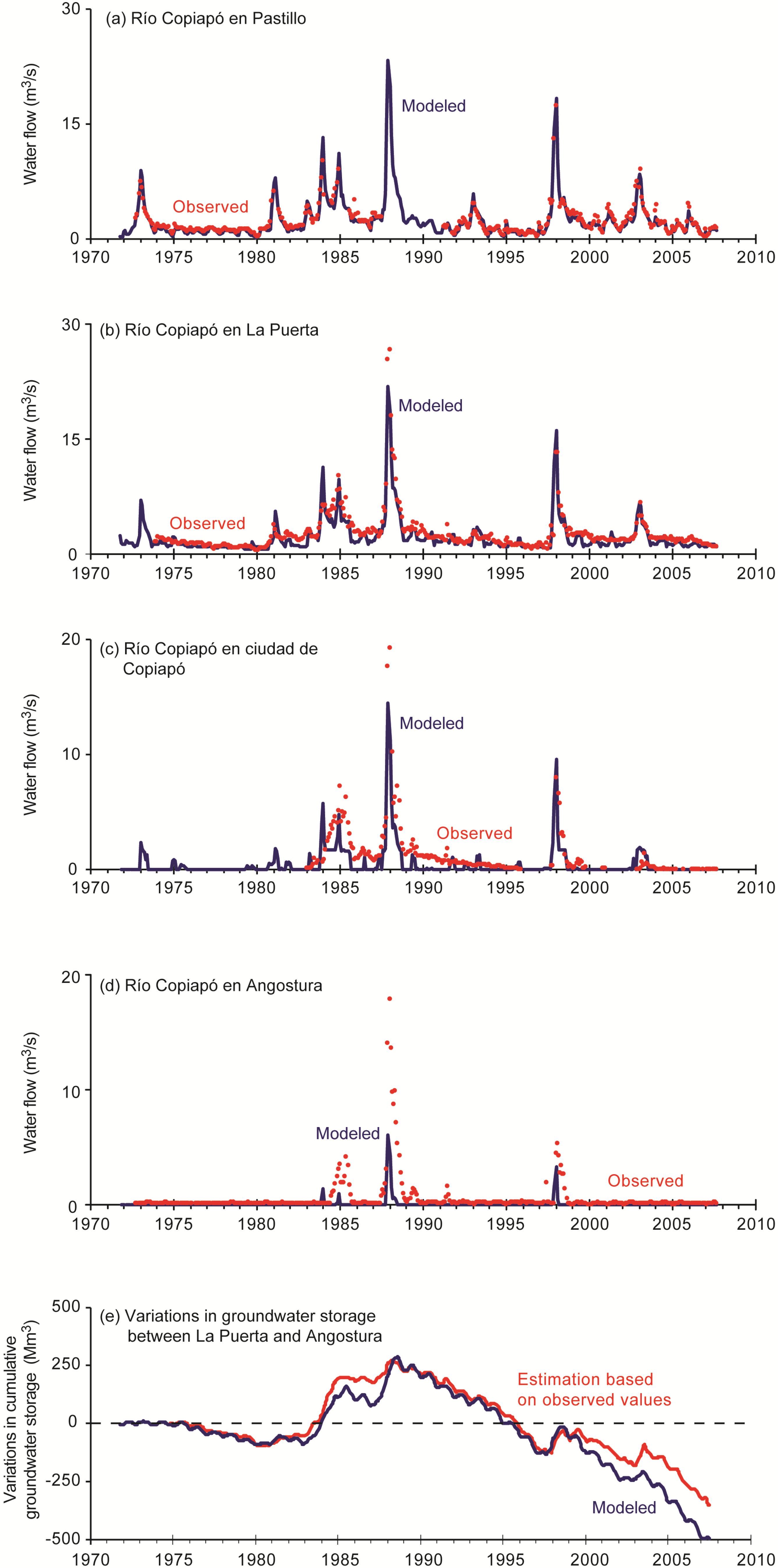
| Surface waters | ||||
|---|---|---|---|---|
| Fluvial stations | Obs. Flow (m3/s) * | Sim. Flow (m3/s) * | E (-) ** | Sim./obs. cumulative flow (m3/s) |
| Río Copiapó en Pastillo | 2.29 ± 1.85 | 2.46 ± 2.64 | 0.905 | 0.932 |
| Río Copiapó en La Puerta | 2.63 ± 2.66 | 2.19 ± 2.28 | 0.493 | 0.832 |
| Río Copiapó en ciudad de Copiapó | 1.47 ± 2.68 | 0.48 ± 1.34 | 0.568 | 0.509 |
| Río Copiapó en Angostura | 0.53 ± 1.69 | 0.06 ± 0.43 | 0.071 | 0.114 |
| Groundwater | ||||
| Sector | Obs. Volume (Mm3) * | Sim. Volume (Mm3)* | Maximum Volume (Mm3) | Sim./obs. Cumulative Volume (Mm3) |
| Sector 1a | 24 ± 3 | 16 ± 9 | 34 | 0.728 |
| Sector 1b | 39 ± 2 | 44 ± 7 | 48 | 1.125 |
| Sector 1c | 38 ± 5 | 38 ± 6 | 66 | 1.104 |
| Sector 1d | 142 ± 9 | 123 ± 29 | 163 | 0.923 |
| Sector 2a | 545 ± 3 | 538 ± 20 | 588 | 0.994 |
| Sector 3a | 187 ± 20 | 176 ± 20 | 257 | 0.941 |
| Sector 3b | 189 ± 18 | 190 ± 23 | 256 | 1.012 |
| Sector 4a | 1036 ± 35 | 1027 ± 55 | 1024 | 0.993 |
| Sector 5a | 2378 ± 56 | 2395 ± 47 | 2583 | 1.009 |
| Sector 6a | 1603 ± 22 | 1607 ± 18 | 1663 | 1.005 |
| Sector 6b | 3131 ± 5 | 3130 ± 6 | 3262 | 1.002 |
| Total | 9944 | |||
3.4. Integrated Water Resource Management Scenarios

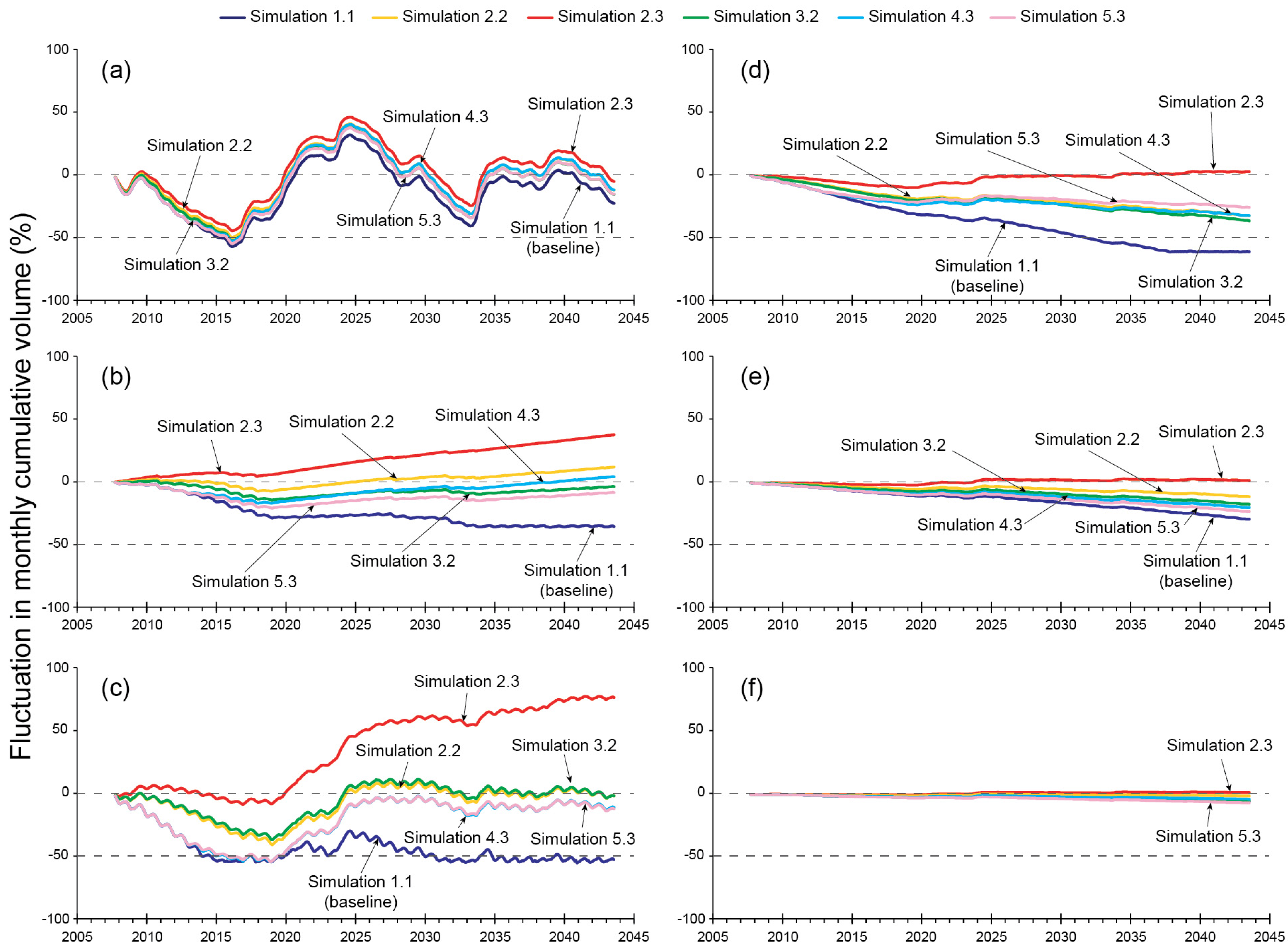
3.5. Impacts of Water Resource Management on Energy Requirements for Water Supply
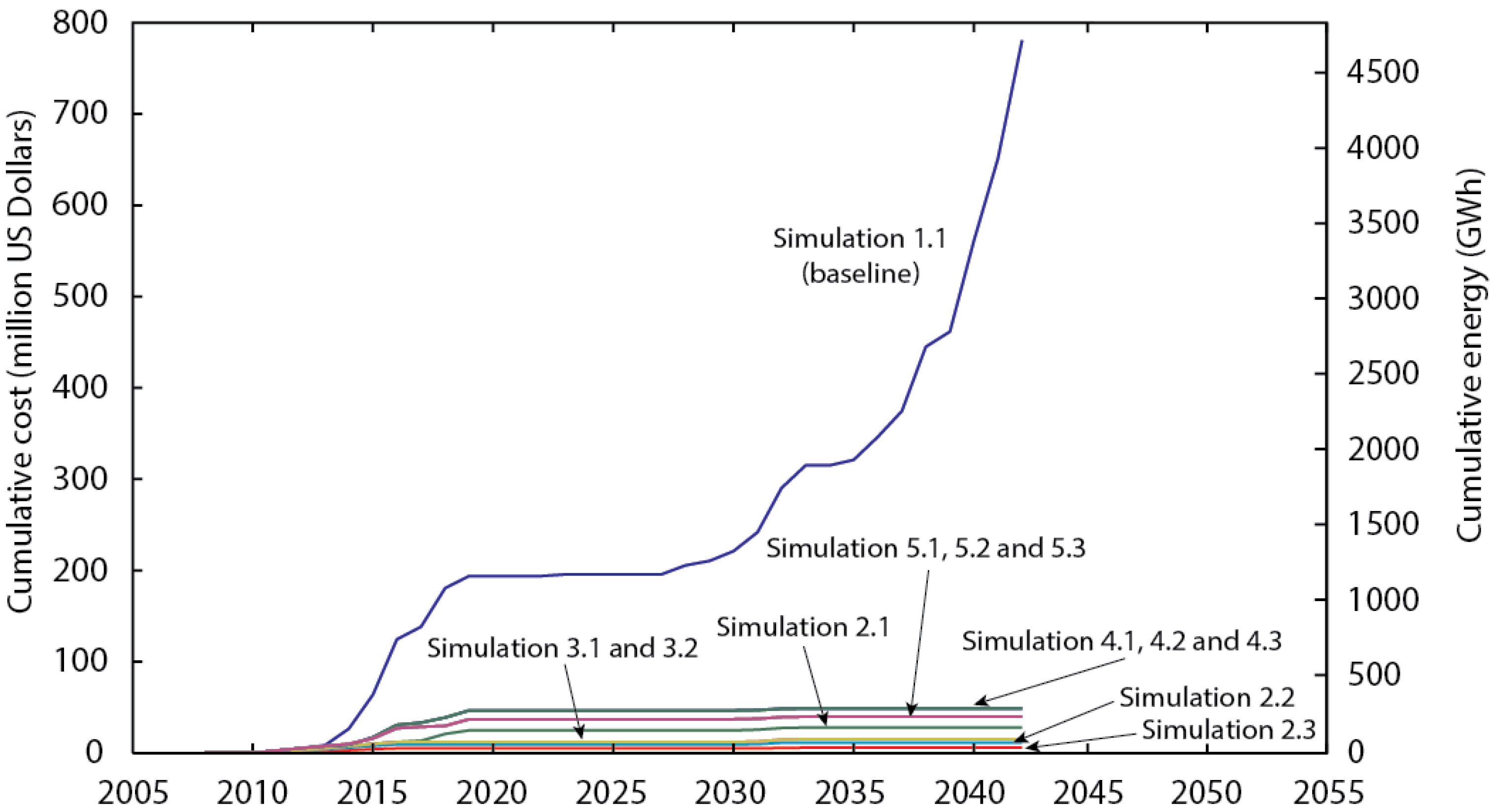
| Scenario | Simulation | Energy for SWRO (GWh) * | Total Energy Required (GWh) * | Cost ($ Million US Dollars) ** |
|---|---|---|---|---|
| 1. Current status. | 1.1 | 2006 (1584–3695) | 4702 (4280–6391) | 781 (710–1061) |
| 2. Uniform reduction of water demand. | 2.1 | 122 (97–225) | 168 (143–271) | 28 (24–45) |
| 2.2 | 52 (41–95) | 70 (59–114) | 12 (10–19) | |
| 2.3 | 26 (20–48) | 35 (30–57) | 6 (5–9) | |
| 3. Segmented reduction of water demand. | 3.1 | 67 (53–123) | 91 (77–147) | 15 (13–24) |
| 3.2 | 67 (53–123) | 91 (77–147) | 15 (13–24) | |
| 4. Water resource management with uniform reduction of water demand and translocation of water between aquifer zones. | 4.1 | 207 (163–381) | 294 (250–468) | 49 (42–78) |
| 4.2 | 207 (164–382) | 294 (250–468) | 49 (42–78) | |
| 4.3 | 207 (163–381) | 294 (250–468) | 49 (42–78) | |
| 5. Water resource management with segmented reduction of water demand and translocation of water between aquifer zones. | 5.1 | 171 (135–316) | 241 (205–385) | 40 (34–64) |
| 5.2 | 171 (135–316) | 241 (205–385) | 40 (34–64) | |
| 5.3 | 171 (135–316) | 241 (205–385) | 40 (34–64) |
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Global Water Partnership. Towards Water Security: A Framework for Action; Global Water Partnership: Stockholm, Sweden, 2000. [Google Scholar]
- Gallego-Ayala, J.; Juízo, D. Integrating stakeholders’ preferences into water resources management planning in the incomati river basin. Water Resour. Manag. 2014, 28, 527–540. [Google Scholar]
- Coelho, A.C.; Labadie, J.W.; Fontane, D.G. Multicriteria decision support system for regionalization of integrated water resources management. Water Resour. Manag. 2012, 26, 1325–1346. [Google Scholar] [CrossRef]
- Malik, R.P.S. Water-energy nexus in resource-poor economies: The Indian experience. Int. J. Water Resour. D 2002, 18, 47–58. [Google Scholar] [CrossRef]
- Sima, L.C.; Kelner-Levine, E.; Eckelman, M.J.; McCarty, K.M.; Elimelech, M. Water flows, energy demand, and market analysis of the informal water sector in Kisumu, Kenya. Ecol. Econ. 2013, 87, 137–144. [Google Scholar] [CrossRef]
- Bindra, S.P.; Hamid, A.; Salem, H.; Hamuda, K.; Abulifa, S. Sustainable integrated water resources management for energy production and food security in Libya. Procedia Technol. 2014, 12, 747–752. [Google Scholar] [CrossRef]
- Vörösmarty, C.J.; Green, P.; Salisbury, J.; Lammers, R.B. Global water resources: Vulnerability from climate change and population growth. Science 2000, 289, 284–288. [Google Scholar]
- Arnell, N.W. Climate change and global water resources: SRES emissions and socio-economic scenarios. Glob. Environ. Chang. 2004, 14, 31–52. [Google Scholar] [CrossRef]
- Pahl-Wostl, C. Transitions towards adaptive management of water facing climate and global change. Water Resour. Manag. 2007, 21, 49–62. [Google Scholar]
- Kalbacher, T.; Delfs, J.; Shao, H.; Wang, W.; Walther, M.; Samaniego, L.; Schneider, C.; Kumar, R.; Musolff, A.; Centler, F.; et al. The IWAS-ToolBox: Software coupling for an integrated water resources management. Environ. Earth Sci. 2012, 65, 1367–1380. [Google Scholar] [CrossRef]
- Hadded, R.; Nouiri, I.; Alshihabi, O.; Maßmann, J.; Huber, M.; Laghouane, A.; Yahiaoui, H.; Tarhouni, J. A decision support system to manage the groundwater of the Zeuss Koutine aquifer using the WEAP-MODFLOW framework. Water Resour. Manag. 2013, 27, 1981–2000. [Google Scholar] [CrossRef]
- Kalbus, E.; Kalbacher, T.; Kolditz, O.; Krüger, E.; Seegert, J.; Röstel, G.; Teutsch, G.; Borchardt, D.; Krebs, P. Integrated water resources management under different hydrological, climatic and socio-economic conditions. Environ. Earth Sci. 2012, 65, 1363–1366. [Google Scholar] [CrossRef]
- Grundmann, J.; Schütze, N.; Gerd H. Schmitz, G.; Al-Shaqsi, S. Towards an integrated arid zone water management using simulation-based optimization. Environ. Earth Sci. 2012, 65, 1381–1394. [Google Scholar] [CrossRef]
- Leidel, M.; Niemann, S.; Hagemann, N. Capacity development as a key factor for integrated water resources management (IWRM): Improving water management in the Western Bug River Basin, Ukraine. Environ. Earth Sci. 2012, 65, 1415–1426. [Google Scholar] [CrossRef]
- Moharram, S.H.; Gad, M.I.; Saafan, T.A.; Khalaf-Allah, S. Optimal groundwater management using genetic algorithm in El-Farafra Oasis, Western Desert, Egypt. Water Resour. Manag. 2012, 26, 927–948. [Google Scholar] [CrossRef]
- Akhbari, M.; Grigg, N.S. A framework for an agent-based model to manage water resources conflicts. Water Resour. Manag. 2013, 27, 4039–4052. [Google Scholar] [CrossRef]
- Razavi-Toosi, S.L.; Samani, J.M.V. Evaluating water transfer projects using analytic network process (ANP). Water Resour. Manag. 2012, 26, 1999–2014. [Google Scholar]
- European Commission. EU Water Framework Directive; (Directive 2000/60/EC); European Parliament and Commission: Brussels, Belgium, 22 December 2000. [Google Scholar]
- Andreu, J.; Ferrer-Polo, J.; Pérez, M.A.; Solera, A. Decision support system for drought planning and management in the Jucar river basin, Spain. In Proceedings of the 18th World IMACS/MODSIM Congress, Cairns, Australia, 13–17 July 2009.
- Wilkinson, R. Integrating water and energy resource management: Progress and opportunities. In Proceedings of the World Environmental and Water Resources Congress, Tampa, FL, USA, 15–19 May 2007.
- Mo, W.; Nasiri, F.; Eckelman, M.J.; Zhang, Q.; Zimmerman, J.B. Measuring the embodied energy in drinking water supply systems: A case study in the Great Lakes Region. Environ. Sci. Technol. 2010, 44, 9516–9521. [Google Scholar] [CrossRef]
- Buytaert, W.; Friesen, J.; Liebe, J.; Ludwig, R. Assessment and management of water resources in developing, semi-arid and arid regions. Water Resour. Manag. 2012, 26, 927–948. [Google Scholar] [CrossRef]
- División de Ingeniería Hidráulica y Ambiental, Pontificia Universidad Católica de Chile (DICTUC). Análisis Integrado de Gestión en Cuenca del río Copiapó; DICTUC: Santiago, Chile, 2010. [Google Scholar]
- Oyarzún, J.; Oyarzún, R. Sustainable development threats, inter-sector conflicts and environmental policy requirements in the arid, mining rich, Northern Chile territory. Sustain. Dev. 2011, 19, 263–274. [Google Scholar]
- Instituto Nacional de Estadísticas (INE). CHILE: Proyecciones y Estimaciones de Población 1950–2050; INE: Santiago, Chile, 2005. [Google Scholar]
- The National Map Viewer and Download Platform. Available online: http://nationalmap.gov/viewer.html (accessed on 1 August 2014).
- Fetter, C.W. Applied Hydrogeology, 4th ed.; Prentice Hall, Inc.: Upper Saddle River, NJ, USA, 2001. [Google Scholar]
- Andreu, J.; Capilla, J.; Sanchis, E. AQUATOOL: A generalized decision support-system for water-resources planning and operational management. J. Hydrol. 1996, 177, 269–291. [Google Scholar] [CrossRef]
- Ochoa-Rivera, J.C.; Andreu, J.; García-Bartual, R. Influence of inflows modeling on management simulation of water resources system. J. Water Res. Pl. ASCE 2007, 133, 106–116. [Google Scholar]
- Paredes-Arquiola, J.; Andreu, J.; Martín-Monerris, M.; Solera, A. Water quantity and quality models applied to the Jucar River basin, Spain. Water Resour. Manag. 2010, 24, 2759–2779. [Google Scholar]
- Paredes-Arquiola, J.; Martínez-Capel, F.; Solera, A.; Aguilella, V. Implementing environmental flows in complex water resources systems—Case study: The Duero River basin, Spain. River Res. Appl. 2011, 29, 451–468. [Google Scholar]
- Pulido-Velázquez, M.; Sahuquillo, A.; Andreu, J. An efficient conceptual model to simulate surface water body-aquifer interaction in conjunctive use management models. Water Resour. Res. 2007, 43. [Google Scholar] [CrossRef]
- Nash, J.E.; Sutcliffe, J.V. River flow forecasting through conceptual models part I–A discussion of principles. J. Hydrol. 1970, 10, 282–290. [Google Scholar] [CrossRef]
- Legates, D.R.; McCabe, G.J., Jr. Evaluating the use of “goodness-of-fit” measures in hydrologic and hydroclimatic model validation. Water Resour. Res. 1999, 35, 233–241. [Google Scholar] [CrossRef]
- Fowler, H.J.; Blenkinsop, S.; Tebaldi, C. Linking climate change modelling to impacts studies: Recent advances in downscaling techniques for hydrological modelling. Int. J. Climatol. 2007, 27, 1547–1578. [Google Scholar] [CrossRef]
- Mahmoudi, H.; Ouagued, A.; Ghaffour, N. Capacity building strategies and policy for desalination using renewable energies in Algeria. Renew. Sust. Energ. Rev. 2009, 13, 921–926. [Google Scholar] [CrossRef]
- Shaffer, D.L.; Yip, N.Y.; Gilron, J.; Elimelech, M. Seawater desalination for agriculture by integrated forward and reverse osmosis: Improved product water quality for potentially less energy. J. Membr. Sci. 2012, 415–416, 1–8. [Google Scholar]
- Ghaffour, N.; Missimer, T.M.; Amy, G.L. Technical review and evaluation of the economics of water desalination: Current and future challenges for better water supply sustainability. Desalination 2013, 309, 197–207. [Google Scholar] [CrossRef]
- Terink, W.; Immerzeel, W.W.; Droogers, P. Climate change projections of precipitation and reference evapotranspiration for the Middle East and Northen Africa until 2050. Int. J. Climatol. 2013, 33, 3055–3072. [Google Scholar] [CrossRef]
- Earman, S.; Dettinger, M. Potential impacts of climate change on groundwater resources—A global review. J. Water Clim. Chang. 2011, 2, 213–229. [Google Scholar] [CrossRef]
- IPCC. Summary for Policymakers. In Climate Change 2013: The Physical Science Basis; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Centro de Despacho Económico de Carga–Estadísticas e informes. Available online: https://www.cdec-sic.cl/est_opera_privada.php (accessed on 2 May 2014).
- Karagiannis, I.C.; Soldatos, P.G. Water desalination cost literature: Review and assessment. Desalination 2008, 223, 448–456. [Google Scholar] [CrossRef]
- Lazarova, V.; Choo, K.; Cornel, P. Water-Energy Interactions in Water Reuse; IWA Publishing: London, UK, 2012. [Google Scholar]
- Olsson, G. Water and Energy. Threads and Opportunities; IWA Publishing: London, UK, 2012. [Google Scholar]
- Duffie, J.A.; Beckham, W.A. Solar Engineering of Thermal Processes, 3rd ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2006. [Google Scholar]
- Gleick, P.H. Water and energy. Annu. Rev. Energy Environ. 1994, 19, 267–299. [Google Scholar] [CrossRef]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Suárez, F.; Muñoz, J.F.; Fernández, B.; Dorsaz, J.-M.; Hunter, C.K.; Karavitis, C.A.; Gironás, J. Integrated Water Resource Management and Energy Requirements for Water Supply in the Copiapó River Basin, Chile. Water 2014, 6, 2590-2613. https://doi.org/10.3390/w6092590
Suárez F, Muñoz JF, Fernández B, Dorsaz J-M, Hunter CK, Karavitis CA, Gironás J. Integrated Water Resource Management and Energy Requirements for Water Supply in the Copiapó River Basin, Chile. Water. 2014; 6(9):2590-2613. https://doi.org/10.3390/w6092590
Chicago/Turabian StyleSuárez, Francisco, José F. Muñoz, Bonifacio Fernández, Jean-Marc Dorsaz, Christian K. Hunter, Christos A. Karavitis, and Jorge Gironás. 2014. "Integrated Water Resource Management and Energy Requirements for Water Supply in the Copiapó River Basin, Chile" Water 6, no. 9: 2590-2613. https://doi.org/10.3390/w6092590
APA StyleSuárez, F., Muñoz, J. F., Fernández, B., Dorsaz, J.-M., Hunter, C. K., Karavitis, C. A., & Gironás, J. (2014). Integrated Water Resource Management and Energy Requirements for Water Supply in the Copiapó River Basin, Chile. Water, 6(9), 2590-2613. https://doi.org/10.3390/w6092590