Effects of Soil and Water Conservation Measures on Groundwater Levels and Recharge
Abstract
:1. Introduction
2. Materials and Methods
2.1. Experimental Conditions and Equipment of Simulated Rainfall Experiments


2.2. Experimental Materials and Monitoring Methods
2.3. MODFLOW
3. Results and Discussion
3.1. Model Calibration and the Key Parameters for Different Measures of Soil and Water Conservation
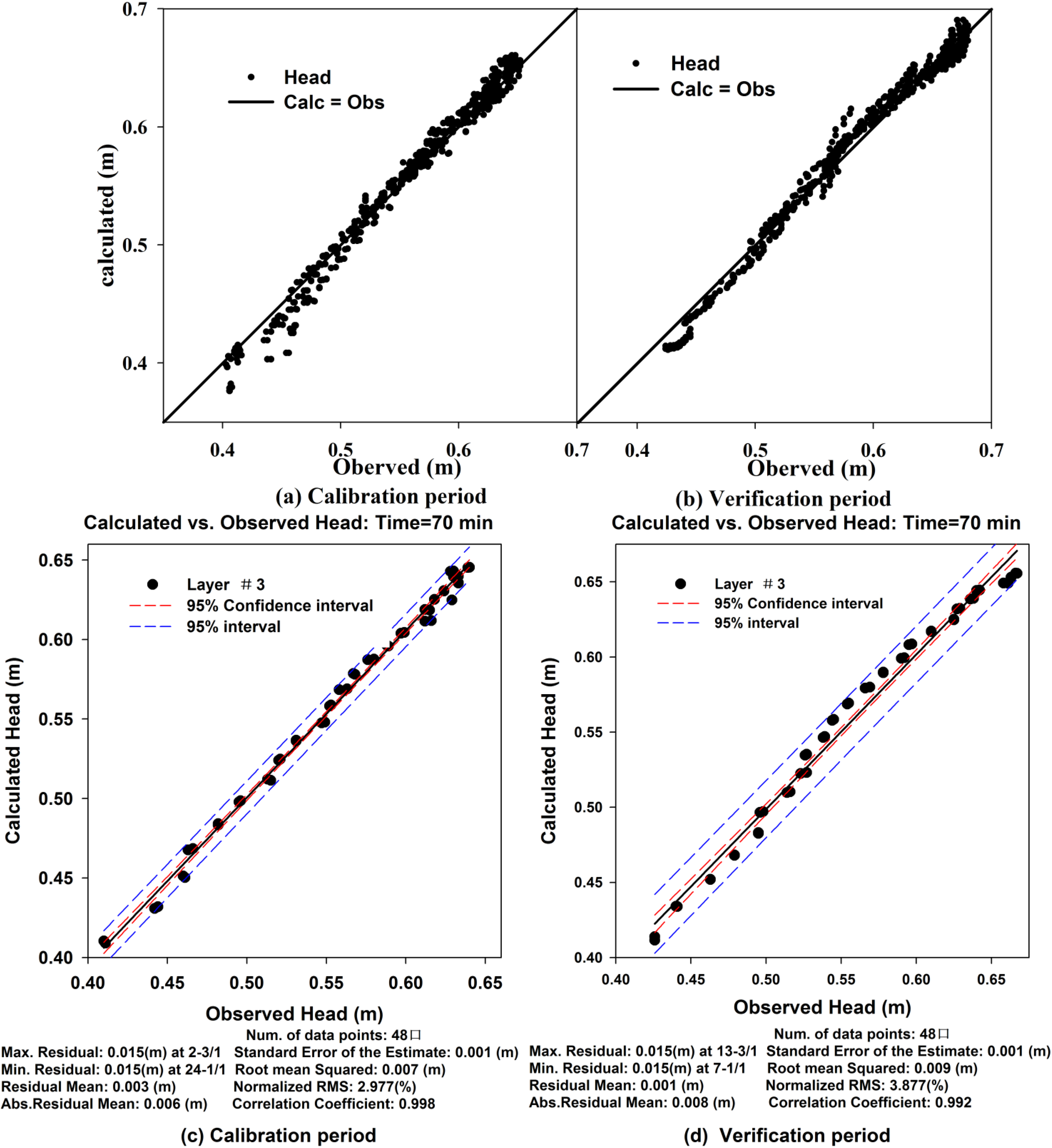

| The Key Parameters | α (10−5 m/s) | Sy1 | Sy2 | Sy3 |
|---|---|---|---|---|
| Bare Slope | 0.68 | 0.36 | 0.36 | 0.36 |
| Grassland | 1.72 | 0.22 | 0.22 | 0.27 |
| Straw Mulching | 1.92 | 0.072 | 0.072 | 0.26 |
3.2. Model Verification for Different Measures of Soil and Water Conservation
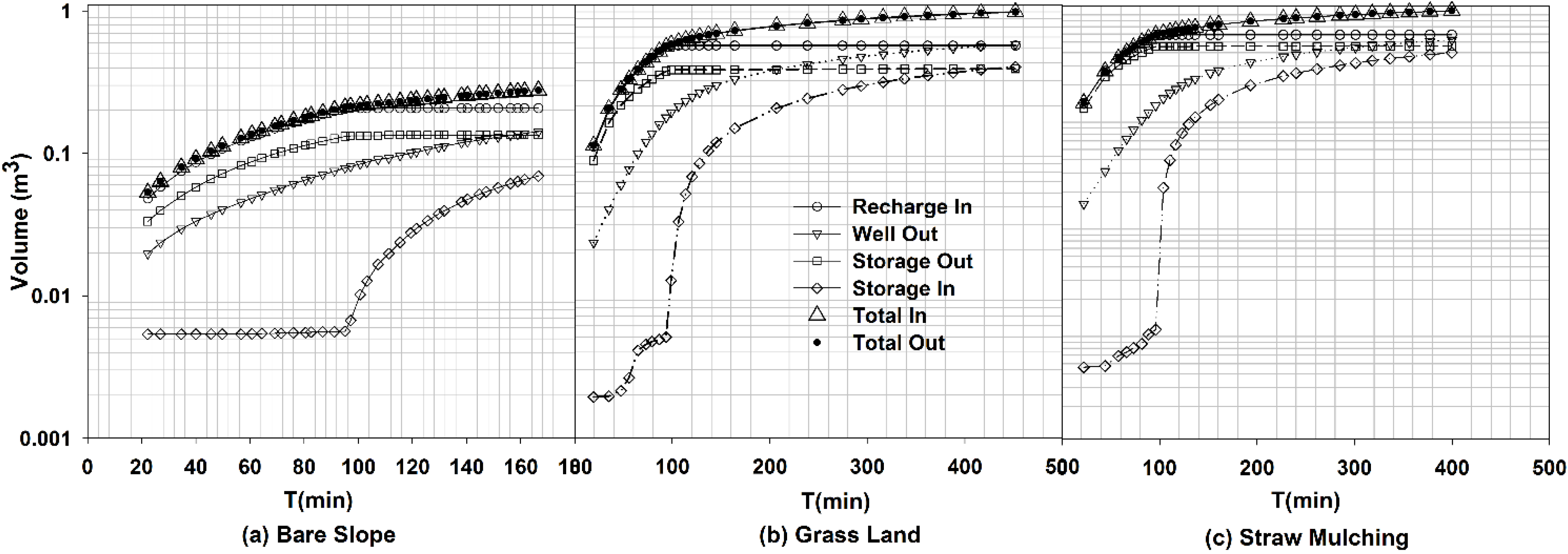
3.2.1. Verification of the Groundwater Balance
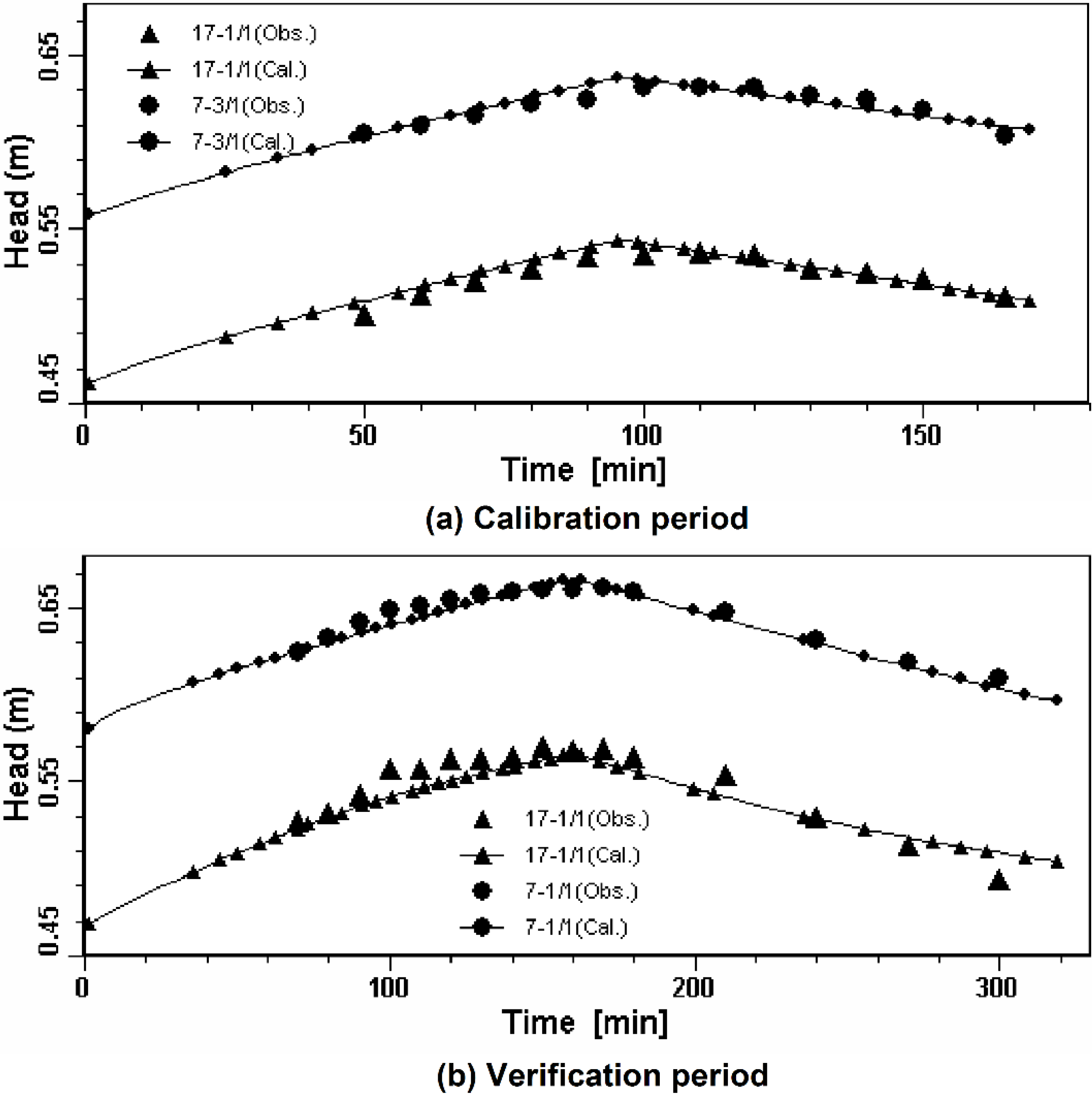
3.2.2. Verification of the Groundwater Level
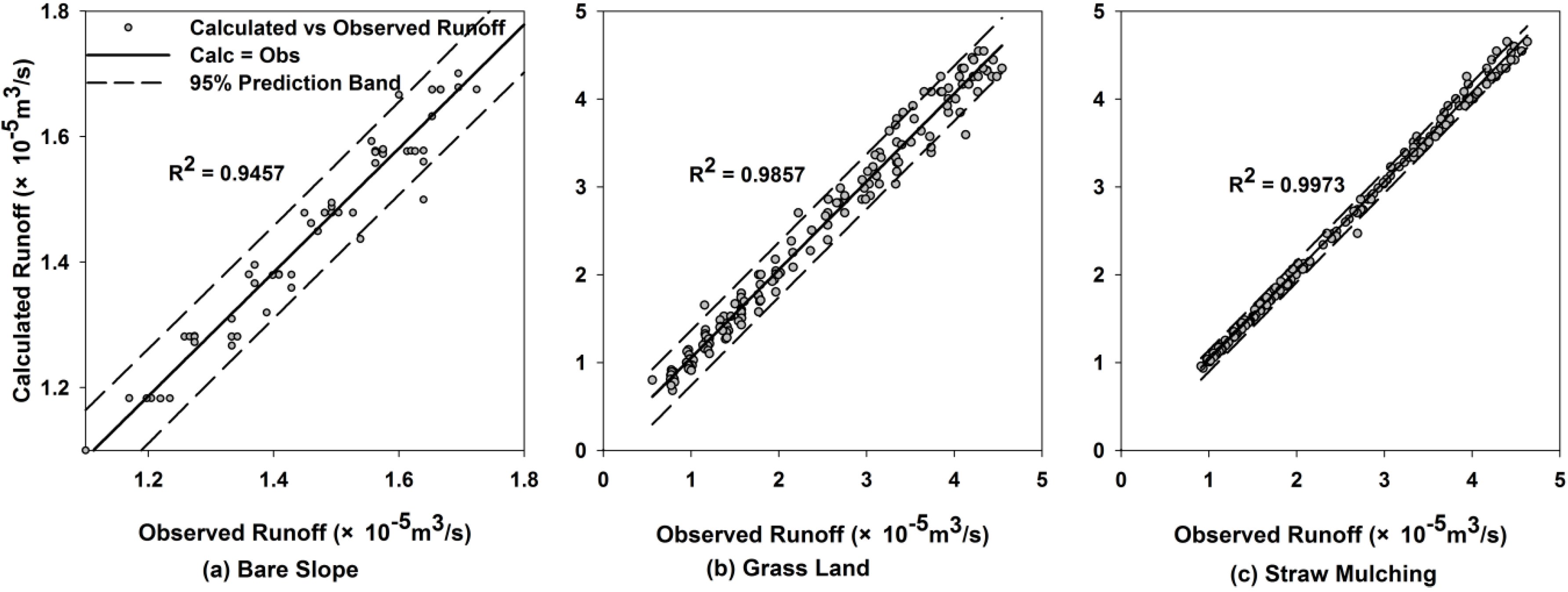
3.2.3. Verification of the Groundwater Runoff
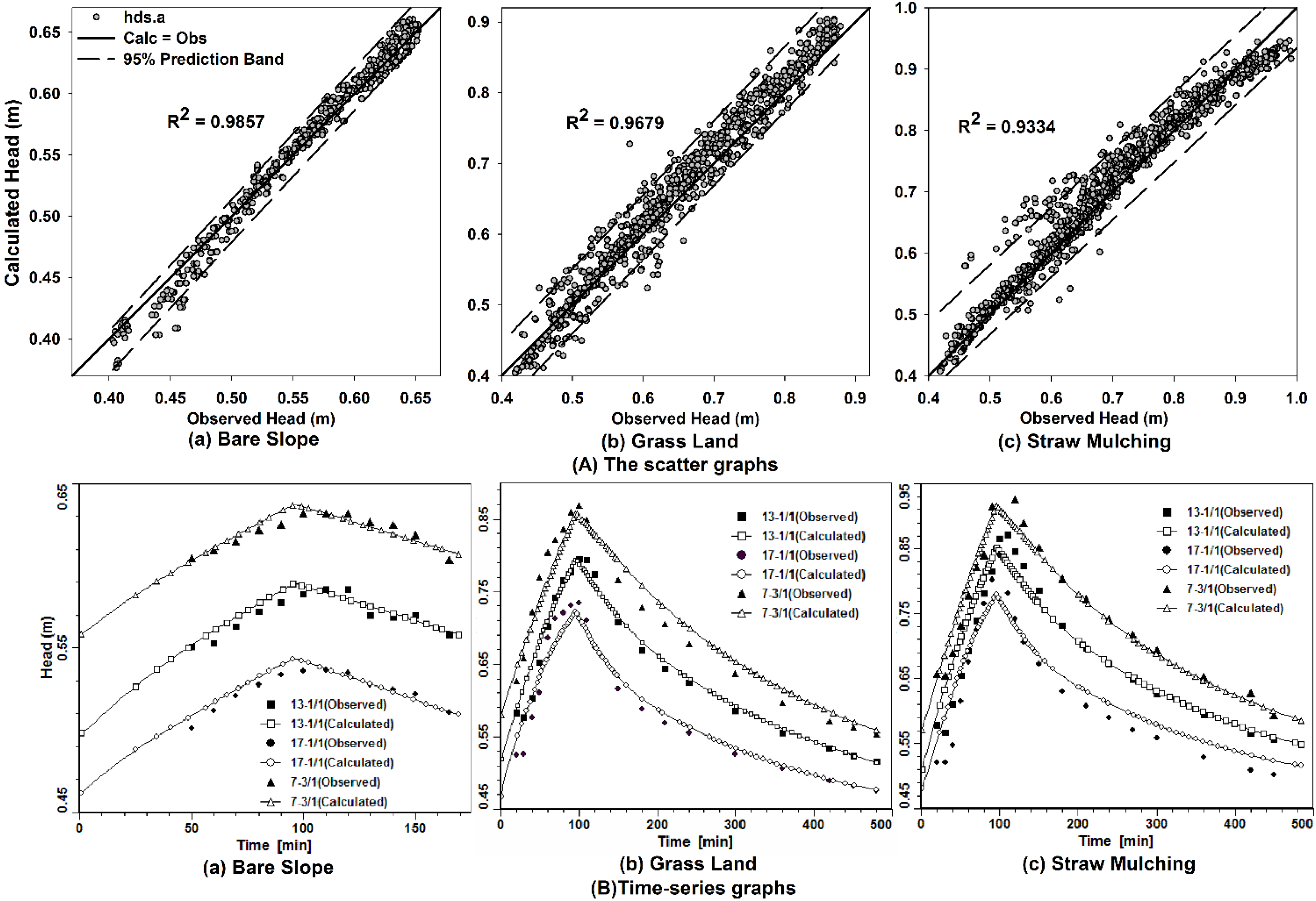
| The Evaluation Indexes | RM/m | ARM/m | SEE/m | RMS/m | NRMS/% | Cor |
|---|---|---|---|---|---|---|
| Bare Slope | 0.0002 | 0.007 | 0.001 | 0.010 | 4.146 | 0.996 |
| Grassland | 0.012 | 0.021 | 0.003 | 0.025 | 8.336 | 0.981 |
| Straw Mulching | 0.018 | 0.028 | 0.004 | 0.035 | 9.932 | 0.983 |
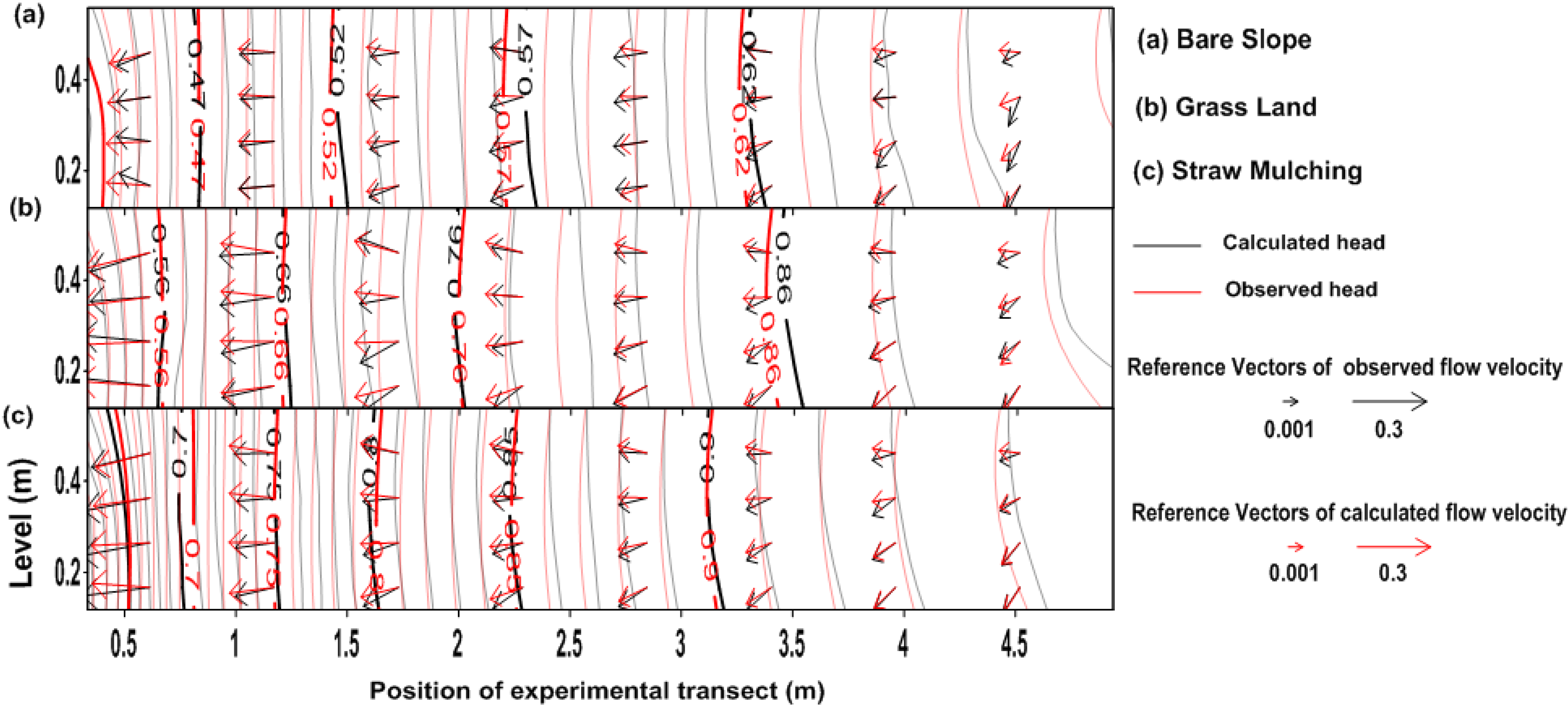
3.2.4. Verification of the Groundwater Flow Field
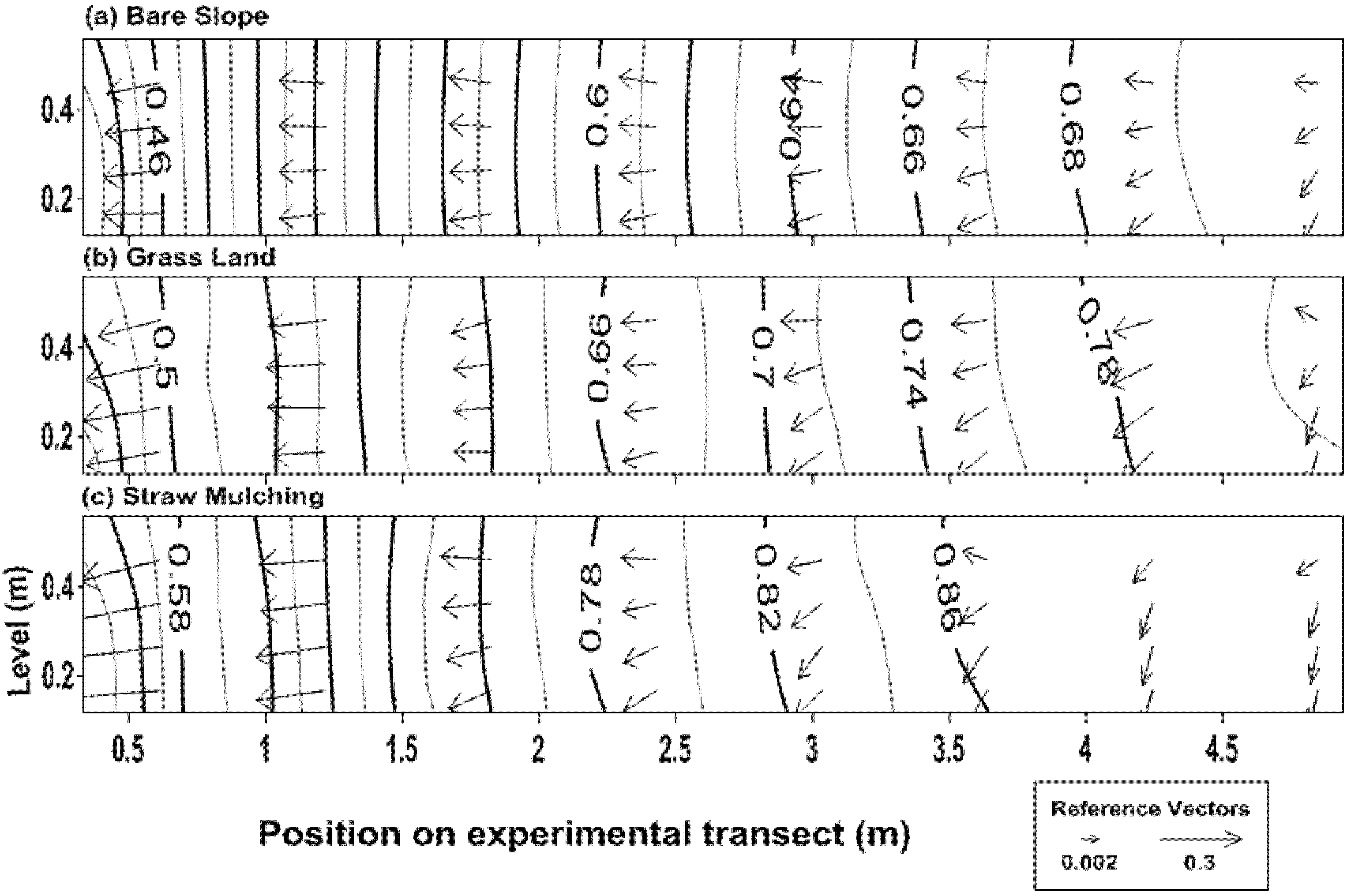
3.3. The Impact of Soil and Water Conservation Measures Construction on Groundwater
3.3.1. Response of Groundwater Recharge and Runoff

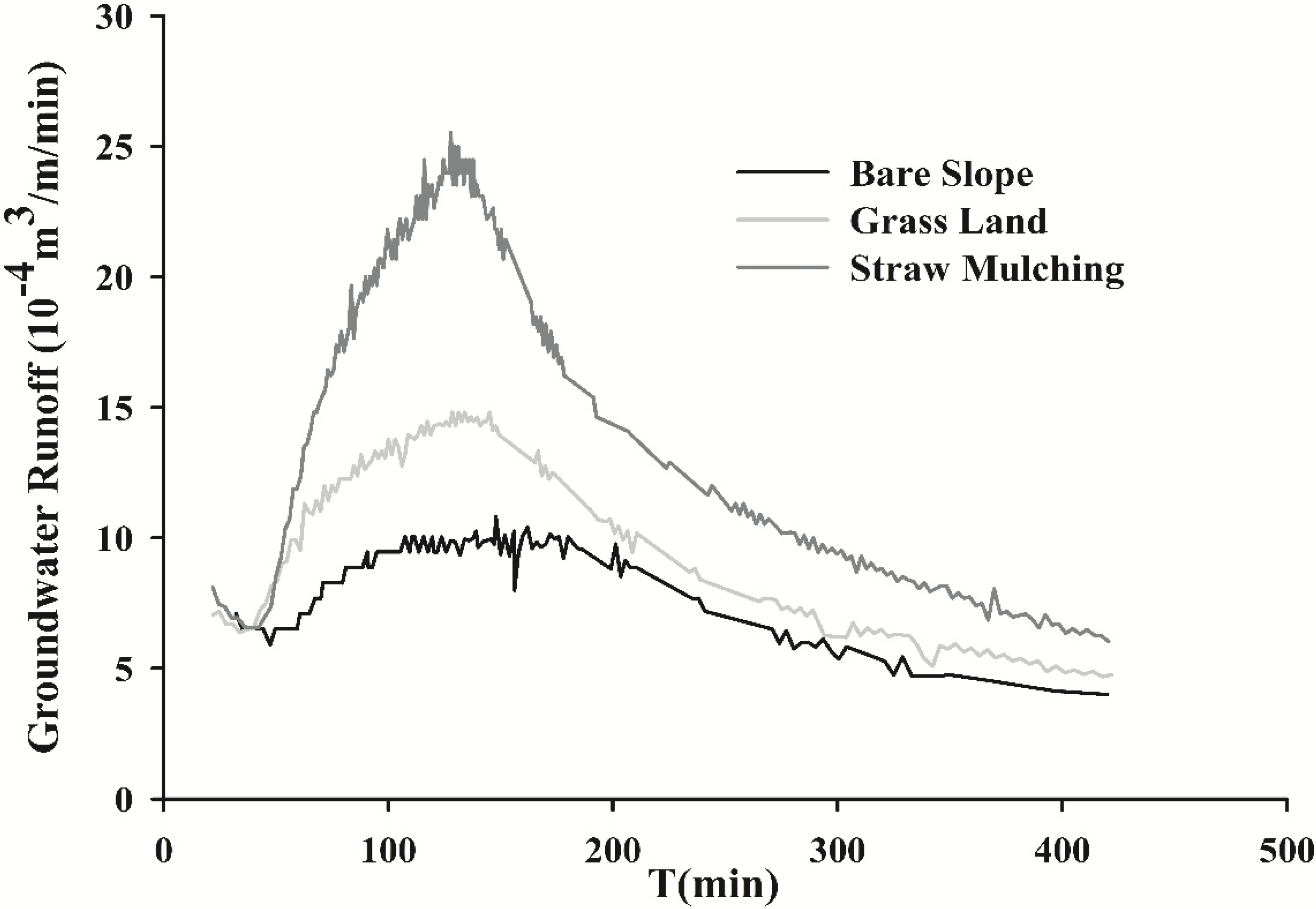
| Scenario | Runoff | ||
|---|---|---|---|
| Value (10−4 m3/m/min) | Change (%) | ||
| Average | Bare Slope | 8.29 | 0 |
| Grassland | 10.19 | 22.9 | |
| Straw Mulching | 15.51 | 87.1 | |
| Maximum | Bare Slope | 10.81 | 0 |
| Grassland | 14.82 | 37.1 | |
| Straw Mulching | 25.53 | 136.2 | |
3.3.2. Response of the Groundwater Level





3.3.3. Response of the Stage-Discharge Relationship of the Groundwater
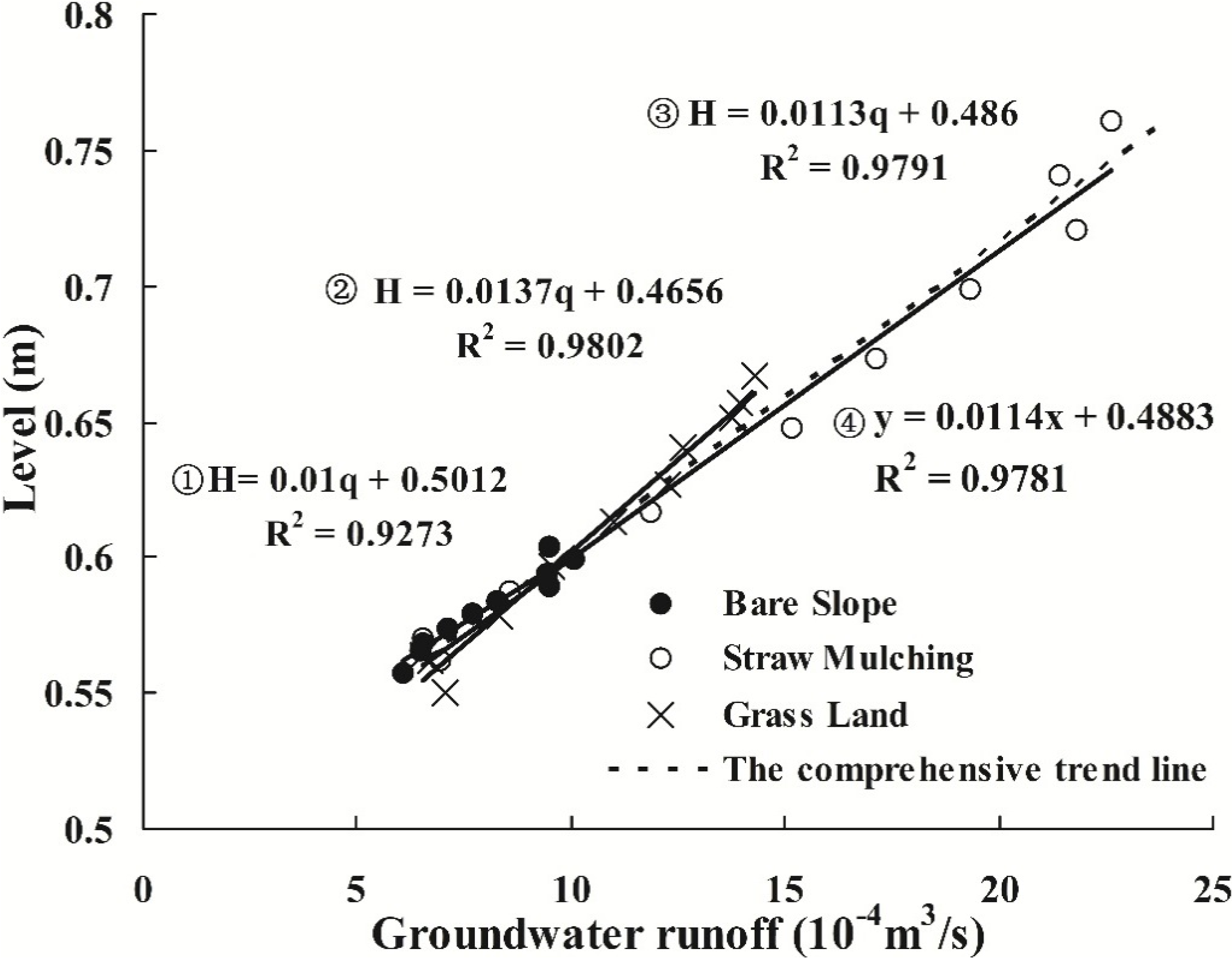
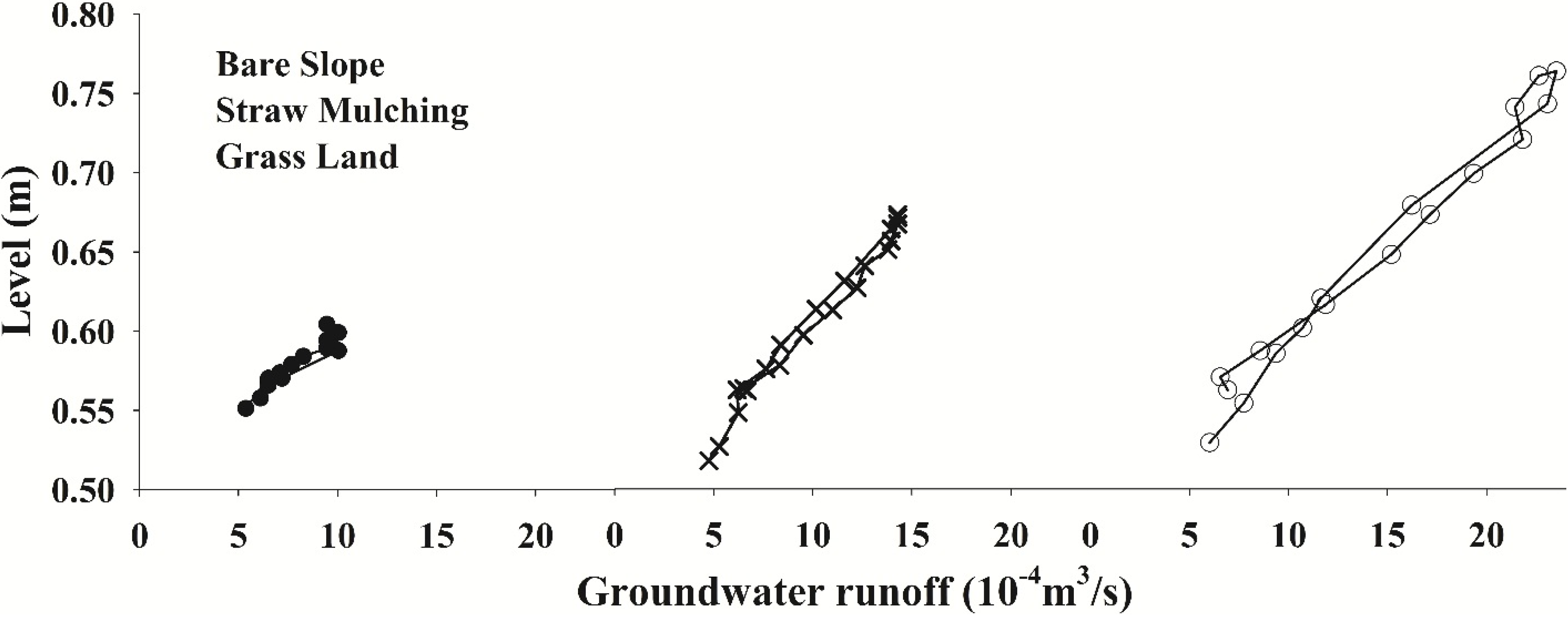
3.4. The Impact of Soil and Water Conservation Measures Destruction on Groundwater
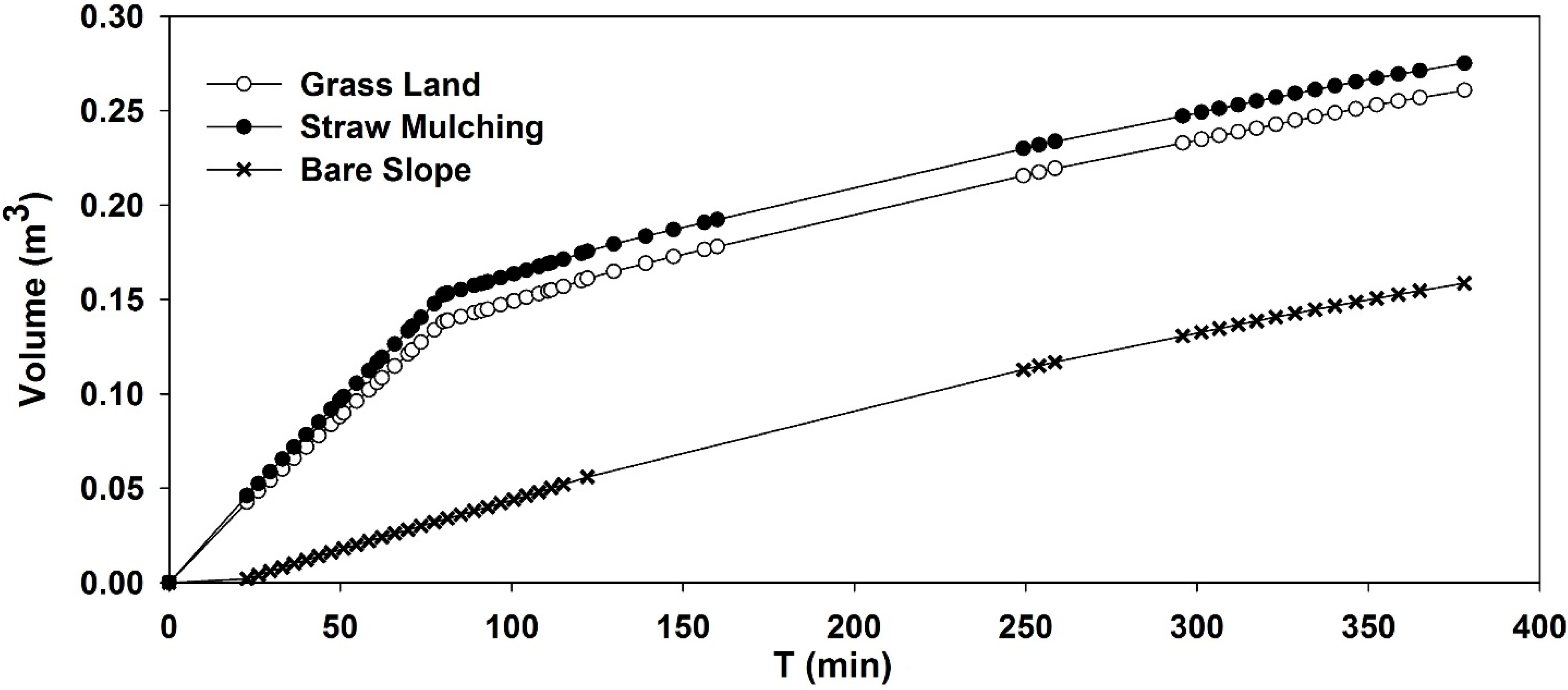

4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Dams, J.; Woldeamlak, S.T.; Batelaan, O. Predicting land-use change and its impact on the groundwater system of the Kleine Nete catchment, Belgium. Hydrol. Earth Syst. Sci. 2008, 12, 1369–1385. [Google Scholar]
- Zhang, Y.K.; Schilling, K.E. Effects of land cover on water table, soil moisture, evapotranspiration, and groundwater recharge: A field observation and analysis. J. Hydrol. 2006, 319, 328–338. [Google Scholar] [CrossRef]
- Eckhardt, D.A.; Stackelberg, P.E. Relation of ground-water quality to land use on Long Island, New York. Ground Water 1995, 33, 1019–1033. [Google Scholar] [CrossRef]
- Collin, M.L.; Melloul, A.J. Combined land-use and environmental factors for sustainable groundwater management. Urban Water 2001, 3, 229–237. [Google Scholar] [CrossRef]
- Jeong, C.H. Effect of land use and urbanization on hydrochemistry and contamination of groundwater from Taejon area, Korea. J. Hydrol. 2001, 253, 194–210. [Google Scholar] [CrossRef]
- Scanlon, B.R.; Reedy, R.C.; Stonestrom, D.A.; Prudic, D.E.; Dennehy, K.F. Impact of land use and land cover change on groundwater recharge and quality in the southwestern US. Glob. Change Biol. 2005, 11, 1577–1593. [Google Scholar] [CrossRef]
- Adekalu, K.; Olorunfemi, I.; Osunbitan, J. Grass mulching effect on infiltration, surface runoff and soil loss of three agricultural soils in Nigeria. Bioresour. Technol. 2007, 98, 912–917. [Google Scholar] [CrossRef] [PubMed]
- Adams, J.E. Influence of mulches on runoff, erosion, and soil moisture depletion. Soil Sci. Soc. Am. J. 1966, 30, 110–114. [Google Scholar] [CrossRef]
- Barnett, A.; Diseker, E.G.; Richardson, E. Evaluation of mulching methods for erosion control on newly prepared and seeded highway backslopes. Agron. J. 1967, 59, 83–85. [Google Scholar] [CrossRef]
- Le Maitre, D.C.; Scott, D.F.; Colvin, C. Review of Information on Interactions between Vegetation and Groundwater; Water Research Commission: Pretoria, South Africa, 1999; Volume 25, pp. 137–152. [Google Scholar]
- Huang, J.; Wu, P.T.; Zhao, X.N. Effects of rainfall intensity, underlying surface and slope gradient on soil infiltration under simulated rainfall experiments. Catena 2012, 25, 137–152. [Google Scholar]
- Black, P.E. Hydrograph responses to geomorphic model watershed characteristics and precipitation variables. J. Hydrol. 1972, 17, 309–329. [Google Scholar] [CrossRef]
- Colman, E.A. Vegetation and watershed management. Soil Sci. 1954, 77, 256. [Google Scholar] [CrossRef]
- Branson, F.A.; Gifford, G.F.; Owen, J.R. Rangeland Hydrology; Society for Range Management: Denver, CO, USA, 1972. [Google Scholar]
- Marston, R.B. Ground cover requirements for summer storm runoff control on aspen sites in northern Utah. J. For. 1952, 50, 303–307. [Google Scholar]
- Moukana, J.A.; Koike, K. Geostatistical model for correlating declining groundwater levels with changes in land cover detected from analyses of satellite images. Comput. Geosci. 2008, 34, 1527–1540. [Google Scholar] [CrossRef]
- He, B.; Wang, Y.; Takase, K.; Mouri, G.; Razafindrabe, B.H. Estimating land use impacts on regional scale urban water balance and groundwater recharge. Water Resour. Manag. 2009, 23, 1863–1873. [Google Scholar] [CrossRef]
- Cho, J.; Barone, V.; Mostaghimi, S. Simulation of land use impacts on groundwater levels and streamflow in a Virginia watershed. Agric. Water Manag. 2009, 96, 1–11. [Google Scholar] [CrossRef]
- Zeigler, B.P.; Praehofer, H.; Kim, T.G. Theory of Modeling and Simulation; John Wiley: New York, NY, USA, 1976. [Google Scholar]
- Suzuki, N.; Murasawa, K.; Sakurai, T.; Nansai, K.; Matsuhashi, K.; Moriguchi, Y.; Tanabe, K.; Nakasugi, O.; Morita, M. Geo-referenced multimedia environmental fate model (G-CIEMS): Model formulation and comparison to the generic model and monitoring approaches. Environ. Sci. Technol. 2004, 38, 5682–5693. [Google Scholar] [CrossRef] [PubMed]
- Xu, X.; Huang, G.H.; Qu, Z.Y.; Pereira, L.S. Using MODFLOW and GIS to assess changes in groundwater dynamics in response to water saving measures in irrigation districts of the Upper Yellow River Basin. Water Resour. Manag. 2011, 25, 2035–2059. [Google Scholar] [CrossRef]
- Wang, H.; Gao, J.E.; Zhang, S.L.; Zhang, M.J.; Li, X.H. Modeling the impact of soil and water conservation on surface and ground water based on the SCS and Visual Modflow. Plos One 2013. [Google Scholar] [CrossRef]
- Zhang, W.Z. The Calculation of the Groundwater Unsteady Flow and Appraisal of Groundwater Resources; Science Press: Beijing, China, 1983. [Google Scholar]
- Liang, W.; Liu, L.; Pan, P. Simulation of nitate-nitrogen transfer in trough scale. Lake Sci. 2007, 19, 710–717. [Google Scholar]
- Visual MODFLOW v. 4.1 User’s Manual; Waterloo Hydrogeologic, Inc.: Waterloo, ON, Canada, 2005.
- Mohanty, S.; Jha, M.K.; Kumar, A.; Panda, D. Comparative evaluation of numerical model and artificial neural network for simulating groundwater flow in Kathajodi-Surua Inter-Basin of Odisha, India. J. Hydrol. 2013, 495, 38–51. [Google Scholar] [CrossRef]
- Wang, S.; Shao, J.; Song, X.; Zhang, Y.; Huo, Z.; Zhou, X. Application of MODFLOW and geographic information system to groundwater flow simulation in North China Plain, China. Environ. Geol. 2008, 55, 1449–1462. [Google Scholar] [CrossRef]
- Freeze, R.A.; Cherry, J.A. Groundwater; Prentice-Hall: Englewood Cliffs, NJ, USA, 1977. [Google Scholar]
- Pei, Y.S. Soil moisture movement and specific yield under groundwater levels increasing uniformly. Hydrogeol. Eng. Geol. 1983, 4, 1–7. [Google Scholar]
- Zhang, W.Z.; Cai, M.J. Indoor water test and numerical simulation of specific yield of homogeneous. Eng. J. Wuhan Univ. 1988, 2, 1–11. [Google Scholar]
- Zhang, W.Z.; Zhang, Y.F. specific yield and freedom porosity of soil. J. Irrig. Drain. Eng. 1983, 3, 1–16. [Google Scholar]
- Lei, Z.D.; Xie, S.C.; Yang, S.X.; Li, H.Z. The preliminary investigation of the specific yield. J. Hydraul. Eng. 1984, 5, 10–17. [Google Scholar]
- Paul, M.J. Impact of land-use patterns on distributed groundwater recharge and discharge. Chin. Geogr. Sci. 2006, 16, 229–235. [Google Scholar]
- Jain, S.; Chalisgaonkar, D. Setting up stage-discharge relations using ANN. J. Hydrol. Eng. 2000, 5, 428–433. [Google Scholar] [CrossRef]
- Habib, E.H.; Meselhe, E.A. Stage–discharge relations for low-gradient tidal streams using data-driven models. J. Hydraul. Eng. 2006, 132, 482–492. [Google Scholar] [CrossRef]
- Kumar, A. Stage-Discharge Relationship; Springer Science+Business Media BV: Chandigarh, India, 2011. [Google Scholar]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, H.; Gao, J.; Li, X.; Wang, H.; Zhang, Y. Effects of Soil and Water Conservation Measures on Groundwater Levels and Recharge. Water 2014, 6, 3783-3806. https://doi.org/10.3390/w6123783
Wang H, Gao J, Li X, Wang H, Zhang Y. Effects of Soil and Water Conservation Measures on Groundwater Levels and Recharge. Water. 2014; 6(12):3783-3806. https://doi.org/10.3390/w6123783
Chicago/Turabian StyleWang, Hong, Jianen Gao, Xinghua Li, Hongjie Wang, and Yuanxing Zhang. 2014. "Effects of Soil and Water Conservation Measures on Groundwater Levels and Recharge" Water 6, no. 12: 3783-3806. https://doi.org/10.3390/w6123783
APA StyleWang, H., Gao, J., Li, X., Wang, H., & Zhang, Y. (2014). Effects of Soil and Water Conservation Measures on Groundwater Levels and Recharge. Water, 6(12), 3783-3806. https://doi.org/10.3390/w6123783




