An Experimental Investigation on Inclined Negatively Buoyant Jets
Abstract
:Notations
| A | Cross-sectional area, m2 |
| Bo | Buoyancy flux the nozzle, m4/s3 |
| D | Mixing tank diameter, m |
| do | Nozzle diameter, m |
| g | Acceleration due to gravity, m/s2 |
| g΄ | Effective acceleration due to gravity, m/s2 |
| H | Mixing tank depth, m |
| K | Constant |
| k | Slope coeff. (kxi, ky ,kxy ,kym, kxym, kxe), m |
| L | Mixing tank length, m |
| l | Characteristic length scale (lM,lM) |
| Mo | Momentum flux at the nozzle, m4/s2 |
| Qo | Volume flux at the nozzle, m3/s |
| S | Nozzle salinity percentage, % |
| uo | Nozzle velocity, m/s |
| W | Mixing tank width, m |
| Xe | Edge point horizontal distance, m |
| Xi | Jet impact point distance, m |
| Xy | Horizontal distance to jet centerline max. level, m |
| Xym | Horizontal distance to maximum jet edge level, m |
| Y | Trajectory centerline maximum, m |
| Ym | Maximum jet edge level, m |
| Greek Symbols | |
|---|---|
| α | Empirical coefficient |
| θ | Initial jet angle, ° |
| ρo | Effluent density, kg/m3 |
| ρa | Ambient density, kg/m3 |
| Ψ | function |
| Subscripts | |
|---|---|
| a | Ambient |
| 0 | Reference value |
| Exponents | |
|---|---|
| m | Empirical coefficient |
| n | Empirical coefficient |
| Notations | |
|---|---|
| Cip. | Cipollina et al. (2005) |
| Kik. | Kikkert et al. (2007) |
| LA | Light attenuation system |
| LIF | Laser induced fluorescence system |
| Non-dimensional Numbers | |
|---|---|
| F | Jet densimetric Froude number |
| R | Jet initial Reynolds number |
1. Introduction
1.1. Background and Previous Studies
1.2. Objectives
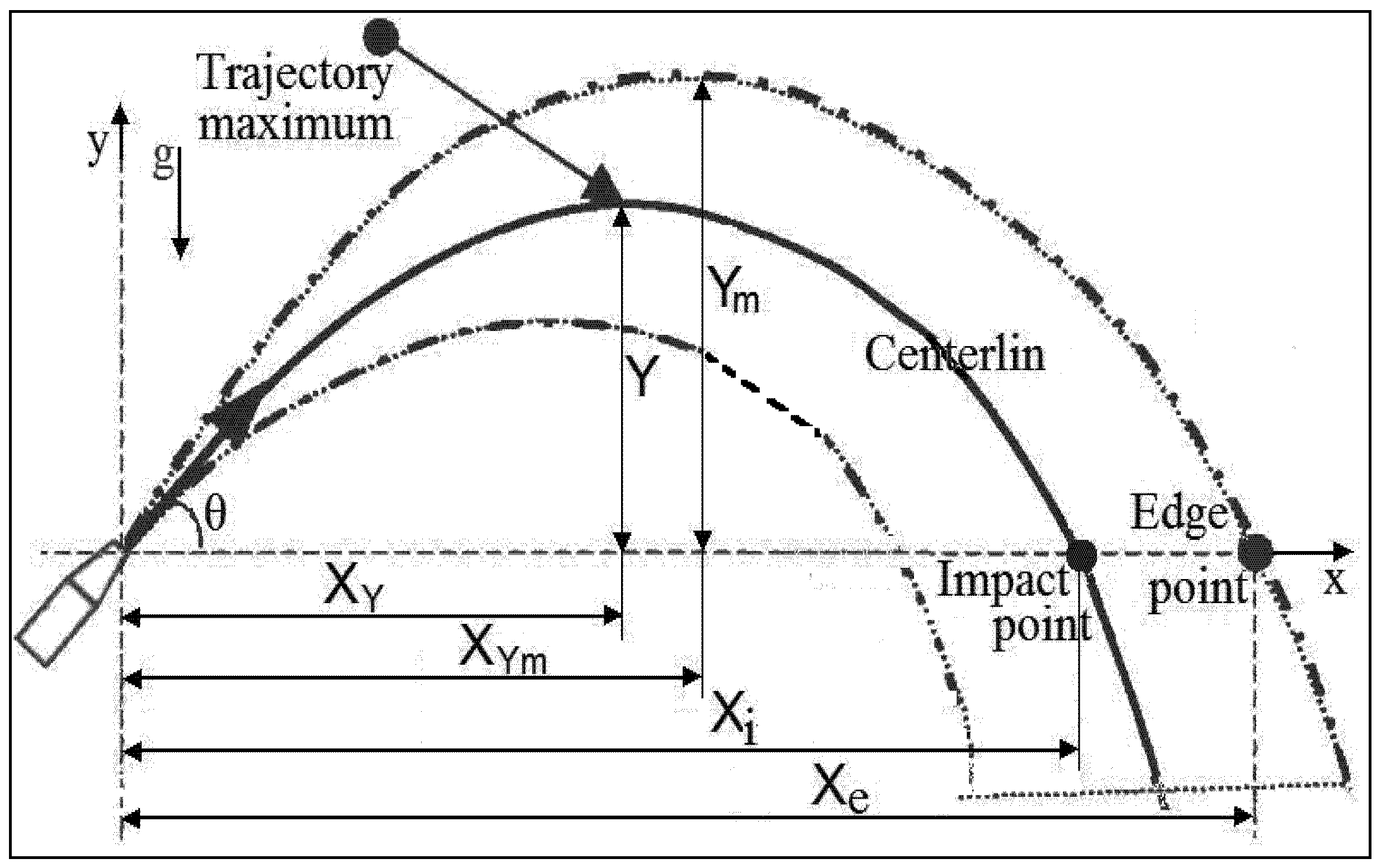
2. Dimensional Considerations and Review of Main Jet Properties
2.1. Dimensional Analysis of Negatively Buoyant Jets

 is the modified acceleration due to gravity. A dimensional analysis involving Qo, Mo, and Bo yields two length scales that may be used to normalize the above-mentioned geometric quantities and to develop empirically based predictive relationships [24]:
is the modified acceleration due to gravity. A dimensional analysis involving Qo, Mo, and Bo yields two length scales that may be used to normalize the above-mentioned geometric quantities and to develop empirically based predictive relationships [24]:
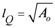 and if the nozzle is circular
and if the nozzle is circular  .
.


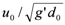 ). Thus, (Equation 4) can be rewritten:
). Thus, (Equation 4) can be rewritten: 
 ; Similar equations may be developed for the other geometric jet quantities Ym, Xy, Xym, and Xe, but with different values of the coefficient k.
; Similar equations may be developed for the other geometric jet quantities Ym, Xy, Xym, and Xe, but with different values of the coefficient k.2.2. Previous Results for Geometric Jet Quantities
3. Laboratory Experiments
3.1. Experimental Setup

3.2. Experimental Procedure and Data Collected
| Case | θ0 | S (%) | d0 (cm) | Qo (L/min) | Qo × 10−5 (m3/s) | uo (m/s) | ρo (kg/m3) | R | F | Y (cm) | Xy (cm) | Ym (cm) | Xym (cm) | Xe (cm) |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 30 | 4 | 0.48 | 0.97 | 1.6 | 0.9 | 1023.7 | 3681 | 25 | 8.5 | 19.5 | 11 | 25 | 51 |
| 2 | 30 | 6 | 0.48 | 1.20 | 2.0 | 1.1 | 1034.8 | 4458 | 26 | 10 | 21.5 | 13.5 | 25.5 | 47 |
| 3 | 30 | 2 | 0.48 | 1.00 | 1.7 | 0.9 | 1011.1 | 3861 | 34 | 8 | 18 | 11 | 23 | 37 |
| 4 | 30 | 6 | 0.33 | 0.88 | 1.5 | 1.7 | 1034.8 | 4755 | 49 | 11 | 30 | 15.5 | 35 | 57 |
| 5 | 30 | 6 | 0.48 | 2.37 | 4.0 | 2.2 | 1034.8 | 8805 | 52 | 17 | 45 | 23.5 | 50 | 82 |
| 6 | 30 | 4 | 0.48 | 2.05 | 3.4 | 1.9 | 1023.7 | 7779 | 53 | 17.5 | 39 | 22 | 49 | 83 |
| 7 | 30 | 2 | 0.48 | 1.75 | 2.9 | 1.6 | 1011.1 | 6757 | 60 | 19 | 47 | 27.5 | 59 | 85.5 |
| 8 | 30 | 4 | 0.33 | 1.02 | 1.7 | 2.0 | 1023.7 | 5630 | 67 | 15.5 | 41.5 | 20 | 50 | 71 |
| 9 | 30 | 6 | 0.23 | 0.58 | 1.0 | 2.3 | 1034.8 | 4497 | 80 | 14.5 | 28 | 18.5 | 32 | 53.5 |
| 10 | 30 | 6 | 0.33 | 1.45 | 2.4 | 2.8 | 1034.8 | 7835 | 81 | 18 | 44 | 25 | 49 | 84 |
| 11 | 30 | 4 | 0.33 | 1.41 | 2.4 | 2.7 | 1023.7 | 7783 | 92 | 19 | 48 | 25.5 | 57 | 90 |
| 12 | 30 | 4 | 0.23 | 0.60 | 1.0 | 2.4 | 1023.7 | 4752 | 97 | 9 | 19 | 11.5 | 22 | 41 |
| 13 | 45 | 6 | 0.48 | 0.96 | 1.6 | 0.9 | 1034.8 | 3566 | 21 | 10 | 15.5 | 14 | 18.5 | 36 |
| 14 | 45 | 4 | 0.48 | 1.25 | 2.1 | 1.2 | 1023.7 | 4744 | 32 | 16 | 27.5 | 20.5 | 31.5 | 52 |
| 15 | 45 | 2 | 0.48 | 1.00 | 1.7 | 0.9 | 1011.1 | 3861 | 34 | 8 | 12 | 11 | 12.5 | 22.5 |
| 16 | 45 | 6 | 0.48 | 2.38 | 4.0 | 2.2 | 1034.8 | 8842 | 52 | 25 | 38 | 33.5 | 48 | 75 |
| 17 | 45 | 4 | 0.33 | 0.82 | 1.4 | 1.6 | 1023.7 | 4526 | 54 | 17 | 29 | 21.5 | 37 | 54 |
| 18 | 45 | 4 | 0.48 | 2.10 | 3.5 | 1.9 | 1023.7 | 7969 | 54 | 25 | 44.5 | 33 | 52 | 81 |
| 19 | 45 | 6 | 0.33 | 0.98 | 1.6 | 1.9 | 1034.8 | 5296 | 55 | 17.5 | 25 | 23 | 31 | 57 |
| 20 | 45 | 2 | 0.48 | 1.75 | 2.9 | 1.6 | 1011.1 | 6757 | 60 | 28 | 41.5 | 37 | 45 | 77.5 |
| 21 | 45 | 2 | 0.33 | 0.80 | 1.3 | 1.6 | 1011.1 | 4493 | 70 | 8 | 13 | 11 | 15.5 | 23.5 |
| 22 | 45 | 6 | 0.23 | 0.52 | 0.9 | 2.1 | 1034.8 | 4032 | 71 | 16 | 25 | 20.5 | 28.5 | 50 |
| 23 | 45 | 6 | 0.33 | 1.46 | 2.4 | 2.8 | 1034.8 | 7890 | 81 | 29.5 | 46 | 37 | 56.5 | 91 |
| 24 | 45 | 4 | 0.23 | 0.51 | 1.0 | 2.0 | 1023.7 | 4039 | 82 | 17 | 30 | 21 | 34.5 | 55 |
| 25 | 45 | 4 | 0.33 | 1.40 | 2.3 | 2.7 | 1023.7 | 7728 | 92 | 26 | 51 | 34.5 | 62 | 94 |
| 26 | 45 | 6 | 0.15 | 0.24 | 0.4 | 2.3 | 1034.8 | 2853 | 96 | 15 | 25 | 19 | 26 | 42.5 |
| 27 | 60 | 4 | 0.48 | 1.00 | 1.7 | 0.9 | 1023.7 | 3795 | 26 | 14.5 | 14.5 | 18 | 16 | 32 |
| 28 | 60 | 6 | 0.48 | 1.22 | 2.0 | 1.1 | 1034.8 | 4532 | 27 | 17.5 | 15 | 23.5 | 19 | 35 |
| 29 | 60 | 6 | 0.33 | 0.70 | 1.2 | 1.4 | 1034.8 | 3783 | 39 | 17 | 18.5 | 21.5 | 21.5 | 39 |
| 30 | 60 | 2 | 0.48 | 1.25 | 2.1 | 1.2 | 1011.1 | 4826 | 43 | 17 | 17.5 | 25 | 23.5 | 36.5 |
| 31 | 60 | 4 | 0.48 | 1.75 | 2.9 | 1.6 | 1023.7 | 6641 | 45 | 29 | 27 | 37 | 34.5 | 63 |
| 32 | 60 | 6 | 0.48 | 2.20 | 3.7 | 2.0 | 1034.8 | 8173 | 48 | 33 | 27 | 41 | 31.5 | 58 |
| 33 | 60 | 4 | 0.33 | 0.86 | 1.4 | 1.7 | 1023.7 | 4747 | 56 | 26 | 26 | 34.5 | 31.5 | 53 |
| 34 | 60 | 2 | 0.48 | 1.70 | 2.8 | 1.6 | 1011.1 | 6564 | 58 | 34 | 35.5 | 43 | 42.5 | 69 |
| 35 | 60 | 2 | 0.33 | 0.80 | 1.3 | 1.6 | 1011.1 | 4493 | 70 | 13.5 | 14 | 16 | 16.5 | 29.5 |
| 36 | 60 | 6 | 0.33 | 1.27 | 2.1 | 2.5 | 1034.8 | 6863 | 71 | 31 | 31.5 | 40 | 36 | 47 |
| 37 | 60 | 4 | 0.33 | 1.10 | 1.8 | 2.1 | 1023.7 | 6072 | 72 | 38 | 35 | 45 | 40 | 75 |
| 38 | 60 | 6 | 0.23 | 0.60 | 1.0 | 2.4 | 1034.8 | 4652 | 82 | 25.5 | 28 | 31.5 | 31 | 52 |
| 39 | 60 | 4 | 0.23 | 0.57 | 1.0 | 2.3 | 1023.7 | 4514 | 92 | 26 | 27.5 | 31 | 33 | 56 |
| 40 | 30 | 2 | 0.33 | 1.20 | 2.0 | 2.3 | 1011.1 | 6739 | 105 | 14 | 26.5 | 17 | 31 | 59 |
| 41 | 30 | 6 | 0.23 | 0.82 | 1.4 | 3.3 | 1034.8 | 6358 | 113 | 22 | 41 | 27.5 | 48 | 79 |
| 42 | 30 | 6 | 0.15 | 0.30 | 0.5 | 2.8 | 1034.8 | 3566 | 120 | 8 | 24 | 12 | 29 | 50 |
| 43 | 30 | 2 | 0.33 | 1.80 | 3.0 | 3.5 | 1011.1 | 10109 | 158 | 25.5 | 48 | 35.5 | 66.5 | 96.5 |
| 44 | 30 | 4 | 0.23 | 1.00 | 1.7 | 4.0 | 1023.7 | 7920 | 162 | 20 | 50 | 25 | 58 | 89.5 |
| 45 | 30 | 6 | 0.15 | 0.42 | 0.7 | 4.0 | 1034.8 | 4993 | 168 | 12 | 32 | 15 | 39 | 63.5 |
| 46 | 30 | 2 | 0.23 | 0.85 | 1.4 | 3.4 | 1011.1 | 6849 | 184 | 9 | 23.5 | 11.5 | 27.5 | 55 |
| 47 | 30 | 2 | 0.23 | 1.00 | 1.7 | 4.0 | 1011.1 | 8058 | 216 | 20 | 53 | 25.5 | 58 | 102 |
| 48 | 30 | 2 | 0.15 | 0.35 | 0.6 | 3.3 | 1011.1 | 4324 | 220 | 8.5 | 23 | 11 | 28 | 41 |
| 49 | 30 | 4 | 0.15 | 0.65 | 1.1 | 6.1 | 1023.7 | 7893 | 306 | 13 | 28 | 16 | 33 | 53 |
| 50 | 30 | 4 | 0.15 | 1.00 | 1.7 | 9.4 | 1023.7 | 12143 | 471 | 18 | 38 | 22.5 | 44.5 | 72 |
| 51 | 30 | 2 | 0.15 | 0.85 | 1.4 | 8.0 | 1011.1 | 10502 | 535 | 21.5 | 50 | 27.5 | 56 | 92.5 |
| 52 | 45 | 6 | 0.23 | 0.76 | 1.3 | 3.0 | 1034.8 | 5892 | 104 | 24 | 41 | 27.5 | 48 | 82 |
| 53 | 45 | 2 | 0.33 | 1.45 | 2.4 | 2.8 | 1011.1 | 8143 | 127 | 30 | 42.5 | 40 | 52.5 | 88.5 |
| 54 | 45 | 2 | 0.23 | 0.60 | 1.0 | 2.4 | 1011.1 | 4835 | 130 | 11 | 20 | 16 | 26 | 40.5 |
| 55 | 45 | 4 | 0.23 | 0.90 | 1.7 | 3.6 | 1023.7 | 7128 | 145 | 30 | 51.5 | 36.5 | 57 | 98 |
| 56 | 45 | 6 | 0.15 | 0.38 | 0.6 | 3.6 | 1034.8 | 4518 | 152 | 22 | 31 | 28 | 36 | 59 |
| 57 | 45 | 2 | 0.23 | 0.85 | 1.4 | 3.4 | 1011.1 | 6849 | 184 | 32 | 49 | 41 | 57 | 97 |
| 58 | 45 | 2 | 0.15 | 0.38 | 0.6 | 3.6 | 1011.1 | 4695 | 239 | 14 | 17 | 17.5 | 19 | 37 |
| 59 | 45 | 4 | 0.15 | 0.65 | 1.1 | 6.1 | 1023.7 | 7893 | 306 | 18 | 24.5 | 24 | 30 | 49 |
| 60 | 45 | 4 | 0.15 | 1.00 | 1.7 | 9.4 | 1023.7 | 12143 | 471 | 26.5 | 37 | 33 | 44.5 | 67.5 |
| 61 | 45 | 2 | 0.15 | 0.80 | 1.3 | 7.5 | 1011.1 | 9884 | 504 | 33.5 | 46.5 | 40 | 53 | 90 |
| 62 | 60 | 2 | 0.23 | 0.50 | 0.8 | 2.0 | 1011.1 | 4029 | 108 | 17.5 | 16.5 | 23 | 22 | 36 |
| 63 | 60 | 6 | 0.15 | 0.28 | 0.5 | 2.6 | 1034.8 | 3329 | 112 | 20 | 19 | 26 | 21 | 37 |
| 64 | 60 | 6 | 0.23 | 0.82 | 1.4 | 3.3 | 1034.8 | 6358 | 113 | 36 | 36 | 45 | 41 | 70 |
| 65 | 60 | 2 | 0.33 | 1.35 | 2.3 | 2.6 | 1011.1 | 7582 | 118 | 32.5 | 34 | 43 | 42.5 | 29.5 |
| 66 | 60 | 4 | 0.23 | 0.87 | 1.5 | 3.5 | 1023.7 | 6890 | 141 | 36 | 39 | 45 | 47.5 | 86.5 |
| 67 | 60 | 2 | 0.23 | 0.70 | 1.2 | 2.8 | 1011.1 | 5641 | 151 | 37.5 | 34.5 | 43.5 | 45 | 87 |
| 68 | 60 | 6 | 0.15 | 0.49 | 0.8 | 4.6 | 1034.8 | 5825 | 196 | 29.5 | 27 | 37 | 32 | 53 |
| 69 | 60 | 2 | 0.15 | 0.38 | 0.6 | 3.6 | 1011.1 | 4695 | 239 | 15 | 11.5 | 18.5 | 15.5 | 31 |
| 70 | 60 | 4 | 0.15 | 0.60 | 1.0 | 5.7 | 1023.7 | 7286 | 282 | 15 | 15.5 | 20 | 17 | 33 |
| 71 | 60 | 4 | 0.15 | 0.85 | 1.4 | 8.0 | 1023.7 | 10322 | 400 | 30 | 28.5 | 38.5 | 34.5 | 50 |
| 72 | 60 | 2 | 0.15 | 0.90 | 1.5 | 8.5 | 1011.1 | 11120 | 567 | 37.5 | 34 | 45.5 | 41.5 | 72 |
4. Results and Discussion
4.1. General Jet Development
4.2. Jet Trajectory Analysis
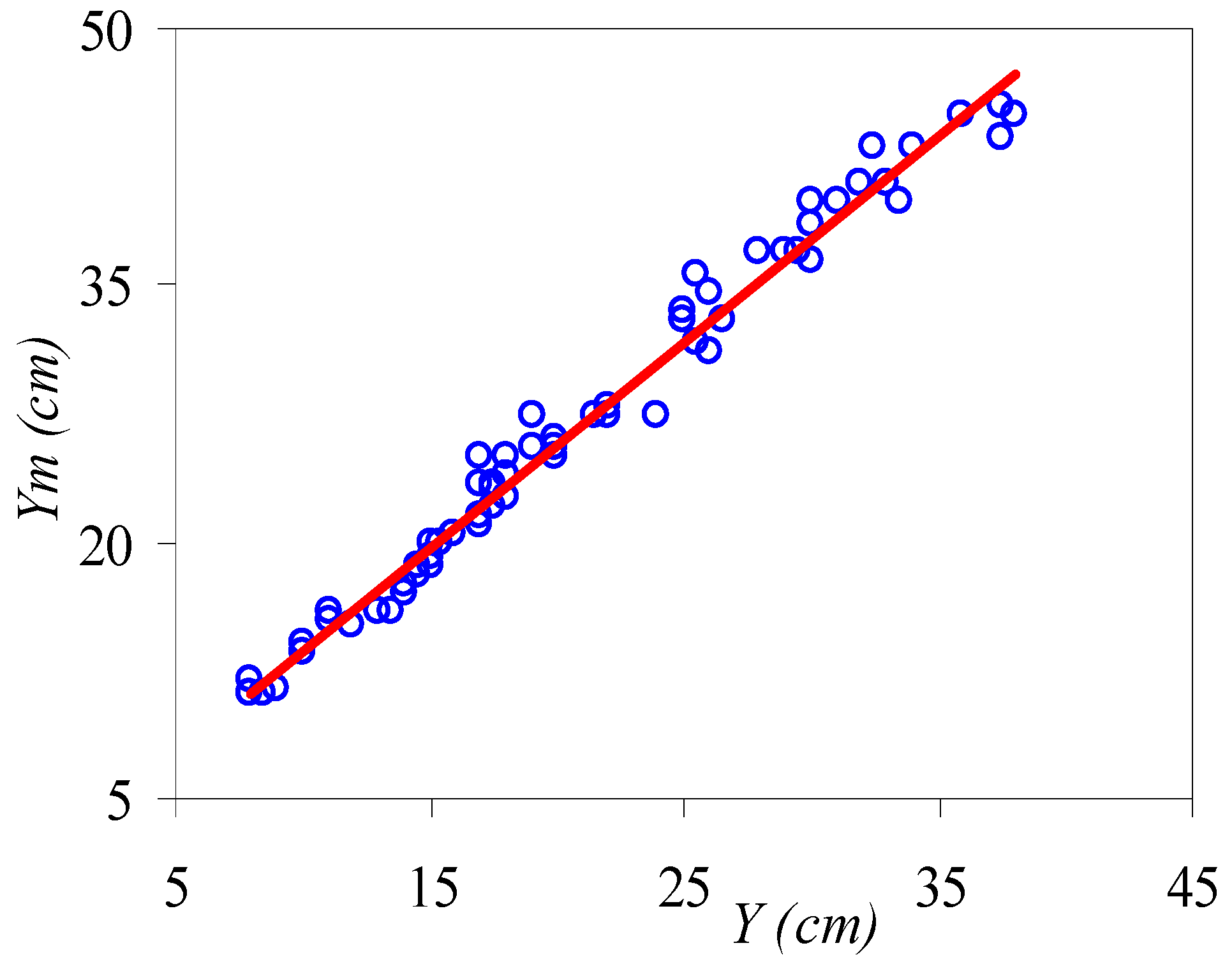

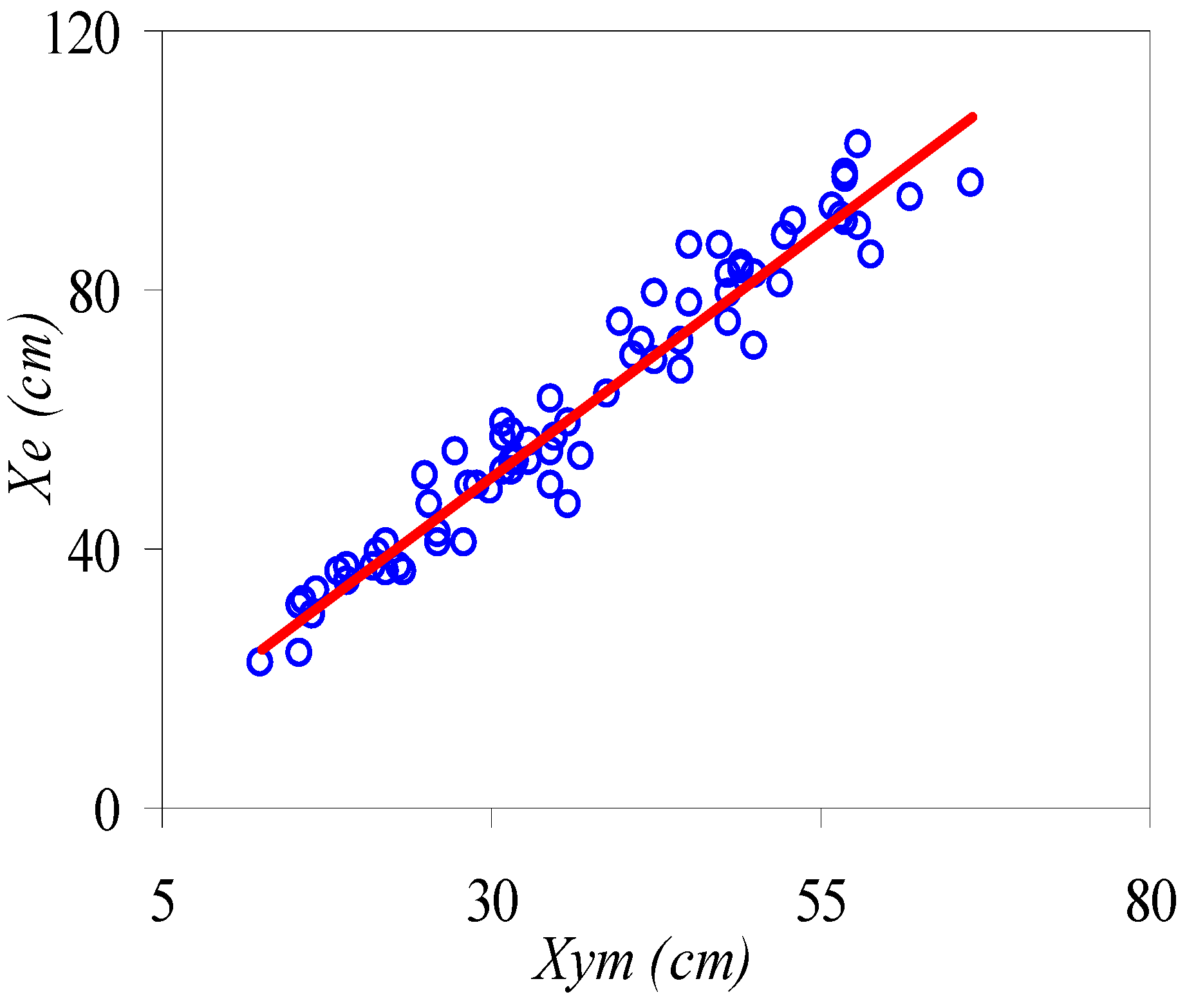
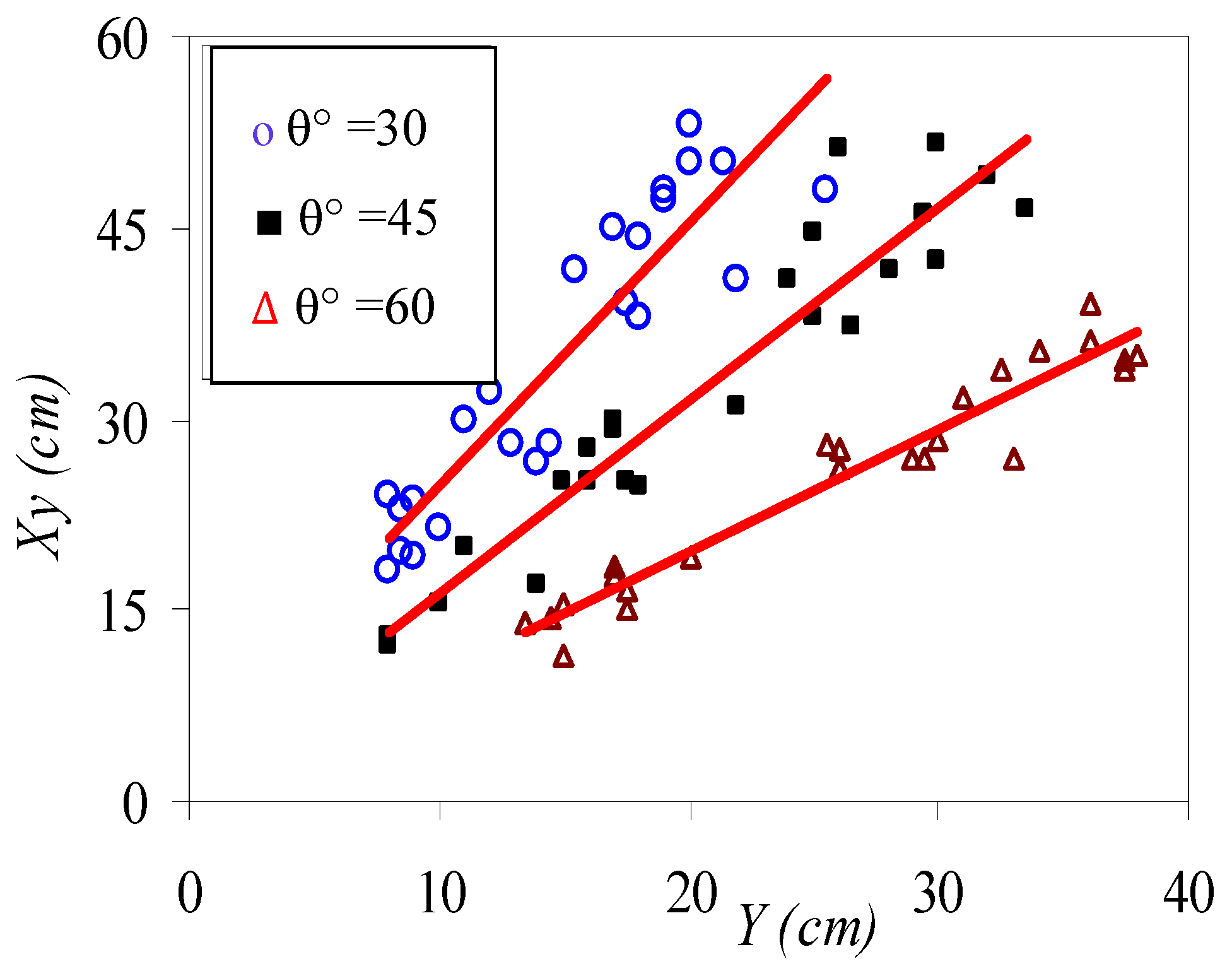
4.3. Densimetric Froude Number Dependence

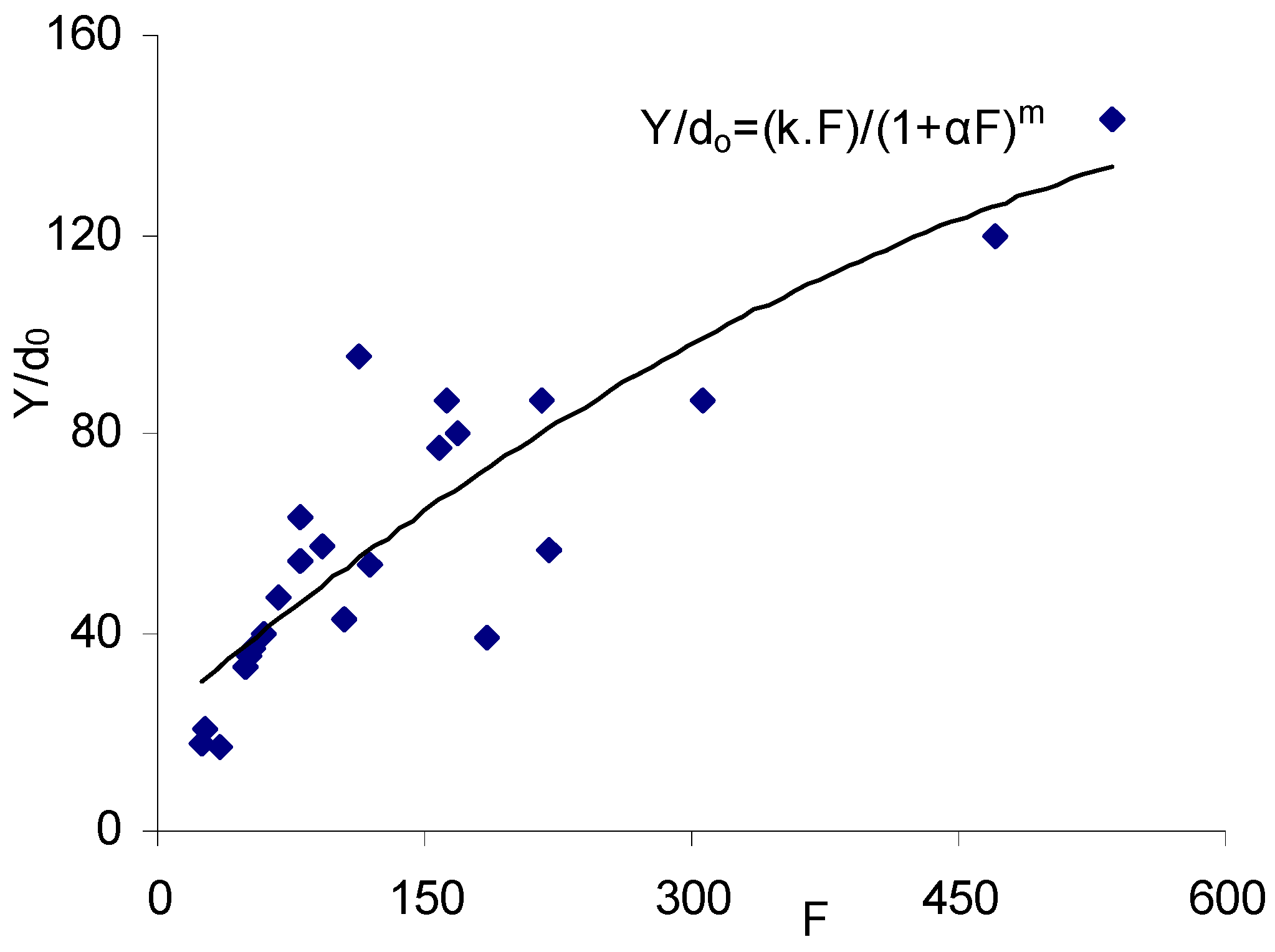
 , with n < 1. Based on the theoretical constraints and the empirical observations, the following equation was proposed to describe Y/do as a function of F over the entire range of experimental data,
, with n < 1. Based on the theoretical constraints and the empirical observations, the following equation was proposed to describe Y/do as a function of F over the entire range of experimental data,
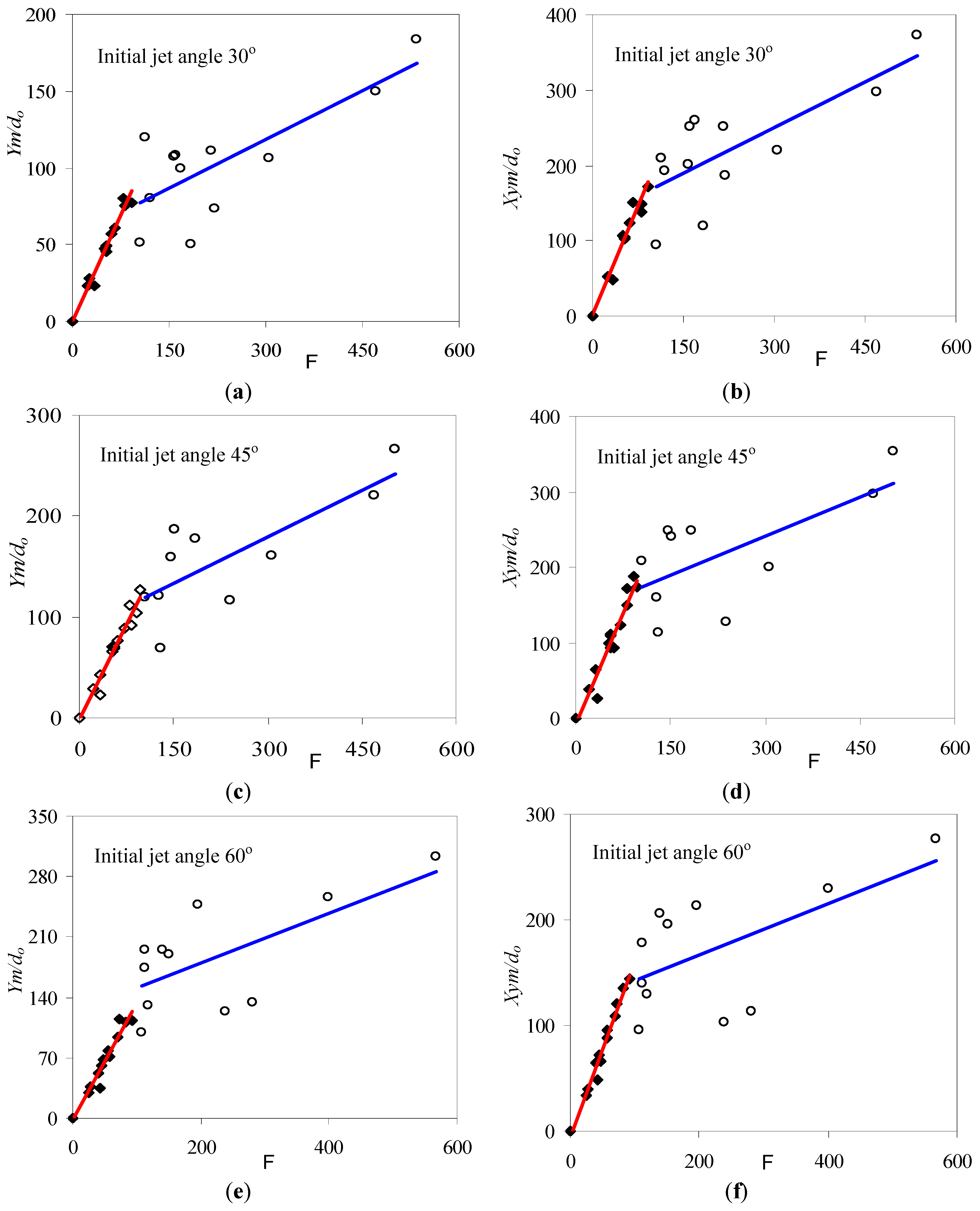
4.4. Relationships for Jet Quantities
| Study angle ( θ0) | ky | kym | kxe | ||||||
|---|---|---|---|---|---|---|---|---|---|
| 30° | 45° | 60° | 30° | 45° | 60° | 30° | 45° | 60° | |
| Zeitoun et al. [28] | - | - | - | 1.04 | 1.56 | 2.13 | - | - | - |
| Lindberg [14] | - | - | - | 1.29 | 1.58 | 2.14 | - | - | - |
| Bloomfield & Kerr [29] | - | - | - | 0.89 | 1.24 | 1.63 | - | - | - |
| Cipollina et al. [15] | 0.79 | 1.17 | 1.77 | 1.08 | 1.61 | 2.32 | 3.03 | 2.82 | 2.25 |
| Kikkert LA (1) [30] | 0.61 | 1.1 | 1.6 | 1.07 | 1.71 | 2.28 | 3.18 | 3.32 | 2.79 |
| Kikkert LIF (2) [30] | - | 1.21 | 1.76 | 1.21 | 1.78 | 2.45 | - | - | - |
| Kikkert et al. (3) [16] | 0.63 | 1.14 | 1.70 | 1.07 | 1.66 | 2.27 | 2.96 | 3.05 | 2.72 |
| Papakonstantis et al. [18] | - | - | - | - | 1.45 | 1.99 | - | - | - |
| Papakonstantis [31] | - | 1.17 | 1.68 | - | 1.58 | 2.14 | - | 3.16 | 2.75 |
| Christodoulou & Papak. [20] | 0.62 | 1.27 | 1.89 | 1.12 | 1.59 | 2.14 | 3.18 | 3.16 | 2.75 |
| Papakonstantis et al. [31] | - | - | - | - | 1.58 | 2.14 | - | 3.78 | 3.57 |
| This study: F < 100 | 0.69 | 1.0 | 1.4 | 0.92 | 1.3 | 1.7 | 3.12 | 2.90 | 2.66 |
| F > 100 | 0.17 | 0.26 | 0.24 | 0.21 | 0.31 | 0.29 | 0.65 | 0.53 | 0.36 |
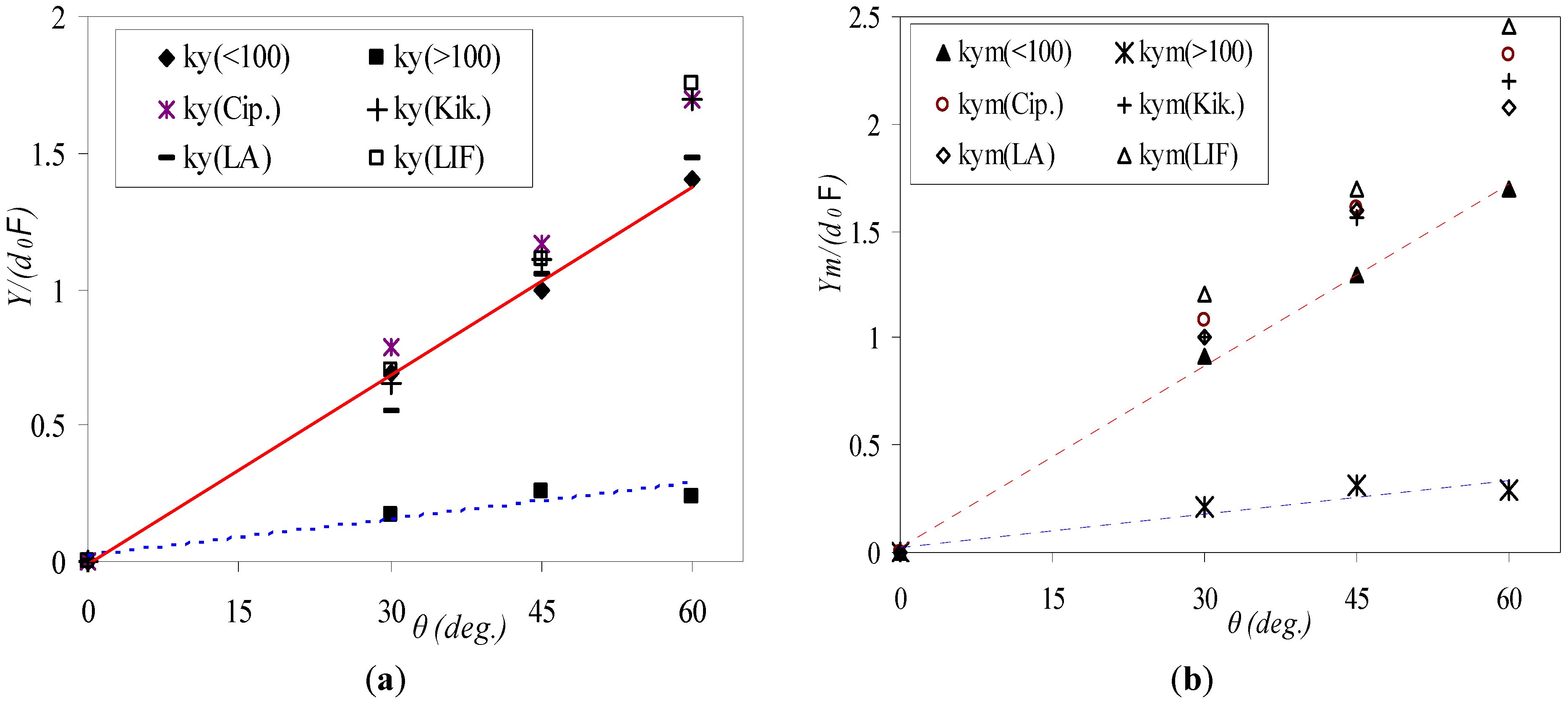
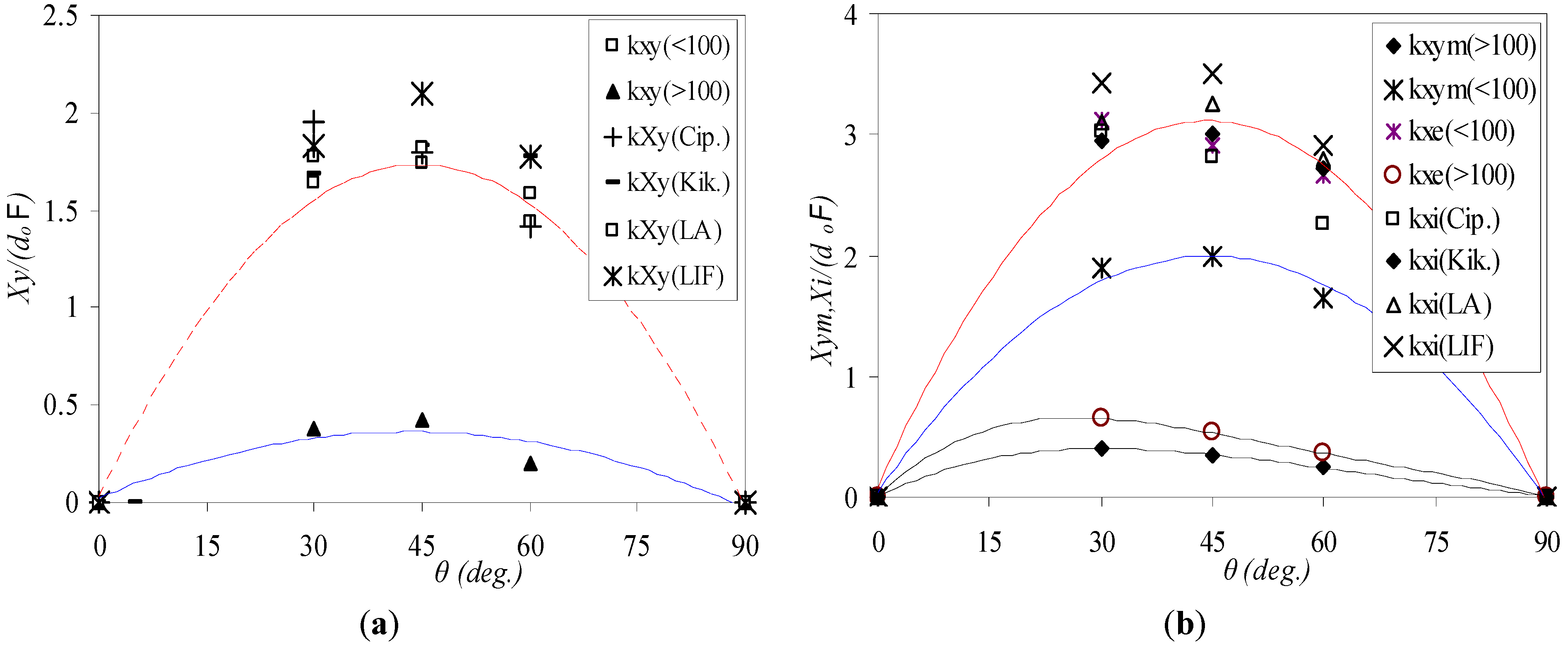
5. Conclusions
Acknowledgments
References
- Turner, J.S. Jets and plumes with negative or reversing buoyancy. J. Fluid Mech. 1966, 26, 779–792. [Google Scholar] [CrossRef]
- Abraham, G. Jets with negative buoyancy in homogeneous fluids. J. Hydraul. Res. 1967, 5, 236–248. [Google Scholar]
- Tong, S.S.; Stolzenbach, K.D. Submerged Discharges of Dense Effluent; Report No. 243; Massachusetts Institute of Technology: Cambridge, MA, USA, 1979. [Google Scholar]
- James, W.P.; Vergara, I.; Kim, K. Dilution of a dense vertical jet. J. Environ. Eng. 1983, 109, 1273–1283. [Google Scholar] [CrossRef]
- McLellan, T.N.; Randall, R. Measurement of brine jet height and dilution. J. Waterw. Port Coast Ocean Eng. 1986, 112, 200–216. [Google Scholar] [CrossRef]
- Baines, W.D.; Turner, J.S.; Campbell, I.H. Turbulent fountains in an open chamber. J. Fluid Mech. 1990, 212, 557–592. [Google Scholar] [CrossRef]
- Roberts, P.J.W.; Toms, G. Inclined dense jets inflowing current. J. Hydraul. Eng. 1987, 113, 323–341. [Google Scholar] [CrossRef]
- Roberts, P.J.W.; Toms, G. Ocean outfall system for dense and buoyant effluents. J. Environ. Eng. 1988, 114, 1175–1191. [Google Scholar] [CrossRef]
- Roberts, P.J.W.; Ferrier, A.; Daviero, G. Mixing in inclined dense jets. J. Hydraul. Eng. 1997, 123, 693–699. [Google Scholar] [CrossRef]
- Zhang, H.; Baddour, R.E. Maximum penetration of vertical round dense jets at small and large Froude numbers. J. Hydraul. Eng. 1998, 124, 550–553. [Google Scholar] [CrossRef]
- Zeitoun, M.A.; Reid, R.O.; McHilhenny, W.F.; Mitchell, T.M. Model Studies of Outfall Systems for Desalination Plants; Research and Development Progress Report No. 804; Office of Saline Water,U.S. Department of the Interior: Washington, DC, USA, 1972. [Google Scholar]
- Pincince, A.B.; List, E.J. Disposal of brine into an estuary. J. Water Polllut. Control Fed. 1973, 45, 2335–2344. [Google Scholar]
- Demetriou, J.D. Turbulent Diffusion of Vertical Water Jets with Negative Buoyancy (In Greek). Ph.D. Dissertation, National Technical University of Athens, Athens, Greece, 1978. [Google Scholar]
- Lindberg, W.R. Experiments on negatively buoyant jets, with and without cross-flow. In Recent Research Advances in the Fluid Mechanics of Turbulent Jets and Plumes; Davies, P.A., Valente Neves, M.J., Eds.; Springer: Berlin, Germany, 1994; Volume 255, pp. 131–145. [Google Scholar]
- Cipollina, A.; Brucato, A.; Grisafi, F.; Nicosia, S. Bench scale investigation of inclined dense jets. J. Hydraul. Eng. 2005, 131, 1017–1022. [Google Scholar] [CrossRef]
- Kikkert, G.A.; Davidson, M.J.; Nokes, R.I. Inclined negatively buoyant discharges. J. Hydraul. Eng. 2007, 133, 545–554. [Google Scholar] [CrossRef]
- Jirka, G.H. Integral model for turbulent buoyant jets in unbounded stratified flows. Part 2: Plane jet dynamics resulting from multiport diffuser jets. Environ. Fluid Mech. 2006, 6, 43–100. [Google Scholar] [CrossRef]
- Papakonstantis, I.; Kampourelli, M.; Christodoulou, G. Height of rise of inclined and vertical negatively buoyant jets. In Proceedings of 32nd IAHR Congress, Venice, Italy, 1–6 July 2007 [CD-ROM]; IAHR (The International Association for Hydro-Environment Engineering and Research): Madrid, Spain, 2004. [Google Scholar]
- Jirka, G.H. Improved discharge configuration for brine effluents from desalination plants. J. Hydraulic Eng. 2008, 134, 116–120. [Google Scholar] [CrossRef]
- Christodoulou, G.C.; Papakonstantis, I.G. Simplified estimates of trajectory of inclined negatively buoyant jets. In Environmental Hydraulics; Taylor & Francis: London, UK, 2010; pp. 165–170. [Google Scholar]
- Ferrari, S.; Querzoli, G. Mixing and re-entrainment in a negatively buoyant jet. J. Hydraul. Res. 2010, 48, 632–640. [Google Scholar] [CrossRef]
- Papakonstantis, I.G.; Christodoulou, G.C.; Papanicolaou, P.N. Inclined negatively buoyant jets 1: Geometrical characteristics. J. Hydraul. Res. 2011, 49, 3–12. [Google Scholar] [CrossRef]
- Sanchez, M.D. Near-Field Evolution and Mixing of a Negatively Buoyant Jet Consisting of Brine from a Desalination Plant. Master’s Thesis, Water Resources Engineering, Department of Building and Environmental Technology, Lund University, Lund, Sweden, 2009. [Google Scholar]
- Fischer, H.B.; List, E.J.; Koh, R.C.Y.; Imberger, J.; Brooks, N.H. Mixing in Inland and Coastal Waters; Academic Press: New York, NY, USA, 1979. [Google Scholar]
- Wright, S.J. Buoyant jets in density-stratified crossflow. J. Hydraul. Eng. 1984, 110, 643–656. [Google Scholar] [CrossRef]
- Doneker, R.L.; Jirka, G.H. Cormix User Manual 6.0E: A Hydrodynamic Mixing Zone Model and Decision Support System for Pollutant Discharges into Surface Waters; U.S. Environmental Protection Agency: Washington, DC, USA, 2007. [Google Scholar]
- Fan, L.-N.; Brooks, N.H. Dilution of Waste Gas Discharge from Campus Buildings; Technical Memo 68-1; California Institute of Technology: Pasadena, CA, USA, 1968. [Google Scholar]
- Zeitoun, M.A.; McHilhenny, W.F.; Reid, R.O. Conceptual Designs of Outfall Systems for Desalination Plants; Research and Development Progress Report No. 550; Office of Saline Water, U.S. Department of the Interior: Washington, DC, USA, 1970. [Google Scholar]
- Bloomfield, L.J.; Kerr, R.C. A theoretical model of a turbulent fountain. J. Fluid Mech. 2000, 424, 197–216. [Google Scholar] [CrossRef]
- Kikkert, G.A. Buoyant Jets with Two and Three Dimensional Trajectories. Ph.D. Dissertation, University of Canterbury, Christchurch, New Zealand, 2006. [Google Scholar]
- Papakonstantis, I.G. Turbulent Round Negatively Buoyant Jets at an Angle in a Calm Homogeneous Ambient (in Greek). Ph.D. Dissertation, School of Civil Engineering, National Technical University of Athens, Athens, Greece, 2009. [Google Scholar]
© 2012 by the authors; licensee MDPI, Basel, Switzerland. This article is an open-access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Bashitialshaaer, R.; Larson, M.; Persson, K.M. An Experimental Investigation on Inclined Negatively Buoyant Jets. Water 2012, 4, 720-738. https://doi.org/10.3390/w4030720
Bashitialshaaer R, Larson M, Persson KM. An Experimental Investigation on Inclined Negatively Buoyant Jets. Water. 2012; 4(3):720-738. https://doi.org/10.3390/w4030720
Chicago/Turabian StyleBashitialshaaer, Raed, Magnus Larson, and Kenneth M. Persson. 2012. "An Experimental Investigation on Inclined Negatively Buoyant Jets" Water 4, no. 3: 720-738. https://doi.org/10.3390/w4030720
APA StyleBashitialshaaer, R., Larson, M., & Persson, K. M. (2012). An Experimental Investigation on Inclined Negatively Buoyant Jets. Water, 4(3), 720-738. https://doi.org/10.3390/w4030720







