Coastal Flooding in the Solent: An Integrated Analysis of Defences and Inundation
Abstract
:1. Introduction
- Describe a coastal flood modelling methodology, where flood simulations account for real flood defence characteristics and a full range of defence failure mechanisms (ranging from simple overflow or outflanking, through to full breaching).
- Simulate synthetic coastal floods within the Solent, to indicate flood event impacts under present and 21st century sea levels across this range of failure mechanisms.
- Compare the flood consequences for different failure mechanisms in these floods.
2. The Solent Case-Study Region and Initial Assessment of Coastal Flood Risk
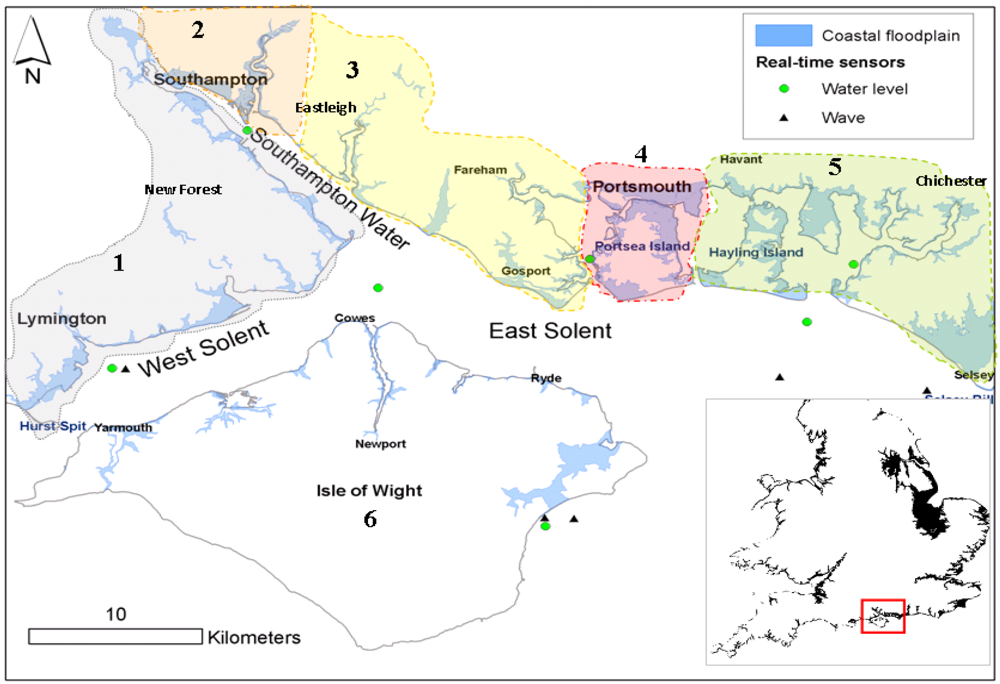
3. Methods
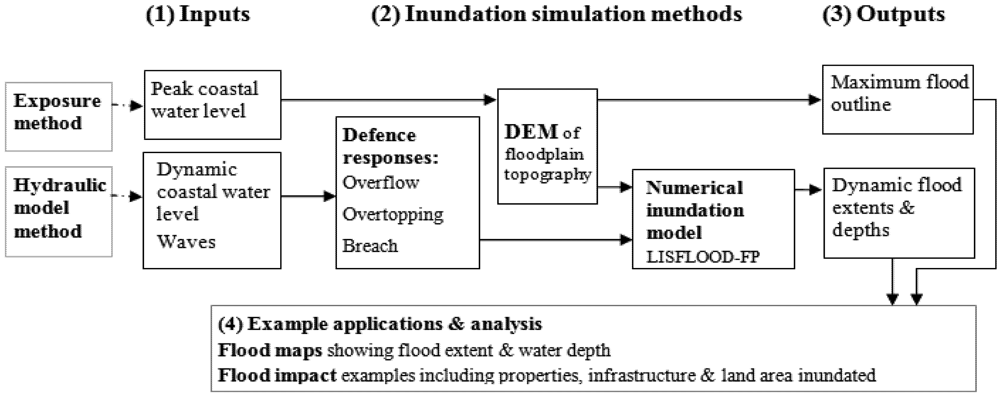
3.1. Regional Topography, Water Level and Defence Datasets
| Return period (years) | 1 | 10 | 20 | 50 | 100 | 200 | 1000 | MHWS | 10th Match 2008 |
|---|---|---|---|---|---|---|---|---|---|
| Elevation (mAOD) | 2.56 | 2.81 | 2.88 | 2.98 | 3.05 | 3.12 | 3.28 | 1.97 | 2.77 |
3.2. The Planar Water Level Method
3.3. Hydraulic Modelling
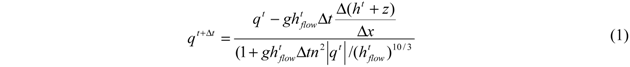
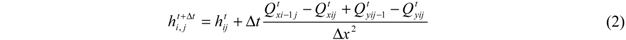
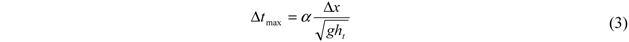
| Model | Domain size | DEM resolution (m) and file size (MB) | Number of inflow points | Time-step (seconds) | Run-time to simulate 14 hours of real-time flooding (minutes) | ||
|---|---|---|---|---|---|---|---|
| Km2 | columns, rows | ||||||
| Solent | 105.7 | 1227,846 | 50 | 3.87 | 4909 | 4.2–6.3 | 15 |
| City of Portsmouth | 15.2 | 150, 168 | 50 | 0.01 | 595 | 5.4–8 | <1 |
3.3.1. Overflow
3.3.2. Overtopping
3.3.3. Breach Analysis
3.4. Model Validation
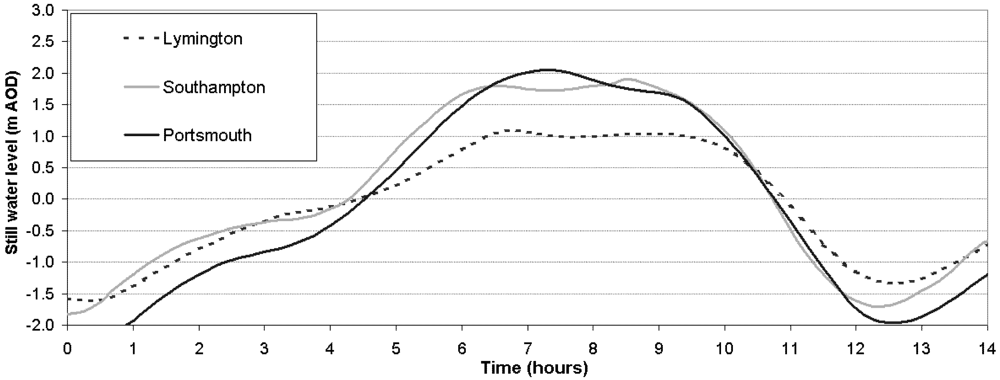
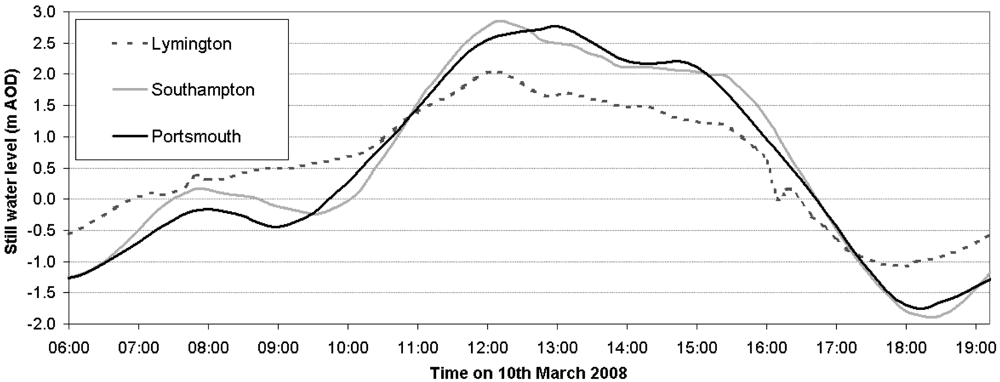
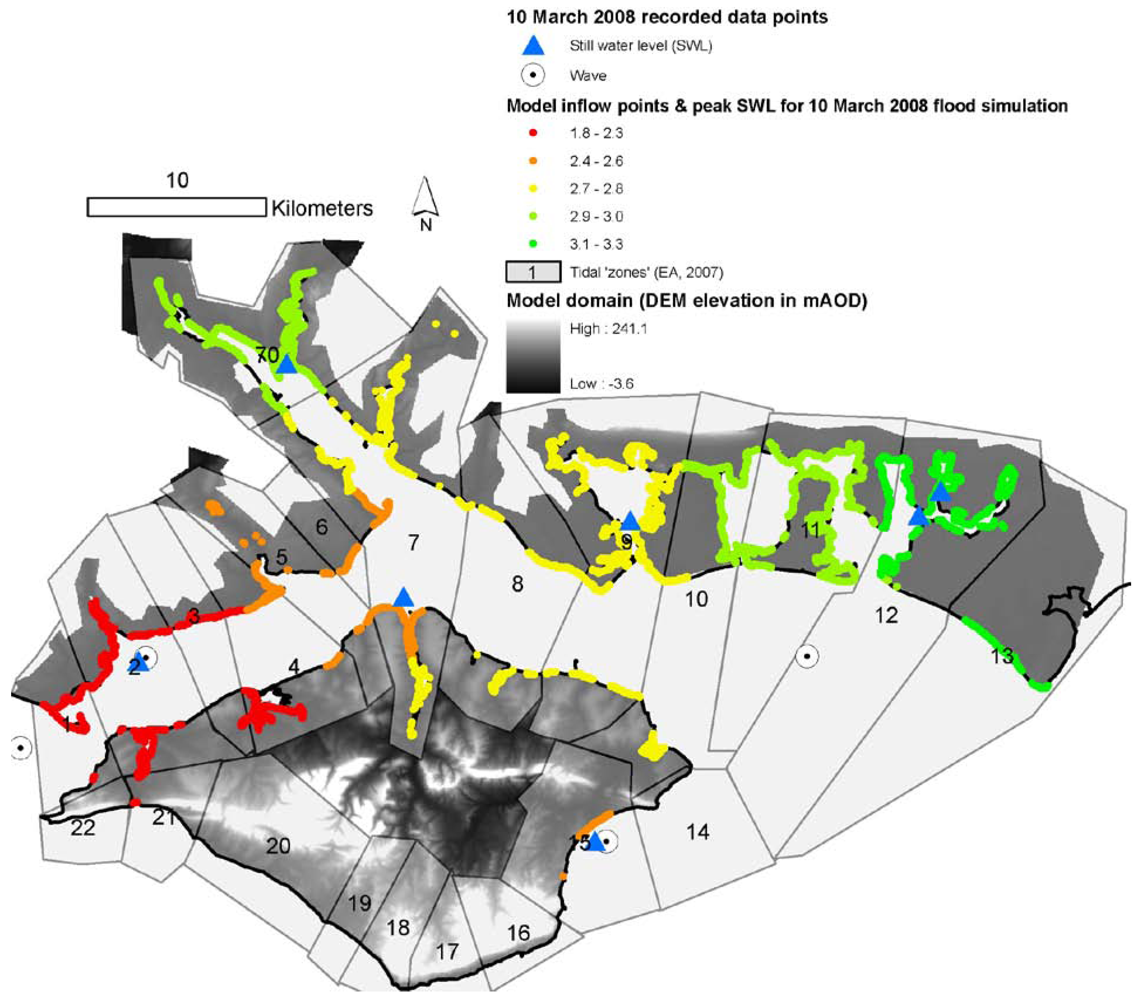
3.4.1. 10th March 2008 Regional Flood Event
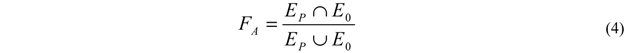

| Test type | Criteria | Result |
|---|---|---|
| Detection of observed floods | The model indicated flooding at 29 out of 30 locations where floods were reported (the location missed was on a tidal river where additional sources of floodwater may have occurred). At 27 locations, some description of flooding was available to allow comparison with the model; which is summarised below. | |
| Flood extent fit scores | Mean FA value (for the 27 compared locations) over-predictionsunder-predictionsneither definitive over or under-prediction | 0.656813 |
| Number of locations with a good fit (FA > 0.75) 2 were slight over-predictions, 1 was a slight under-prediction Number of locations with a moderate fit (FA = 0.50 to 0.75) 2 over-predictions, 3 under-predictions Number of locations with a poor fit (FA < 0. 50) 2 were over-predictions, 4 were under-predictions | 12 7 8 | |
| Flood depth | All modelled depths were within 30 cm of observed flood depths. | |

3.4.2. 17th December 1989 Flood Event (Breach Failure)
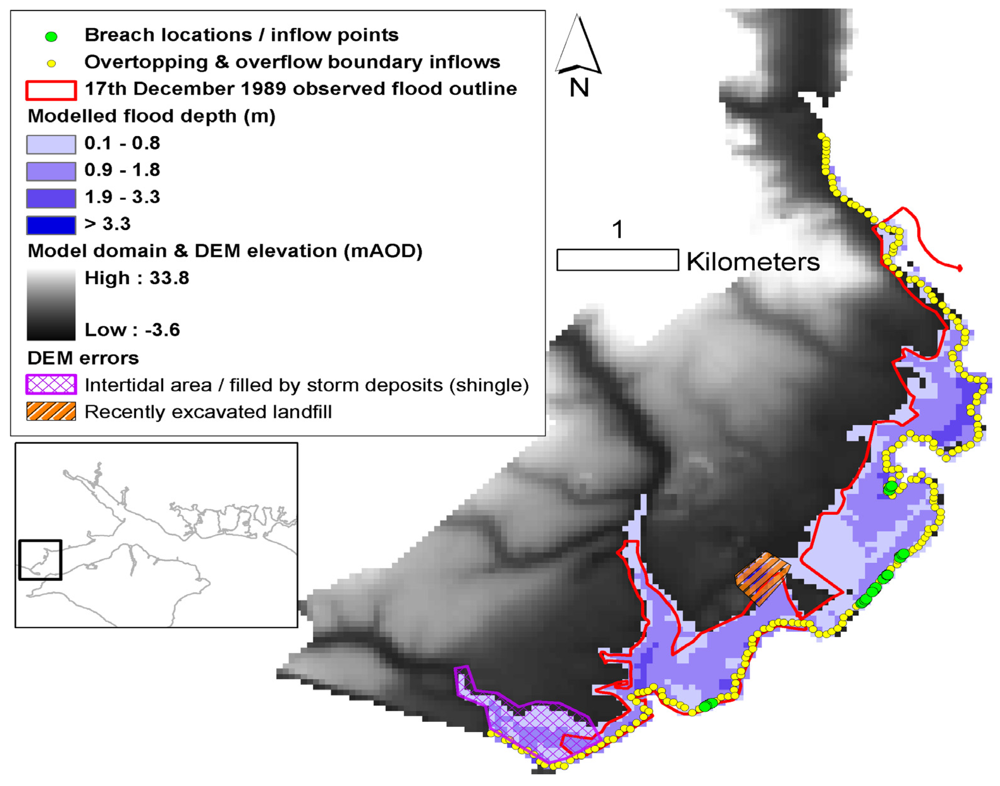
4. Results
4.1. Simulation of an Extreme Present-Day Flood Event
| REGION (see Figure 1) | Location | Exposure (numbers in brackets is land area inundated to >1m depth) | Properties flooded by hydraulic simulations
Total (number in brackets are properties inundated to >1 m depth) | ||||
|---|---|---|---|---|---|---|---|
| Floodplain area (km2) | Properties | Breach of all defences and max. waves | Max. waves | 1 in 1 yr waves | No waves | ||
| 1 | New Forest | 14.1
(6.6) | 736
(134) | 639
(147) | 591
(112) | 430
(55) | 329
(34) |
| 2 | City of Southampton | 2.0
(0.1) | 2,148
(249) | 978
(21) | 973
(21) | 919
(21) | 715
(10) |
| 3 | Eastleigh, Fareham & Gosport | 7.2
(2.4) | 1,789
(106) | 1,395
(109) | 1,321
(93) | 1,163
(91) | 564
(52) |
| 4 | City of Portsmouth | 13.8
(4.9) | 14,055
(3,483) | 10,922
(2,734) | 6,437
(459) | 4,660
(110) | 1,923
(8) |
| 5 | Havant & Chichester | 29.8
(13.2) | 3,781
(1,657) | 3,168
(613) | 2,995
(448) | 2,602
(329) | 1,033
(90) |
| 6 | Isle of Wight (all) | 11.4 (5.6) | 617
(155) | 505
(114) | 377
(67) | 354
(67) | 255
(25) |
| Total | Solent | 78.3 (32.8) | 23,126 (5,784) | 17,607 (3,728) | 12,694 (1,200) | 10,128 (673) | 4,816 (219) |
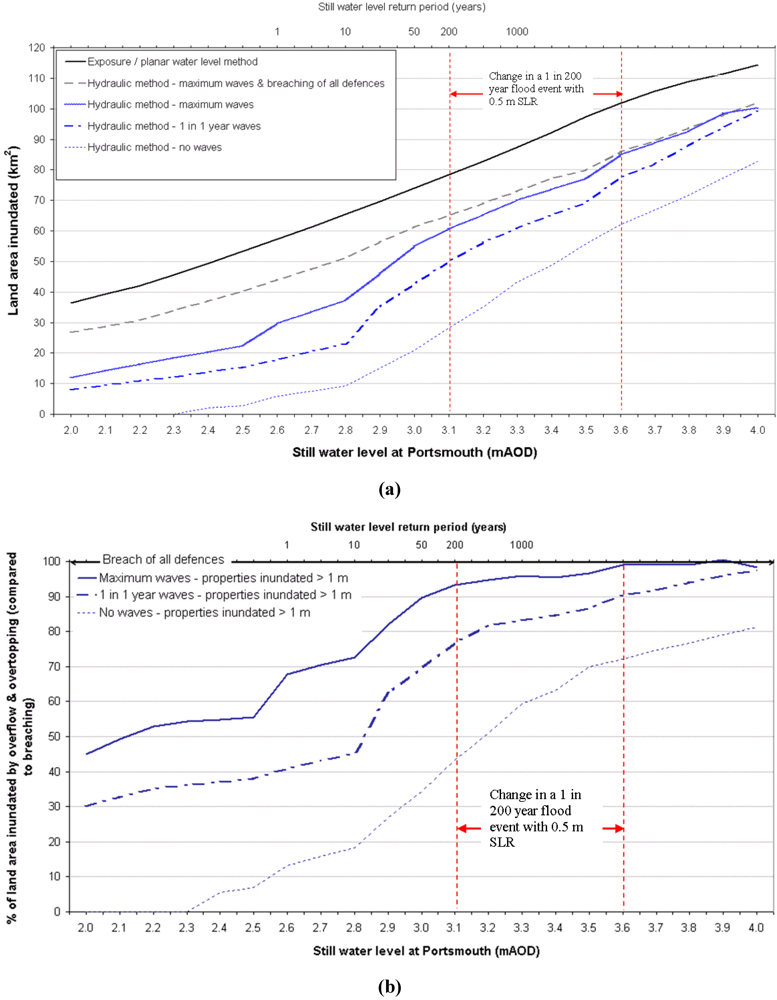
4.2. Coastal Flood Simulations Across a Range of Loadings
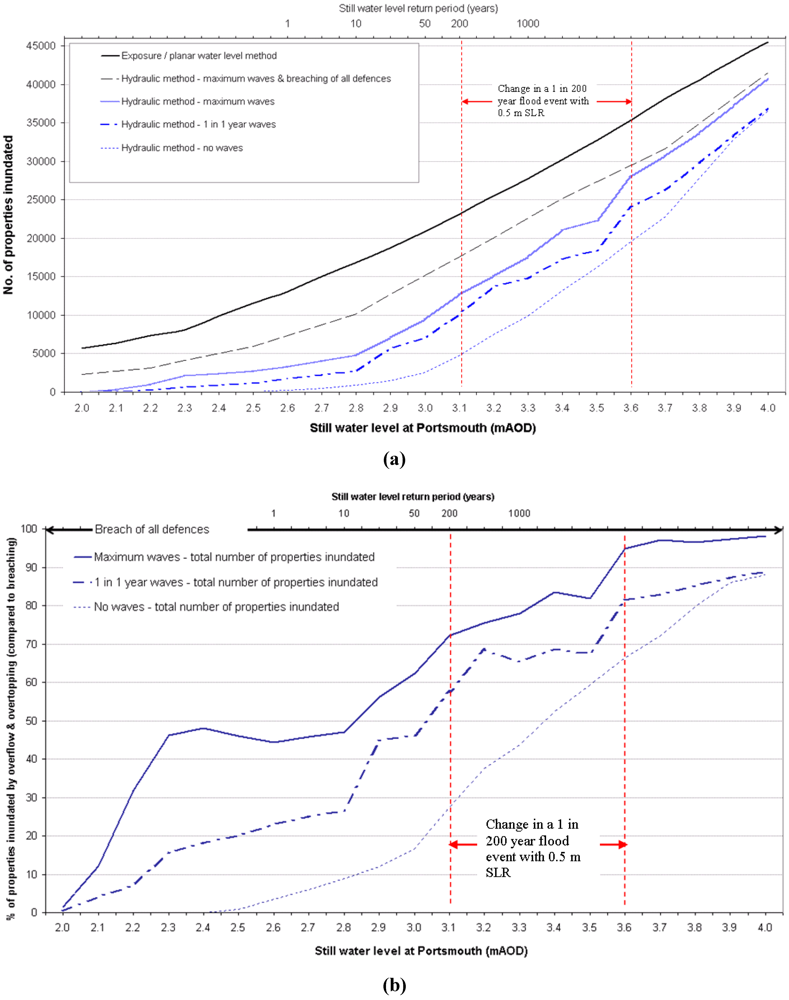
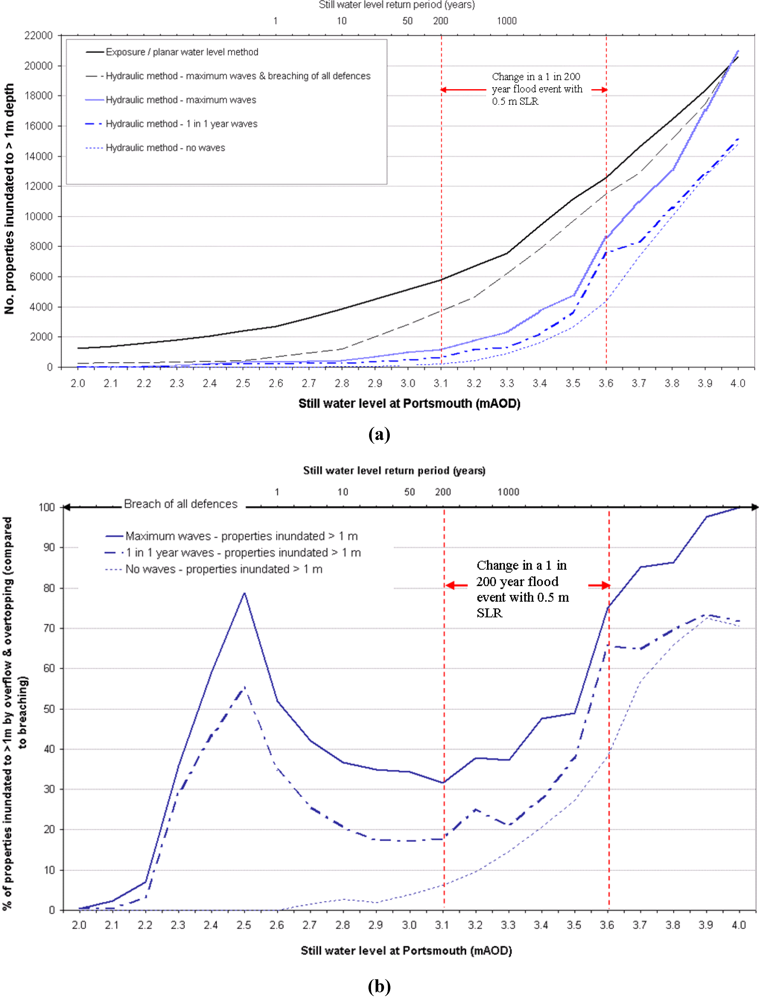
5. Discussion
5.1. Application of the Methodology
5.2. Flood Impacts in the Solent
5.3. Directions for Further Research
6. Conclusions
Acknowledgments
References
- Van Koningsveld, M.; Mulder, J.P.M.; Stive, M.J.F.; van der Valk, L.; van der Weck, A.W. Living with sea-level rise and climate change: A case study of the netherlands. J. Coast. Res. 2008, 24, 367–379. [Google Scholar]
- Dawson, R.J.; Ball, T.; Werritty, J.; Werritty, A.; Hall, J.W.; Roche, N. Assessing the effectiveness of non-structural flood management measures in the thames estuary under conditions of socio-economic and environmental change. Glob. Environ. Change 2011, 21, 628–646. [Google Scholar]
- Gerritsen, H. What happened in 1953? The big flood in the netherlands in retrospect. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2005, 363, 1271–1291. [Google Scholar]
- Baxter, P.J. The east coast big flood, 31 January–1 February 1953: A summary of the human disaster. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2005, 363, 1293–1312. [Google Scholar]
- McRobie, A.; Spencer, T.; Gerritsen, H. The big flood: North sea storm surge. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2005, 363, 1263–1270. [Google Scholar]
- Bütow, H. Die große flut in Hamburg: Eine Chronik der Katastrophe vom Februar 1962; Verlag: Hansestadt, German, 1963. [Google Scholar]
- Von Storch, H.; Woth, K.; Gönnert, G. Storm surges—The case of Hamburg, Germany. ESSP OSC Panel Session on “GEC, Natural Disasters, and Their Implications for Human Security in Coastal Urban Areas”. Available online: http://www.safecoast.nl/editor/databank/File/hamburg-storms.pdf (accessed on 26 January 2012).
- Battjes, J.; Gerritsen, H. Coastal modelling for flood defence. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Eng. Sci. 2002, 360, 1461–1475. [Google Scholar]
- Bates, P.D.; Dawson, R.J.; Hall, J.W.; Horritt, M.S.; Nicholls, R.J.; Wicks, J.; Mohamed Ahmed Ali Mohamed, H. Simplified two-dimensional numerical modelling of coastal flooding and example applications. Coast. Eng. 2005, 52, 793–810. [Google Scholar]
- Brown, J.D.; Spencer, T.; Moeller, I. Modeling storm surge flooding of an urban area with particular reference to modeling uncertainties: A case study of Canvey Island, United Kingdom. Water Resour. Res. 2007, 43, W06402. [Google Scholar] [CrossRef]
- Gallien, T.W.; Schubert, J.E.; Sanders, B.F. Predicting tidal flooding of urbanized embayments: A modeling framework and data requirements. Coast. Eng. 2011, 58, 567–577. [Google Scholar]
- Smith, R.A.E.; Bates, P.D.; Hayes, C. Evaluation of a coastal flood inundation model using hard and soft data. Environ. Model. Softw. 2012, 30, 35–46. [Google Scholar]
- Narayan, S.; Hanson, S.; Nicholls, R.J.; Clarke, D.; Willems, P.; Ntegaka, V.; Monbaliu, J. A holistic assessment of coastal flood systems using system diagrams and the source-pathway-receptor (spr) concept. J. Nat. Hazards Earth Syst. Sci. 2012, in press. [Google Scholar]
- Dawson, R.; Peppe, R.; Wang, M. An agent-based model for risk-based flood incident management. Nat. Hazards 2011, 59, 167–189. [Google Scholar]
- RMS. Hurricane Katrina: Profile of a Super Cat, Lessons and Implications for Catastrophe, Risk Management Solutions; Risk Management Solutions; Risk Management Solutions, Inc.: Newark, CA, USA, 2005. Available online: http://www.Rms.Com (accessed on 14 June 2011).
- Hanson, S.; Nicholls, R.; Ranger, N.; Hallegatte, S.; Corfee-Morlot, J.; Herweijer, C.; Chateau, J. A global ranking of port cities with high exposure to climate extremes. Clim. Change 2011, 104, 89–111. [Google Scholar]
- Lumbroso, D.M.; Vinet, F. A comparison of the causes, effects and aftermaths of the coastal flooding of england in 1953 and france in 2010. Nat. Hazards Earth Syst. Sci. 2011, 11, 2321–2333. [Google Scholar]
- Kolen, B.; Slomp, R.; van Balen, W.; Terpstra, T.; Bottema, M.; Nieuwenhuis, S. Learning from French Experiences with Storm Xynthia–Damages after a Flood; ©HKV LIJN IN WATER: Waterdienst, The Netherlands, 2010. [Google Scholar]
- Muir Wood, R.; Bateman, W. Uncertainties and constraints on breaching and their implications for flood loss estimation. Philos. Trans. R. Soc. A. Math. Phys. Eng. Sci. 2005, 363, 1423–1430. [Google Scholar]
- Haigh, I.; Nicholls, R.; Wells, N. Rising sea levels in the English Channel 1900 to 2100. Proc. ICE Maritime Engineer. 2011, 164, 81–92. [Google Scholar]
- Menéndez, M.; Woodworth, P.L. Changes in extreme high water levels based on a quasi-global tide-gauge data set. J. Geophys. Res. 2010, 115, C10011. [Google Scholar] [CrossRef]
- Wahl, T.; Jensen, J.; Frank, T.; Haigh, I. Improved estimates of mean sea level changes in the german bight over the last 166 years. Ocean Dyn. 2011, 61, 701–715. [Google Scholar]
- De Moel, H.; van Alphen, J.; Aerts, J.C.J.H. Flood maps in Europe—Methods, availability and use. Nat. Hazards Earth Syst. Sci. 2009, 9, 289–301. [Google Scholar]
- Reeve, D.E.; Burgess, K.A. A method for the assesment of coastal flood risk. IMA J. Manag. Math. 1993, 5, 197–209. [Google Scholar]
- Hall, J.; Dawson, R.J.; Sayers, P.B.; Rosu, C.; Chatterton, J.B.; Deakin, R.A. A methodology for national-scale flood risk assessment. Water Marit. Eng. ICE 2003, 156, 235–247. [Google Scholar]
- Dawson, R.; Hall, J. Adaptive importance sampling for risk analysis of complex infrastructure systems. Proc. R. Soc. A Math. Phys. Eng. Sci. 2006, 462, 3343–3362. [Google Scholar]
- Gouldby, B.; Sayers, P.; Mulet-Marti, J.; Hassan, M.; Benwell, D. A methodology for regional-scale flood risk assessment. Water Manag. 2008, 161, 169–182. [Google Scholar]
- EA. Flooding in England: A National Assessment of Flood Risk; Environment Agency: Rotherham, UK, 2009. Available online: http://publications.Environment-agency.Gov.Uk/pdf/geho0609bqds-e-e.Pdf (accessed on 23 April 2012).
- Defra. Shoreline Management Plan Guidance Volume 1: Aims and Requirements; DEFRA: London, UK, 2006. Available online: http://www.Defra.Gov.Uk/publications/files/pb11726-smpg-vol1–060308.Pdf (accessed on 25 January 2012).
- Motyka, J.M.; Brampton, A.H. Coastal Management: Mapping of Littoral Cells Ref: SR328; HR Wallingford: Oxfordshire, UK, 1993. [Google Scholar]
- DEFRA; Environment Agency, Best Practice in Coastal Flood Forecasting: R&d Technical Report FD2206/TR1; HR Wallingford Report TR 132; DEFRA: London, UK, 2004.
- Bradbury, A.; Kidd, R. Hurst spit stabilisation scheme: Design and construction of beach recharge. In 33rd MAFF Conference of River and Coastal Engineers, Keele University, Staffordshire, UK, 1–3 July 1998.
- Haigh, I.; Butcher, P.; Harris, J.M.; Cooper, N.J.; Trip, I. Developing an improved understanding of storm surges in the solent. In Proceedings of the39th DEFRA Flood and Coastal Management Conference, York, UK, 29 June–1 July 2004.
- Law, C.R. Storm surges in the English Channel. Hydrogr. J. 1975, 2, 30–34. [Google Scholar]
- Haigh, I. Extreme Sea Levels in the English Channel. Ph.D Dissertation, University of Southampton, Hampshire, UK, 2009. [Google Scholar]
- Ruocco, A.; Nicholls, R.; Haigh, I.; Wadey, M. Reconstructing coastal flood occurrence combining sea level and media sources: A case study of the Solent, UK since 1935. Nat. Hazards 2011, 59, 1773–1796. [Google Scholar]
- Pugh, D.T. Tides, surges and mean sea-level. In A Handbook for Engineers and Scientists; Wiley: Chichester, UK; Volume 1987.
- Wells, N.C.; Baldwin, D.J.; Wang, J.Y.; Collins, M.B. Modelling of extreme storm surge events in the English Channel for the period 14–18 December 1989. Glob. Atmos. Ocean Syst. 2001, 7, 275–294. [Google Scholar]
- Mason, T.; Bradbury, A.; Poate, T.; Newman, R. Nearshore wave climate of the English Channel—Evidence for bi-modal seas. In 31st International Conference on Coastal Engineering, Hamburg, Germany, 31 Auguest–5 September 2008; Jane, M.S., Ed.; World Scientific: Singapore; Hamburg, Germany, 2009; pp. 605–616. [Google Scholar]
- Evans, E. Foresight: Scientific Summary. Volume ii, Managing Future Risks; Office of Science and Technology: London, UK, 2004. [Google Scholar]
- NFDC. Cabinet 06/07/09 Report A: North Solent Shoreline Management Plan; New Forest District Council: Hampshire, UK, 6 July 2009. Available online: http://www.Newforest.Gov.Uk/committeedocs/cab/cdr05054.Pdf (accessed on 15 December 2011).
- Portsmouth City Council (PCC). Portsmouth City Local Plan, First Review 2001–2011. Supplementary Planning Guidance: Flood Protection. Approved by the Executive Member for Planning, Regeneration, Economic Development and Property 6th February 2004; Portsmouth City Council: Wales, UK, 2004. Available online: http://www.portsmouth.gov.uk/media/Flood_Protection_SPG.pdf (accessed on 26 August 2009).
- RIBA; ICE. Facing up to Rising Sea-Levels: Retreat? Defend? Attack? The Future of Our Coastal and Estuarine Cities; Royal Institute for British Architects; Institute of Civil Engineers: London, UK, 2009. Available online: http://www.buildingfutures.org.uk/assets/downloads/Facing_Up_To_Rising_Sea_Levels.pdf (accessed on 31 January 2010).
- Atkins. Strategic Flood Risk Assessment for the Partnership for Urban South Hampshire; Document Number: 5049258/72/DG/048; Atkins limited: London, UK, 2007. Available online: http://www.environment-agency.gov.uk/static/documents/Research/PUSH_SFRA.pdf (accessed on 24 April 2012).
- OS. Ordnance Survey Land-Form Profile™ User Guide; V4.0–5/2001© Crown Copyright; Ordnance Survey: Southampton, UK, 2001. Available online: http://www.ordnancesurvey.co.uk (Accessed on 6 July 2011).
- CCO. Strategic Regional Coastal Monitoring Programmes; Channel Coastal Observatory: Southampton, UK, 2011. Available online: http://www.channelcoast.org (accessed on 23 April 2012).
- Xharde, R.; Long, B.; Forbes, D. Accuracy and limitations of AIrborne Lidar surveys in coastal environment. In Proceedings of theGeoscience and Remote Sensing Symposium, 2006; IGARSS 2006. IEEE International Conference, Denver, CO, USA, 31 July–4 August 2006; pp. 2412–2415, ISBN: 0-7803-9510-7.
- Webster, T.L.; Forbes, D.L.; MacKinnon, E.; Roberts, D. Flood-risk mapping for storm-surge events and sea-level rise using lidar for southeast new brunswick. Can. J. Remote Sens. 2006, 32, 194–211. [Google Scholar]
- Fewtrell, T.J.; Bates, P.D.; Horritt, M.; Hunter, N.M. Evaluating the effect of scale in flood inundation modelling in urban environments. Hydrol. Process. 2008, 22, 5107–5118. [Google Scholar]
- De Mers, M.N. Fundamentals of Geographic Information Systems, 4th ed; John Wiley Sons Inc.: New York, NY, USA, 2009. [Google Scholar]
- Purvis, M.J.; Bates, P.D.; Hayes, C.M. A probabilistic methodology to estimate future coastal flood risk due to sea level rise. Coast. Eng. 2008, 55, 1062–1073. [Google Scholar]
- Dixon, M.J.; Tawn, J.A. Extreme Sea-Levels at the UK a-Class Sites: Site-by-Site Analysis; Pol Internal Document Number 65; Proudman Oceanographic Laboratory: Liverpool, UK, 1994. Available online: http://www.pol.ac.uk/ntslf/pdf/id65.pdf (accessed on 23 April 2012).
- Dixon, M.J.; Tawn, J.A. Extreme Sea-Levels at the UK a-Class Sites: Optimal Site-Bysite Analysis and Spatial Analyses for the East Coast; Pol Internal Document Number 72; Proudman Oceanographic Laboratory: Liverpool, UK, 1995; p. 298. [Google Scholar]
- Dixon, M.J.; Tawn, J.A. Estimates of Extreme Sea Conditions—Final Report, Spatial Analysis for the UK Coast; Pol Internal Document Number 112; Proudman Oceanographic Laboratory: Liverpool, UK, 1997; p. 217. [Google Scholar]
- Haigh, I.D.; Nicholls, R.; Wells, N. A comparison of the main methods for estimating probabilities of extreme still water levels. Coast. Eng. 2010, 57, 838–849. [Google Scholar]
- Flood Risk Mapping and Data Management Team, Environment Agency Extreme Water Levels: Bournemouth to Littlehampton; EA: Rotherham, UK, 2007.
- EA, Coastal Flood Boundary Conditions for UK Mainland and Islands; Project: Sc060064/tr2: Design sea Levels; EA: Bristol, UK, 2011; p. 142.
- Haigh, I. Quantification of sea-level rise at the Southampton and Portsmouth tide gauges. Personal Communication.
- NFDC. North Solent Shoreline Management Plan, Appendix c Baseline Process Understanding, c.1 Assessment of Shoreline Dynamics; New Forest District Council: Hampshire, UK, 2010. Available online: http://www.newforest.gov.uk/media/adobe/o/s/C1_Coastal_Processes _Literature_Review_%28final_draft%29.pdf (accessed on 30 June 2011).
- CERC, Coastal Engineering Research Center 1984: Shore Protection Manual; Department of the Army, Waterways Experiment Station, Corps of Engineers, Coastal Engineering Research Center, Vicksburg, United States Government Printing Office: Washington, DC, USA, 1984; p. 652.
- Dawson, R.; Hall, J. Probabilistic condition characterisation of coastal structures using imprecise information. In Proceedings of the 28th International Conference Coastal Engineering Cardiff, UK, 8–12 July 2002; McKee-Smith, J., Ed.; World Scientific: Hackensack, NJ, USA; Cardiff, UK, 2003; pp. 2348–2359. [Google Scholar]
- Flikweert, J.; Simm, J. Improving performance targets for flood defence assets. J. Flood Risk Manag. 2008, 1, 201–212. [Google Scholar]
- Lowe, J.A.; Howard, T.; Pardaens, A.; Tinker, J.; Holt, J.; Wakelin, S.; Glenn, M.; Leake, J.; Wolf, J.; Horsburgh, K.; et al. UK Climate Projections Science Report: Marine and Coastal Projections; Met Office Hadley Centre: Exeter, UK, 2009; p. 99. [Google Scholar]
- Bates, P.D.; Horritt, M.S.; Fewtrell, T.J. A simple inertial formulation of the shallow water equations for efficient two-dimensional flood inundation modelling. J. Hydrol. 2010, 387, 33–45. [Google Scholar]
- Bates, P.D.; de Roo, A.P.J. A simple raster-based model for flood inundation simulation. J. Hydrol. 2000, 236, 54–77. [Google Scholar]
- Hunter, N.M.; Horritt, M.S.; Bates, P.D.; Wilson, M.D.; Werner, M.G.F. An adaptive time step solution for raster-based storage cell modelling of floodplain inundation. Adv. Water Resour. 2005, 28, 975–991. [Google Scholar]
- Dawson, R.J.; Hall, J.W.; Bates, P.D.; Nicholls, R.J. Quantified analysis of the probability of flooding in the thames estuary under imaginable worst-case sea level rise scenarios. Int. J. Water Resour. Dev. 2005, 21, 577–591. [Google Scholar]
- Hunter, N.M.; Bates, P.D.; Horritt, M.S.; Wilson, M.D. Simple spatially-distributed models for predicting flood inundation: A review. Geomorphology 2007, 90, 208–225. [Google Scholar]
- Fewtrell, T.J.; Duncan, A.; Sampson, C.C.; Neal, J.C.; Bates, P.D. Benchmarking urban flood models of varying complexity and scale using high resolution terrestrial lidar data. Phys. Chem. Earth Parts A/B/C 2011, 36, 281–291. [Google Scholar] [CrossRef]
- Hunter, N.M.; Bates, P.D.; Neelz, S.; Pender, G.; Villanueva, I.; Wright, N.G.; Liang, D.; Falconer, R.A.; Lin, B.; Waller, S.; et al. Benchmarking 2D hydraulic models for urban flooding. Proc. Inst. Civil Engineers Water Manag. 2008, 161, 13–30. [Google Scholar]
- Pullen, T.; Allsop, N.W.H.; Bruce, T.; Kortenhaus, A.; Schuttrumpf, H.; van der Meer, J. Eurotop, Wave Overtopping of Sea Defences and Related Structures—Assessment Manual. 2007. Available online: www.overtopping-manual.com (accessed on 24 April 2012).
- Owen, M.W. Design of Seawalls Allowing for Wave Overtopping; Report Ex 924; HR Wallingford: Oxfordshire, UK, 1980. [Google Scholar]
- TAWTechnical Report—Wave Run-Up and Wave Overtopping at Dikes; Technical advisory committee for flood defence in the Netherlands (TAW)TAW: Delft, The Netherlands, 2002.
- Gouldby, B.; Kingston, G.; Wills, M.; Van Gelder, P.; Buijis, F.; Kortenhaus, A. Flood Defence Reliability Calculator: Reliable User Manual; Floodsite Report Number: T07–10–08; January 2008.
- Allsop, W.; Kortenhaus, A.; Morris, M. Failure Mechanisms for Flood Defence Structures; Floodsite Report Number: T04–06–01; HR Wallingford Ltd.: Oxfordshire, UK, 2007. [Google Scholar]
- Vrouwenvelder, A.C.W.M.; Steenbergen, H.M.G.M.; Slijkhuis, K.A.H. Theoretical Manual of Pc-Ring, Part B: Statistical Models (in dutch); No. 98-con-r1431. Delft, The Netherland, 2001. [Google Scholar]
- Terzaghi, K.; Peck, R.B. Soil Mechanics in Engineering Practice; John Wiley & Sons: New York, NY, USA, 1967; p. 729. [Google Scholar]
- Powell, K.A. Predicting the Short Term Profile Response for Shingle Beaches; Hydraulics Research Report sr219; HR Wallingford: Oxfordshire, UK, 1990. [Google Scholar]
- DEFRA; EA, Performance and Reliability of Flood and Coastal Defences; R&d Technical Report Fd2318/tr 2; Buijis, F.; Simm, J.; Wallis, M.; Sayers, P. (Eds.) DEFRA: London, UK, 2005.
- Morris, M.; Hassan, M.; Kortenhaus, A.; Visser, P. Breaching Processes: A State of the Art Review; Floodsite Report Number t06–06–03, Revision Number 5_2_p01; FLOODsite Consortium: Oxfordshire, UK, 2009. [Google Scholar]
- Morris, M.; Hanson, G.; Hassan, M. Improving the accuracy of breach modelling: Why are we not progressing faster? J. Flood Risk Manag. 2008, 1, 150–161. [Google Scholar] [CrossRef]
- Wadey, M.; Nicholls, R.J.; Haigh, I.; Hutton, C. Creating a coastal flood event dataset for the solent region-the 10th March 2008 storm surge event. 2012; in preparation. [Google Scholar]
- Cope, S. Details of the breach of the medmerry/selsey shingle barrier during the storm on 10 March 2008. Personal Communication, New Forest District Council/Channel Coastal Observatory: Southampton, UK.
- EA, Solent & South Downs Area Report 10 March Tidal Flooding Event, Hampshire & Isle of Wight: A report of events, Consequences and Conclusions, Environment Agency: Rotherham, UK, 2010; Version 2.
- Wadey, M.P. Photograph taken by Matthew Wadey from the Channel Coastal Observatory overlooking Southampton Docks. National Oceanography Centre, Southampton, UK, 2:05PM, 10 March 2008.
- Evacuations as waves flood park. BBC World News. 10 March 2008. Available online: http://news.bbc.co.uk/1/hi/england/sussex/7287880.stm (accessed on 25 January 2012).
- Emsworth Residents Association. Flooding in Emsworth, Borough of Havant, during a Storm Surge in March 2008; Emsworth_Flood_2008_1.jpg. 10 March 2008. Available online: http://www.floods.emsworthhants.org.uk/ (accessed on 24 April 2012).
- NRA, Lymington/Pennington Flood Investigation–Interim Report; Report for National Rivers Authority Southern Region; Ea Report Reference Number: IMP/sch/008/5054, Southern Projects Ltd.: Jersey, UK, 1990.
- Stripling, S.; Bradbury, A.P.; Cope, S.N.; Brampton, A.H. Understanding Barrier Beaches; R&D Technical Report Fd1925/Tr; JOINT Defra/ea Flood and Coastal Erosion Risk Management R&D Programme: London, UK, 2008. Available online: http://sciencesearch.defra.gov.uk (accessed on 24 April 2012).
- Nicholls, R. The Stability of the Shingle Beaches in the Eastern Half of Christchurch Bay. Ph.D Dissertation, University of Southampton, Hampshire, UK, 1985. [Google Scholar]
- Nicholls, R.J.; Webber, N.B. The past, present and future evolution of hurst castle spit, Hampshir. Prog. Oceanogr. 1987, 18, 119–137. [Google Scholar]
- Jonkman, S.N.; Penning-Rowsell, E. Human instability in flood flows. JAWRA J. Am. Water Resour. Assoc. 2008, 44, 1208–1218. [Google Scholar]
- Pachauri, R.K.; Reisinger, A. (Eds.) Contribution of Working Groups I, II and III to the Fourth Assessment Report of the Intergovernmental Panel on Climate Change; IPCC, Climate Change 2007: Synthesis Report; IPCC: IPCC, Geneva, Switzerland, 2007. Available online: http://www.ipcc.ch/publications_and_data/ar4/syr/en/contents.html (accessed on 24 April 2012).
- Flather, R.A. Existing operational oceanography. Coast. Eng. 2000, 41, 13–40. [Google Scholar]
- Ray, J.; Coulling, E.; Evans, S.Y.; Spink, K. Developing a practical tool for coastal flood forecasting and warning. ICE Coastal Management: Innovative Coastal Zone Management.Sustainable Engineering for a Dynamic Coast, Belfast, UK, 2011. [Google Scholar]
- Wadey, M.; Nicholls, R.; Hutton, C. Threat of coastal inundation in the solent: Real-time forecasting. ICE Coastal Management: Innovative Coastal Zone Management. Sustainable Engineering for a Dynamic Coast, Belfast, UK, 2011. [Google Scholar]
- DEFRA; EA, Improving Flood Warnings: Final Report: Improving Institutional and Social Responses to Flooding; Science Report: Sc060019-Work Package 1a; Twigger-ross, C.; Fernández-bilbao, A.; Tapsell, S.; Walker, G.; Watson, N.; Collaborators, C.; Rose, P.; Dade, H.; Deeming, E. (Eds.) DEFRA: London, UK, 2009; p. 67, ISBN: 978-1-84911-058-7.
- Pappenberger, F.; Beven, K.J. Ignorance is bliss: Or seven reasons not to use uncertainty analysis. Water Resour. Res. 2006, 42, W05302. [Google Scholar] [CrossRef]
© 2012 by the authors; licensee MDPI, Basel, Switzerland. This article is an open-access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Wadey, M.P.; Nicholls, R.J.; Hutton, C. Coastal Flooding in the Solent: An Integrated Analysis of Defences and Inundation. Water 2012, 4, 430-459. https://doi.org/10.3390/w4020430
Wadey MP, Nicholls RJ, Hutton C. Coastal Flooding in the Solent: An Integrated Analysis of Defences and Inundation. Water. 2012; 4(2):430-459. https://doi.org/10.3390/w4020430
Chicago/Turabian StyleWadey, Matthew P., Robert J. Nicholls, and Craig Hutton. 2012. "Coastal Flooding in the Solent: An Integrated Analysis of Defences and Inundation" Water 4, no. 2: 430-459. https://doi.org/10.3390/w4020430
APA StyleWadey, M. P., Nicholls, R. J., & Hutton, C. (2012). Coastal Flooding in the Solent: An Integrated Analysis of Defences and Inundation. Water, 4(2), 430-459. https://doi.org/10.3390/w4020430





