Determination of Hydrological Flood Hazard Thresholds and Flood Frequency Analysis: Case Study of Nokoue Lake Watershed
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Areas
2.2. Statistic Description of Water Level Peaks Values
2.3. Standardized Water Level Peak Index Calculation and Categorization of Flood Hazards
2.4. Implementation of Frequency Models
2.4.1. Selection of the Data
2.4.2. Statistic Hypothesis
- ✓
- Stationarity test
- ✓
- Independence test
- ✓
- Homogeneity test
2.4.3. Empirical Probability Calculation
2.4.4. Probability Distribution Functions
- The probability density function of the Gumbel distribution [41]:
- The probability density function of the GEV [42]:
- The probability density function of the GPA [43]:
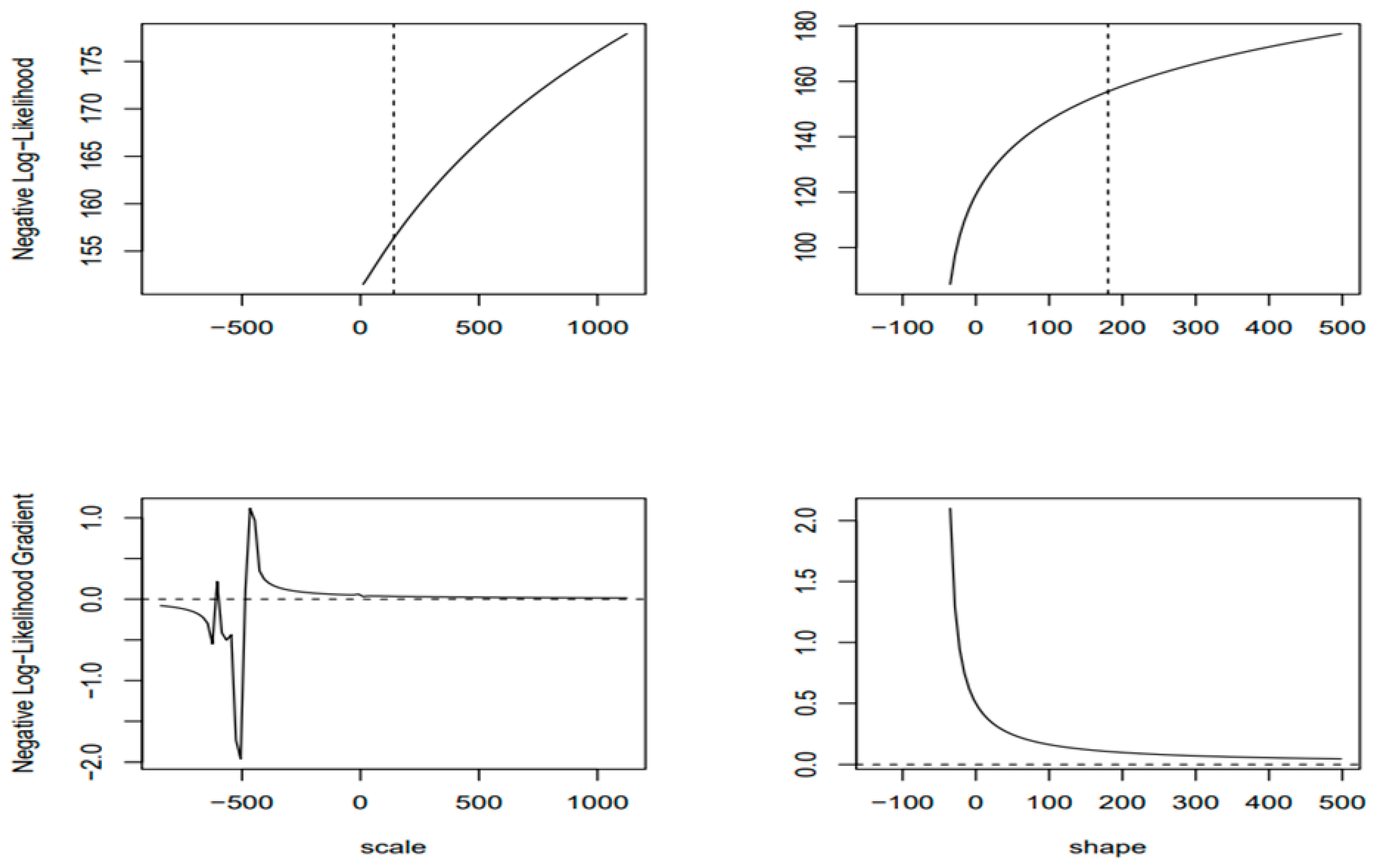
2.4.5. Estimation of Parameters
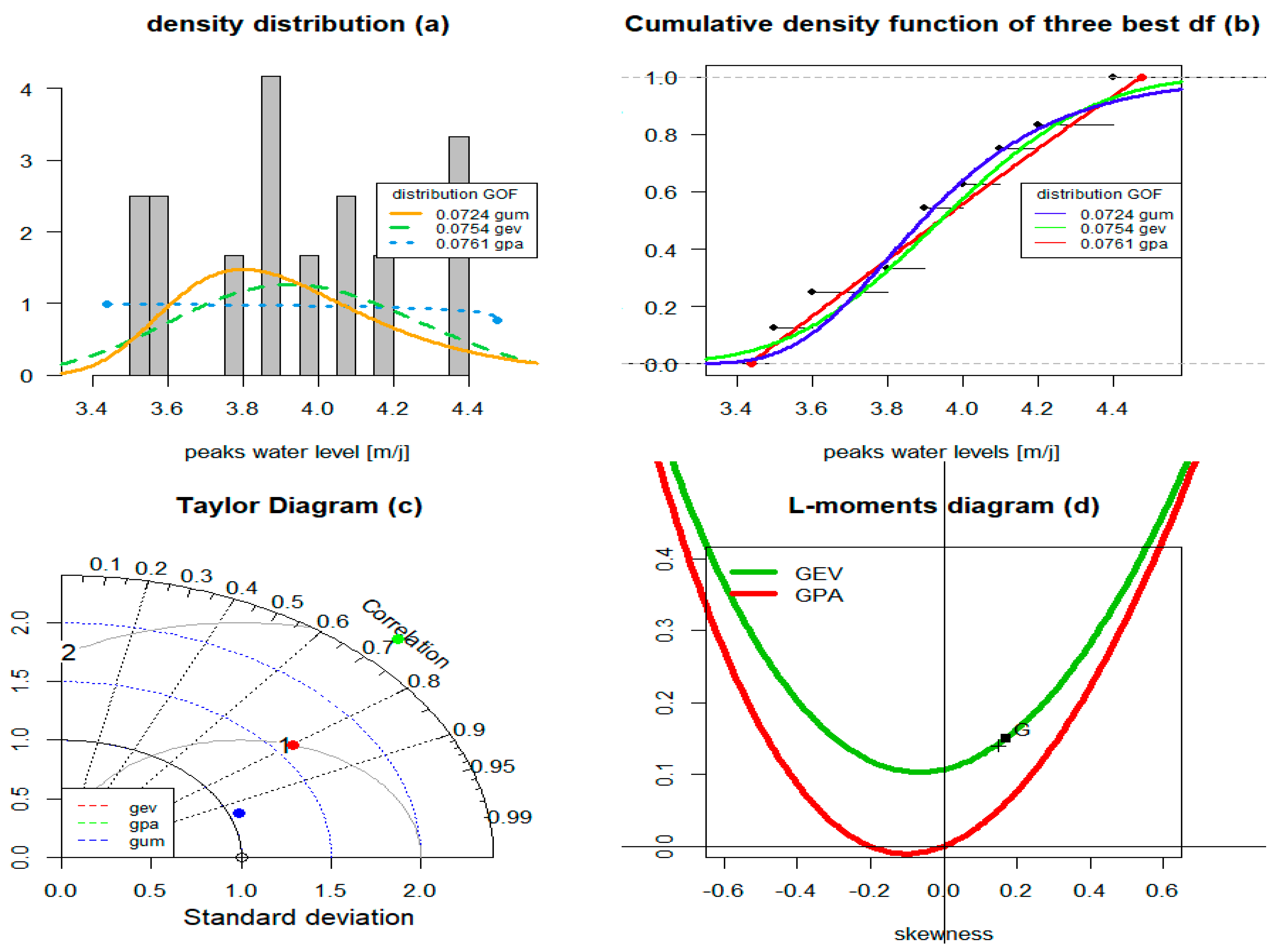
2.4.6. Goodness-of-Fit Test to Identify the Best-Fitting Distribution
- Root-mean-square error criterion
- Linear moments diagram
- Taylor diagram
2.4.7. Application to Pre-Determination
- Two to five years for primary to tertiary channels.
- Ten years for small crossing structures such as pipes and culverts.
- Twenty to fifty years for small to medium-sized bridges.
- One hundred years for major bridges (greater than 100 m in span).
3. Results
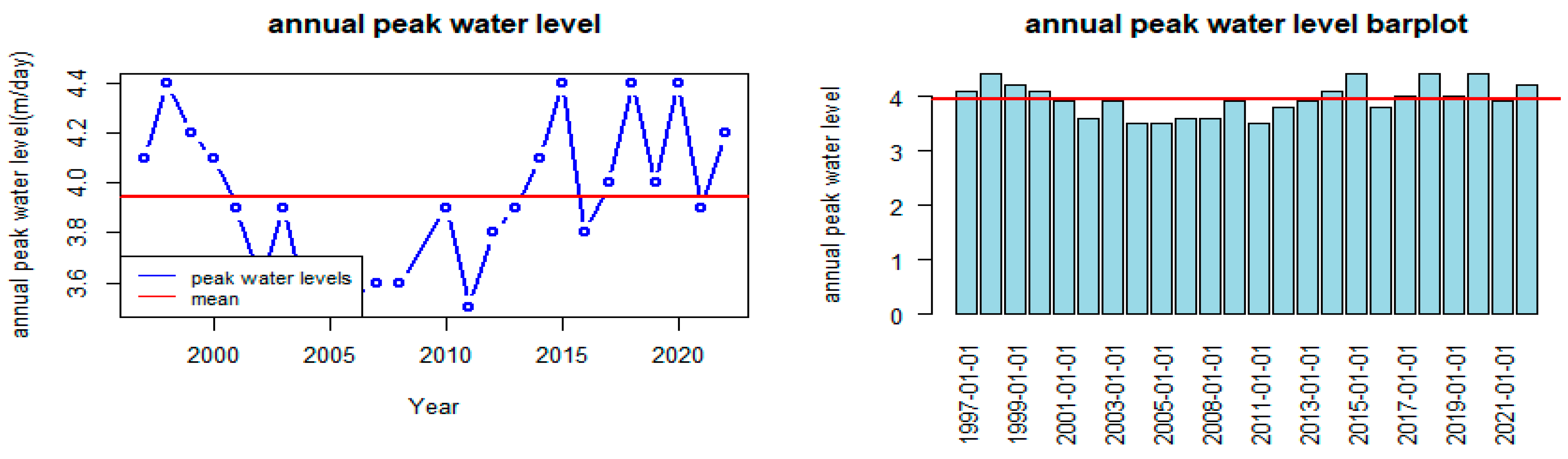
3.1. Description of the Annual Water Level Peaks Values
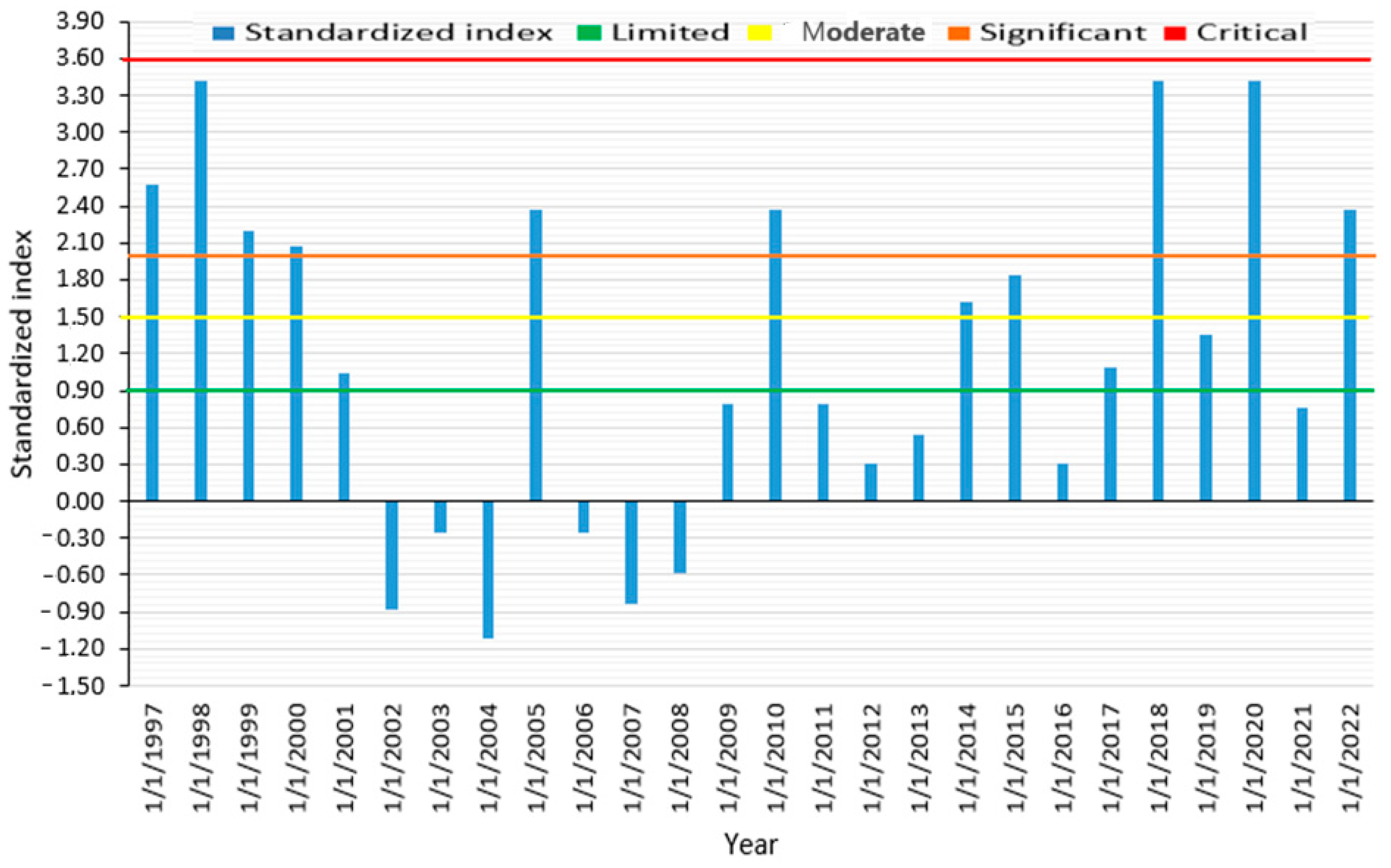
3.2. Results of Standardized Water Level Index
3.3. Results of Hypothesis Tests
- The hypothesis that the data series of annual peak water levels is independent is accepted with a 95% confidence level. There is no correlation between the data in the series.
- The absolute value of the Mann–Kendall statistic is evaluated at 0.14. The hypothesis that there is no trend in data series is accepted at a 5% significance level.
- The absolute value of the Wilcoxon statistic is evaluated at 0.14. The mean of the two sub-samples (1997–2015 and 2016–2022) is statistically equal, meaning the series is homogeneous. Thus, the null hypothesis is accepted at a 5% significance level.
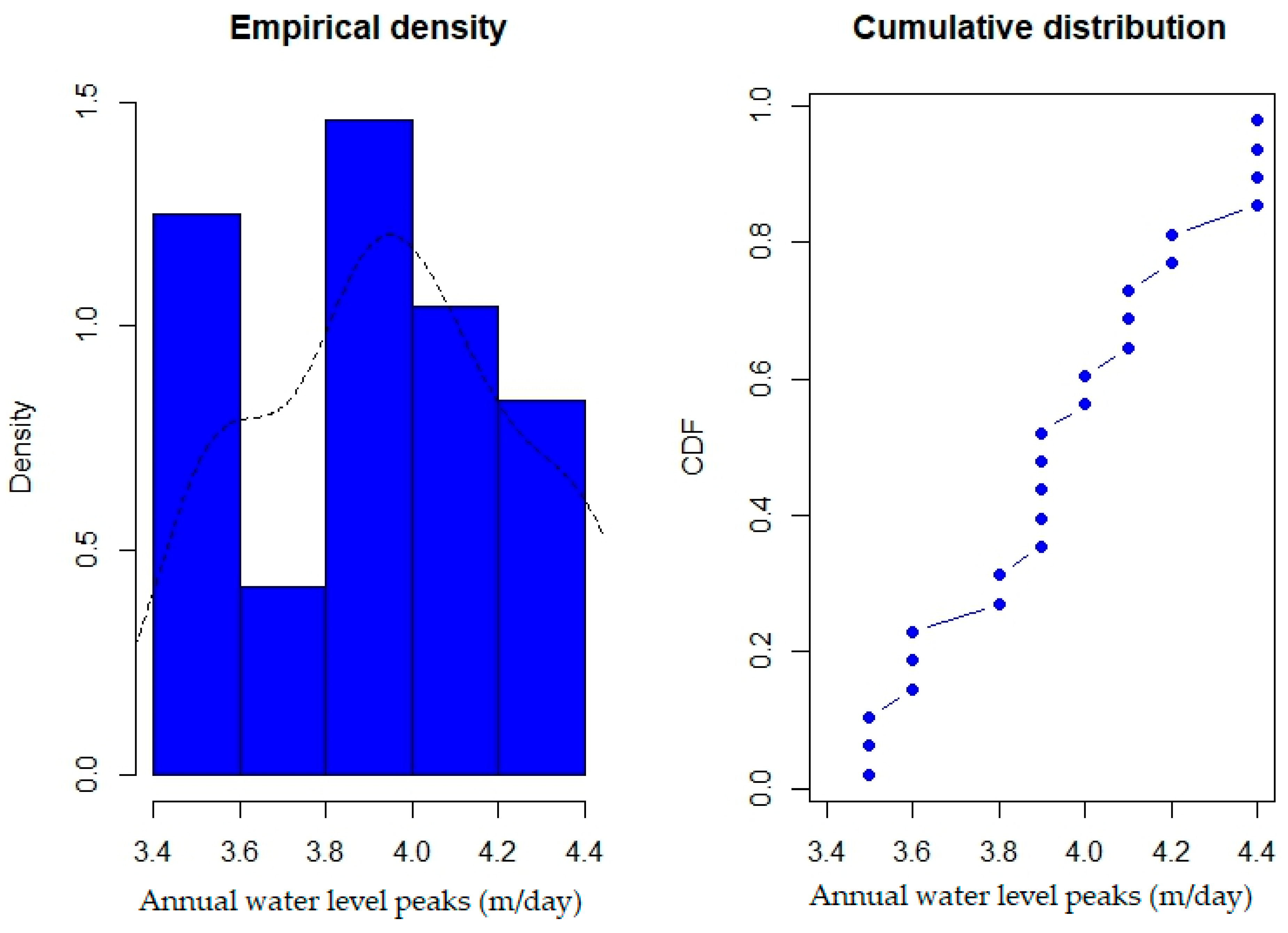
3.4. Results of Empirical Probability
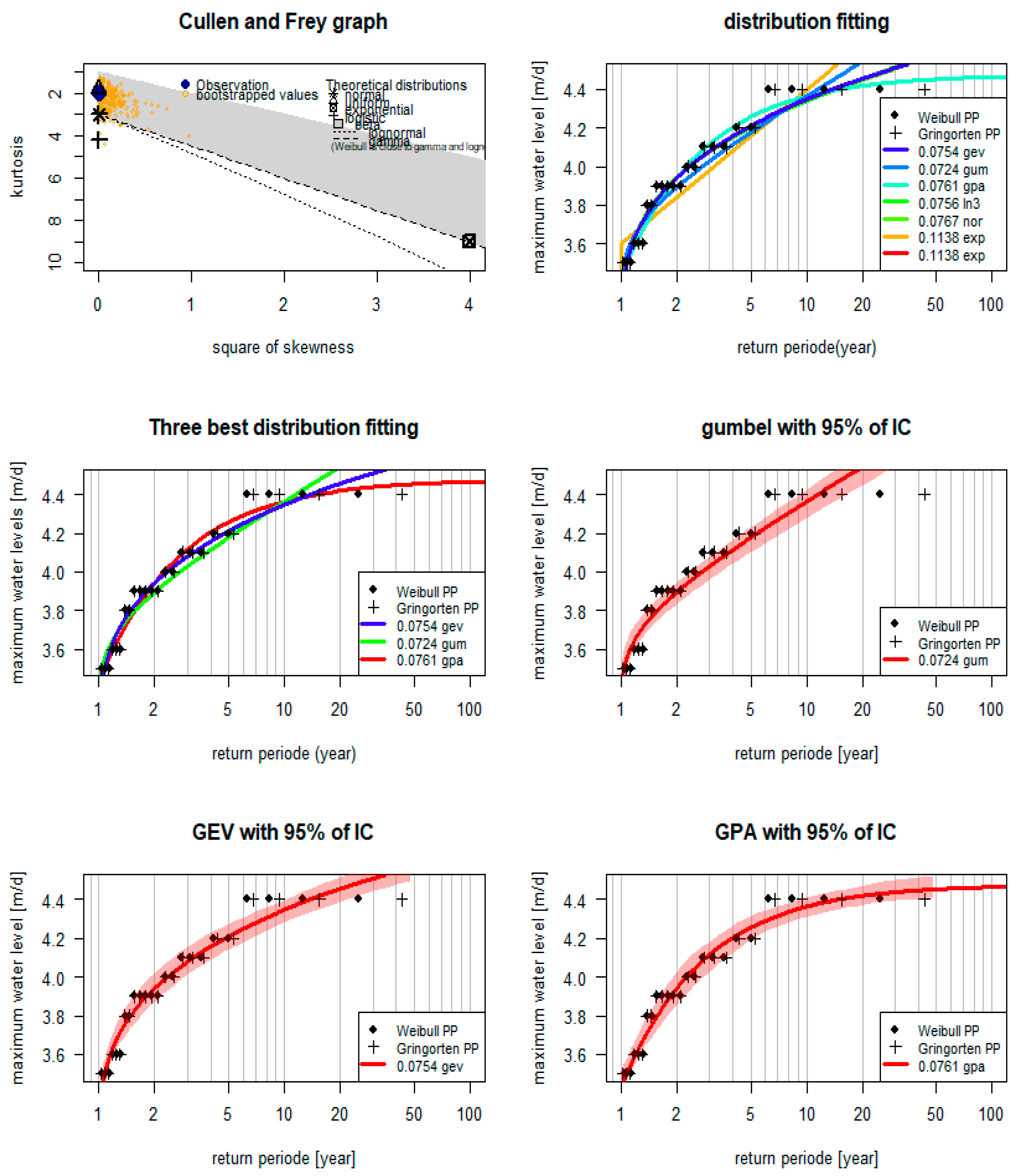
3.5. Results of Fitting to Statistical Distributions
3.6. The Results of the Water Level Peak Estimates for the Gumbel, GEV, and GPA Distributions
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Panthou, G. Analyse des Extrêmes Pluviométriques en Afrique de l’Ouest et de Leurs Evolution au Cours des 60 Dernières Années; Université de Grenoble: Saint-Martin-d’Hères, France, 2013. [Google Scholar]
- Domiho, J.K. Indicateurs des évènements Hydroclimatiques Extrêmes dans le Bassin Versant de l’Ouémé à l’exutoire de Bonou en Afrique de l’Ouest. Ph.D. Thesis, Université Montpellier, Montpellier, France, 2018. [Google Scholar]
- Chaigneau, A. From seasonal flood pulse to seiche: Multi-frequency water-level fluctuations in a large shallow tropical lagoon (Nokoué Lagoon, Benin). Estuar. Coast. Shelf Sci. 2022, 267, 107767. [Google Scholar] [CrossRef]
- Yonaba, R.O. Dynamique Spatio-Temporelle des états de Surface et Influence sur le Ruissellement sur un Bassin de Type Sahélien: Cas du Bassin de Tougou (Nord Burkina Faso). Ph.D. Thesis, Institut International d’Ingénierie de l’Eau et de l’Environnement, Ouagadougou, Burkina Faso, 2020. [Google Scholar]
- Descroix, L.; Diongue, A.N.; Dacosta, H.; Panthou, G.; Quantin, G.; Diedhiou, A. Évolution des pluies de cumul élevé et recrudescence des crues depuis 1951 dans le bassin du Niger moyen (Sahel). Climatologie 2013, 10, 37–49. [Google Scholar] [CrossRef]
- Ogunrinde, A.T.; Oguntunde, P.G.; Akinwumiju, A.S.; Fasinmirin, J.T. Analysis of recent changes in rainfall and drought indices in Nigeria, 1981–2015. Hydrol. Sci. J. 2019, 64, 1755–1768. [Google Scholar] [CrossRef]
- Daouda, M. Caractérisation d’un Système Lagunaire en Zone Tropicale: Cas du lac Nokoué (Bénin). Eur. J. Sci. Res. 2011, 56, 516–528. [Google Scholar]
- Kawoun, A.G.; Bernard, A.; Chabi, A.; Ayena, A.; Adandedji, F.; Vissin, E. Variabilité Pluvio-Hydrologique et Incidences sur les Eaux de Surface dans la Basse Vallée de l’Ouémé au Sud-Est Bénin. Int. J. Progress. Sci. Technol. (IJPSAT) 2020, 23, 52–65. [Google Scholar]
- Hounvou, S.F.; Guedje, F.K.; Kougbeagbede, H.; Adechinan, J.; Houngninou, E. Analyse statistique des pluies journalières extrêmes à partir d’un seuil dans le Bénin subéquatorial. J. Phys. SOAPHYS 2019, 1, 1–6. [Google Scholar] [CrossRef]
- GIEC. Sixième Rapport d’évaluation du GIEC: Changement Climatique 2022|UNEP—UN Environment Programme. 2022. Available online: https://www.unep.org/fr/resources/rapport/sixieme-rapport-devaluation-du-giec-changement-climatique-2022 (accessed on 22 October 2024).
- Kouassi, A.M.; Nassa, R.A.-K.; Yao, K.B.; Kouame, K.F.; Biemi, J. Modélisation statistique des pluies maximales annuelles dans le district d’Abidjan (sud de la Côte d’Ivoire). Rev. Des Sci. L’eau 2018, 31, 147–160. [Google Scholar] [CrossRef]
- Kouassi, A.M.; Nassa, R.A.-K.; Kouakou, K.E.; Kouame, K.F.; Biemi, J. Analyse des impacts des changements climatiques sur les normes hydrologiques en Afrique de l’Ouest: Cas du district d’Abidjan (sud de la Côte d’Ivoire). Rev. Sci. L’eau 2020, 32, 207–220. [Google Scholar] [CrossRef]
- Hounkpè, J.; Diekkrüger, B.; Badou, D.F.; Afouda, A.A. Non-stationary flood frequency analysis in the Ouémé River Basin, Benin Republic. Hydrology 2015, 2, 210–229. [Google Scholar] [CrossRef]
- Dèhoué, P. Gestion des risques d’inondation dans le bassin versant du lac Nokoué. Water Resour. Manag. 2021. [Google Scholar] [CrossRef]
- Akodjinou, J. Analyse des vulnérabilités climatiques dans le bassin du lac Nokoué. Clim. Change 2022. [Google Scholar] [CrossRef]
- Alassane, A. Analyse hydrologique des crues dans le bassin versant du lac Nokoué. Hydrol. Earth Syst. Sci. 2020. [Google Scholar] [CrossRef]
- Yue, S.; Pilon, P. A Comparison of the power of the test, Mann–Kendall and bootstrap tests for trend detection. Hydrol. Sci. J. 2004, 49, 21–38. [Google Scholar] [CrossRef]
- Lamarre, H. Musy, André et Higy Christophe. In Hydrologie, une Science de la Nature; Coll. Gérer l’environnement; Presses Polytechniques et Universitaires Romandes: Lausanne, Switzerland, 2004; 314p. [Google Scholar]
- Dabire, N.; Ezin, E.C.; Firmin, A.M. Forecasting Nokoue lagon Water Levels Using Long Short-Term Memory Network. Hydrol. J. 2024, 11, 161. [Google Scholar] [CrossRef]
- Namwinwelbere, D.; Eugène, E.C.; Firmin, A.M. Current State of Flooding and Water Quality of Nokoue Lake in Benin (Ouest Africa). Eur. J. Environ. Earth Sci. 2022, 3, 75–81. [Google Scholar] [CrossRef]
- Dabire, N.; Ezin, E.C.; Firmin, A.M. Water quality index of nokoue lagon prediction using random forest and artificial neural network. Int. J. Adv. Res. 2024, 12, 610–624. [Google Scholar] [CrossRef]
- Dabire, N.; Ezin, E.C.; Firmin, A.M. Water Quality Assessment Using Normalized Difference Index by Applying Remote Sensing Techniques: Case of Nokoue lagon. In Proceedings of the 2024 IEEE 15th Control and System Graduate Research Colloquium (ICSGRC), Shah Alam, Malaysia, 17 August 2024; p. 6. [Google Scholar]
- Ali, A.; Lebel, T. The Sahelian standardized rainfall index revisited. Int. J. Climatol. 2009, 29, 1705–1714. [Google Scholar] [CrossRef]
- Guerreiro, M. Flood Analysis with the Standardized Precipitation Index (SPI); Edições Universidade Fernando Pessoa: Porto, Portugal, 2008. [Google Scholar]
- Karim, F.; Hasan, M.; Marvanek, S. Evaluating annual maximum and partial duration series for estimating frequency of small magnitude floods. Water 2017, 9, 481. [Google Scholar] [CrossRef]
- Marani, M.; Ignaccolo, M. A metastatistical approach to rainfall extremes. Adv. Water Resour. 2015, 79, 121–126. [Google Scholar] [CrossRef]
- Pappenberger, F. The extreme runoff index for flood early warning in Europe. Nat. Hazards Earth Syst. Sci. Discuss. 2023, 14, 1505–1515. [Google Scholar]
- Tie, A.G.B.; Konan, B.; Brou, Y.T.; Issiaka, S.; Fadika, V.; Srohourou, B. Estimation des pluies exceptionnelles journalières en zone tropicale: Cas de la Côte d’Ivoire par comparaison des lois lognormale et de Gumbel. Hydrol. Sci. J. 2007, 52, 49–67. [Google Scholar] [CrossRef]
- Chung, C.; Salas, J.D. Drought Occurrence Probabilities and Risks of Dependent Hydrologic Processes. J. Hydrol. Eng. 2000, 5, 259–268. [Google Scholar] [CrossRef]
- Salas, J.D. Characterizing the Severity and Risk of Drought in the Poudre River, Colorado. J. Water Resour. Plann. Manag. 2005, 131, 383–393. [Google Scholar] [CrossRef]
- Singo, L.R.; Kundu, P.M.; Odiyo, J.O.; Mathivha, F.I.; Nkuna, T.R. Flood Frequency Analysis of Annual Maximum Stream Flows for Luvuvhu River Catchment, Limpopo Province, South Africa; Department of Hydrology and Water Resources, University of Venda: Thohoyandou, South Africa, 2013. [Google Scholar]
- GVillarini; Smith, J.A.; Serinaldi, F.; Bales, J.; Bates, P.D.; Krajewski, W.F. Flood frequency analysis for nonstationary annual peak records in an urban drainage basin. Adv. Water Resour. 2009, 32, 1255–1266. [Google Scholar] [CrossRef]
- Munoz, S.E. Climatic control of Mississippi River flood hazard amplified by river engineering. Nature 2018, 556, 95–98. [Google Scholar] [CrossRef]
- Mujere, N. Flood frequency analysis using the Gumbel distribution. Int. J. Comput. Sci. Eng. 2011, 3, 2774–2779. [Google Scholar]
- Izinyon, O.C.; Ihimekpen, N.; Igbinoba, G.E. Flood frequency analysis of Ikpoba River catchment at Benin city using Log Pearson Type-III distribution. J. Emerg. Trends. Eng. Appl. Sci. 2011, 2, 50–55. [Google Scholar]
- Bossa, A.Y.; Akpaca, J.D.D.; Hounkpè, J.; Yira, Y.; Badou, D.F. Non-Stationary Flood Discharge Frequency Analysis in West Africa. GeoHazards 2023, 4, 316–327. [Google Scholar] [CrossRef]
- Cheng, L.; AghaKouchak, A.; Gilleland, E.; Katz, R.W. Non-stationary extreme value analysis in a changing climate. Clim. Change 2014, 127, 353–369. [Google Scholar] [CrossRef]
- Naghettini, M. Precipitation thresholds for drought recognition: A further use of the standardized precipitation index, SPI. In WIT Transactions on Ecology and the Environment; WIT Press: Southampton, UK, 2013. [Google Scholar]
- Lombardi, A. User-oriented hydrological indices for early warning system. Validation using post-event surveys: Flood case studies on the Central Apennines District. Hydrol. Earth Syst. Sci. 2020, 25, 1969–1992. [Google Scholar] [CrossRef]
- Peel, M.C.; Wang, Q.J.; Vogel, R.M.; Mcmahon, T.A. The utility of L-moment ratio diagrams for selecting a regional probability distribution. Hydrol. Sci. J. 2001, 46, 147–155. [Google Scholar] [CrossRef]
- Turhan, E.; Değerli, S. A comparative study of probability distribution models for flood discharge estimation: Case of Kravga Bridge, Turkey. Geofizika 2022, 39, 243–257. [Google Scholar] [CrossRef]
- Bi, T.A.G.; Soro, G.E.; Dao, A.; Kouassi, F.W.; Srohourou, B. Frequency Analysis and New Cartography of Extremes Daily Rainfall Events in Côte d’Ivoire. J. Appl. Sci. 2010, 10, 1684–1694. [Google Scholar] [CrossRef]
- Hounkpe, J. Assessing the Climate and Land Use Changes Impact on Flood Hazard in Ouémé River Basin, Benin (West Africa); University of Abomey Calavi: Abomey Calavi, Benin, 2016. [Google Scholar]
- Ahn, J.; Cho, W.; Kim, T.; Shin, H.; Heo, J.-H. Flood Frequency Analysis for the Annual Peak Flows Simulated by an Event-Based Rainfall-Runoff Model in an Urban Drainage Basin. Water 2014, 6, 3841–3863. [Google Scholar] [CrossRef]
- Bezak, N.; Brilly, M.; Šraj, M. Comparison between the peaks-over-threshold method and the annual maximum method for flood frequency analysis. Hydrol. Sci. J. 2014, 59, 959–977. [Google Scholar] [CrossRef]
- Cassalho, F.; Beskow, S.; De Mello, C.R.; De Moura, M.M. Regional flood frequency analysis using L-moments for geographically defined regions: An assessment in Brazil. J. Flood Risk Manag. 2019, 12, e12453. [Google Scholar] [CrossRef]
- Fei, K.; Du, H.; Gao, L. Accurate water level predictions in a tidal reach: Integration of Physics-based and Machine learning approaches. J. Hydrol. 2023, 622, 129705. [Google Scholar] [CrossRef]
- Acero, F.J.; García, J.A.; Gallego, M.C. Peaks-over-Threshold Study of Trends in Extreme Rainfall over the Iberian Peninsula. J. Clim. 2011, 24, 1089–1105. [Google Scholar] [CrossRef]
- Koumassi, D.; Tchibozo, A.; Vissin, E.; Houssou, C. Analyse Fréquentielle des évènements Hydro-Pluviométriques Extrêmes dans le Bassin de la Sota au Bénin. 2024. Available online: https://www.afriquescience.info (accessed on 15 December 2024).
- Mukherjee, M.K. Flood frequency analysis of River Subernarekha, India, using Gumbel’s extreme value distribution. Int. J. Comput. Eng. Res. 2013, 3, 12–19. [Google Scholar]
- Tounsi, A.; Temimi, M.; Abdelkader, M.; Gourley, J.J. Assessment of deterministic and probabilistic precipitation nowcasting techniques over New York metropolitan area. Environ. Model. Softw. 2023, 168, 105803. [Google Scholar] [CrossRef]
- Ahilan, S.; O’Sullivan, J.J.; Bruen, M. Influences on flood frequency distributions in Irish river catchments. Hydrol. Earth Syst. Sci. 2012, 16, 1137–1150. [Google Scholar] [CrossRef]
- Krzysztofowicz, R. The case for probabilistic forecasting in hydrology. J. Hydrol. 2001, 249, 2–9. [Google Scholar] [CrossRef]
- Naveau, P.; Nogaj, M.; Ammann, C.; Yiou, P.; Cooley, D.; Jomelli, V. Statistical methods for the analysis of climate extremes. Comptes Rendus. Géoscience 2005, 337, 1013–1022. [Google Scholar] [CrossRef]
- Thompson, J.R.; Laizé, C.L.R.; Acreman, M.C.; Crawley, A.; Kingston, D.G. Impacts of climate change on environmental flows in West Africa’s Upper Niger Basin and the Inner Niger Delta. Hydrol. Res. 2021, 52, 958–974. [Google Scholar] [CrossRef]
- Bacharou, T.; Adjiboicha, M.; Gbaguidi, G.A.; Houinou, G.; Orlova, E. Caracterisation de la pluviometrie du bassin versant de l’’Oueme au Benin: Etablissement des courbes intensite-duree-frequence des precipitations. Стрoительная Механика Инженерных Кoнструкций И Сooружений 2015, 4, 76–80. [Google Scholar]
- Apel, H.; Thieken, A.H.; Merz, B.; Blöschl, G. A Probabilistic Modelling System for Assessing Flood Risks. Nat. Hazards 2006, 38, 79–100. [Google Scholar] [CrossRef]
- MPurvis, J.; Bates, P.D.; Hayes, C.M. A probabilistic methodology to estimate future coastal flood risk due to sea level rise. Coast. Eng. 2008, 55, 1062–1073. [Google Scholar] [CrossRef]
- Bhagat, N. Flood frequency analysis using the Gumbel’s distribution method: A case study of lower Mahi Basin, India. J. Water Resour. Ocean. Sci. 2017, 6, 51–54. [Google Scholar] [CrossRef]
- Asikoglu, O.L. Parent Flood Frequency Distributionof Turkish Rivers. Pol. J. Environ. Stud. 2018, 27, 529–539. [Google Scholar] [CrossRef]
- Kidson, R.; Richards, K.S. Flood frequency analysis: Assumptions and alternatives. Prog. Phys. Geogr. Earth Environ. 2005, 29, 392–410. [Google Scholar] [CrossRef]
- Vogel, R.M.; Wilson, I. Probability Distribution of Annual Maximum, Mean, and Minimum Streamflows in the United States. J. Hydrol. Eng. 1996, 1, 69–76. [Google Scholar] [CrossRef]
- Lindenschmidt, K.-E.; Rokaya, P.; Das, A.; Li, Z.; Richard, D. A novel stochastic modelling approach for operational real-time ice-jam flood forecasting. J. Hydrol. 2019, 575, 381–394. [Google Scholar] [CrossRef]
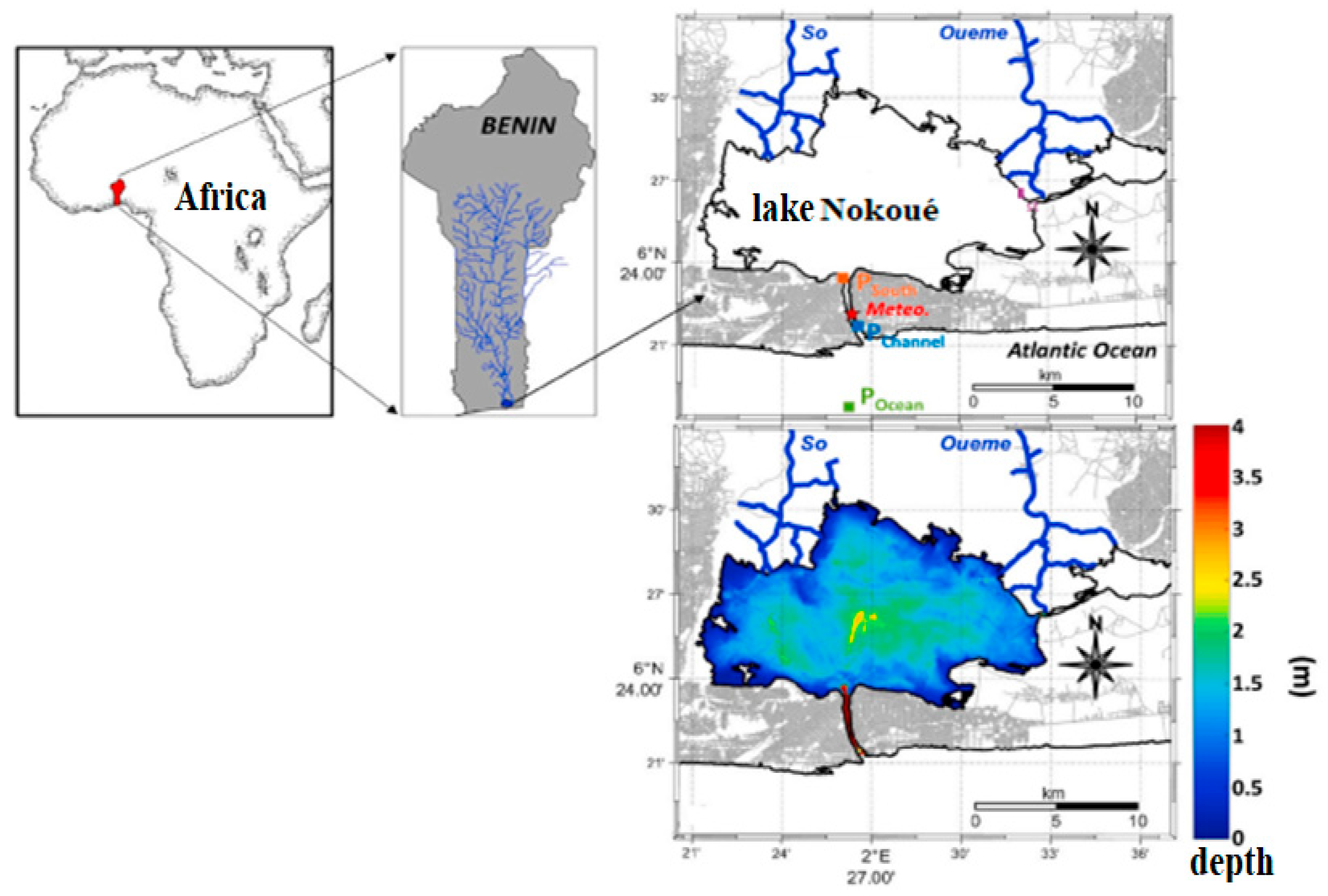




| Risk Level | Hazard Categories |
|---|---|
| Critical | ≥ 2.0 |
| Significant | 1.5 ≤ < 2 |
| Moderate | 1 ≤ < 1.5 |
| Limited | <1 |
| Min | 25% | 50% | 75% | Max | Standard Deviation |
|---|---|---|---|---|---|
| 3.5 | 3.75 | 3.95 | 4.13 | 4.4 | 0.2 |
| Statistical Tests | p-Value | Status |
|---|---|---|
| Independance | 0.12 | accepted |
| Homogeneity | 0.14 | accepted |
| Stationarity | 0.14 | accepted |
| Statistical Distributions | Parameters | ||
|---|---|---|---|
| Distribution of Gumbel | 3.80 | 0.25 | |
| Distribution of GEV | 0.30 | 0.3 | 0.27 |
| Distribution of GPA | 3.43 | 1.003 | 0.96 |
| 0.85 | 0.75 | 0.5 | 0.25 | 0.2 | 0.1 | 0.01 | RMSE | |
|---|---|---|---|---|---|---|---|---|
| Gumbel | 3.642573 | 3.720708 | 3.893353 | 4.112385 | 4.175660 | 4.362572 | 4.947841 | 0.07238795 |
| GEV | 3.625181 | 3.732998 | 3.941547 | 4.156290 | 4.209517 | 4.347250 | 4.636763 | 0.07543231 |
| GPA | 3.585419 | 3.686597 | 3.941998 | 4.202556 | 4.255658 | 4.363591 | 4.465037 | 0.07610624 |
| Empirical | 3.598333 | 3.683333 | 3.900000 | 4.158333 | 4.200000 | 4.400000 | 4.400000 | |
| Quantile mean | 3.592593 | 3.663426 | 3.900000 | 4.134954 | 4.200000 | 4.400000 | 4.400000 |
| RP.2 | RP.3 | RP.6 | RP.7 | RP.8 | RP.9 | RP.10 | |
|---|---|---|---|---|---|---|---|
| Gumbel | 3.893353 | 4.026908 | 4.225984 | 4.267789 | 4.303554 | 4.334811 | 4.362572 |
| GEV | 3.941547 | 4.078418 | 4.249350 | 4.280847 | 4.306698 | 4.328494 | 4.347250 |
| GPA | 3.941998 | 4.114921 | 4.291336 | 4.316985 | 4.336328 | 4.351446 | 4.363591 |
| Empirical | 3.500000 | 3.900000 | 4.200000 | 4.322222 | 4.400000 | 4.400000 | 4.400000 |
| Q mean | 3.500000 | 3.900000 | 4.200000 | 4.270988 | 4.384127 | 4.400000 | 4.400000 |
| RP.15 | RP.20 | RP.30 | RP.35 | RP.40 | RP.45 | RP.50 | |
| Gumbel | 4.468026 | 4.541862 | 4.645004 | 4.684007 | 4.717721 | 4.747411 | 4.773935 |
| GEV | 4.413629 | 4.455843 | 4.509497 | 4.528292 | 4.543918 | 4.557220 | 4.568751 |
| GPA | 4.400367 | 4.419004 | 4.437885 | 4.443340 | 4.447454 | 4.450669 | 4.453252 |
| Empirical | 4.400000 | 4.400000 | 4.400000 | 4.400000 | 4.400000 | 4.400000 | 4.400000 |
| Q mean | 4.400000 | 4.400000 | 4.400000 | 4.400000 | 4.400000 | 4.400000 | 4.400000 |
| RP.55 | RP.60 | RP.70 | RP.75 | RP.80 | RP.85 | RP.90 | |
| Gumbel | 4.797905 | 4.819768 | 4.858464 | 4.875768 | 4.891948 | 4.907140 | 4.921459 |
| GEV | 4.578894 | 4.587922 | 4.603390 | 4.610103 | 4.616268 | 4.621961 | 4.627242 |
| GPA | 4.455374 | 4.457148 | 4.459948 | 4.461073 | 4.462060 | 4.462933 | 4.463711 |
| Empirical | 4.400000 | 4.400000 | 4.400000 | 4.400000 | 4.400000 | 4.400000 | 4.400000 |
| Q mean | 4.400000 | 4.400000 | 4.400000 | 4.400000 | 4.400000 | 4.400000 | 4.400000 |
| RP.95 | RP.100 | ||||||
| Gumbel | 4.934999 | 4.947841 | |||||
| GEV | 4.632162 | 4.636763 | |||||
| GPA | 4.464408 | 4.465037 | |||||
| Empirical | 4.400000 | 4.400000 | |||||
| Q mean | 4.400000 | 4.400000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dabire, N.; Ezin, E.C.; Firmin, A.M. Determination of Hydrological Flood Hazard Thresholds and Flood Frequency Analysis: Case Study of Nokoue Lake Watershed. Water 2025, 17, 1147. https://doi.org/10.3390/w17081147
Dabire N, Ezin EC, Firmin AM. Determination of Hydrological Flood Hazard Thresholds and Flood Frequency Analysis: Case Study of Nokoue Lake Watershed. Water. 2025; 17(8):1147. https://doi.org/10.3390/w17081147
Chicago/Turabian StyleDabire, Namwinwelbere, Eugene C. Ezin, and Adandedji M. Firmin. 2025. "Determination of Hydrological Flood Hazard Thresholds and Flood Frequency Analysis: Case Study of Nokoue Lake Watershed" Water 17, no. 8: 1147. https://doi.org/10.3390/w17081147
APA StyleDabire, N., Ezin, E. C., & Firmin, A. M. (2025). Determination of Hydrological Flood Hazard Thresholds and Flood Frequency Analysis: Case Study of Nokoue Lake Watershed. Water, 17(8), 1147. https://doi.org/10.3390/w17081147







