Performance of Emitters in Drip Irrigation Systems Using Computational Fluid Dynamic Analysis †
Abstract
1. Introduction
2. Materials and Methods
2.1. Numerical Method
2.2. Case Study
3. Results
3.1. Discharge Exponent
3.2. Flow Rate and Cross-Section Area Relation
3.3. Velocity Field Analysis
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Saccone, D.; De Marchis, M. Optimization of the design of labyrinth emitter for agriculture irrigation using computational fluid dynamic analysis. In Proceedings of the AlP Conference Proceedings, Thessaloniki, Greece, 14–18 March 2018; AlP Publishing LLC: Atlanta, GA, USA, 2018; Volume 2040, No. 1. p. 140013. [Google Scholar]
- De Marchis, M.; Milici, B.; Freni, G. Pressure-discharge law of local tanks connected to a water distribution network: Experimental and mathematical results. Water 2015, 7, 4701–4723. [Google Scholar] [CrossRef]
- Bruno, F.; De Marchis, M.; Milici, B.; Saccone, D.; Traina, F. A pressure monitoring system for water distribution networks based on arduino microcontroller. Water 2021, 13, 2321. [Google Scholar] [CrossRef]
- De Marchis, M.; Milici, B. Leakage Estimation in Water Distribution Network: Effect of the Shape and Size Cracks. Water Resour. Manag. 2019, 33, 1167–1183. [Google Scholar] [CrossRef]
- Yurdem, H.; Demir, V.; Mancuhan, A. Development of a simplified model for predicting the optimum lengths of drip irrigation laterals with coextruded cylindrical in-line emitters. Biosyst. Eng. 2015, 137, 22–35. [Google Scholar] [CrossRef]
- Li, Y.; Li, G.; Qiu, X.; Wang, J. Modeling of hydraulic characteristics through labyrinth emitter in drip irrigation using computational fluid dynamics. Trans. CSAE 2005, 21, 3. [Google Scholar]
- Wang, W.; Wang, F.; Zhao, F. Simulation of unsteady flow in labyrinth emitter of drip irrigation system. In Computers in Agriculture and Natural Resources, 4th World Congress Conference Proceedings; American Society of Agricultural and Biological Engineers: St. Joseph, MI, USA, 2006. [Google Scholar]
- Zhang, J.; Zhao, W.; Wei, Z.; Tang, Y.; Lu, B. Numerical and experimental study on hydraulic performance of emitters with arc labyrinth channels. Comput. Electron. Agric. 2007, 56, 120–129. [Google Scholar] [CrossRef]
- Zhao, W.; Zhang, J.; Tang, Y.; Wei, Z.; Lu, B. Research on transitional flow characteristics of labyrinth channel emitter. In Computer and Computing Technologies in Agriculture II, Volume 2; Springer: Boston, MA, USA, 2008. [Google Scholar]
- Wang, L.; Wei, Z.; Deng, T.; Tang, Y. Step-by-step CFD design method of pressure compensating emitter. Trans. Chin. Soc. Agric. Eng. 2012, 28, 86–92. [Google Scholar]
- Wei, Z.; Cao, M.; Liu, X.; Tang, Y.; Lu, B. Flow Behaviour Analysis and Experimental Investigation for Emitter Microchannels. Chin. J. Mech. Eng. 2012, 25, 729–737. [Google Scholar] [CrossRef]
- Celik, H.K.; Karayel, D.; Lupeanu, M.E.; Rennie, A.E.W.; Akinci, I. Determination of head losses in drip irrigation laterals with cylindrical in-line type emitters through CFD analysis. Bulg. J. Agric. Sci. 2015, 21, 703–710. [Google Scholar]
- Souza, W.J.; Sinobas, L.R.; Sánchez, R.; Botrel, T.A.; Coelho, R.D. Prototype emitter for use in subsurface drip irrigation: Manufacturing, hydraulic evaluation and experimental analyses. Biosyst. Eng. 2014, 128, 41–51. [Google Scholar] [CrossRef]
- Liu, X.; He, X.; Zhang, L. Simplified method for estimating discharge of microporous ceramic emitters for drip irrigation. Biosyst. Eng. 2022, 219, 38–55. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, L.; Sun, Y.; Tong, X.; He, X.; Wei, Y. A novel variable discharge emitter for irrigation and salt-leaching. Biosyst. Eng. 2024, 246, 178–182. [Google Scholar] [CrossRef]
- Chen, X.; Wei, Z.; Wei, C.; He, K. Effect of compensation chamber structure on the hydraulic performance of pressure compensating drip emitters. Biosyst. Eng. 2022, 214, 107–121. [Google Scholar] [CrossRef]
- Li, H.; Li, P.; Li, J.; Jiang, Y.; Huang, X. Influence of micro/nano aeration on the diversity of the microbial community in drip irrigation to reduce emitter clogging. Biosyst. Eng. 2023, 235, 116–130. [Google Scholar] [CrossRef]
- Yang, B.; Wang, F.; Wang, J.; Wang, C.; Qiu, X. Numerical simulation and optimisation of the inlet structure of dentiform emitters in drip-irrigation systems. Biosyst. Eng. 2024, 246, 183–190. [Google Scholar] [CrossRef]
- Dallagi, H.; Ait-Mouheb, N.; Soric, A.; Boiron, O. Simulation of the flow characteristics of a labyrinth milli-channel used in drip irrigation. Biosyst. Eng. 2024, 239, 114–129. [Google Scholar] [CrossRef]
- Senyigit, U.; Cruz, R.L.; Rodriguez-Sinobas, L.; Souza de Jesus, W. Changes on emitter discharge under different water temperature and pressure. J. Food Agric. Environ. 2012, 10, 718–720. [Google Scholar]
- Senyigit, U.; Ilkhan, M.S. The effects of water temperature on discharge and uniformity parameters of emitters with different discharges, types and distances. Tarim Bilim. Derg. 2017, 23, 223–233. [Google Scholar]
- Lv, C.; Niu, W.; Du, Y.; Sun, J.; Dong, A.; Wu, M.; Mu, F.; Zhu, J.; Siddique, K.H.M. A meta-analysis of labyrinth channel emitter clogging characteristics under Yellow. River water drip tape irrigation. Agric. Water Manag. 2024, 291, 1–10. [Google Scholar] [CrossRef]
- Li, C.; Li, Z.; Du, P.; Ma, J.; Li, S. Mechanism Analysis of the Influence of Structural Parameters on the Hydraulic Performance of the Novel Y-Shaped Emitter. Agriculture 2023, 13, 1160. [Google Scholar] [CrossRef]
- Li, Y.; Feng, X.; Han, X.; Sun, Y.; Li, H. Machine Learning Approach to Predict Flow Regime Index of a Stellate Water-Retaining Labyrinth Channel Emitter. Agronomy 2023, 13, 1063. [Google Scholar] [CrossRef]
- Li, Y.; Feng, X.; Han, X.; Sun, Y.; Liu, Y.; Yao, M.; Liu, H.; He, Q.; Li, H. Analysis of internal flow characteristics and determination of the core structural parameters of a stellate labyrinth channel irrigation emitter. Biosyst. Eng. 2023, 231, 1–19. [Google Scholar] [CrossRef]
- Qiu, X.; Chen, G.; Wang, H.; Wang, C.; Wang, J. Vertical optimisation of tooth shape to improve the anti-clogging performance of emitters in drip irrigation systems. Biosyst. Eng. 2023, 233, 193–203. [Google Scholar] [CrossRef]
- Li, Y.; Feng, X.; Liu, Y.; Han, X.; Liu, H.; Sun, Y.; Li, H.; Xie, Y. Research on Hydraulic Properties and Energy Dissipation Mechanism of the Novel Water-Retaining Labyrinth Channel Emitters. Agronomy 2022, 12, 1708. [Google Scholar] [CrossRef]
- Seo, B.S.; Lee, S.; Lee, J.H.; Kim, D.S.; Seo, Y.J.; Kim, D.W.; Choi, W. Efficient two-way fluid–structure interaction simulation for performance prediction of pressure-compensating emitter. Biosyst. Eng. 2024, 244, 53–66. [Google Scholar] [CrossRef]
- von Westarp, S.; Chieng, S.; Schreier, H. A comparison between low-cost drip irrigation, conventional drip irrigation, and hand watering in Nepal. Agric. Water Manag. 2004, 64, 143–160. [Google Scholar] [CrossRef]
- Chamba, D.; Zubelzu, S.; Juana, L. Energy, cost and uniformity in the design of drip irrigation systems. Biosyst. Eng. 2019, 178, 200–218. [Google Scholar] [CrossRef]
- ISO 9261; Agricultural Irrigation Equipment, Emitters and Emitting Pipe, Specification and Test Methods. ISO: Geneva, Switzerland, 2004.
- ANSYS Inc. ANSYS 2024 User Guide; ANSYS Inc.: Canonsburg, PA, USA, 2024; Available online: https://www.ansys.com (accessed on 24 February 2025).
- Li, Y.; Yang, P.; Xu, T.; Ren, S.; Lin, X.; Wei, R.; Xu, H. CFD and digital particle tracking to assess flow characteristics in the labyrinth flow path of a drip irrigation emitter. Irrig. Sci. 2008, 26, 427–438. [Google Scholar] [CrossRef]

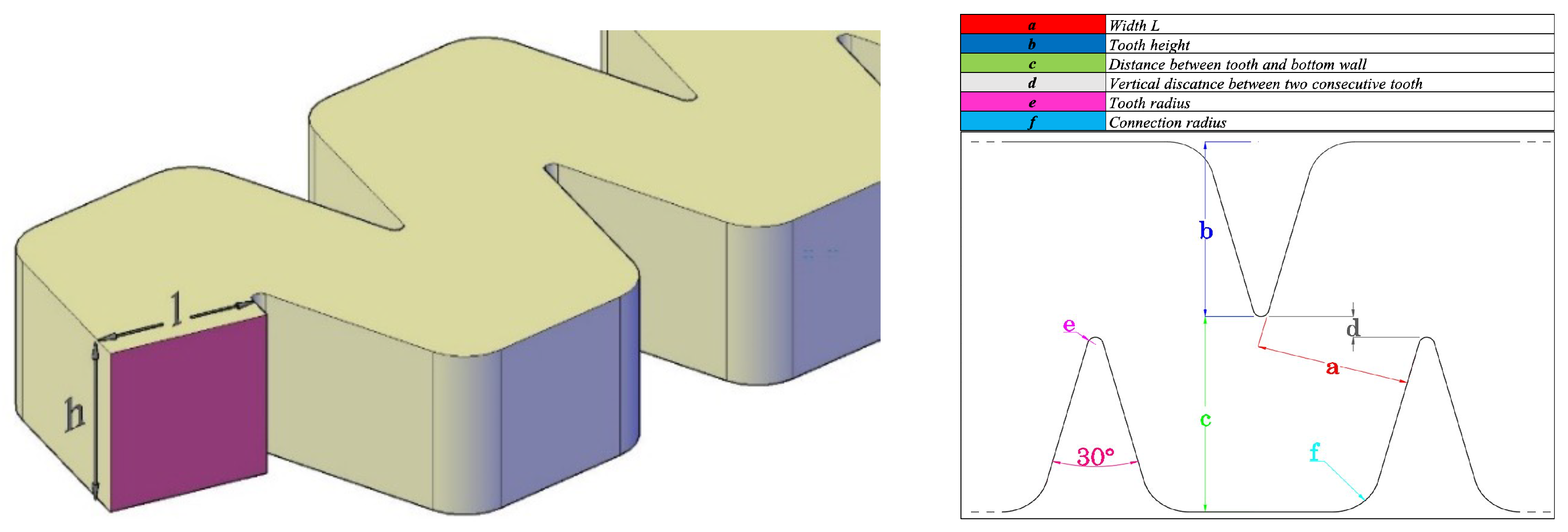


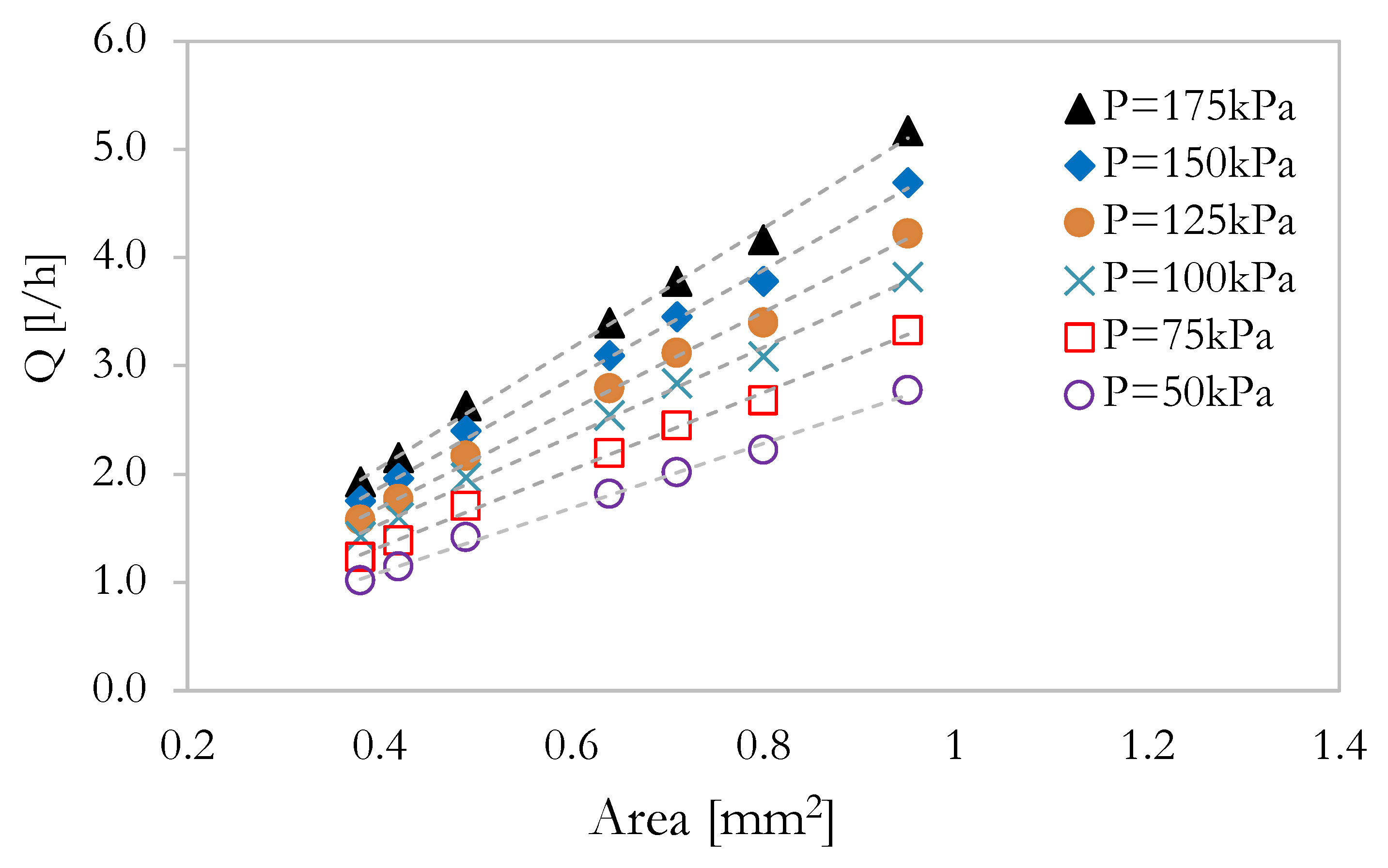

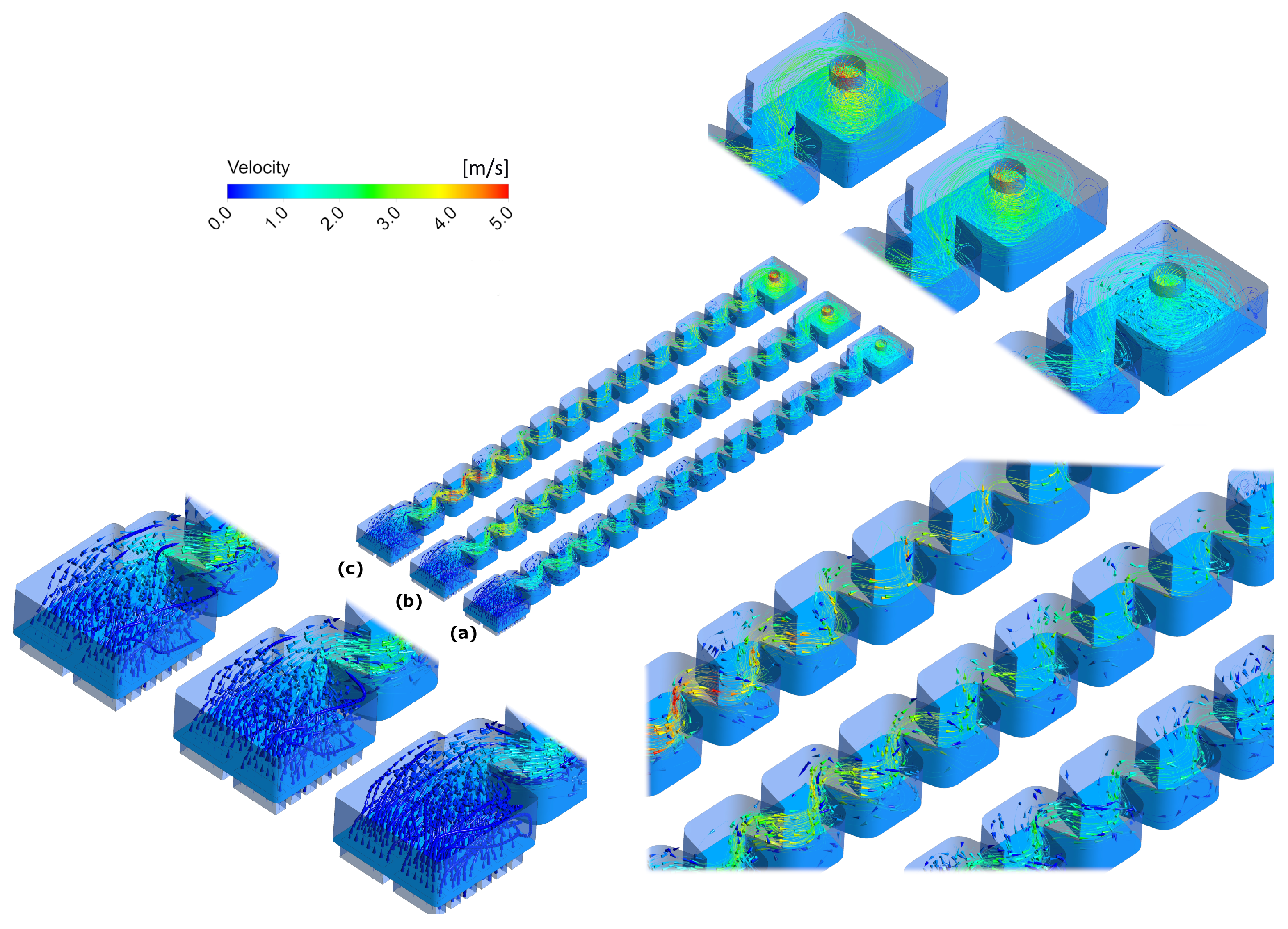
| Case | Width L [mm] | Height h [mm] | Area A [mm2] |
|---|---|---|---|
| 1 | 0.63 | 0.60 | 0.378 |
| 2 | 0.65 | 0.65 | 0.422 |
| 3 | 0.70 | 0.70 | 0.490 |
| 4 | 0.80 | 0.80 | 0.640 |
| 5 | 0.84 | 0.85 | 0.714 |
| 6 | 0.89 | 0.90 | 0.801 |
| 7 | 1.00 | 0.95 | 0.950 |
| Pressure [kPa] | ||||||
|---|---|---|---|---|---|---|
| 50 | 75 | 100 | 125 | 150 | 175 | |
| Case | Flow Rate q [L/h] | |||||
| 1 | 1.0100 | 1.2271 | 1.4240 | 1.5728 | 1.7500 | 1.9272 |
| 2 | 1.1420 | 1.3829 | 1.6010 | 1.7625 | 1.9560 | 2.1495 |
| 3 | 1.4160 | 1.7047 | 1.9650 | 2.1609 | 2.3960 | 2.6312 |
| 4 | 1.8120 | 2.1926 | 2.5370 | 2.7886 | 3.0910 | 3.3934 |
| 5 | 2.0130 | 2.4441 | 2.8350 | 3.1132 | 3.4480 | 3.7829 |
| 6 | 2.2200 | 2.6722 | 3.0800 | 3.3992 | 3.7800 | 4.1608 |
| 7 | 2.7740 | 3.3262 | 3.8230 | 4.2203 | 4.6940 | 5.1677 |
| Case | x | k |
|---|---|---|
| 1 | 0.4990 | 0.1450 |
| 2 | 0.4986 | 0.1613 |
| 3 | 0.4945 | 0.2079 |
| 4 | 0.4883 | 0.2601 |
| 5 | 0.4972 | 0.2864 |
| 6 | 0.4952 | 0.3166 |
| 7 | 0.4904 | 0.4025 |
| Case | ||
|---|---|---|
| 1 | 5.54 | 0.158 |
| 2 | 5.03 | 0.139 |
| 3 | 4.52 | 0.119 |
| 4 | 4.09 | 0.102 |
| 5 | 3.57 | 0.104 |
| 6 | 2.98 | 0.105 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
De Marchis, M.; Bruno, F.; Saccone, D.; Napoli, E. Performance of Emitters in Drip Irrigation Systems Using Computational Fluid Dynamic Analysis. Water 2025, 17, 689. https://doi.org/10.3390/w17050689
De Marchis M, Bruno F, Saccone D, Napoli E. Performance of Emitters in Drip Irrigation Systems Using Computational Fluid Dynamic Analysis. Water. 2025; 17(5):689. https://doi.org/10.3390/w17050689
Chicago/Turabian StyleDe Marchis, Mauro, Federica Bruno, Domenico Saccone, and Enrico Napoli. 2025. "Performance of Emitters in Drip Irrigation Systems Using Computational Fluid Dynamic Analysis" Water 17, no. 5: 689. https://doi.org/10.3390/w17050689
APA StyleDe Marchis, M., Bruno, F., Saccone, D., & Napoli, E. (2025). Performance of Emitters in Drip Irrigation Systems Using Computational Fluid Dynamic Analysis. Water, 17(5), 689. https://doi.org/10.3390/w17050689







