Global Sensitivity Analysis of Slope Stability Considering Effective Rainfall with Analytical Solutions
Abstract
1. Introduction
2. Theoretical Background and Methodology
2.1. One-Dimensional Richards Equation
2.2. Analytical Solution of Effective Rainfall
2.3. Slope Stability with Effective Rainfall
2.3.1. Shear Strength of Unsaturated Soil
2.3.2. Shear Strength of Soil in the Transient Saturated Zone
2.3.3. Slope Stability
2.4. Global Sensitivity Analysis
2.4.1. Sobol Method
2.4.2. DT Method
2.4.3. RS-HDMR Method
3. Illustrative Examples
4. Results and Discussion
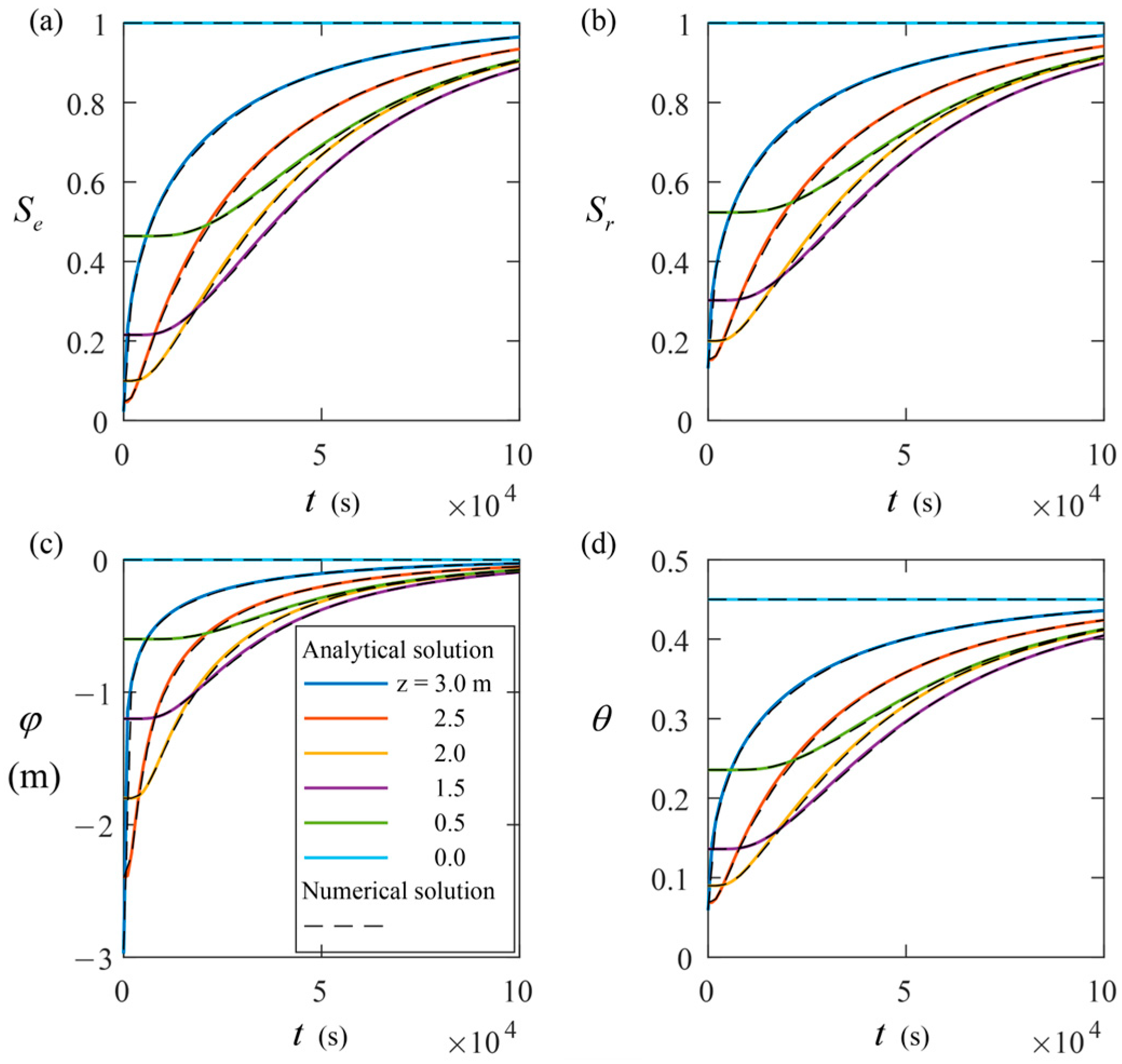
4.1. Analytical and Numerical Solutions
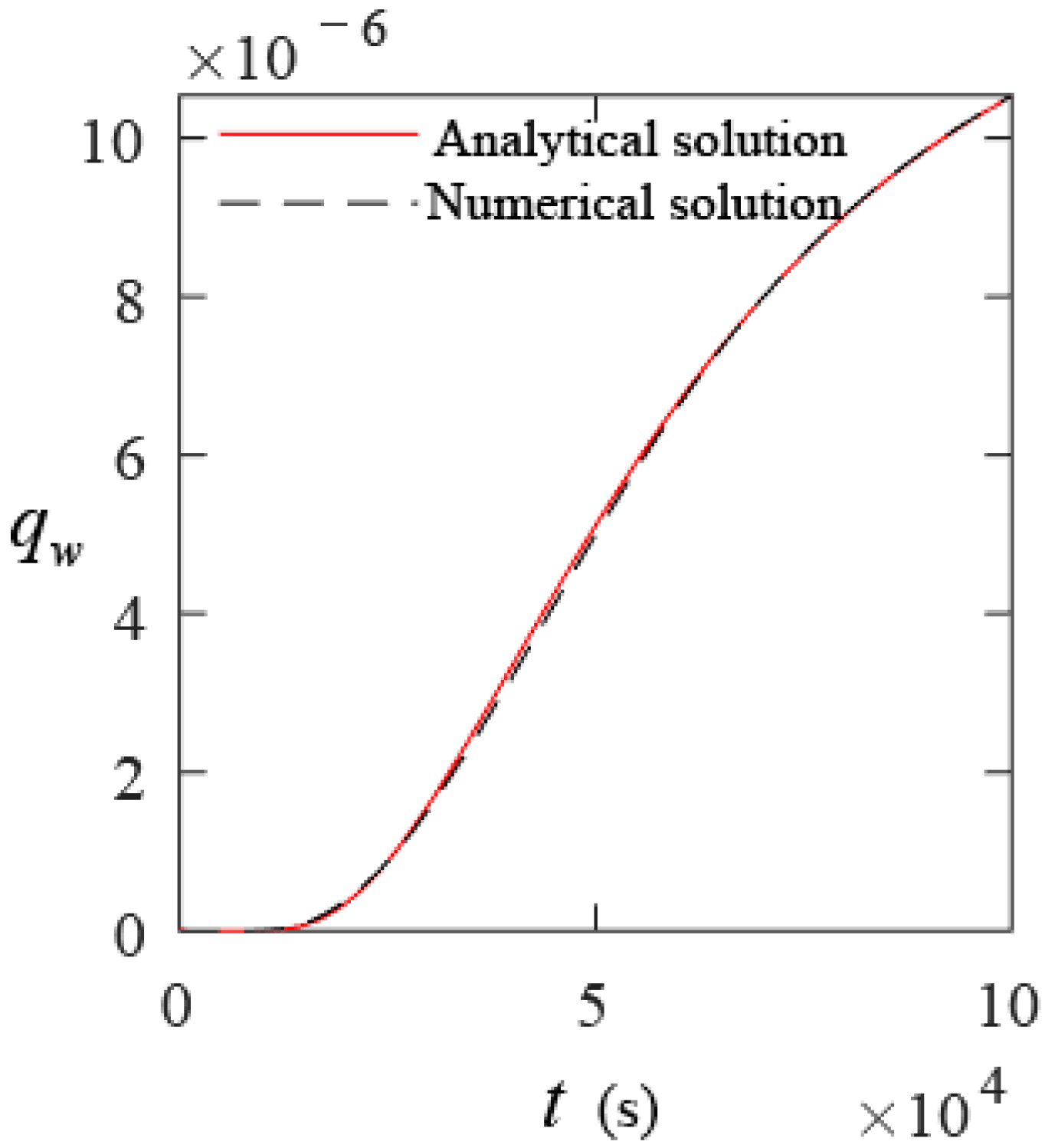
4.2. Temporal Evolution of Effective Rainfall
4.3. Spatiotemporal Distribution of Shear Strength
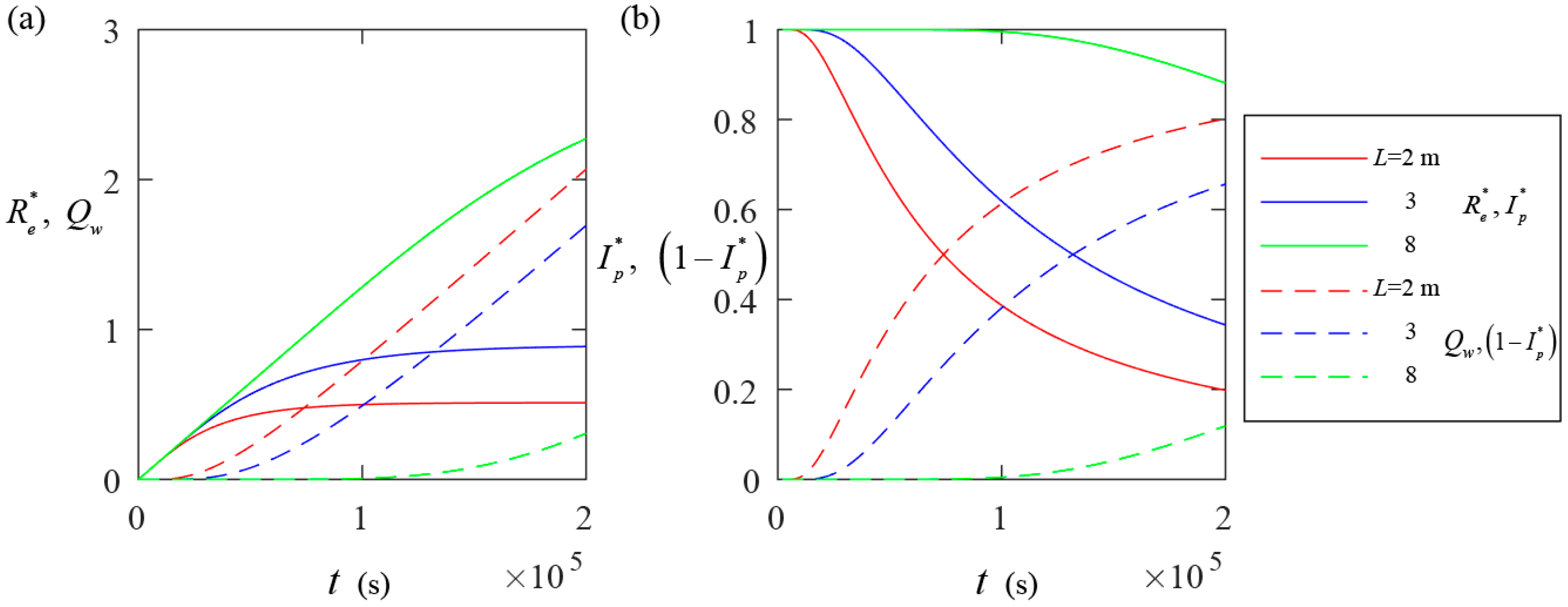
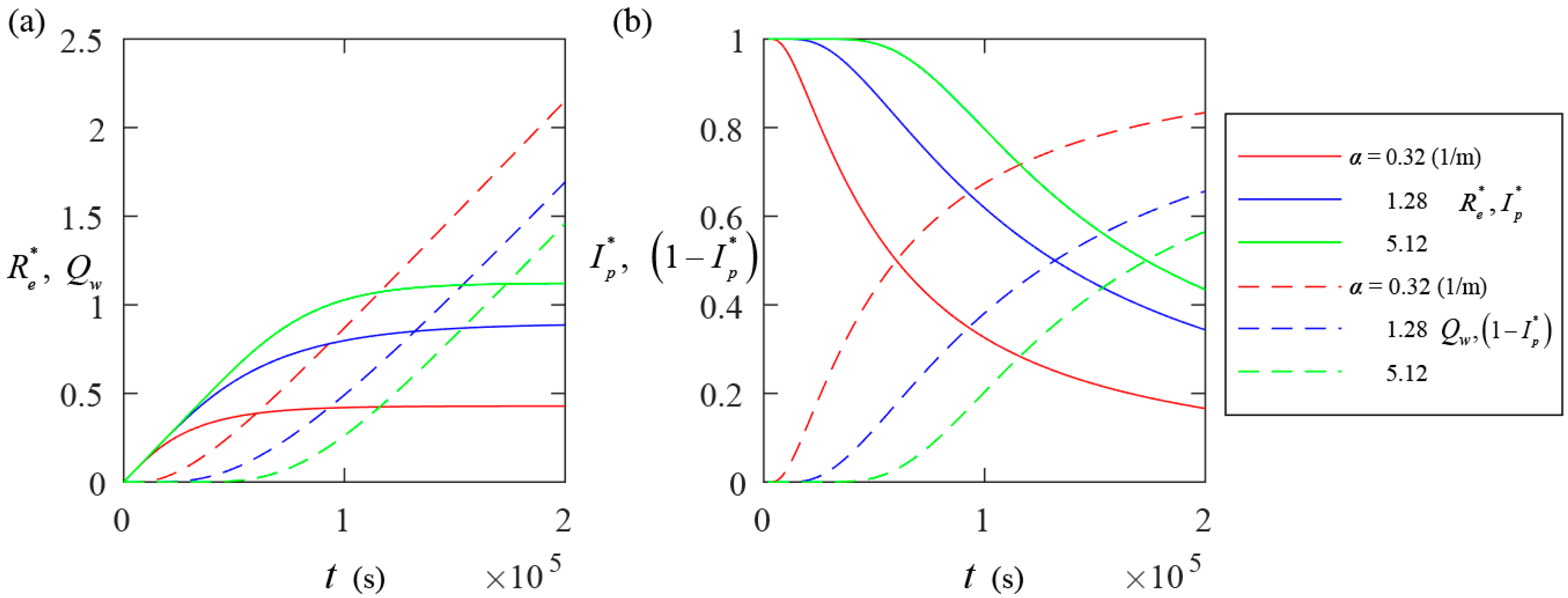
4.4. Sensitivity of Slope Stability
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Rabie, M. Comparison study between traditional and finite element methods for slopes under heavy rainfall. HBRC J. 2014, 10, 160–168. [Google Scholar] [CrossRef]
- Jian, W.B.; Hu, H.R.; Luo, Y.H.; Tang, W.Y. Experimental study on deterioration of granitic residual soil strength in wetting-drying cycles. J. Eng. Geol. 2017, 25, 592–597. [Google Scholar] [CrossRef]
- Zeng, L.; Shi, Z.N.; Fu, H.Y.; Bian, H.B. Influence of rainfall infiltration on distribution characteristics of slope transient saturated zone. China J. Highw. Transp. 2017, 30, 25–34. [Google Scholar] [CrossRef]
- Qiu, X.; Jiang, H.B.; Ou, J.; Liu, Z.W.; Chen, M. Stability analysis method of “transient” saturated-unsaturated slope. China J. Highw. Transp. 2020, 33, 63–75. [Google Scholar] [CrossRef]
- Peiffer, L.; Inguaggiato, C.; Wurl, J.; Fletcher, J.M.; Martínez, M.G.O.; Martínez, D.C.; Legrand, D.; Morales, P.H.; Reinoza, C.E.; Tchamabé, B.C.; et al. Geochemistry of coastal geothermal systems from southern Baja California peninsula (Mexico): Fluid origins, water-rock interaction and tectonics. Chem. Geol. 2024, 670, 122316. [Google Scholar] [CrossRef]
- Shu, B.; Chen, J.J.; Xue, H. Experimental study of the change of pore structure and strength of granite after fluid-rock interaction in CO2-EGS. Renew. Energy 2024, 220, 119635. [Google Scholar] [CrossRef]
- Abd, A.I.; Fattah, Y.M.; Mekkiyah, H. Relationship between the Matric Suction and the Shear Strength in Unsaturated Soil. Case Stud. Constr. Mater. 2020, 13, e00441. [Google Scholar] [CrossRef]
- Antonello, T.; Luigi, P.; Enrico, C. Rainfall Threshold for Shallow Landslide Triggering Due to Rising Water Table. Water 2022, 14, 2966. [Google Scholar] [CrossRef]
- Duc, B.T.; Ha, N.D.; Kim, N.T.; Hong, L.L.; Osamu, W.; Kazunori, H.; Akihiko, W.; Shinro, A. Geometry and the Mechanism of Landslide Occurrence in a Limestone Area–Case Examples of Landslides in Vietnam and from Europe, China, and Japan. J. Disaster Res. 2021, 16, 646–657. [Google Scholar] [CrossRef]
- Qiu, X.; Jiang, H.B.; Ou, J.; Fu, H.Y.; Ma, J.Q. Numerical analysis of formation conditions and evolution characteristics of transient saturation zone of a slope under rainfall conditions. Shuili Xuebao 2020, 51, 1525–1535. [Google Scholar] [CrossRef]
- Zivaljevic, S.; Tomanovic, Z.; Radulovic, M. Analysis of the triggering mechanism of landslide in the village Podi, Montenegro. Arab. J. Geosci. 2021, 14, 56. [Google Scholar] [CrossRef]
- Zeng, L.; Bian, H.B.; Shi, Z.N.; He, Z.M. Forming condition of transient saturated zone and its distribution in residual slope under rainfall conditions. J. Cent. South Univ. 2017, 24, 1866–1880. [Google Scholar] [CrossRef]
- Miller, C.J.; Yesiller, N.; Yaldo, K.; Merayyan, S. Impact of soil type and compaction conditions on soil water characteristic. J. Geotech. Geoenviron. Eng. 2002, 128, 733–742. [Google Scholar] [CrossRef]
- Dou, H.Q.; Han, T.C.; Gong, X.N.; Zhang, J. Probabilistic slope stability analysis considering the variability of hydraulic conductivity under rainfall infiltration–redistribution conditions. Eng. Geol. 2014, 183, 1–13. [Google Scholar] [CrossRef]
- Jian, W.B.; Huang, C.H.; Luo, Y.H.; Nie, W. Experimental study on wetting front migration induced by rainfall infiltration in unsaturated eluvial and residual soil. Rock Soil Mech. 2020, 41, 1123–1133. [Google Scholar] [CrossRef]
- Tang, D.; Jiang, Z.M.; Yuan, T.; Li, Y. Stability analysis of soil slope subjected to perched water condiiton. KSCE J. Civ. Eng. 2020, 24, 2581–2590. [Google Scholar] [CrossRef]
- Qiu, X.; Li, J.H.; Jiang, H.B.; Ou, J.; Ma, J.Q. Evolution of the Transient Saturated Zone and Stability Analysis of Slopes under Rainfall Conditions. KSCE J. Civ. Eng. 2022, 26, 1618–1631. [Google Scholar] [CrossRef]
- Crozier, M.J. Landslides: Causes, Consequences & Environment; Croom Helm: London, UK, 1997. [Google Scholar]
- Kim, I.M.; Lee, J.S. An Analysis of Landslide Risk Using the Change in the Volumetric Water Content Gradient in the Soil Layer Per Unit Time of Effective Cumulative Rainfall. Water 2023, 15, 1699. [Google Scholar] [CrossRef]
- Lee, E.M. A Statistical Analysis of Long-term trends in UK Effective Rainfall: Implications for Deep-seated Landsliding. Q. J. Eng. Geol. Hydrogeol. 2020, 53, 587–597. [Google Scholar] [CrossRef]
- Jian, W.B.; Xu, X.T.; Zheng, M.Z.; Liu, K.; Lai, S.Q. On effective rainfall of slope instability. Rock Soil Mech. 2013, 34, 247–251. [Google Scholar] [CrossRef]
- Shakoor, A.; Smithmyer, A.J. An analysis of storm-induced landslides in colluvial soils overlying mudrock sequences, southeastern Ohio, USA. Eng. Geol. 2005, 78, 257–274. [Google Scholar] [CrossRef]
- Ahmed, A.; Ugai, K.; Yang, Q.Q. Assessment of 3D Slope Stability Analysis Methods Based on 3D Simplified Janbu and Hovland Methods. Int. J. Geomech. 2012, 12, 81–89. [Google Scholar] [CrossRef]
- Yang, H.X.; Guo, N.; Lu, F.; Zhou, S.K. Slope Stability Analysis and Sliding Surface Search Model Based on Swedish Slice Method. Soil Mech. Found. Eng. 2024, 61, 342–350. [Google Scholar] [CrossRef]
- Gardner, W.R. Some steady-state solutions of the unsaturated moisture flow equation with application to evaporation from a water table. Soil Sci. 1958, 85, 228–232. [Google Scholar] [CrossRef]
- Srivastava, R.; Yeh, T.C.J. Analytical solutions for one-dimensional transient infiltration toward the water-table in homogeneous and layered soils. Water Resour. Res. 1991, 27, 753–762. [Google Scholar] [CrossRef]
- Fredlund, D.G.; Morgenstern, N.R.; Widger, R.A. The shear strength of unsaturated soils. Can. Geotech. J. 1978, 15, 313–321. [Google Scholar] [CrossRef]
- Sobol, I.M. Global sensitivity indices for nonlinear mathematical models and their Monte Carlo estimates. Math. Comput. Simul. 2001, 55, 271–280. [Google Scholar] [CrossRef]
- Xia, C.A.; Tong, J.X.; Hu, B.X.; Wu, X.J.; Alberto, G. Assessment of alternative adsorption models and global sensitivity analysis to characterize hexavalent chromium loss from soil to surface runoff. Hydrol. Process. 2018, 32, 3140–3157. [Google Scholar] [CrossRef]
- Borgonovo, E. A new uncertainty importance measure. Reliab. Eng. Syst. Saf. 2006, 92, 771–784. [Google Scholar] [CrossRef]
- Li, G.Y.; Rabitz, H.; Yelvington, P.E.; Oluwole, O.O.; Bacon, F.; Kolb, C.E.; Schoendorf, J. Global sensitivity analysis for systems with independent and/or correlated inputs. J. Phys. Chem. A 2010, 114, 6022–6032. [Google Scholar] [CrossRef] [PubMed]
- Ho, D.Y.F.; Fredlund, D.G. Increase in strength due to suction for two HONG-KONG soils. In Proceedings of the ASCE Geotechnical Conference on Engineering and Construction in Tropical and Residual Soils, Honolulu, HI, USA, 11–15 January 1982. [Google Scholar]
- Yang, D.S. Study on Strength Attenuation Mechanism of Granite Residual Soil Under Rainfall Condition—A Case Study of Xiyanshan Granite in Pucheng County, Fujian Province. Master’s Thesis, Chengdu University of Technology, Chengdu, China, 2023. [Google Scholar] [CrossRef]
- Wang, W.W. Strength Characteristics and Engineering Application of Unsaturated Granite Residual Soil. Master’s Thesis, Fuzhou University, Fuzhou, China, 2022. [Google Scholar] [CrossRef]
- Zhou, X.W.; Luo, X.C. Identification and physical mechanical property comparison between completely decomposed granite and granite residual soil. J. Yangtze River Sci. Res. Inst. 2022, 39, 1–7. [Google Scholar] [CrossRef]
- Jamaluddin, D.; Nujid, M.M.; Ahmad, A.; Sadikon, S.F.; Ahmad, F. Shear strength parameters of granitic and sedimentary residual soils from the Northern and the Peninsular Malaysia. IOP Conf. Ser. Earth Environ. Sci. 2021, 920, 012016. [Google Scholar] [CrossRef]
- Bai, H.L.; Feng, W.K.; Li, S.Q.; Ye, L.Z.; Wu, Z.T.; Hu, R.; Dai, H.C.; Hu, Y.P.; Yi, X.Y.; Deng, P.C. Flow-slide characteristics and failure mechanism of shallow landslides in granite residual soil under heavy rainfall. J. Mt. Sci. 2022, 19, 1541–1557. [Google Scholar] [CrossRef]
- Tsutsumi, D.; Fujita, M. Field observations, experiments, and modeling of sediment production from freeze and thaw action on a bare, weathered granite slope in a temperate region of Japan. Geomorphology 2016, 267, 37–47. [Google Scholar] [CrossRef]
- Yamakawa, Y.; Kosugi, K.; Masaoka, N.; Sumida, J.; Tani, M.; Mizuyama, T. Estimation of Soil Thickness Distribution on a Granitic Hillslope using Electrical Resistivity Method. Int. J. Eros. Control Eng. 2010, 3, 20–26. [Google Scholar] [CrossRef]
- Dou, H.Q.; Xie, S.H.; Chen, F.; Wang, H.; Chen, F.Q.; Jian, W.B. Study on shear characteristics and a mechanics model of granite residual soil–rock interface. Bull. Eng. Geol. Environ. 2023, 82, 212. [Google Scholar] [CrossRef]
- Ohtsu, H.; Masuda, H.; Kitaoka, T.; Takahashi, K.; Yabe, M.; Soralump, S.; Maeda, Y. A Simulation of Surface Runoff and Infiltration due to Torrential Rainfall Based on Field Monitoring Results at a Slope Comprising Weathered Granite. Geotech. Eng. J. SEAGS AGSSEA 2015, 46, 12–21. [Google Scholar] [CrossRef]
- Pooya, S.; Md, J.M.N.; Yasmin, A.; Afshin, A. Shear Strength of Unsaturated Malaysian Granitic Residual Soil. J. Test. Eval. 2019, 47, 20170305. [Google Scholar] [CrossRef]
- Xu, J.; Ni, Y. Prediction of grey-catastrophe destabilization time of a granite residual soil slope under rainfall. Bull. Eng. Geol. Environ. 2019, 78, 5687–5693. [Google Scholar] [CrossRef]
- Geng, J.S.; Sun, Q.; Zhang, Y.C.; Yan, C.G.; Zhang, W.Q. Electric-field response based experimental investigation of unsaturated soil slope seepage. J. Appl. Geophys. 2017, 138, 154–160. [Google Scholar] [CrossRef]
- Li, T.; Liu, G.D.; Wang, C.; Wang, X.W.; Li, Y. The Probability and Sensitivity Analysis of Slope Stability Under Seepage Based on Reliability Theory. Geotech. Geol. Eng. 2020, 38, 3469–3479. [Google Scholar] [CrossRef]
- Liang, J.X.; Sui, W.H. Sensitivity Analysis of Anchored Slopes under Water Level Fluctuations: A Case Study of Cangjiang Bridge—Yingpan Slope in China. Appl. Sci. 2021, 11, 7137. [Google Scholar] [CrossRef]
- Lin, H.J.; Li, L.; Qiang, Y.; Zhang, Y.; Liang, S.Y. Sensitivity analysis of slope stability based on eXtreme gradient boosting and SHapley Additive exPlanations: An exploratory study. Heliyon 2024, 10, e35871. [Google Scholar] [CrossRef]
- Lei, D.X.; Zhang, Y.P.; Lu, Z.G.; Lin, H.; Fang, B.W. Slope Stability Prediction Using Principal Component Analysis and Hybrid Machine Learning Approaches. Appl. Sci. 2024, 14, 6526. [Google Scholar] [CrossRef]

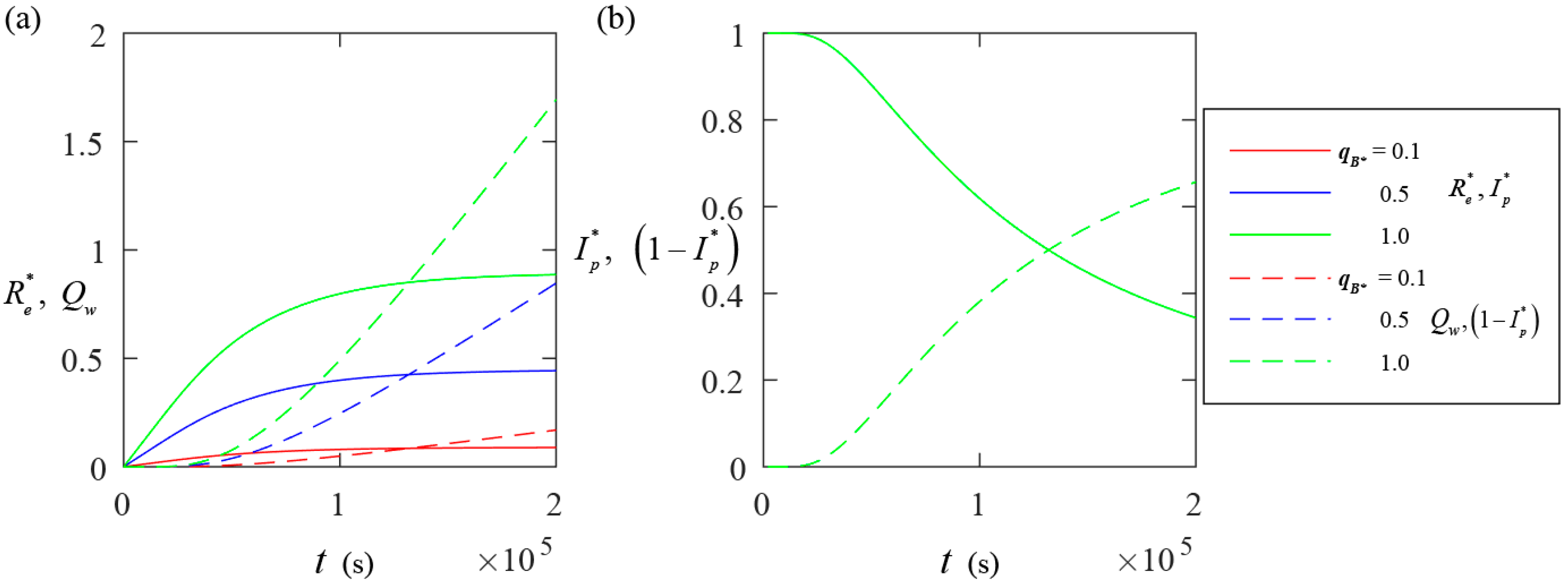


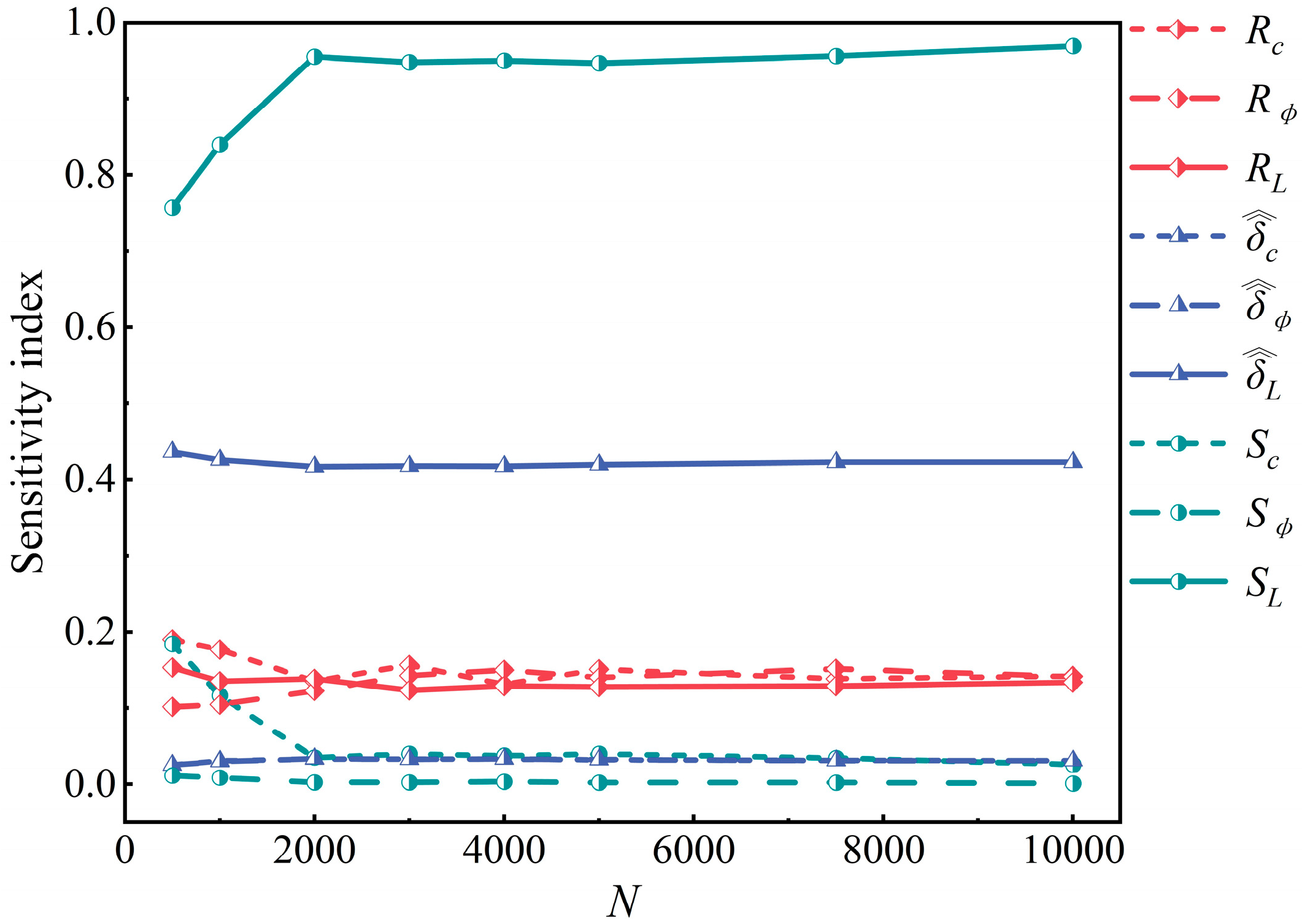

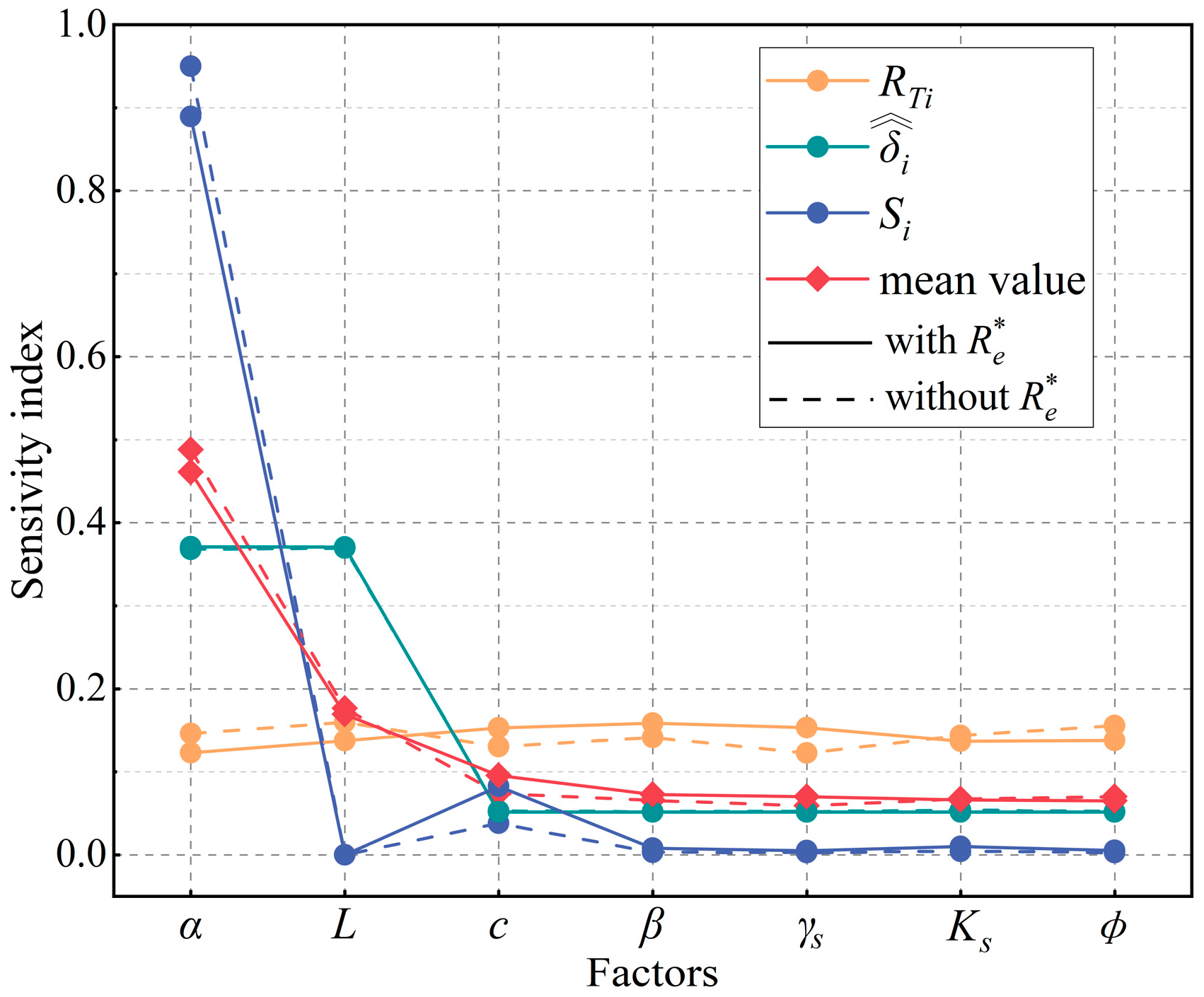
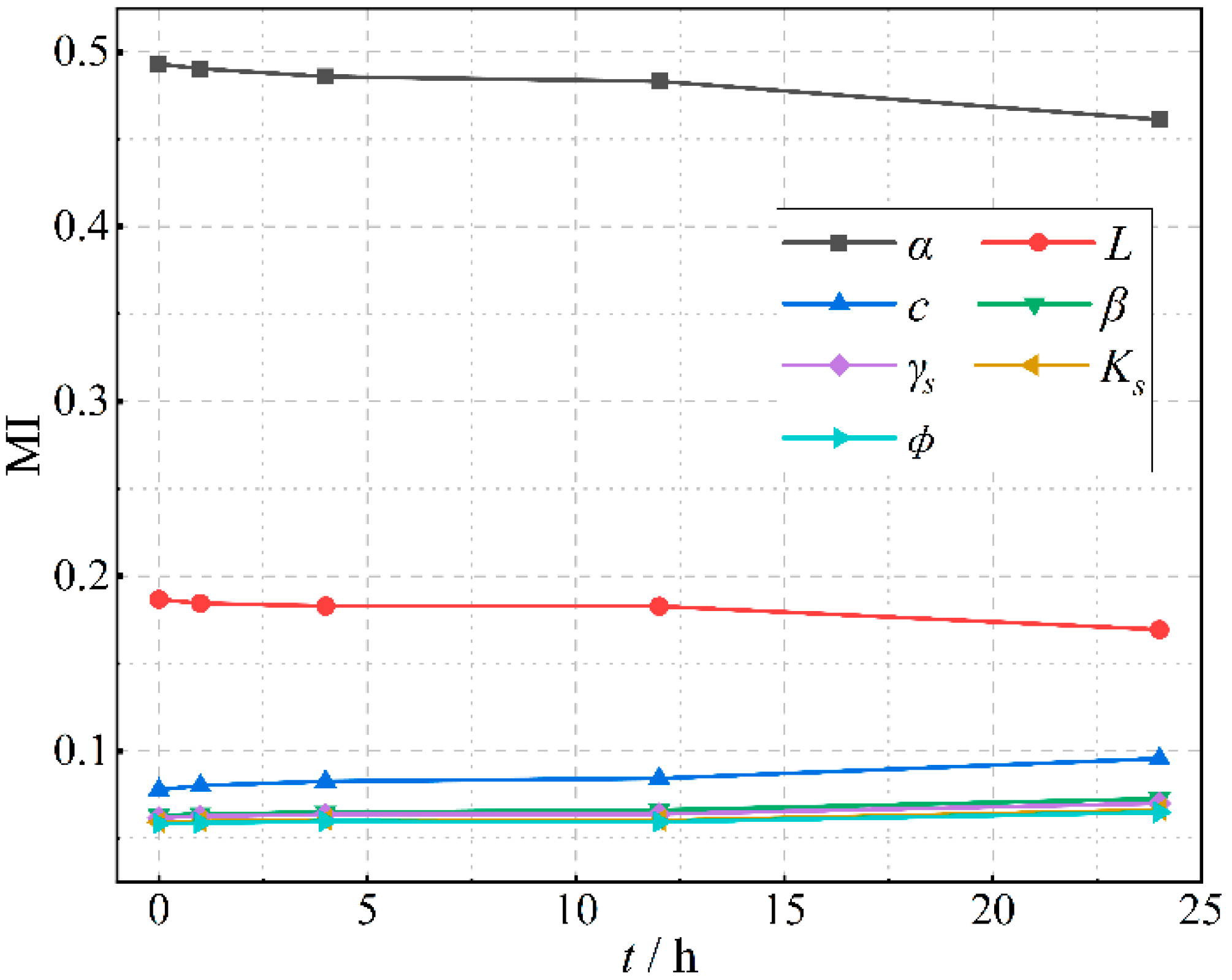
| Parameter | Value | Unit |
|---|---|---|
| c | 28.9 | kPa |
| ϕ | 33.4 | ° |
| ϕb | 15.3 | ° |
| β | 30 | ° |
| γs | 24.89 | kN/m3 |
| Ks | 1.29 × 10−5 | m/d |
| qB* | 0.1, 0.5, 1.0 | mm/d |
| α | 0.32, 1.28, 5.12 | L/m |
| L | 2, 3, 8 | m |
| θs | 0.45 | - |
| θr | 0.05 | - |
| Factor | Distribution | Shape Parameters for PDF | Unit | References | |
|---|---|---|---|---|---|
| c | lognormal | υ, σ | 25.000, 3.333 | kPa | [36] |
| ϕ | 25.000, 1.667 | ° | [2] | ||
| β | 40.000, 3.333 | ° | [37,38] | ||
| γs | 19.000, 0.333 | kN/m3 | [39,40] | ||
| Ks | 1.296, 0.215 | m/d | [37,41] | ||
| α | uniform | , | 0.320, 5.120 | L/m | [42] |
| L | 0.500, 8.000 | m | [37,43] | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xia, C.-A.; Zhang, J.-Q.; Wang, H.; Jian, W.-B. Global Sensitivity Analysis of Slope Stability Considering Effective Rainfall with Analytical Solutions. Water 2025, 17, 141. https://doi.org/10.3390/w17020141
Xia C-A, Zhang J-Q, Wang H, Jian W-B. Global Sensitivity Analysis of Slope Stability Considering Effective Rainfall with Analytical Solutions. Water. 2025; 17(2):141. https://doi.org/10.3390/w17020141
Chicago/Turabian StyleXia, Chuan-An, Jing-Quan Zhang, Hao Wang, and Wen-Bin Jian. 2025. "Global Sensitivity Analysis of Slope Stability Considering Effective Rainfall with Analytical Solutions" Water 17, no. 2: 141. https://doi.org/10.3390/w17020141
APA StyleXia, C.-A., Zhang, J.-Q., Wang, H., & Jian, W.-B. (2025). Global Sensitivity Analysis of Slope Stability Considering Effective Rainfall with Analytical Solutions. Water, 17(2), 141. https://doi.org/10.3390/w17020141







