Designing the Spigot Structure of Hydrocyclones to Reduce Fine Particle Misplacement in Underflow
Abstract
1. Introduction
2. Numerical Methods
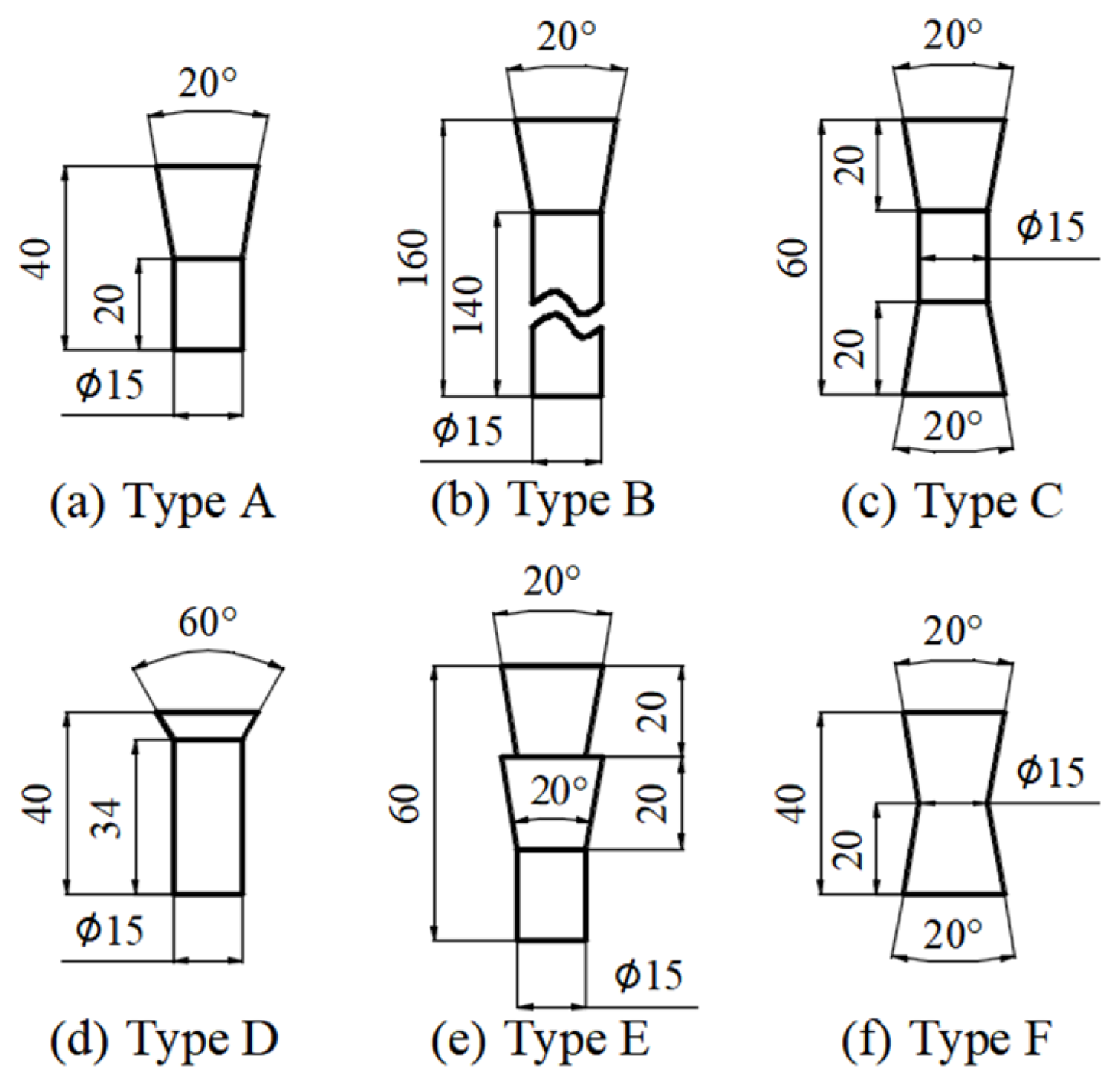
2.1. Geometry and Meshes
2.2. Model Description
2.2.1. Turbulence Model
2.2.2. Multiphase Flow Model
2.3. Simulation Conditions
3. Results and Discussion
3.1. Flow Pattern
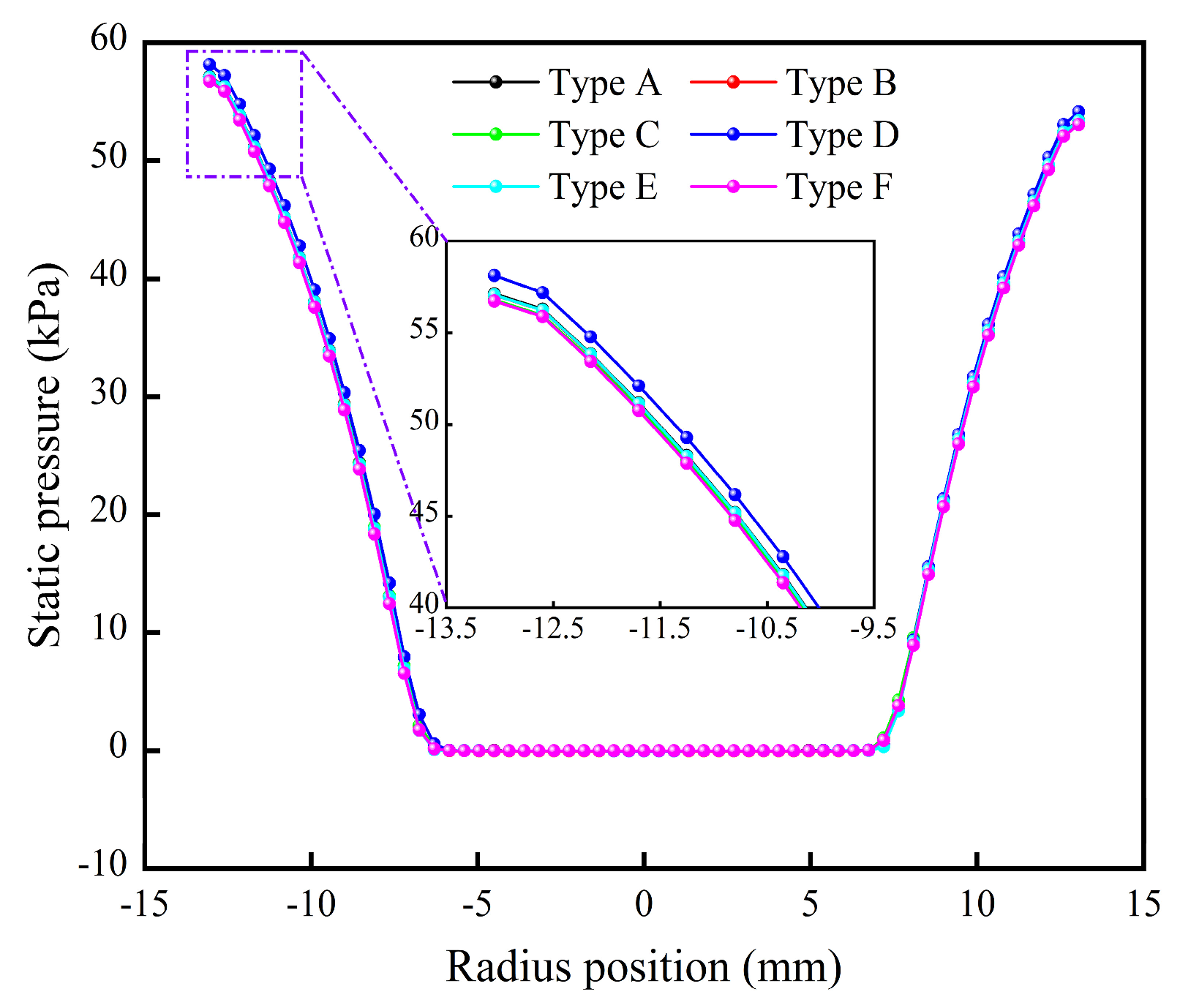
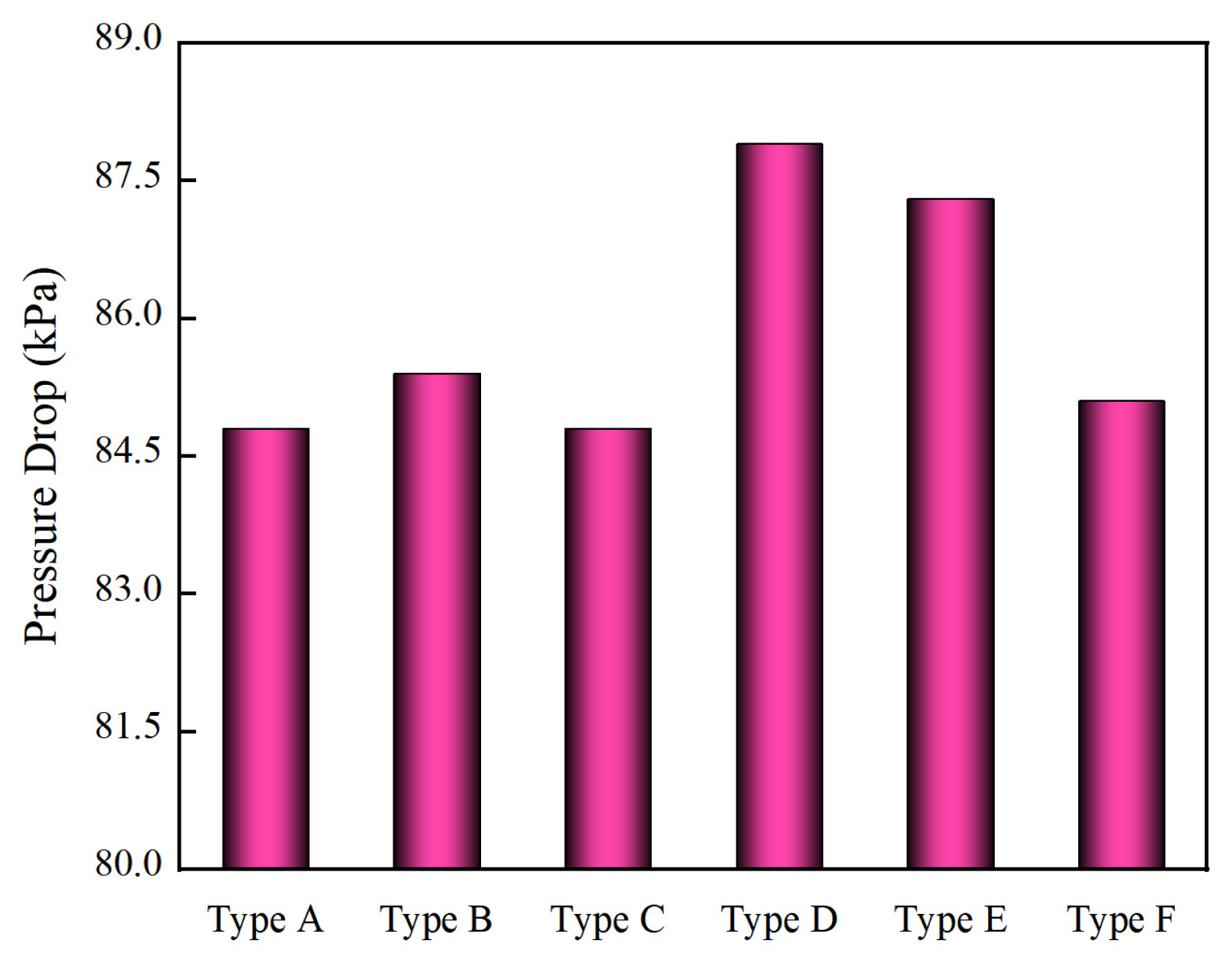
3.1.1. Static Pressure
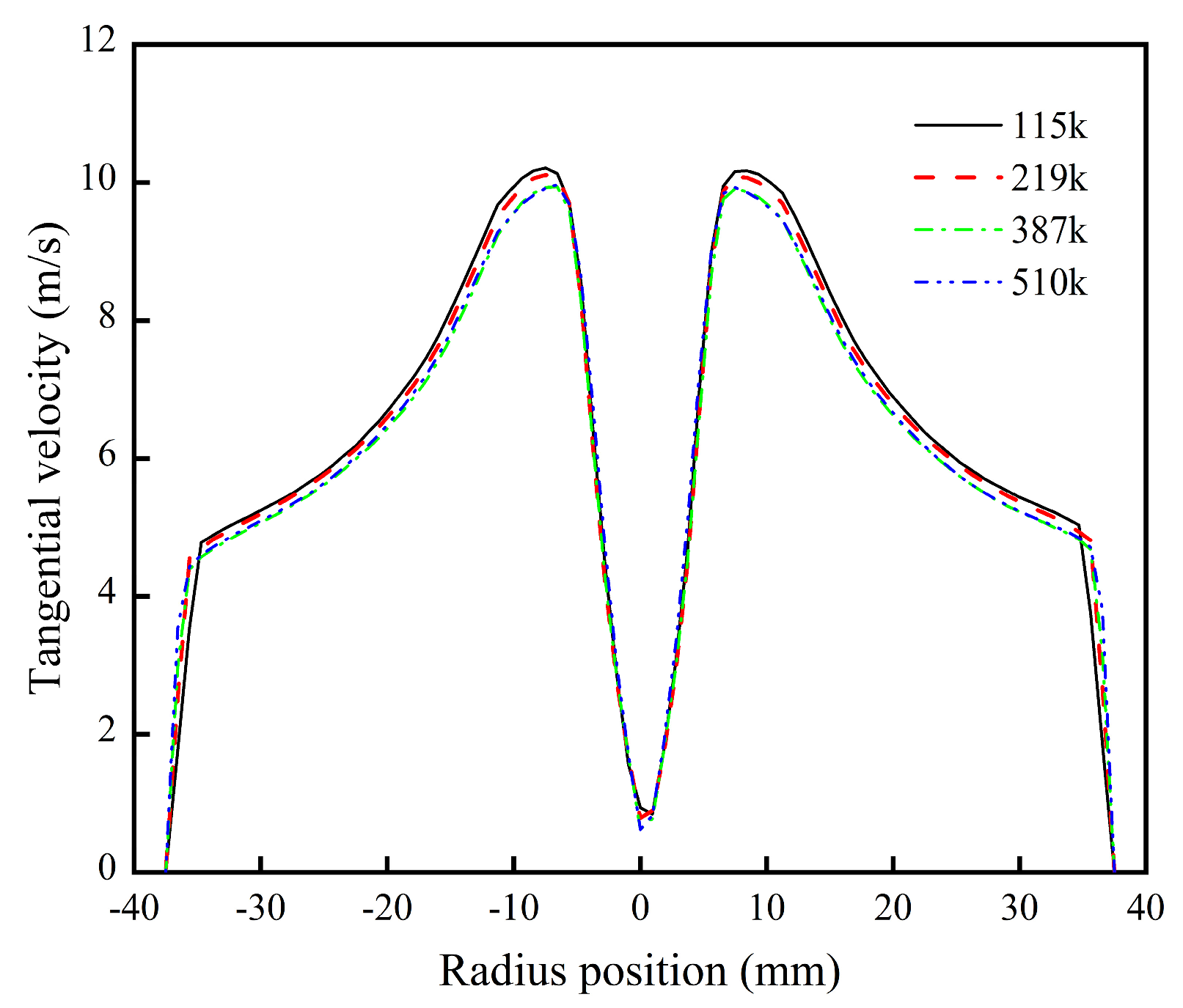
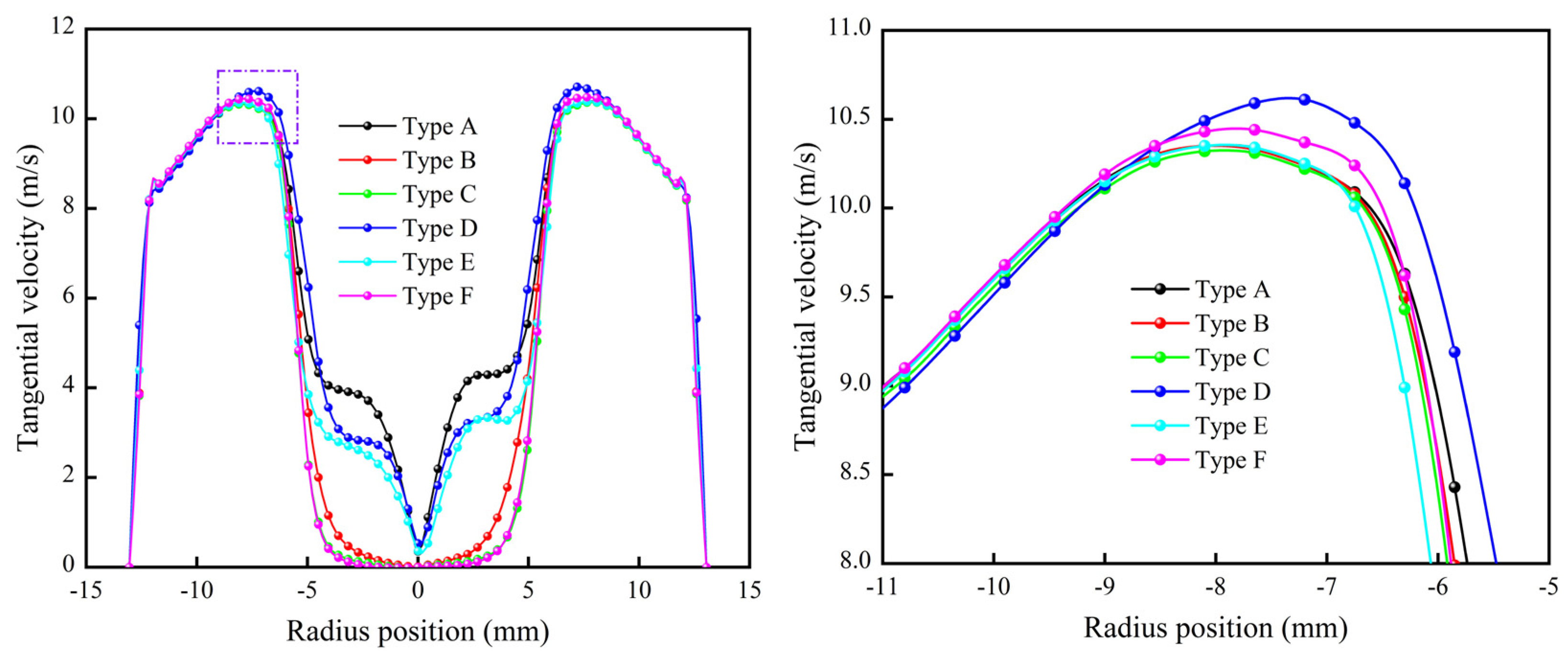
3.1.2. Tangential Velocity
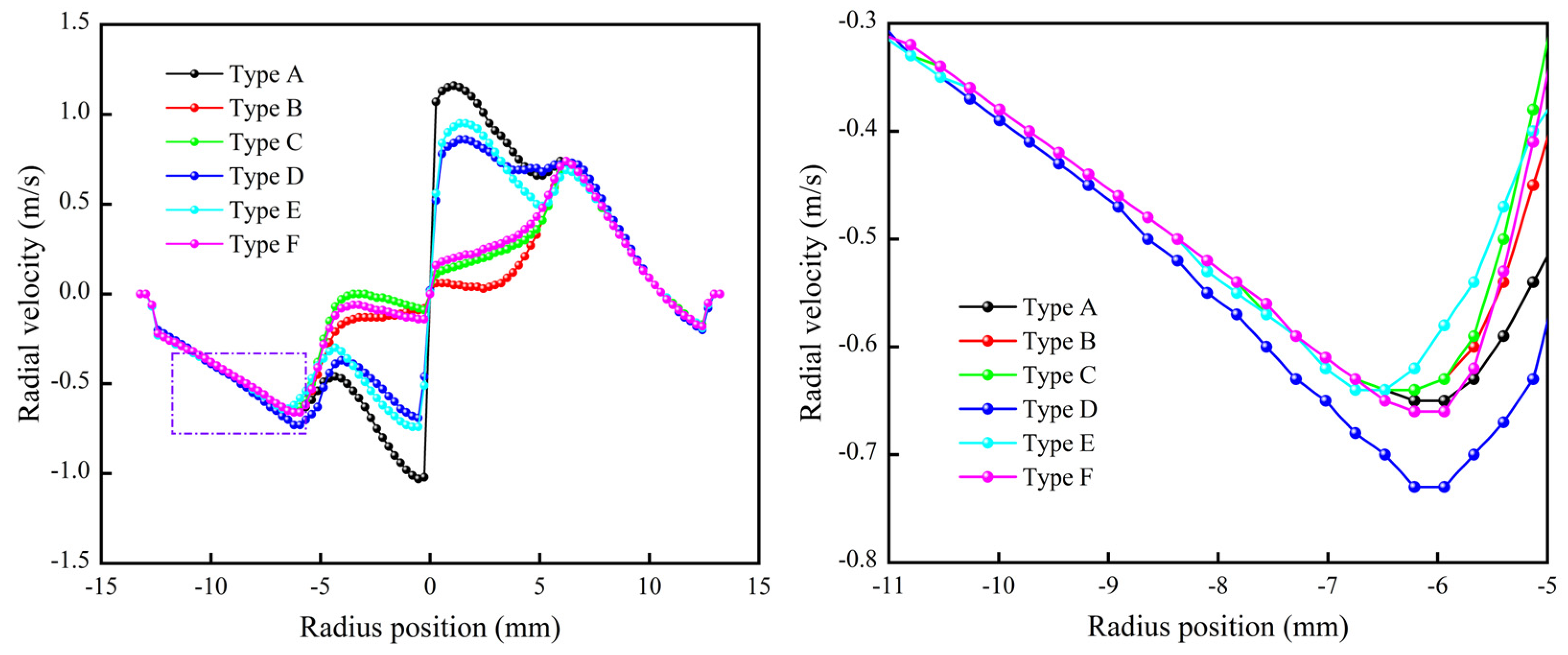
3.1.3. Radial Velocity
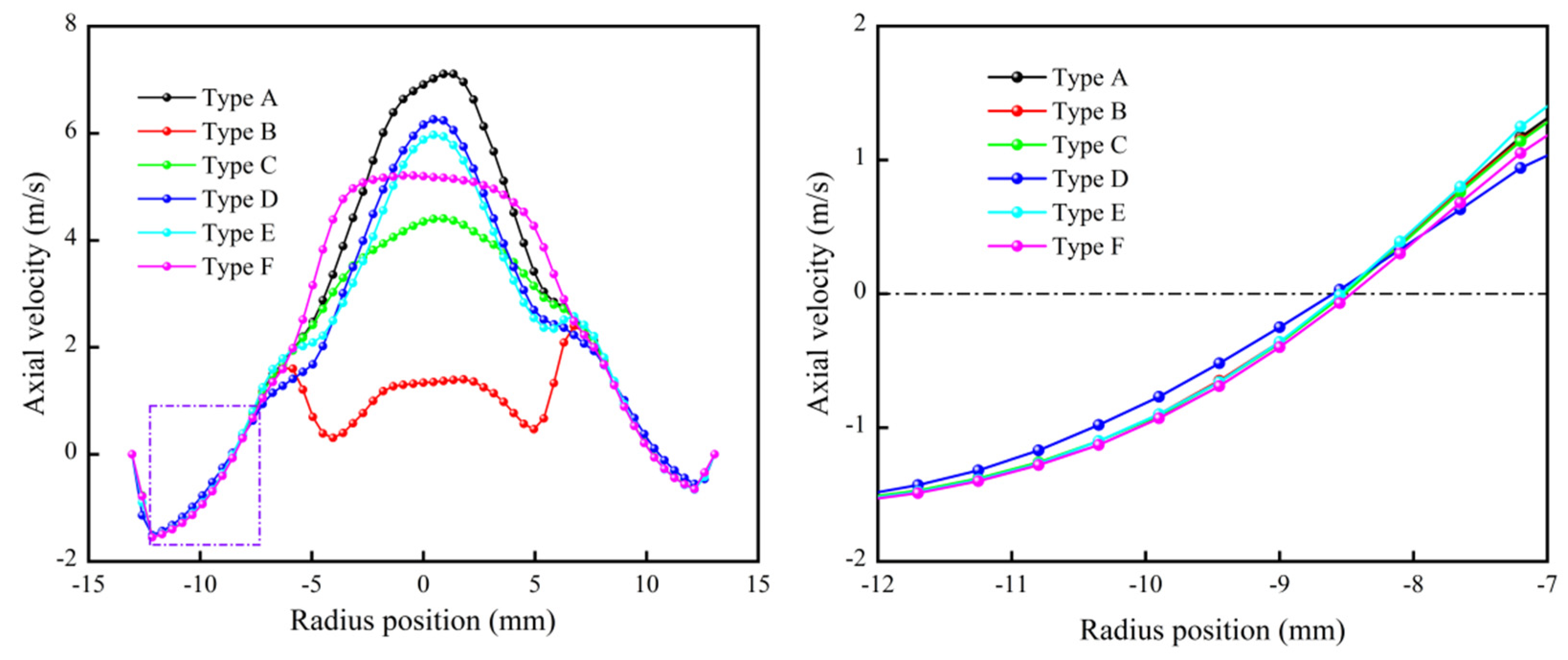
3.1.4. Axial Velocity
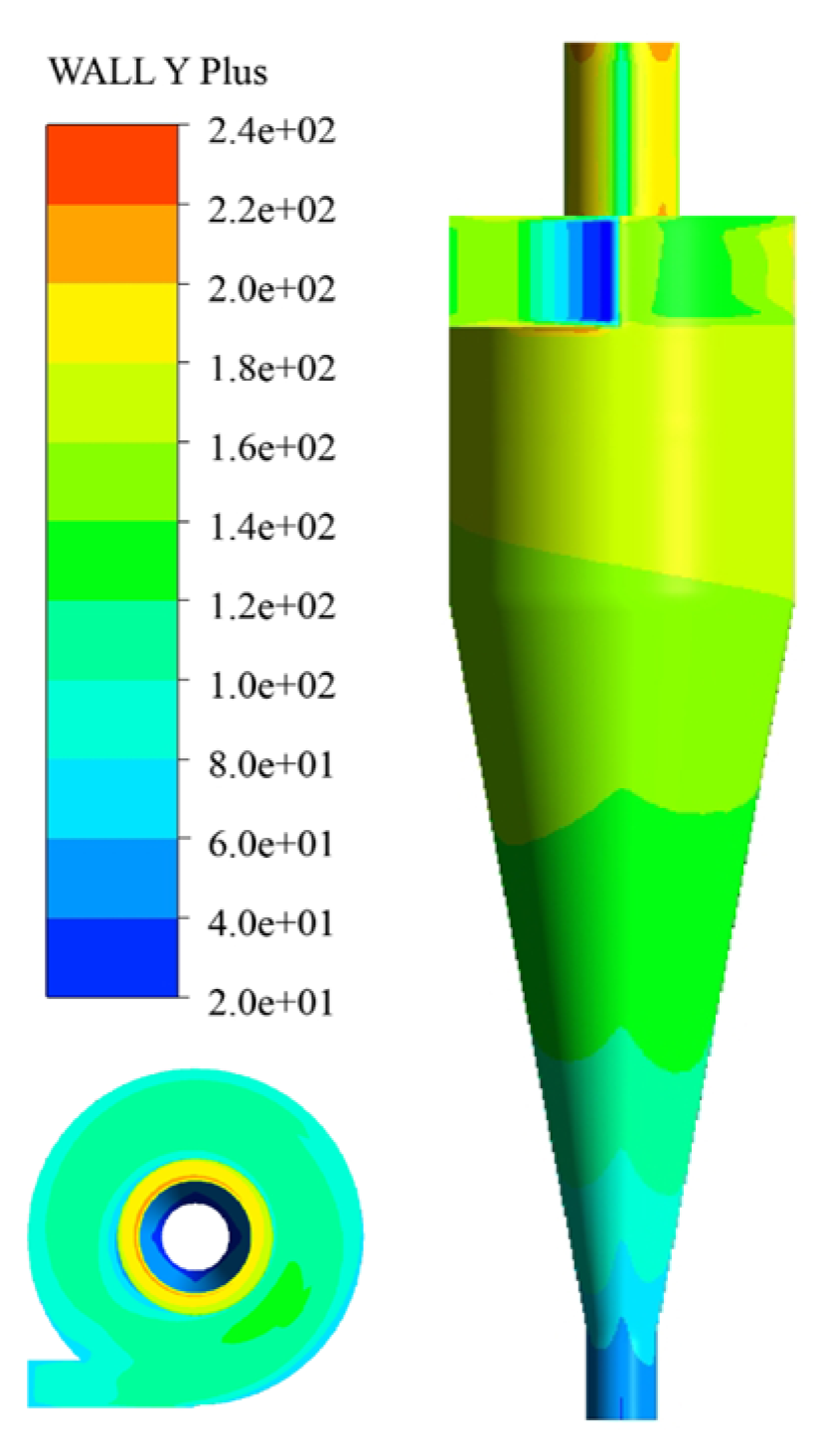
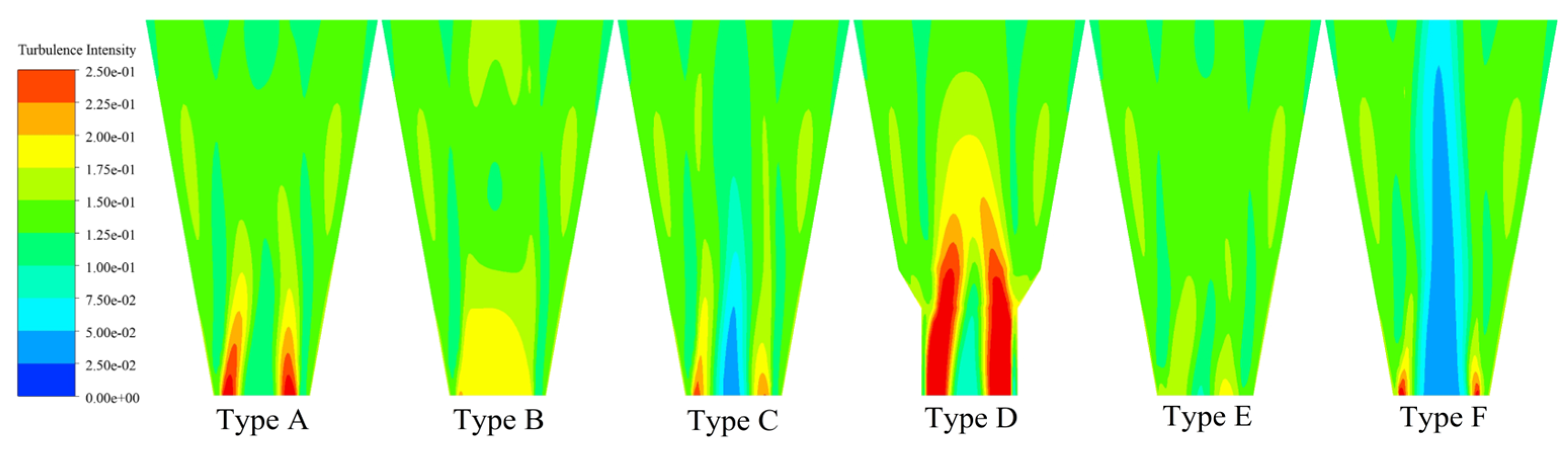
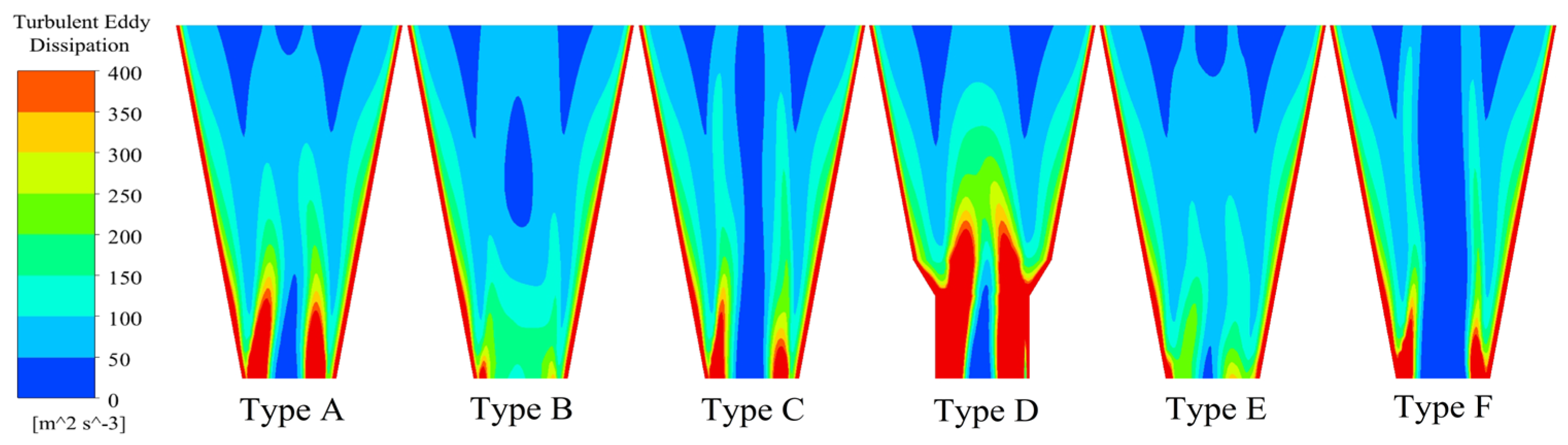
3.1.5. Turbulent Flow
3.2. Separation Performance
3.2.1. Separation Efficiency
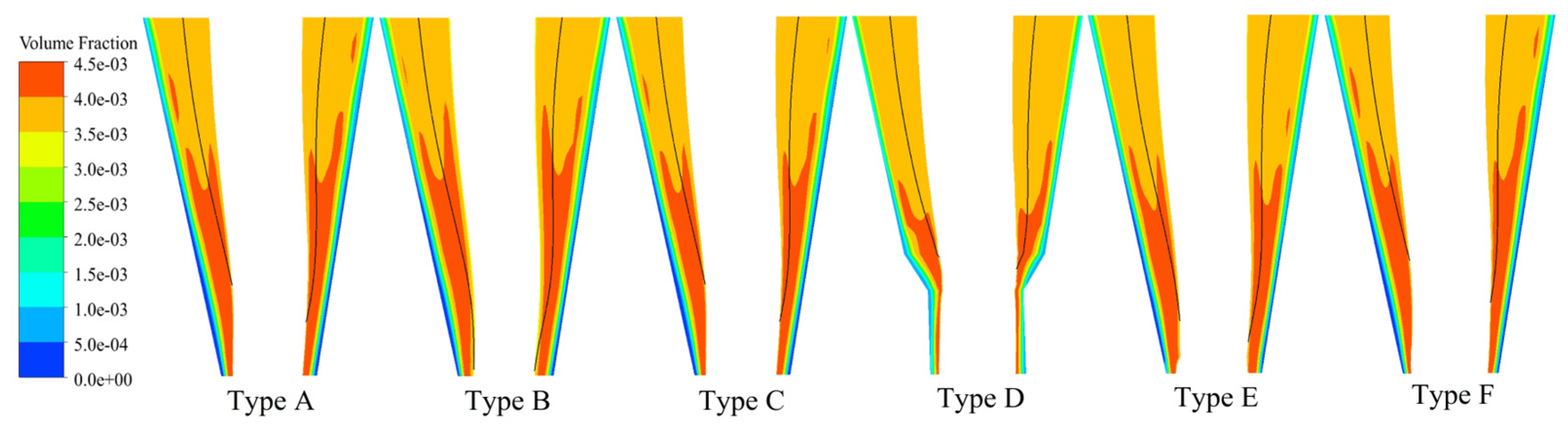
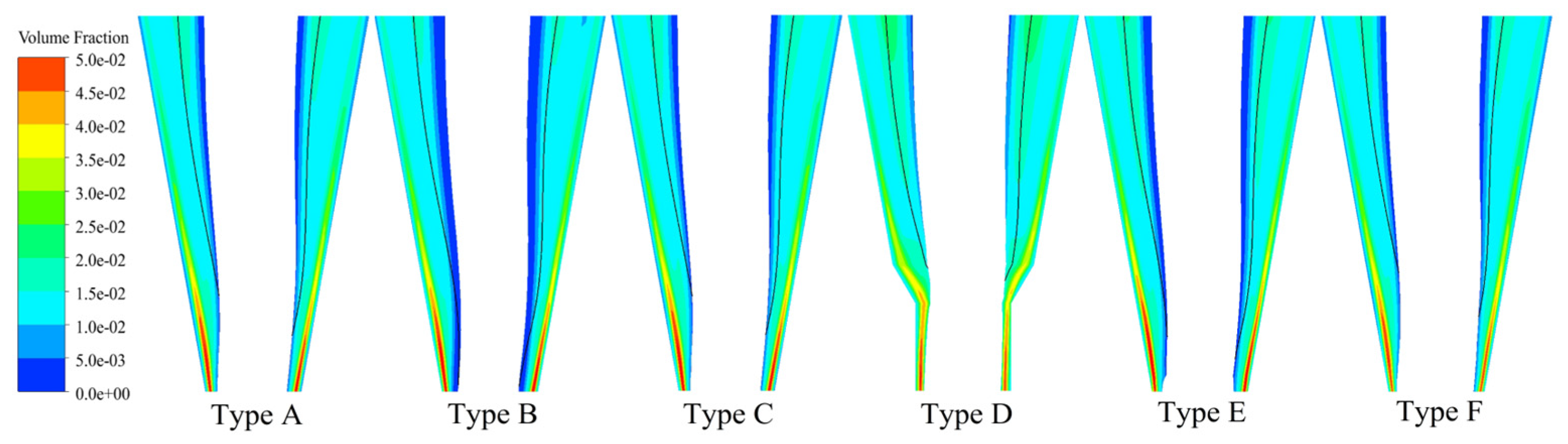
3.2.2. Particle Spatial Distribution
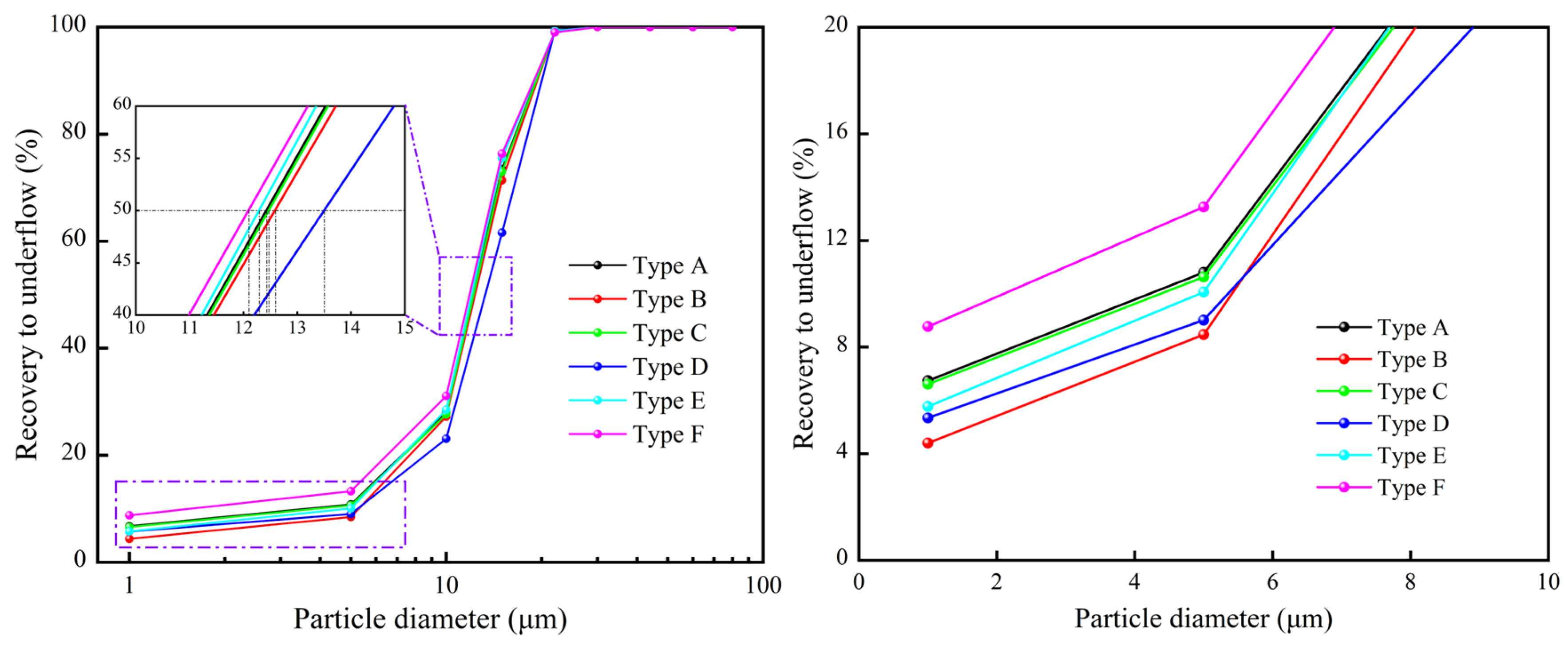
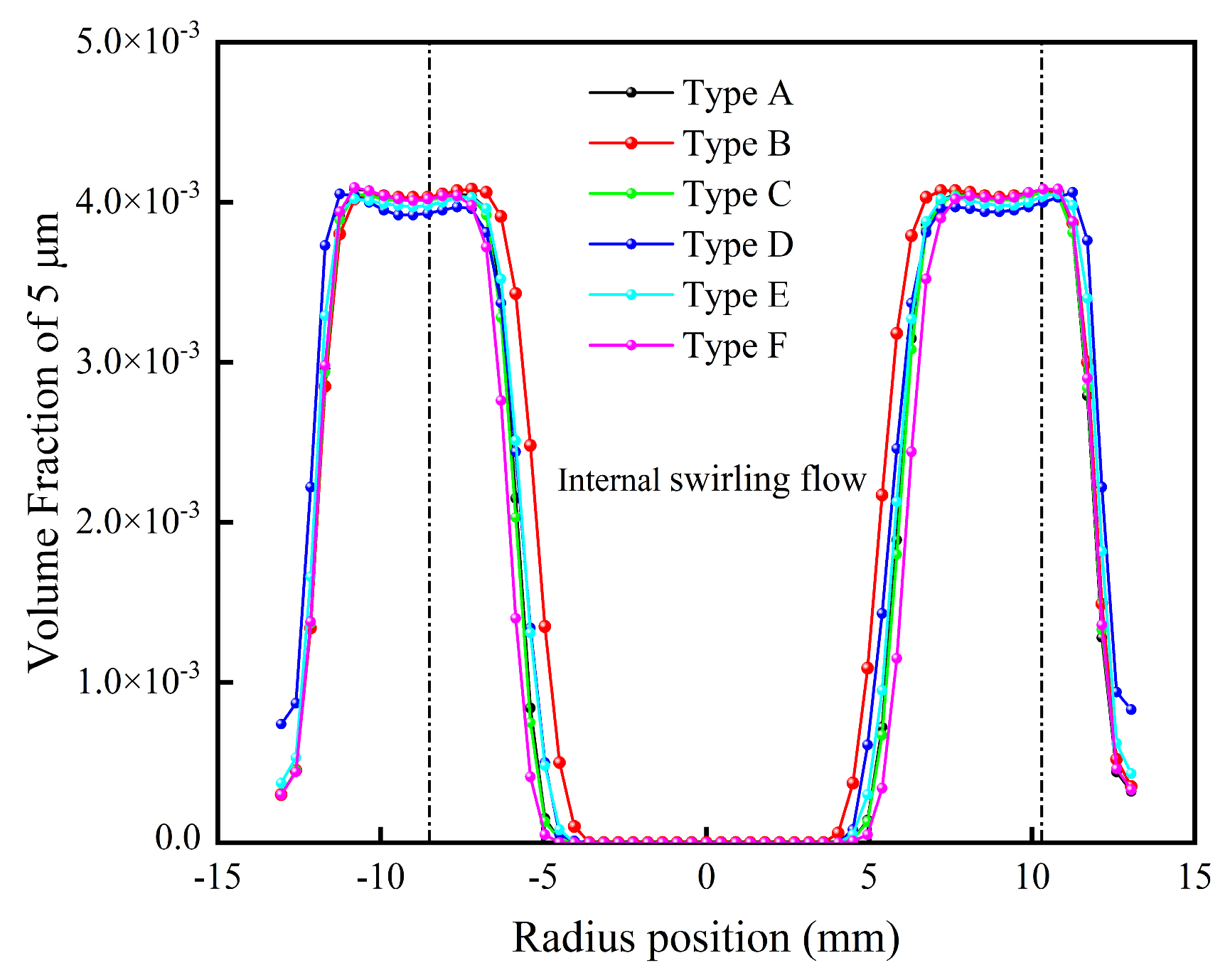
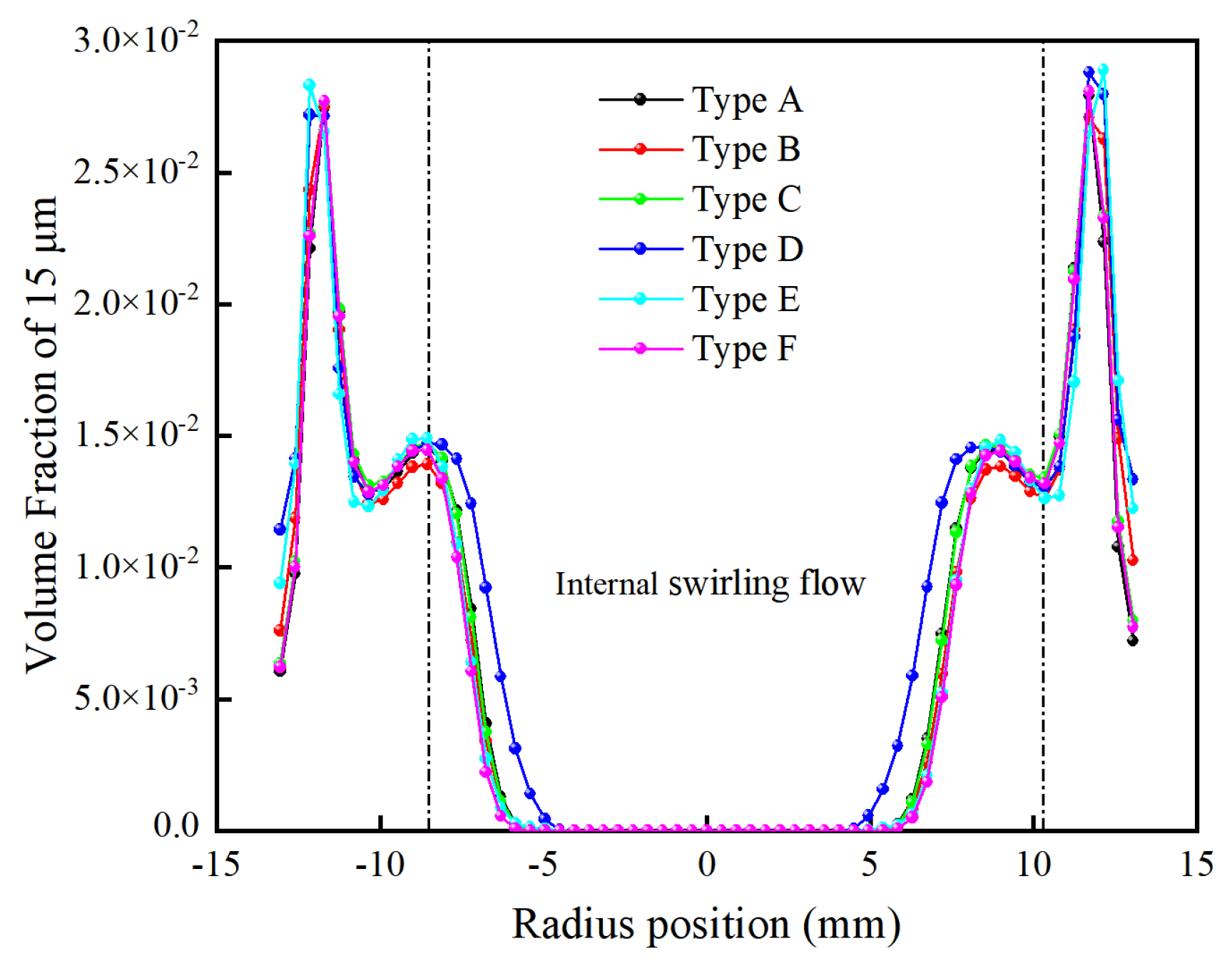
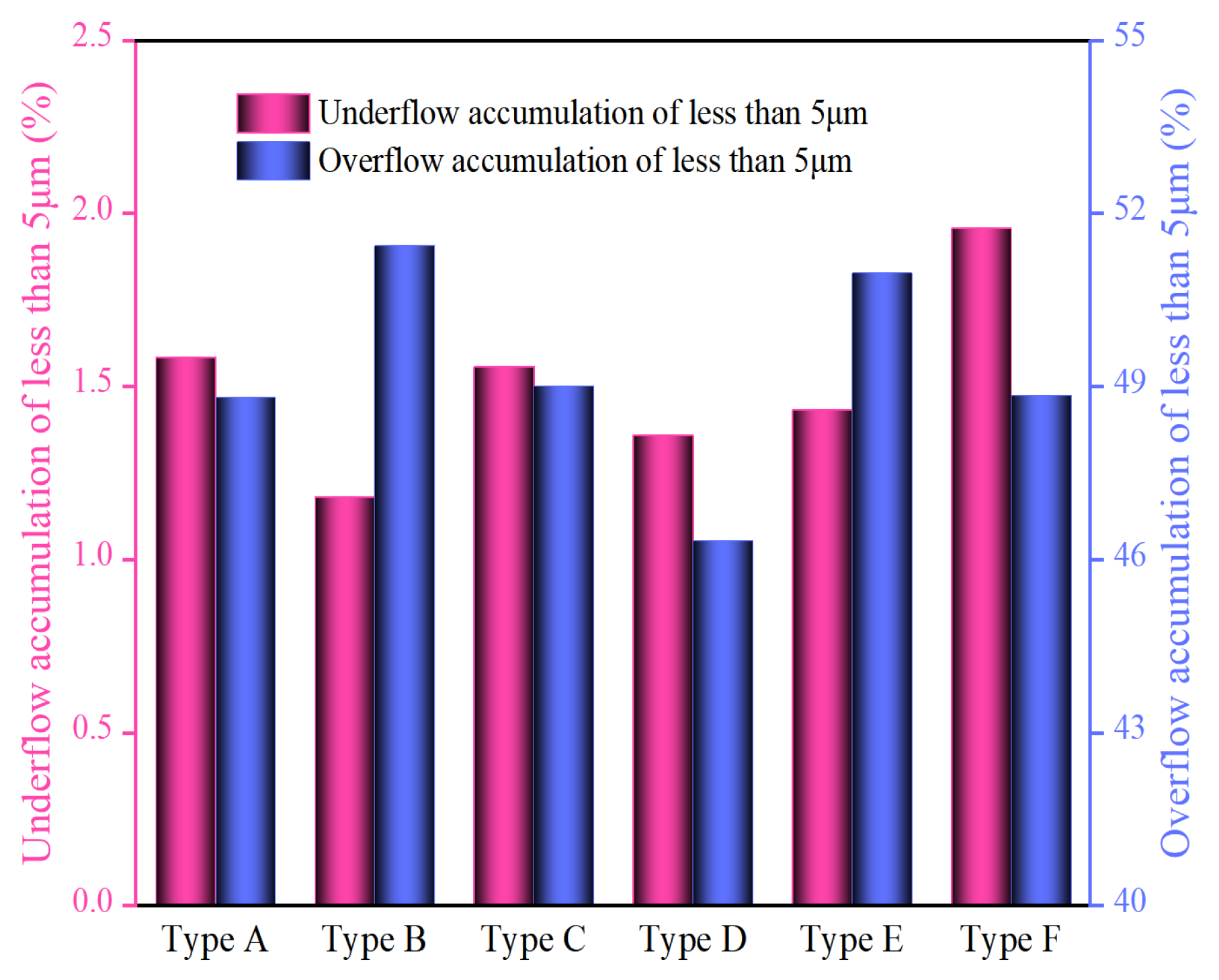
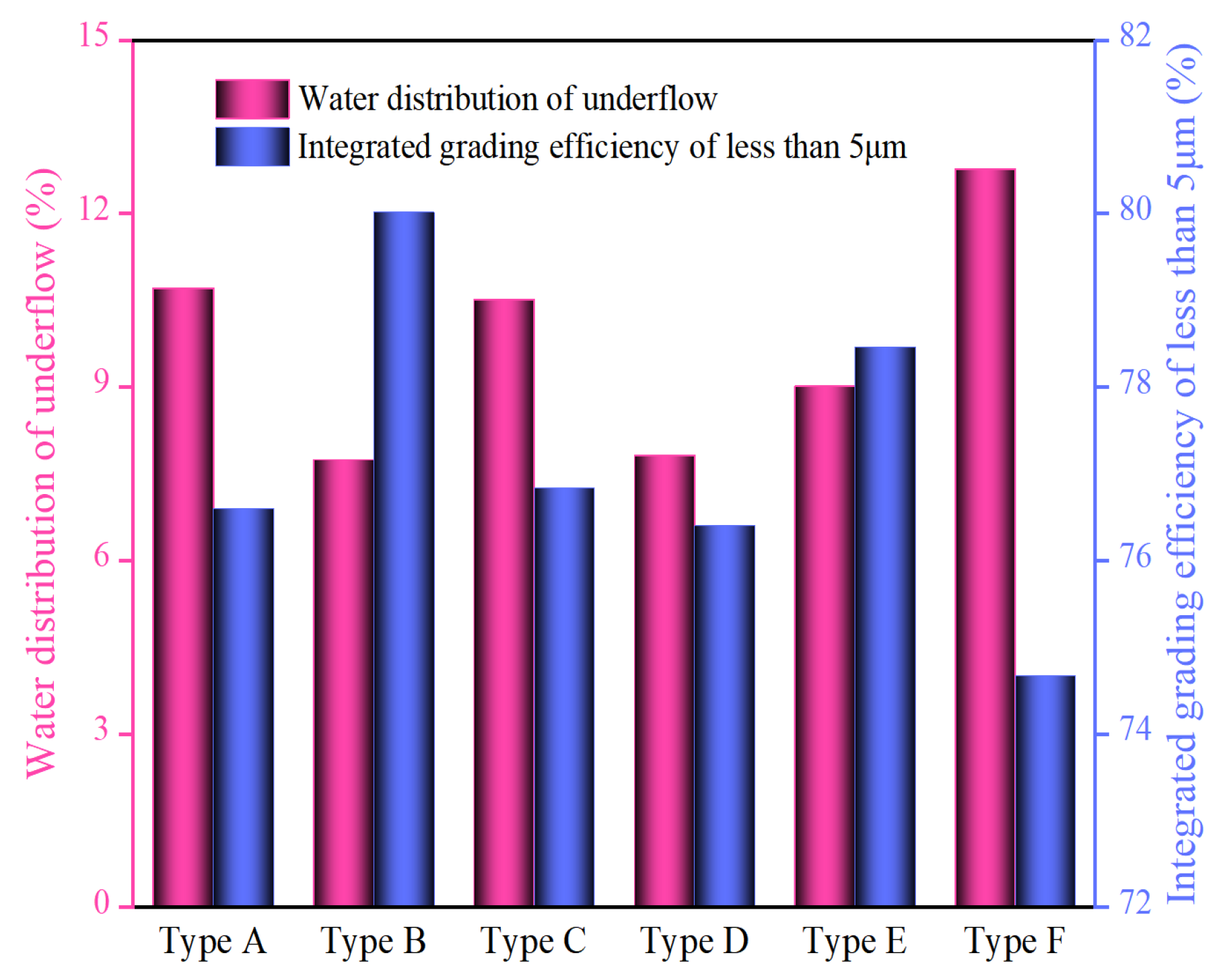
3.2.3. Separation Performance of Fine Particles
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- da Silva, J.T.T.; Bicalho, I.C.; Ribeiro, G.P.; Ataíde, C.H. Hydrocyclone Applied in the Physical Processing of Phosphate Concentrate Containing Rare Earth Elements. Miner. Eng. 2020, 155, 106402. [Google Scholar] [CrossRef]
- Dai, L.; Xu, D.; Wei, A.; Shan, M.; Tao, P.; Deng, Z.; Li, J.; Chen, J.; Wang, H. Purification of Hydrogenated Oil by Microchannel Separation Coupling Hydrocyclone. Process Saf. Environ. Prot. 2023, 180, 981–991. [Google Scholar] [CrossRef]
- Zhang, C.; Lu, S. Study on the Separation Mechanism of Coal and Gangue Particles during Coal Slime Classification in a Hydrocyclone. Powder Technol. 2023, 424, 118566. [Google Scholar] [CrossRef]
- Khatri, N.; Khatri, K.K.; Sharma, A. Enhanced Energy Saving in Wastewater Treatment Plant Using Dissolved Oxygen Control and Hydrocyclone. Environ. Technol. Innov. 2020, 18, 100678. [Google Scholar] [CrossRef]
- Tang, Z.; Yu, L.; Wang, F.; Li, N.; Chang, L.; Cui, N. Effect of Particle Size and Shape on Separation in a Hydrocyclone. Water 2018, 11, 16. [Google Scholar] [CrossRef]
- Ji, L.; He, L.; Chu, K.; Kuang, S. How Particles with Sizes Close to Cut Size Affect the Multiphase Flows and Performance of Hydrocyclones. Ind. Eng. Chem. Res. 2021, 60, 18477–18489. [Google Scholar] [CrossRef]
- Gonçalves, S.M.; Ullmann, G.; Morimoto, M.G.; de Souza Barrozo, M.A.; Vieira, L.G.M. Effect of Rheology and Solids Concentration on Hydrocyclones Performance: A Study Involving the Design Variables of an Optimized Hydrocyclone. J. Pet. Sci. Eng. 2022, 210, 110019. [Google Scholar] [CrossRef]
- Zhang, Y.; Yang, M.; Liu, P. Sediment-Containing Sewage Separation Using Intermittent-Discharge Columnar Hydrocyclones. Water 2020, 12, 2883. [Google Scholar] [CrossRef]
- Palaniandy, S.; Yahyaei, M.; Powell, M. Assessment of Hydrocyclone Operation in Gravity Induced Stirred Mill Circuits. Miner. Eng. 2017, 108, 83–92. [Google Scholar] [CrossRef]
- Gonçalves, S.M.; Kyriakidis, Y.N.; Ullmann, G.; De Souza Barrozo, M.A.; Vieira, L.G.M. Design of an Optimized Hydrocyclone for High Efficiency and Low Energy Consumption. Ind. Eng. Chem. Res. 2020, 59, 16437–16449. [Google Scholar] [CrossRef]
- Izquierdo, J.; Sukunza, X.; Espinazo, P.; Vicente, J.; Aguado, R.; Olazar, M. In Depth Characterisation of Hydrocyclones: Ascertaining the Effect of Geometry and Operating Conditions on Their Performance. Adv. Powder Technol. 2023, 34, 104025. [Google Scholar] [CrossRef]
- Tian, J.; Ni, L.; Song, T.; Olson, J.; Zhao, J. An Overview of Operating Parameters and Conditions in Hydrocyclones for Enhanced Separations. Sep. Purif. Technol. 2018, 206, 268–285. [Google Scholar] [CrossRef]
- Cui, B.; Zhang, C.; Zhao, Q.; Hou, D.; Wei, D.; Song, T.; Feng, Y. Study on Interaction Effects between the Hydrocyclone Feed Flow Rate and the Feed Size Distribution. Powder Technol. 2020, 366, 617–628. [Google Scholar] [CrossRef]
- Pérez, D.; Cornejo, P.; Rodríguez, C.; Concha, F. Transition from Spray to Roping in Hydrocyclones. Miner. Eng. 2018, 123, 71–84. [Google Scholar] [CrossRef]
- Dubey, R.K.; Climent, E.; Banerjee, C.; Majumder, A.K. Performance Monitoring of a Hydrocyclone Based on Underflow Discharge Angle. Int. J. Miner. Process. 2016, 154, 41–52. [Google Scholar] [CrossRef]
- Su, T.; Zhang, Y. Effect of the Vortex Finder and Feed Parameters on the Short-Circuit Flow and Separation Performance of a Hydrocyclone. Processes 2022, 10, 771. [Google Scholar] [CrossRef]
- Ji, L.; Kuang, S.; Yu, A. Numerical Investigation of Hydrocyclone Feed Inlet Configurations for Mitigating Particle Misplacement. Ind. Eng. Chem. Res. 2019, 58, 16823–16833. [Google Scholar] [CrossRef]
- Jiang, L.; Liu, P.; Zhang, Y.; Li, X.; Yang, X.; Hou, D.; Chen, B. Effect of Cone Section Combination Form on the Separation Performance of a Biconical Hydrocyclone. Powder Technol. 2023, 419, 118325. [Google Scholar] [CrossRef]
- Dou, D.; Qiu, Z.; Yang, J. Parameter Optimization of an Industrial Water Injection Hydrocyclone in the Taixi Coal Preparation Plant. Int. J. Coal Prep. Util. 2022, 42, 2357–2365. [Google Scholar] [CrossRef]
- Kyriakidis, Y.N.; Silva, D.O.; Barrozo, M.A.S.; Vieira, L.G.M. Effect of Variables Related to the Separation Performance of a Hydrocyclone with Unprecedented Geometric Relationships. Powder Technol. 2018, 338, 645–653. [Google Scholar] [CrossRef]
- Silva, N.K.G.; Silva, D.O.; Vieira, L.G.M.; Barrozo, M.A.S. Effects of Underflow Diameter and Vortex Finder Length on the Performance of a Newly Designed Filtering Hydrocyclone. Powder Technol. 2015, 286, 305–310. [Google Scholar] [CrossRef]
- Ye, J.; Xu, Y.; Song, X.; Yu, J. Numerical Modelling and Multi-Objective Optimization of the Novel Hydrocyclone for Ultra-Fine Particles Classification. Chem. Eng. Sci. 2019, 207, 1072–1084. [Google Scholar] [CrossRef]
- Zhang, C.; Cui, B.; Wei, D.; Lu, S. Effects of Underflow Orifice Diameter on the Hydrocyclone Separation Performance with Different Feed Size Distributions. Powder Technol. 2019, 355, 481–494. [Google Scholar] [CrossRef]
- Yamamoto, T.; Oshikawa, T.; Yoshida, H.; Fukui, K. Improvement of Particle Separation Performance by New Type Hydro Cyclone. Sep. Purif. Technol. 2016, 158, 223–229. [Google Scholar] [CrossRef]
- Tian, J.; Ni, L.; Song, T.; Shen, C.; Yao, Y.; Zhao, J. Numerical Study of Foulant-Water Separation Using Hydrocyclones Enhanced by Reflux Device: Effect of Underflow Pipe Diameter. Sep. Purif. Technol. 2019, 215, 10–24. [Google Scholar] [CrossRef]
- Hou, D.; Cui, B.; Zhao, Q.; Wei, D.; Song, Z.; Feng, Y. Research on the Structure of the Cylindrical Hydrocyclone Spigot to Mitigate the Misplacement of Particles. Powder Technol. 2021, 387, 61–71. [Google Scholar] [CrossRef]
- Hou, D.; Zhao, Q.; Liu, P.; Jiang, L.; Cui, B.; Wei, D. Effects of Bottom Profile on the Circulation and Classification of Particles in Cylindrical Hydrocyclones. Adv. Powder Technol. 2023, 34, 104050. [Google Scholar] [CrossRef]
- Pathak, S.S.; Mishra, S.; Tyeb, M.H.; Majumder, A.K. Spigot Design Modification to Alleviate Roping in Hydrocyclones. Mining Metall. Explor. 2022, 39, 761–775. [Google Scholar] [CrossRef]
- Jiang, L.; Liu, P.; Yang, X.; Zhang, Y.; Wang, H. Designing W-Shaped Apex for Improving the Separation Efficiency of a Full-Column Hydrocyclone. Sep. Sci. Technol. 2020, 55, 1724–1740. [Google Scholar] [CrossRef]
- Jiang, L.; Liu, P.; Yang, X.; Zhang, Y.; Li, X.; Zhang, Y.; Wang, H. Experimental Research on the Separation Performance of W-Shaped Hydrocyclone. Powder Technol. 2020, 372, 532–541. [Google Scholar] [CrossRef]
- Han, T.; Liu, H.; Xiao, H.; Chen, A.; Huang, Q. Experimental Study of the Effects of Apex Section Internals and Conical Section Length on the Performance of Solid–Liquid Hydrocyclone. Chem. Eng. Res. Des. 2019, 145, 12–18. [Google Scholar] [CrossRef]
- Vega-Garcia, D.; Cilliers, J.J.; Brito-Parada, P.R. CFD Modelling of Particle Classification in Mini-Hydrocyclones. Sep. Purif. Technol. 2020, 251, 117253. [Google Scholar] [CrossRef]
- Liu, L.; Zhao, L.; Reifsnyder, S.; Gao, S.; Jiang, M.; Huang, X.; Jiang, M.; Liu, Y.; Rosso, D. Analysis of Hydrocyclone Geometry via Rapid Optimization Based on Computational Fluid Dynamics. Chem. Eng. Technol. 2021, 44, 1693–1707. [Google Scholar] [CrossRef]
- Li, Y.; Tao, S.; Song, X.; Zhao, Y. Study on Internal Flow Characteristics of Hydrocyclone with Guide Vanes. Sustainability 2023, 15, 5350. [Google Scholar] [CrossRef]
- Vysyaraju, R.; Pukkella, A.K.; Subramanian, S. Computational Investigation of a Novel Hydrocyclone for Fines Bypass Reduction. Powder Technol. 2022, 395, 501–515. [Google Scholar] [CrossRef]
- Jing, J.; Zhang, S.; Qin, M.; Luo, J.; Shan, Y.; Cheng, Y.; Tan, J. Numerical Simulation Study of Offshore Heavy Oil Desanding by Hydrocyclones. Sep. Purif. Technol. 2021, 258, 118051. [Google Scholar] [CrossRef]
- Durango-Cogollo, M.; Garcia-Bravo, J.; Newell, B.; Gonzalez-Mancera, A. CFD Modeling of Hydrocyclones-A Study of Efficiency of Hydrodynamic Reservoirs. Fluids 2020, 5, 118. [Google Scholar] [CrossRef]
- Zhang, C.; Wei, D.; Cui, B.; Li, T.; Luo, N. Effects of Curvature Radius on Separation Behaviors of the Hydrocyclone with a Tangent-Circle Inlet. Powder Technol. 2017, 305, 156–165. [Google Scholar] [CrossRef]
- Fang, X.; Wang, G.; Zhong, L.; Qiu, S.; Wang, D. Adaptability Analysis of Operating Parameters of Hydrate Hydrocyclone Separator Based on a CFD Simulation. Sep. Sci. Technol. 2022, 57, 979–989. [Google Scholar] [CrossRef]
- Padhi, M.; Mangadoddy, N.; Sreenivas, T.; Vakamalla, T.R.; Mainza, A.N. Study on Multi-Component Particle Behaviour in a Hydrocyclone Classifier Using Experimental and Computational Fluid Dynamics Techniques. Sep. Purif. Technol. 2019, 229, 115698. [Google Scholar] [CrossRef]
- Vakamalla, T.R.; Mangadoddy, N. The Dynamic Behaviour of a Large-Scale 250-Mm Hydrocyclone: A CFD Study. Asia-Pacific J. Chem. Eng. 2019, 14, e2287. [Google Scholar] [CrossRef]
- Liu, P.; Fu, W.; Jiang, L.; Zhang, Y.; Li, X.; Yang, X.; Chen, B. Effect of Back Pressure on the Separation Performance of a Hydrocyclone. Powder Technol. 2022, 409, 117823. [Google Scholar] [CrossRef]

| Structure Parameters | Size |
|---|---|
| Diameter of cylinder section, D (mm) | 75 |
| Diameter of inlet, a × b (mm) | 10 × 26 |
| Height of body, H (mm) | 320 |
| Height of outer vortex finder, H1 (mm) | 40 |
| Height of cylinder section, H2 (mm) | 90 |
| Height of cone section, H3 (mm) | 170 |
| Cone angle (°) | 20 |
| Diameter of vortex finder, do (mm) | 25 |
| Insertion depth of vortex finder, h (mm) | 50 |
| Diameter of spigot, ds (mm) | 15 |
| Size Interval (μm) | Mean Size (μm) | Yield (%) | Volume Fraction (%) |
|---|---|---|---|
| 0–2 | 1 | 4.35 | 0.174 |
| 2–7 | 5 | 8.47 | 0.339 |
| 7–12 | 10 | 9.94 | 0.398 |
| 12–18 | 15 | 12.34 | 0.494 |
| 18–25 | 22 | 18.18 | 0.727 |
| 25–35 | 30 | 15.79 | 0.632 |
| 35–50 | 44 | 14.33 | 0.573 |
| 50–70 | 60 | 9.83 | 0.393 |
| 70–90 | 80 | 6.77 | 0.271 |
| Type A | Type B | Type C | Type D | Type E | Type F | |
|---|---|---|---|---|---|---|
| d50 (μm) | 12.5 | 12.7 | 12.5 | 13.6 | 12.4 | 11.9 |
| Ep (μm) | 3.20 | 3.25 | 3.15 | 3.60 | 3.15 | 3.15 |
| I | 0.256 | 0.256 | 0.252 | 0.265 | 0.254 | 0.252 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, P.; Chen, B.; Hou, D.; Yang, X.; Zhang, W.; Lu, Y. Designing the Spigot Structure of Hydrocyclones to Reduce Fine Particle Misplacement in Underflow. Water 2024, 16, 1070. https://doi.org/10.3390/w16071070
Liu P, Chen B, Hou D, Yang X, Zhang W, Lu Y. Designing the Spigot Structure of Hydrocyclones to Reduce Fine Particle Misplacement in Underflow. Water. 2024; 16(7):1070. https://doi.org/10.3390/w16071070
Chicago/Turabian StyleLiu, Peikun, Bo Chen, Duanxu Hou, Xinghua Yang, Wei Zhang, and Yuanli Lu. 2024. "Designing the Spigot Structure of Hydrocyclones to Reduce Fine Particle Misplacement in Underflow" Water 16, no. 7: 1070. https://doi.org/10.3390/w16071070
APA StyleLiu, P., Chen, B., Hou, D., Yang, X., Zhang, W., & Lu, Y. (2024). Designing the Spigot Structure of Hydrocyclones to Reduce Fine Particle Misplacement in Underflow. Water, 16(7), 1070. https://doi.org/10.3390/w16071070





