The Impacts of River Channel Blockages Caused by Sliding Embankment Collapses during Earthquakes
Abstract
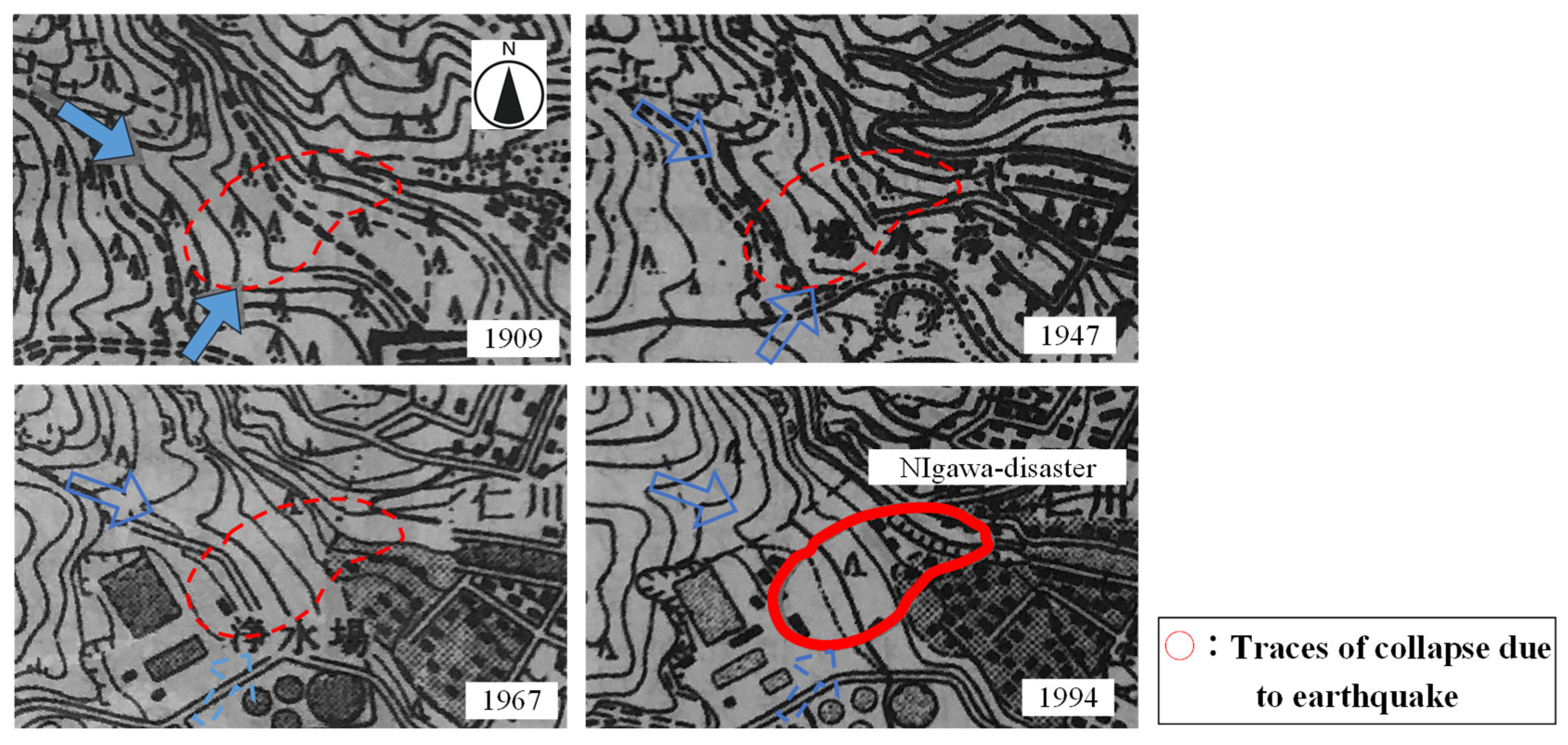
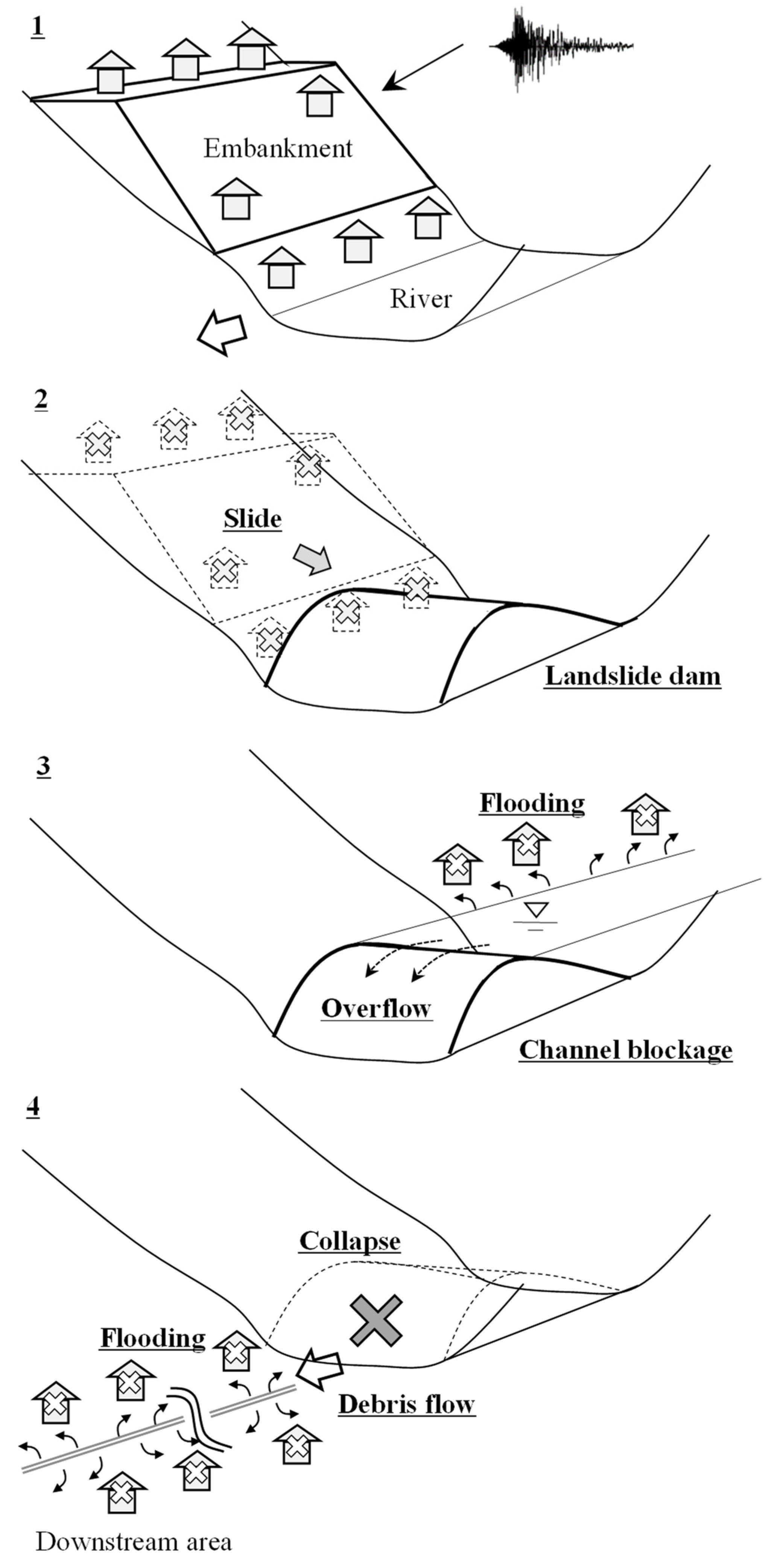
1. Introduction
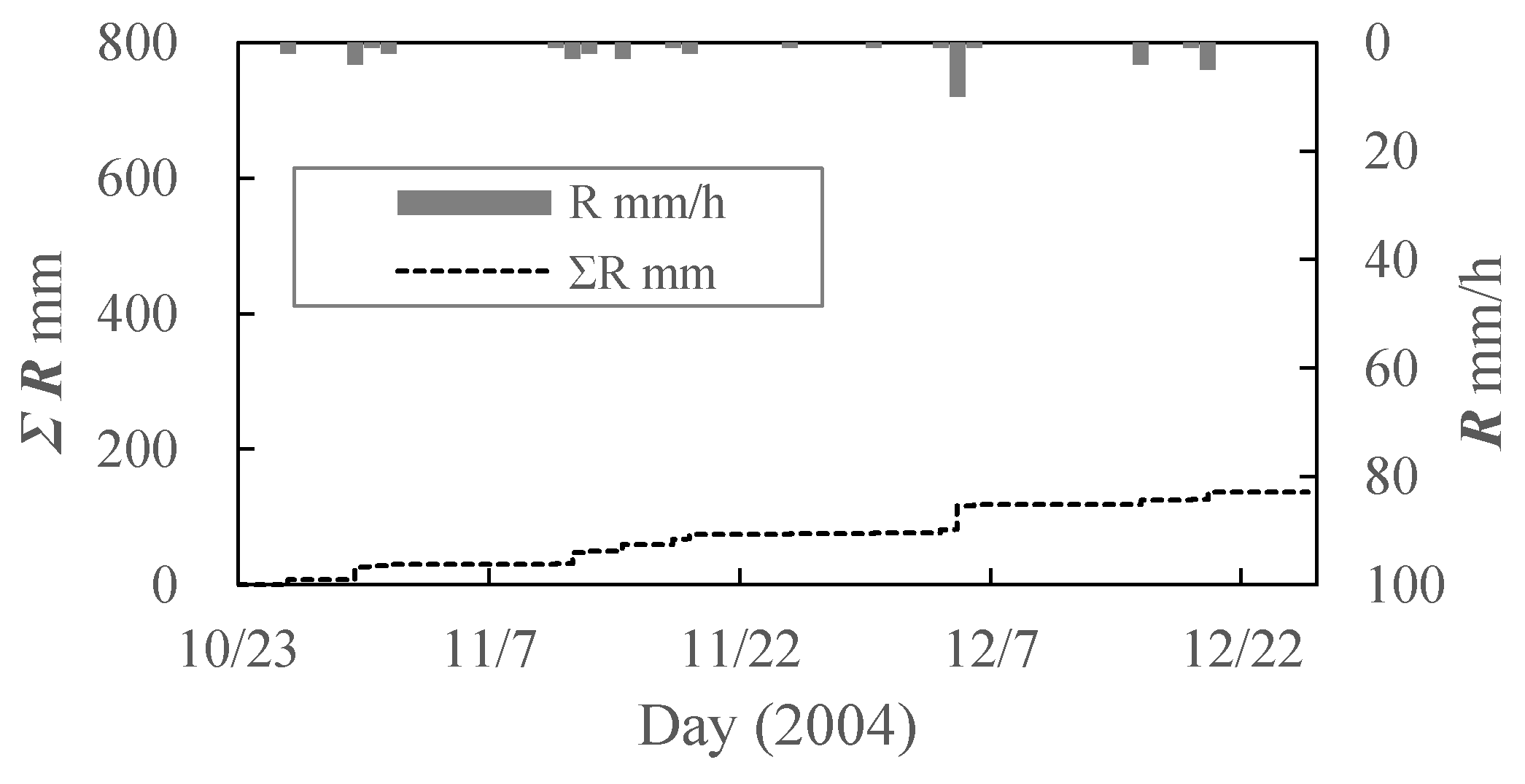
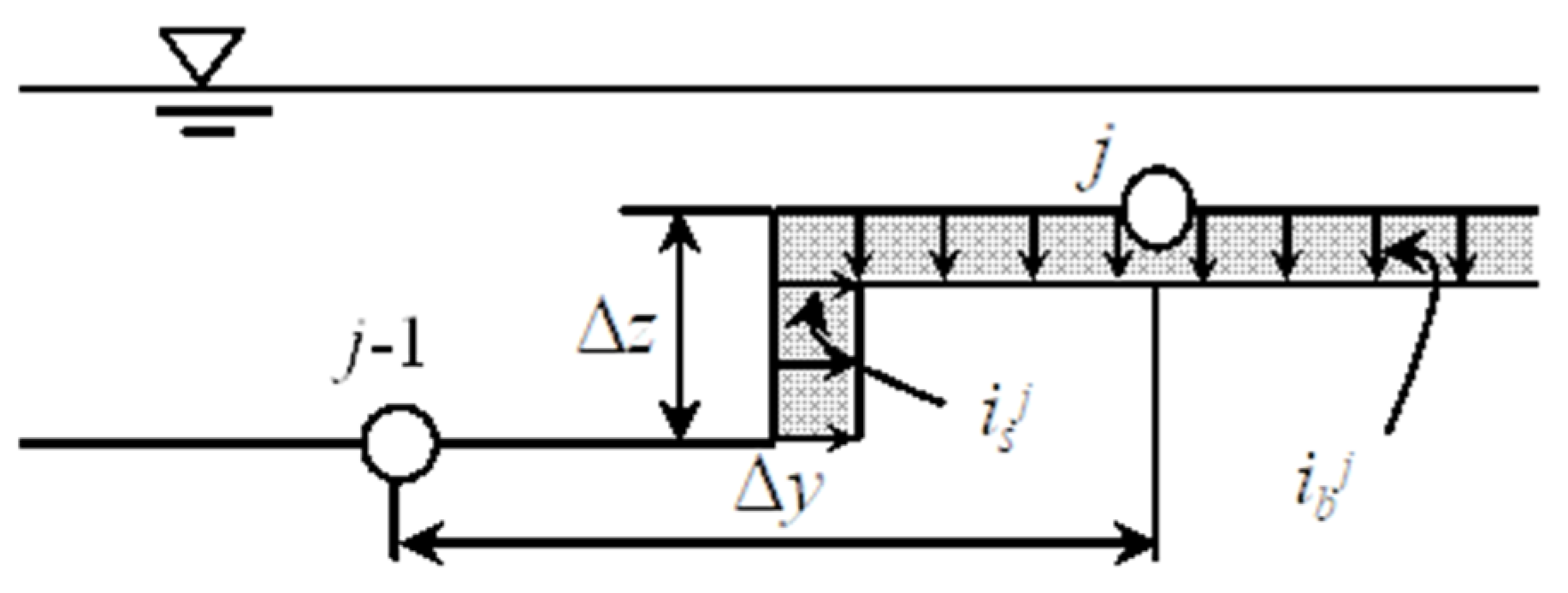
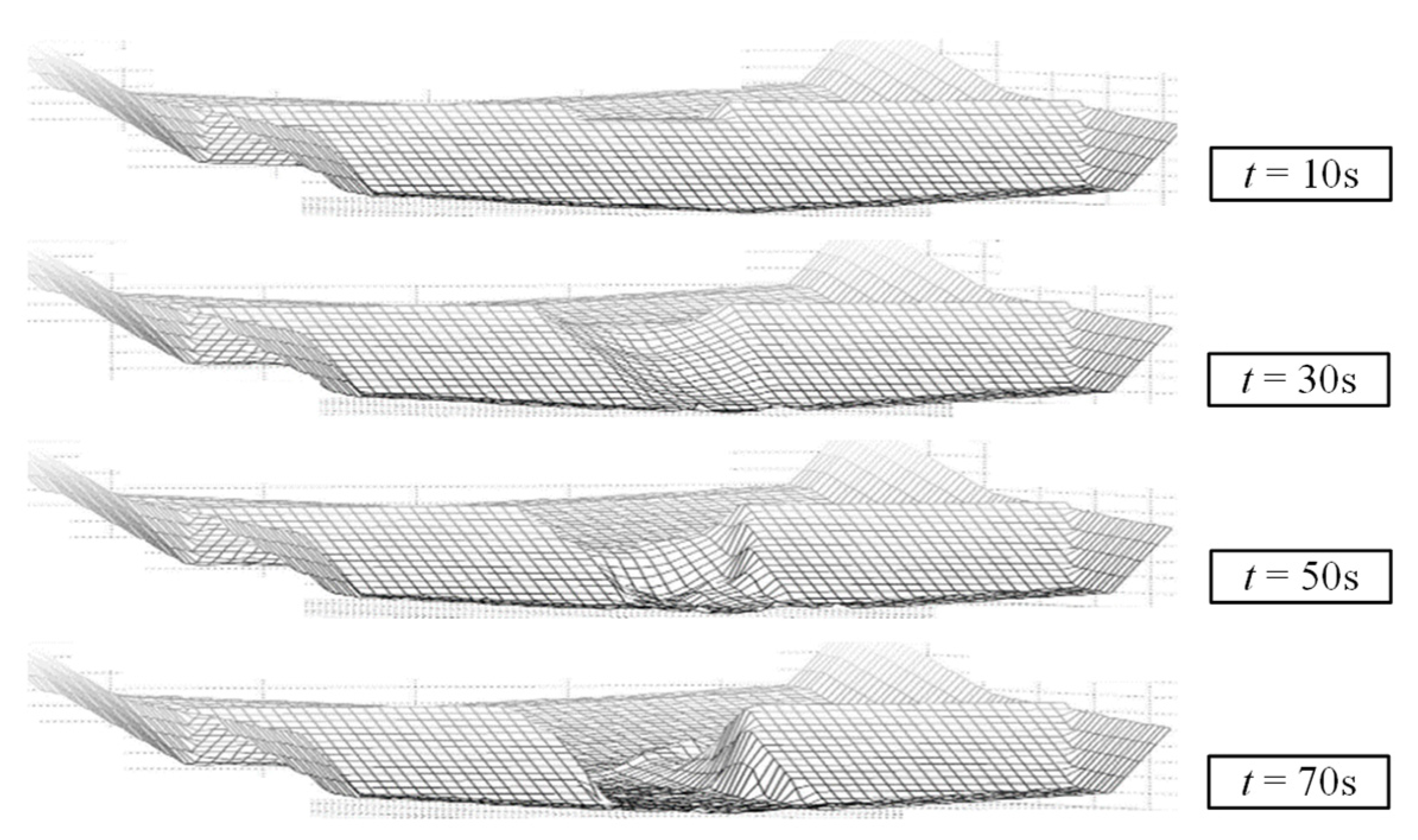
2. Prediction of Deposition Shape during River Channel Blockage by Embankments
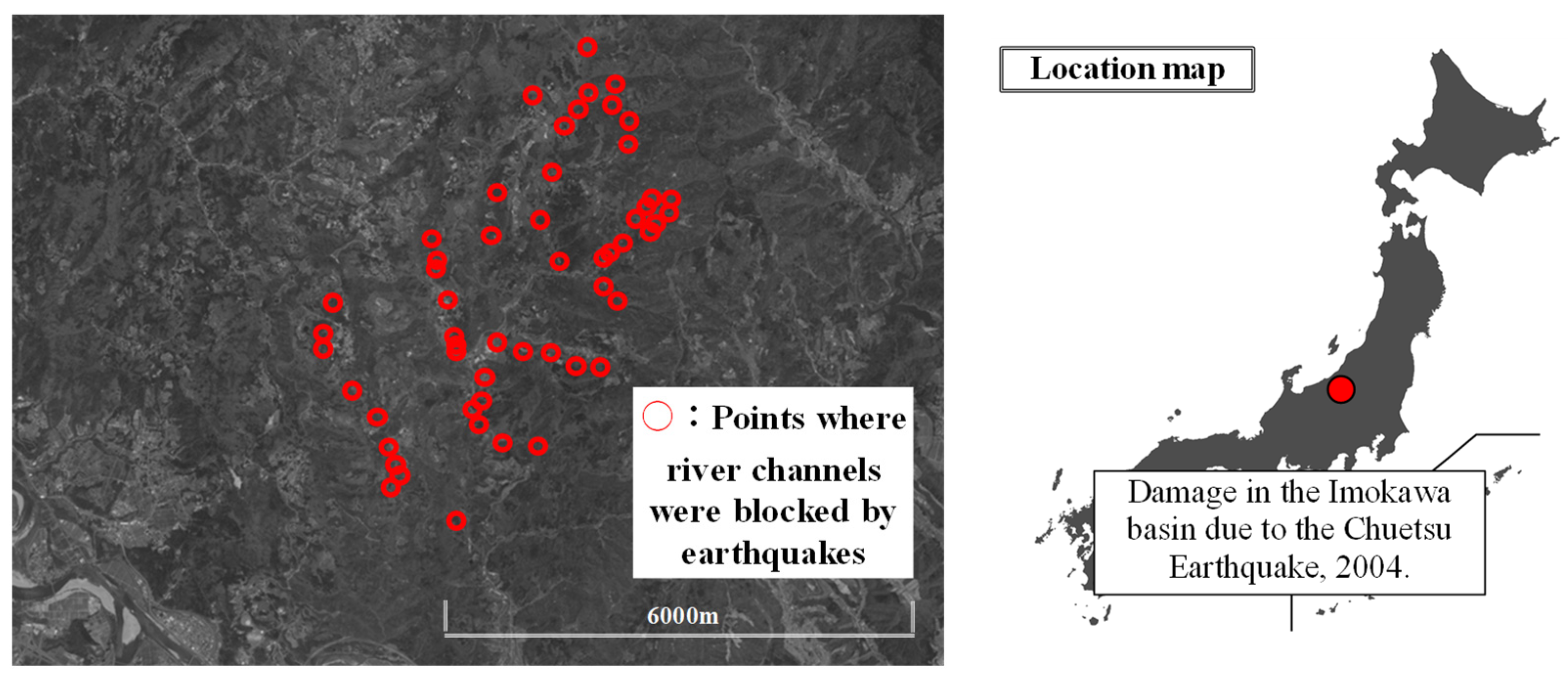
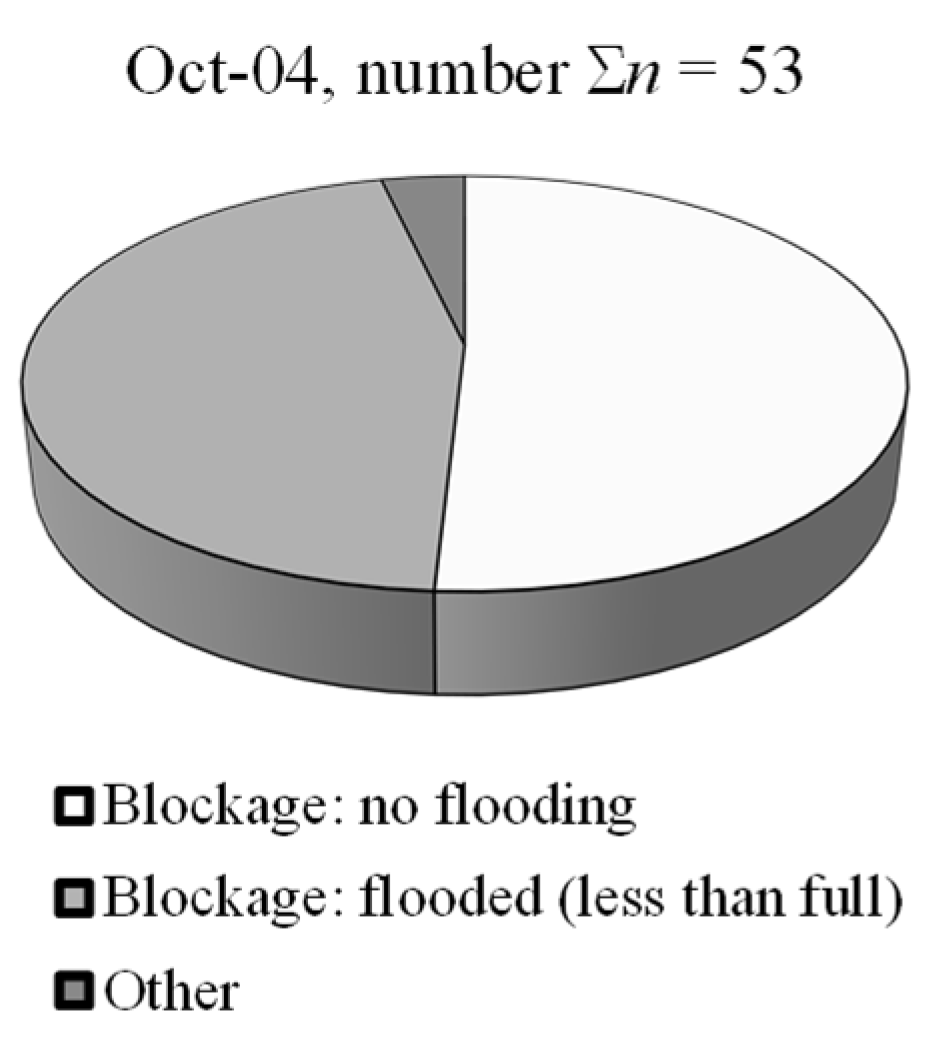
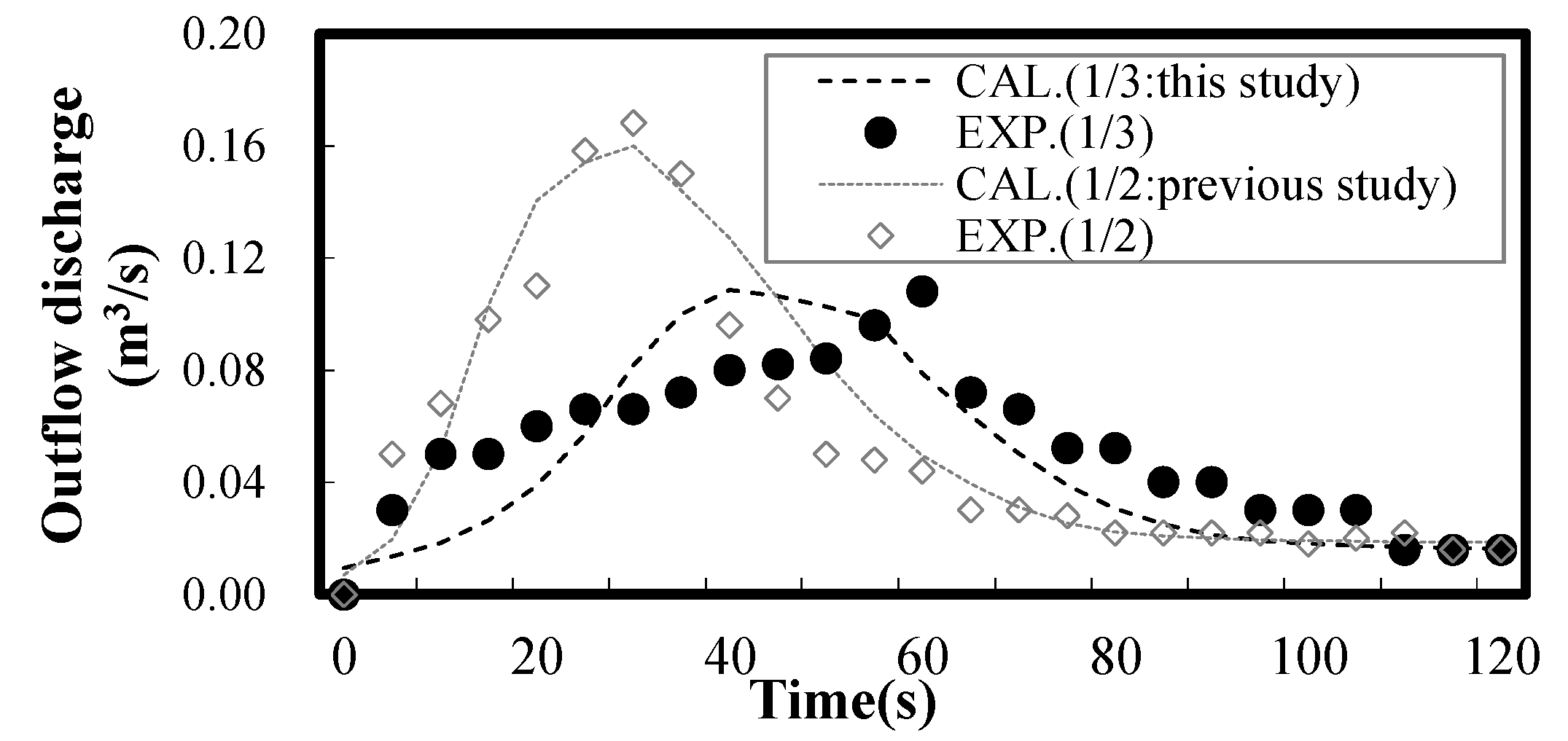
3. Prediction of the Flood Outflow Risk When a Landslide Dam Breaches
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhou, Y.; Sheng, Q.; Li, N.; Fu, X. The dynamic mechanical properties of a hard rock under true triaxial damage-controlled dynamic cyclic loading with different loading rates: A case study. Rock Mech. Rock Eng. 2022, 55, 2471–2492. [Google Scholar] [CrossRef]
- Djalante, R. Key assessments from the IPCC special report on global warming of 1.5 °C and the implications for the Sendai framework for disaster risk reduction. Prog. Disaster Sci. 2019, 1, 100001. [Google Scholar] [CrossRef]
- Bai, S.; Lu, P.; Thiebes, B. Comparing characteristics of rainfall and earthquake-triggered landslides in the upper Minjiang catchment, China. Eng. Geol. 2020, 268, 105518. [Google Scholar] [CrossRef]
- Haque, U.; Da Silva, P.F.; Devoli, G.; Pilz, J.; Zhao, B.; Khaloua, A.; Wilopo, W.; Andersen, P.; Lu, P.; Lee, J.; et al. The human cost of global warming: Deadly landslides and their triggers (1995–2014). Sci. Total Environ. 2019, 682, 673–684. [Google Scholar] [CrossRef]
- Domènech, G.; Fan, X.; Scaringi, G.; van Asch, T.W.; Xu, Q.; Huang, R.; Hales, T.C. Modelling the role of material depletion, grain coarsening and revegetation in debris flow occurrences after the 2008 Wenchuan earthquake. Eng. Geol. 2019, 250, 34–44. [Google Scholar] [CrossRef]
- Zhou, W.W.; Fang, J.; Tang, C.; Yang, G. Empirical relationships for the estimation of debris flow runout distances on depositional fans in the Wenchuan earthquake zone. J. Hydrol. 2019, 577, 123932. [Google Scholar] [CrossRef]
- Ciccarese, G.; Mulas, M.; Alberoni, P.P.; Truffelli, G.; Corsini, A. Debris flows rainfall thresholds in the Apennines of Emilia-Romagna (Italy) derived by the analysis of recent severe rainstorms events and regional meteorological data. Geomorphology 2020, 358, 107097. [Google Scholar] [CrossRef]
- Liu, S.; Wei, L.; Hu, K. Topographical and geological variation of effective rainfall for debris-flow occurrence from a large-scale perspective. Geomorphology 2020, 358, 107134. [Google Scholar] [CrossRef]
- Zhang, S.J.; Xu, C.X.; Wei, F.Q.; Hu, K.H.; Xu, H.; Zhao, L.Q.; Zhang, G.P. A physics-based model to derive rainfall intensity-duration threshold for debris flow. Geomorphology 2020, 351, 106930. [Google Scholar] [CrossRef]
- Guo, Z.X.; Cao, S.Y.; Liu, X.N.; Fang, D. Experimental study on parameters affecting the river-blocking due to debris flow. J. Hydraul. Eng. 2004, 11, 39–45. (In Chinese) [Google Scholar] [CrossRef]
- Yan, Z.W.; Wei, Y.Q.; Cai, H. Formation mechanism and stability analysis of barrier dam. Chin. J. Geol. Hazard Control 2009, 20, 55–59. (In Chinese) [Google Scholar] [CrossRef]
- Chang, D.S.; Zhang, L.M.; Xu, Y.; Huang, R.Q. Field testing of erodibility of two landslide dams triggered by the 12 May Wenchuan earthquake. Landslides 2011, 8, 321–332. [Google Scholar] [CrossRef]
- Cui, P.; Chen, X.Q.; Zhu, Y.Y.; Su, F.H.; Wei, F.Q.; Han, Y.S.; Liu, H.J.; Zhuang, J.Q. The Wenchuan earthquake (May 12, 2008), Sichuan province, China, and resulting geohazards. Nat. Hazards 2011, 56, 19–36. [Google Scholar] [CrossRef]
- Chen, C.G.; Yao, L.K.; Yang, Q.H.; Liu, C.R. Study of river-blocking due to debris flow based on weir flow theory. J. Nat. Disasters 2012, 21, 131–135. (In Chinese) [Google Scholar] [CrossRef]
- Cui, P.; Zhou, G.G.D.; Zhu, X.H.; Zhang, J.Q. Scale amplification of natural debris flows caused by cascading landslide dam failures. Geomorphology 2013, 182, 173–189. [Google Scholar] [CrossRef]
- Liu, C.R.; Zhao, S.G. Experimental study on mechanism of large river blocking by debris flow. J. Chongqing Jiaotong Univ. (Nat. Sci.) 2016, 35, 90–95. [Google Scholar]
- Imaizumi, F.; Osanai, N.; Kato, M.; Koike, M.; Kosugi, K.; Sakai, Y.; Sakaguchi, H.; Satofuka, Y.; Takayama, S.; Tanaka, T.; et al. Debris flow disaster in Atami, Shizuoka Prefecture, in July 2021. J. Jpn. Soc. Eros. Control Eng. 2022, 74, 34–42. [Google Scholar]
- Ministry of Land, Infrastructure, Transport and Tourism. Embankment Regulation Law. Available online: https://www.mlit.go.jp/toshi/toshi_tobou_tk_000076.html (accessed on 10 January 2023).
- Ministry of Land, Infrastructure, Transport and Tourism. Basic Survey Implementation Guidelines (Designation of Regulatory Areas), Commentary. 2023. Available online: https://www.mlit.go.jp/toshi/toshi_tobou_tk_000086.html (accessed on 10 January 2023).
- Nishimoto, H. A Study on Changes in the Names of Sediment Transport Phenomena and Debris Flows. Ph.D. Dissertation, The University of Tokyo, Tokyo, Japan, 2010. [Google Scholar]
- Central Disaster Management Council. 1847 Zenkoji Earthquake Report; Central Disaster Management Council: Japan, 2007; 201p.
- Sanada Treasure Museum. “Earthquake Post-World Secular Language Seed”. Available online: https://www.iida-museum.org/user/nature/earthquake/dishin.html (accessed on 10 January 2023).
- Liu, Y.; Chen, X.J.; Hu, M. Three-dimensional large deformation modeling of landslides in spatially variable and strain-softening soils subjected to seismic loads. Can. Geotech. J. 2022, 60, 426–437. [Google Scholar] [CrossRef]
- Chen, X.Y.; Zhang, L.L.; Yang, H.Q. Probabilistic runout analysis of landslide considering spatial variability. In Proceedings of the 7th International Symposium on Geotechnical Safety and Risk (ISGSR), Taipei, Taiwan, 11–13 December 2019; pp. 745–749. [Google Scholar]
- Fenton, G.A.; Griffiths, D.V. Risk Assessment in Geotechnical Engineering; John Wiley & Sons Inc.: New York, NY, USA, 2008. [Google Scholar]
- Shirouzu, T. Twenty years from the Great Hanshin Awaji earthquake. Rev. Consum. Co-Oper. Stud. 2015, 470, 62–64. [Google Scholar]
- Sasa, K. An earthquake induced rapid landslide in Nikawa, Nishinomiya-city, Japan. Soil Mech. Found. Eng. 1996, 44, 83–85. [Google Scholar]
- Kikuyama, H.; Yokoyama, S. Earthquake disasters of geotechnical structures due to the 1995 southern Hyogo prefecture earthquake. Annu. Rep. Fukada Geol. Inst. Found. 2015, 16, 75–96. [Google Scholar]
- Minstry of Land, Infrastructure, Transport and Tourism. Large-Scale Sediment Disasters Occurrence Situation—Proposal. Available online: https://www.mlit.go.jp/common/001024314.pdf (accessed on 10 January 2023).
- Zheng, H.; Shi, Z.; Shen, D.; Peng, M.; Hanley, K.J.; Ma, C.; Zhang, L. Recent advances in stability and failure mechanisms of landslide dams. Front. Earth Sci. 2021, 9, 659935. [Google Scholar] [CrossRef]
- Fan, X.; Dufresne, A.; Subramanian, S.S.; Strom, A.; Hermanns, R.; Tacconi Stefanelli, C.; Hewitt, K.; Yunus, A.P.; Dunning, S.; Capra, L.; et al. The formation and impact of landslide dams—State of the art. Earth-Sci. Rev. 2020, 203, 103116. [Google Scholar] [CrossRef]
- Asada, A. A method for estimating and increasing the stability of artificial housing ground during earthquake. J. Jpn. Soc. Soil Mech. Found. Eng. Soils Found. 1978, 22, 191–202. [Google Scholar] [CrossRef]
- Asada, A. Natural disasters and geotechnical engineering in the Ttohoku region: A decade after the Miyagi offshore earthquake. Soil Mech. Found. Eng. 1989, 37, 57–62. [Google Scholar]
- Furuchi, Y.; Kunioki, G.; Ishizawa, T.; Yamamoto, J. Physico-mechanical characteristics of collapsed embankment during the Noto peninsula earthquake. J. Earthq. Eng. 2007, 1007–1010. [Google Scholar] [CrossRef]
- Yanagisawa, E.; Yasuda, S.; Yoshida, N.; Wakamatsu, K. Residential damage, disaster investigation report of the 1993 Kushiro-oki earthquake & Noto peninsula earthquake. Jpn. Geotech. Soc. 1994, 180625004, 231–275. [Google Scholar]
- Ministry of Land, Infrastructure, Transport and Tourism. Regarding the Damage and Response to the 2023 Noto Peninsula Earthquake. Available online: https://www.mlit.go.jp/saigai/saigai_240101.html (accessed on 10 January 2023).
- Yokoyama, O.; Uchida, T.; Kinoshita, A. Classification of landslide dams based on the duration until failure. J. Jpn. Soc. Eros. Control Eng. 2016, 68, 14–23. [Google Scholar]
- Hata, Y.; Ichii, K.; Tokida, K. A probabilistic evaluation of the size of earthquake-induced slope failure for an embankment. Georisk: Assess. Manage. Risk Eng. Syst. Geo-Hazards 2012, 6, 73–88. [Google Scholar] [CrossRef]
- Hata, Y.; Ichii, K.; Tokida, K. a study on the collapse range of slopes during earthquakes. J. Jpn. Soc. Civ. Eng. Ser. C 2007, 63, 677–690. [Google Scholar]
- Kadota, K.; Sato, S.; Motohashi, A.; Tougo, T.; Kaneko, S. Investigation of the factors causing the collapse of residential ground by black and red soils damaged by the Kumamoto earthquake. J. Geotech. Eng. 2023, 18, 193–210. [Google Scholar]
- Nakamura, S.; Sendo, N.; Umemura, J.; Otsuka, S.; Toyoda, H. Geotechnical disasters in the Nakadori and Iwaki regions of Fukushima prefecture caused by the 2011 Tohoku-pacific ocean earthquake. J. Geotech. Eng. 2012, 7, 91–101. [Google Scholar]
- Costa, J.E.; Schuster, R.L. The Formation and Failure of Landslide Dams. Geol. Soc. Am. Bull. 1988, 100. Available online: https://pubs.usgs.gov/of/1987/0392/report.pdf (accessed on 10 January 2023).
- Takahashi, Y.; Masunari, M. A study on the scale of debris flow caused by the collapse of a landslide dam. Ann. Disaster Prev. Res. Inst. Kyoto Univ. 1988, B-2, 1–12. [Google Scholar]
- Mizuyama, T.; Mori, S.; Sakaguchi, T.; Inoue, K. Landslide dams in japan and countermeasures. Kokin Shoin 2011, 9784772261104, 4–11. [Google Scholar]
- Harada, N.; Kosugi, K.; Satofuka, Y.; Mizuyama, T. Prediction of landslide dam formation affected by topographical and geological conditions. J. Jpn. Soc. Civ. Eng. B1 2015, 71, I_1267–I_1272. [Google Scholar]
- Kainthura, P.; Sharma, N. Hybrid machine learning approach for landslide prediction, Uttarakhand, India. Sci. Rep. 2022, 12, 20101. [Google Scholar] [CrossRef]
- Zhou, C.; Yin, K.; Cao, Y.; Ahmed, B.; Fu, X. A novel method for landslide displacement prediction by integrating advanced computational intelligence algorithms. Sci. Rep. 2018, 8, 7287. [Google Scholar] [CrossRef]
- Nakamura, H.; Tsunaki, R.; Ishihama, S. simulation model for debris movement of landslides. Proc. Japan-China Symp. Landslides Debris Flows Niigata 1989, BA0769199X, 81–86. [Google Scholar]
- Sako, Y.; Mori, T.; Nakamura, H.; Yusa, N. Prediction of landslide dam shape formed by deep-seated landslides. J. Jpn. Soc. Eros. Control Eng. 2015, 68, 44–51. [Google Scholar]
- Harada, N.; Kosugi, K.; Satofuka, Y.; Mizuyama, T. prediction of landslide-dam height and length based on previous data. J. Jpn. Soc. Eros. Control Eng. 2016, 68, 47–50. [Google Scholar]
- Satofuka, Y.; Yoshino, K.; Mizuyama, T.; Ogawa, N.; Uchikawa, T.; Mori, T. A study on the prediction method of flood discharge due to the collapse of a landslide dam. J. Hydraul. Eng. 2007, 51, 901–906. [Google Scholar] [CrossRef][Green Version]
- Yang, M.; Cai, Q.; Li, Z.; Yang, J. Uncertainty analysis on flood routing of embankment dam breach due to overtopping failure. Sci. Rep. 2023, 13, 20151. [Google Scholar] [CrossRef] [PubMed]
- Nobarinia, M.; Kalateh, F.; Nourani, V.; Babaeian Amini, A. Dam failure peak outflow prediction through GEP-SVM meta models and uncertainty analysis. Water Supply 2021, 21, 3387–3401. [Google Scholar] [CrossRef]
- Harada, N.; Akazawa, F.; Hayami, S.; Yanagisaki, T.; Satofuka, Y.; Fujimoto, M.; Tsutsumi, D.; Miyata, H. Field experiment on landslide dam failure in the hill valley of ashitsuke river basin. J. Jpn. Soc. Eros. Control Eng. 2015, 67, 41–48. [Google Scholar]
- Harada, N.; Akazawa, F.; Hayami, S.; Satofuka, Y. Numerical simulation of landslide dam deformation caused by erosion. Adv. River Sediment Res. 2013, 1107–1116. [Google Scholar] [CrossRef]
- Hayashi, T. Quantification—Theory and Methods; Asakura Shoten: Tokyo, Japan, 1993; 233p. [Google Scholar]
- Geospatial Information Authority of Japan. Maps. Available online: https://maps.gsi.go.jp (accessed on 10 January 2023).
- NTT DATA. Satellite Images. Available online: https://www.nttdata.com/ (accessed on 10 January 2023).
- Ministry of Land, Infrastructure, Transport and Tourism. Available online: https://www.hrr.mlit.go.jp/yuzawa/sabo/chuetsu/pamphlet/pamphlet01.pdf (accessed on 10 January 2023).
- Ministry of Land, Infrastructure, Transport and Tourism. Available online: https://www.hrr.mlit.go.jp/yuzawa/sabo/chuetsu/taisaku/iinkai_2nd/genjou.pdf (accessed on 10 January 2023).
- Yoneyama, T.; Yanagida, M.; Ikeda, H.; Iseya, F.; Medai, K.; Kotama, Y.; Tamai, H. Are the terrace deposits along the Mogami river, Niigata prefecture, former Yamakoshi village, dammed by landslides (landslide dams)? J. Geol. Soc. Japan 2007, 116, 5. [Google Scholar]
- Japan Meteorological Agency. Available online: https://www.data.jma.go.jp/obd/stats/etrn (accessed on 10 January 2023).
- Takahashi, Y.; Nakagawa, I. Prediction of stony debris flow induced by severe rainfall. Jpn. Soc. Eros. Control Eng. 1991, 44, 12–19. [Google Scholar]
- Takahashi, Y.; Nakagawa, K.; Satofuka, Y. Sediment flushing using a reverse-flow system. J. Hydraul. Eng. 2002, 46, 785–790. [Google Scholar] [CrossRef][Green Version]
- Ashida, K.; Egashira, S.; Kamoto, M. Study on erosion and channel changes in mountainous watersheds (part 2). Ann. Disaster Prev. Res. Inst. Kyoto Univ. 1983, B26, 353–361. [Google Scholar]
- Shimizu, Y.; Itakura, T. Calculation of flow and bed deformation with a general nonorthogonal coordinates system. Proc. XXIV IAHR Congr. Madr. Spain 1991, C-2, 241–248. [Google Scholar]
- Takahashi, T.; Satofuka, Y. Simulation model for channel variation braided channel reach. Ann. Disaster Prev. Res. Inst. Kyoto Univ. 1999, B-2, 189–200. [Google Scholar]
- Koike, T.; Sato, G.; Komori, J. Implementation of the global-scale earth issues international science and technology cooperation program and towards the social implementation of research achievements. J. Jpn. Soc. Landslide Sci. 2021, 58, 193–195. [Google Scholar] [CrossRef]
- Zhang, G.; Yao, T.; Xie, H.; Wang, W.; Yang, W. An inventory of glacial lakes in the third pole region and their changes in response to global warming. Glob. Planet. Chang. 2015, 131, 148–157. [Google Scholar] [CrossRef]

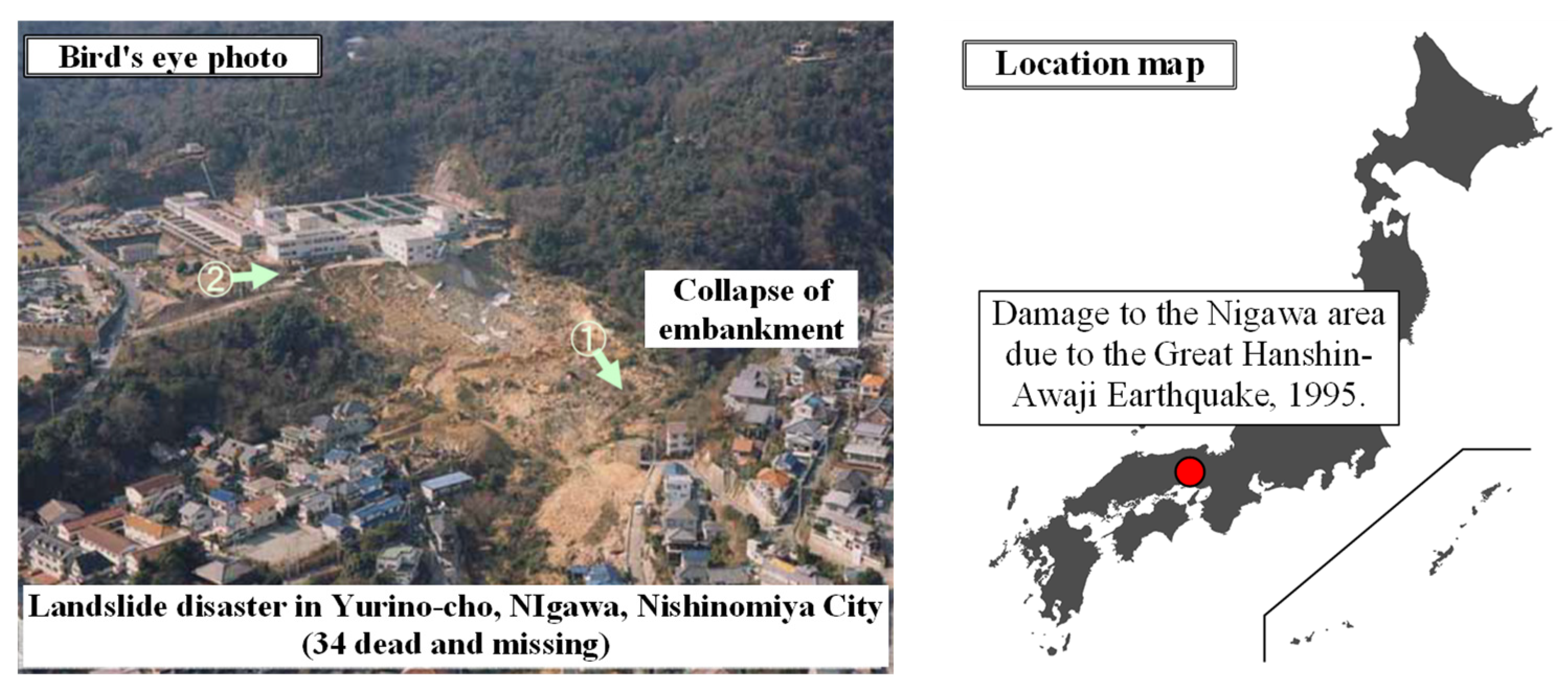

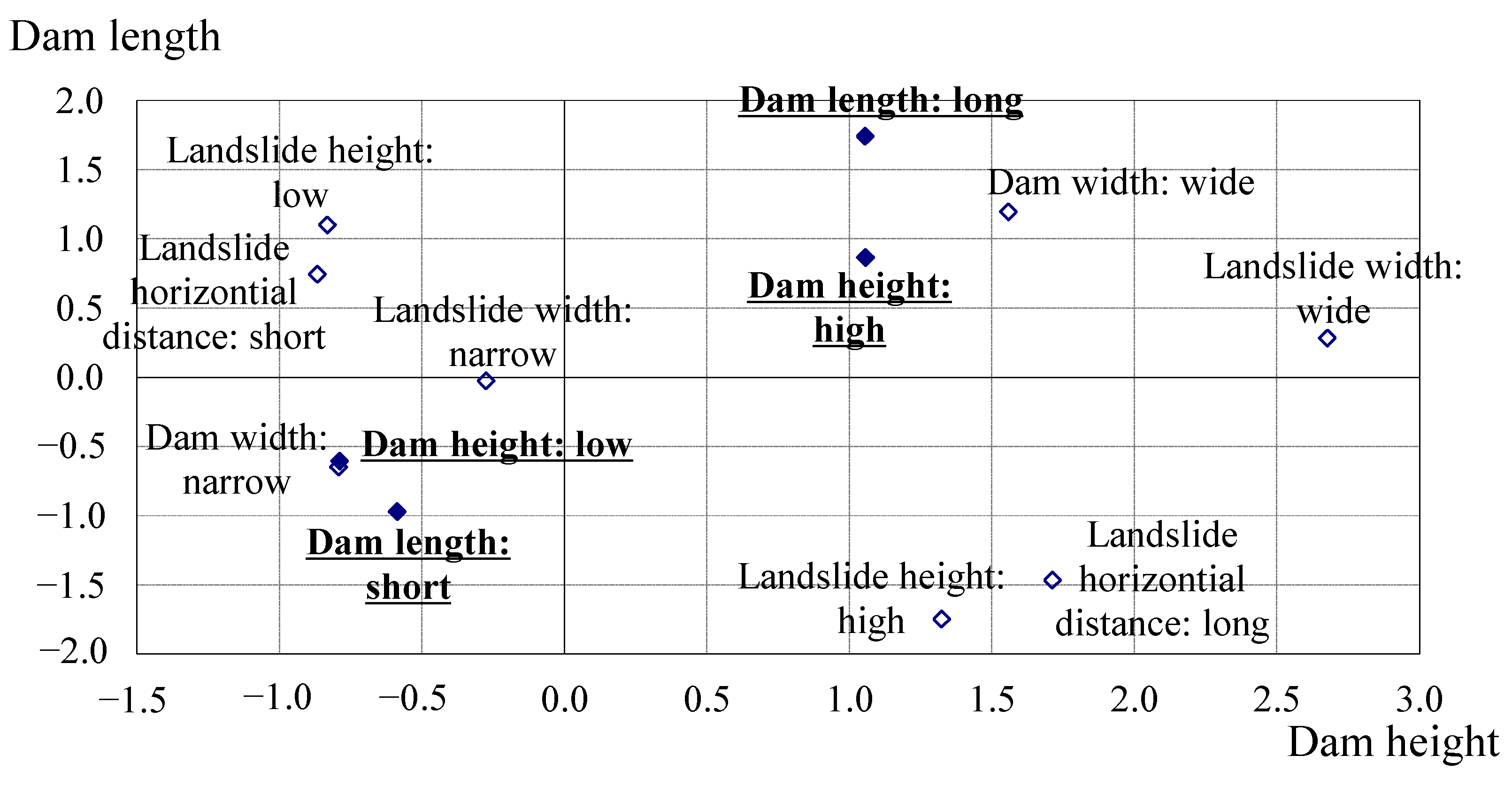



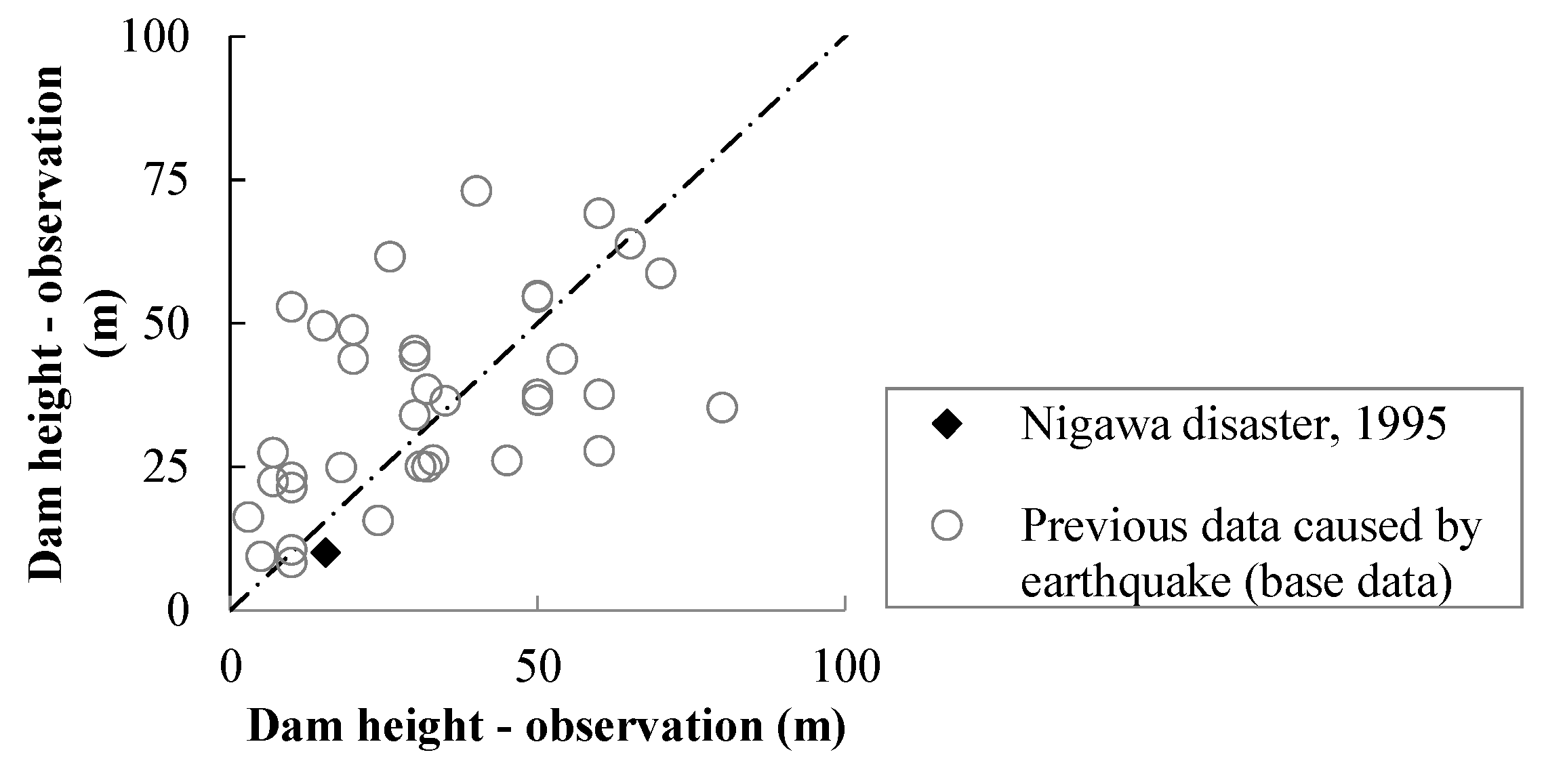
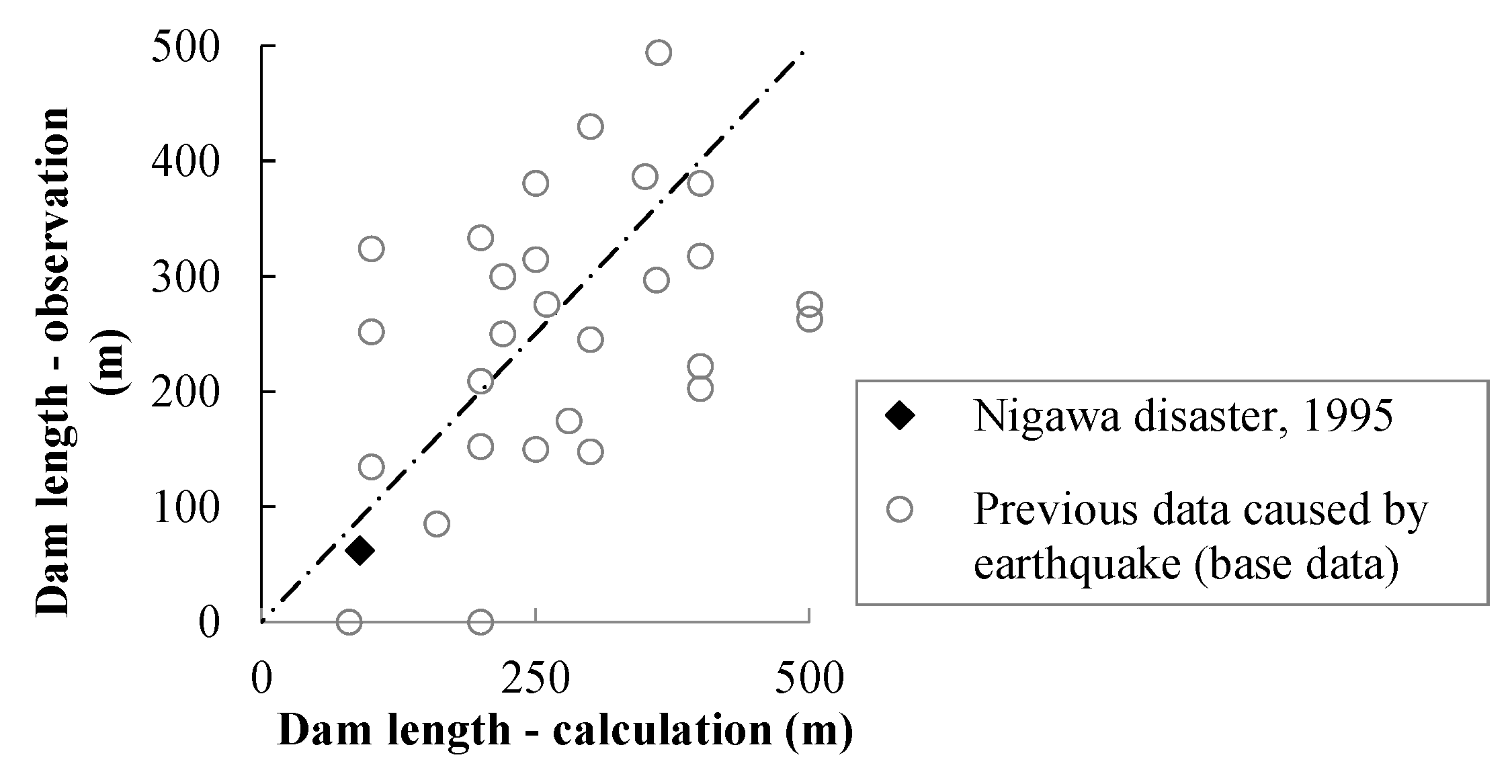


| α1 | β1 | γ1 | δ1 | |
|---|---|---|---|---|
| Earthquake Hearthquake | 0.11 | 0.014 | 0.032 | 0.039 |
| Heavy rain Hheavy_rain | 0.081 | 0.0039 | 0.063 | 0.35 |
| α2 | β2 | γ2 | ε2 | |
| Earthquake Learthquake | 0.27 | −0.0043 | 0.98 | 0.0001 |
| Heavy rain Lheavy_rain | 0.44 | 0.012 | 0.23 | 0.51 |
| h1 | l1 | w1 | A1 | B1 | |
|---|---|---|---|---|---|
| Nigawa-disaster, 1995 | ~50 m | ~150 m | 20–50 m | ~10,000 m2 (/100,000) | ~100 m |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Harada, N.; Satofuka, Y.; Mizuyama, T. The Impacts of River Channel Blockages Caused by Sliding Embankment Collapses during Earthquakes. Water 2024, 16, 822. https://doi.org/10.3390/w16060822
Harada N, Satofuka Y, Mizuyama T. The Impacts of River Channel Blockages Caused by Sliding Embankment Collapses during Earthquakes. Water. 2024; 16(6):822. https://doi.org/10.3390/w16060822
Chicago/Turabian StyleHarada, Norio, Yoshifumi Satofuka, and Takahisa Mizuyama. 2024. "The Impacts of River Channel Blockages Caused by Sliding Embankment Collapses during Earthquakes" Water 16, no. 6: 822. https://doi.org/10.3390/w16060822
APA StyleHarada, N., Satofuka, Y., & Mizuyama, T. (2024). The Impacts of River Channel Blockages Caused by Sliding Embankment Collapses during Earthquakes. Water, 16(6), 822. https://doi.org/10.3390/w16060822





