Modeling of Water Resource Dynamics in SUR Systems Through Generalized Conformable Calculus in Arid Sites in Mexico
Abstract
1. Introduction
Strategic Models for Managing Water Resources
2. Methods
- 1.
- .
- 2.
- .
- 3.
- .
- 4.
- .
- 5.
- .
SUR Model and Conformable Mathematical Extensions
- and .
- is continuous in .
- is differentiable in .
3. Dynamic Systems Analysis Through Conformable Functions and Autonomous Models
3.1. Parameter Fitting
3.2. Selection of Conformable Functions
- Modified Sinusoidal Function: The function , defined on , has been included due to its ability to capture oscillatory phenomena and its injectivity in (it is important to note that since , this function tends to zero only when ).
- Exponential Function: The function , defined on , is included for its capacity to describe exponential growth and decay, which is fundamental in dynamic systems modeling.
- Quadratic Function: The function , defined on , is especially useful for capturing quadratic and nonlinear behaviors in dynamic systems.
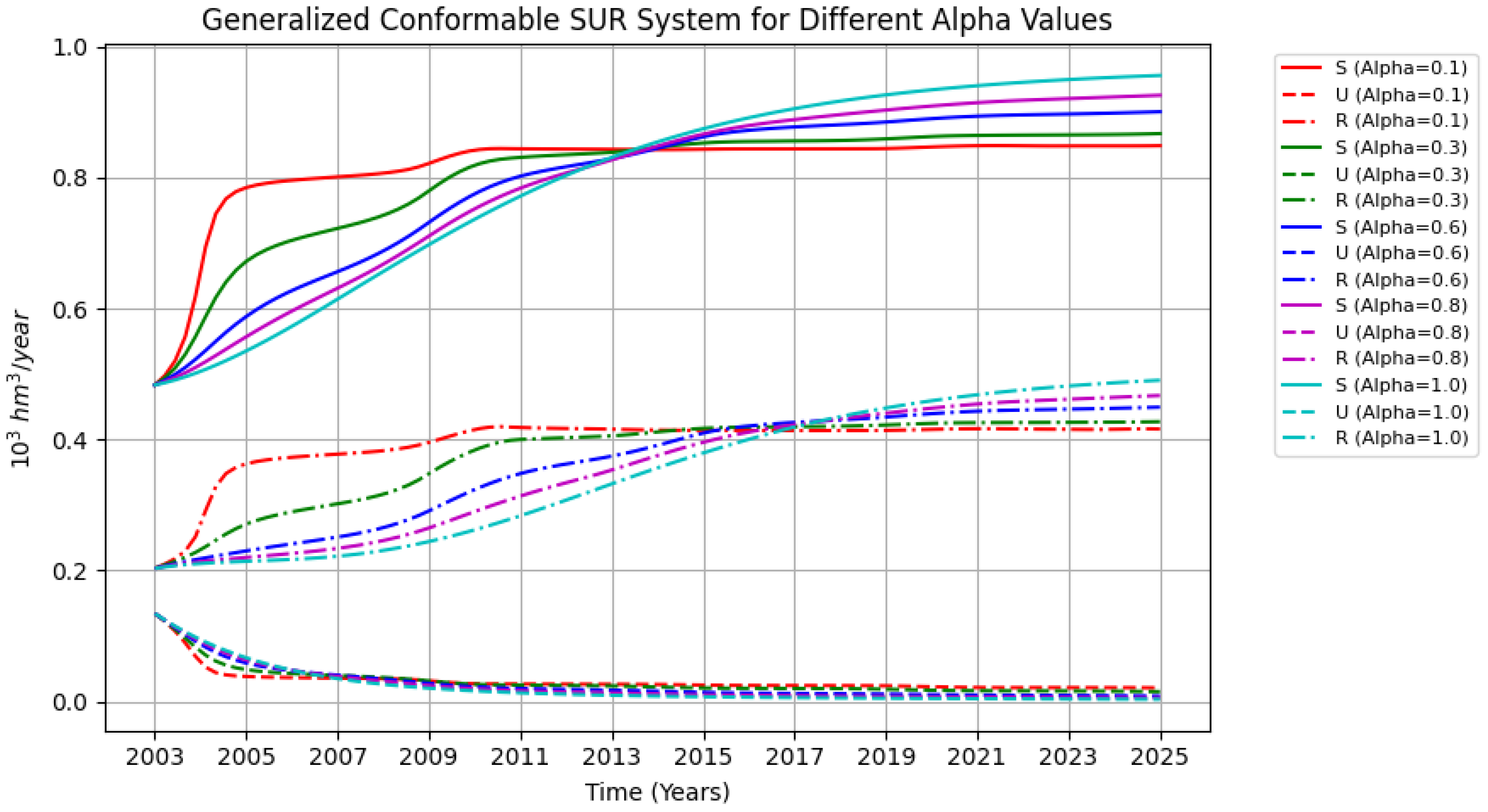
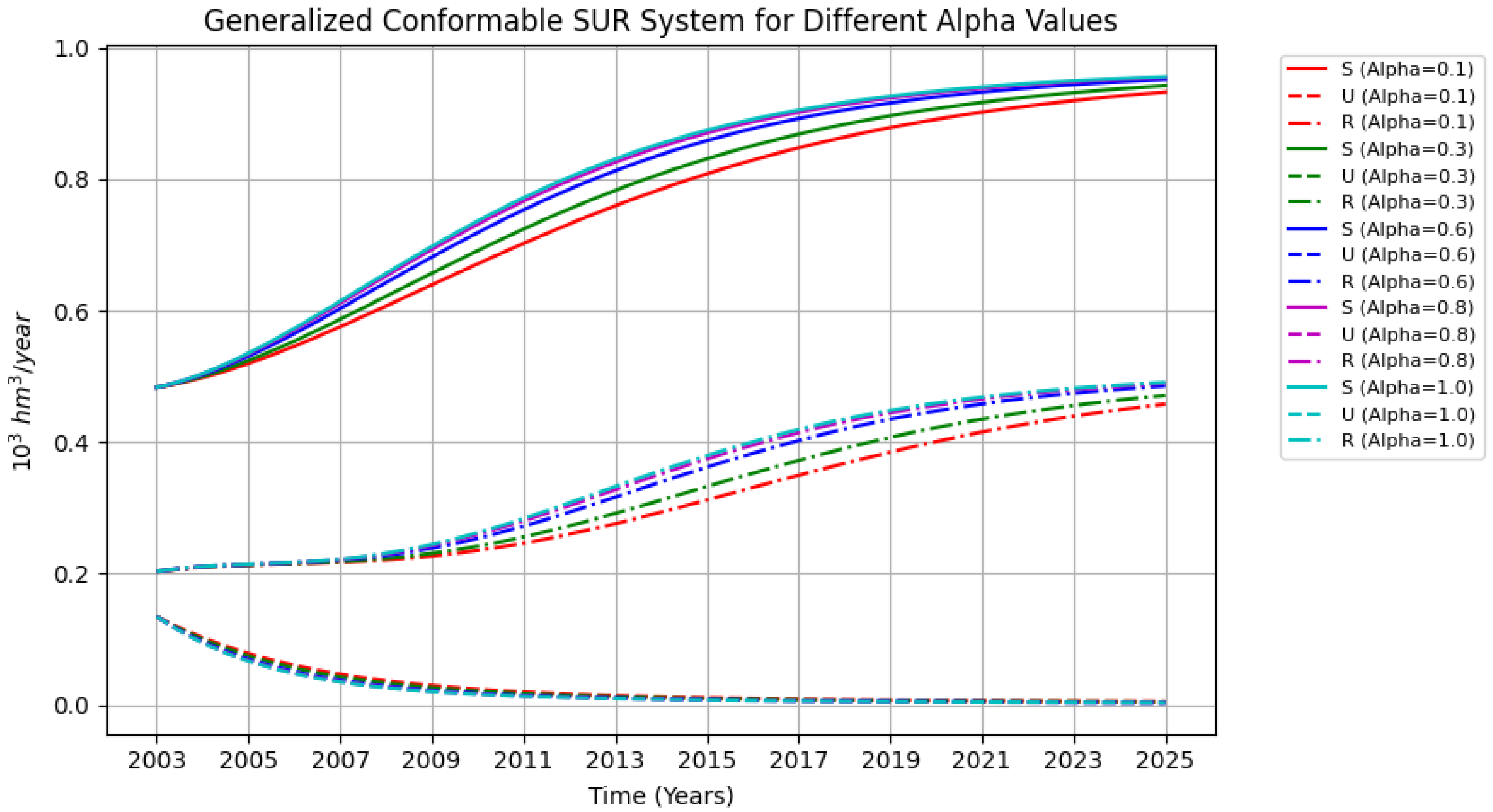
3.3. Homogeneous Case 1
3.4. Homogeneous Case 2
3.5. Homogeneous Case 3
3.6. Study of Equilibrium and Stability of the Model
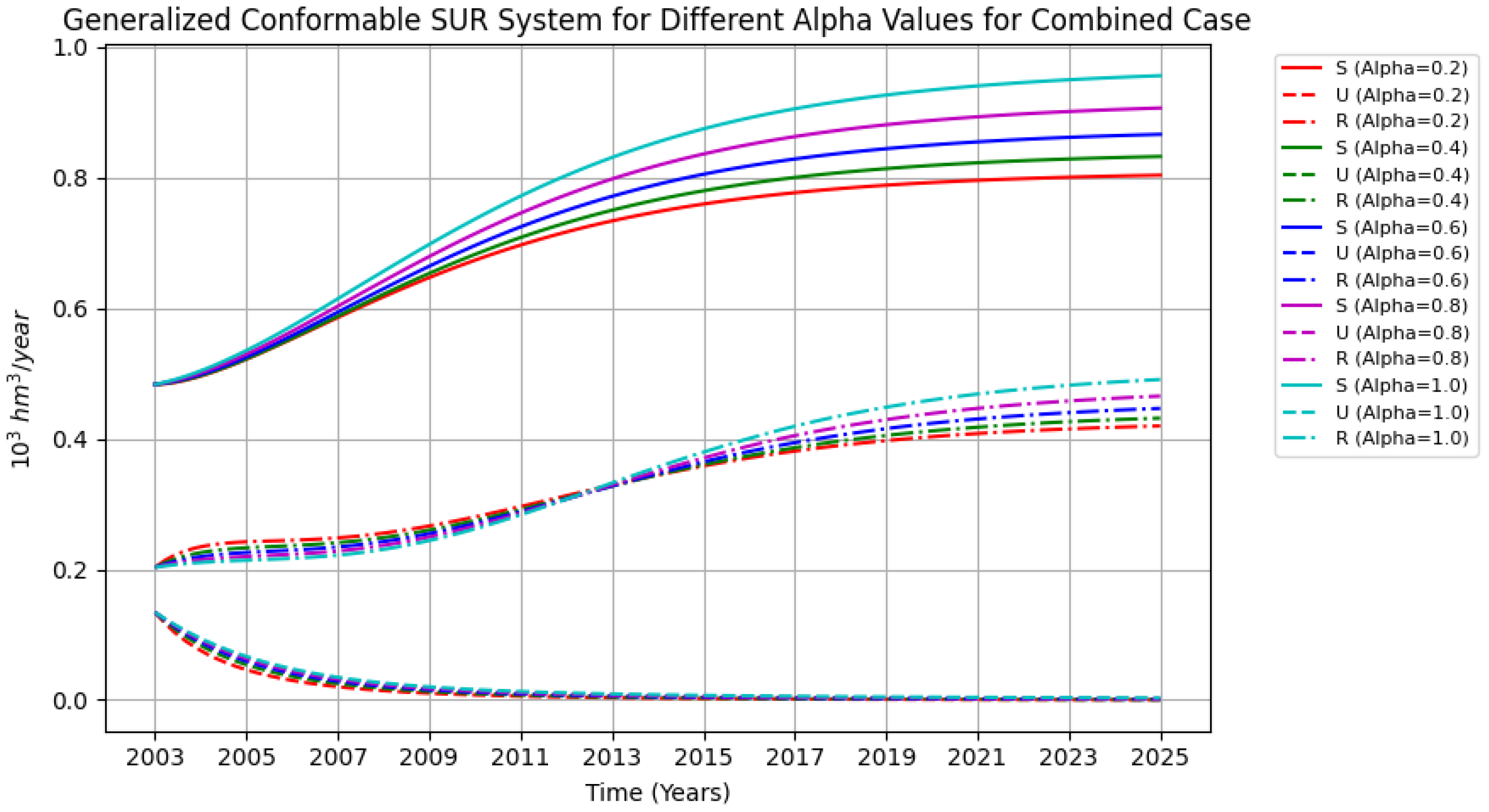
3.7. Combined Case
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zewde, N.T.; Denboba, M.A.; Tadesse, S.A.; Getahun, Y.S. Predicting runoff and sediment yields using soil and water assessment tool (SWAT) model in the Jemma Subbasin of Upper Blue Nile, Central Ethiopia. Environ. Chall. 2024, 14, 100806. [Google Scholar] [CrossRef]
- Huang, Y.P.; Tsai, H.P.; Chiang, L.C. Integration of UAV Digital Surface Model and HEC-HMS Hydrological Model System in iRIC Hydrological Simulation—A Case Study of Wu River. Drones 2024, 8, 178. [Google Scholar] [CrossRef]
- El-Bagoury, H.; Gad, A. Integrated hydrological modeling for watershed analysis, flood prediction, and mitigation using meteorological and morphometric data, SCS-CN, HEC-HMS/RAS, and QGIS. Water 2024, 16, 356. [Google Scholar] [CrossRef]
- Gutiérrez-Corona, J.N.; Itzá-Ortiz, B.A.; Torres-Mendoza, A.; Tzatchkov, V.G.; Quezada-Téllez, L.A. Mathematical modeling for water supply by means of natural supply sources: The case of Pachuca de Soto, Hidalgo. Sustain. Water Resour. Manag. 2024, 10, 63. [Google Scholar] [CrossRef]
- Song, L. Dynamic Modeling and Simulation of Option Pricing Based on Fractional Diffusion Equations with Double Derivatives. Comput. Econ. 2024, 1–21. [Google Scholar] [CrossRef]
- López Gunn, E.; Rica, M.; Zugasti, I.; Hernaez, O.; Pulido-Velazquez, M.; Sanchis-Ibor, C. Use of the DELPHI method to assess the potential role of Enhanced Information Systems in Mediterranean groundwater management and governance. Water Policy 2024, wp2024033. [Google Scholar] [CrossRef]
- Lin, C.Y.; Alegria, M.E.O.; Dhakal, S.; Zipper, S.; Marston, L. PyCHAMP: A crop-hydrological-agent modeling platform for groundwater management. Environ. Model. Softw. 2024, 181, 106187. [Google Scholar] [CrossRef]
- Hassanzadeh, A.; Vázquez-Suñé, E.; Valdivielso, S.; Corbella, M. WaterpyBal: A comprehensive open-source python library for groundwater recharge assessment and water balance modeling. Environ. Model. Softw. 2024, 172, 105934. [Google Scholar] [CrossRef]
- Kenway, S.; Gregory, A.; McMahon, J. Urban water mass balance analysis. J. Ind. Ecol. 2011, 15, 693–706. [Google Scholar] [CrossRef]
- Gogo-Abite, I.; Chopra, M.; Wanielista, M. Integrated Surface–Groundwater Model for Storm-Water Harvesting Using Basic Mass Balance Principles. J. Irrig. Drain. Eng. 2013, 139, 55–65. [Google Scholar] [CrossRef]
- Bear, J.; Verruijt, A. Modeling Groundwater Flow and Pollution; Springer: Dordrecht, The Netherlands, 1987. [Google Scholar]
- Bear, J.; Cheng, A.H.D. Modeling Groundwater Flow and Contaminant Transport; Springer Science & Business Media: Berlin, Germany, 2010. [Google Scholar]
- Ntona, M.M.; Busico, G.; Mastrocicco, M.; Kazakis, N. Modeling groundwater and surface water interaction: An overview of current status and future challenges. Sci. Total Environ. 2022, 846, 157355. [Google Scholar] [CrossRef]
- Bhadula, R.C.; Pokhariyal, G.P.; Sisodia, M.; Mamgain, K.; Agarwal, I.; Bahuguna, A. Mathematical Model of Artificial Groundwater Recharge. Res. Sq. 2024. preprint. [Google Scholar] [CrossRef]
- Zhang, J.; Liang, X.; Zhang, Y.K.; Chen, X.; Ma, E.; Schilling, K. Groundwater responses to recharge and flood in riparian zones of layered aquifers: An analytical model. J. Hydrol. 2022, 614, 128547. [Google Scholar] [CrossRef]
- Celaya, S.; Fuente, I.; Rábago, D.; Quindós, L.; Sainz, C. Application of a mathematical model to an artificial aquifer under different recharge/discharge conditions using 222Rn as a tracer. Groundw. Sustain. Dev. 2022, 17, 100753. [Google Scholar] [CrossRef]
- Agnieszka, T. Numerical modeling and rational methods of water supply network operations in environmental engineering systems. Appl. Water Sci. 2023, 13, 18. [Google Scholar]
- Conejos Fuertes, P.; Martínez Alzamora, F.; Hervás Carot, M.; Alonso Campos, J. Building and exploiting a Digital Twin for the management of drinking water distribution networks. Urban Water J. 2020, 17, 704–713. [Google Scholar] [CrossRef]
- Keyhanpour, M.J.; Jahromi, S.H.M.; Ebrahimi, H. System dynamics model of sustainable water resources management using the Nexus Water-Food-Energy approach. Ain Shams Eng. J. 2021, 12, 1267–1281. [Google Scholar] [CrossRef]
- Haque, A.; Salama, A.; Lo, K.; Wu, P. Surface and groundwater interactions: A review of coupling strategies in detailed domain models. Hydrology 2021, 8, 35. [Google Scholar] [CrossRef]
- Nagata, K.; Shoji, I.; Arima, T.; Otsuka, T.; Kato, K.; Matsubayashi, M.; Omura, M. Practicality of integrated water resources management (IWRM) in different contexts. Int. J. Water Resour. Dev. 2022, 38, 897–919. [Google Scholar] [CrossRef]
- Diaz, M.; Sinicyn, G.; Grodzka-Łukaszewska, M. Modelling of groundwater–surface water interaction applying the hyporheic flux model. Water 2020, 12, 3303. [Google Scholar] [CrossRef]
- Nie, X.; Fan, T.; Wang, B.; Li, Z.; Shankar, A.; Manickam, A. Big data analytics and IoT in operation safety management in under water management. Comput. Commun. 2020, 154, 188–196. [Google Scholar] [CrossRef]
- INEGI. Climatología. 2024. Available online: https://www.inegi.org.mx/temas/climatologia/ (accessed on 11 November 2024).
- Khalil, R.; Al Horani, M.; Yousef, A.; Sababheh, M. A new definition of fractional derivative. J. Comput. Appl. Math. 2014, 264, 65–70. [Google Scholar] [CrossRef]
- Gateaux, R. Sur les fonctionnelles continues et les fonctionnelles analytiques. CR Acad. Sci. Paris 1913, 157, 65. [Google Scholar]
- Zhao, D.; Luo, M. General conformable fractional derivative and its physical interpretation. Calcolo 2017, 54, 903–917. [Google Scholar] [CrossRef]
- Smith, A.B.; Johnson, T.E.; Martinez, C.J. Understanding Surface Water Dynamics in Changing Environments. Water Resour. Res. 2021, 57, e2020WR028476. [Google Scholar] [CrossRef]
- Fleckenstein, J.H.; Krause, S.; Hannah, D.M. Groundwater–Surface Water Interactions: Recent Advances and Interdisciplinary Challenges. Water 2020, 12, 291. [Google Scholar]
- McDonald, J.A.; Newell, C.J.; Adamson, D.T.; Stroo, H.F. Modeling PFAS Fate and Transport in Groundwater, With and Without Precursor Transformation. Groundwater 2021, 59, 645–658. [Google Scholar]
- Ríos-Sánchez, K.I.; Chamizo-Checa, S.; Galindo-Castillo, E.; Acevedo-Sandoval, O.A.; González-Ramírez, C.A.; Hernández-Flores, M.d.l.L.; Otazo-Sánchez, E.M. The Groundwater Management in the Mexico Megacity Peri-Urban Interface. Sustainability 2024, 16, 4801. [Google Scholar] [CrossRef]
- Galindo, E.; Otazo, E.M.; Reyes, L.R.; Arellano, S.M.; Gordillo, A.; González, C.A. Balance hídrico y afectaciones a la recarga para el año 2021 en el acuífero Cuautitlán Pachuca. GeoFocus. Int. Rev. Geogr. Inf. Sci. Technol. 2010, 10, 65–90. [Google Scholar]
- Comisión Nacional del Agua. Sistema Nacional de Información del Agua (SINA). 2024. Available online: https://www.gob.mx/conagua/acciones-y-programas/sistema-nacional-de-informacion-del-agua-sina (accessed on 11 December 2024).

| Parameters | ||||||||
|---|---|---|---|---|---|---|---|---|
| 0.14886328 | 0.14639151 | 0.14391975 | 0.14144799 | 0.13897623 | 0.13650446 | 0.13156094 | 0.12661742 | |
| −0.20284103 | −0.21271435 | −0.22258766 | −0.23246098 | −0.2423343 | −0.25220762 | −0.27195425 | −0.29170089 | |
| 0.00084089 | 0.00080652 | 0.00077214 | 0.00073777 | 0.00070339 | 0.00066902 | 0.00060027 | 0.00053152 | |
| −0.29854531 | −0.38507868 | −0.52330165 | −0.77925168 | −1.41489504 | −5.70163156 | 1.29597977 | 0.6287696 | |
| 0.0008847 | 0.00089837 | 0.00091374 | 0.00093116 | 0.00095106 | 0.00097402 | 0.00103243 | 0.00111672 | |
| 0.37523903 | 0.38367073 | 0.39280021 | 0.40271782 | 0.41353024 | 0.42536427 | 0.45273679 | 0.48648716 | |
| 0.53066379 | 0.55362582 | 0.57658785 | 0.59954988 | 0.62251191 | 0.64547394 | 0.69139801 | 0.73732207 | |
| −0.19895248 | −0.19098379 | −0.1830151 | −0.17504641 | −0.16707771 | −0.15910902 | −0.14317164 | −0.12723425 | |
| 0.23789292 | 0.26443861 | 0.2909843 | 0.31753 | 0.34407569 | 0.37062138 | 0.42371277 | 0.47680415 |
| Parameters | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 0.31012 | 0.28074 | 0.25414 | 0.23007 | 0.20827 | 0.18854 | 0.17068 | 0.15451 | 0.13987 | 0.12662 | |
| −0.71435 | −0.64668 | −0.58542 | −0.52997 | −0.47977 | −0.43432 | −0.39318 | −0.35594 | −0.32222 | −0.29170 | |
| 0.00130 | 0.00118 | 0.00107 | 0.00097 | 0.00087 | 0.00079 | 0.00072 | 0.00065 | 0.00059 | 0.00053 | |
| 0.62802 | 0.62810 | 0.62819 | 0.62827 | 0.62835 | 0.62844 | 0.62852 | 0.62860 | 0.62869 | 0.62877 | |
| 0.00112 | 0.00112 | 0.00112 | 0.00112 | 0.00112 | 0.00112 | 0.00112 | 0.00112 | 0.00112 | 0.00112 | |
| 0.48683 | 0.48679 | 0.48675 | 0.48671 | 0.48668 | 0.48664 | 0.48660 | 0.48656 | 0.48652 | 0.48649 | |
| 1.80808 | 1.63656 | 1.48132 | 1.34080 | 1.21361 | 1.09849 | 0.99428 | 0.89997 | 0.81459 | 0.73732 | |
| −0.31129 | −0.28183 | −0.25516 | −0.23102 | −0.20916 | −0.18936 | −0.17145 | −0.15522 | −0.14053 | −0.12723 | |
| 1.16959 | 1.05861 | 0.95815 | 0.86723 | 0.78494 | 0.71046 | 0.64304 | 0.58202 | 0.52679 | 0.47680 |
| Parameters | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 461,105 | 409,871 | 358,637 | 307,404 | 256,170 | 204,936 | 153,702 | 102,468 | 51,234 | 0.12662 | |
| −1,061,922 | −943,931 | −825,940 | −707,948 | −589,957 | −471,966 | −353,974 | −235,983 | −117,992 | −0.29170 | |
| 1931 | 1716 | 1502 | 1287 | 1073 | 858.13761 | 643.60334 | 429.06907 | 214.53480 | 0.00053 | |
| 0.62710 | 0.62710 | 0.62710 | 0.62710 | 0.62710 | 0.62710 | 0.62710 | 0.62710 | 0.62710 | 0.62877 | |
| 0.00112 | 0.00112 | 0.00112 | 0.00112 | 0.00112 | 0.00112 | 0.00112 | 0.00112 | 0.00112 | 0.00112 | |
| 0.48724 | 0.48724 | 0.48724 | 0.48724 | 0.48724 | 0.48724 | 0.48724 | 0.48724 | 0.48724 | 0.48649 | |
| 2,692,333 | 2,393,185 | 2,094,037 | 1,794,890 | 1,495,741 | 1,196,593 | 897,445 | 598,297 | 299,149 | 0.73732 | |
| −462,223 | −410,865 | −359,507 | −308,149 | −256,791 | −205,433 | −154,074 | −102,716 | −51,358 | −0.12723 | |
| 1,742,245 | 1,548,662 | 1,355,080 | 1,161,497 | 967,914 | 774,331 | 580,749 | 387,166 | 193,583 | 0.47680 |
| Point | Eigenvalue | Type of Point |
|---|---|---|
| 0.0011, 0.5130, −1.5579 | Saddle | |
| −0.0011, 0.5130, −1.5579 | Saddle | |
| −9.7851, −5.1296, −0.9852 | Attractor | |
| −9.8064, −5.1296, −0.9852 | Attractor |
| Parameters | |||||
|---|---|---|---|---|---|
| 20,493.71 | 15,370.35 | 10,246.94 | 5123.528 | 0.126618 | |
| −47,196.8 | −35,397.7 | −23,598.6 | −11,799.4 | −0.2917 | |
| 85.80082 | 64.35074 | 42.90753 | 21.45403 | 0.000531 | |
| −0.18249 | −0.49781 | −4.43568 | 1.162429 | 0.62877 | |
| 0.000943 | 0.000969 | 0.001003 | 0.00105 | 0.001117 | |
| 0.404317 | 0.419543 | 0.437649 | 0.459535 | 0.486488 | |
| 119,659.9 | 89,745.18 | 59,830.33 | 29,915.54 | 0.737322 | |
| −20,543.4 | −15,407.6 | −10,271.8 | −5135.94 | −0.12723 | |
| 77,433.47 | 58,075.23 | 38,716.95 | 19,358.73 | 0.476803 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gutierrez-Corona, J.N.; Quezada-Téllez, L.A.; Torres-Mendoza, A.; Fernandez-Anaya, G. Modeling of Water Resource Dynamics in SUR Systems Through Generalized Conformable Calculus in Arid Sites in Mexico. Water 2024, 16, 3691. https://doi.org/10.3390/w16243691
Gutierrez-Corona JN, Quezada-Téllez LA, Torres-Mendoza A, Fernandez-Anaya G. Modeling of Water Resource Dynamics in SUR Systems Through Generalized Conformable Calculus in Arid Sites in Mexico. Water. 2024; 16(24):3691. https://doi.org/10.3390/w16243691
Chicago/Turabian StyleGutierrez-Corona, Josué Neftalí, Luis Alberto Quezada-Téllez, Arturo Torres-Mendoza, and Guillermo Fernandez-Anaya. 2024. "Modeling of Water Resource Dynamics in SUR Systems Through Generalized Conformable Calculus in Arid Sites in Mexico" Water 16, no. 24: 3691. https://doi.org/10.3390/w16243691
APA StyleGutierrez-Corona, J. N., Quezada-Téllez, L. A., Torres-Mendoza, A., & Fernandez-Anaya, G. (2024). Modeling of Water Resource Dynamics in SUR Systems Through Generalized Conformable Calculus in Arid Sites in Mexico. Water, 16(24), 3691. https://doi.org/10.3390/w16243691








