Analysis of Fill Dam Using Finite Element Method and Comparison with Monitoring Results
Abstract
1. Introduction
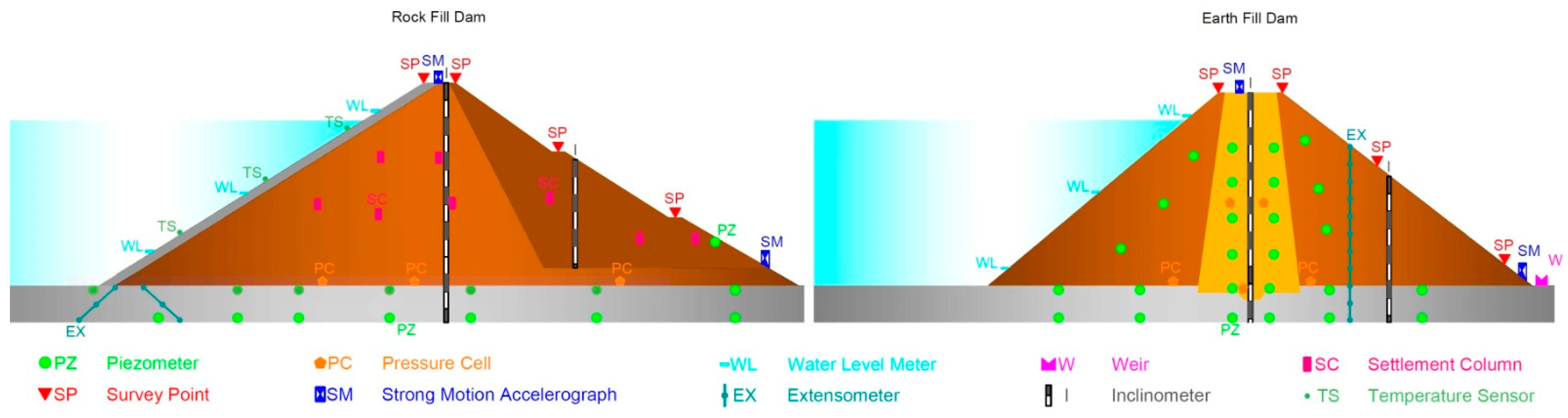
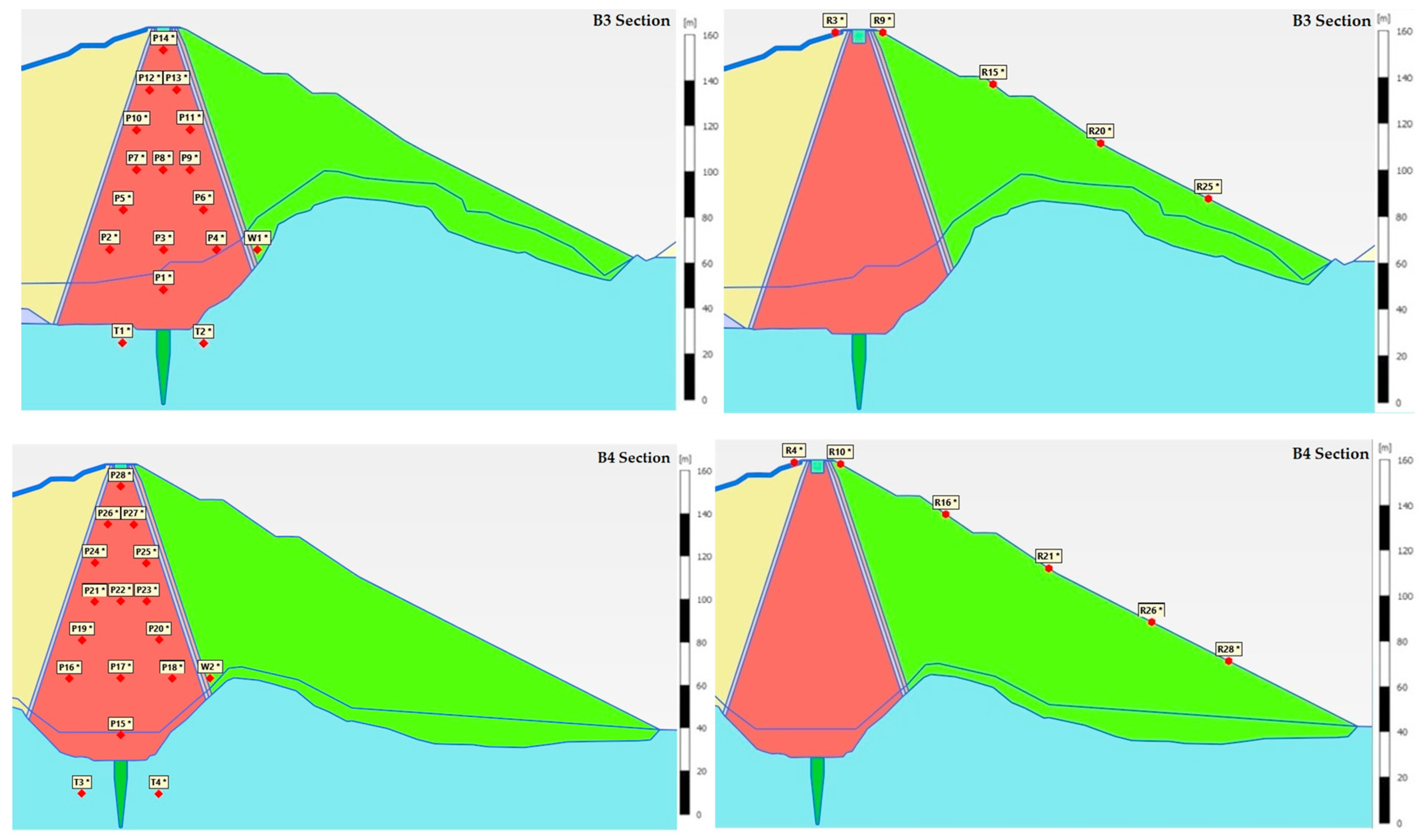
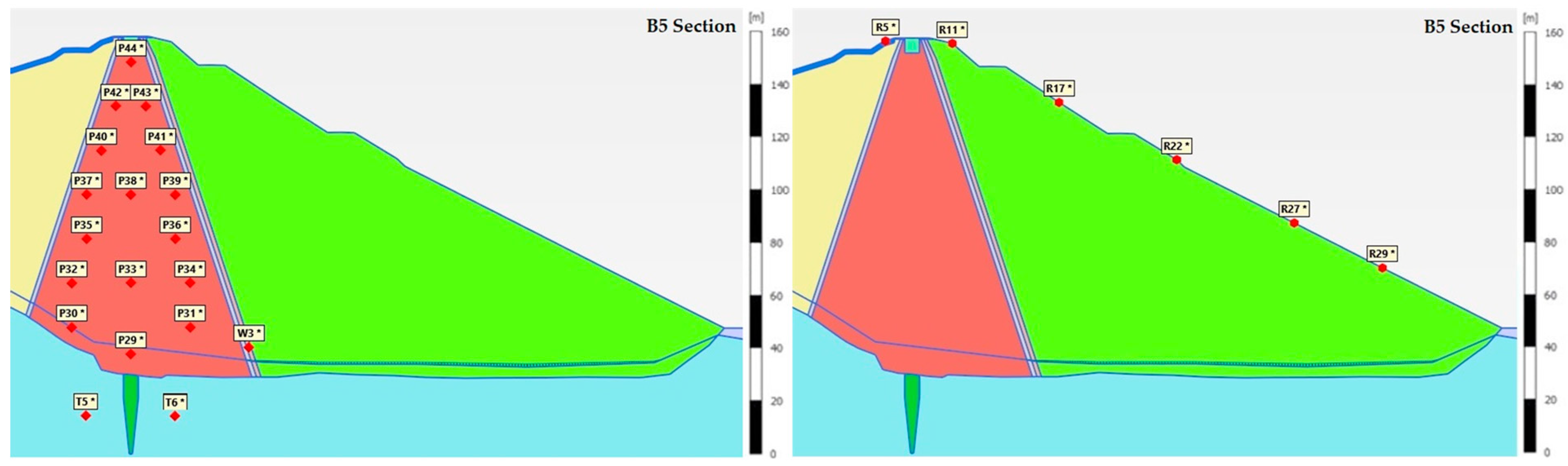
2. Monitoring and Inspection of Fill Dams
2.1. Piezometers
2.2. Survey Points
2.3. Extensometers
2.4. Water Pressure Meters
2.5. Inclinometers
2.6. Settlement Meters
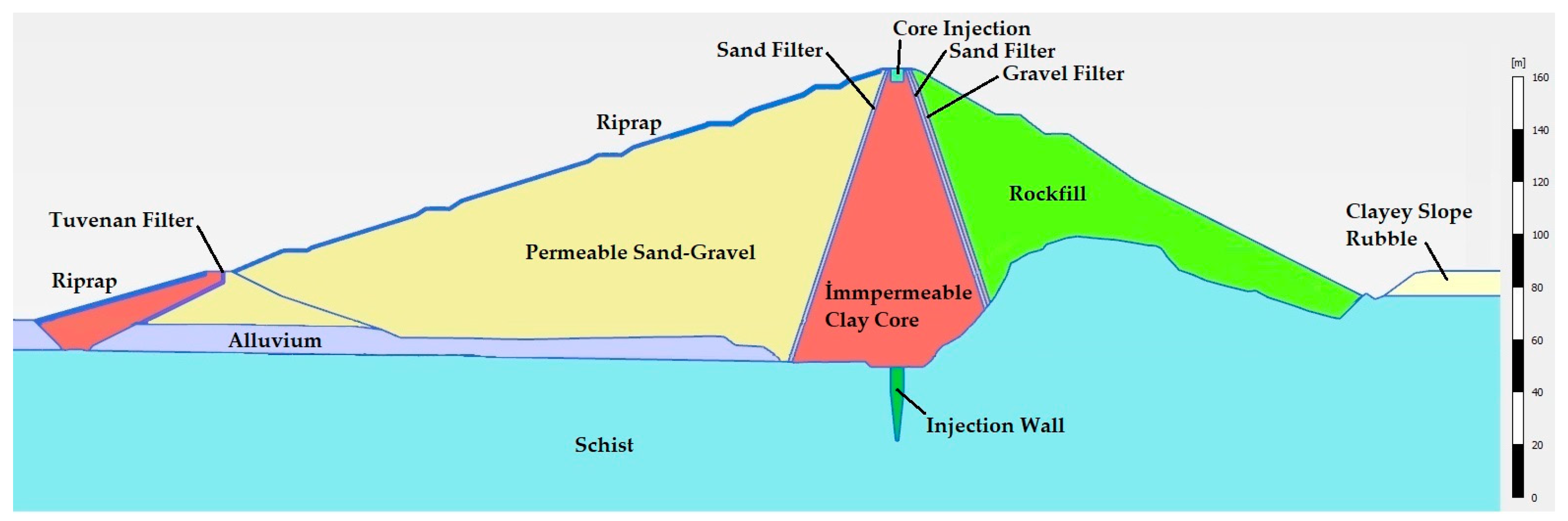
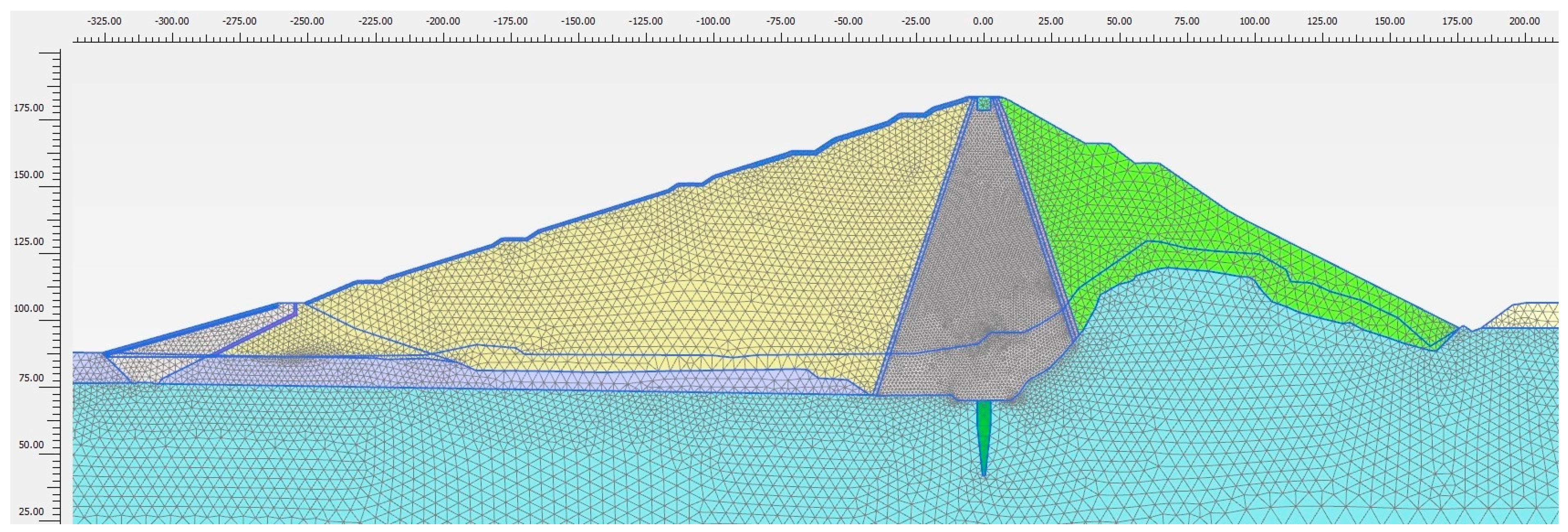
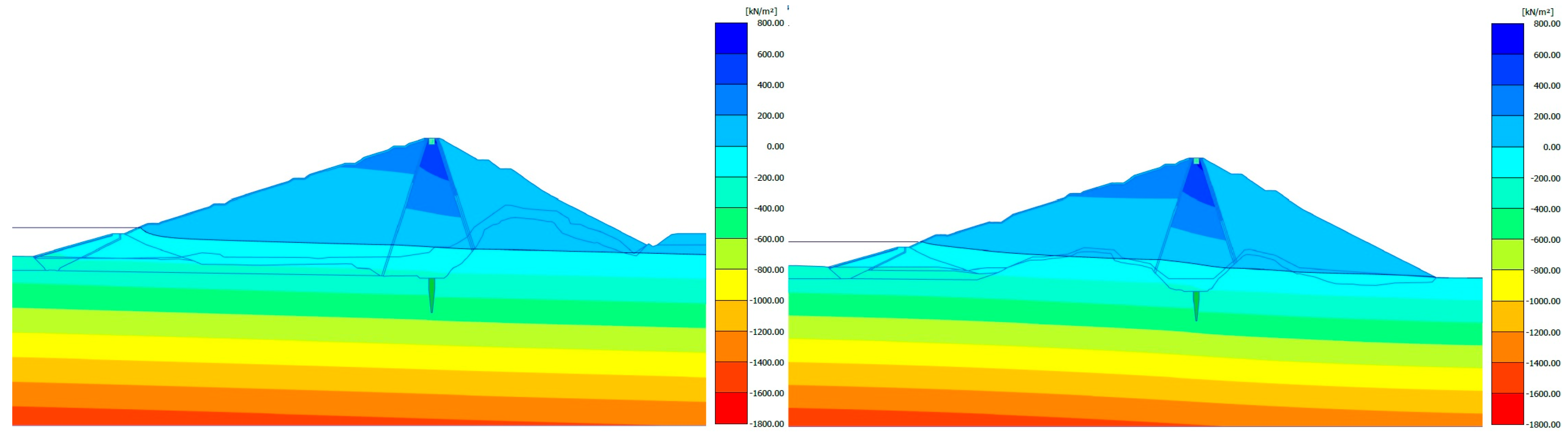
3. Method and Application
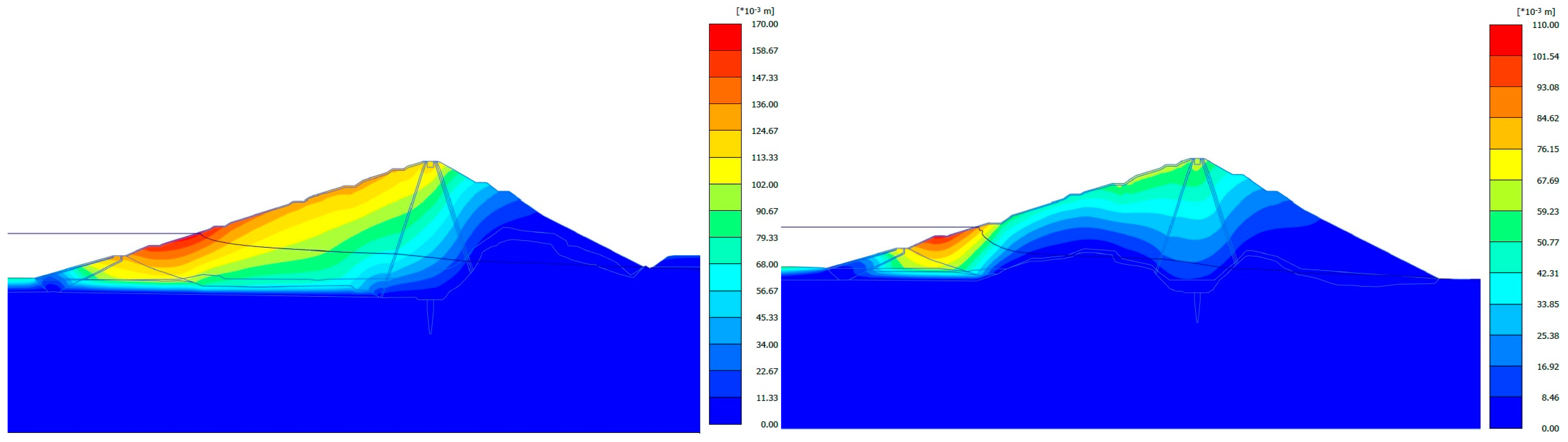
4. Analysis Results
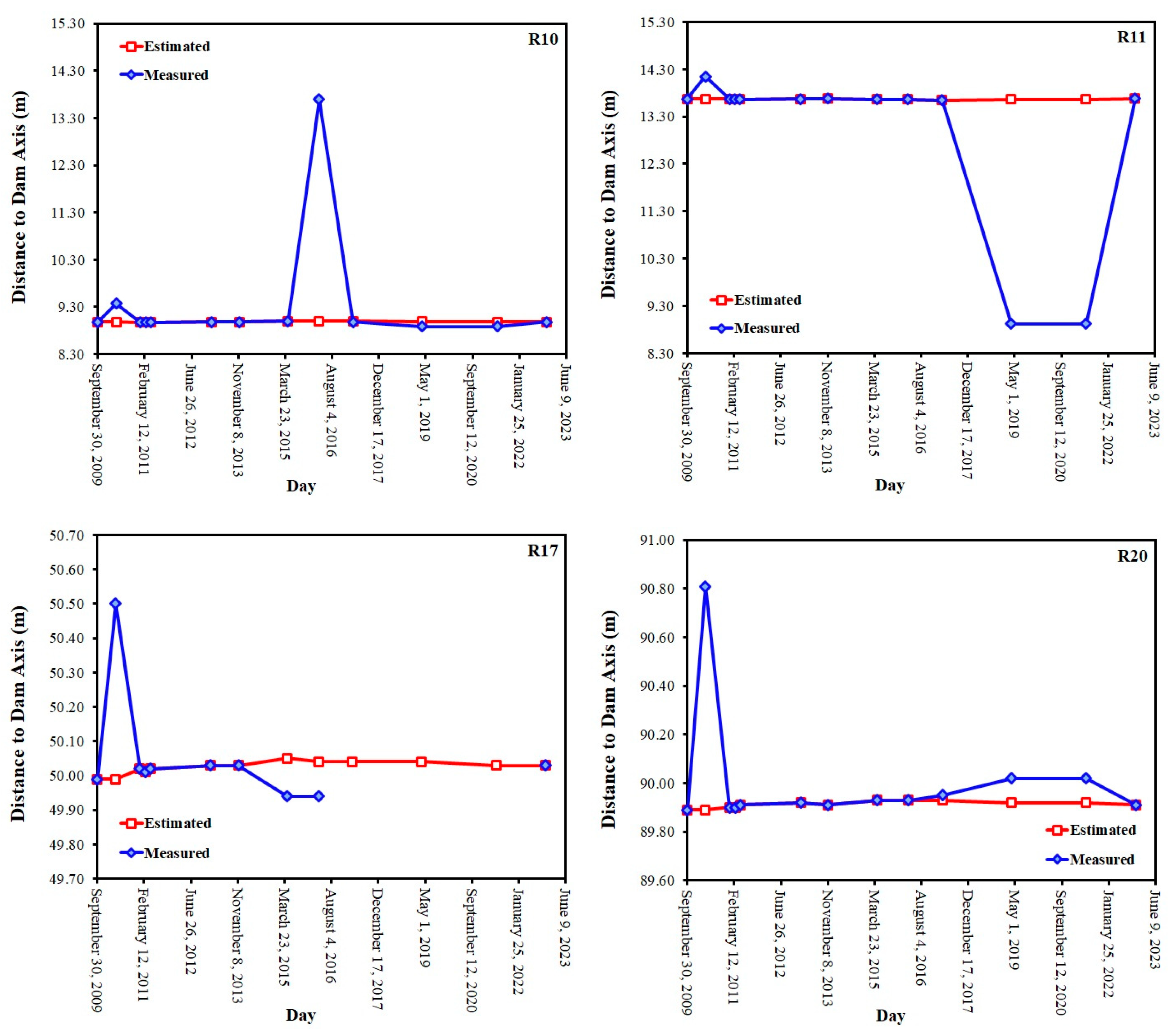
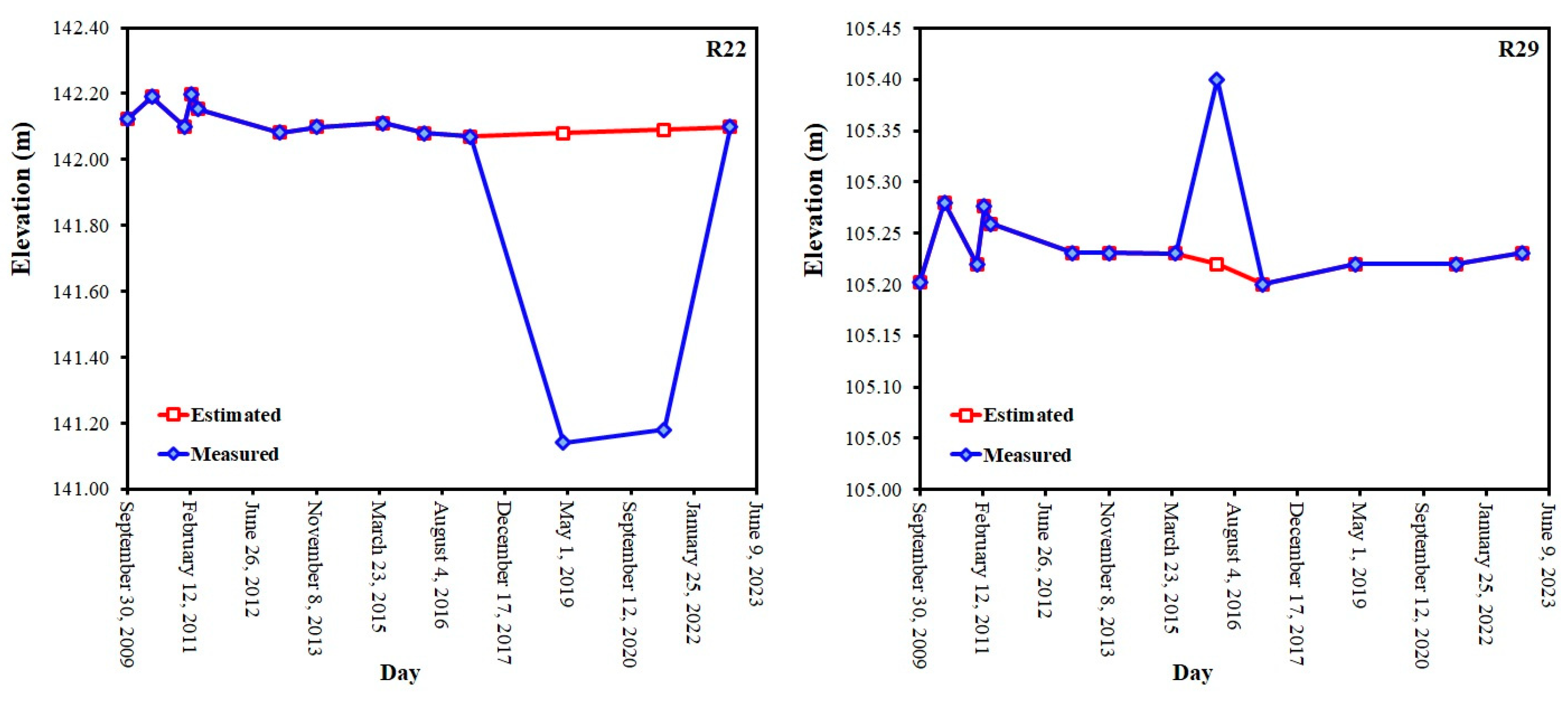
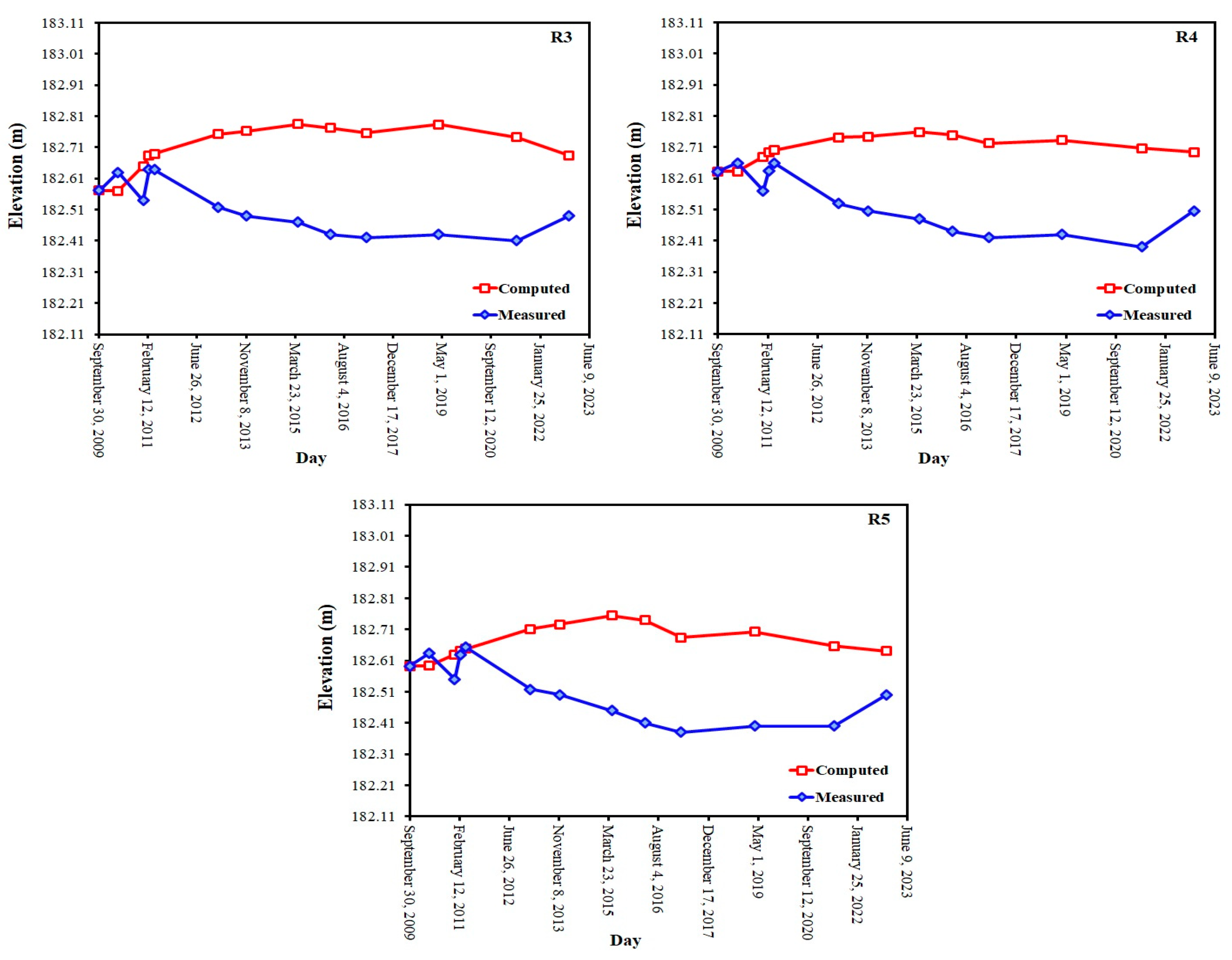
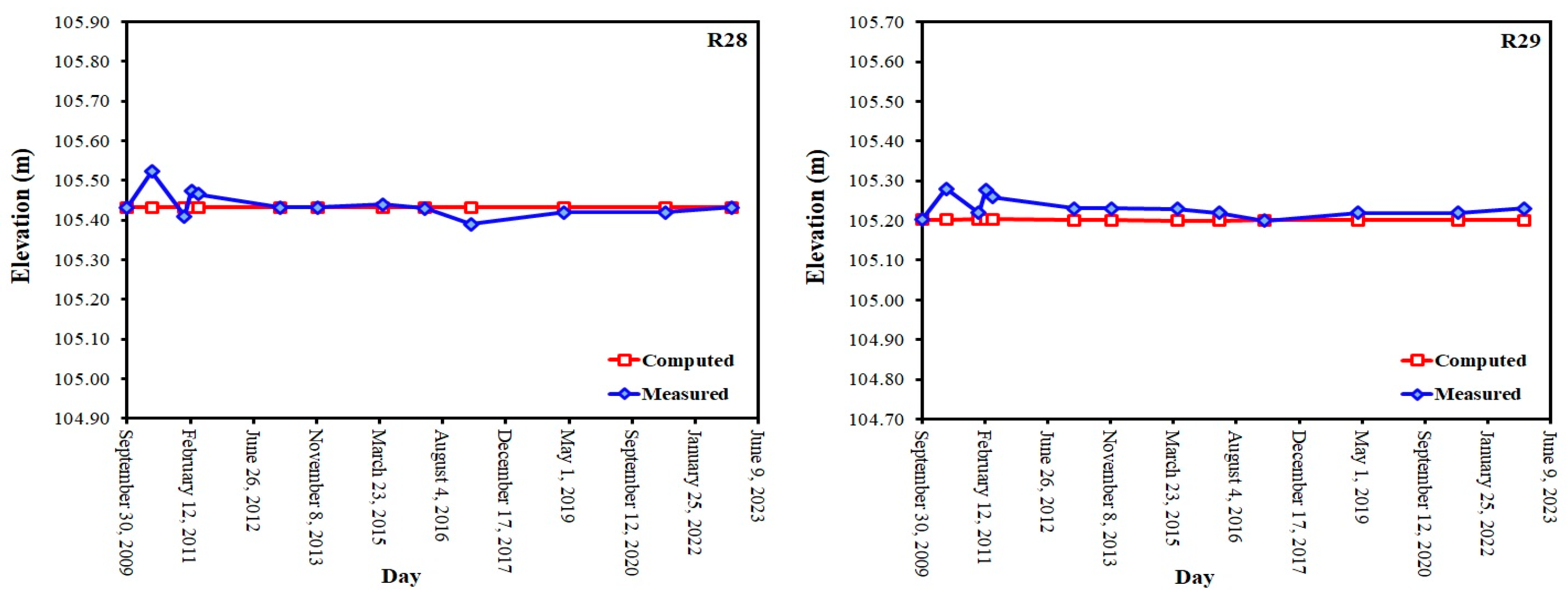
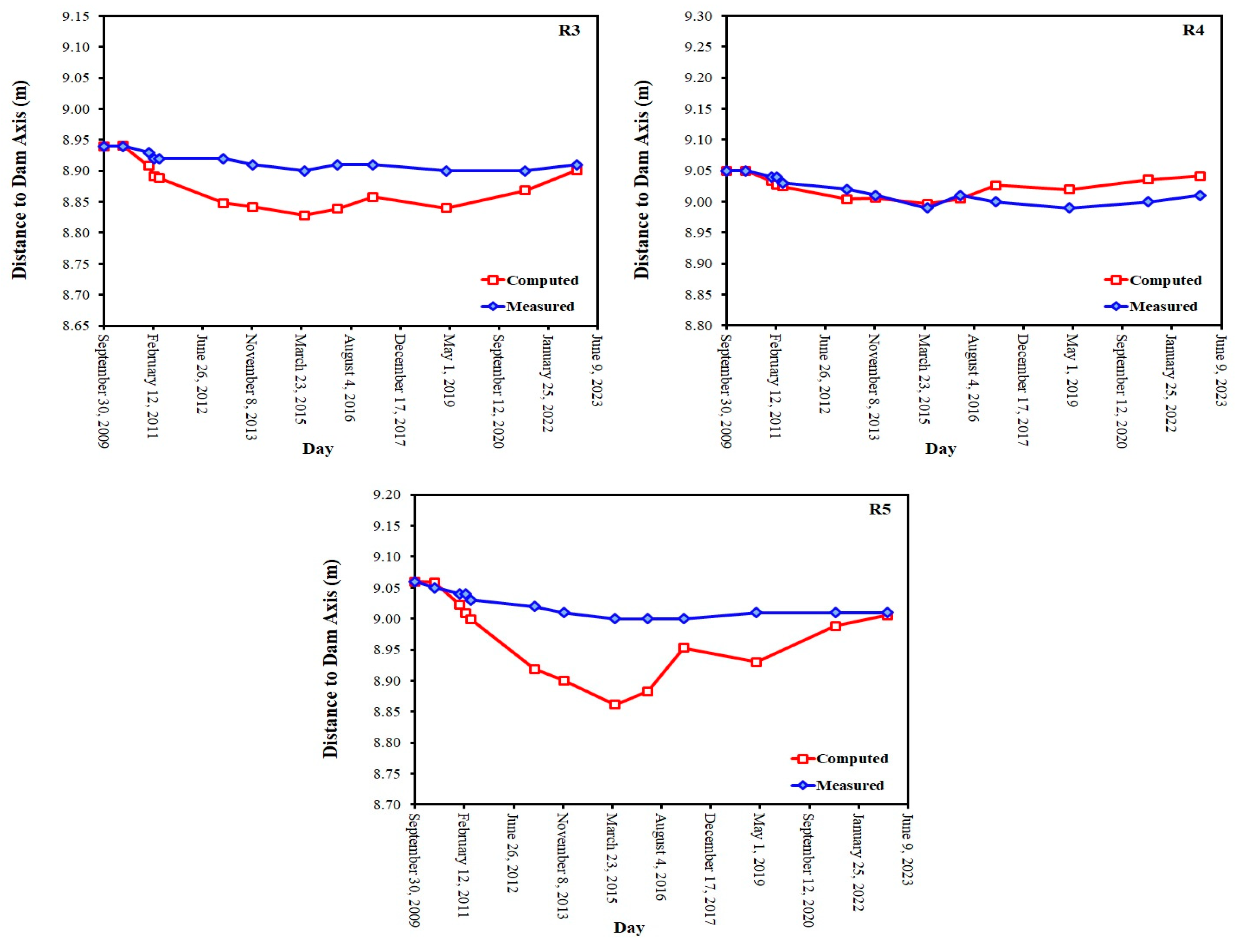
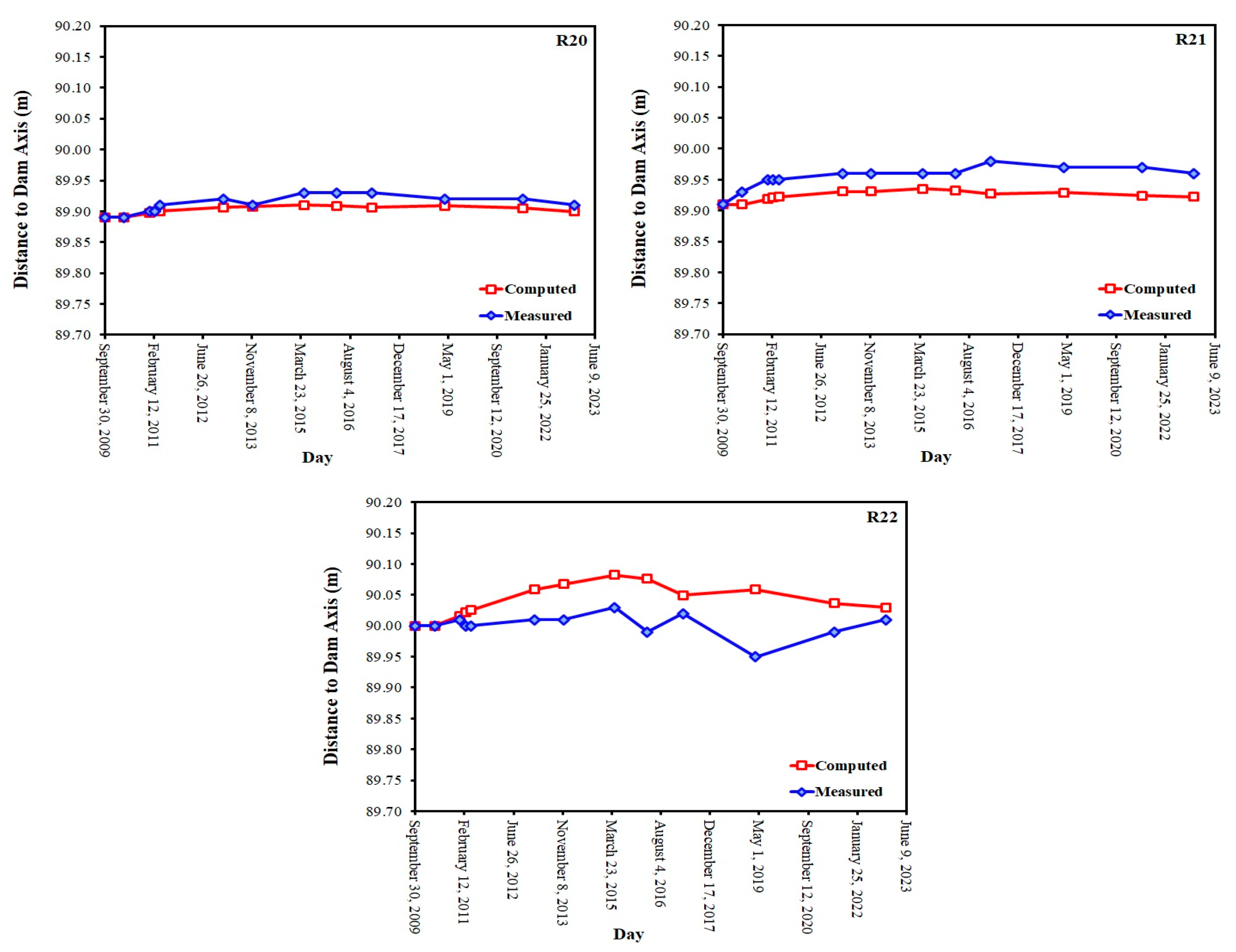
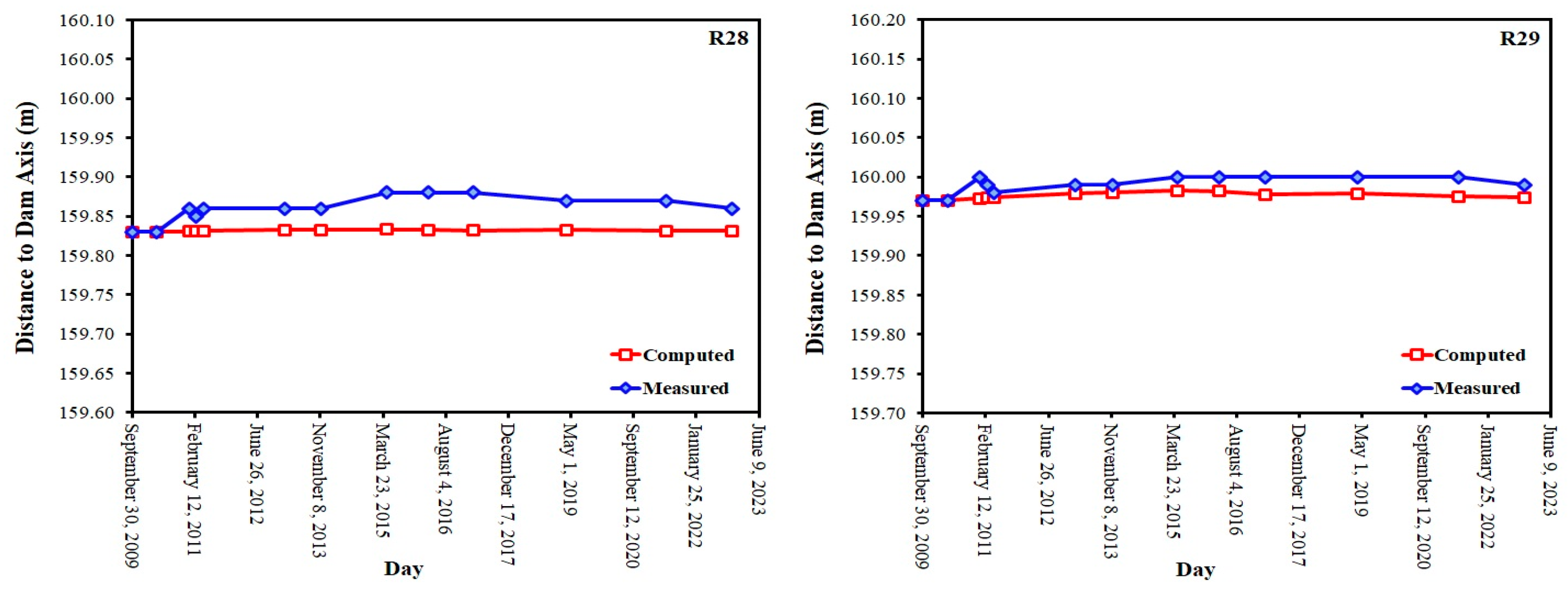
4.1. Comparison and Evaluation of Analysis Results of Survey Points and Measurement Results
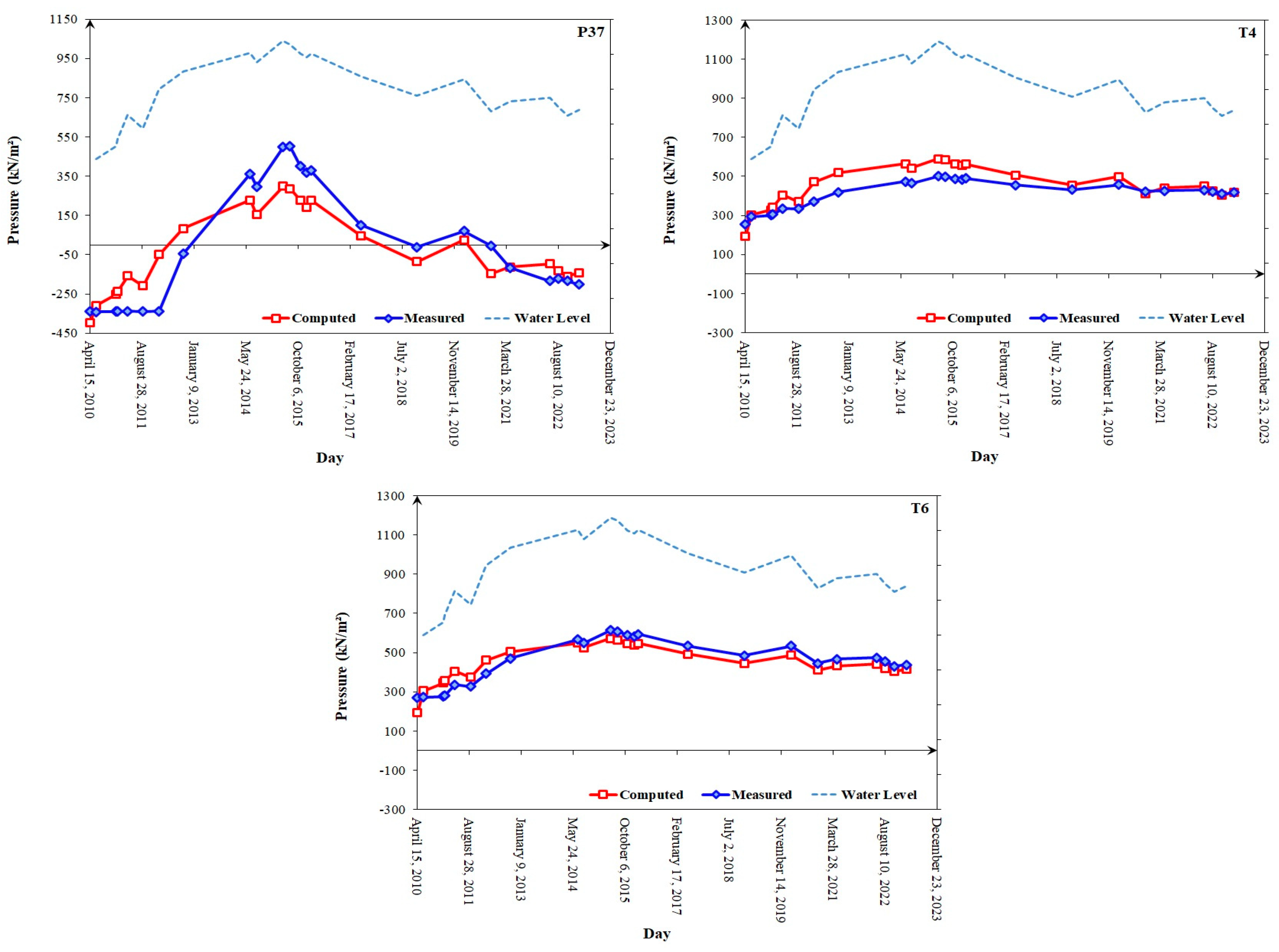
4.2. Comparison and Evaluation of Analysis Results of Piezometers and Dam Measurement Results
5. Results and Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yıllar Itibariyle Yapımı Tamamlanan Baraj Sayıları 1936–2022. Available online: https://dsi.gov.tr/Sayfa/Detay/1847 (accessed on 3 March 2024).
- Ağıralioğlu, N. Atatürk Barajı ve Türkiye’ye Etkileri, 1st ed.; Scala: İstanbul, Turkey, 2014; pp. 83–84. [Google Scholar]
- Uzel, T.; Erkaya, H.; Hoşbaş, R.G.; Gülal, E. Barajların güvenliği: Uygulamadan örnekler. In Proceedings of the International 2nd National Symposium on Dam Safety, Eskişehir, Turkey, 13–15 May 2009. [Google Scholar]
- You, L.; Li, C.; Min, X.; Xiaolei, T. Review of Dam-break Research of Earth-rock Dam Combining with Dam Safety Management. In Proceedings of the 2012 International Conference on Modern Hydraulic Engineering, Nanjing, China, 9–11 March 2012. [Google Scholar]
- Yenigün, K.; Yüzgül, F. Baraj elemanlarının tasarım ve yapım aşamasında baraj güvenliği kıstasıyla irdelenmesi; Ilısu Barajı Örneği. In Proceedings of the International Participation Fourth National Symposium On Dam Safety, Elazığ, Turkey, 9–11 October 2014. [Google Scholar]
- Abay, O.; Baykan, N.; Baykan, N.O. Tarih boyunca barajların elden çıkma nedenleri. In Proceedings of the 4. Su Yapıları Sempozyumu, Antalya, Turkey, 19–21 November 2015. [Google Scholar]
- Dam Failure. Available online: https://en.wikipedia.org/wiki/Dam_failure (accessed on 20 December 2023).
- Zhong, D.; Sun, Y.; Li, M. Dam break threshold value and risk probability assessment for an earth dam. Nat. Hazards 2011, 59, 129–147. [Google Scholar] [CrossRef]
- Amouzou, G.Y.; Soulaïmani, A. Numerical Algorithms for Elastoplacity: Finite Elements Code Development and Implementation of the Mohr–Coulomb Law. Appl. Sci. 2021, 11, 4637. [Google Scholar] [CrossRef]
- Shahzadi, G.; Soulaïmani, A. Deep Neural Network and Polynomial Chaos Expansion-Based Surrogate Models for Sensitivity and Uncertainty Propagation: An Application to a Rockfill Dam. Water 2021, 13, 1830. [Google Scholar] [CrossRef]
- Halil, İ.A. Ön Yüzü Beton Kaplı Sakarya Akçay Barajı’nın Sonlu Elemanlar Yöntemiyle Tasarımı ve Arazide Yapılan Ölçüm-lerle Karşılaştırılması. Master’s Thesis, Sakarya University, Sakarya, Turkey, 2021. [Google Scholar]
- Li, Y.; Zhang, H.; Yuan, Y.; Lan, L.; Su, Y. Research on Failure Modes and Causes of 100-m-High Core Wall Rockfill Dams. Water 2024, 16, 1809. [Google Scholar] [CrossRef]
- Klun, M.; Kryžanowski, A. Dynamic monitoring as a part of structural health monitoring of dams. Arch. Civ. Eng. 2022, 68, 569–578. [Google Scholar] [CrossRef]
- Wieland, M.; Kirchen, G.F. Long-term dam safety monitoring of Punt dal Gall arch dam in Switzerland. Front. Struct. Civ. Eng. 2012, 6, 76–83. [Google Scholar] [CrossRef]
- Ağıralioğlu, N. Baraj Güvenliği, 1st ed.; Beta: İstanbul, Turkey, 2011; pp. 120–142. [Google Scholar]
- Zhuang, W.; Liu, Y.; Zhang, R.; Ho, S.; Yang, Q. Study on deformation mechanism and parameter inversion of a reservoir bank slope during initial impoundment. Acta Geotech. 2023, 18, 4353–4374. [Google Scholar] [CrossRef]
- Aksu, S.; Ağca, E. Baraj emniyeti rehberi ve bölge taşkın planları. In Proceedings of the International 2nd National Symposium on Dam Safety, Eskişehir, Turkey, 13–15 May 2009. [Google Scholar]
- Baykan, N.O.; Yaşar, M.; Saf, B. Safety regulations and measuring devices in dams. In Proceedings of the International 2nd National Symposium on Dam Safety, Eskişehir, Turkey, 13–15 May 2009. [Google Scholar]
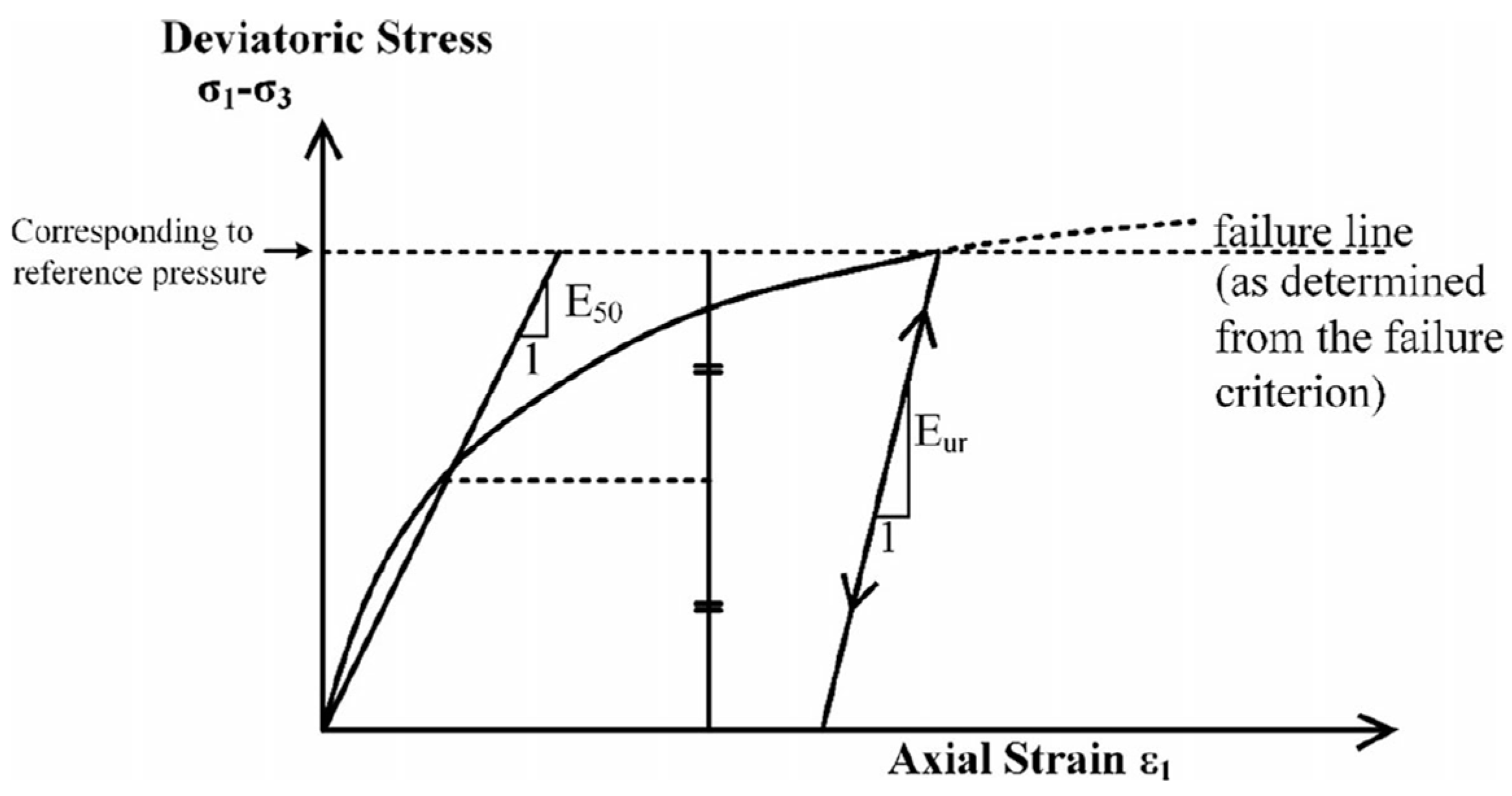
- Wu, J.T.H.; Tung, S.C.-Y. Determination of Model Parameters for the Hardening Soil Model. Transp. Infrastruct. Geotechnol. 2019, 7, 55–68. [Google Scholar] [CrossRef]
- Schanz, T.; Vermeer, P.A.; Bonnier, P.G. The Hardening Soil Model: Formulation and Verification. Available online: https://www.academia.edu/7922212/The_hardening_soil_model_Formulation_and_verification (accessed on 1 July 2024).
- Plaxis, B.V. Plaxis Version 8 Material Models Manual; Balkema: Rotterdam, The Netherlands, 2002. [Google Scholar]





| Dam | Year | Country | Death | Collapse Reason |
|---|---|---|---|---|
| Derna dam | 2023 | Libya | 18,000–20,000 | Body collapse |
| Brumadinho dam | 2019 | Brazil | 270 | Weak regulatory structures and regulatory gaps |
| Koshi | 2008 | Nepal | 250 | Extreme rainfall |
| Kantale | 1986 | Siri Lanka | 180 | Faulty operation |
| Val di Stava | 1985 | Italy | 268 | Faulty design and construction |
| Machchu 2 | 1979 | India | 5000 | Water exceeding the dam |
| Banqiao/Shimantan | 1975 | China | 171,000 | Extreme rainfall |
| Canyon Lake | 1972 | USA | 238 | Flooding |
| Buffalo Creek | 1972 | USA | 125 | Extreme rainfall |
| Sempor | 1967 | Indonesia | >2000 | Water exceeding the dam |
| Vratsa | 1966 | Bulgaria | 107 | Mud and water flooding |
| Vaiont | 1963 | İtaly | 2000 | Water exceeding the dam |
| Panshet | 1961 | India | 1000 | Body collapse |
| Malpesset | 1959 | France | 423 | Under construction |
| Vega de Tera | 1959 | Spain | 144 | Body collapse |
| Sella Zerbino | 1935 | İtaly | 111 | Geological instability/flood |
| St. Francis | 1928 | USA | 600 | Geological instability |
| Gleno | 1923 | İtaly | 356 | Faulty design and construction |
| Tigra | 1917 | India | 1000 | Water seepage from the foundation |
| South Fork | 1889 | USA | 2209 | Extreme rainfall |
| Mill Nehri | 1874 | USA | 139 | Faulty design |
| Dale Dike | 1864 | England | 244 | Faulty construction |
| Puentes | 1802 | Spain | 608 | Soft soil |
| Material | Material Model | Drainage Type | γunsat γsat (kN/m3) | E50ref (kN/m2) | kx − ky (m/day) | c′ref (kN/m2) | φ′ (°) | ψ′ (°) |
|---|---|---|---|---|---|---|---|---|
| Injection Wall—cover Injection | L.E. 1 | Non-porous | 24.00 | 20,000 | - | - | - | - |
| Alluvium | H.S. 2 | Drained | 19.00 20.00 | 5000 | 3.024 | 5 | 30 | 0 |
| Schist | H.S. | Drained | 27.00 28.00 | 50,000,000 | 30.24 | 20,000 | 30 | 0 |
| Clayey slope rubble | H.S. | Drained | 19.00 20.00 | 20,000 | 0.0864 | 10 | 35 | 5 |
| Impermeable Clay core | H.S. | Undrained-A | 18.60 19.60 | 25,000 | 0.007957 | 120.6 | 20 | 0 |
| Permeable Sand–gravel | H.S. | Drained | 16.90 17.90 | 30,000 | 8.64 | 0 | 36 | 6 |
| Sand filter | H.S. | Drained | 16.70 17.70 | 25,000 | 86.4 | 0 | 37 | 7 |
| Gravel filter | H.S. | Drained | 17.10 18.10 | 32,000 | 864 | 0 | 38 | 8 |
| Tuvenan filter | H.S. | Drained | 16.90 17.90 | 30,000 | 8.64 | 0 | 37 | 7 |
| Rockfill | H.S. | Drained | 26.50 27.50 | 200,000 | 864 | 0 | 42 | 12 |
| Riprap | H.S. | Drained | 26.50 27.50 | 200,000 | 8640 | 0 | 42 | 12 |
| R3 | R4 | R5 | |||||
|---|---|---|---|---|---|---|---|
| Day | Water Level (m) | Measured Values (m) | Computed Values (m) | Measured Values (m) | Computed Values (m) | Measured Values (m) | Computed Values (m) |
| 30 September 2009 | 0.00 | 182.572 | 182.572 | 182.632 | 182.632 | 182.592 | 182.592 |
| 13 April 2010 | 0.00 | 182.630 | 182.571 | 182.660 | 182.632 | 182.633 | 182.593 |
| 27 December 2010 | 108.53 | 182.540 | 182.650 | 182.570 | 182.679 | 182.550 | 182.629 |
| 22 February 2011 | 120.55 | 182.638 | 182.684 | 182.633 | 182.693 | 182.629 | 182.640 |
| 18 April 2011 | 125.55 | 182.639 | 182.689 | 182.660 | 182.700 | 182.653 | 182.648 |
| 24 January 2013 | 154.36 | 182.518 | 182.752 | 182.529 | 182.741 | 182.518 | 182.711 |
| 15 November 2013 | 158.70 | 182.490 | 182.763 | 182.506 | 182.744 | 182.501 | 182.726 |
| 21 April 2015 | 167.01 | 182.470 | 182.785 | 182.480 | 182.758 | 182.450 | 182.755 |
| 18 March 2016 | 162.00 | 182.430 | 182.772 | 182.440 | 182.749 | 182.410 | 182.739 |
| 16 March 2017 | 145.90 | 182.420 | 182.756 | 182.420 | 182.722 | 182.380 | 182.684 |
| 25 March 2019 | 152.80 | 182.430 | 182.783 | 182.430 | 182.732 | 182.400 | 182.702 |
| 1 June 2021 | 134.29 | 182.410 | 182.743 | 182.390 | 182.706 | 182.400 | 182.656 |
| 9 November 2022 | 124.81 | 182.490 | 182.685 | 182.506 | 182.694 | 182.501 | 182.641 |
| R20 | R21 | R22 | |||||
|---|---|---|---|---|---|---|---|
| Day | Water Level (m) | Measured Values (m) | Computed Values (m) | Measured Values (m) | Computed Values (m) | Measured Values (m) | Computed Values (m) |
| 30 September 2009 | 0.00 | 141.202 | 141.202 | 141.442 | 141.442 | 142.122 | 142.122 |
| 13 April 2010 | 0.00 | 141.245 | 141.202 | 141.494 | 141.442 | 142.190 | 142.122 |
| 27 December 2010 | 108.53 | 141.190 | 141.200 | 141.420 | 141.439 | 142.100 | 142.125 |
| 22 February 2011 | 120.55 | 141.232 | 141.199 | 141.516 | 141.439 | 142.199 | 142.125 |
| 18 April 2011 | 125.55 | 141.249 | 141.199 | 141.501 | 141.438 | 142.153 | 142.125 |
| 24 January 2013 | 154.36 | 141.154 | 141.197 | 141.424 | 141.435 | 142.082 | 142.124 |
| 15 November 2013 | 158.70 | 141.157 | 141.196 | 141.434 | 141.435 | 142.099 | 142.123 |
| 21 April 2015 | 167.01 | 141.160 | 141.195 | 141.430 | 141.434 | 142.110 | 142.121 |
| 18 March 2016 | 162.00 | 141.120 | 141.196 | 141.360 | 141.434 | 142.080 | 142.122 |
| 16 March 2017 | 145.90 | 141.120 | 141.197 | 141.370 | 141.436 | 142.070 | 142.122 |
| 25 March 2019 | 152.80 | 141.120 | 141.196 | 141.370 | 141.435 | 142.080 | 142.122 |
| 1 June 2021 | 134.29 | 141.120 | 141.197 | 141.370 | 141.436 | 142.090 | 142.122 |
| 9 November 2022 | 124.81 | 141.157 | 141.198 | 141.434 | 141.437 | 142.099 | 142.121 |
| R28 | R29 | ||||
|---|---|---|---|---|---|
| Day | Water Level (m) | Measured Values (m) | Computed Values (m) | Measured Values (m) | Computed Values (m) |
| 30 September 2009 | 0.00 | 141.202 | 141.202 | 141.442 | 141.442 |
| 13 April 2010 | 0.00 | 141.245 | 141.202 | 141.494 | 141.442 |
| 27 December 2010 | 108.53 | 141.190 | 141.200 | 141.420 | 141.439 |
| 22 February 2011 | 120.55 | 141.232 | 141.199 | 141.516 | 141.439 |
| 18 April 2011 | 125.55 | 141.249 | 141.199 | 141.501 | 141.438 |
| 24 January 2013 | 154.36 | 141.154 | 141.197 | 141.424 | 141.435 |
| 15 November 2013 | 158.70 | 141.157 | 141.196 | 141.434 | 141.435 |
| 21 April 2015 | 167.01 | 141.160 | 141.195 | 141.430 | 141.434 |
| 18 March 2016 | 162.00 | 141.120 | 141.196 | 141.360 | 141.434 |
| 16 March 2017 | 145.90 | 141.120 | 141.197 | 141.370 | 141.436 |
| 25 March 2019 | 152.80 | 141.120 | 141.196 | 141.370 | 141.435 |
| 1 June 2021 | 134.29 | 141.120 | 141.197 | 141.370 | 141.436 |
| 9 November 2022 | 124.81 | 141.157 | 141.198 | 141.434 | 141.437 |
| R3 | R4 | R5 | |||||
|---|---|---|---|---|---|---|---|
| Day | Water Level (m) | Measured Values (m) | Computed Values (m) | Measured Values (m) | Computed Values (m) | Measured Values (m) | Computed Values (m) |
| 30 September 2009 | 0.00 | 8.94 | 8.940 | 9.05 | 9.050 | 9.06 | 9.060 |
| 13 April 2010 | 0.00 | 8.94 | 8.940 | 9.05 | 9.050 | 9.05 | 9.059 |
| 27 December 2010 | 108.53 | 8.93 | 8.908 | 9.04 | 9.033 | 9.04 | 9.022 |
| 22 February 2011 | 120.55 | 8.92 | 8.891 | 9.04 | 9.027 | 9.04 | 9.009 |
| 18 April 2011 | 125.55 | 8.92 | 8.888 | 9.03 | 9.025 | 9.03 | 8.999 |
| 24 January 2013 | 154.36 | 8.92 | 8.848 | 9.02 | 9.004 | 9.02 | 8.919 |
| 15 November 2013 | 158.70 | 8.91 | 8.842 | 9.01 | 9.006 | 9.01 | 8.900 |
| 21 April 2015 | 167.01 | 8.90 | 8.828 | 8.99 | 8.996 | 9.00 | 8.861 |
| 18 March 2016 | 162.00 | 8.91 | 8.839 | 9.01 | 9.005 | 9.00 | 8.883 |
| 16 March 2017 | 145.90 | 8.91 | 8.858 | 9.00 | 9.027 | 9.00 | 8.953 |
| 25 March 2019 | 152.80 | 8.90 | 8.840 | 8.99 | 9.020 | 9.01 | 8.930 |
| 1 June 2021 | 134.29 | 8.90 | 8.868 | 9.00 | 9.035 | 9.01 | 8.988 |
| 9 November 2022 | 124.81 | 8.91 | 8.901 | 9.01 | 9.041 | 9.01 | 9.006 |
| R20 | R21 | R22 | |||||
|---|---|---|---|---|---|---|---|
| Day | Water Level (m) | Measured Values (m) | Computed Values (m) | Measured Values (m) | Computed Values (m) | Measured Values (m) | Computed Values (m) |
| 30 September 2009 | 0.00 | 89.89 | 89.890 | 89.91 | 89.910 | 90.00 | 90.000 |
| 13 April 2010 | 0.00 | 89.89 | 89.890 | 89.93 | 89.910 | 90.00 | 90.000 |
| 27 December 2010 | 108.53 | 89.9 | 89.897 | 89.95 | 89.918 | 90.01 | 90.016 |
| 22 February 2011 | 120.55 | 89.9 | 89.900 | 89.95 | 89.921 | 90.00 | 90.022 |
| 18 April 2011 | 125.55 | 89.91 | 89.900 | 89.95 | 89.922 | 90.00 | 90.026 |
| 24 January 2013 | 154.36 | 89.92 | 89.907 | 89.96 | 89.931 | 90.01 | 90.059 |
| 15 November 2013 | 158.70 | 89.91 | 89.908 | 89.96 | 89.931 | 90.01 | 90.067 |
| 21 April 2015 | 167.01 | 89.93 | 89.910 | 89.96 | 89.935 | 90.03 | 90.083 |
| 18 March 2016 | 162.00 | 89.93 | 89.909 | 89.96 | 89.933 | 89.99 | 90.076 |
| 16 March 2017 | 145.90 | 89.93 | 89.906 | 89.98 | 89.927 | 90.02 | 90.050 |
| 25 March 2019 | 152.80 | 89.92 | 89.909 | 89.97 | 89.929 | 89.95 | 90.059 |
| 1 June 2021 | 134.29 | 89.92 | 89.905 | 89.97 | 89.924 | 89.99 | 90.036 |
| 9 November 2022 | 124.81 | 89.91 | 89.899 | 89.96 | 89.922 | 90.01 | 90.029 |
| R28 | R29 | ||||
|---|---|---|---|---|---|
| Day | Water Level (m) | Measured Values (m) | Computed Values (m) | Measured Values (m) | Computed Values (m) |
| 30 September 2009 | 0.00 | 159.83 | 159.830 | 159.97 | 159.970 |
| 13 April 2010 | 0.00 | 159.83 | 159.830 | 159.97 | 159.970 |
| 27 December 2010 | 108.53 | 159.86 | 159.831 | 160.00 | 159.973 |
| 22 February 2011 | 120.55 | 159.85 | 159.831 | 159.99 | 159.973 |
| 18 April 2011 | 125.55 | 159.86 | 159.831 | 159.98 | 159.974 |
| 24 January 2013 | 154.36 | 159.86 | 159.832 | 159.99 | 159.979 |
| 15 November 2013 | 158.70 | 159.86 | 159.833 | 159.99 | 159.980 |
| 21 April 2015 | 167.01 | 159.88 | 159.833 | 160.00 | 159.983 |
| 18 March 2016 | 162.00 | 159.88 | 159.833 | 160.00 | 159.982 |
| 16 March 2017 | 145.90 | 159.88 | 159.832 | 160.00 | 159.978 |
| 25 March 2019 | 152.80 | 159.87 | 159.832 | 160.00 | 159.979 |
| 1 June 2021 | 134.29 | 159.87 | 159.832 | 160.00 | 159.975 |
| 9 November 2022 | 124.81 | 159.86 | 159.831 | 159.99 | 159.974 |
| P29 Elevation: 76 m Dam Axis | P15 Elevation: 78 m Dam Axis | P1 Elevation: 85 m Dam Axis | |||||
|---|---|---|---|---|---|---|---|
| Day | Water Level (m) | Measured Values (m) | Computed Values (m) | Measured Values (m) | Computed Values (m) | Measured Values (m) | Computed Values (m) |
| 15 April 2010 | 0.00 | 269.182 | −13.305 | 1.898 | −30.882 | 279.317 | −85.684 |
| 15 June 2010 | 100.00 | 270.014 | 103.581 | 2.462 | 88.753 | 281.410 | 49.766 |
| 21 December 2010 | 107.38 | 272.719 | 159.598 | 2.872 | 116.783 | 284.130 | 103.104 |
| 6 January 2011 | 111.24 | 276.777 | 176.210 | 2.821 | 135.142 | 289.936 | 104.156 |
| 12 April 2011 | 125.23 | 322.749 | 252.550 | 3.693 | 214.872 | 346.694 | 177.576 |
| 9 September 2011 | 117.50 | 319.629 | 204.360 | 2.103 | 170.209 | 333.982 | 139.146 |
| 8 February 2012 | 139.80 | 372.591 | 346.335 | 4.513 | 310.458 | 401.526 | 317.345 |
| 26 September 2012 | 150.00 | 448.004 | 421.692 | 7.080 | 379.844 | 477.268 | 379.853 |
| 3 July 2014 | 160.50 | 545.806 | 493.654 | 7.539 | 448.420 | 561.823 | 443.309 |
| 2 September 2014 | 155.16 | 533.838 | 458.551 | 6.528 | 414.810 | 544.012 | 413.201 |
| 15 May 2015 | 167.53 | 591.464 | 533.020 | 8.052 | 488.802 | 611.004 | 481.203 |
| 22 July 2015 | 165.55 | 589.205 | 526.480 | 7.539 | 480.783 | 606.805 | 472.757 |
| 27 October 2015 | 160.16 | 572.870 | 494.964 | 7.539 | 447.806 | 586.407 | 442.076 |
| 30 December 2015 | 158.24 | 567.477 | 479.013 | 8.549 | 435.622 | 580.119 | 430.801 |
| 9 February 2016 | 160.22 | 607.424 | 495.562 | 9.062 | 446.896 | 589.561 | 440.346 |
| 31 May 2017 | 147.07 | 525.297 | 400.908 | 5.548 | 361.028 | 531.468 | 363.396 |
| 22 November 2018 | 136.06 | 483.387 | 319.815 | 6.498 | 286.176 | 484.905 | 296.374 |
| 19 February 2020 | 145.63 | 526.612 | 388.919 | 9.062 | 351.114 | 534.098 | 354.265 |
| 31 October 2020 | 126.98 | 451.747 | 263.510 | 8.052 | 226.605 | 440.934 | 185.594 |
| 2 May 2021 | 132.70 | 464.832 | 297.030 | 9.575 | 265.265 | 470.306 | 204.387 |
| 21 May 2022 | 135.01 | 467.310 | 312.023 | 10.088 | 246.550 | 481.879 | 211.358 |
| 13 August 2022 | 129.51 | 465.788 | 278.289 | 9.108 | 246.785 | 461.843 | 194.200 |
| 8 November 2022 | 124.83 | 446.179 | 251.096 | 9.062 | 213.317 | 438.304 | 177.121 |
| 1 March 2023 | 128.04 | 451.157 | 269.291 | 10.103 | 235.862 | 439.928 | 188.843 |
| P37 Elevation: 130 m Upstream-15 m | T4 Elevation: 55 m Downstream-15 m | T6 Elevation: 55 m Downstream-15 m | |||||
|---|---|---|---|---|---|---|---|
| Day | Water Level (m) | Measured Values (m) | Computed Values (m) | Measured Values (m) | Computed Values (m) | Measured Values (m) | Computed Values (m) |
| 15 April 2010 | 0.00 | −340.182 | −398.412 | 255.037 | 193.924 | 270.504 | 194.954 |
| 15 June 2010 | 100.00 | −340.678 | −309.076 | 293.736 | 302.963 | 273.226 | 305.511 |
| 21 December 2010 | 107.38 | −339.630 | −252.429 | 300.992 | 328.845 | 276.274 | 345.165 |
| 6 January 2011 | 111.24 | −339.465 | −235.634 | 303.949 | 343.642 | 281.662 | 356.251 |
| 12 April 2011 | 125.23 | −338.638 | −158.898 | 335.075 | 404.019 | 336.308 | 404.952 |
| 9 September 2011 | 117.50 | −340.458 | −208.173 | 334.053 | 370.141 | 327.055 | 374.049 |
| 8 February 2012 | 139.80 | −337.315 | −48.661 | 372.011 | 472.167 | 392.637 | 461.352 |
| 26 September 2012 | 150.00 | −45.963 | 83.246 | 419.441 | 519.294 | 469.695 | 503.996 |
| 3 July 2014 | 160.50 | 361.518 | 227.228 | 473.872 | 563.973 | 565.466 | 547.773 |
| 2 September 2014 | 155.16 | 297.789 | 153.018 | 463.652 | 542.458 | 548.598 | 525.075 |
| 15 May 2015 | 167.53 | 497.690 | 298.287 | 501.308 | 590.410 | 612.803 | 571.340 |
| 22 July 2015 | 165.55 | 500.984 | 286.034 | 498.080 | 583.574 | 607.362 | 564.801 |
| 27 October 2015 | 160.16 | 401.612 | 228.508 | 487.859 | 562.655 | 590.495 | 546.608 |
| 30 December 2015 | 158.24 | 369.757 | 192.389 | 484.631 | 555.230 | 583.421 | 537.288 |
| 9 February 2016 | 160.22 | 378.545 | 228.407 | 488.935 | 562.857 | 593.759 | 546.806 |
| 31 May 2017 | 147.07 | 100.418 | 46.275 | 455.584 | 506.354 | 533.362 | 492.410 |
| 22 November 2018 | 136.06 | −10.733 | −86.326 | 432.456 | 454.653 | 485.476 | 445.189 |
| 19 February 2020 | 145.63 | 70.161 | 25.702 | 457.735 | 499.708 | 533.362 | 486.318 |
| 31 October 2020 | 126.98 | −4.678 | −148.114 | 422.237 | 411.916 | 444.663 | 411.149 |
| 2 May 2021 | 132.70 | −116.444 | −112.863 | 426.002 | 440.003 | 465.886 | 431.796 |
| 21 May 2022 | 135.01 | −183.096 | −95.802 | 429.767 | 449.890 | 472.961 | 440.697 |
| 13 August 2022 | 129.51 | −173.179 | −133.008 | 421.162 | 426.466 | 453.370 | 420.205 |
| 8 November 2022 | 124.83 | −183.096 | −160.672 | 410.406 | 402.246 | 429.425 | 403.546 |
| 1 March 2023 | 128.04 | −201.829 | −142.093 | 417.397 | 418.898 | 437.044 | 414.958 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sarayli, S.; Sert, S.; Sonmez, O. Analysis of Fill Dam Using Finite Element Method and Comparison with Monitoring Results. Water 2024, 16, 2387. https://doi.org/10.3390/w16172387
Sarayli S, Sert S, Sonmez O. Analysis of Fill Dam Using Finite Element Method and Comparison with Monitoring Results. Water. 2024; 16(17):2387. https://doi.org/10.3390/w16172387
Chicago/Turabian StyleSarayli, Suleyman, Sedat Sert, and Osman Sonmez. 2024. "Analysis of Fill Dam Using Finite Element Method and Comparison with Monitoring Results" Water 16, no. 17: 2387. https://doi.org/10.3390/w16172387
APA StyleSarayli, S., Sert, S., & Sonmez, O. (2024). Analysis of Fill Dam Using Finite Element Method and Comparison with Monitoring Results. Water, 16(17), 2387. https://doi.org/10.3390/w16172387






