Drivers of Daily Water Level Fluctuation of Shallow Groundwater in the Inner Delta of the River Danube
Abstract
1. Introduction
Aims
- Do the diurnal patterns of the groundwater level and temperature exhibit mutually consistent characteristics, and if so, are there any spatial attributes of these ?
- What factors contribute to the development of diurnal periodicity in SGW, and what are its drivers?
- Is the Szigetköz area susceptible to forecasted changes in climatic conditions and/or human activity concerning groundwater levels and the extent (thickness) of the unsaturated zone? What specific alterations in groundwater levels might be anticipated in the future? Are there spatial variations within the area’s response to climatic changes?
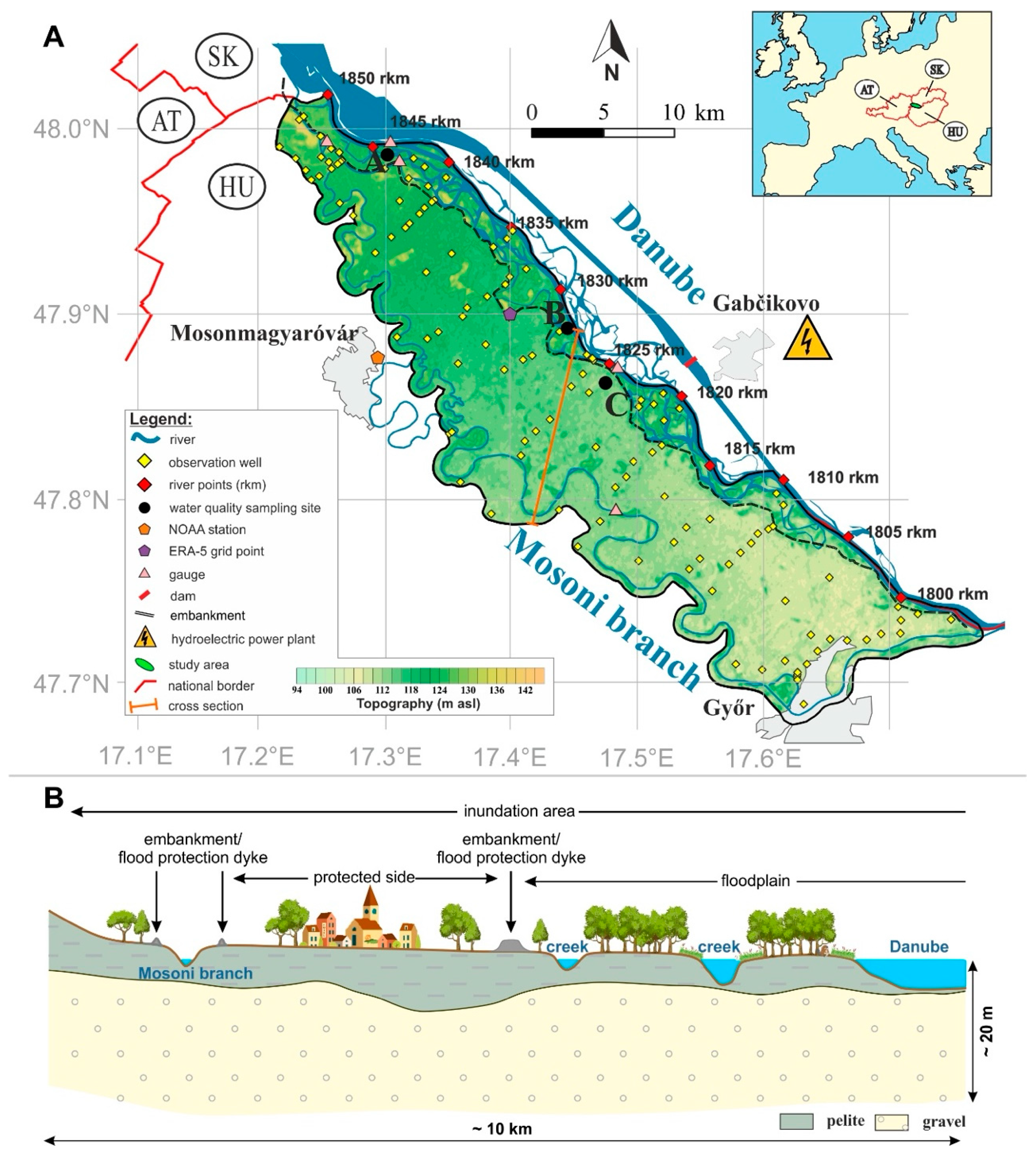
2. Materials and Methods
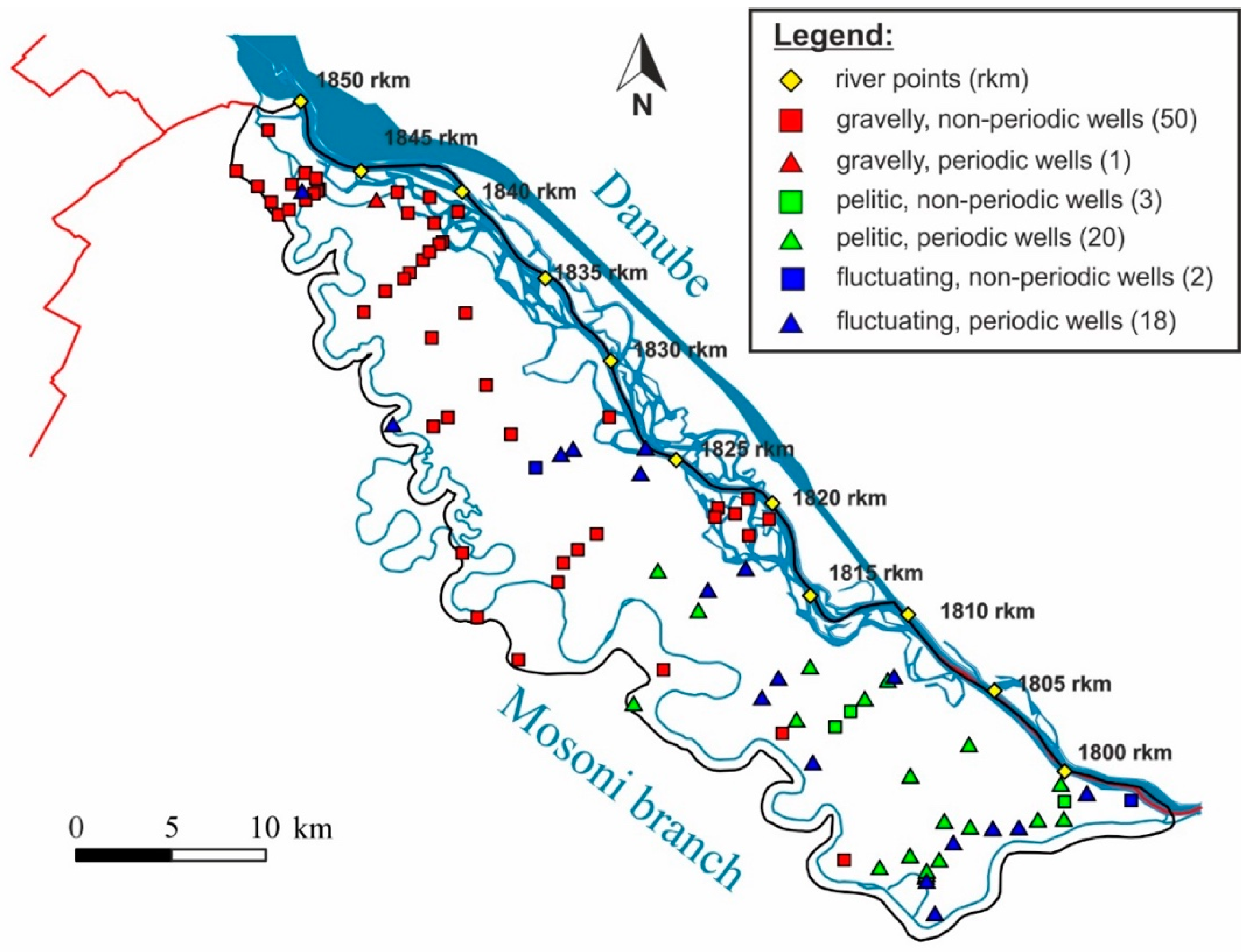
2.1. Study Area—Geological Conditions
2.2. Data
2.3. Steps of the Study in Identifying the Main Driving Factors of the Diurnal Periodicity of Groundwater Levels
- First, it was investigated whether a significant linear relationship exists between the surface explanatory variables and the subsurface response variables at each SGW well using Pearson’s correlation coefficient [50], including cross-correlation on an hourly and diurnal basis to obtain those parameters that are in close relation with the water levels. If any periodic pattern is observed in a SGW level time series resembling that of the meteorological parameters, it may be suspected that a causal relationship may obtain between them, and that this will be driven mainly by insolation/radiation [51,52]. In order to draw general conclusions from any such particular instance of the coincidence between periodic meteorological behavior and SGW, a thorough investigation is required as to the extent to which temperature, PET, relative humidity, and air pressure are able to explain the variance in the SGW levels. It is suggested that this investigation precedes that of the periodic patterns.
- The periodic behavior of the response parameters was assessed using wavelet spectrum analysis (Section 2.4) to determine what percentage of the investigated time period is a diurnal period present in the SGW levels and temperature records, and to try to relate this behavior to local geology.
- Using wavelet transform coherence (WTC, Section 2.4), we investigated whether the periodic signals of the independent explanatory parameters are transferred to the subsurface. This is necessary to determine the delay in the change in SGW levels caused by the given surface processes.
2.4. Wavelet Spectrum Analysis and Wavelet Transform Coherence
- The presence of the coherent periods in time, which meant that significant periodic behavior—coherence—at a certain frequency was transformed into a percentage, while taking the presence of the coherence/period throughout the whole investigated time as 100%;
- The maximum global wavelet power, which is the average cross-wavelet power in the frequency domain (averages over time) [61];
- The phase differences between the pairs of water quality parameters and meteorological parameters, which show which series is the leading one in this relationship [43].
3. Results and Discussion
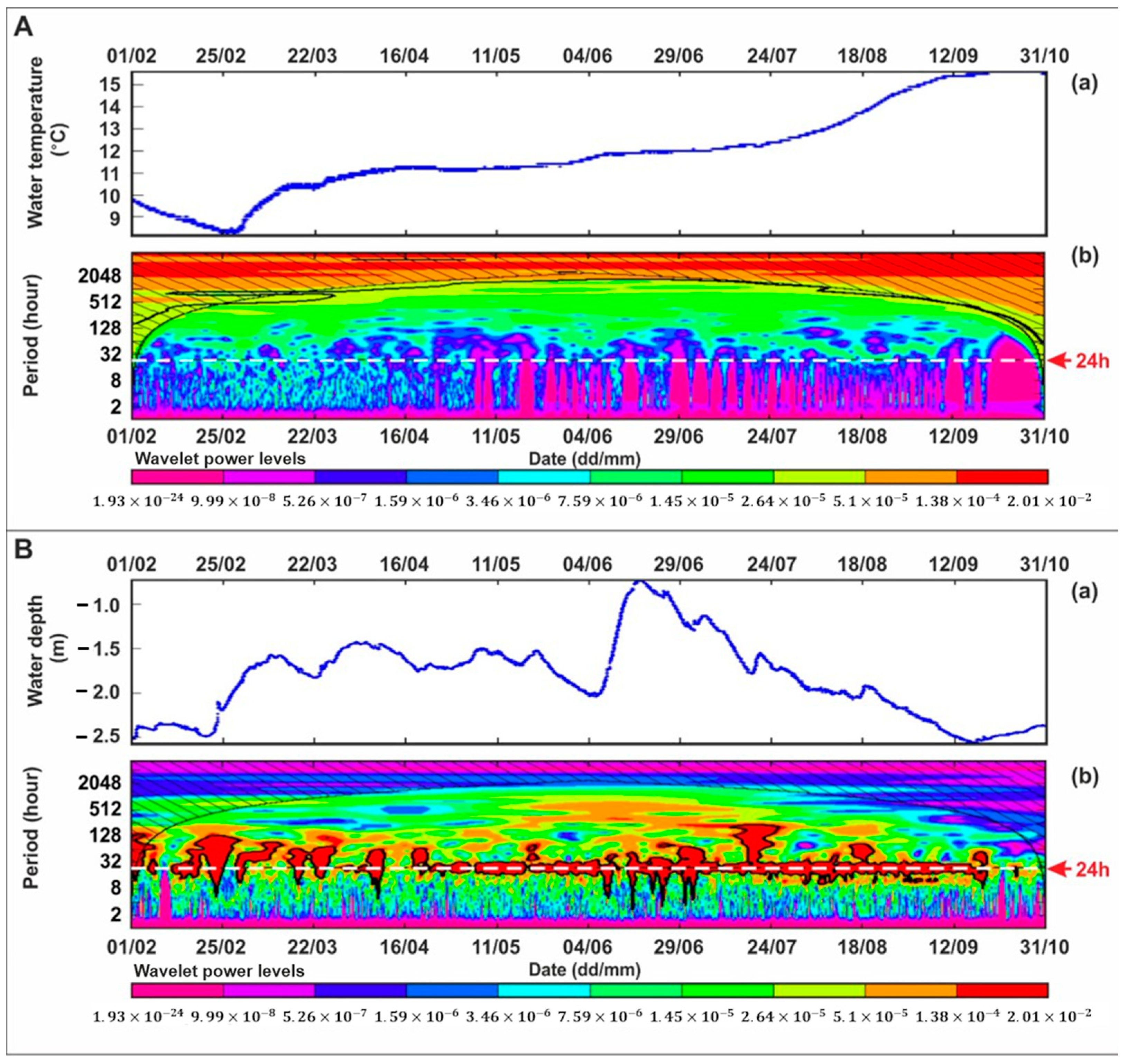
3.1. Analysis of Shallow Groundwater Table Fluctuation
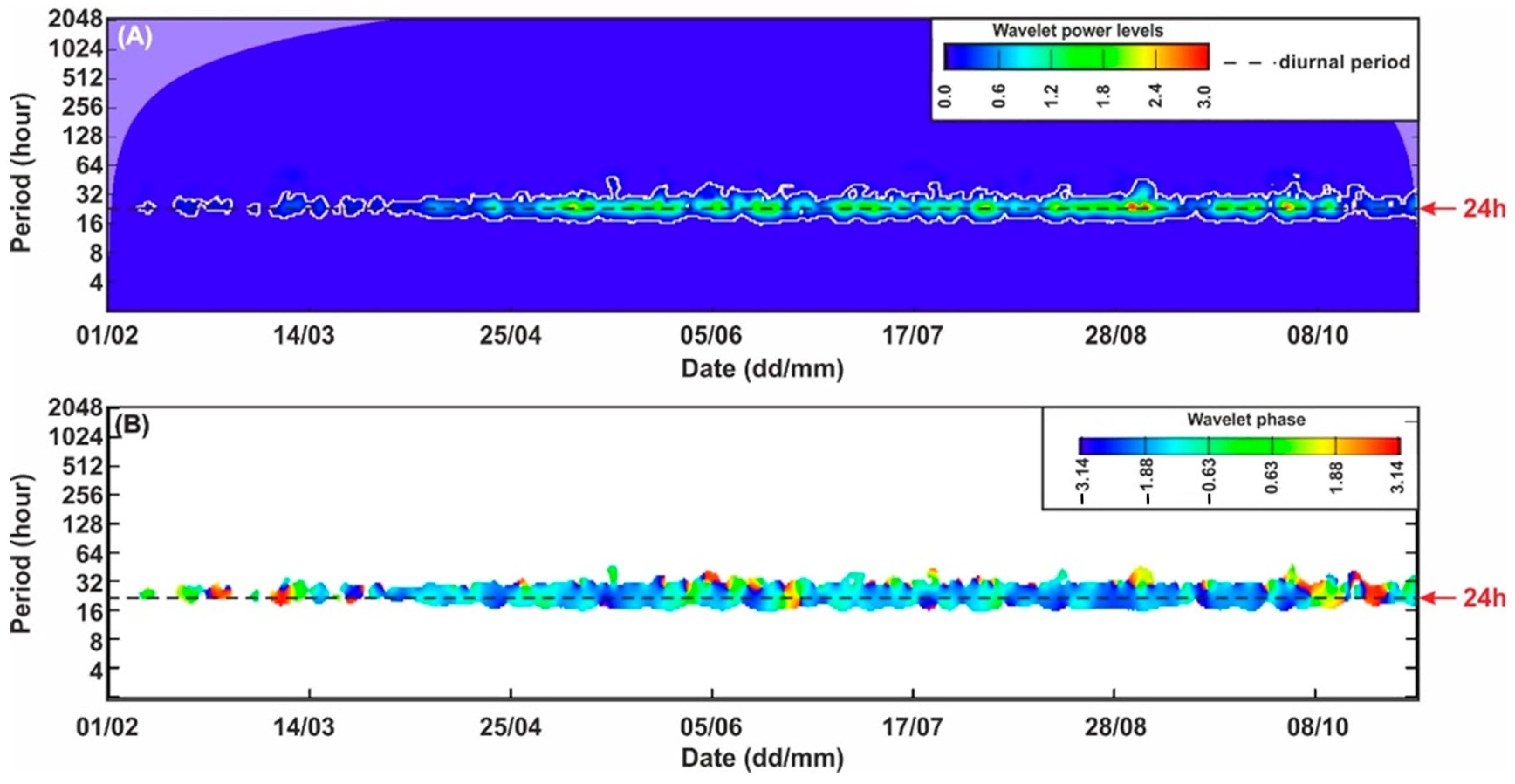
3.2. Detection of the Effect of Surface Processes in the Groundwater by Periodicity Analysis
3.3. Relationship between the Intensity of Evapotranspiration and the Periodic Behavior of Shallow Groundwater Fluctuation
3.4. Temporal Relationship between Evapotranspiration and Groundwater Level Fluctuation
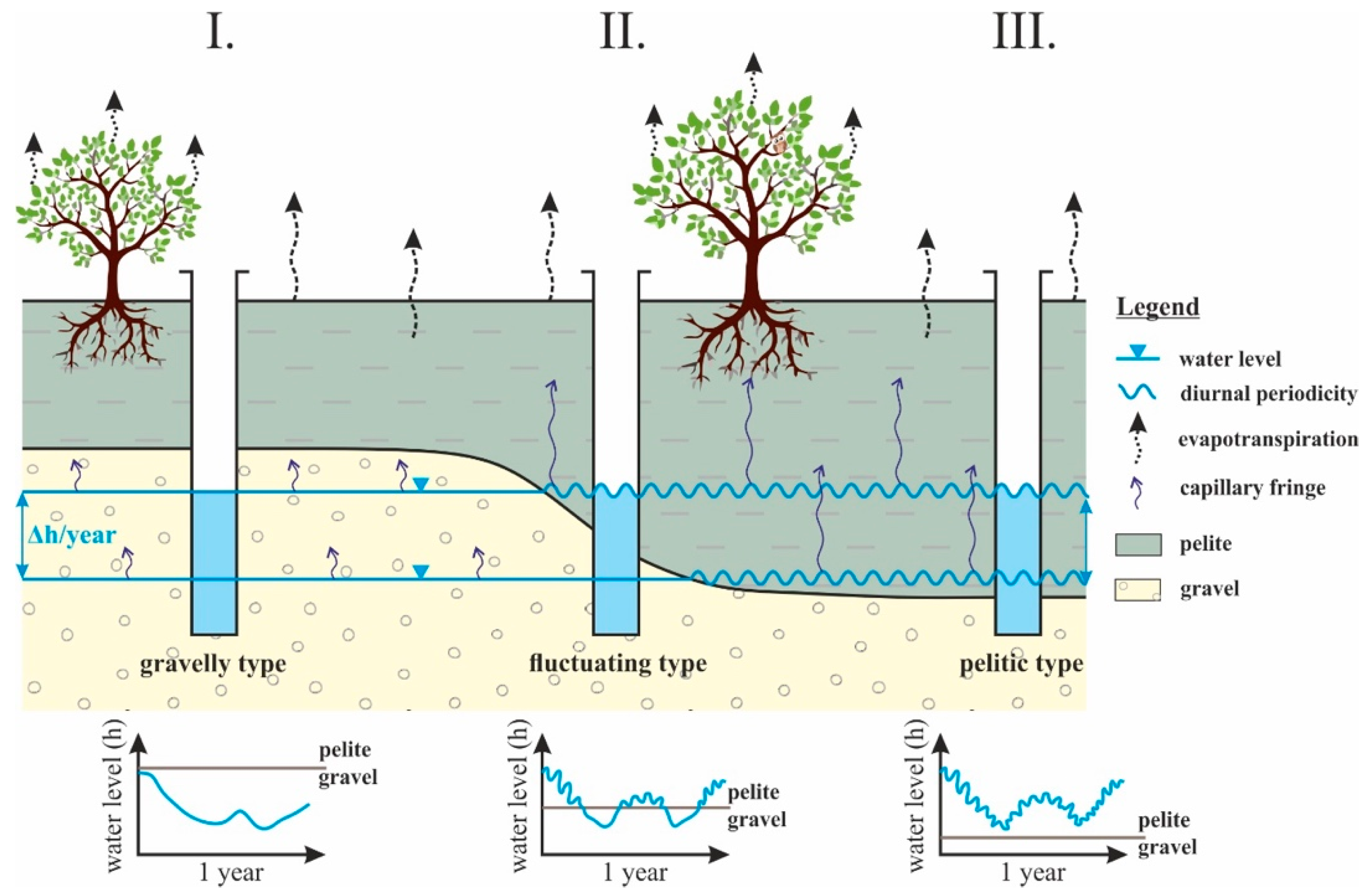
3.5. Capillary Fringe as a ”Conveyor Belt”
- The higher reaction time to changes in the SGW table due to the smaller pore space;
- The fact that the water can reach the root zone, due to the great extent of capillary action, enabling plants to take up water [81].
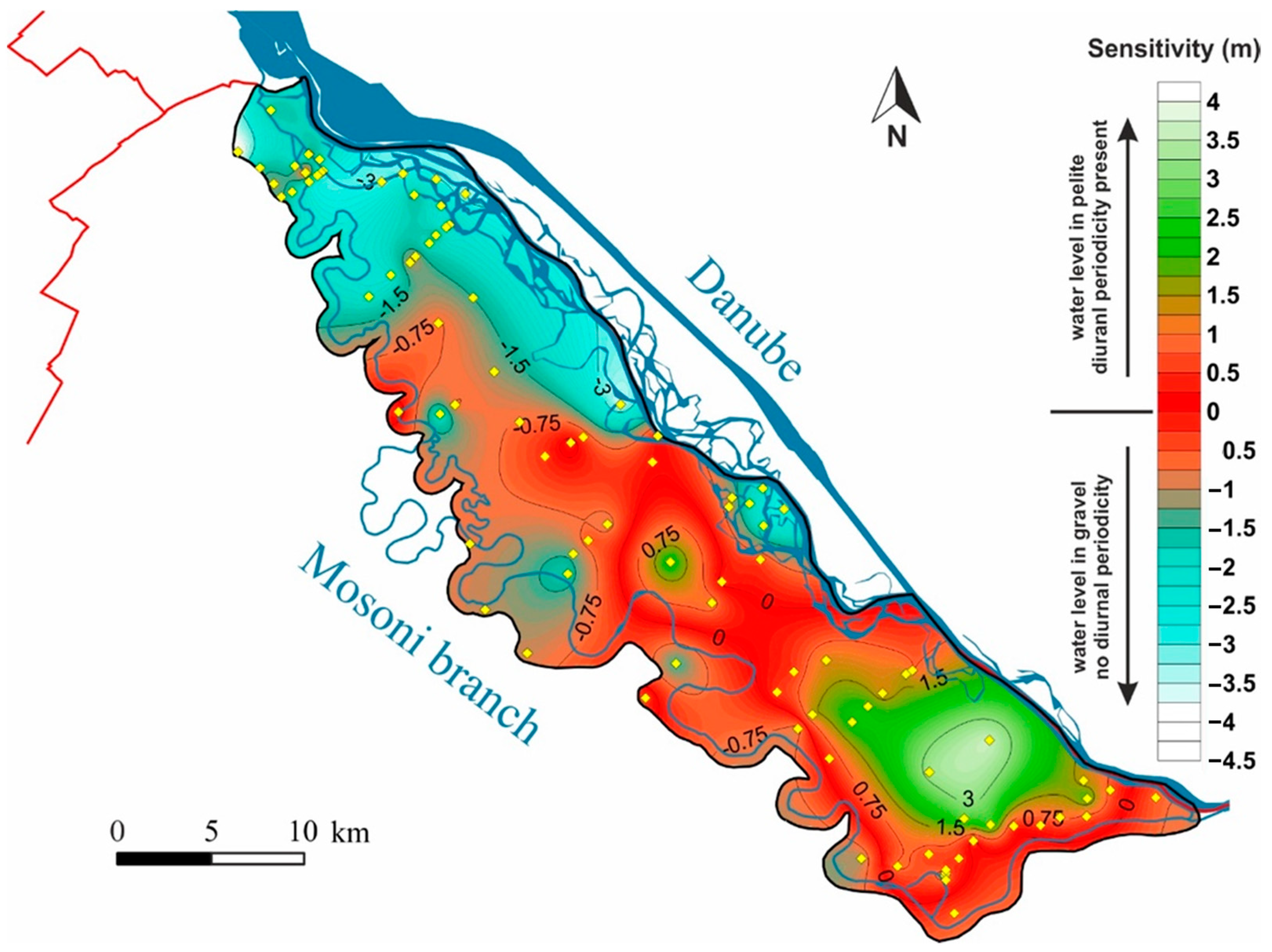
3.6. Sensitivity of Unsaturated Zone to Possible Future Changes in Groundwater Level
- Due to the lack of moisture in the upper layers of the soil, the productivity and actual yield of agriculture decreases.
- The altered moisture conditions may affect natural ecological communities, changing the plant community and, as a result, the habitat as well. A habitat change of such a nature usually results in a decrease in biodiversity [95] and contributes to the spreading of pioneer and invasive species [96], which can then alter the landscape [86].
- The changing water balance may affect the physical and chemical properties of soils and may even cause the degradation of the soil, which can be to the further detriment of the water balance of the soil.
- Groundwater levels decreasing due to localized anthropogenic effects or major evapotranspiration resulting from climate change (e.g., drought) may affect vulnerable water tables directly, and these events thus have a serious negative impact on groundwater supply.
4. Conclusions and Outlook
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Alley, W.M.; Healy, R.W.; LaBaugh, J.W.; Reilly, T.E. Flow and Storage in Groundwater Systems. Science 2002, 296, 1985–1990. [Google Scholar] [CrossRef] [PubMed]
- Siebert, S.; Burke, J.; Faures, J.M.; Frenken, K.; Hoogeveen, J.; Döll, P.; Portmann, F.T. Groundwater use for irrigation—A global inventory. Hydrol. Earth Syst. Sci. 2010, 14, 1863–1880. [Google Scholar] [CrossRef]
- MacDonald, A.M.; Bonsor, H.C.; Dochartaigh, B.É.Ó.; Taylor, R.G. Quantitative maps of groundwater resources in Africa. Environ. Res. Lett. 2012, 7, 024009. [Google Scholar] [CrossRef]
- Hughes, J.D.; Petrone, K.C.; Silberstein, R.P. Drought, groundwater storage and stream flow decline in southwestern Australia. Geophys. Res. Lett. 2012, 39, L03408. [Google Scholar] [CrossRef]
- Wada, Y.; van Beek, L.P.H.; van Kempen, C.M.; Reckman, J.W.T.M.; Vasak, S.; Bierkens, M.F.P. Global depletion of groundwater resources. Geophys. Res. Lett. 2010, 37, L20402. [Google Scholar] [CrossRef]
- Xanke, J.; Liesch, T. Quantification and possible causes of declining groundwater resources in the Euro-Mediterranean region from 2003 to 2020. Hydrogeol. J. 2022, 30, 379–400. [Google Scholar] [CrossRef]
- Qi, P.; Zhang, G.; Xu, Y.J.; Wang, L.; Ding, C.; Cheng, C. Assessing the Influence of Precipitation on Shallow Groundwater Table Response Using a Combination of Singular Value Decomposition and Cross-Wavelet Approaches. Water 2018, 10, 598. [Google Scholar] [CrossRef]
- Tóth, Á.; Baják, P.; Szijártó, M.; Tiljander, M.; Korkka-Niemi, K.; Hendriksson, N.; Mádl-Szőnyi, J. Multimethodological Revisit of the Surface Water and Groundwater Interaction in the Balaton Highland Region—Implications for the Overlooked Groundwater Component of Lake Balaton, Hungary. Water 2023, 15, 1006. [Google Scholar] [CrossRef]
- Czauner, B.; Erőss, A.; Szkolnikovics-Simon, S.; Markó, Á.; Baják, P.; Trásy-Havril, T.; Szijártó, M.; Szabó, Z.; Hegedűs-Csondor, K.; Mádl-Szőnyi, J. From basin-scale groundwater flow to integrated geofluid research in the hydrogeology research group of Eötvös Loránd University, Hungary. J. Hydrol. X 2022, 17, 100142. [Google Scholar] [CrossRef]
- Galsa, A.; Tóth, Á.; Szijártó, M.; Pedretti, D.; Mádl-Szőnyi, J. Interaction of basin-scale topography- and salinity-driven groundwater flow in synthetic and real hydrogeological systems. J. Hydrol. 2022, 609, 127695. [Google Scholar] [CrossRef]
- Barthel, R.; Banzhaf, S. Groundwater and Surface Water Interaction at the Regional-scale—A Review with Focus on Regional Integrated Models. Water Resour. Manag. 2016, 30, 1–32. [Google Scholar] [CrossRef]
- Kalbus, E.; Reinstorf, F.; Schirmer, M. Measuring methods for groundwater–surface water interactions: A review. Hydrol. Earth Syst. Sci. 2006, 10, 873–887. [Google Scholar] [CrossRef]
- Garamhegyi, T.; Kovács, J.; Pongrácz, R.; Tanos, P.; Hatvani, I.G. Investigation of the climate-driven periodicity of shallow groundwater level fluctuations in a Central-Eastern European agricultural region. Hydrogeol. J. 2018, 26, 677–688. [Google Scholar] [CrossRef]
- Magyar, N.; Hatvani, I.G.; Arató, M.; Trásy, B.; Blaschke, A.P.; Kovács, J. A New Approach in Determining the Decadal Common Trends in the Groundwater Table of the Watershed of Lake “Neusiedlersee”. Water 2021, 13, 290. [Google Scholar] [CrossRef]
- Han, Z.; Huang, S.; Huang, Q.; Leng, G.; Wang, H.; Bai, Q.; Zhao, J.; Ma, L.; Wang, L.; Du, M. Propagation dynamics from meteorological to groundwater drought and their possible influence factors. J. Hydrol. 2019, 578, 124102. [Google Scholar] [CrossRef]
- Molnar, S.; Molnar, M.; Csabragi, A. Impact Assessment Of Mitigation Strategies in the Hungarian Agriculture. J. Agric. Inform. 2011, 2, 10–17. [Google Scholar] [CrossRef][Green Version]
- Alexander, L.V.; Zhang, X.; Peterson, T.C.; Caesar, J.; Gleason, B.; Klein Tank, A.M.G.; Haylock, M.; Collins, D.; Trewin, B.; Rahimzadeh, F.; et al. Global observed changes in daily climate extremes of temperature and precipitation. J. Geophys. Res. Atmos. 2006, 111, 1–22. [Google Scholar] [CrossRef]
- Kurylyk, B.L.; MacQuarrie, K.T.B.; Voss, C.I. Climate change impacts on the temperature and magnitude of groundwater discharge from shallow, unconfined aquifers. Water Resour. Res. 2014, 50, 3253–3274. [Google Scholar] [CrossRef]
- Whitehead, P.G.; Wilby, R.L.; Battarbee, R.W.; Kernan, M.; Wade, A.J. A review of the potential impacts of climate change on surface water quality. Hydrol. Sci. J. 2009, 54, 101–123. [Google Scholar] [CrossRef]
- Bouraoui, F.; Vachaud, G.; Li, L.Z.X.; Le Treut, H.; Chen, T. Evaluation of the impact of climate changes on water storage and groundwater recharge at the watershed scale. Clim. Dyn. 1999, 15, 153–161. [Google Scholar] [CrossRef]
- Spane, F.A. Considering barometric pressure in groundwater flow investigations. Water Resour. Res. 2002, 38, 14-11–14-18. [Google Scholar] [CrossRef]
- Robins, N.S.; Finch, J.W. Groundwater flood or groundwater-induced flood? Q. J. Eng. Geol. Hydrogeol. 2012, 45, 119–122. [Google Scholar] [CrossRef]
- Li, B.; Rodell, M.; Famiglietti, J. Groundwater Variability across Temporal and Spatial Scales in the Central and Northeastern U.S. J. Hydrol. 2015, 525, 769–780. [Google Scholar] [CrossRef]
- Blöschl, G.; Bierkens, M.F.P.; Chambel, A.; Cudennec, C.; Destouni, G.; Fiori, A.; Kirchner, J.W.; McDonnell, J.J.; Savenije, H.H.G.; Sivapalan, M.; et al. Twenty-three unsolved problems in hydrology (UPH)—A community perspective. Hydrol. Sci. J. 2019, 64, 1141–1158. [Google Scholar] [CrossRef]
- Liu, S.; Huang, S.; Xie, Y.; Leng, G.; Huang, Q.; Wang, L.; Xue, Q. Spatial-temporal changes of rainfall erosivity in the loess plateau, China: Changing patterns, causes and implications. CATENA 2018, 166, 279–289. [Google Scholar] [CrossRef]
- Edenhofer, O.; Seyboth, K. Intergovernmental Panel on Climate Change (IPCC); United Nations: Vienna, Austria, 2013; pp. 48–56. [Google Scholar]
- Molnár, S.; Molnar, M. Comprehensive assessment of climate change policies and measures in Hungary: Concerns and tasks in an underestimated challenge. Idojaras 2012, 116, 297–321. [Google Scholar]
- Zeng, R.; Cai, X. Analyzing streamflow changes: Irrigation-enhanced interaction between aquifer and streamflow in the Republican River basin. Hydrol. Earth Syst. Sci. 2014, 18, 493–502. [Google Scholar] [CrossRef]
- Jaramillo, M. Riverbank filtration: An efficient and economical drinking-water treatment technology. DYNA 2012, 79, 148–157. [Google Scholar]
- Chapman, D.V.; Bradley, C.; Gettel, G.M.; Hatvani, I.G.; Hein, T.; Kovács, J.; Liska, I.; Oliver, D.M.; Tanos, P.; Trásy, B.; et al. Developments in water quality monitoring and management in large river catchments using the Danube River as an example. Environ. Sci. Policy 2016, 64, 141–154. [Google Scholar] [CrossRef]
- Winter, T.C.; Harvey, J.W.; Franke, O.L.; Alley, W.M. Ground Water and Surface Water: A Single Resource; Circular 1139; U.S. Department of the Interior, U.S. Geological Survey: Reston, VA, USA, 1998. [Google Scholar]
- Apurv, T.; Sivapalan, M.; Cai, X. Understanding the Role of Climate Characteristics in Drought Propagation. Water Resour. Res. 2017, 53, 9304–9329. [Google Scholar] [CrossRef]
- Taormina, R.; Chau, K.-W.; Sethi, R. Artificial neural network simulation of hourly groundwater levels in a coastal aquifer system of the Venice lagoon. Eng. Appl. Artif. Intell. 2012, 25, 1670–1676. [Google Scholar] [CrossRef]
- Trásy, B.; Magyar, N.; Havril, T.; Kovács, J.; Garamhegyi, T. The Role of Environmental Background Processes in Determining Groundwater Level Variability—An Investigation of a Record Flood Event Using Dynamic Factor Analysis. Water 2020, 12, 2336. [Google Scholar] [CrossRef]
- Smith, S.E.; Büttner, G.; Szilagyi, F.; Horvath, L.; Aufmuth, J. Environmental Impacts of River Diversion: Gabcikovo Barrage System. J. Water Resour. Plan. Manag. 2000, 126, 138–145. [Google Scholar] [CrossRef]
- Jansky, L.; Pachova, N.I.; Murakami, M. The Danube: A case study of sharing international waters. Glob. Environ. Chang. 2004, 14, 39–49. [Google Scholar] [CrossRef]
- Völgyesi, I. A Kisalföld talajvíz-és rétegvíz helyzete. Hidrol. Közlöny 1994, 74, 260–268. [Google Scholar]
- Trásy, B.; Garamhegyi, T.; Laczkó-Dobos, P.; Kovács, J.; István Gábor, H. Geostatistical screening of flood events in the groundwater levels of the diverted inner delta of the Danube River: Implications for river bed clogging. Open Geosci. 2018, 10, 64–78. [Google Scholar] [CrossRef]
- Kovács, J.; Márkus, L.; Szalai, J.; Kovács, I.S. Detection and evaluation of changes induced by the diversion of River Danube in the territorial appearance of latent effects governing shallow-groundwater fluctuations. J. Hydrol. 2015, 520, 314–325. [Google Scholar] [CrossRef]
- Trásy, B.; József, K.; István Gábor, H.; Timea, H.; Tibor, N.; Péter, S.; Csaba, S. Assessment of the interaction between surface- and sub-surface waters after the diversion in the inner delta of the River Danube using multivariate statistics. Anthropocene 2018, 22, 14. [Google Scholar] [CrossRef]
- Deák, J.; László, F.; Liebe, P. A felszín alatti vizek utánpótlódásának, áramlási viszonyainak, szintjének és minőségének változása. In Proceedings of the A Szigetközi Környezeti Monitoring Eredményei, Mosonmagyaróvár, Budapest, Hungary, 20–21 February 2002. [Google Scholar]
- ÉDUKÖVIZIG. (North-Transdanubian Water Directorate); ÉDUKÖVIZIG: Győr, Hungary, 2014. [Google Scholar]
- Trásy, B. The Effects of River-Diversion on the Behavior of Shallow Groundwater in the Szigetköz Inner Delta of the River Danube (Hungary). Ph.D. Thesis, Eötvös Loránd University, Budapest, Hungary, 2018. [Google Scholar]
- Scharek, P.; Don, G.; Horvath, I.; Toth, G. Results of the modern depositional process and hydrogeologic investigations in Szigetkoz, Hungary. Operation of a geologic monitoring system by the geological institute of Hungary. Acta Geol. Hung. 2000, 43, 85–106. [Google Scholar]
- EC. EU Water Framework Directive; EC Directive; EC: Maastricht, The Netherlands, 2000; Volume 60. [Google Scholar]
- Allen, R.G.; Pereira, L.S.; Raes, D.; Smith, M. Crop Evapotranspiration-Guidelines for Computing Crop Water Requirements; FAO Irrigation and Drainage Paper 56; FAO: Rome, Italy, 1998; Volume 300, p. D05109. [Google Scholar]
- Allen, R.G.; Pruitt, W.O.; Wright, J.L.; Howell, T.A.; Ventura, F.; Snyder, R.; Itenfisu, D.; Steduto, P.; Berengena, J.; Yrisarry, J.B. A recommendation on standardized surface resistance for hourly calculation of reference ETo by the FAO56 Penman-Monteith method. Agric. Water Manag. 2006, 81, 1–22. [Google Scholar] [CrossRef]
- Available online: https://cds.climate.copernicus.eu (accessed on 2 February 2018).
- Available online: https://www.ncei.noaa.gov (accessed on 2 February 2018).
- Pearson, K. Note on Regression and Inheritance in the Case of Two Parents. Proc. R. Soc. Lond. 1895, 58, 240–242. [Google Scholar]
- Pörtge, K.-H. Tagesperiodische Schwankungen des Abflusses in Kleinen Einzugsgebieten als Ausdruck Komplexer Wasser-und Stoffflüsse; E. Goltze: Berlin, Germany, 1996. [Google Scholar]
- Dingman, S.L. Physical Hydrology; Waveland Press: Long Grove, IL, USA, 2015. [Google Scholar]
- Wang, F.; Wang, X.; Zhao, Y.; Yang, Z. Long-term changes of water level associated with chlorophyll a concentration in Lake Baiyangdian, North China. Procedia Environ. Sci. 2012, 13, 1227–1237. [Google Scholar] [CrossRef][Green Version]
- Nalley, D.; Adamowski, J.; Khalil, B.; Biswas, A. Inter-annual to inter-decadal streamflow variability in Quebec and Ontario in relation to dominant large-scale climate indices. J. Hydrol. 2016, 536, 426–446. [Google Scholar] [CrossRef]
- Salamalikis, V.; Argiriou, A.A.; Dotsika, E. Periodicity analysis of δ18O in precipitation over Central Europe: Time–frequency considerations of the isotopic ‘temperature’ effect. J. Hydrol. 2016, 534, 150–163. [Google Scholar] [CrossRef]
- Sen, A.K.; Kern, Z. Wavelet analysis of low-frequency variability in oak tree-ring chronologies from east Central Europe. Open Geosci. 2016, 8, 478–483. [Google Scholar] [CrossRef]
- Grinsted, A.; Moore, J.C.; Jevrejeva, S. Application of the cross wavelet transform and wavelet coherence to geophysical time series. Nonlinear Process. Geophys. 2004, 11, 561–566. [Google Scholar] [CrossRef]
- Kovács, J.; Hatvani, I.G.; Korponai, J.; Kovács, I.S. Morlet wavelet and autocorrelation analysis of long-term data series of the Kis-Balaton water protection system (KBWPS). Ecol. Eng. 2010, 36, 1469–1477. [Google Scholar] [CrossRef]
- Morlet, J.; Arens, G.; Fourgeau, E.; Glard, D. Wave propagation and sampling theory—Part I: Complex signal and scattering in multilayered media. Geophysics 1982, 47, 203–221. [Google Scholar] [CrossRef]
- Hatvani, I.G.; Kern, Z.; Leél-Őssy, S.; Demény, A. Speleothem stable isotope records for east-central Europe: Resampling sedimentary proxy records to obtain evenly spaced time series with spectral guidance. Earth Syst. Sci. Data 2018, 10, 139–149. [Google Scholar] [CrossRef]
- Rösch, A.; Schmidbauer, H. WaveletComp 1.1: A guided Tour through the R Package. 2014. Available online: http://www.hs-stat.com/projects/WaveletComp/WaveletComp_guided_tour.pdf (accessed on 2 February 2018).
- Torrence, C.; Compo, G.P. A practical guide to wavelet analysis. Bull. Am. Meteorol. Soc. 1998, 79, 61–78. [Google Scholar] [CrossRef]
- R Core Team. R: A Language and Environment for Statistical Computing; R Foundation for Statistical Computing: Vienna, Austria, 2021. [Google Scholar]
- Kovács, J.; Márkus, L.; Szalai, J.; Barcza, M.; Bernáth, G.; Kovácsné Székely, I.; Halupka, G. Exploring Potentially Hazardous Areas for Water Quality Using Dynamic Factor Analysis. In Water Quality; Kostas, V., Dimitra, V., Eds.; IntechOpen: Rijeka, Croatia, 2012; Chapter 9. [Google Scholar]
- Nagy, B.; Ignéczi, Á.; Kovács, J.; Szalai, Z.; Mari, L. Shallow ground temperature measurements on the highest volcano of the Earth, the Mt. Ojos del Salado, Arid Andes, Chile. Permafr. Periglac. Process. 2018; in press. [Google Scholar]
- Karátson, D. Pannon enciklopédia. In Magyarország Földje–Kitekintéssel a Kárpát-Medence Egészére (Pannonian Encyclopedia: The Land of Hungary with an Outlook to the Whole Carpathian Basin); Kertek: Budapest, Hungary, 2000. [Google Scholar]
- Thornthwaite, C.W. An approach toward a rational classification of climate. Geogr. Rev. 1948, 38, 55–94. [Google Scholar] [CrossRef]
- Gribovszki, Z.; Szilágyi, J.; Kalicz, P. Diurnal fluctuations in shallow groundwater levels and streamflow rates and their interpretation—A review. J. Hydrol. 2010, 385, 371–383. [Google Scholar] [CrossRef]
- Hatvani, I.G.; Clement, A.; Korponai, J.; Kern, Z.; Kovács, J. Periodic signals of climatic variables and water quality in a river–eutrophic pond–wetland cascade ecosystem tracked by wavelet coherence analysis. Ecol. Indic. 2017, 83, 21–31. [Google Scholar] [CrossRef]
- Kollet, S.J.; Maxwell, R.M. Capturing the influence of groundwater dynamics on land surface processes using an integrated, distributed watershed model. Water Resour. Res. 2008, 44, W02402. [Google Scholar] [CrossRef]
- Fredlund, D.G.; Rahardjo, H. An Overview of Unsaturated Soil Behaviour. In Proceedings of the ASCE Specialty Session Unsaturated Soil Properties, Dallas, TX, USA, 24–28 October 1993. [Google Scholar]
- Fredlund, D. The scope of unsaturated soil mechanics: An overview. In Proceedings of the First International Conference on Unsaturated Soils, Paris, France, 6–8 September 1995. [Google Scholar]
- De Vries, D. Simultaneous transfer of heat and moisture in porous media. Eos Trans. Am. Geophys. Union 1958, 39, 909–916. [Google Scholar]
- Domenico, P.A.; Schwartz, F.W. Physical and Chemical Hydrogeology; Wiley: New York, NY, USA, 1998; Volume 506. [Google Scholar]
- Kozeny, J. Das tägliche periodische steigen und fallen des grundwasserspiegels. Die Wasserwirtsch. 1933, 31, 424–427. [Google Scholar]
- Ubell, K. Über die gesetzmassigkeiten des grundwassergangs and des grundwasserhaushalts in flachlandgebieten. Wasserwirtsch. Wassertech. 1961, 11, 366–372. [Google Scholar]
- Meyboom, P. Three observations on streamflow depletion by phreatophytes. J. Hydrol. 1965, 2, 248–261. [Google Scholar] [CrossRef]
- Reigner, I.C. A method of estimating steamflow loss by evapotranspiration from the riparian zone. For. Sci. 1966, 12, 130–139. [Google Scholar]
- Bond, B.J.; Jones, J.A.; Moore, G.; Phillips, N.; Post, D.; McDonnell, J.J. The zone of vegetation influence on baseflow revealed by diel patterns of streamflow and vegetation water use in a headwater basin. Hydrol. Process. 2002, 16, 1671–1677. [Google Scholar] [CrossRef]
- White, W.N. A Method of Estimating Ground-Water Supplies Based on Discharge by Plants and Evaporation from Soil: Results of Investigations in Escalante Valley, Utah; US Government Printing Office: Washington, DC, USA, 1932; Volume 659. [Google Scholar]
- Rodríguez-Iturbe, I.; Porporato, A. Ecohydrology of Water-Controlled Ecosystems: Soil Moisture and Plant Dynamics; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Bauer, P.; Thabeng, G.; Stauffer, F.; Kinzelbach, W. Estimation of the evapotranspiration rate from diurnal groundwater level fluctuations in the Okavango Delta, Botswana. J. Hydrol. 2004, 288, 344–355. [Google Scholar] [CrossRef]
- Nachabe, M.; Shah, N.; Ross, M.; Vomacka, J. Evapotranspiration of Two Vegetation Covers in a Shallow Water Table Environment. Soil Sci. Soc. Am. J. 2005, 69, 492–499. [Google Scholar] [CrossRef]
- Thal-Larsen, J.H. Fluctuations in the level of the phreatic surface with an atmospheric deposit in the form of dew. Bodenkd. Forsch. 1934, 4, 223–233. [Google Scholar]
- Moreo, M.T.; Andraski, B.J.; Garcia, C.A. Groundwater Discharge by Evapotranspiration, Flow of Water in Unsaturated Soil, and Stable Isotope Water Sourcing in Areas of Sparse Vegetation, Amargosa Desert, Nye County, Nevada; 2328-0328; US Geological Survey: Reston, VA, USA, 2017. [Google Scholar]
- Euliss, N.H.; Mushet, D.M. Water-level fluctuation in wetlands as a function of landscape condition in the prairie pothole region. Wetlands 1996, 16, 587–593. [Google Scholar] [CrossRef]
- Loheide, S.P.; Butler, J.J.; Gorelick, S.M. Estimation of groundwater consumption by phreatophytes using diurnal water table fluctuations: A saturated-unsaturated flow assessment. Water Resour. Res. 2005, 41, W07030. [Google Scholar] [CrossRef]
- Seneviratne, S.I.; Corti, T.; Davin, E.L.; Hirschi, M.; Jaeger, E.B.; Lehner, I.; Orlowsky, B.; Teuling, A.J. Investigating soil moisture–climate interactions in a changing climate: A review. Earth-Sci. Rev. 2010, 99, 125–161. [Google Scholar] [CrossRef]
- Hirschi, M.; Mueller, B.; Dorigo, W.; Seneviratne, S.I. Using remotely sensed soil moisture for land–atmosphere coupling diagnostics: The role of surface vs. root-zone soil moisture variability. Remote Sens. Environ. 2014, 154, 246–252. [Google Scholar] [CrossRef]
- Heiler, G.; Hein, T.; Schiemer, F.; Bornette, G. Hydrological connectivity and flood pulses as the central aspects for the integrity of a river-floodplain system. Regul. Rivers Res. Manag. 1995, 11, 351–361. [Google Scholar] [CrossRef]
- Hudon, C. Impact of water level fluctuations on St. Lawrence River aquatic vegetation. Can. J. Fish. Aquat. Sci. 1997, 54, 2853–2865. [Google Scholar] [CrossRef]
- Trebitz, A.S. Characterizing Seiche and Tide-driven Daily Water Level Fluctuations Affecting Coastal Ecosystems of the Great Lakes. J. Great Lakes Res. 2006, 32, 102–116. [Google Scholar] [CrossRef]
- Schälchli, U. The clogging of coarse gravel river beds by fine sediment. In Sediment/Water Interactions: Proceedings of the Fifth International Symposium; Hart, B.T., Sly, P.G., Eds.; Springer: Dordrecht, The Netherlands, 1992; pp. 189–197. [Google Scholar]
- Lewandowski, J.; Lischeid, G.; Nützmann, G. Drivers of water level fluctuations and hydrological exchange between groundwater and surface water at the lowland River Spree (Germany): Field study and statistical analyses. Hydrol. Process. 2009, 23, 2117–2128. [Google Scholar] [CrossRef]
- Van Tol, G.; Dobben, H.v.; Schmidt, P.; Klap, J. Biodiversity of Dutch forest ecosystems as affected by receding groundwater levels and atmospheric deposition. Biodivers. Conserv. 1998, 7, 221–228. [Google Scholar]
- Pyšek, P.; Richardson, D.M. Invasive species, environmental change and management, and health. Annu. Rev. Environ. Resour. 2010, 35, 25–55. [Google Scholar] [CrossRef]
- Trásy-Havril, T.; Szkolnikovics-Simon, S.; Mádl-Szőnyi, J. How Complex Groundwater Flow Systems Respond to Climate Change Induced Recharge Reduction? Water 2022, 14, 3026. [Google Scholar] [CrossRef]
- Dillon, P.; Pavelic, P.; Page, D.; Beringen, H.; Ward, J. Managed Aquifer Recharge: An Introduction; Waterlines Report Series no. 13, February 2009; National Water Commisssion: Canberra, Australia, 2009. [Google Scholar]
- Imig, A.; Szabó, Z.; Halytsia, O.; Vrachioli, M.; Kleinert, V.; Rein, A. A review on risk assessment in managed aquifer recharge. Integr. Environ. Assess. Manag. 2022, 18, 1513–1529. [Google Scholar] [CrossRef] [PubMed]
- Szabó, Z.; Szijártó, M.; Tóth, Á.; Mádl-Szőnyi, J. The Significance of Groundwater Table Inclination for Nature-Based Replenishment of Groundwater-Dependent Ecosystems by Managed Aquifer Recharge. Water 2023, 15, 1077. [Google Scholar] [CrossRef]
- Simon, S.; Déri-Takács, J.; Szijártó, M.; Szél, L.; Mádl-Szőnyi, J. Wetland Management in Recharge Regions of Regional Groundwater Flow Systems with Water Shortage, Nyírség Region, Hungary. Water 2023, 15, 3589. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Trásy, B.; Magyar, N.; Hatvani, I.G.; Garamhegyi, T.; Kovács-Székely, I.; Kovács, J.; Trásy-Havril, T. Drivers of Daily Water Level Fluctuation of Shallow Groundwater in the Inner Delta of the River Danube. Water 2024, 16, 2011. https://doi.org/10.3390/w16142011
Trásy B, Magyar N, Hatvani IG, Garamhegyi T, Kovács-Székely I, Kovács J, Trásy-Havril T. Drivers of Daily Water Level Fluctuation of Shallow Groundwater in the Inner Delta of the River Danube. Water. 2024; 16(14):2011. https://doi.org/10.3390/w16142011
Chicago/Turabian StyleTrásy, Balázs, Norbert Magyar, István Gábor Hatvani, Tamás Garamhegyi, Ilona Kovács-Székely, József Kovács, and Tímea Trásy-Havril. 2024. "Drivers of Daily Water Level Fluctuation of Shallow Groundwater in the Inner Delta of the River Danube" Water 16, no. 14: 2011. https://doi.org/10.3390/w16142011
APA StyleTrásy, B., Magyar, N., Hatvani, I. G., Garamhegyi, T., Kovács-Székely, I., Kovács, J., & Trásy-Havril, T. (2024). Drivers of Daily Water Level Fluctuation of Shallow Groundwater in the Inner Delta of the River Danube. Water, 16(14), 2011. https://doi.org/10.3390/w16142011







