The Feedback from a Beach Berm during Post-Storm Recovery and How to Improve the Berm’s Restorative Efficiency
Abstract
1. Introduction
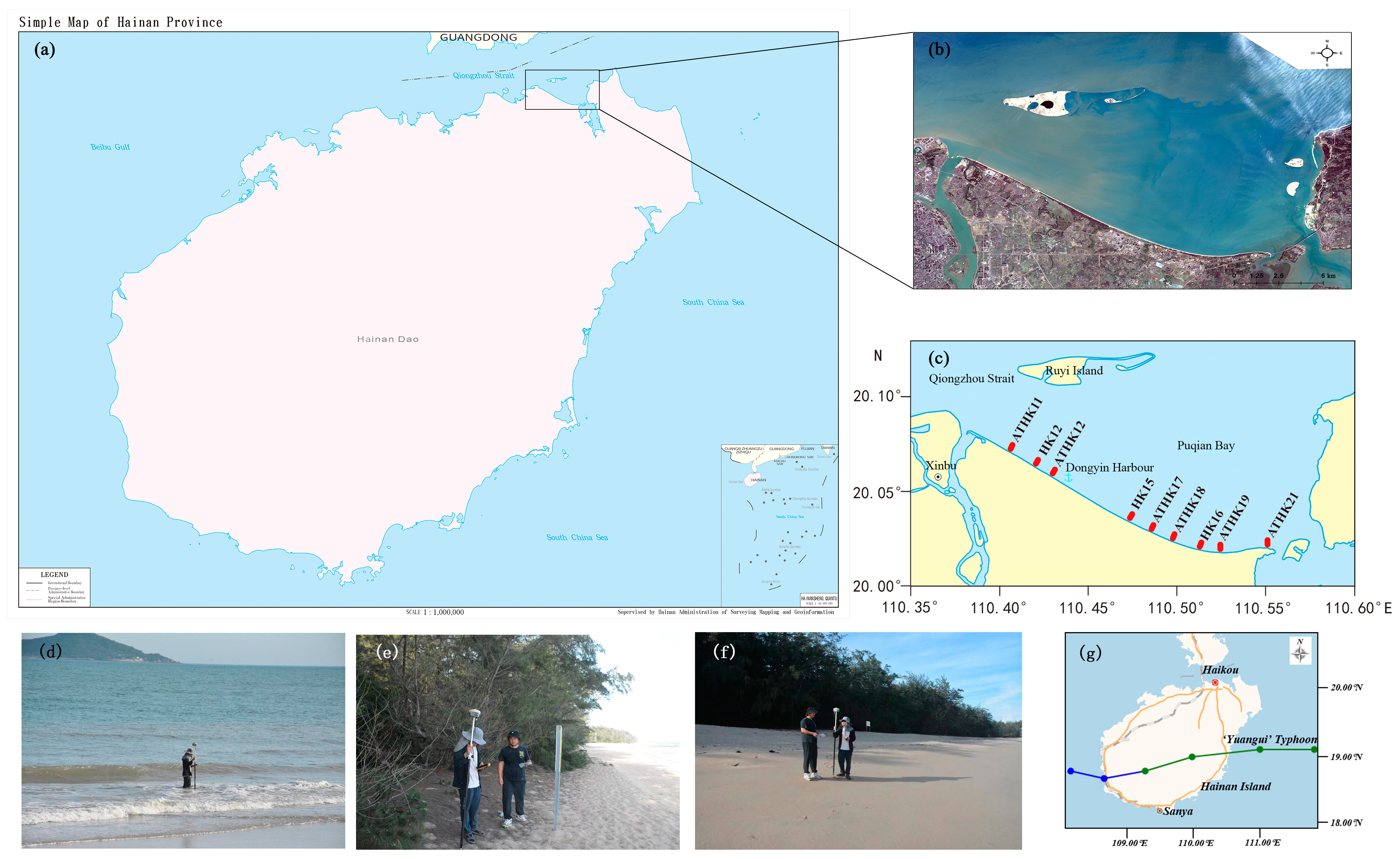
2. Study Site
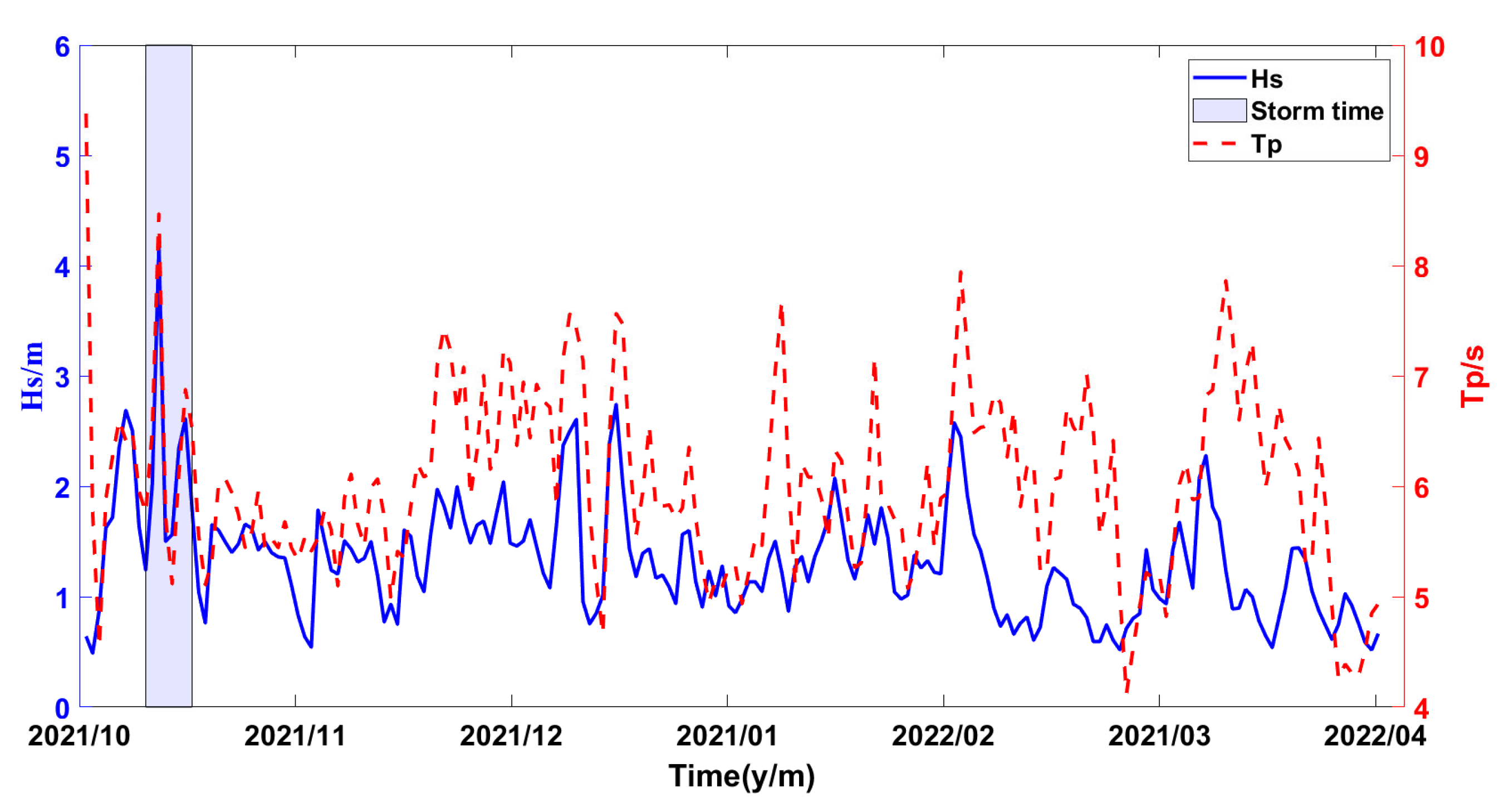
3. Methods
3.1. RTK-GPS Profiles Survey
3.2. XBeach Model
3.3. Dynamic Database
3.4. Model Setup
3.5. Model Validation
4. Results
4.1. Volume Change
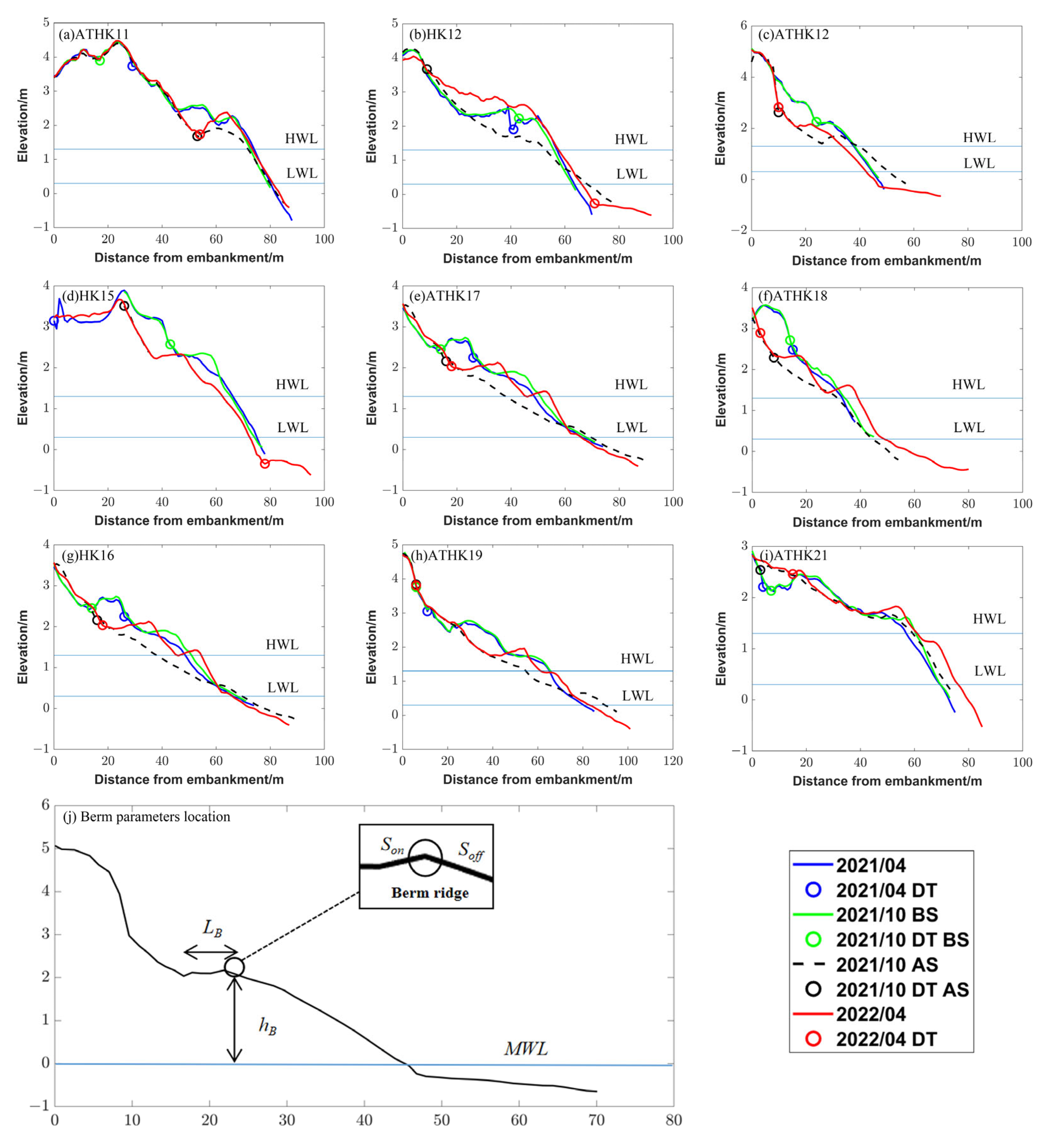
4.2. Berm Recovery
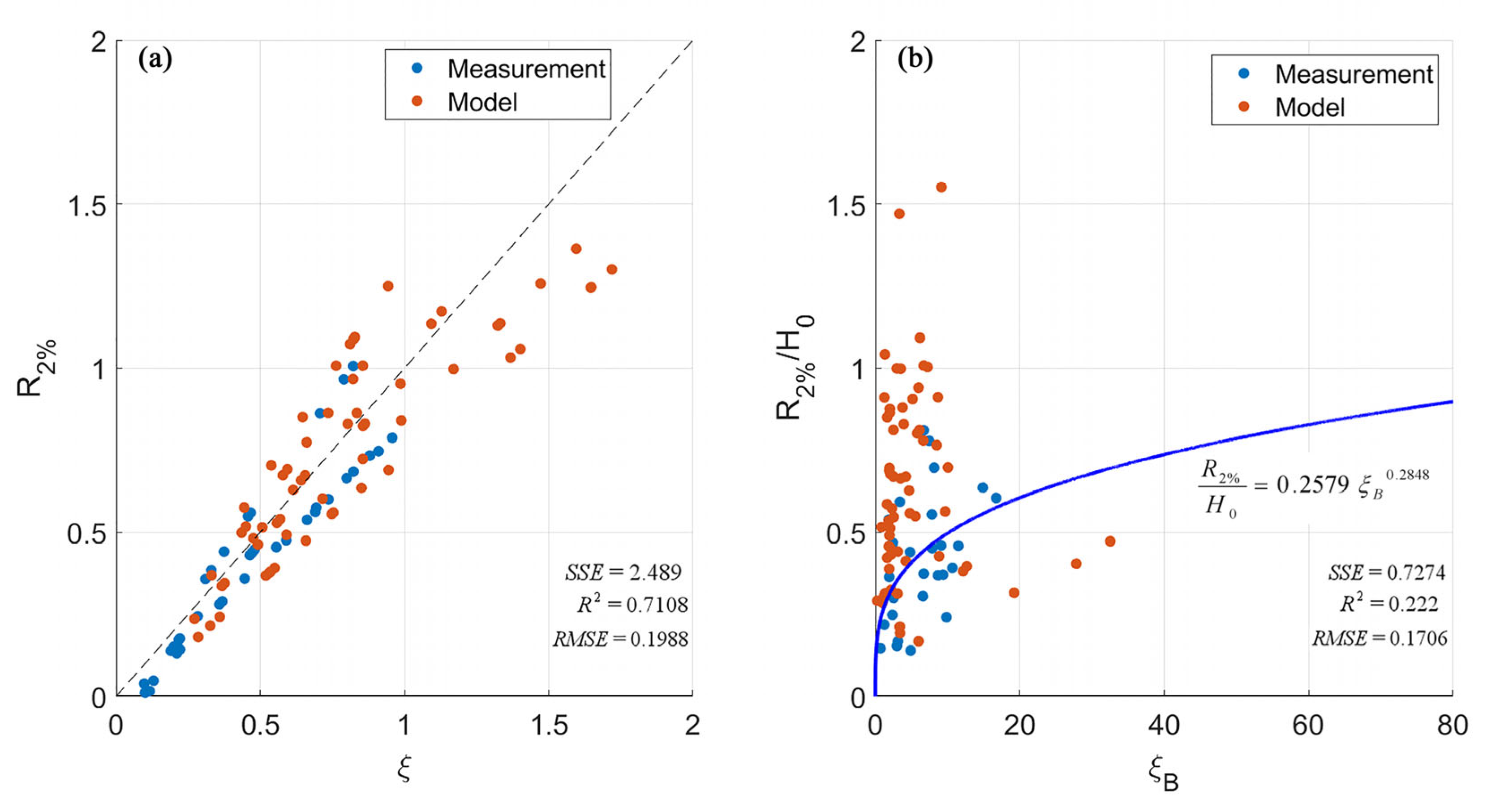
4.3. How the Berm Effects Wave Run-Up and Volume Change
5. Discussion: How to Improve Coast Resilience
6. Conclusions
- Supratidal erosion and intertidal accretion are induced by storm waves in the profile. The recovery efficiency of berm elevation exceeds that of the beach berm platform width, with the intertidal zone demonstrating the fastest overall recovery efficiency while that above the HWL is slower.
- The height of the berm ridge is shifted landward and increased during storm waves, while it is lowered and shifted seaward during moderate waves. This movement is governed by a periodic cycle aligned with the circulation of the storm–moderate waves.
- Under the influence of storms, the slopes on both sides of the berm ridge point change in opposite directions. However, during the accumulation of moderate waves, the slopes on both sides of the berm ridge gradually change in the opposite direction.
- The empirical wave run-up model of Stockdon neglects berm conditions, resulting in the underestimation of smooth and wide berm platforms and the overestimation of non-platform berms. The new model, which combines the berm ridge and platform width, reflects the dynamic interaction between waves and profiles. The new model modifies the elevation of the run-up peaks to align more closely with the berm ridge point.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Smart, L.S.; Vukomanovic, J.; Sills, E.O.; Sanchez, G. Cultural ecosystem services caught in a ‘coastal squeeze’ between sea level rise and urban expansion. Glob. Environ. Chang. 2021, 66, 102209. [Google Scholar] [CrossRef]
- Rangel-Buitrago, N.; Williams, A.T.; Anfuso, G. Hard protection structures as a principal coastal erosion management strategy along the Caribbean coast of Colombia. A chronicle of pitfalls. Ocean Coast. Manag. 2017, 156, 58–75. [Google Scholar] [CrossRef]
- Shi, J.; Feng, X.; Toumi, R.; Zhang, C.; Hodges, K.I.; Tao, A.; Zhang, W.; Zheng, J. Global increase in tropical cyclone ocean surface waves. Nat. Commun. 2024, 15, 174. [Google Scholar] [CrossRef]
- Clifton, G.A.; Dafforn, K.A.; Bishop, M.J. The ecological benefits of adding topographic complexity to seawalls vary across estuarine gradients. Ecol. Eng. J. Ecotechnol. 2022, 182, 106735. [Google Scholar] [CrossRef]
- Zhou, Y.; Feng, X.; Liu, M.; Wang, W. Influence of Beach Erosion during Wave Action in Designed Artificial Sandy Beach Using XBeach Model: Profiles and Shoreline. J. Mar. Sci. Eng. 2023, 11, 984. [Google Scholar] [CrossRef]
- Peng, S.; Tang, C. Blending the analytic hierarchy process and fuzzy logical systems in scenic beauty assessment of check dams in streams. Water 2015, 7, 6983–6998. [Google Scholar] [CrossRef]
- Djuma, H.; Bruggeman, A.; Camera, C.; Eliades, M.; Kostarelos, K. The impact of a check dam on groundwater recharge and sedimentation in an ephemeral stream. Water 2017, 9, 813. [Google Scholar] [CrossRef]
- Kuang, C.; Ma, Y.; Han, X.; Pan, S.; Zhu, L. Experimental observation on beach evolution process with presence of artificial submerged sand bar and reef. J. Mar. Sci. Eng. 2020, 8, 1019. [Google Scholar] [CrossRef]
- Kuang, C.; Mao, X.; Gu, J.; Niu, H.; Ma, Y.; Yang, Y.; Qiu, R.; Zhang, J. Morphological processes of two artificial submerged shore-parallel sandbars for beach nourishment in a nearshore zone. Ocean Coast Manag. 2019, 179, 104870. [Google Scholar] [CrossRef]
- Koster, L. Humplike Nourishing of the Shoreface. A Study on More Efficient Nourishing of the Shoreface. 2006. Available online: https://repository.tudelft.nl/record/uuid:e354c6da-99bb-4419-8419-31654896027f (accessed on 9 April 2024).
- Dette, H.H.; Larson, M.; Murphy, J.; Newe, J.; Peters, K.; Reniers AJ, H.M.; Steetzel, H. Application of prototype flume tests for beach nourishment assessment. Coast. Eng. 2002, 47, 137–177. [Google Scholar] [CrossRef]
- Žilinskas, G.; Pupienis, D.; Jarmalavičius, D. Possibilities of regeneration of Palanga coastal zone. J. Environ. Eng. Landsc. Manag. 2010, 18, 92–101. [Google Scholar] [CrossRef]
- Pan, Y.; Yin, S.; Chen, Y.; Yang, Y.; Xu, C.; Xu, Z. An experimental study on the evolution of a submerged berm under the effects of regular waves in low-energy conditions. Coast. Eng. 2022, 176, 104169. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, C.; Zhao, S.; Qi, H.; Cai, F.; Zheng, J. Equilibrium configurations of sandy-muddy transitional beaches on South China coasts: Role of waves in formation of sand-mud transition boundary. Coast. Eng. 2024, 187, 104401. [Google Scholar] [CrossRef]
- Zhu, J.; Shi, F.; Cai, F.; Wang, Q.; Qi, H.; Zhang, C.; Liu, J.; Liu, G.; Lei, G. Influences of beach berm height on beach response to storms: A numerical study. Appl. Ocean Res. 2022, 121, 103090. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, C.; Song, J.; Chi, S.; Zhao, S.; Qi, H.; Shi, J. Tide-modulated wave characteristics and breaking regimes in the intertidal zone of a dissipative beach. Ocean Eng. 2022, 266, 113055. [Google Scholar] [CrossRef]
- You, Z.; Laine, R.; Hanslow, D.J.; Baldock, T.; Wiecek, D. Field measurements of beach-dune dynamic profiles to assess erosion hazard on the coast of NSW, Australia. Coast. Eng. Proc. 2014, 34, 23. [Google Scholar] [CrossRef]
- Chen, J.M. Numerical simulation of tidal current and sediment movement in Ruyi island of Haikou bay. Water 2018, 15, 2516. [Google Scholar]
- Duo, E.; Sanuy, M.; Jiménez, J.A.; Ciavola, P. How good are symmetric triangular synthetic storms to represent real events for coastal hazard modelling. Coast. Eng. 2020, 159, 103728. [Google Scholar] [CrossRef]
- Van Ormondt, M.; Nelson, T.R.; Hapke, C.J.; Roelvink, D. Morphodynamic modelling of the wilderness breach, Fire Island, New York. Part I: Model set-up and validation. Coast. Eng. 2020, 157, 103621. [Google Scholar] [CrossRef]
- Bennett, W.G.; Karunarathna, H.; Reeve, D.E.; Mori, N. Computational modelling of morphodynamic response of a macro-tidal beach to future climate variabilities. Mar. Geol. 2019, 415, 105960. [Google Scholar] [CrossRef]
- Didier, D.; Baudry, J.; Bernatchez, P.; Dumont, D.; Sadegh, M.; Bismuth, E.; Bandet, M.; Dugas, S.; Sévigny, C. Multihazard simulation for coastal flood mapping: Bathtub versus numerical modelling in an open estuary, Eastern Canada. J. Flood Risk Manag. 2019, 12 (Suppl. S1), e12505. [Google Scholar] [CrossRef]
- Galappatti, G.; Vreugdenhil, C.B. A depth-integrated model for suspended sediment transport. J. Hydraul. Res. 1985, 23, 359–377. [Google Scholar] [CrossRef]
- Roelvink, D.; Reniers, A.; Van Dongeren, A.; van Thiel De Vries, J.; McCall, R.; Lescinski, J. Modelling storm impacts on beaches, dunes and barrier islands. Coast. Eng. 2009, 56, 1133–1152. [Google Scholar] [CrossRef]
- Lu, Y.; Li, S.; Zuo, L.; Liu, H.; Roelvink, J. Advances in sediment transport under combined action of waves and currents. Int. J. Sediment Res. 2015, 30, 351–360. [Google Scholar] [CrossRef]
- Stockdon, H.F.; Sallenger, A.H., Jr.; Holman, R.A.; Howd, P.A. A simple model for the spatially-variable coastal response to hurricanes. Mar. Geol. 2007, 238, 1–20. [Google Scholar] [CrossRef]
- Cao, Y.G.; Bai, Y.C.; Chen, Y.Z.; Ouyang, Y.Z.; Feng, Y.; Jiang, L.; Liao, S.; Xiao, Z. Analysis of siltation trend and mechanism in the eastern sea area of Haikou Bay, Hainan, China. Oceanol. Limnol. Sin. 2022, 53, 906–916. [Google Scholar]
- Chen, L.H.; Lin, G.Y.; Gong, W.P. The impact of artificial island construction on the headland vortex and bed level change in headland-bay coastal systems: A case study in Puqian Bay, Hainan Island. Haiyang Xuebao 2019, 41, 41–50. [Google Scholar]
- Li, Y.; Zhang, C.; Dai, W.; Chen, D.; Sui, T.; Xie, M.; Chen, S. Laboratory investigation on morphology response of submerged artificial sandbar and its impact on beach evolution under storm wave condition. Mar. Geol. 2022, 443, 106668. [Google Scholar] [CrossRef]
- Battjes, J.A. Surf similarity. Coast. Eng. 1974, 1974, 466–480. [Google Scholar]
- Stockdon, H.F.; Holman, R.A.; Howd, P.A.; Sallenger, J.A. Empirical parameterization of setup, swash, and runup. Coast. Eng. 2006, 53, 573–588. [Google Scholar] [CrossRef]
- Park, H.; Cox, D.T.; Petroff, C.M. An empirical solution for tsunami run-up on compound slopes. Nat. Hazards 2015, 76, 1727–1743. [Google Scholar] [CrossRef]




| Parameter | ATHK11 | HK12 | ATHK12 | HK15 | HK16 | ATHK17 | ATHK18 | ATHK19 | ATHK21 |
|---|---|---|---|---|---|---|---|---|---|
| CFL | 0.9 | 0.9 | 0.9 | 0.9 | 0.9 | 0.9 | 0.9 | 0.9 | 0.9 |
| morfac | 10 | 10 | 50 | 10 | 10 | 10 | 10 | 10 | 10 |
| reposeangle | 40 | 40 | 40 | 40 | 40 | 40 | 40 | 40 | 40 |
| wetslp | 1.0 | 0.1 | 0.1 | 0.2 | 0.2 | 0.2 | 0.2 | 0.2 | 0.2 |
| dryslp | 2.0 | 1.0 | 0.8 | 0.7 | 0.5 | 0.2 | 0.5 | 0.2 | 1.0 |
| D50 | 0.4 | 0.4 | 0.4 | 0.4 | 0.4 | 0.4 | 0.4 | 0.4 | 0.4 |
| por | 0.4 | 0.4 | 0.4 | 0.4 | 0.4 | 0.4 | 0.4 | 0.4 | 0.4 |
| facAs | 1.0 | 1.0 | 0.7 | 0.8 | 0.8 | 0.5 | 0.8 | 0.8 | 0.8 |
| fasSK | 0.1 | 0.5 | 0.1 | 0.5 | 0.5 | 0.6 | 0.5 | 0.6 | 0.5 |
| Volume/m3 | Profile | October 2021 | Novermber 2021 | December 2021 | January 2022 | February 2022 | March 2022 | April 2022 |
|---|---|---|---|---|---|---|---|---|
| Over HWL | ATHK11 | 125.45 | 128.45 | 129.70 | 123.84 | 126.10 | 126.05 | 133.52 |
| HK12 | 67.24 | 69.68 | 77.68 | 85.30 | 85.53 | 85.50 | 94.48 | |
| ATHK12 | 55.97 | 63.22 | 63.51 | 62.31 | 62.96 | 62.54 | 62.60 | |
| HK15 | 82.22 | 86.13 | 87.11 | 85.30 | 84.61 | 86.60 | 88.82 | |
| ATHK17 | 23.69 | 24.30 | 28.97 | 33.32 | 34.39 | 34.53 | 31.60 | |
| ATHK18 | 46.53 | 48.38 | 53.66 | 56.92 | 57.90 | 58.81 | 54.51 | |
| HK16 | 37.93 | 38.05 | 37.71 | 51.02 | 52.78 | 52.89 | 45.97 | |
| ATHK19 | 69.05 | 70.27 | 69.54 | 72.23 | 73.83 | 75.58 | 75.87 | |
| ATHK21 | 44.51 | 47.36 | 49.32 | 56.78 | 57.67 | 57.03 | 47.95 | |
| Between HWL and LWL | ATHK11 | 73.94 | 73.83 | 72.24 | 73.14 | 70.46 | 69.23 | 76.33 |
| HK12 | 59.14 | 59.17 | 65.00 | 69.28 | 69.15 | 69.16 | 61.35 | |
| ATHK12 | 48.03 | 52.11 | 47.21 | 52.12 | 46.55 | 49.97 | 41.65 | |
| HK15 | 65.27 | 64.99 | 68.08 | 66.19 | 69.62 | 68.25 | 67.05 | |
| ATHK17 | 38.96 | 47.17 | 45.10 | 44.66 | 49.03 | 48.38 | 43.92 | |
| ATHK18 | 46.37 | 48.14 | 5.95 | 56.66 | 56.83 | 56.14 | 51.63 | |
| HK16 | 51.63 | 51.60 | 52.66 | 59.05 | 56.17 | 56.12 | 57.76 | |
| ATHK19 | 69.32 | 71.97 | 71.56 | 77.75 | 77.78 | 76.73 | 72.63 | |
| ATHK21 | 64.68 | 64.69 | 71.83 | 72.89 | 72.86 | 72.48 | 69.40 |
| Slope | Year/Month | ATHK11 | HK12 | ATHK12 | HK15 | ATHK17 | ATHK18 | ATHK19 | HK16 | ATHK21 |
|---|---|---|---|---|---|---|---|---|---|---|
| Soff | April 2021 | 0.075 | 0.121 | 0.113 | 0.046 | 0.059 | 0.048 | 0.110 | 0.095 | 0.008 |
| October 2021 BS | 0.087 | 0.116 | 0.074 | 0.122 | 0.093 | 0.022 | 0.020 | 0.083 | 0.002 | |
| October 2021 AS | 0.021 | 0.067 | 0.066 | 0.026 | 0.065 | 0.025 | 0.022 | 0.036 | 0.001 | |
| April 2022 | 0.138 | 0.049 | 0.077 | 0.075 | 0.133 | 0.069 | 0.053 | 0.118 | 0.005 | |
| Son | April 2021 | −0.015 | −0.062 | −0.032 | −0.007 | 0.060 | 0.038 | 0.028 | 0.035 | −0.031 |
| October 2021 BS | −0.011 | −0.011 | −0.013 | −0.010 | −0.002 | −0.004 | −0.003 | 0.016 | −0.100 | |
| October 2021 AS | −0.041 | −0.013 | −0.054 | −0.001 | 0.028 | 0.043 | −0.008 | 0.014 | 0.052 | |
| April 2022 | −0.035 | −0.014 | −0.025 | −0.012 | −0.023 | −0.006 | −0.003 | −0.019 | −0.062 |
| Parameters | LB | hB | tanβoff | tanβon | R2% | ξB |
|---|---|---|---|---|---|---|
| Volume change above HWL | 0.055 | 0.391 | 0.423 | 0.211 | 0.357 | 0.241 |
| Volume change between HWL and LWL | 0.397 | 0.408 | 0.289 | 0.246 | 0.234 | 0.287 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, Y.; Zhou, Y.; Zeng, W.; Feng, W.; Jiang, Y. The Feedback from a Beach Berm during Post-Storm Recovery and How to Improve the Berm’s Restorative Efficiency. Water 2024, 16, 1955. https://doi.org/10.3390/w16141955
Zhu Y, Zhou Y, Zeng W, Feng W, Jiang Y. The Feedback from a Beach Berm during Post-Storm Recovery and How to Improve the Berm’s Restorative Efficiency. Water. 2024; 16(14):1955. https://doi.org/10.3390/w16141955
Chicago/Turabian StyleZhu, Yu, Yingtao Zhou, Weite Zeng, Weibing Feng, and Yuanshu Jiang. 2024. "The Feedback from a Beach Berm during Post-Storm Recovery and How to Improve the Berm’s Restorative Efficiency" Water 16, no. 14: 1955. https://doi.org/10.3390/w16141955
APA StyleZhu, Y., Zhou, Y., Zeng, W., Feng, W., & Jiang, Y. (2024). The Feedback from a Beach Berm during Post-Storm Recovery and How to Improve the Berm’s Restorative Efficiency. Water, 16(14), 1955. https://doi.org/10.3390/w16141955






