Assessment of the Midseason Crop Coefficient for the Evaluation of the Water Demand of Young, Grafted Hazelnut Trees in High-Density Orchards
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Site Description and Tree Sampling
- 625 trees ha−1, spaced 4 m between rows and 4 m on the row, used as a control treatment (the common density used by farmers);
- 1250 trees ha−1, spaced 4 m × 2 m;
- 2500 trees ha−1, spaced 4 m × 1 m.
2.2. Manual Measurements
2.3. Evaluation of the Hazelnut Orchard’s Crop Coefficients Using the FAO66 Method
Evaluation of Tree Transpiration Tr
- To calculate the using Equation (3);
- To derive the values of the product from Equation (4). Substituting the values, obtained from Equation (9), the radiation extinction coefficients were evaluated, and the coefficients of Equation (5) were determined for the hazelnut orchard.
2.4. Estimation of the Hazelnut Orchard’s Crop Coefficients from the Fraction of Ground Cover and Height (Allen and Pereira [16])
3. Results
3.1. Application of the FAO66 Method
3.2. Application of the Allen and Pereira Method [15]
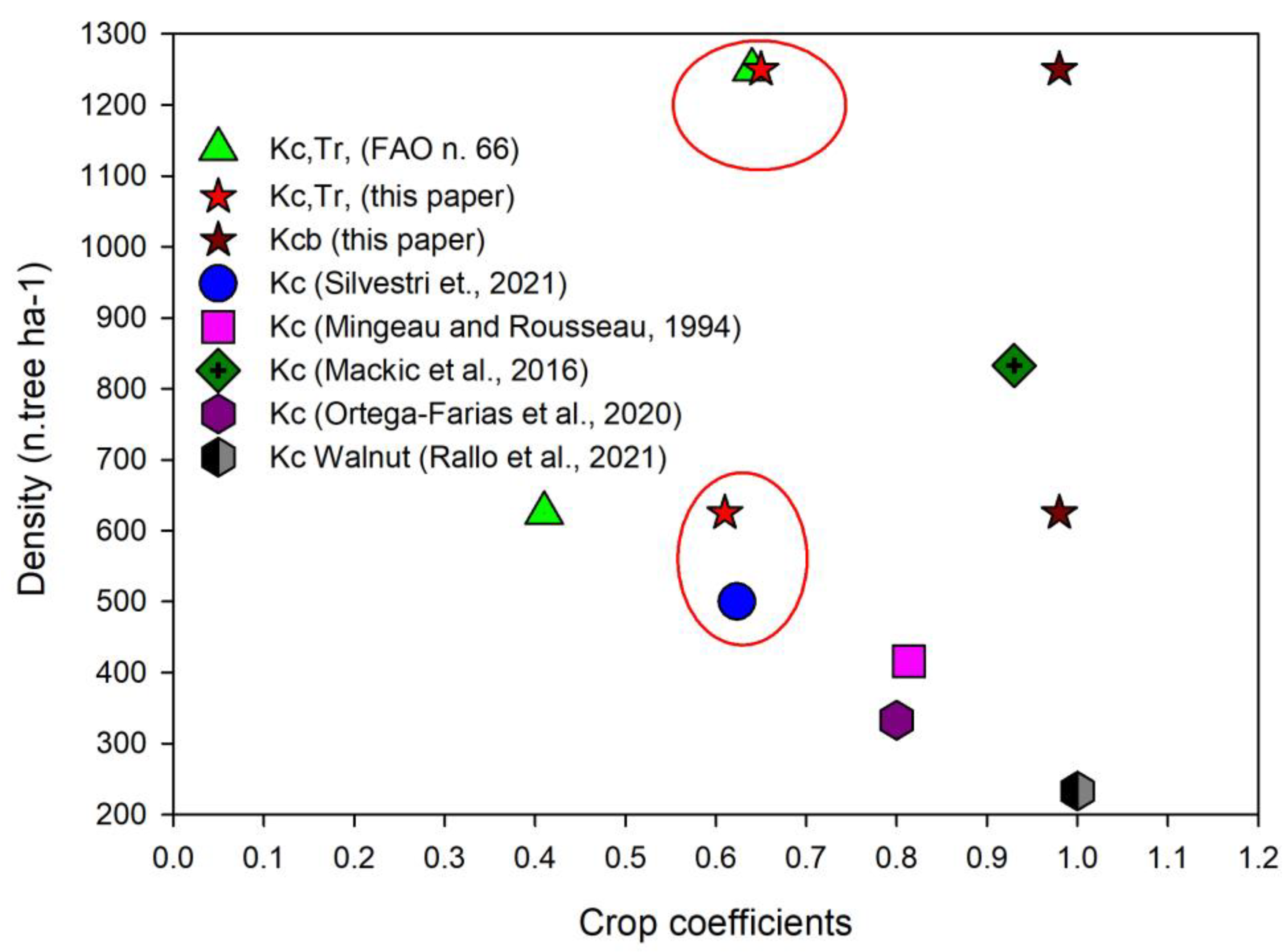
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Noto, L.V.; Cipolla, G.; Pumo, D.; Francipane, A. Climate Change in the Mediterranean Basin (Part II): A Review of Challenges and Uncertainties in Climate Change Modeling and Impact Analyses. Water Resour. Manag. 2023. [Google Scholar] [CrossRef]
- Vergni, L.; Vinci, A.; Todisco, F. Effectiveness of the new standardized deficit distance index and other meteorological indices in the assessment of agricultural drought impacts in central Italy. J. Hydrol. 2021, 603, 126986. [Google Scholar] [CrossRef]
- Vergni, L.; Vinci, A.; Todisco, F.; Santaga, F.S.; Vizzari, M. Comparing Sentinel-1, Sentinel-2, and Landsat-8 data in the early recognition of irrigated areas in central Italy. J. Agric. Eng. 2021, 52, 1265. [Google Scholar] [CrossRef]
- Vinci, A.; Vergni, L.; Todisco, F.; Mannocchi, F. Analysis of rainfed alfalfa evapotranspiration measured by an eddy covariance system. J. Agric. Eng. 2013, 44, 779–784. [Google Scholar] [CrossRef]
- Levidow, L.; Zaccaria, D.; Maia, R.; Vivas, R.; Todorovic, M.; Scardigno, A. Improving water-efficient irrigation: Prospects and difficulties of innovative practices. Agric. Water Manag. 2014, 146, 84–94. [Google Scholar] [CrossRef]
- Allen, R.G.; Pereira, L.S.; Raes, D.; Smith, M. Crop evapotranspiration. guidelines for computing crop water requirements. In FAO Irrigation and Drainage Paper; FAO: Rome, Italy, 1998; p. 300. Available online: https://www.fao.org/3/X0490E/x0490e00.htm#Contents (accessed on 10 January 2023).
- Steduto, P.; Hsiao, T.C.; Fereres, E.; Raes, D. Crop yield response to water. In FAO Irrigation and Drainage Paper; FAO: Rome, Italy, 2012; Available online: https://www.fao.org/3/i2800e/i2800e00.htm (accessed on 10 January 2023).
- Pereira, L.S.; Paredes, P.; Lopez-Urrea, R.; Hunsaker, D.J.; Mota, M.; Mohammadi Shad, Z. Standard single and basal crop coefficients for vegetable crops, an update of FAO56 crop water requirements approach. Agric. Water Manag. 2021, 241, 106197. [Google Scholar] [CrossRef]
- Rallo, G.; Paço, T.A.; Puig, A.; Paredes, P.; Massai, R.; Provenzano, G.; Pereira, L.S. Updated single and dual crop coefficients for tree and vine fruit crops. Agric. Water Manag. 2021, 250, 106645. [Google Scholar] [CrossRef]
- Mačkić, K.; Pejić, B.; Belić, M.; Janković, D.; Pavlović, L. Hazelnut (Corylus avellana L.) response to microsprinkler irrigation in climatic conditions of Vojvodina province. Res. J. Agric. Sci. 2016, 48, 1–7. [Google Scholar]
- Ortega-Farias, S.; Villalobos-Soublett, E.; Riveros-Burgos, C.; Zúñiga, M.; Ahumada-Orellana, L.E. Effect of irrigation cut-off strategies on yield, water productivity and gas exchange in a drip-irrigated hazelnut (Corylus avellana L. cv. Tonda di Giffoni) orchard under semiarid conditions. Agric. Water Manag. 2020, 238, 106173. [Google Scholar] [CrossRef]
- Silvestri, C.; Bacchetta, L.; Bellincontro, A.; Cristofori, V. Advances in cultivar choice, hazelnut orchard management, and nut storage to enhance product quality and safety: An overview. J. Sci. Food Agric. 2021, 101, 27–43. [Google Scholar] [CrossRef]
- Mingeau, M.; Rousseau, P. Water use of hazelnut trees as measured with lysimeters. Acta Hotic. 1992, 351, 315–322. [Google Scholar] [CrossRef]
- Portarena, S.; Gavrichkova, O.; Brugnoli, E.; Battistelli, A.; Proietti, S.; Moscatello, S.; Famiani, F.; Tombesi, S.; Zadra, C.; Farinelli, D. Carbon allocation strategies and water uptake in young grafted and own-rooted hazelnut (Corylus avellana L.) cultivars. Tree Physiol. 2022, 42, 939–957. [Google Scholar] [CrossRef] [PubMed]
- Allen, R.G.; Pereira, L.S. Estimating crop coefficients from fraction of ground cover and height. Irrig. Sci. 2009, 28, 17–34. [Google Scholar] [CrossRef]
- Tombesi, A. Effect of Light Penetration on High-Density Filbert Planting; Institute of Fruit Culture, University of Perugia: Perugia, Italy, 1977; Volume 32, p. 33. [Google Scholar]
- Spectrum Technologies Inc. WatchDog 2000 Series Mini Stations product Manual. Available online: https://www.specmeters.com/assets/1/22/2000_Series_Mini_Loggers_2021.pdf (accessed on 13 February 2023).
- Vinci, A.; Traini, C.; Farinelli, D.; Brigante, R. Assessment of the geometrical characteristics of hazelnut intensive orchard by an Unmanned Aerial Vehicle (UAV). In Proceedings of the 2022 IEEE Workshop on Metrology for Agriculture and Forestry (MetroAgriFor), Perugia, Italy, 3–5 November 2022; pp. 218–222. [Google Scholar] [CrossRef]
- Vinci, A.; Brigante, R.; Traini, C.; Farinelli, D. Geometrical Characterization of Hazelnut Trees in an Intensive Orchard by an Unmanned Aerial Vehicle (UAV) for Precision Agriculture Applications. Remote Sens. 2023, 15, 541. [Google Scholar] [CrossRef]
- Sarkar, S.; Cazenave, A.B.; Oakes, J.; McCall, D.; Thomason, W.; Abbott, L.; Balota, M. Aerial high-throughput phenotyping of peanut leaf area index and lateral growth. Sci. Rep. 2021, 11, 21661. [Google Scholar] [CrossRef] [PubMed]
- Mathews, A.J.; Jensen, J.L. Visualizing and quantifying vineyard canopy LAI using an unmanned aerial vehicle (UAV) collected high density structure from motion point cloud. Remote Sens. 2013, 5, 2164–2183. [Google Scholar] [CrossRef]
- Ilniyaz, O.; Du, Q.; Shen, H.; He, W.; Feng, L.; Azadi, H.; Kurban, A.; Chen, X. Leaf area index estimation of pergola-trained vineyards in arid regions using classical and deep learning methods based on UAV-based RGB images. Comput. Electron. Agric. 2023, 207, 107723. [Google Scholar] [CrossRef]
- Farinelli, D.; Boco, M.; Tombesi, A. Influence of canopy density on fruit growth and flower formation. Acta Hortic. 2005, 686, 247–252. [Google Scholar] [CrossRef]
- SigmaScan Pro5, Inpixon Richmond, California (USA). Available online: https://systat.co.uk/ (accessed on 14 February 2023).
- Souto, C.; Lagos, O.; Holzapfel, E.; Ruybal, C.; Bryla, D.R.; Vidal, G. Evaluating a Surface Energy Balance Model for Partially Wetted Surfaces: Drip and Micro-Sprinkler Systems in Hazelnut Orchards (Corylus avellana L.). Water 2022, 14, 4011. [Google Scholar] [CrossRef]
- Testi, L.; Goldhamer, D.A.; Iniesta, F.; Salinas, M. Crop Water Stress Index Is a Sensitive Water Stress Indicator in Pistachio Trees. Irrig. Sci. 2008, 26, 395–405. [Google Scholar] [CrossRef]
- Orgaz, F.; Testi, L.; Villalobos, F.J.; Fereres, E. Water requirements of olive orchards II: Determination of crop coefficients for irrigation scheduling. Irrig. Sci. 2006, 24, 77–84. [Google Scholar] [CrossRef]
- Allen, R.G.; Pereira, L.S.; Smith, M.; Raes, D.; Wright, J.L. FAO-56 dual crop coefficient method for estimating evaporation from soil and application extensions. J. Irrig. Drain. Eng. 2005, 131, 2–13. [Google Scholar] [CrossRef]
- Pereira, L.S.; Allen, R.G.; Smith, M.; Raes, D. Crop evapotranspiration estimation with FAO56: Past and future. Agric. Water Manag. 2015, 147, 4–20. [Google Scholar] [CrossRef]
- Pereira, L.S.; Paredes, P.; Melton, F.; Johnson, L.; Wang, T.; López-Urrea, R.; Cancela, J.J.; Allen, R.G. Prediction of crop coefficients from fraction of ground cover and height. Background and validation using ground and remote sensing data. Agric. Water Manag. 2021, 241, 106197. [Google Scholar] [CrossRef]
- Pereira, L.S.; Paredes, P.; Hunsaker, D.J.; Lopez-Urrea, R.; Mohammadi Shad, Z. Standard single and basal crop coefficients for field crops. Updates and advances to the FAO56 crop water requirements method. Agric. Water Manag. 2021, 243, 106466. [Google Scholar] [CrossRef]
- Calvo, F.E.; Trentacoste, E.R.; Silvente, S.T. Vegetative growth, yield, and crop water productivity response to different irrigation regimes in high density walnut orchards (Juglans regia L.) in a semi-arid environment in Argentina. Agric. Water Manag. 2022, 274, 107969. [Google Scholar] [CrossRef]
- Portarena, S.; Leonardi, L.; Scartazza, A.; Lauteri, M.; Baldacchini, C.; Farinelli, D.; Famiani, F.; Ciolfi, M.; Brugnoli, E. Combining analysis of fatty acid composition and δ13C in extra-virgin olive oils as affected by harvest period and cultivar: Possible use in traceability studies. Food Control 2019, 105, 151–158. [Google Scholar] [CrossRef]
- Cincera, I.; Frioni, T.; Ughini, V.; Poni, S.; Farinelli, D.; Tombesi, S. Intra-specific variability of stomatal sensitivity to vapour pressure deficit in Corylus avellana L.: A candidate factor influencing different adaptability to different climates? J. Plant Physiol. 2019, 232, 241–247. [Google Scholar] [CrossRef]
- Di Lena, B.; Curci, G.; Vergni, L.; Farinelli, D. Climatic Suitability of Different Areas in Abruzzo, Central Italy, for the Cultivation of Hazelnut. Horticulturae 2022, 8, 580. [Google Scholar] [CrossRef]
- Altieri, G.; Maffia, A.; Pastore, V.; Amato, M.; Celano, G. Use of high-resolution multispectral UAVs to calculate projected ground area in Corylus avellana L. tree orchard. Sensors 2022, 22, 7103. [Google Scholar] [CrossRef]
| 625 Trees/ha | 1250 Trees/ha | 2500 Trees/ha | |
|---|---|---|---|
| Equations (3)–(8) | 0.41 c | 0.64 a | 0.61 b |
| Equations (3), (4), (17) and (18) | 0.61 b | 0.65 ab | 0.74 a |
| DAFm | Qd | fc | |
|---|---|---|---|
| 625 trees/ha | 1.56 | 0.535 | 0.74 |
| 2.54 | 0.635 | ||
| 2.78 | 0.837 | ||
| 2.66 | 0.835 | ||
| 2.49 | 0.856 | ||
| 2.38 | 0.799 | ||
| 1.60 | 0.882 | ||
| 2.47 | 0.856 | ||
| 5.63 | 0.768 | ||
| 3.99 | 0.644 | ||
| 4.56 | 0.535 | ||
| 1250 trees/ha | 3.13 | 0.863 | 0.795 |
| 1.47 | 0.828 | ||
| 1.48 | 0.714 | ||
| 1.96 | 0.856 | ||
| 2.35 | 0.737 | ||
| 1.80 | 0.844 | ||
| 2.40 | 0.934 | ||
| 2.41 | 0.806 | ||
| 5.89 | 0.532 | ||
| 3.26 | 0.889 | ||
| 7.01 | 0.748 | ||
| 2500 trees/ha | 4.50 | 0.829 | 0.895 |
| 6.41 | 0.858 | ||
| 2.58 | 0.895 | ||
| 4.48 | 0.902 | ||
| 5.06 | 0.885 | ||
| 3.51 | 0.912 | ||
| 2.81 | 0.920 | ||
| 4.26 | 0.943 | ||
| 3.85 | 0.936 | ||
| 4.09 | 0.935 | ||
| 4.53 | 0.897 | ||
| 3.62 | 0.824 |
| 625 trees/ha | 1250 trees/ha | 2500 trees/ha | |
|---|---|---|---|
| Equation (13) | 0.98 b | 0.98 b | 1.04 a |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vinci, A.; Traini, C.; Portarena, S.; Farinelli, D. Assessment of the Midseason Crop Coefficient for the Evaluation of the Water Demand of Young, Grafted Hazelnut Trees in High-Density Orchards. Water 2023, 15, 1683. https://doi.org/10.3390/w15091683
Vinci A, Traini C, Portarena S, Farinelli D. Assessment of the Midseason Crop Coefficient for the Evaluation of the Water Demand of Young, Grafted Hazelnut Trees in High-Density Orchards. Water. 2023; 15(9):1683. https://doi.org/10.3390/w15091683
Chicago/Turabian StyleVinci, Alessandra, Chiara Traini, Silvia Portarena, and Daniela Farinelli. 2023. "Assessment of the Midseason Crop Coefficient for the Evaluation of the Water Demand of Young, Grafted Hazelnut Trees in High-Density Orchards" Water 15, no. 9: 1683. https://doi.org/10.3390/w15091683
APA StyleVinci, A., Traini, C., Portarena, S., & Farinelli, D. (2023). Assessment of the Midseason Crop Coefficient for the Evaluation of the Water Demand of Young, Grafted Hazelnut Trees in High-Density Orchards. Water, 15(9), 1683. https://doi.org/10.3390/w15091683









