Design the Water Tariff Structure: Application and Assessment of a Model to Balance Sustainability, Cost Recovery and Wise Use
Abstract
:1. Introduction
2. State of the Art
3. Model
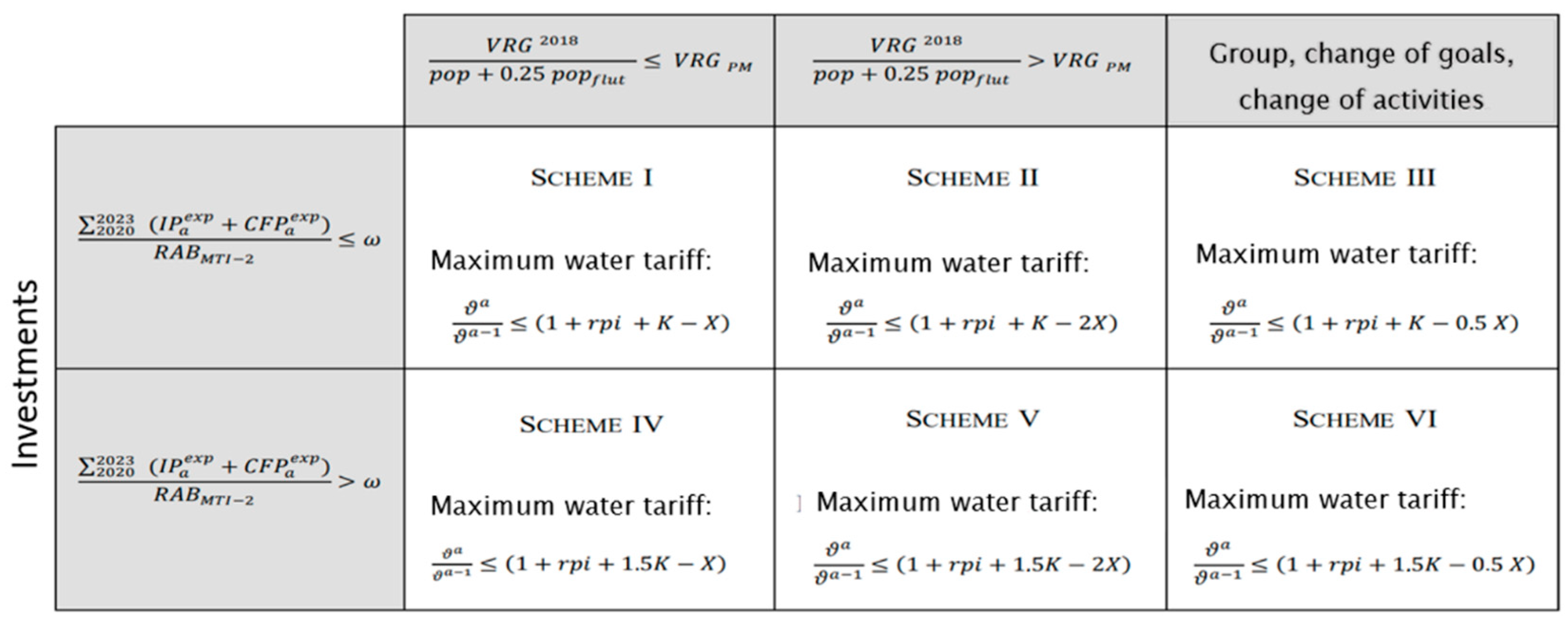
3.1. Model Constraints
- ensuring a facilitated and continuous water supply for the largest families, with significant discounts aimed at guaranteeing everyone a sufficient volume of drinking water in the order of 50 L per inhabitant per day, equal to 18.25 cubic meters of water per year;
- increasing the level of severity towards those who do not pay, so as not to jeopardize innovation in the sector due to physiological arrears;
- encouraging the sustainable use of water resources through measures aimed at reducing water waste.
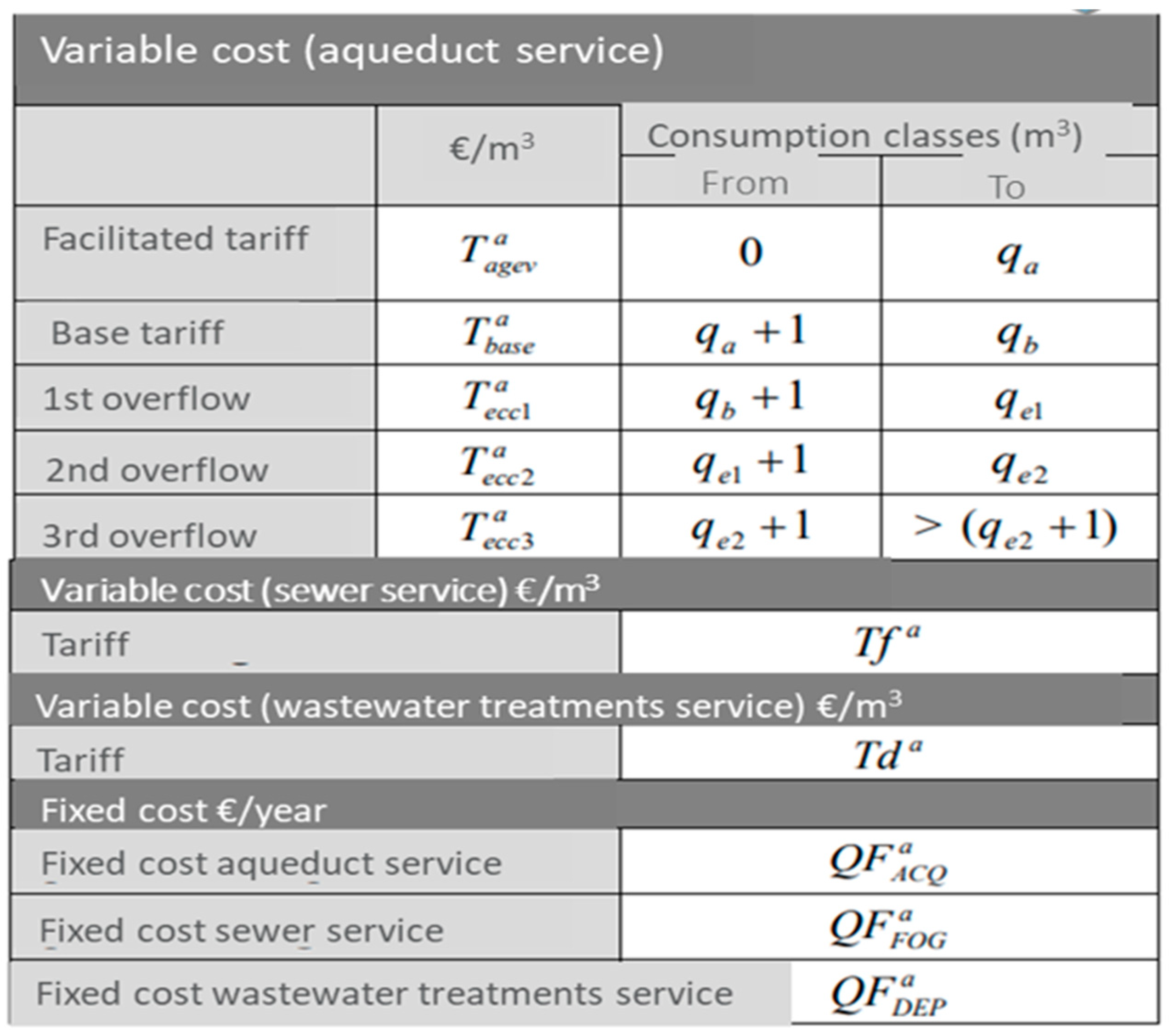
- refers to the revenues generated by the application of the pre-existing tariffs to the original articulation of the scale variables (consumption ranges);
- refers to the revenues generated by the application of the new tariffs to the new articulation of the scale variables (consumption ranges), determined starting from the re-modulation of the pre-existing variables based on the criteria established by the ARERA.
3.2. Model Definition
4. Results and Discussion
4.1. Results
- resident domestic use;
- non-resident domestic use;
- non domestic use.
4.2. Discussion
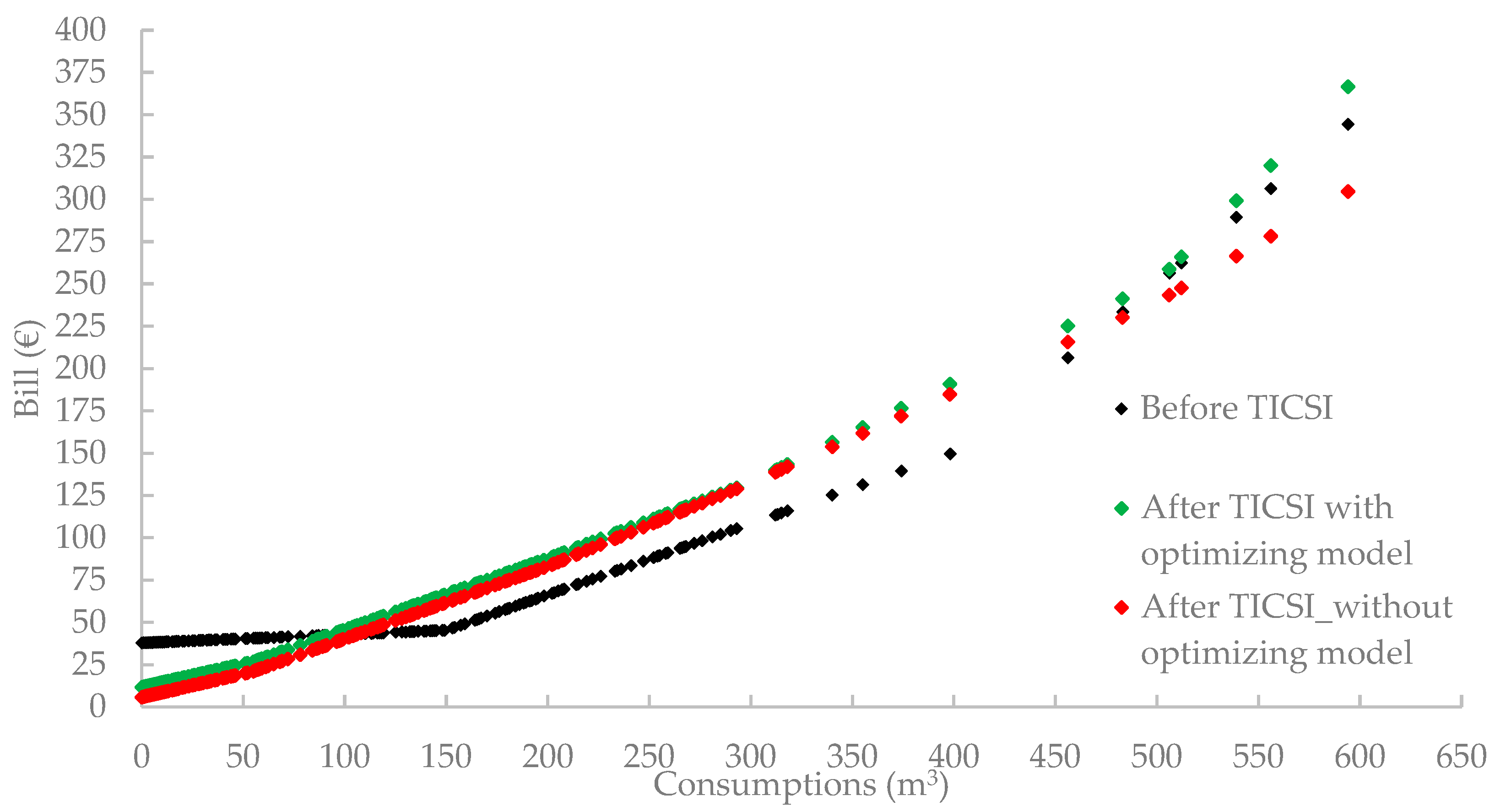
- The reduced tariff (Tred), the basic tariff (Tbas) and the first excess tariff (Texc,1) do not involve a significant difference in terms of payment to be paid as consumption increases compared to the scenario in which the TICSI is applied without resorting to the optimization model. In fact, in Figure 5 it can be seen that, for consumption up to about 300 m3, the green curve roughly coincides with the red one;
- The last two excess tariffs (Texc,2 and Texc,3) determine higher payments to be paid in proportion to consumption compared to the scenario in which the TICSI is applied without resorting to the proposed model;
- The Texc,3 tariff involves a significant increase in spending for less virtuous users, exceeding the costs relating to both the pre-TICSI scenario and the post-TICSI scenario in the absence of the model.
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- United Nations (UN). Transforming our World: The 2030 Agenda for Sustainable Development. Available online: https://sdgs.un.org/2030agenda (accessed on 23 December 2022).
- Vollmer, D.; Harrison, I.J. H2O ≠ CO2: Framing and responding to the global water crisis. Environ. Res. Lett. 2021, 16, 011005. [Google Scholar] [CrossRef]
- Mishra, B.K.; Kumar, P.; Saraswat, C.; Chakraborty, S.; Gautam, A. Water Security in a Changing Environment: Concept, Challenges and Solutions. Water 2021, 13, 490. [Google Scholar] [CrossRef]
- Salehi, M. Global water shortage and potable water safety; Today’s concern and tomorrow’s crisis. Environ. Int. 2022, 158, 106936. [Google Scholar] [CrossRef] [PubMed]
- Leão, A.S.; Sipert, S.A.; Medeiros, D.L.; Cohim, E.B. Water footprint of drinking water: The consumptive and degradative use. J. Clean. Prod. 2022, 355, 131731. [Google Scholar] [CrossRef]
- Wang, R.; Zimmerman, J. Hybrid analysis of blue water consumption and water scarcity implications at the global, national, and basin levels in an increasingly globalized world. Environ. Sci. Technol. 2016, 50, 5143–5153. [Google Scholar] [CrossRef]
- Koronkevich, N.I.; Barabanova, E.A.; Zaitseva, I.S. Assessment of Modern Water Consumption in the World and on Continents and Its Impact on the Annual River Runoff. Her. Russ. Acad. Sci. 2022, 92, 199–206. [Google Scholar] [CrossRef]
- European Environment Agency. Drought and Excessive Water Consumption in Europe. Available online: https://www.eea.europa.eu/it/pressroom/newsreleases/siccita-e-consumo-eccessivo-di-acqua-in-europa (accessed on 24 December 2022).
- Rinnovabili.it. Thirsty Italy, in Second Place in Europe for Water Consumption per Person. Available online: https://www.rinnovabili.it/ambiente/acqua/litalia-assetata-al-secondo-posto-in-europa-per-consumi-dacqua-a-testa/ (accessed on 24 December 2022).
- Istat. Istat Statistics on Water—Years 2019–2021. Available online: https://www.istat.it/it/files//2022/03/REPORTACQUA2022.pdf (accessed on 22 December 2022).
- Ben Zaied, Y.; Taleb, L.; Ben Lahouel, B.; Managi, S. Sustainable Water Demand Management and Incentive Tariff: Evidence from a Quantile-on-Quantile Approach. Environ. Model. Assess. 2022, 27, 967–980. [Google Scholar] [CrossRef]
- Dinar, A.; Subramanian, A. Policy implications from water pricing experiences in various countries. Water Policy 1998, 1, 239–250. [Google Scholar] [CrossRef]
- Nesticò, A.; De Mare, G.; Frusciante, B.; Dolores, L. Construction Costs Estimate for Civil Works. A Model for the Analysis During the Preliminary Stage of the Project. In LNCS; Gervasi, O., Murgante, B., Misra, S., Borruso, G., Torre, C.M., Rocha, A.M.A.C., Taniar, D., Apduhan, B.O., Stankova, E., Cuzzocrea, A., Eds.; Springer: Cham, Switzerland, 2017; Volume 10408, pp. 202–217. [Google Scholar] [CrossRef]
- Dolores, L.; Macchiaroli, M.; De Mare, G. Financial Impacts of the Energy Transition in Housing. Sustainability 2022, 14, 4876. [Google Scholar] [CrossRef]
- De Mare, G.; Manganelli, B.; Nesticò, A. The Economic Evaluation of Investments in the Energy Sector: A Model for the Optimization of the Scenario Analyses. In Computational Science and Its Applications—ICCSA 2013; Lecture Notes in Computer Science; Springer: Berlin, Heidelberg, 2013; Volume 7972. [Google Scholar] [CrossRef]
- Bashar, A.M.; Nozari, H.; Marofi, S.; Mohamadi, M.; Ahadiiman, A. Investigation of factors affecting rural drinking water consumption using intelligent hybrid models. Water Sci. Eng. 2022, in press. [CrossRef]
- Arbues, F.; Villanua, I. Potential for pricing policies in water resource management: Estimation of urban residential water demand in Zaragoza, Spain. Urban Stud. 2006, 43, 2421–2442. [Google Scholar] [CrossRef]
- Domene, E.; Saurí, D. Urbanisation and water consumption: Influencing factors in the metropolitan region of Barcelona. Urban Stud. 2006, 43, 1605–1623. [Google Scholar] [CrossRef]
- Inman, D.; Jeffrey, P. A review of residential water conservation tool performance and influences on implementation effectiveness. Urban Water J. 2006, 3, 127–143. [Google Scholar] [CrossRef] [Green Version]
- March, H.; Saurí, D. The suburbanization of water scarcity in the Barcelona metropolitan region: Sociodemographic and urban changes influencing domestic water consumption. Prof. Geog. 2010, 62, 32–45. [Google Scholar] [CrossRef]
- Olmstead, S.M.; Stavins, R.N. Comparing price and nonprice approaches to urban water conservation. Water Resour. Res. 2009, 45, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Stavenhagen, M.; Buurman, J.; Tortajada, C. Saving water in cities: Assessing policies for residential water demand management in four cities in Europe. Cities 2018, 79, 187–195. [Google Scholar] [CrossRef]
- OCDE. The Price of Water: Trends in OECD Countries; Organisation for Economic Co-operation and Development: Paris, France, 1999; pp. 1–174. [Google Scholar]
- Marzano, R.; Rouge, C.; Garrone, P.; Grilli, L.; Harou, J.J.; Pulido-Velazquez, M. Determinants of the price response to residential water tariffs: Meta-analysis and beyond. Environ. Model Softw. 2018, 101, 236–248. [Google Scholar] [CrossRef]
- Klassert, C.; Sigel, K.; Klauer, B.; Gawel, E. Increasing Block Tariffs in an Arid Developing Country: A Discrete/Continuous Choice Model of Residential Water Demand in Jordan. Water 2018, 10, 248. [Google Scholar] [CrossRef] [Green Version]
- Monteiro, H.; Roseta-Palma, C. Pricing for scarcity? An efficiency analysis of increasing block tariffs. Water Resour. Res. 2011, 47, 1–11. [Google Scholar] [CrossRef]
- Organization for Economic Co-operation and Development (OECD). Managing Water for All: An OECD Perspective on Pricing and Financing; OECD: Paris, France, 2009; Available online: https://www.oecd.org/env/42350563.pdf (accessed on 4 January 2023).
- Fuente, D. The design and evaluation of water tariffs: A systematic review. Utilities Policy 2019, 61, 100975. [Google Scholar] [CrossRef]
- De Mare, G.; Manganelli, B.; Nesticò, A. Dynamic Analysis of the Property Market in the City of Avellino (Italy). In Computational Science and Its Applications—ICCSA 2013; Lecture Notes in Computer Science; Springer: Berlin, Heidelberg, 2013; Volume 7973. [Google Scholar] [CrossRef]
- De Mare, G.; Nesticò, A.; Macchiaroli, M.; Dolores, L. Market Prices and Institutional Values. In LNCS; Gervasi, O., Murgante, B., Misra, S., Garau, C., Blečić, I., Taniar, D., Apduhan, B.O., Rocha, A.M.A.C., Tarantino, E., Torre, C.M., Eds.; Springer: Cham, Switzerland, 2017; Volume 10409, pp. 430–440. [Google Scholar] [CrossRef]
- Nieswiadomy, M.L. Estimating urban residential water demand: Effects of price structure, conservation, and education. Water Resour. Res. 1992, 28, 609–615. [Google Scholar] [CrossRef]
- Olmstead, S.M.; Hanemann, M.; Stavins, R.N. Water Demand under Alternative Price Structures. J. Environ. Econ. Manag. 2007, 54, 181–198. [Google Scholar] [CrossRef] [Green Version]
- Ratnasiri, S.; Wilson, C.; Athukorala, W.; Garcia-Valiñas, M.A.; Torgler, B.; Gifford, R. Effectiveness of two pricing structures on urban water use and conservation: A quasi-experimental investigation. Environ. Econ. Policy Stud. 2018, 20, 547–560. [Google Scholar] [CrossRef]
- Sahin, O.; Bertone, E.; Beal, C. A systems approach for assessing water conservation potential through demand-based water tariffs. J. Clean. Prod. 2017, 148, 773–784. [Google Scholar] [CrossRef] [Green Version]
- García-Valiñas, M.Á.; Suárez-Fernández, S. Are Economic Tools Useful to Manage Residential Water Demand? A Review of Old Issues and Emerging Topics. Water 2022, 14, 2536. [Google Scholar] [CrossRef]
- The European Parliament and the Council of The European Union. Directive 2000/60/EC of the European Parliament and of the Council of 23 October 2000 Establishing a Framework for Community Action in the Field of Water Policy. Available online: https://eur-lex.europa.eu/resource.html?uri=cellar:5c835afb-2ec6-4577-bdf8-756d3d694eeb.0004.02/DOC_1&format=PDF (accessed on 5 January 2023).
- Commission of the European Communities. COM(2000)477—Communication from the Commission to the Council, the European Parliament and the Economic and Social Committee: Pricing Policies for Enhancing the Sustainability of Water Resources. Brussels. 2000. Available online: https://eur-lex.europa.eu/LexUriServ/LexUriServ.do?uri=COM:2000:0477:FIN:EN:PDF (accessed on 5 January 2023).
- Commission of the European Communities. COM(2014)177—Communication from the Commission: On the European Citizens’ Initiative “Water and Sanitation are a Human right! Water is a Public good, not a Commodity!”. Brussels. 2014. Available online: https://ec.europa.eu/transparency/documents-register/detail?ref=COM(2014)177&lang=it (accessed on 5 January 2023).
- Regulatory Authority for Energy, Networks and Environment (ARERA). Deliberation 665/2017/R/idr of 28 September 2017, Attachment A—Integrated Text on Water Services Tariffs (TICSI). Available online: https://www.arera.it/allegati/docs/17/665-17all.pdf (accessed on 5 January 2023).
- Regulatory Authority for Energy, Networks and Environment (ARERA). Deliberation 636/2018/R/IDR5 of 5 December 2018—Initiation of the Procedure for Monitoring the Application of the Tariff Structure Criteria Contained in the Integrated Text on Water Services Tariffs (TICSI). Available online: https://www.arera.it/allegati/docs/18/636-18.pdf (accessed on 5 January 2023).
- Levenberg, K. A method for the solution of certain non-linear problems in least squares. Q. Appl. Math. 1944, 2, 164–168. [Google Scholar] [CrossRef] [Green Version]
- Abdi, H. The method of least squares. Encycl. Meas. Stat. 2007, 1, 530–532. [Google Scholar]
- Jiang, B.N. On the least-squares method. Comput. Methods Appl. Mech. Eng. 1998, 152, 239–257. [Google Scholar] [CrossRef]
- Suárez-Varela, M.; Martinez-Espineira, R.; González-Gómez, F. An analysis of the price escalation of non-linear water tariffs for domestic uses in Spain. Util. Policy 2015, 34, 82–93. [Google Scholar] [CrossRef] [Green Version]
- Wichelns, D. Enhancing the performance of water prices and tariff structures in achieving socially desirable outcomes. Int. J. Water Resour. Dev. 2013, 29, 310–326. [Google Scholar] [CrossRef]
- Hoque, S.F.; Wichelns, D. State-of-the-art review: Designing urban water tariffs to recover costs and promote wise use. Int. J. Water Resour. Dev. 2013, 29, 472–491. [Google Scholar] [CrossRef]
- Renzetti, S. Evaluating the welfare effects of reforming municipal water prices. J. Environ. Econ. Manag. 1992, 22, 147–163. [Google Scholar] [CrossRef]
- García-Valiñas, M.Á. Efficiency and equity in natural resources pricing: A proposal for urban water distribution service. Environ. Resour. Econ. 2005, 32, 183–204. [Google Scholar] [CrossRef]
- Diakité, D.; Semenov, A.; Thomas, A. A proposal for social pricing of water supply in Côte d’Ivoire. J. Dev. Econ. 2009, 88, 258–268. [Google Scholar] [CrossRef] [Green Version]
- García-Valiñas, M.A.; Martínez-Espiñeira, R.; González-Gómez, F. Affordability of residential water tariffs: Alternative measurement and explanatory factors in southern Spain. J. Environ. Econ. Manag. 2010, 91, 2696–2706. [Google Scholar] [CrossRef]
- Rinaudo, J.D.; Neverre, N.; Montginoul, M. Simulating the impact of pricing policies on residential water demand: A southern France case study. Water Resour. Manag. 2012, 26, 2057–2068. [Google Scholar] [CrossRef] [Green Version]
- Reynaud, A. Assessing the impact of full cost recovery of water services on European households. Water Resour. Econ. 2016, 14, 65–78. [Google Scholar] [CrossRef]
- Garcia, S.; Reynaud, A. Estimating the benefits of efficient water pricing in France. Resour. Energy Econ. 2004, 26, 1–25. [Google Scholar] [CrossRef]
- Hoffman, J.J.; Du Plessis, J.A. A model to assess water tariffs as part of water demand management. Water Sa 2013, 39, 423–428. [Google Scholar] [CrossRef] [Green Version]
- Ahmad, S.; Prashar, D. Evaluating municipal water conservation policies using a dynamic simulation model. Water Resour. Manag. 2010, 24, 3371–3395. [Google Scholar] [CrossRef]
- Rosenberg, D.E. Residential water demand under alternative rate structures: Simulation approach. J. Water Resour. Plan. Manag. 2010, 136, 395–402. [Google Scholar] [CrossRef] [Green Version]
- Yates, D.N.; Lavin, F.V.; Purkey, D.P.; Guerrero, S.; Hanemann, M.; Sieber, J. Using economic and other performance measures to evaluate a municipal drought plan. Water Policy 2013, 15, 648–668. [Google Scholar] [CrossRef] [Green Version]
- Nauges, C.; Whittington, D. Evaluating the performance of alternative municipal water tariff designs: Quantifying the tradeoffs between equity, economic efficiency, and cost recovery. World Dev. 2017, 91, 125–143. [Google Scholar] [CrossRef] [Green Version]
- Wolak, F.A. Designing nonlinear price schedules for urban water utilities to balance revenue and conservation goals. Natl. Bur. Econ. Res. 2016, 22503, 1–41. [Google Scholar] [CrossRef]
- Chicoine, D.L.; Deller, S.C.; Ramamurthy, G. Water demand estimation under block rate pricing: A simultaneous equation approach. Water Resour. Res. 1986, 22, 859–863. [Google Scholar] [CrossRef]
- Nieswiadomy, M.L.; Molina, D.J. Urban water demand estimates under increasing block rates. Growth Chang. 1988, 19, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Saleth, R.M.; Dinar, A. Satisfying Urban Thirst: Water Supply Augmentation and Pricing Policy in Hyderabad City, India; World Bank Publications: Washington, DC, USA, 1997; Volume 395. [Google Scholar]
- Höglund, L. Household demand for water in Sweden with implications of a potential tax on water use. Water Resour. Res. 1999, 35, 3853–3863. [Google Scholar] [CrossRef]
- Pint, E.M. Household responses to increased water rates during the California drought. Land Econ. 1999, 75, 246–266. [Google Scholar] [CrossRef]
- Hansen, L.G. Water and energy price impacts on residential water demand in Copenhagen. Land Econ. 1996, 47, 66–79. [Google Scholar] [CrossRef]
- Hewitt, J.A.; Hanemann, W.M. A discrete/continuous choice approach to residential water demand under block rate pricing. Land Econ. 1995, 71, 173–192. [Google Scholar] [CrossRef]
- Regulatory Authority for Energy, Networks and Environment (ARERA). Deliberation 580/2019/R/IDR of 27 December 2019, Attachment A—Water Tariff Method 2020–2023—MTI-3. Available online: https://www.arera.it/allegati/docs/19/mti3_ti.pdf (accessed on 21 February 2023).
- Legendre, A.M. Nouvelles Méthodes Pour la Détermination des Orbites des Comètes; Histoire de l’Académie Royale des Sciences: Paris, France, 1805. [Google Scholar]
- Stigler, S.M. Gauss and the invention of least squares. Ann Statist. 1981, 9, 465–474. [Google Scholar] [CrossRef]
- Abadie, J.; Guigou, J. Numerical Experiments with the GRG Method; North-Holland Publishing Company: Amsterdam, Holland, 1970. [Google Scholar]
- Lasdon, L.S. Optimization Theory for Large Systems; Courier Corporation: North Chelmsford, MA, USA, 2002; pp. 1–560. [Google Scholar]
- Gould, N.I.; Orban, D.; Toint, P.L. CUTEst: A constrained and unconstrained testing environment with safe threads for mathematical optimization. Comput. Optim. Appl. 2015, 60, 545–557. [Google Scholar] [CrossRef]
- Pontryagin, L.S. Mathematical Theory of Optimal Processes; CRC Press: Boca Raton, FL, USA, 1987. [Google Scholar]
- Macchiaroli, M.; Dolores, L.; Nicodemo, L.; De Mare, G. Energy Efficiency in the Management of the Integrated Water Service. A Case Study on the White Certificates Incentive System. In LNCS; Gervasi, O., Murgante, B., Misra, S., Garau, C., Blečić, I., Taniar, D., Apduhan, B.O., Rocha, A.M.A.C., Tarantino, E., Torre, C.M., Eds.; Springer: Cham, Switzerland, 2021; Volume 12956, pp. 202–217. [Google Scholar] [CrossRef]
- Dolores, L.; Macchiaroli, M.; De Mare, G. Financial Targets for the Sponsee and the Sponsor in the Restoration/Recovery of the Historical and Architectural Heritage. In SIST; Bevilacqua, C., Calabrò, F., Della Spina, L., Eds.; Springer: Cham, Switzerland, 2020; Volume 177, pp. 155–165. [Google Scholar] [CrossRef]


| Tariff | Consumption Ranges (m3/Years) | QV (EUR/m3) | QF (EUR/Users) | |
|---|---|---|---|---|
| Min | Max | |||
| Tred | 0 | 150 | 0.0500 | 38.000 |
| Tbas | 151 | 400 | 0.4200 | |
| Texc,1 | >400 | 1.0000 | ||
| Annual revenues (EUR) | 5417 | 9766 | ||
| Tariff | Consumption Ranges (m3/Years) | QV (EUR/m3) | QF (EUR/Users) | |
|---|---|---|---|---|
| Min | Max | |||
| Tred | 0 | 55 | 0.2720 | 11.820 |
| Tbas | 56 | 200 | 0.4200 | |
| Texc,1 | 201 | 300 | 0.4510 | |
| Texc,2 | 301 | 500 | 0.5931 | |
| Texc,3 | >500 | 1.2258 | ||
| Annual revenues (EUR) | 12,147 | 3038 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Macchiaroli, M.; Dolores, L.; De Mare, G. Design the Water Tariff Structure: Application and Assessment of a Model to Balance Sustainability, Cost Recovery and Wise Use. Water 2023, 15, 1309. https://doi.org/10.3390/w15071309
Macchiaroli M, Dolores L, De Mare G. Design the Water Tariff Structure: Application and Assessment of a Model to Balance Sustainability, Cost Recovery and Wise Use. Water. 2023; 15(7):1309. https://doi.org/10.3390/w15071309
Chicago/Turabian StyleMacchiaroli, Maria, Luigi Dolores, and Gianluigi De Mare. 2023. "Design the Water Tariff Structure: Application and Assessment of a Model to Balance Sustainability, Cost Recovery and Wise Use" Water 15, no. 7: 1309. https://doi.org/10.3390/w15071309






