2D Microfluidic Devices for Pore-Scale Phenomena Investigation: A Review
Abstract
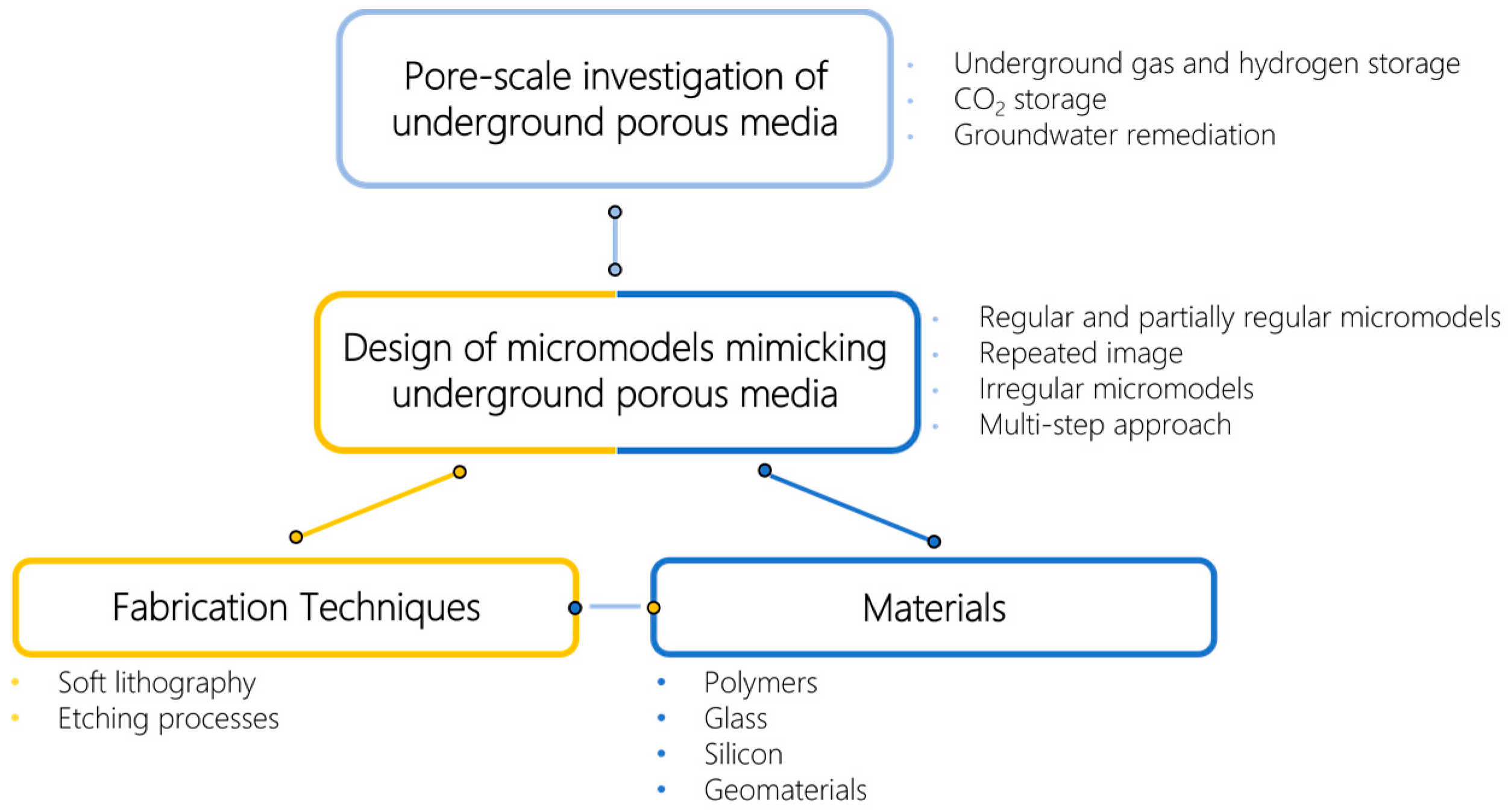
:1. Introduction
2. Design of Micromodels Mimicking Underground Porous Media
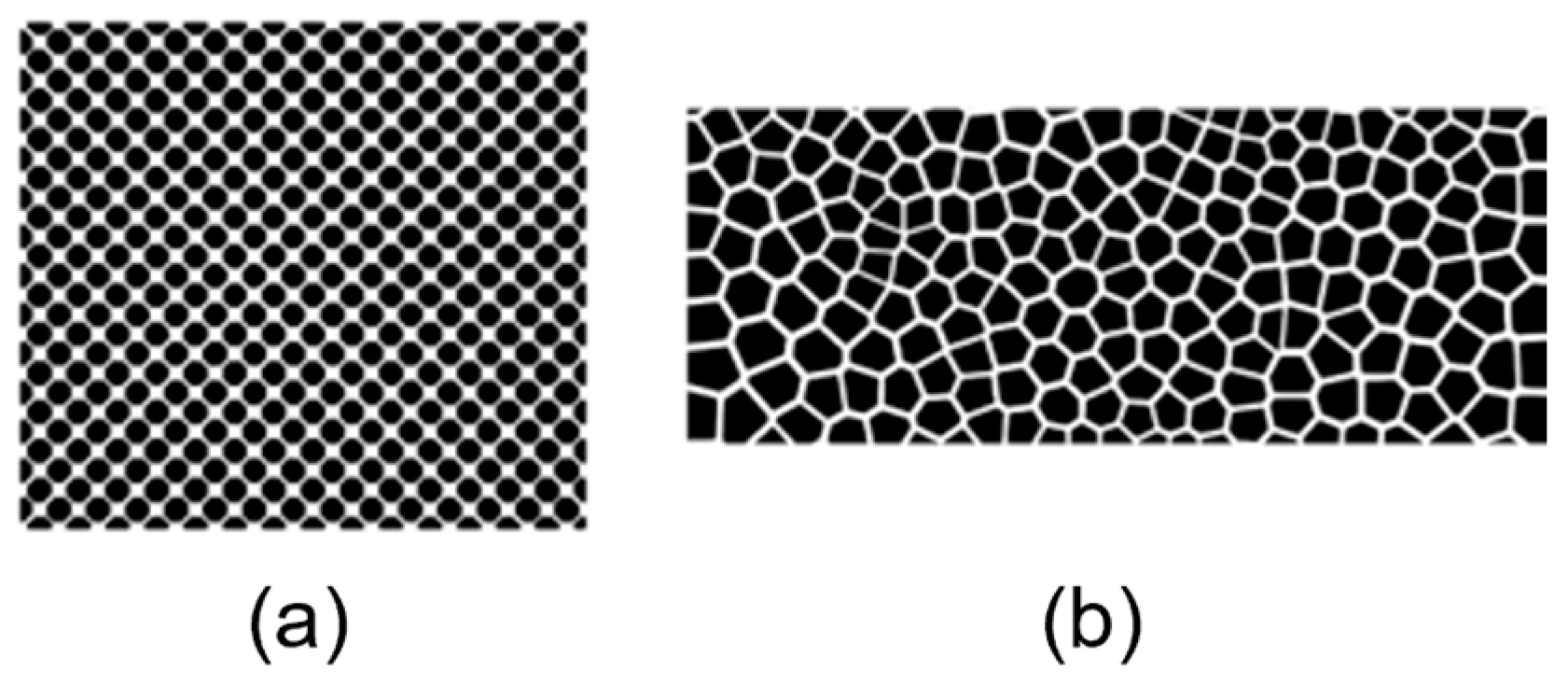
2.1. Regular and Partially Regular Micromodels
2.2. Repeated Image
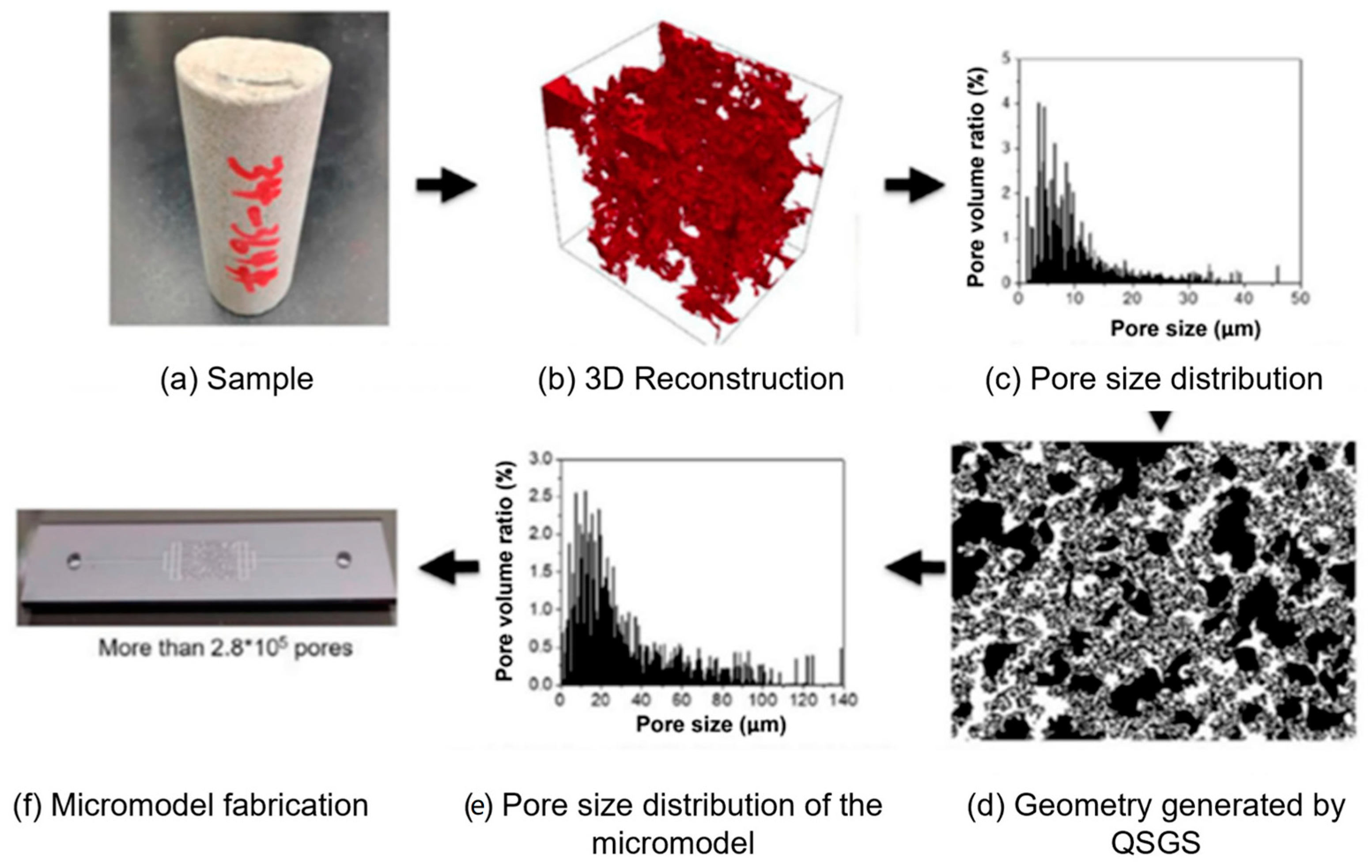
2.3. Irregular Micromodels
2.4. Multi-Step Approach
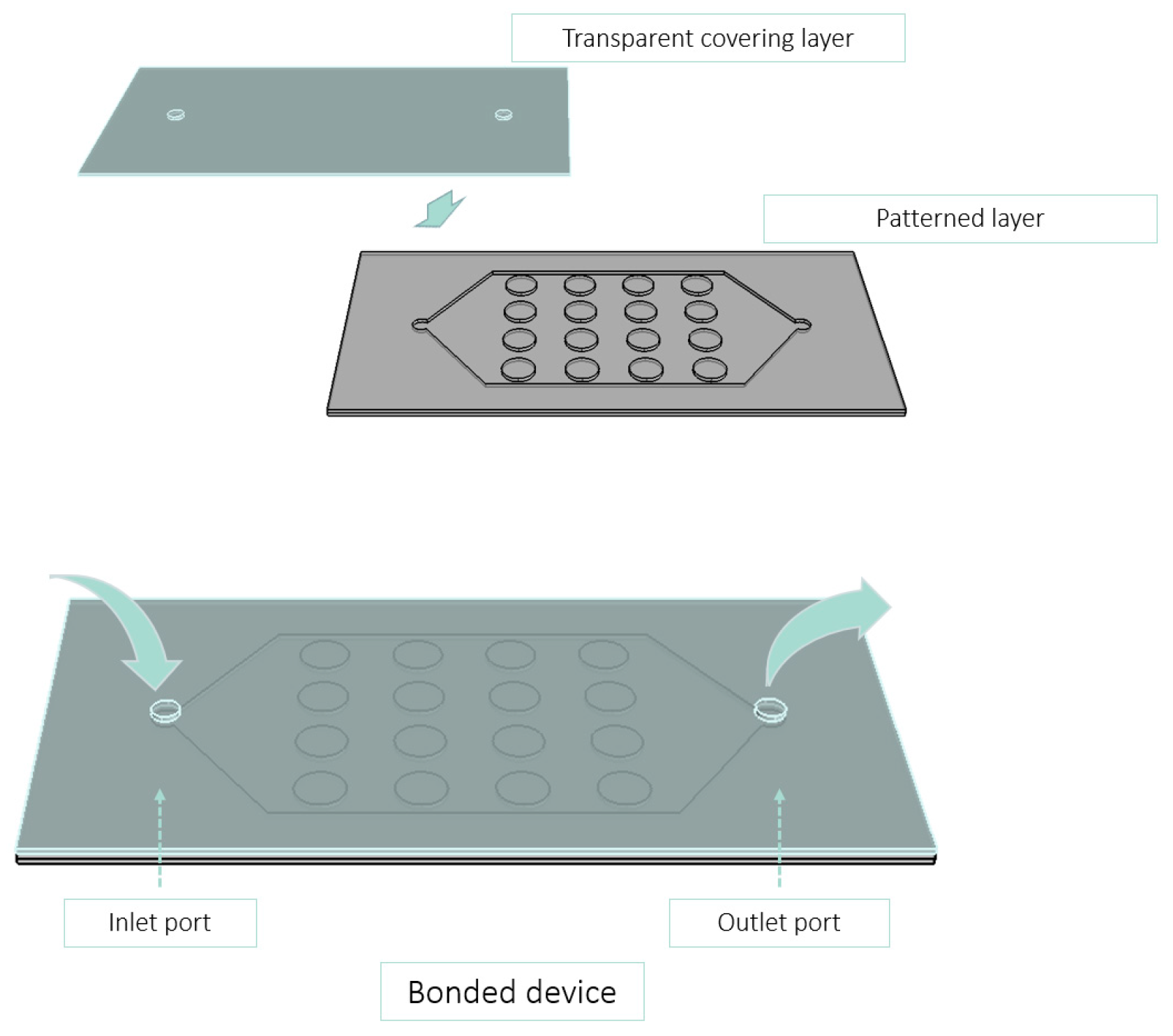
2.5. Inlet and Outlet
3. Micromodel Fabrication Techniques
3.1. Soft Lithography
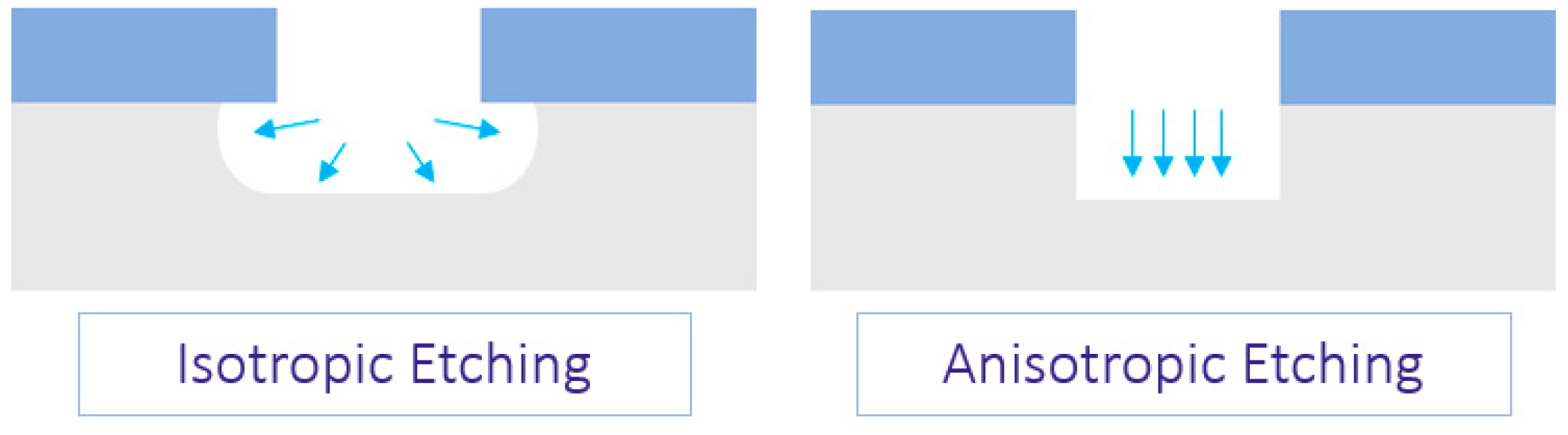
3.2. Etching
4. Micromodel Materials
4.1. Thermo- and Photocurable Polymers: PDMS and Thiolene-Based Resin
4.1.1. PDMS
4.1.2. Thiolene-Based Resin
4.2. Thermoplastic Polymers: PMMA and COC
4.2.1. PMMA
4.2.2. COC
4.3. Glass
4.4. Silicon
4.5. Geomaterials
5. Applications
5.1. Polymers
5.2. Glass
5.3. Silicon
| Material | Fabrication Techniques | Reference | Pattern Geometry | Pattern Dimensions | Channel Depth | Smallest Channel Width |
|---|---|---|---|---|---|---|
| PDMS | Soft- Lithography | Xu et al. [43] | Voronoi diagrams; Eight periodic networks | - | 14.6 μm | 4 µm |
| Casting on etched silicon | Kunz et al. [30] | Random network of rounded pillars | L = 25 mm, W = 1 mm | - | 160 μm | |
| Soft Lithography | Park et al. [78] | Uniform network | L = 49 mm, W = 30 mm | 50 µm | 120 µm | |
| Thiolene resin | Casting on PDMS mold | Kenzhekhanov S. and Yin X. [47] | Voronoi diagram | L = 5 mm, W = 15 mm | 10 µm | 8 μm |
| PMMA | LIGA process | Tsakiroglou, and Avraam [59] | Uniform squared network | L = 20 mm, W = 15 mm | 17.7 μm | 10 μm |
| Laser ablation | Sell et al. [81] | Serpentine | - | 50 μm | 100 μm | |
| COC | Lithography | Hsu et al. [82] | Uniform network and fracture | L = 30 mm, W = 30 mm | 100 µm | 200 µm |
| Glass | Wet etching | Amarasinghe et al. [74] | Random network | L = 51.2 mm, W = 7 mm | 40 µm | - |
| Wet etching | Van Rooijen et al. [88] | Irregular network | - | 20 µm | 50 µm | |
| Silicon | Reactive Ion Etching | Gunda et al. [17] | Delaunay triangulation | L = 35 mm, W = 5 mm | 41 µm | 25 µm |
| Wet etching | Keller et al. [67] | Real thin section image | L = 5.09 mm W = 5.09 mm | 15 µm | 3 µm | |
| Deep Reactive Ion Etching | Lysyy et al. [72] | Real thin section image | L = 28 mm, W = 22 mm | 30 µm | 10 µm | |
| Geomaterial | Laser etching | Gerami et al. [68] | Coal cleat structure | - | 112 µm–381 µm | 10 µm |
| Material | Reference | Application | Pressure | Temperature |
|---|---|---|---|---|
| PDMS | Xu et al. [43] | Investigation of the effects of pore geometry and interfacial tension on two-phase flow | 0.28–1.38 bar | 25 °C |
| Kunz et al. [30] | Comparison of numerical simulations with micromodel experiments | 1 bar | - | |
| Park et al. [78] | Pore-scale mixing and reactions between an iron sulfate solution and simulated groundwaters | Atmospheric pressure | 22 °C | |
| Thiolene resin | Kenzhekhanov S. and Yin X. [47] | Water and surfactant flooding displacement efficiencies in water-wet and oil-wet micromodels | 1 bar | 22 °C |
| PMMA | Sell et al. [81] | Measurement of the diffusion coefficient of carbon dioxide in water and brine | 5−50 bar | 26 °C |
| COC | Hsu et al. [82] | Drainage/Imbibition test in a fractured porous medium | 85 bar | 45 °C |
| Glass | Amarasinghe et al. [74] | Pore-scale CO2 convective mixing analysis in water and oil/water systems | 100 bar | 50 °C |
| Van Rooijen et al. [88] | Hydrogen-brine contact angle measurement for underground hydrogen storage | 10 bar | Ambient Temperature | |
| Silicon | Keller et al. [67] | Observation NAPL flow in water and air at the pore scale | Atmospheric pressure | Ambient Temperature |
| Lysyy et al. [72] | Description of pore-scale multiphase hydrogen flow in an aquifer storage scenario | 5 bar | 20 °C | |
| Geomaterial | Gerami et al. [68] | Contact angle measurements in coal fractures | up to 64 bar | 20 °C |
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| COC | Cyclic Olefin Copolymer |
| DRIE | Deep Reactive Ion Etching |
| EOR | Enhanced Oil Recovery |
| Micro-CT | Micro-Computed Tomography |
| NAPL | Non-Aqueous Phase Liquid |
| PDMS | Poly-Di-Methyl-Siloxane |
| PMMA | Poly-Methyl-Meth-Acrylate |
| RIE | Reactive Ion Etching |
| QSGS | Quartet Structure Generation Set |
References
- Konangi, S.; Palakurthi, N.K.; Karadimitriou, N.K.; Comer, K.; Ghia, U. Comparison of pore-scale capillary pressure to macroscale capillary pressure using direct numerical simulations of drainage under dynamic and quasi-static conditions. Adv. Water Resour. 2021, 147, 103792. [Google Scholar] [CrossRef]
- Massimiani, A.; Panini, F.; Marasso, S.L.; Vasile, N.; Quaglio, M.; Coti, C.; Barbieri, D.; Verga, F.; Pirri, C.F.; Viberti, D. Design, Fabrication, and Experimental Validation of Microfluidic Devices for the Investigation of Pore-Scale Phenomena in Underground Gas Storage Systems. Micromachines 2023, 14, 308. [Google Scholar] [CrossRef] [PubMed]
- Anderson, T. Applications of additive manufacturing to rock analogue fabrication. In Proceedings of the SPE Annual Technical Conference and Exhibition, Dubai, United Arab Emirates, 26–28 September 2016. [Google Scholar]
- Kong, L.; Ostadhassan, M.; Zamiran, S.; Liu, B.; Li, C.; Marino, G.G. Geomechanical upscaling methods: Comparison and verification via 3D printing. Energies 2019, 12, 382. [Google Scholar] [CrossRef] [Green Version]
- Suzuki, A.; Watanabe, N.; Li, K.; Horne, R.N. Fracture network created by 3-D printer and its validation using CT images. Water Resour. Res. 2017, 53, 6330–6339. [Google Scholar] [CrossRef]
- Ahkami, M.; Roesgen, T.; Saar, M.O.; Kong, X.-Z. High-resolution temporo-ensemble PIV to resolve pore-scale flow in 3D-printed fractured Porous Media. Transp. Porous Media 2019, 129, 467–483. [Google Scholar] [CrossRef]
- Yiotis, A.; Karadimitriou, N.K.; Zarikos, I.; Steeb, H. Pore-scale effects during the transition from capillary- to viscosity-dominated flow dynamics within microfluidic porous-like domains. Sci. Rep. 2021, 11, 3891. [Google Scholar] [CrossRef]
- Soulaine, C.; Maes, J.; Roman, S. Computational microfluidics for geosciences. Front. Water 2021, 3, 643714. [Google Scholar] [CrossRef]
- Jahanbakhsh, A.; Wlodarczyk, K.L.; Hand, D.P.; Maier, R.R.J.; Maroto-Valer, M.M. Review of Microfluidic Devices and Imaging Techniques for Fluid Flow Study in Porous Geomaterials. Sensors 2020, 20, 4030. [Google Scholar] [CrossRef]
- Karadimitriou, N.K.; Hassanizadeh, S.M. A review of micromodels and their use in two-phase flow studies. Vadose Zone J. 2012, 11, vzj2011.0072. [Google Scholar] [CrossRef] [Green Version]
- Wu, M.; Xiao, F.; Johnson-Paben, R.M.; Retterer, S.T.; Yin, X.; Neeves, K.B. Single- and two-phase flow in microfluidic porous media analogs based on Voronoi tessellation. Lab Chip 2012, 12, 253–261. [Google Scholar] [CrossRef]
- Heidari, S.; Ahmadi, M.; Esmaeilzadeh, F.; Mowla, D. Oil recovery from fractured reservoirs using in situ and preformed particle gels in micromodel structures. J. Pet. Explor. Prod. Technol. 2019, 9, 2309–2317. [Google Scholar] [CrossRef] [Green Version]
- Buchgraber, M.; Clemens, T.; Castanier, L.M.; Kovscek, A.R. A microvisual study of the displacement of viscous oil by polymer solutions. SPE Reserv. Eval. Eng. 2011, 14, 269–280. [Google Scholar] [CrossRef]
- Chen, Y.; Li, Y.; Valocchi, A.J.; Christensen, K.T. Lattice Boltzmann simulations of liquid CO2 displacing water in a 2D heterogeneous micromodel at reservoir pressure conditions. J. Contam. Hydrol. 2018, 212, 14–27. [Google Scholar] [CrossRef] [PubMed]
- Karadimitriou, N.K. Two-Phase Flow Experimental Studies in Micro-Models. Ph.D. Thesis, Utrecht University, Utrecht, The Netherlands, 2013. [Google Scholar]
- Joekar-Niasar, V.; Hassanizadeh, S.M.; Dahle, H.K. Non-equilibrium effects in capillarity and interfacial area in two-phase flow: Dynamic pore-network modelling. J. Fluid Mech. 2010, 655, 38–71. [Google Scholar] [CrossRef]
- Gunda, N.S.K.; Bera, B.; Karadimitriou, N.K.; Mitra, S.K.; Hassanizadeh, S.M. Reservoir-on-a-Chip (ROC): A new paradigm in reservoir engineering. Lab Chip 2011, 11, 3785–3792. [Google Scholar] [CrossRef] [Green Version]
- Porter, M.L.; Jiménez-Martínez, J.; Martinez, R.; McCulloch, Q.; Carey, J.W.; Viswanathan, H.S. Geo-material microfluidics at reservoir conditions for subsurface energy resource applications. Lab Chip 2015, 15, 4044–4053. [Google Scholar] [CrossRef]
- Lindquist, W.B.; Lee, S.-M.; Coker, D.A.; Jones, K.W.; Spanne, P. Medial axis analysis of void structure in three-dimensional tomographic images of porous media. J. Geophys. Res. Solid Earth 1996, 101, 8297–8310. [Google Scholar] [CrossRef] [Green Version]
- Raeini, A.Q.; Bijeljic, B.; Blunt, M.J. Generalized network modeling: Network extraction as a coarse-scale discretization of the void space of porous media. Phys. Rev. E 2017, 96, 013312. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Viberti, D.; Peter, C.; Borello, E.S.; Panini, F. Pore structure characterization through path-finding and lattice Boltzmann simulation. Adv. Water Resour. 2020, 141, 103609. [Google Scholar] [CrossRef]
- Panini, F.; Salina Borello, E.; Peter, C.; Viberti, D. Application of a* Algorithm for Tortuosity and Effective Porosity Estimation of 2D Rock Images. In Advanced Problem in Mechanics II: Proceedings of the XLVIII International Summer School-Conference “Advanced Problems in Mechanics”, 2020, St. Petersburg, Russia; Springer International Publishing: Cham, The Netherlands, 2020; pp. 519–530. [Google Scholar]
- Wang, M.; Wang, J.; Pan, N.; Chen, S. Mesoscopic predictions of the effective thermal conductivity for microscale random porous media. Phys. Rev. E 2007, 75, 036702. [Google Scholar] [CrossRef] [Green Version]
- Borello, E.S.; Peter, C.; Panini, F.; Viberti, D. Application of A∗ algorithm for microstructure and transport properties characterization from 3D rock images. Energy 2022, 239, 122151. [Google Scholar] [CrossRef]
- Lei, W.; Liu, T.; Xie, C.; Yang, H.; Wu, T.; Wang, M. Enhanced oil recovery mechanism and recovery performance of micro-gel particle suspensions by microfluidic experiments. Energy Sci. Eng. 2020, 8, 986–998. [Google Scholar] [CrossRef] [Green Version]
- Gaol, C.L.; Wegner, J.; Ganzer, L. Real structure micromodels based on reservoir rocks for enhanced oil recovery (EOR) applications. Lab Chip 2020, 20, 2197–2208. [Google Scholar] [CrossRef] [PubMed]
- Anbari, A.; Chien, H.; Datta, S.S.; Deng, W.; Weitz, D.A.; Fan, J. Microfluidic model porous media: Fabrication and applications. Small 2018, 14, e1703575. [Google Scholar] [CrossRef] [PubMed]
- Schneider, M.H.; Tabeling, P. Lab-on-chip methodology in the energy industry: Wettability patterns and their impact on fluid displacement in oil reservoir models. Am. J. Appl. Sci. 2011, 8, 927–932. [Google Scholar] [CrossRef]
- Bartolo, D.; Degré, G.; Nghe, P.; Studer, V. Microfluidic stickers. Lab Chip 2007, 8, 274–279. [Google Scholar] [CrossRef]
- Kunz, P.; Zarikos, I.M.; Karadimitriou, N.K.; Huber, M.; Nieken, U.; Hassanizadeh, S.M. Study of multi-phase flow in porous media: Comparison of SPH simulations with micro-model experiments. Transp. Porous Media 2015, 114, 581–600. [Google Scholar] [CrossRef] [Green Version]
- Deshmukh, S.S.; Goswami, A. Recent developments in hot embossing—A review. Mater. Manuf. Process. 2021, 36, 501–543. [Google Scholar] [CrossRef]
- Schomburg, W.K.; Vollmer, J.; Bustgens, B.; Fahrenberg, J.; Hein, H.; Menz, W. Microfluidic components in LIGA technique. J. Micromech. Microeng. 1994, 4, 186–191. [Google Scholar] [CrossRef]
- Marasso, S.L.; Mombello, D.; Cocuzza, M.; Casalena, D.; Ferrante, I.; Nesca, A.; Poiklik, P.; Rekker, K.; Aaspollu, A.; Ferrero, S.; et al. A polymer Lab-on-a-Chip for genetic analysis using the arrayed primer extension on microarray chips. Biomed. Microdevices 2014, 16, 661–670. [Google Scholar] [CrossRef]
- Tsao, C.-W. Polymer microfluidics: Simple, Low-cost fabrication process bridging academic lab research to commercialized production. Micromachines 2016, 7, 225. [Google Scholar] [CrossRef] [Green Version]
- Quaglio, M.; Bianco, S.; Castagna, R.; Cocuzza, M.; Pirri, C. Elastomeric nanocomposite based on carbon nanotubes for Polymerase Chain Reaction device. Microelectron. Eng. 2011, 88, 1860–1863. [Google Scholar] [CrossRef]
- Vitale, A.; Quaglio, M.; Marasso, S.L.; Chiodoni, A.; Cocuzza, M.; Bongiovanni, R. Direct photolithography of perfluoropolyethers for solvent-resistant microfluidics. Langmuir 2013, 29, 15711–15718. [Google Scholar] [CrossRef] [PubMed]
- Vitale, A.; Quaglio, M.; Chiodoni, A.; Bejtka, K.; Cocuzza, M.; Pirri, C.F.; Bongiovanni, R. Oxygen-Inhibition Lithography for the Fabrication of Multipolymeric Structures. Adv. Mater. 2015, 27, 4560–4565. [Google Scholar] [CrossRef] [PubMed]
- Bertana, V.; Potrich, C.; Scordo, G.; Scaltrito, L.; Ferrero, S.; Lamberti, A.; Perrucci, F.; Pirri, C.F.; Pederzolli, C.; Cocuzza, M.; et al. 3D-printed microfluidics on thin poly(methyl methacrylate) substrates for genetic applications. J. Vac. Sci. Technol. B 2018, 36, 01A106. [Google Scholar] [CrossRef]
- Raj, M.K.; Chakraborty, S. PDMS microfluidics: A mini review. J. Appl. Polym. Sci. 2020, 137, 48958. [Google Scholar] [CrossRef]
- Xia, Y.; Whitesides, G.M. Soft lithography. Angew. Chem. Int. Ed. 1998, 37, 550–575. [Google Scholar] [CrossRef]
- McDonald, J.C.; Duffy, D.C.; Anderson, J.R.; Chiu, D.T.; Wu, H.; Schueller, O.J.A.; Whitesides, G.M. Fabrication of microfluidic systems in poly(dimethylsiloxane). Electrophor. Int. J. 2000, 21, 27–40. [Google Scholar] [CrossRef]
- Cottin, C.; Bodiguel, H.; Colin, A. Influence of wetting conditions on drainage in porous media: A microfluidic study. Phys. Rev. E 2011, 84, 026311. [Google Scholar] [CrossRef]
- Xu, W.; Ok, J.T.; Xiao, F.; Neeves, K.B.; Yin, X. Effect of pore geometry and interfacial tension on water-oil displacement efficiency in oil-wet microfluidic porous media analogs. Phys. Fluids 2014, 26, 093102. [Google Scholar] [CrossRef]
- Wägli, P.; Homsy, A.; de Rooij, N. Norland optical adhesive (NOA81) microchannels with adjustable wetting behavior and high chemical resistance against a range of mid-infrared-transparent organic solvents. Sens. Actuators B Chem. 2011, 156, 994–1001. [Google Scholar] [CrossRef]
- Li, R.; Lv, X.; Deng, Y. NOA 81 fabricated microfluidic chip for SH-SY5Y cell culture. In Proceedings of the 2015 IEEE International Conference on Mechatronics and Automation (ICMA), Beijing, China, 2–5 August 2015; pp. 994–998. [Google Scholar]
- Wägli, P.; Guélat, B.; Homsy, A.; De Rooij, N. Microfluidic devices made of UV-curable glue (NOA81) for fluorescence detection based applications. In Proceedings of the 14th International Conference on Miniaturized Systems for Chemistry and Life Sciences, Groningen, The Netherlands, 3–7 October 2010; pp. 3–7. [Google Scholar]
- Kenzhekhanov, S. Chemical EOR Process Visualization Using NOA81 Micromodels; Colorado School of Mines: Golden, CO, USA, 2016. [Google Scholar]
- Becker, H.; Locascio, L.E. Polymer microfluidic devices. Talanta 2002, 56, 267–287. [Google Scholar] [CrossRef] [PubMed]
- Heng, Q.; Tao, C.; Tie-Chuan, Z. Surface roughness analysis and improvement of micro-fluidic channel with excimer laser. Microfluid. Nanofluid. 2006, 2, 357–360. [Google Scholar] [CrossRef]
- Fiorini, G.S.; Chiu, D.T. Disposable microfluidic devices: Fabrication, function, and application. Biotechniques 2005, 38, 429–446. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hong, T.-F.; Ju, W.-J.; Wu, M.-C.; Tai, C.-H.; Tsai, C.-H.; Fu, L.-M. Rapid prototyping of PMMA microfluidic chips utilizing a CO2 laser. Microfluid. Nanofluid. 2010, 9, 1125–1133. [Google Scholar] [CrossRef]
- Gomez, D.; Goenaga, I.; Lizuain, I.; Ozaita, M. Femtosecond laser ablation for microfluidics. Opt. Eng. 2005, 44, 051105. [Google Scholar]
- Arnold, J.; Dasbach, U.; Ehrfeld, W.; Hesch, K.; Löwe, H. Combination of excimer laser micromachining and replication processes suited for large scale production. Appl. Surf. Sci. 1995, 86, 251–258. [Google Scholar] [CrossRef]
- Chatenever, A.; Calhoun, J.C.J. Visual examinations of fluid behavior in porous media—Part I. J. Pet. Technol. 1952, 4, 149–156. [Google Scholar] [CrossRef]
- Chuoke, R.L.; Van Meurs, P.; Van Der Poel, C. The instability of slow, immiscible, viscous liquid-liquid displacements in permeable media. Trans. AIME 1959, 216, 188–194. [Google Scholar] [CrossRef]
- Corapcioglu, M.Y.; Fedirchuk, P. Glass bead micromodel study of solute transport. J. Contam. Hydrol. 1999, 36, 209–230. [Google Scholar] [CrossRef]
- Iliescu, C.; Chen, B.; Miao, J. On the wet etching of Pyrex glass. Sens. Actuators A Phys. 2008, 143, 154–161. [Google Scholar] [CrossRef]
- Avraam, D.G.; Kolonis, G.B.; Roumeliotis, T.C.; Constantinides, G.; Payatakes, A.C. Steady-state two-phase flow through planar and nonplanar model porous media. Transp. Porous Media 1994, 16, 75–101. [Google Scholar] [CrossRef]
- Tsakiroglou, C.D.; Avraam, D. Fabrication of a new class of porous media models for visualization studies of multiphase flow processes. J. Mater. Sci. 2002, 37, 353–363. [Google Scholar] [CrossRef]
- Giordano, N.; Cheng, J. Microfluid mechanics: Progress and opportunities. J. Phys. Condens. Matter 2001, 13, R271–R295. [Google Scholar] [CrossRef] [Green Version]
- Knowles, K.; Van Helvoort, A. Anodic bonding. Int. Mater. Rev. 2006, 51, 273–311. [Google Scholar] [CrossRef]
- Park, D.S.; Cho, M.W.; Lee, H.; Cho, W.S. Micro-grooving of glass using micro-abrasive jet machining. J. Mater. Process. Technol. 2004, 146, 234–240. [Google Scholar] [CrossRef]
- Marasso, S.; Canavese, G.; Cocuzza, M.; Ferrarini, A.; Giuri, E.; Bartolo, S.L.; Mantero, G.; Perrone, D.; Quaglio, M.; Vallini, I. APEX protocol implementation on a lab-on-a-chip for SNPs detection. Microelectron. Eng. 2008, 85, 1326–1329. [Google Scholar] [CrossRef]
- Marasso, S.L.; Giuri, E.; Canavese, G.; Castagna, R.; Quaglio, M.; Ferrante, I.; Perrone, D.; Cocuzza, M. A multilevel Lab on chip platform for DNA analysis. Biomed. Microdevices 2011, 13, 19–27. [Google Scholar] [CrossRef]
- Henley, W.H.; Dennis, P.J.; Ramsey, J.M. Fabrication of microfluidic devices containing patterned microwell arrays. Anal. Chem. 2012, 84, 1776–1780. [Google Scholar] [CrossRef]
- Ku, X.; Zhang, Z.; Liu, X.; Chen, L.; Li, G. Low-cost rapid prototyping of glass microfluidic devices using a micromilling technique. Microfluid. Nanofluid. 2018, 22, 82. [Google Scholar] [CrossRef]
- Keller, A.A.; Blunt, M.J.; Roberts, A.P.V. Micromodel observation of the role of oil layers in three-phase flow. Transp. Porous Media 1997, 26, 277–297. [Google Scholar] [CrossRef]
- Gerami, A.; Armstrong, R.T.; Johnston, B.; Warkiani, M.E.; Mosavat, N.; Mostaghimi, P. Coal-on-a-chip: Visualizing flow in coal fractures. Energy Fuels 2017, 31, 10393–10403. [Google Scholar] [CrossRef]
- Browne, C.A.; Shih, A.; Datta, S.S. Pore-scale flow characterization of polymer solutions in microfluidic porous media. Small 2019, 16, e1903944. [Google Scholar] [CrossRef] [Green Version]
- Poonoosamy, J.; Soulaine, C.; Burmeister, A.; Deissmann, G.; Bosbach, D.; Roman, S. Microfluidic flow-through reactor and 3D Raman imaging for in situ assessment of mineral reactivity in porous and fractured porous media. Lab Chip 2020, 20, 2562–2571. [Google Scholar] [CrossRef]
- Quaglio, M.; Frascella, F.; Marasso, S.L.; Cocuzza, M.; Viberti, D.; Amendola, A.; Carminati, S.; Pirri, C.F. Design and Characterization of Microfluidic Devices for Water/Oil Separation. In Proceedings of the Offshore Mediterranean Conference and Exhibition, Ravenna, Italy, 27–29 March 2019. [Google Scholar]
- Lysyy, M.; Ersland, G.; Fernø, M. Pore-scale dynamics for underground porous media hydrogen storage. Adv. Water Resour. 2022, 163, 104167. [Google Scholar] [CrossRef]
- Tian, J.; Chen, Q.; Qin, C.; Kang, Y.; Jia, N.; Xi, Z. Pore-scale systematic study on the disconnection of bulk gas phase during water imbibition using visualized micromodels. Phys. Fluids 2022, 34, 062015. [Google Scholar] [CrossRef]
- Amarasinghe, W.; Farzaneh, S.; Fjelde, I.; Sohrabi, M.; Guo, Y. A Visual Investigation of CO2 Convective Mixing in Water and Oil at the Pore Scale Using a Micromodel Apparatus at Reservoir Conditions. Gases 2021, 1, 53–67. [Google Scholar] [CrossRef]
- Datta, S.S.; Battiato, I.; Fernø, M.A.; Juanes, R.; Parsa, S.; Prigiobbe, V.; Santanach-Carreras, E.; Song, W.; Biswal, S.L.; Sinton, D. Lab on a chip for a low-carbon future. Lab Chip 2023, 23, 1358–1375. [Google Scholar] [CrossRef] [PubMed]
- Molla, S.; Mostowfi, F. Novel Microfluidic Device for Dew Point Pressure Measurement of Retrograde Gas Condensates Using a Microfluidic Device. Energy Fuels 2021, 35, 11154–11161. [Google Scholar] [CrossRef]
- Wang, X.; Wang, X.; He, T.; Li, Y.; Hou, L. The mechanism of NAPL layer formation in a microfluidic device with dual-permeability: Experiments and numerical simulation. IOP Conf. Ser. Earth Environ. Sci. 2021, 675, 012175. [Google Scholar] [CrossRef]
- Park, S.; Anggraini, T.M.; Chung, J.; Kang, P.K.; Lee, S. Microfluidic pore model study of precipitates induced by the pore-scale mixing of an iron sulfate solution with simulated groundwater. Chemosphere 2021, 271, 129857. [Google Scholar] [CrossRef]
- Sun, Z.; Santamarina, J.C. Haines jumps: Pore scale mechanisms. Phys. Rev. E 2019, 100, 023115. [Google Scholar] [CrossRef] [Green Version]
- Zhao, J.; Yao, G.; Wen, D. Pore-scale simulation of water/oil displacement in a water-wet channel. Front. Chem. Sci. Eng. 2019, 13, 803–814. [Google Scholar] [CrossRef] [Green Version]
- Sell, A.; Fadaei, H.; Kim, M.; Sinton, D. Measurement of CO2 diffusivity for carbon sequestration: A microfluidic approach for reservoir-specific analysis. Environ. Sci. Technol. 2013, 47, 71–78. [Google Scholar] [CrossRef]
- Hsu, S.-Y.; Zhang, Z.-Y.; Tsao, C.-W. Thermoplastic micromodel investigation of two-phase flows in a fractured porous medium. Micromachines 2017, 8, 38. [Google Scholar] [CrossRef] [Green Version]
- Campbell, B.T.; Orr, F.M. Flow visualization for CO2/crude-oil displacements. Soc. Pet. Eng. J. 1985, 25, 665–678. [Google Scholar] [CrossRef]
- Lenormand, R.; Touboul, E.; Zarcone, C. Numerical models and experiments on immiscible displacements in porous media. J. Fluid Mech. 1988, 189, 165–187. [Google Scholar] [CrossRef]
- Kamari, E.; Rashtchian, D.; Shadizadeh, S.R. Micro-model experimental study of fracture geometrical effect on breakthrough time in miscible displacement process. Iran. J. Chem. Chem. Eng. 2011, 30, 1–7. [Google Scholar]
- Buchgraber, M.; Al-Dossary, M.; Ross, C.; Kovscek, A.R. Creation of a dual-porosity micromodel for pore-level visualization of multiphase flow. J. Pet. Sci. Eng. 2012, 86–87, 27–38. [Google Scholar] [CrossRef]
- Khademolhosseini, R.; Jafari, A.; Shabani, M.H. Micro scale investigation of enhanced oil recovery using nano/bio materials. Procedia Mater. Sci. 2015, 11, 171–175. [Google Scholar] [CrossRef] [Green Version]
- Van Rooijen, W. Dynamic Contact Angle Measurement Using Microfluidic Experiments Relevant for Hydrogen Subsurface Storage. Master’s Thesis, Delft University of Technology, Delft, The Netherlands, 2021. [Google Scholar]
- Födisch, H.; Hincapie, R.; Wegner, J.; Ganzer, L. Visualization of connate water replacement during flooding experiments using Glass-Silicon-Glass micromodels. In Proceedings of the 77th EAGE Conference and Exhibition 2015, Madrid, Spain, 1–4 June 2015; European Association of Geoscientists & Engineers: Utrecht, The Netherlands, 2015; pp. 1–3. [Google Scholar]
- Rock, A.; Hincapie, R.; Wegner, J.; Födisch, H.; Ganzer, L. Pore-scale Visualization of Polymer Viscoelasticity using Particle Tracing in Glass-silicon-glass Micromodels. In Proceedings of the 78th EAGE Conference and Exhibition 2016, Online, 30 May –2 June 2016; European Association of Geoscientists & Engineers: Utrecht, The Netherlands, 2016; pp. 1–3. [Google Scholar]
- Saxena, N.; Mavko, G.; Hofmann, R.; Srisutthiyakorn, N. Estimating permeability from thin sections without reconstruction: Digital rock study of 3D properties from 2D images. Comput. Geosci. 2017, 102, 79–99. [Google Scholar] [CrossRef] [Green Version]
- Hussaini, S.R.; Dvorkin, J. Permeability in the thin section. J. Pet. Sci. Eng. 2022, 218, 110959. [Google Scholar] [CrossRef]
- Ishutov, S. Establishing framework for 3D printing porous rock models in curable resins. Transp. Porous Media 2019, 129, 431–448. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Massimiani, A.; Panini, F.; Marasso, S.L.; Cocuzza, M.; Quaglio, M.; Pirri, C.F.; Verga, F.; Viberti, D. 2D Microfluidic Devices for Pore-Scale Phenomena Investigation: A Review. Water 2023, 15, 1222. https://doi.org/10.3390/w15061222
Massimiani A, Panini F, Marasso SL, Cocuzza M, Quaglio M, Pirri CF, Verga F, Viberti D. 2D Microfluidic Devices for Pore-Scale Phenomena Investigation: A Review. Water. 2023; 15(6):1222. https://doi.org/10.3390/w15061222
Chicago/Turabian StyleMassimiani, Alice, Filippo Panini, Simone Luigi Marasso, Matteo Cocuzza, Marzia Quaglio, Candido Fabrizio Pirri, Francesca Verga, and Dario Viberti. 2023. "2D Microfluidic Devices for Pore-Scale Phenomena Investigation: A Review" Water 15, no. 6: 1222. https://doi.org/10.3390/w15061222
APA StyleMassimiani, A., Panini, F., Marasso, S. L., Cocuzza, M., Quaglio, M., Pirri, C. F., Verga, F., & Viberti, D. (2023). 2D Microfluidic Devices for Pore-Scale Phenomena Investigation: A Review. Water, 15(6), 1222. https://doi.org/10.3390/w15061222







