Analysis of Large-Scale Groundwater-Driven Cooling Zones in Rivers Using Thermal Infrared Imagery and Radon Measurements
Abstract
1. Introduction
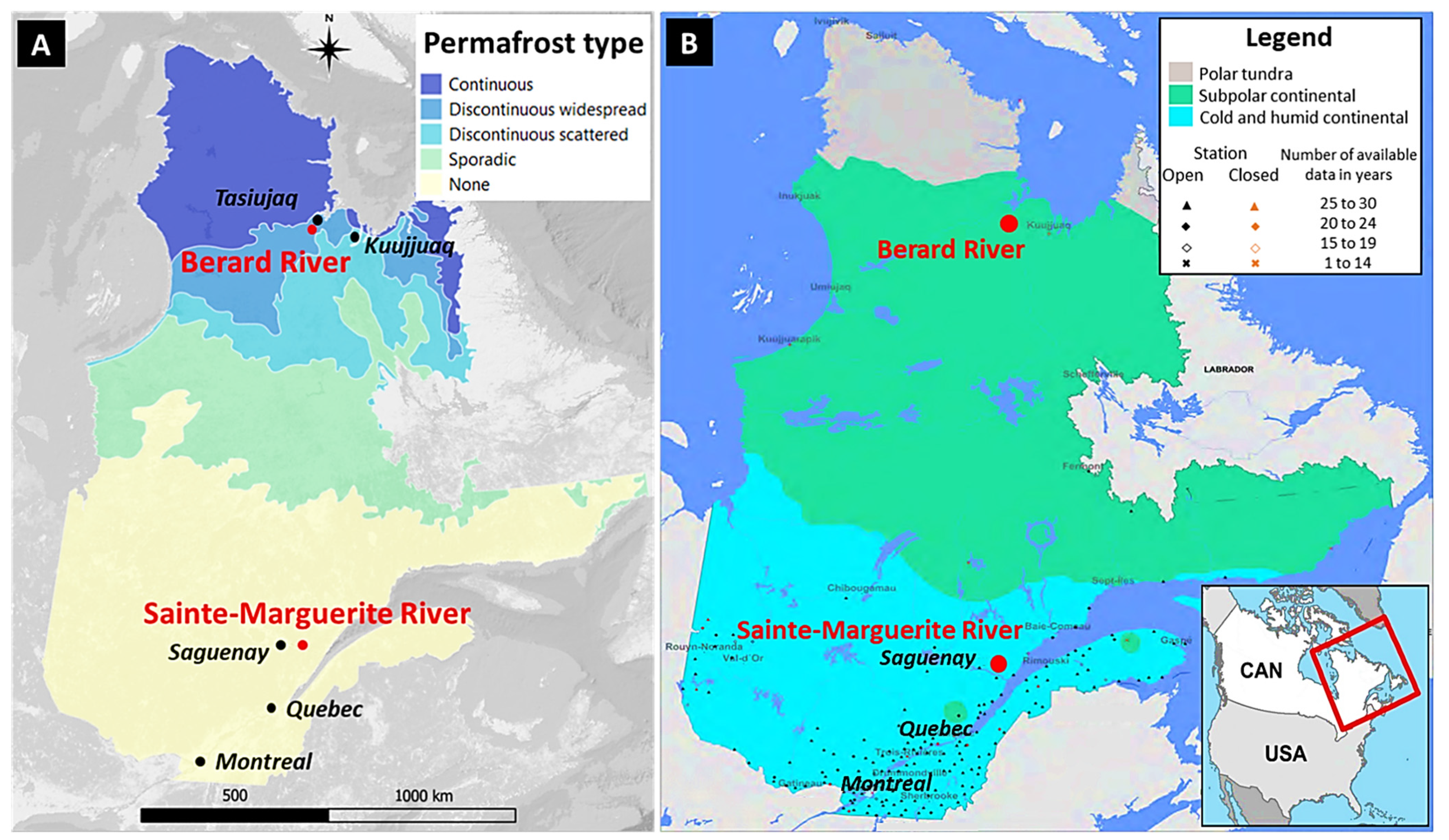
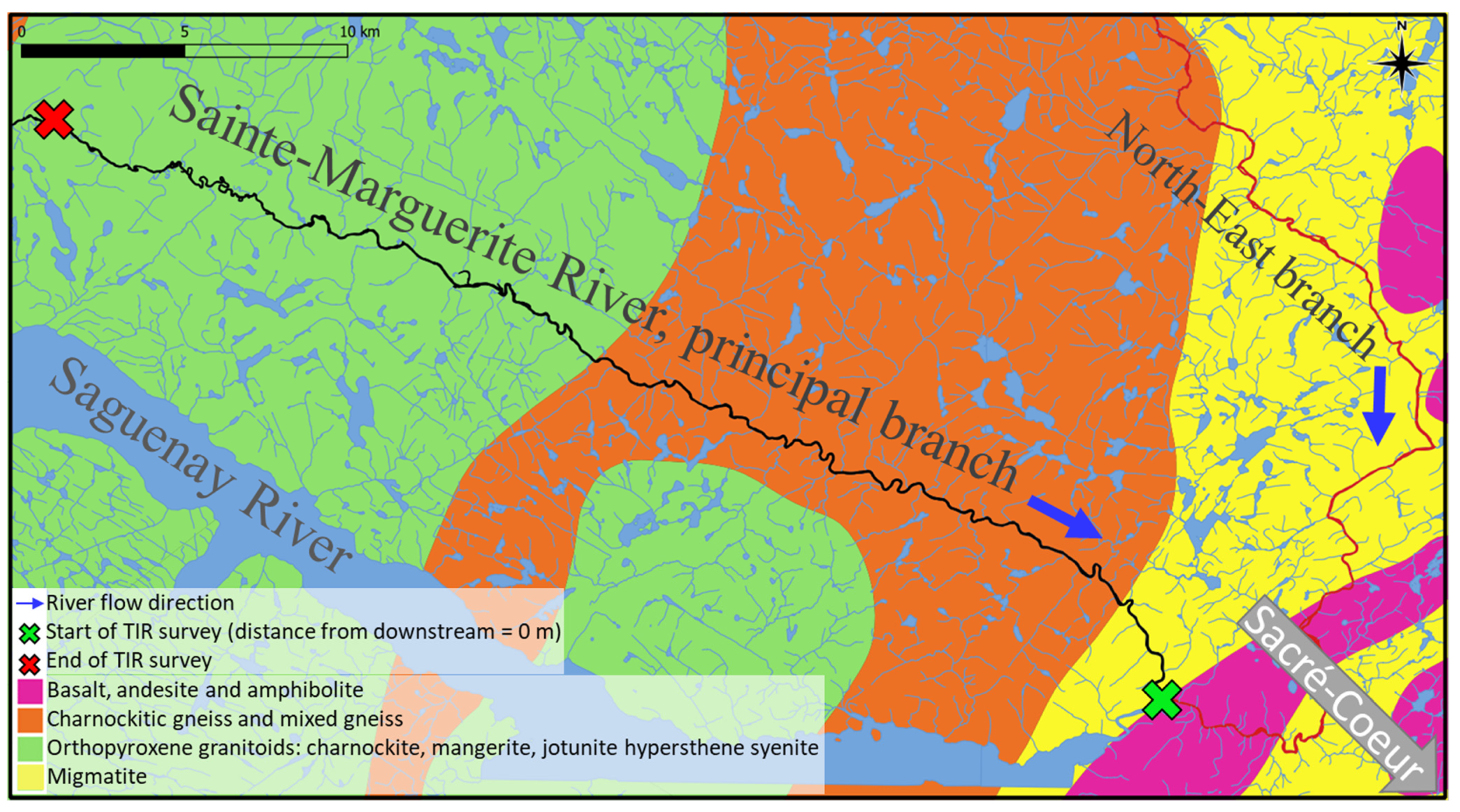
2. Study Area
3. Materials and Methods
3.1. Aerial TIR Imagery
3.2. Radon Measurements
4. Results
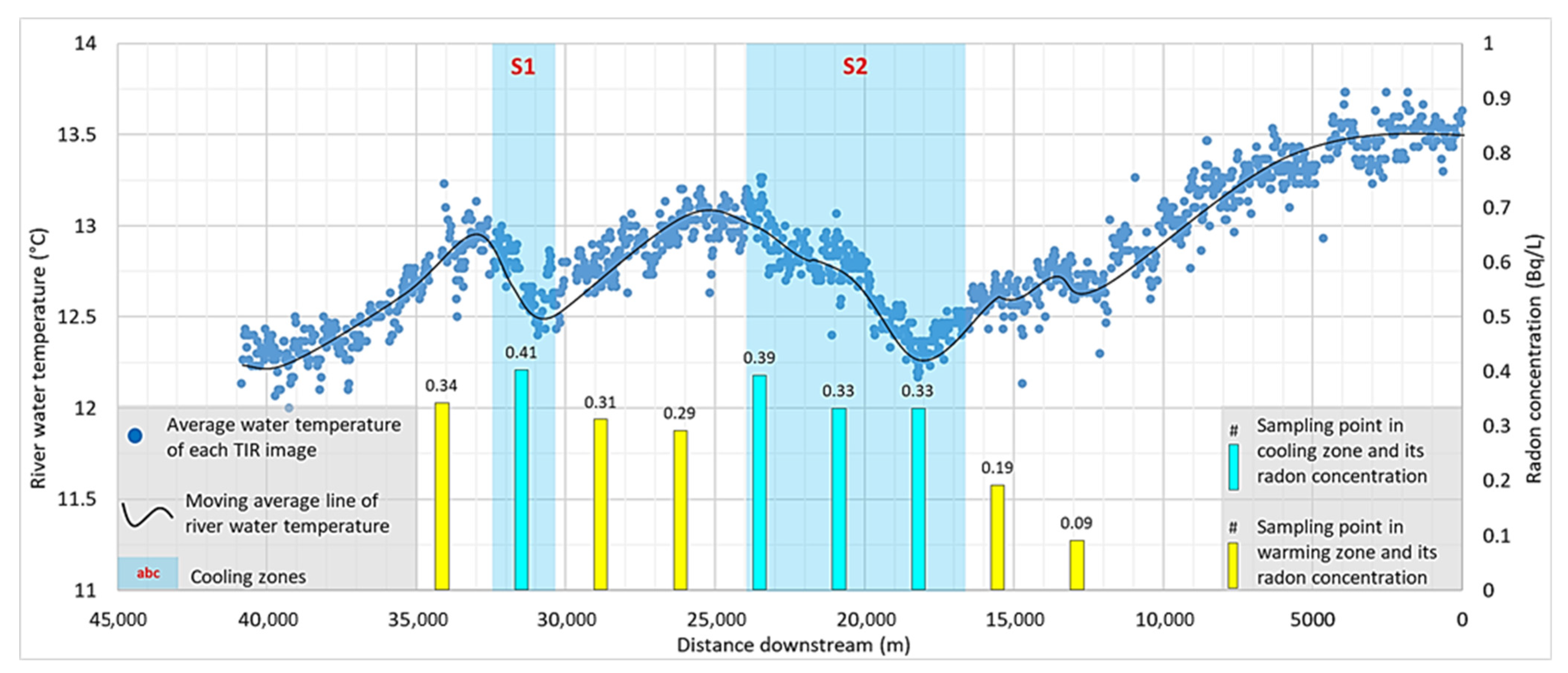
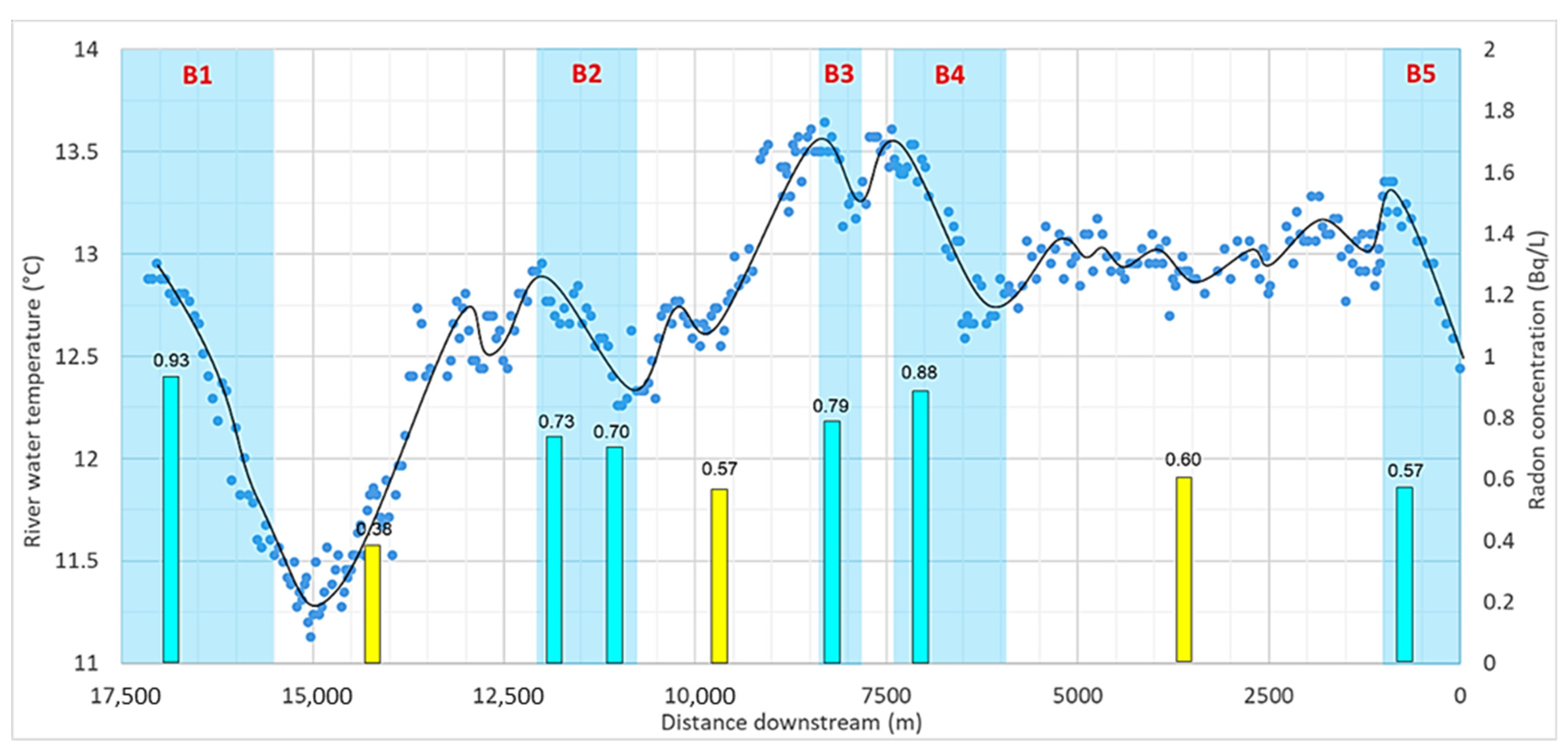
4.1. River Temperature Profile
4.2. Radon Measurements
5. Discussion
5.1. TIR Imaging Method
5.2. Radon Measurements
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Power, G.; Brown, R.S.; Imhof, J.G. Groundwater and fish—Insights from northern North America. Hydrol. Process. 1999, 13, 401–422. [Google Scholar] [CrossRef]
- Anderson, M.P. Heat as a Ground Water Tracer. Groundwater 2005, 43, 951–968. [Google Scholar] [CrossRef]
- Geist, D.R.; Dauble, D.D. Redd site selection and spawning habitat use by fall chinook salmon: The importance of geomorphic features in large rivers. Environ. Manage. 1998, 22, 655–669. [Google Scholar] [CrossRef]
- Baxter, C.V.; Hauer, F.R. Geomorphology, hyporheic exchange, and selection of spawning habitat by bull trout (Salvelinus confluentus). Can. J. Fish. Aquat. Sci. 2000, 57, 1470–1481. [Google Scholar] [CrossRef]
- Dugdale, S.J.; Franssen, J.; Corey, E.; Bergeron, N.E.; Lapointe, M.; Cunjak, R.A. Main stem movement of Atlantic salmon parr in response to high river temperature. Ecol. Freshw. Fish 2015, 25, 429–445. [Google Scholar] [CrossRef]
- Frechette, D.M.; Dugdale, S.J.; Dodson, J.J.; Bergeron, N.E. Understanding summertime thermal refuge use by adult Atlantic salmon using remote sensing, river temperature monitoring, and acoustic telemetry1. Can. J. Fish. Aquat. Sci. 2018, 75, 1999–2010. [Google Scholar] [CrossRef]
- Ebersole, J.L.; Liss, W.J.; Frissell, C.A. Cold water patches in warm streams: Physicochemical characteristics and the influence of shading. J. Am. Water Resour. Assoc. 2003, 39, 355–368. [Google Scholar] [CrossRef]
- Colombo, A.F.; Etkin, D.; Karney, B.W. Climate Variability and the Frequency of Extreme Temperature Events for Nine Sites across Canada: Implications for Power Usage. J. Clim. 1999, 12, 2490–2502. [Google Scholar] [CrossRef]
- Fortin, G.; Acquaotta, F.; Fratianni, S. The evolution of temperature extremes in the Gaspé Peninsula, Quebec, Canada (1974–2013). Theor. Appl. Climatol. 2017, 130, 163–172. [Google Scholar] [CrossRef]
- Breton, M.-P.; Cloutier, G.; Waygood, E.O.D. QUEBEC. In Climate Risks and Adaptation Practices for the Canadian Transportation Sector 2016; Government of Canada: Ottawa, ON, Canada, 2017; pp. 181–216. [Google Scholar]
- Gunn, J.; Snucins, E. Brook charr mortalities during extreme temperature events in Sutton River, Hudson Bay Lowlands, Canada. Hydrobiologia 2010, 650, 79–84. [Google Scholar] [CrossRef]
- Poesch, M.S.; Chavarie, L.; Chu, C.; Pandit, S.N.; Tonn, W. Climate Change Impacts on Freshwater Fishes: A Canadian Perspective. Fisheries 2016, 41, 385–391. [Google Scholar] [CrossRef]
- Willms, T.; Whitworth, G. Mapping of Critical Summer Thermal Refuge Habitats for Chinook Salmon, Coho Salmon, Steelhead and Bull Trout in the Nicola River Watershed—2016; Report submitted to Habitat Stewardship Program for Species at Risk project reference number: 2016HSP7592; Fraser Basin Council: Kamloops, BC, Canada, 2016. [Google Scholar]
- Torgersen, C.; Ebersole, J. Primer for Identifying Cold-Water Refuges to Protect and Restore Thermal Diversity in Riverine Landscapes; Report prepared for region 10, Washington report number: EPA 910-C-12-001; U.S. Environmental Protection Agency: Seattle, DC, USA, 2012. [Google Scholar]
- Mejia, F.H.; Torgersen, C.E.; Berntsen, E.K.; Maroney, J.R.; Connor, J.M.; Fullerton, A.H.; Ebersole, J.L.; Lorang, M.S. Longitudinal, Lateral, Vertical, and Temporal Thermal Heterogeneity in a Large Impounded River: Implications for Cold-Water Refuges. Remote Sens. 2020, 12, 1386. [Google Scholar] [CrossRef]
- Dugdale, S.J.; Bergeron, N.E.; St-Hilaire, A. Spatial distribution of thermal refuges analysed in relation to riverscape hydromorphology using airborne thermal infrared imagery. Remote Sens. Environ. 2015, 160, 43–55. [Google Scholar] [CrossRef]
- Dugdale, S.J.; Bergeron, N.E.; St-Hilaire, A. Temporal variability of thermal refuges and water temperature patterns in an Atlantic salmon river. Remote Sens. Environ. 2013, 136, 358–373. [Google Scholar] [CrossRef]
- Fakhari, M.; Raymond, J.; Martel, R.; Dugdale, S.J.; Bergeron, N. Identification of Thermal Refuges and Water Temperature Patterns in Salmonid-Bearing Subarctic Rivers of Northern Quebec. Geographies 2022, 2, 528–548. [Google Scholar] [CrossRef]
- Wawrzyniak, V.; Piégay, H.; Poirel, A. Longitudinal and temporal thermal patterns of the French Rhône River using Landsat ETM+ thermal infrared images. Aquat. Sci. 2011, 74, 405–414. [Google Scholar] [CrossRef]
- Wu, Y.; Wen, X.; Zhang, Y. Analysis of the exchange of groundwater and river water by using Radon-222 in the middle Heihe Basin of northwestern China. Environ. Geol. 2004, 45, 647–653. [Google Scholar] [CrossRef]
- Ortega, L.; Manzano, M.; Custodio, E.; Hornero, J.; Rodríguez-Arévalo, J. Using 222Rn to identify and quantify groundwater inflows to the Mundo River (SE Spain). Chem. Geol. 2015, 395, 67–79. [Google Scholar] [CrossRef]
- Berth, C.; Bourg, A.C.M. Radon-222 and Chloride as Natural Tracers of the Infiltration of River Water into an Alluvial Aquifer in Which There. Environ. Sci. Technol. 1994, 1994, 794–798. [Google Scholar]
- Hoehn, E.; Von Gunten, H.R. Radon in groundwater: A tool to assess infiltration from surface waters to aquifers. Water Resour. Res. 1989, 25, 1795–1803. [Google Scholar] [CrossRef]
- Rogers, S. Physical Behavior and Geologic Control of Radon in Mountain Streams; Experimental and theoretical geophysics. U.S. Geological Survey. Bulletin 1052-E., Prepared on behalf of the U.S. Atomic Energy Commission; United State Government Printing Office: Washington, DC, USA, 1958. [Google Scholar]
- Schubert, M.; Knoeller, K.; Treutler, H.-C.; Weiss, H.; Dehnert, J. 222Rn as a tracer for the estimation of infiltration of surface waters into aquifers. Radioact. Environ. 2006, 8, 326–334. [Google Scholar]
- Yi, P.; Luo, H.; Chen, L.; Yu, Z.; Jin, H.; Chen, X.; Wan, C.; Ale, A.; Zheng, M.; Hu, Q. Evaluation of groundwater discharge into surface water by using Radon-222 in the Source Area of the Yellow River, Qinghai-Tibet Plateau. J. Environ. Radioact. 2018, 192, 257–266. [Google Scholar] [CrossRef] [PubMed]
- Cook, P.G.; Lamontagne, S.; Berhane, D.; Clark, J.F. Quantifying groundwater discharge to Cockburn River, southeastern Australia, using dissolved gas tracers 222Rn and SF6. Water Resour. Res. 2006, 42, 1–12. [Google Scholar] [CrossRef]
- Corbett, D.R.; Burnett, W.C.; Cable, P.H.; Clark, S.B. Radon tracing of groundwater input into Par Pond, Savannah River Site. J. Hydrol. 1997, 203, 209–227. [Google Scholar] [CrossRef]
- Burnett, W.C.; Peterson, R.N.; Santos, I.R.; Hicks, R.W. Use of automated radon measurements for rapid assessment of groundwater flow into Florida streams. J. Hydrol. 2010, 380, 298–304. [Google Scholar] [CrossRef]
- Kelly, J.L.; Dulai, H.; Glenn, C.R.; Lucey, P.G. Integration of aerial infrared thermography and in situ radon-222 to investigate submarine groundwater discharge to Pearl Harbor, Hawaii, USA. Limnol. Oceanogr. 2019, 64, 238–257. [Google Scholar] [CrossRef]
- Wilson, J.; Rocha, C. A combined remote sensing and multi-tracer approach for localising and assessing groundwater-lake interactions. Int. J. Appl. Earth Obs. Geoinf. 2016, 44, 195–204. [Google Scholar] [CrossRef]
- Malard, F.; Tockner, K.; Ward, J.V. Shifting dominance of subcatchment water sources and flow paths in a glacial floodplain, Val Roseg, Switzerland. Arct. Antarct. Alp. Res. 1999, 31, 135–150. [Google Scholar] [CrossRef]
- ClimateData.ca. Environment and Climate Change Canada Data Servers End-use Licence. Available online: https://eccc-msc.github.io/open-data/licence/readme_en/ (accessed on 1 September 2021).
- Lemieux, J.M.; Fortier, R.; Talbot-Poulin, M.C.; Molson, J.; Therrien, R.; Ouellet, M.; Banvil, D. Occurrence of groundwater in cold environments: Examples from Nunavik, Canada. Hydrogeol. J. 2016, 24, 1497–1513. [Google Scholar] [CrossRef]
- Gouvernement du Québec, “Climate Normals 1981–2010,” Ministère De l’Environnement et de la Lutte Contre les Changements Climatiques. 2021. Available online: https://www.environnement.gouv.qc.ca/climat/normales/climat-qc.htm (accessed on 20 March 2019).
- SIGÉOM. Surface Deposits Map. Système D’information Géominière du Quebec. 2020. Available online: https://sigeom.mines.gouv.qc.ca/signet/classes/I1108_afchCarteIntr (accessed on 3 June 2021).
- Fullerton, A.H.; Torgersen, C.E.; Lawler, J.J.; Faux, R.N.; Steel, E.A.; Beechie, T.J. Rethinking the longitudinal stream temperature paradigm: Region-wide comparison of thermal infrared imagery reveals unexpected complexity of river temperatures. Hydrol. Process. 2015, 29, 4719–4737. [Google Scholar] [CrossRef]
- Elzain, A.A. Measurement of Radon-222 concentration levels in water samples in Sudan. Adv. Appl. Sci. Res. 2014, 5, 229–234. [Google Scholar]
- Pylon. Pylon AB5 Portable Radiation Monitor. Pylon Electronic Inc. 2022. Available online: https://pylonelectronics-radon.com/wp-content/uploads/2019/08/DS138R2-AB7.pdf (accessed on 10 December 2022).
- Rosenberry, D.O.; LaBaugh, J.W. Field Techniques for Estimating Water Fluxes Between Surface Water and Ground Water Techniques and Methods 4—D2; U.S. Geological Survey: Reston, VA, USA, 2008. [Google Scholar]
- Faux, R.N.; Maus, P.; Lachowski, H.; Torgersen, C.E.; Boyd, M.S. New Approaches for Monitoring Stream Temperature: Airborne Thermal Infrared Remote Sensing; US Department of Agriculture Forest Service Engineering, Remote Sensing Applications Center: Salt Lake City, UT, USA, 2001. [Google Scholar]
- Kaandorp, V.P.; Doornenbal, P.J.; Kooi, H.; Broers, H.P.; de Louw, P.G. Temperature buffering by groundwater in ecologically valuable lowland streams under current and future climate conditions. J. Hydrol. X 2019, 3, 100031. [Google Scholar] [CrossRef]
- Dugdale, S.J.; Kelleher, C.A.; Malcolm, I.A.; Caldwell, S.; Hannah, D.M. Assessing the potential of drone-based thermal infrared imagery for quantifying river temperature heterogeneity. Hydrol. Process. 2019, 33, 1152–1163. [Google Scholar] [CrossRef]
- Hahn, E.J.; Gokun, Y.; Andrews Jr, W.M.; Overfield, B.L.; Robertson, H.; Wiggins, A.; Rayens, M.K. Radon potential, geologic formations, and lung cancer risk. Prev. Med. Rep. 2015, 2, 342–346. [Google Scholar] [CrossRef] [PubMed]
- Ershaidat, N.M.; Al-Bataina, B.A.; Al-Shereideh, S.A. Characteristics of soil radon transport in different geological formations. Environ. Geol. 2007, 55, 29–35. [Google Scholar] [CrossRef]
- Drolet, J.-P.; Martel, R.; Poulin, P.; Dessau, J.-C.; Lavoie, D.; Parent, M.; Lévesque, B. An approach to define potential radon emission level maps using indoor radon concentration measurements and radiogeochemical data positive proportion relationships. J. Environ. Radioact. 2013, 124, 57–67. [Google Scholar] [CrossRef]
- Xie, Y.; Cook, P.G.; Shanafield, M.; Simmons, C.T.; Zheng, C. Uncertainty of natural tracer methods for quantifying river-aquifer interaction in a large river. J. Hydrol. 2016, 535, 135–147. [Google Scholar] [CrossRef]






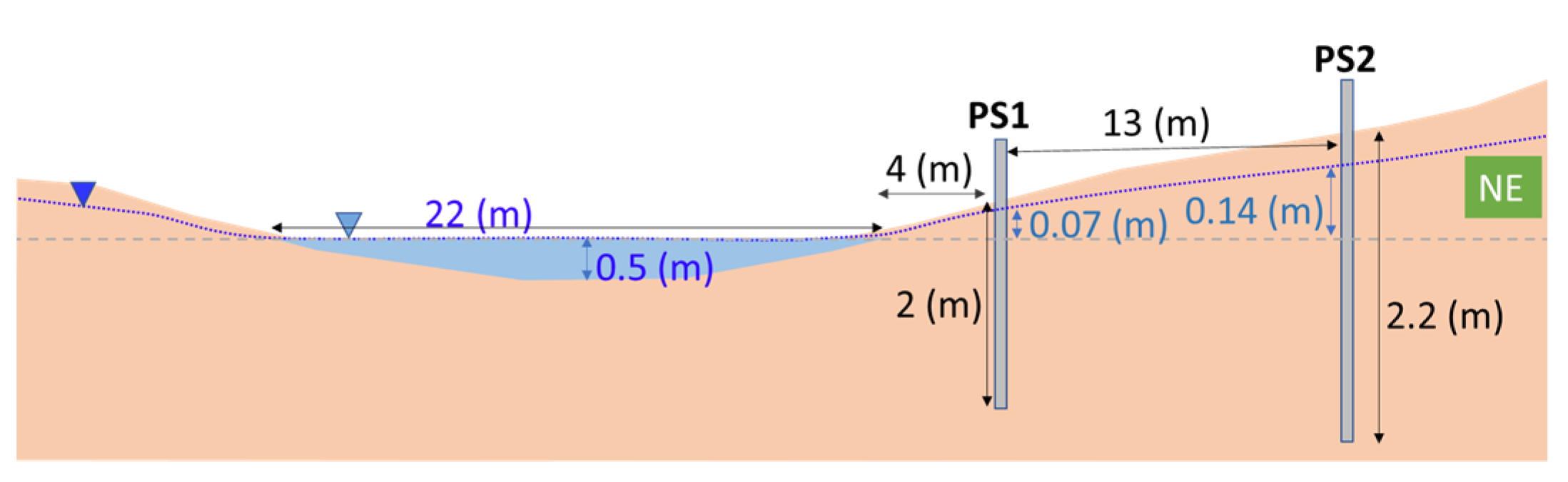

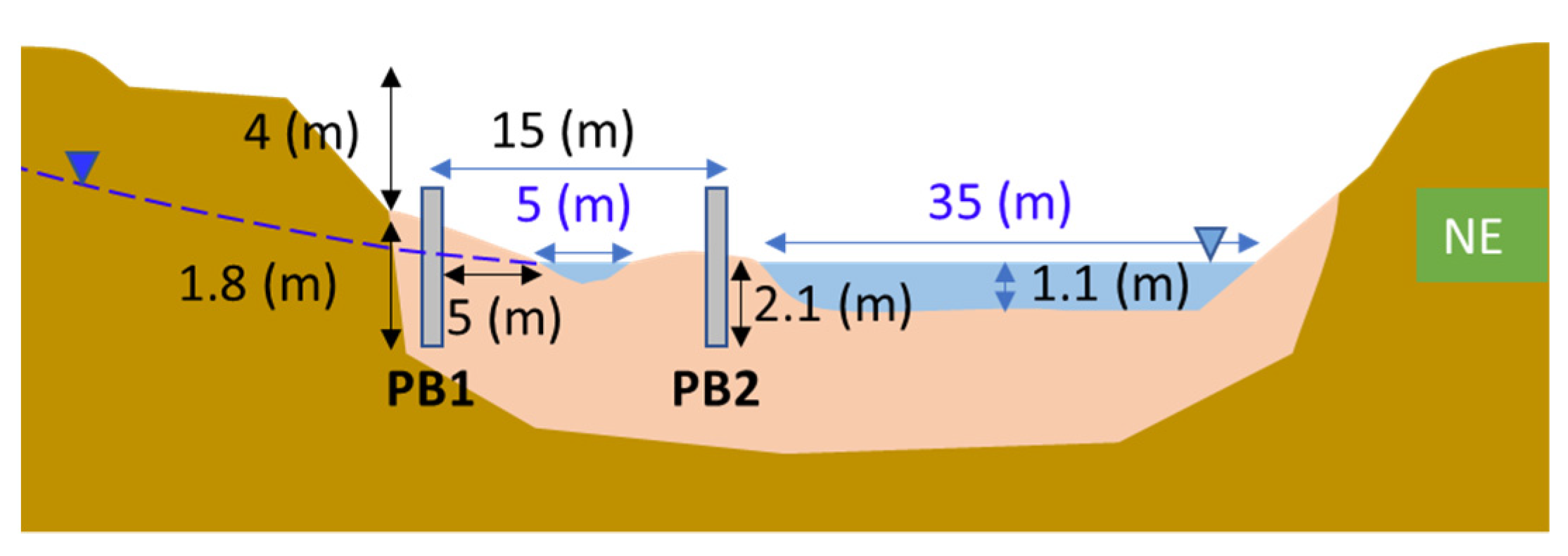
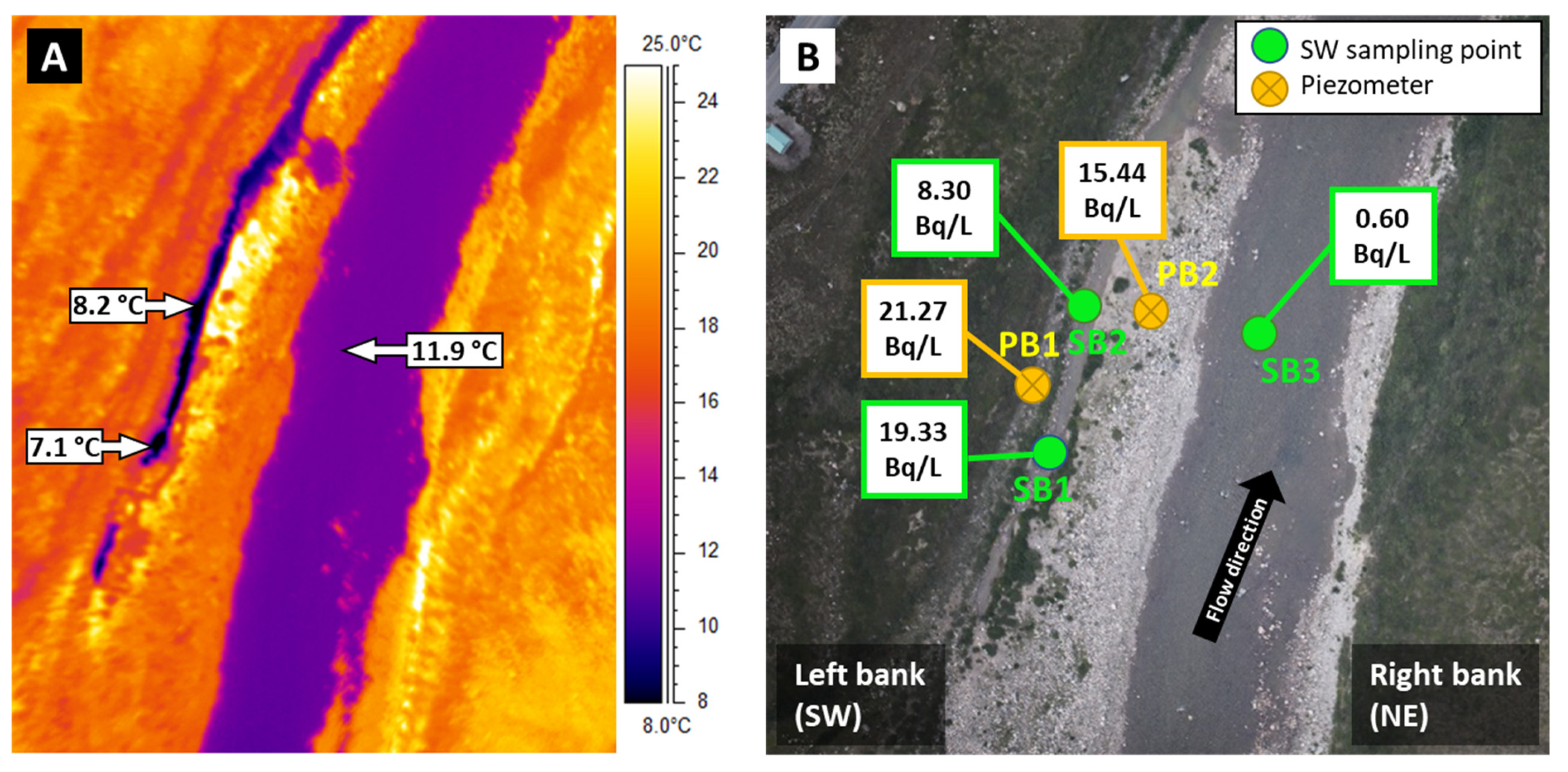
| River | Cooling Zone | Cg (Bq/L) | Cb (Bq/L) | Cs (Bq/L) | f (%) |
|---|---|---|---|---|---|
| St-Marguerite | S1 | 6.23 | 0.34 | 0.41 | 1.1 |
| S2 | 0.29 | 0.34 | 0.9 | ||
| Berard | B1 | 10.85 | - | 0.93 | - |
| B2 | 0.38 | 0.72 | 3.2 | ||
| B3 | 0.57 | 0.79 | 2.1 | ||
| B4 | 0.57 | 0.88 | 3.0 | ||
| B5 | 0.60 | 0.57 | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fakhari, M.; Raymond, J.; Martel, R.; Drolet, J.-P.; Dugdale, S.J.; Bergeron, N. Analysis of Large-Scale Groundwater-Driven Cooling Zones in Rivers Using Thermal Infrared Imagery and Radon Measurements. Water 2023, 15, 873. https://doi.org/10.3390/w15050873
Fakhari M, Raymond J, Martel R, Drolet J-P, Dugdale SJ, Bergeron N. Analysis of Large-Scale Groundwater-Driven Cooling Zones in Rivers Using Thermal Infrared Imagery and Radon Measurements. Water. 2023; 15(5):873. https://doi.org/10.3390/w15050873
Chicago/Turabian StyleFakhari, Milad, Jasmin Raymond, Richard Martel, Jean-Philippe Drolet, Stephen J. Dugdale, and Normand Bergeron. 2023. "Analysis of Large-Scale Groundwater-Driven Cooling Zones in Rivers Using Thermal Infrared Imagery and Radon Measurements" Water 15, no. 5: 873. https://doi.org/10.3390/w15050873
APA StyleFakhari, M., Raymond, J., Martel, R., Drolet, J.-P., Dugdale, S. J., & Bergeron, N. (2023). Analysis of Large-Scale Groundwater-Driven Cooling Zones in Rivers Using Thermal Infrared Imagery and Radon Measurements. Water, 15(5), 873. https://doi.org/10.3390/w15050873







