Experimental Study on Neck Cutoff in Meandering River under Variable Discharges
Abstract
1. Introduction
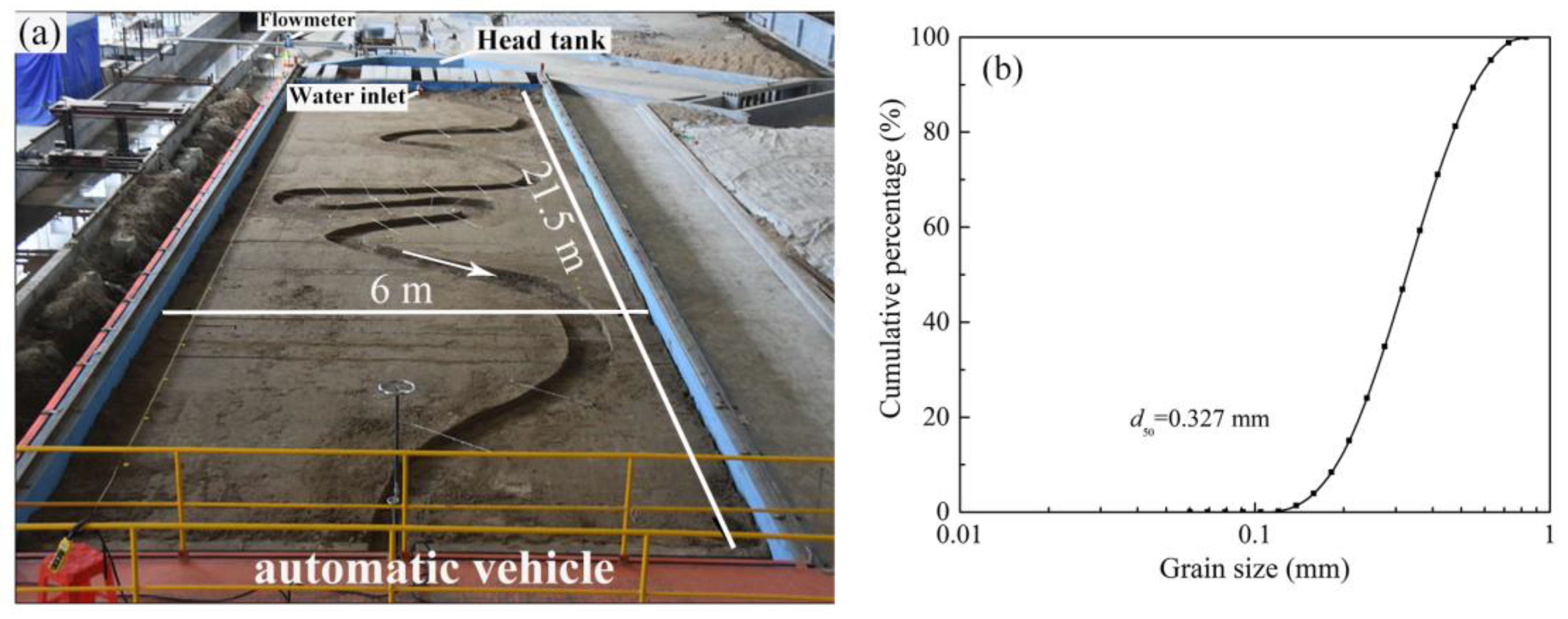
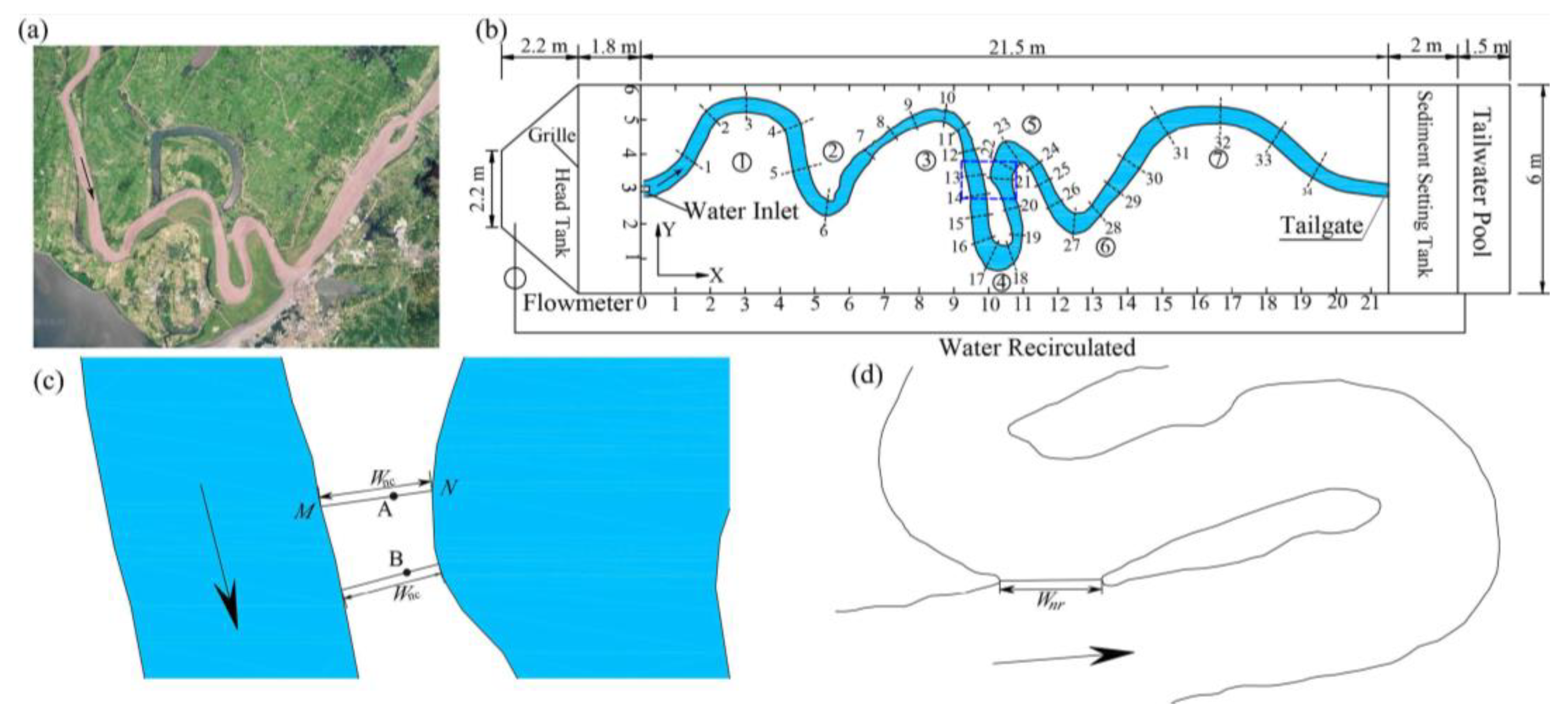
2. Experiment Setup
2.1. Experimental System
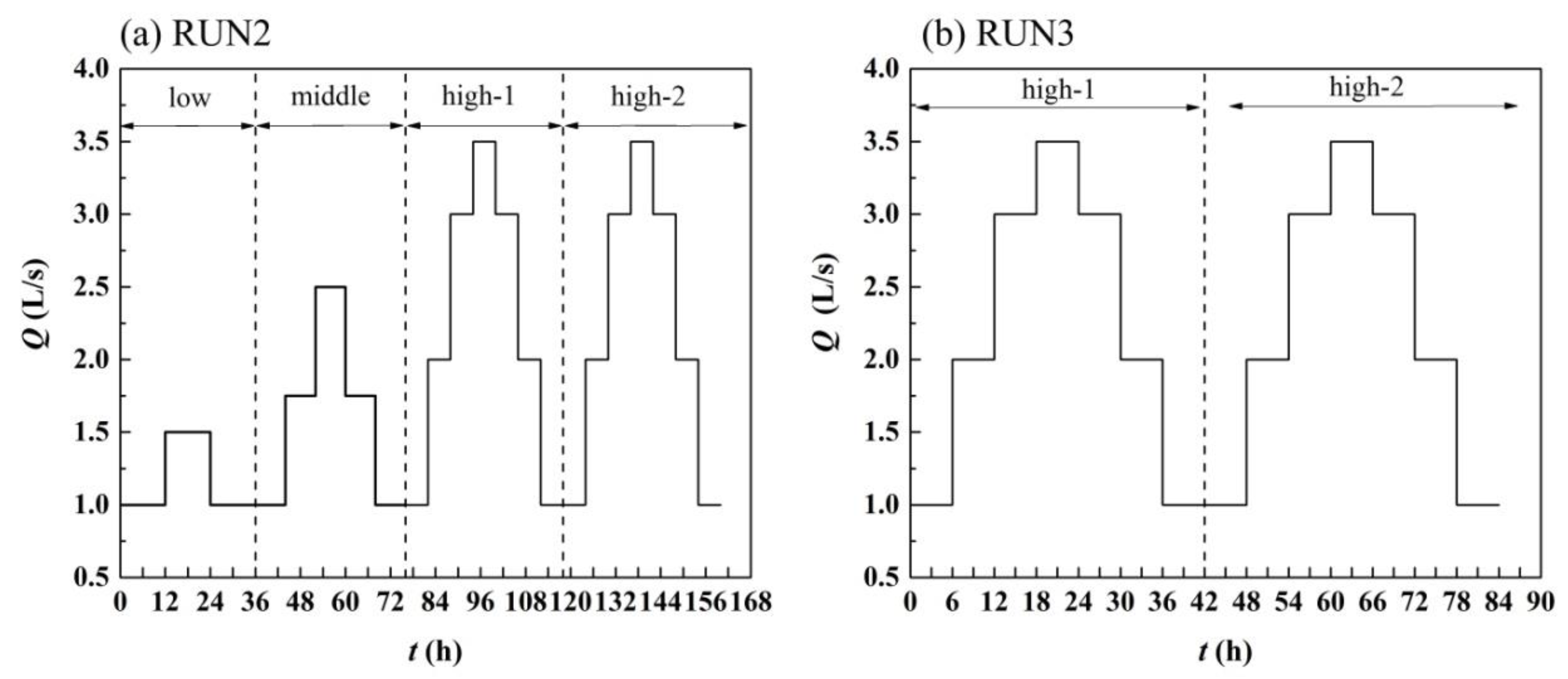
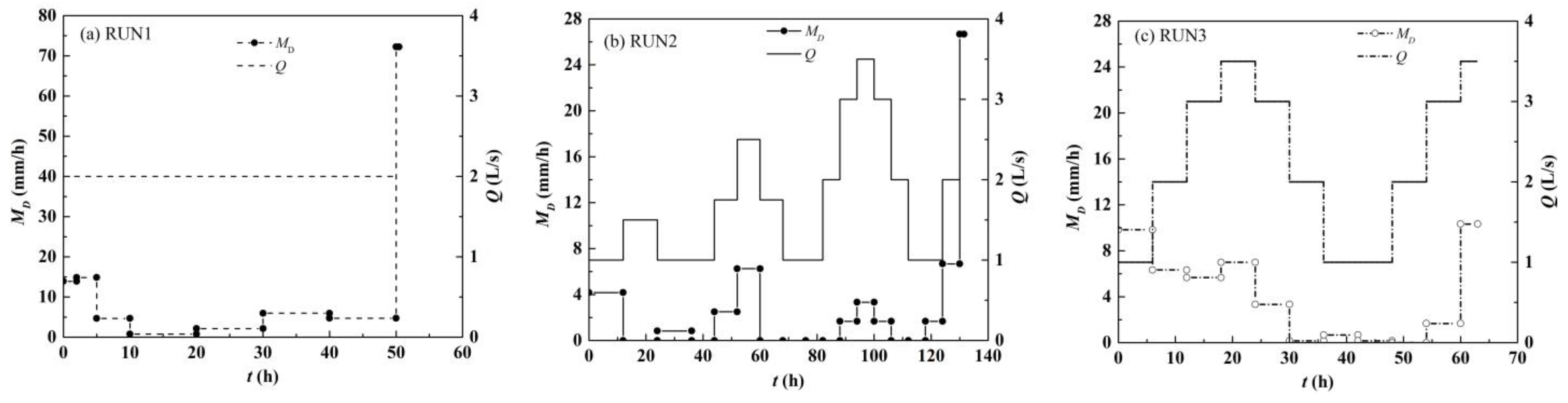
2.2. Method
2.3. Data Collection
3. Results and Analysis
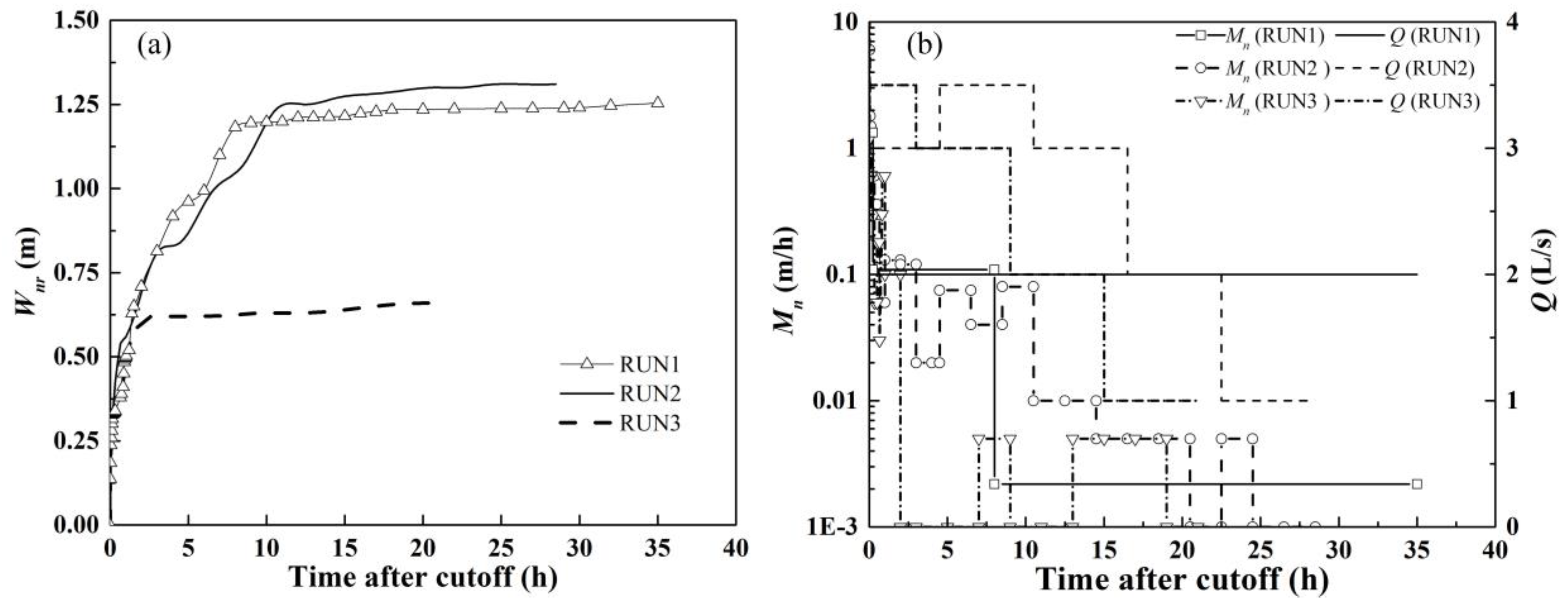
3.1. Neck Width Change before Cutoff
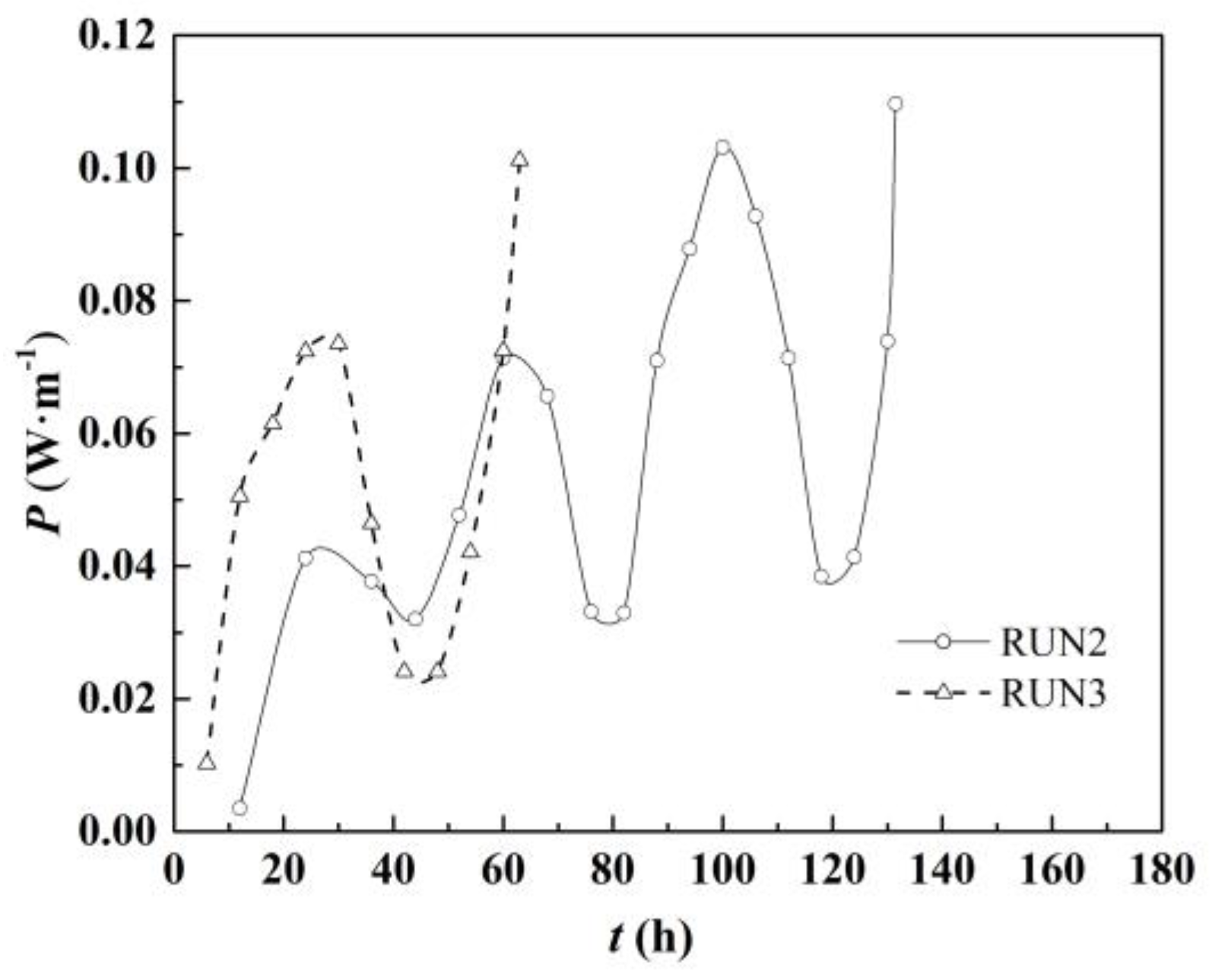
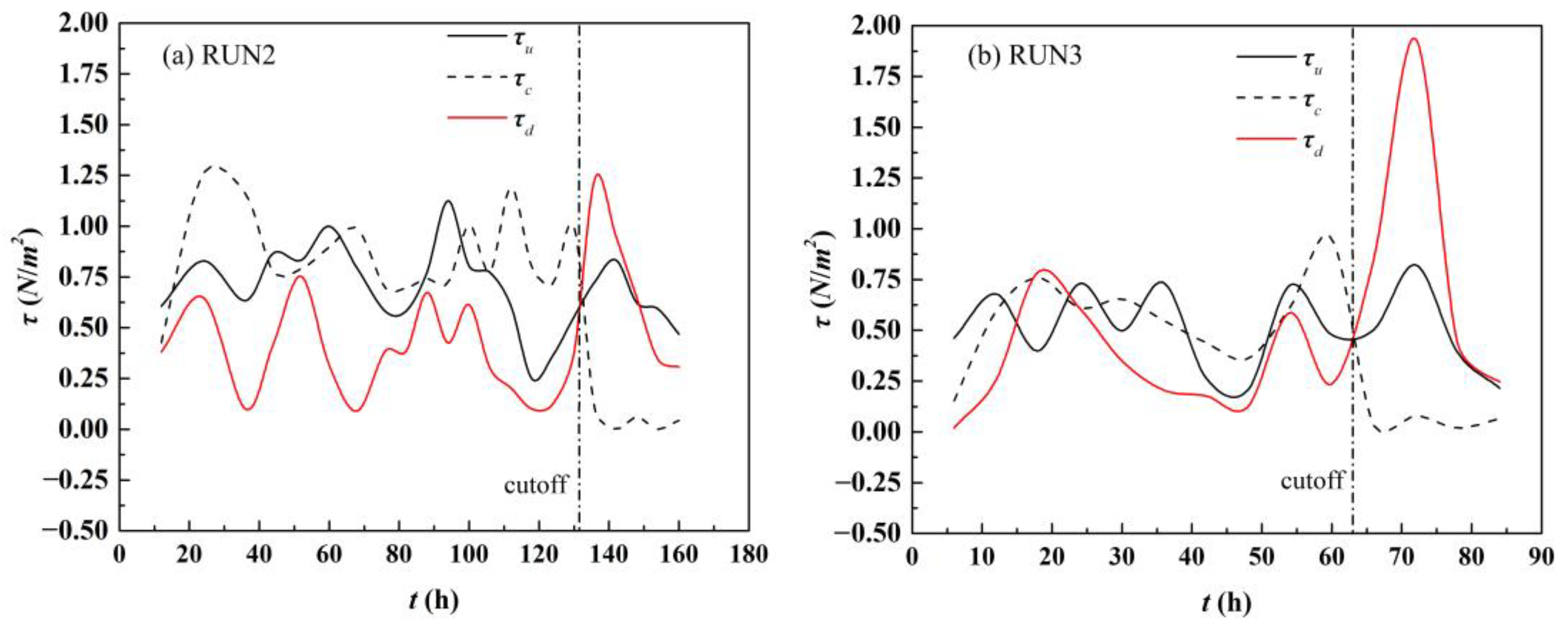
3.2. Change in the Water Level Difference and Stream Power before Cutoff
3.3. Evolution of the New Channel
3.4. Channel Response to Neck Cutoff
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kleinhans, M.G. Sorting out river channel patterns. Prog. Phys. Geogr. 2010, 34, 287–326. [Google Scholar] [CrossRef]
- Yin, X.; Liang, Z.; Chen, J.; Liu, X. River Patterns and Their Changes. J. Sediment. Res. 1999, 13–19. [Google Scholar]
- Schumm, S.A. Patterns of Alluvial Rivers. Annu. Rev. Earth Planet. Sci. 1985, 13, 5–27. [Google Scholar] [CrossRef]
- Güneralp, İ.; Abad, J.D.; Zolezzi, G.; Hooke, J. Advances and challenges in meandering channels research. Geomorphology 2012, 163-164, 1–9. [Google Scholar] [CrossRef]
- Seminara, G. Meanders. J. Fluid Mech. 2006, 554, 271–297. [Google Scholar] [CrossRef]
- Callander, R.A. River Meandering. Ann. Rev. Fluid Mech. 1978, 10, 129–158. [Google Scholar] [CrossRef]
- Parker, G. On the cause and characteristic scales of meandering and braiding in rivers. J. Fluid Mech. 1976, 76, 457–480. [Google Scholar] [CrossRef]
- Richang, T. Preliminary analysis of the cause of meandering river section and experimental study of bed making. Acta Geol. Sin.-Engl. Ed. 1963, 29, 13–21. [Google Scholar]
- Hooke, J.M. Cutoffs galore!: Occurrence and causes of multiple cutoffs on a meandering river. Geomorphology 2004, 61, 225–238. [Google Scholar] [CrossRef]
- Hooke, J.M. River channel adjustment to meander cutoffs on the River Bollin and River Dane, northwest England. Geomorphology 1995, 14, 235–253. [Google Scholar] [CrossRef]
- Micheli, E.R.; Larsen, E.W. River channel cutoff dynamics, Sacramento River, California, USA. River Res. Appl. 2015, 27, 328–344. [Google Scholar] [CrossRef]
- Zinger, J.A.; Rhoads, B.L.; Best, J.L.; Johnson, K.K. Flow structure and channel morphodynamics of meander bend chute cutoffs: A case study of the Wabash River, USA. J. Geophys. Res. Earth Surf. 2013, 118, 2468–2487. [Google Scholar] [CrossRef]
- Qiao, Q.; Chun-guang, L.; He-fang, J.; Ling-xiao, H.; Cheng, Y. Impact of an artificial chute cutoff on the river morphology and flow structure in Sipaikou area of the Upper Yellow River. J. Mt. Sci. 2021, 18, 3275–3290. [Google Scholar] [CrossRef]
- Fuller, I.C.; Large, A.R.G.; Milan, D.J. Quantifying channel development and sediment transfer following chute cutoff in a wandering gravel-bed river. Geomorphology 2003, 54, 307–323. [Google Scholar] [CrossRef]
- Constantine, J.A.; McLean, S.R.; Dunn, T. A mechanism of chute cutoff along large meandering rivers with uniform floodplain topography. Geol. Soc. Am. Bull. 2010, 122, 855–869. [Google Scholar] [CrossRef]
- Eekhout, J.P.C.; Hoitink, A.J.F. Chute cutoff as a morphological response to stream reconstruction: The possible role of backwater. Water Resour. Res. 2015, 51, 3339–3352. [Google Scholar] [CrossRef]
- Viero, D.P.; Dubon, S.L.; Lanzoni, S. Chute cutoffs in meandering rivers: Formative mechanisms and hydrodynamic forcing. Int. Assoc. Sedimentol. Spec. Publ. 2018, 48, 201–230. [Google Scholar]
- Richards, D.; Konsoer, K. Morphologic adjustments of actively evolving highly curved neck cutoffs. Earth Surf. Proc. Land. 2020, 45, 1067–1081. [Google Scholar] [CrossRef]
- Li, Z.; Gao, P.; You, Y.; Finotello, A.; Ielpi, A. Delayed neck cutoff in the meandering Black River of the Qinghai–Tibet plateau. Earth Surf. Proc. Land. 2022, 1–12. [Google Scholar] [CrossRef]
- Li, Z.; Wu, X.; Gao, P. Experimental study on the process of neck cutoff and channel adjustment in a highly sinuous meander under constant discharges. Geomorphology 2018, 327, 215–229. [Google Scholar] [CrossRef]
- Li, Z.; Yang, H.; Xia, J.; Zhou, M.; Wang, Y. Channel morphologic processes of a highly sinuous bend approaching neck cutoff by bank erosion in the middle Yangtze River. Int. J. Sediment Res. 2021, 36, 457–467. [Google Scholar] [CrossRef]
- Gay, G.R.; Gay, H.H.; Gay, W.H.; Martinson, H.A.; Meade, R.H.; Moody, J.A. Evolution of cutoffs across meander necks in Powder River, Montana, USA. Earth Surf. Proc. Land. 2015, 23, 651–662. [Google Scholar] [CrossRef]
- Slowik, M. The influence of meander bend evolution on the formation of multiple cutoffs: Findings inferred from floodplain architecture and bend geometry. Earth Surf. Proc. Land. 2015, 41, 626–641. [Google Scholar] [CrossRef]
- Hooke, J.M. Complexity, self-organisation and variation in behaviour in meandering rivers. Geomorphology 2007, 91, 236–258. [Google Scholar] [CrossRef]
- Gautier, E.; Brunstein, D.; Vauchel, P.; Roulet, M.; Fuertes, O.; Guyot, L.; Darozzes, J.; Bourrel, L. Temporal relations between meander deformation, water discharge and sediment fluxes in the floodplain of the Rio Beni (Bolivian Amazonia). Earth Surf. Proc. Land. 2010, 32, 230–248. [Google Scholar] [CrossRef]
- Thompson, D.M. A Geomorphic Explanation for a Meander Cutoff Following Channel Relocation of a Coarse-Bedded River. Environ. Manag. 2003, 31, 385–400. [Google Scholar] [CrossRef]
- Braudrick, C.A.; Dietrich, W.E.; Leverich, G.T.; Sklar, L.S. Experimental evidence for the conditions necessary to sustain meandering in coarse-bedded rivers. Proc. Natl. Acad. Sci. USA 2009, 106, 16936–16941. [Google Scholar] [CrossRef]
- Visconti, F.; Camporeale, C.; Ridolfi, L. Role of discharge variability on pseudomeandering channel morphodynamics: Results from laboratory experiments. J. Geophys. Res. 2010, 115, 1–18. [Google Scholar] [CrossRef]
- Monegaglia, F.; Tubino, M. The Hydraulic Geometry of Evolving Meandering Rivers. J. Geophys. Res. Earth Surf. 2019, 124, 2723–2748. [Google Scholar] [CrossRef]
- Dulal, K.P.; Shimizu, Y. Experimental simulation of meandering in clay mixed sediments. J. Hydro-Environ. Res. 2010, 4, 329–343. [Google Scholar] [CrossRef]
- Van Dijk, W.M.; van de Lageweg, W.I.; Kleinhans, M.G. Formation of a cohesive floodplain in a dynamic experimental meandering river. Earth Surf. Proc. Land. 2013, 38, 1550–1565. [Google Scholar]
- Peakall, J.; Ashworth, P.J.; Best, J.L. Meander-Bend Evolution, Alluvial Architecture, and the Role of Cohesion in Sinuous River Channels: A Flume Study. J. Sediment. Res. 2007, 77, 197–212. [Google Scholar] [CrossRef]
- Smith, C.E. Modeling high sinuosity meanders in a small flume. Geomorphology 1998, 25, 19–30. [Google Scholar] [CrossRef]
- Dijk, W.M.V.; van de Lageweg, W.I.; Kleinhans, M.G. Experimental meandering river with chute cutoffs. J. Geophys. Res. 2012, 117, 1–18. [Google Scholar]
- Xu, D.; Bai, Y. Experimental study on the bed topography evolution in alluvial meandering rivers with various sinuousnesses. J. Hydro-Environ. Res. 2013, 7, 92–102. [Google Scholar] [CrossRef]
- Song, X.; Xu, G.; Bai, Y.; Xu, D. Experiments on the short-term development of sine-generated meandering rivers. J. Hydro-Environ. Res. 2016, 11, 42–58. [Google Scholar] [CrossRef]
- Eaton, B.C.; Giles, T.R. Assessing the effect of vegetation-related bank strength on channel morphology and stability in gravel-bed streams using numerical models. Earth Surf. Proc. Land. 2009, 34, 712–724. [Google Scholar] [CrossRef]
- Eaton, B.C. Bank stability analysis for regime models of vegetated gravel bed rivers. Earth Surf. Proc. Land. 2006, 31, 1438–1444. [Google Scholar] [CrossRef]
- Tal, M.; Paola, C. Effects of vegetation on channel morphodynamics: Results and insights from laboratory experiments. Earth Surf. Proc. Land. 2010, 35, 1014–1028. [Google Scholar] [CrossRef]
- Polvi, L.E.; Wohl, E.; Merritt, D.M. Modeling the functional influence of vegetation type on streambank cohesion. Earth Surf. Proc. Land. 2014, 39, 1245–1258. [Google Scholar] [CrossRef]
- Millar, R.G. Influence of bank vegetation on alluvial channel patterns. Water Resour. Res. 2000, 36, 1109–1118. [Google Scholar] [CrossRef]
- Gran, K.; Paola, C. Riparian vegetation controls on braided stream dynamics. Water Resour. Res. 2001, 37, 3275–3283. [Google Scholar] [CrossRef]
- Corenblit, D.; Steiger, J.; Gurnell, A.M.; Tabacchi, E.; Roques, L. Control of sediment dynamics by vegetation as a key function driving biogeomorphic succession. Earth Surf. Proc. Land. 2009, 34, 1790–1810. [Google Scholar] [CrossRef]
- Tal, M.; Paola, C. Dynamic single-thread channels maintained by the interaction of flow and vegetation. Geology 2007, 35, 1651–1656. [Google Scholar] [CrossRef]
- Li, Z.; Gao, P.; Wu, X. Processes of neck cutoff and channel adjustment affected by seeding herbaceous vegetation and variable discharges. CATENA 2022, 208, 105731. [Google Scholar] [CrossRef]
- Han, B.; Endreny, T.A. Detailed river stage mapping and head gradient analysisduring meander cutoff in a laboratory river. Water Resour. Res. 2014, 50, 1689–1703. [Google Scholar] [CrossRef]
- Kleinhans, M.G.; van den Berg, J.H. River channel and bar patterns explained and predicted by an empirical and a physics-based method. Earth Surf. Proc. Land. 2011, 36, 721–738. [Google Scholar] [CrossRef]
- Lague, D. The stream power river incision model: Evidence, theory and beyond. Earth Surf. Proc. Land. 2014, 39, 38–61. [Google Scholar] [CrossRef]
- Petit, F.; Gob, F.; Houbrechts, G.; Assani, A.A. Critical specific stream power in gravel-bed rivers. Geomorphology 2005, 69, 92–101. [Google Scholar] [CrossRef]
- Moore, I.D.; Burch, G.J. Sediment Transport Capacity of Sheet and Rill Flow: Application of Unit Stream Power Theory. Water Resour. Res. 1986, 22, 1350–1360. [Google Scholar] [CrossRef]
- Yang, C.T.; Stall, J.B. Applicability of Unit Stream Power Equation. ASCE 1976, 102, 559–568. [Google Scholar] [CrossRef]
- Bendix, J. Stream power influence on southern Californian riparian vegetation. J. Veg. Sci. 1999, 10, 243–252. [Google Scholar] [CrossRef]
- Stolum, H.H. Planform geometry and dynamics of meandering rivers. Geol. Soc. Am. Bull. 1998, 110, 1485–1498. [Google Scholar] [CrossRef]
- Yang, C.T. Unit stream power equations for total load. J. Hydrol. 1979, 40, 123–138. [Google Scholar] [CrossRef]
- Chang, H.H. Analysis of River Meanders. J. Hydraul. Eng. 1984, 110, 37–50. [Google Scholar] [CrossRef]
- Yang, C.T.; Song, C.C.S.; Woldenberg, M.J. Hydraulic geometry and minimum rate of energy dissipation. Water Resour. Res 1981, 17, 1014–1018. [Google Scholar] [CrossRef]
- Xu, G.; Zhao, L.; Yang, C.T. Derivation and verification of minimum energy dissipation rate principle of fluid based on minimum entropy production rate principle. Int. J. Sediment Res. 2016, 31, 16–24. [Google Scholar] [CrossRef]
- Xu, G.; Lian, J. Changes of the entropy, the entropy production and the rate of energy dissipation in river adjustment. Adv. Water Sci. 2004, 11, 3933–4024. [Google Scholar]





| RUN | Initial Slope Sr | Discharge Q (L/s) | Re | Duration t (h) |
|---|---|---|---|---|
| 1 | 1.0‰ | Constant | 7473–8315 | 85.45 |
| 2 | 1.0‰ | Low–middle–high–high | 1454–5157 | 160 |
| 3 | 1.0‰ | High–high | 1436–5369 | 84 |
| Run | Wu (m) | Wd (m) | Wnc (m) | Cutoff Location | Wu/Wd |
|---|---|---|---|---|---|
| 1 | 0.140 | 0.150 | 0.290 | 0.320 m downstream from S13 | 0.93 |
| 2 | 0.190 | 0.070 | 0.260 | 0.029 m upstream from S13 | 2.71 |
| 3 | 0.092 | 0.148 | 0.240 | 0.026 m downstream from S13 | 0.62 |
| RUN | γ (N∙m−3) | Q (m3/s) | Water Head (cm) | Distance (m) | J | P (W∙m−1) |
|---|---|---|---|---|---|---|
| 1 | 9800 | 0.0020 | 2.07 | 7.667 | 0.00270 | 0.0530 |
| 2 | 9800 | 0.0030 | 1.85 | 5.355 | 0.0035 | 0.1097 |
| 3 | 9800 | 0.0035 | 1.58 | 5.355 | 0.0030 | 0.1012 |
| RUN | Sinuosity | Angle of Rotation Downstream (°) | ||
|---|---|---|---|---|
| Initial | Cutoff | Increase Percentage (%) | ||
| 1 | 6.47 | 6.71 | 3.79 | 11 |
| 2 | 6.69 | 7.09 | 5.89 | 11 |
| 3 | 7.03 | 7.11 | 1.16 | 11 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, X.; Hu, X.; Zhang, X. Experimental Study on Neck Cutoff in Meandering River under Variable Discharges. Water 2023, 15, 841. https://doi.org/10.3390/w15050841
Wu X, Hu X, Zhang X. Experimental Study on Neck Cutoff in Meandering River under Variable Discharges. Water. 2023; 15(5):841. https://doi.org/10.3390/w15050841
Chicago/Turabian StyleWu, Xinyu, Xuyue Hu, and Xiang Zhang. 2023. "Experimental Study on Neck Cutoff in Meandering River under Variable Discharges" Water 15, no. 5: 841. https://doi.org/10.3390/w15050841
APA StyleWu, X., Hu, X., & Zhang, X. (2023). Experimental Study on Neck Cutoff in Meandering River under Variable Discharges. Water, 15(5), 841. https://doi.org/10.3390/w15050841




