Measurement of Total Air Entrainment, Disentrainment and Net Entrainment Flow Rates Utilizing Novel Downcomer Incorporating Al-Anzi’s Disentrainment Ring (ADR) in a Confined Plunging Jet Reactor

Abstract

Plunging liquid jet reactor (PLJR) has gained popularity as a feasible and efficient aerator and mixer. However, the measurement of air disentrainment rate (Q_ads), which affects aeration performance, has been overlooked by many researchers. In this work, a newly invented Al-Anzi disentrainment ring (ADR) device was incorporated in CPLJR to experimentally measure Q_ads and its effect on the net air entrainment rate. Furthermore, the effect of new variables (l_ADR and d_s) and old variables (L_j and V_L) on Q_anet were also investigated. Results showed that shorter d_s and l_ADR produced higher Q_anet for the same ADR device. A new net entrainment jet velocity at the impingement point (V_Lnet) was measured at about 651 cm/s, above which bubbles left the base of downcomer as Q_anet. Q_anet increased linearly with V_L; however, Q_ads increased until it reached maximum value, and then decreased. Bubble penetration depth and liquid rise height increased for all V_L until they reached maximum, and then leveled off for the same l_ADR. A significant increase in Q_anet values was achieved with this downcomer in comparison with the conventional one. The increase in Q_anet was measured to be approximately 2.5–15 times of that measured by the standalone downcomer.

1. Introduction

The plunging liquid jet reactor (PLJR) concept was originated with natural environmental phenomena such as waterfalls and carbon dioxide absorption in oceans [1]. Recently, however, the PLJR concept has been used as an aerator in a wide range of applications, such as aerobic wastewater treatment processes, fermentation processes, biological aerated filters, bubble floatation of minerals, chemical stirring and in other applications that require mixing of gas and liquid phases [2,3].

As shown in Figure 1 and Figure 2, PLJR systems can be divided into three categories: (a) unconfined, (b) confined and (c) confined PLJR systems incorporating an annular riser [2,4]. The first two categories have been in use for decades in different applications. The third category, however, was first introduced by Al-Anzi [2] to aerate and mix more fresh water than that aerated by PLJR technologies, at almost no additional cost.

Al-Anzi has been studying PLJR systems since 2004, and recently, with his counterparts at MIT, introduced to its numerous applications a new dimension: the use of PJLR as an outfall or a dispenser for high saline, rejected brine effluent from desalination plants in Kuwait [4,5,6]. The nature of PLJRs enables the rejected brine to be safely discharged into the ambient seawater in an optimum manner that promotes aeration and dilution, simultaneously, at a lower cost. This will increase the dissolved oxygen (DO) concentration levels in the seawater and mix the receiving pool water vigorously to hinder stratification of dense brine layers, thus preserving the flora and fauna of the region, especially those of the shoreline, which is the most vulnerable part of seawater.

PLJRs are efficient and cost-effective due to their multiple advantages over other conventional technologies (e.g. surface and diffused aerators). PLJR is the only technique that requires no air blower/compressors nor any external agitation device. It achieves high oxygen dissolution and rigorous mixing of the surrounding liquid content at very minimal cost [2]. It presents minimal operational challenges and operates free from clogging because all CPLJR parts are connected above the water level. It does not demand heavy maintenance nor high operational costs.

When a falling jet impinges on a liquid pool, descending through a distance L_j (jet length), at a velocity V_L (jet velocity at the impingement), it carries the potential to plunge deeper into the liquid pool until depth H_p (penetration depth), and entrain air bubbles into the liquid phase [5]. Depending on the system’s operating conditions and the liquid’s characteristics, such as density and depth of the receiving pool, the penetration depth of the bubbles is determined. In the case of shallow water, entrained bubbles may reach the bottom and spread radially in an outwards direction. This will then be followed by the rise of the larger/coalesced bubbles to the liquid’s surface [5]. For unconfined PLJR, entrainment of air will occur only if the jet’s velocity is greater than the critical onset velocity, which is often reported to be in the vicinity of 1.5–2.5 m/s [7,8,9] under specific operating conditions. As defined by Bin [10], the critical onset velocity, which Bin referred to as the minimum entrainment velocity V_e, is “the threshold value of the jet velocity at which gas entrainment commences”. Bin also mentioned that “V_e is well defined for coherent viscous laminar jets and is far more ambiguous for turbulent jets”. This critical velocity is a function of many main parameters such as fluid properties, turbulence characteristics and jet angle [7]. The other main variables that are of importance to air entrainment rate (Q_a) are jet velocity (V_L), jet length (L_j), nozzle diameter (d_n), downcomer submergence depth (H_c) and downcomer diameter (D_c).

Many authors have investigated the effect of main parameters such as jet velocity, jet length, submergence depth, penetration depth, jet turbulence, and the effect of nozzle design and downcomer diameter on air entrainment [3,4,7,10,11,12,13,14] and dilution rates [5,6]. A comprehensive review of the research on entrainment of air by plunging jets until 1993 can be found in Bin’s work [10]. The mechanism involved in the air entrainment process was detailed in a study carried out by McKeogh and Ervine [15]. Schmidtke et al. [16] identified different regions of air entrainment (no entrainment, intermittent and continuous entrainment regimes) in similar processes. Air entrainment mechanisms have also been studied by a number of authors with the aid of computational fluid dynamics [17,18,19,20].

Despite the long history of PLJR, dating back to the late 1960s–early 1970s, none of the previous works have presented any method to simultaneously measure air disentrainment rate (Q_ads) and air net entrainment rate (Q_anet) experimentally.

When a jet impinges into a water body, it carries the potential to plunge deeper into the liquid pool and entrain air bubbles from the surrounding headspace into the liquid phase [5]. As the jet velocity impinges on the receiving pool, the entrained air will be broken into a downflow of primary/fine bubbles surrounded by an upflow of larger, coalesced bubbles. Some of the initially entrained bubbles ascend to the water’s surface and into the headspace, going unmeasured. This air is referred to as disentrained air flowrate (Q_ads); the measurement of this air is the subject of this publication. At low jet velocities and short jet lengths, all of the entrained air becomes disentrained, since the jet’s momentum is not powerful enough to carry the bubbles in a downward direction and out of the base of the downcomer. This is due to the superficial velocity of the liquid being lower than the terminal velocity of the bubbles (0.25 m/s) [21].

As the jet velocity increases for fixed operating conditions, the liquid momentum will also increase until the liquid superficial velocity inside the downcomer or the liquid jet velocity is strong enough to push the bubbles out of the base of the downcomer, resulting in net entrained air (Q_anet). In this case, the total entrained air (Q_aT) = net entrained air (Q_anet), since the disentrained air is counterbalanced by the system (whatever is disentrained is re-entrained by the same jet). For unconfined PLJR with a given jet geometry, smooth jets of a viscous liquid will entrain air when the jet velocity exceeds the same critical value (V_e) as defined previously.

The only reference that proposes a technique to measure Q_ads, Q_anet and total air entrainment rate (Q_aT) simultaneously is the recent US patent by Al-Anzi [22], the author of several papers on CPLJR since 2006 and the main author of the current study. The effect of Q_ads on net air entrainment rate has been discussed in the literature for different downcomer lengths and jet velocities [23]; however, none of them has proposed a method to accurately measure different Q_as (Q_anet, Q_ads and Q_aT) experimentally at the same time, until the patent by Al-Anzi [22].

The aim of the current study is to measure Q_ads and Q_aT experimentally and simultaneously for the first time ever, utilizing a CPLJR apparatus with a novel downcomer incorporating an Al-Anzi disentrainment ring (ADR) device. This device is discussed further in the methodology section. Measuring Q_ads accurately is essential to the PLJR concept and of interest to those who work in this field since it is strongly connected to the amount of air escaping from the bottom of the downcomer to the surroundings, known as Q_anet, and the total air entrainment of the system (Q_aT). This, ultimately, will affect the entire performance of CPLJR as an aerator and mixer. The effect of the novel ADR downcomer on Q_anet, Q_ads and Q_aT is examined in the current study. Furthermore, the effect of a range of main variables such as jet velocity at the impingement point (V_L) and L_j on Q_ads, Q_anet, bubble penetration depth (H_p) and liquid rise height (H_R) are also investigated in the current study. Other new variables pertinent to the novel ADR, such as distance from the end of ADR to the receiving pool (d_s) and ADR length (l_ADR), are introduced as main variables, and their effect on Q_ads, Q_anet and Q_aT are investigated in this study. Finally, Q_anet measured by a conventional downcomer is compared to that measured by a downcomer incorporating the ADR device for a range of d_s and l_ADR.

2. Materials and Methods

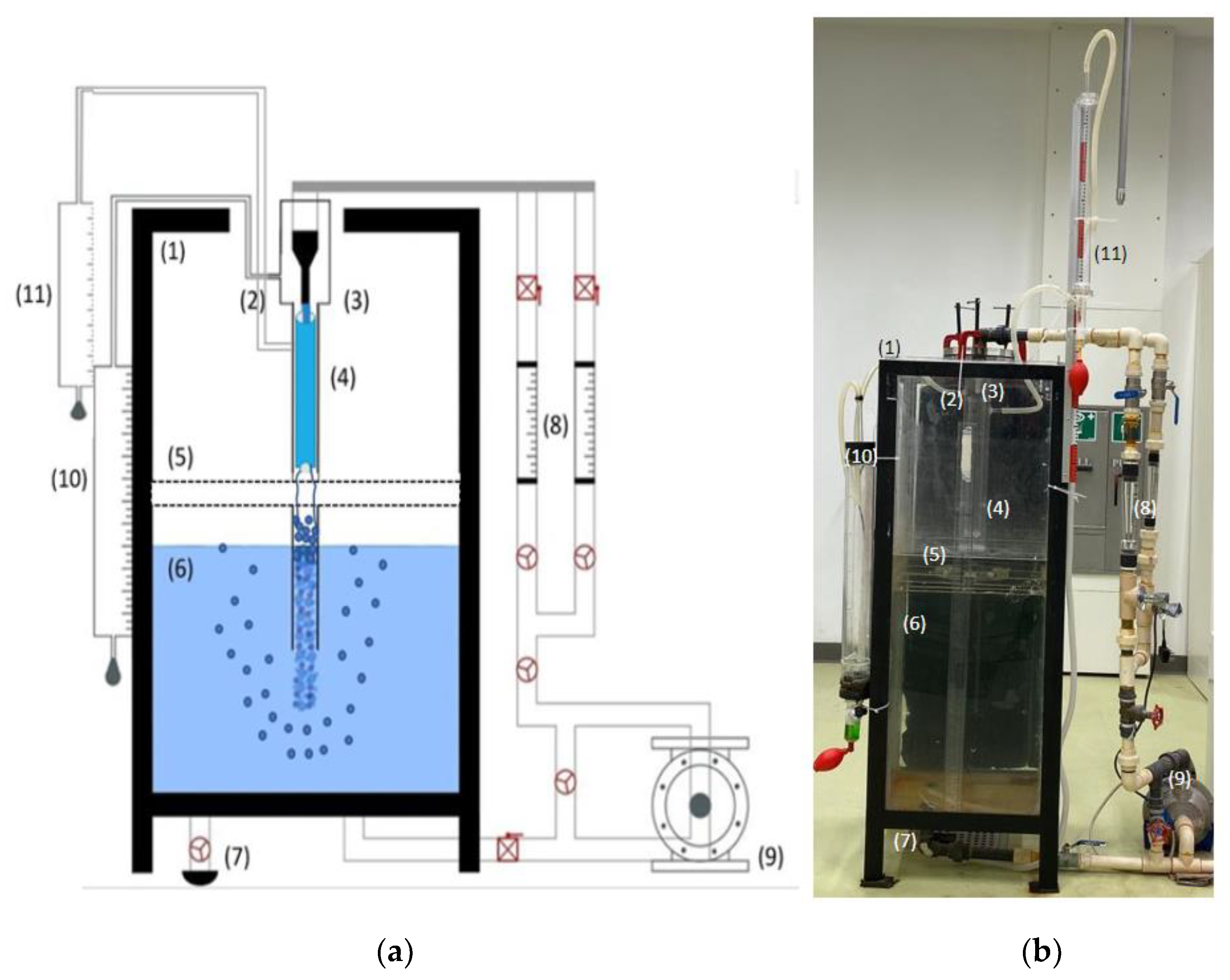
2.1. Confined Plunging Liquid Jet Reactor System

The CPLJR apparatus—similar to that used by the same author earlier—used to generate experimental results is shown in Figure 3 [4]. Water was withdrawn from the base of a reservoir (1.5 × 0.5 × 0.5 m³) by a centrifugal pump, to be recycled through rotameters and a nozzle located at the top of the same reservoir to form a water jet. Two rotameters were used to measure a maximum liquid flowrate of 60 LPM. The nozzle was designed with a length to diameter ratio of 5, according to Ohkawa et al. [24] and Al-Anzi et al. [23], and with a nozzle exit diameter (d_n) of 8.1 mm. The nozzle was placed concentrically in the downcomer column, the diameter (D_c) of which was 7.4 cm, at L_j distances of 36–51 cm from the receiving water body.

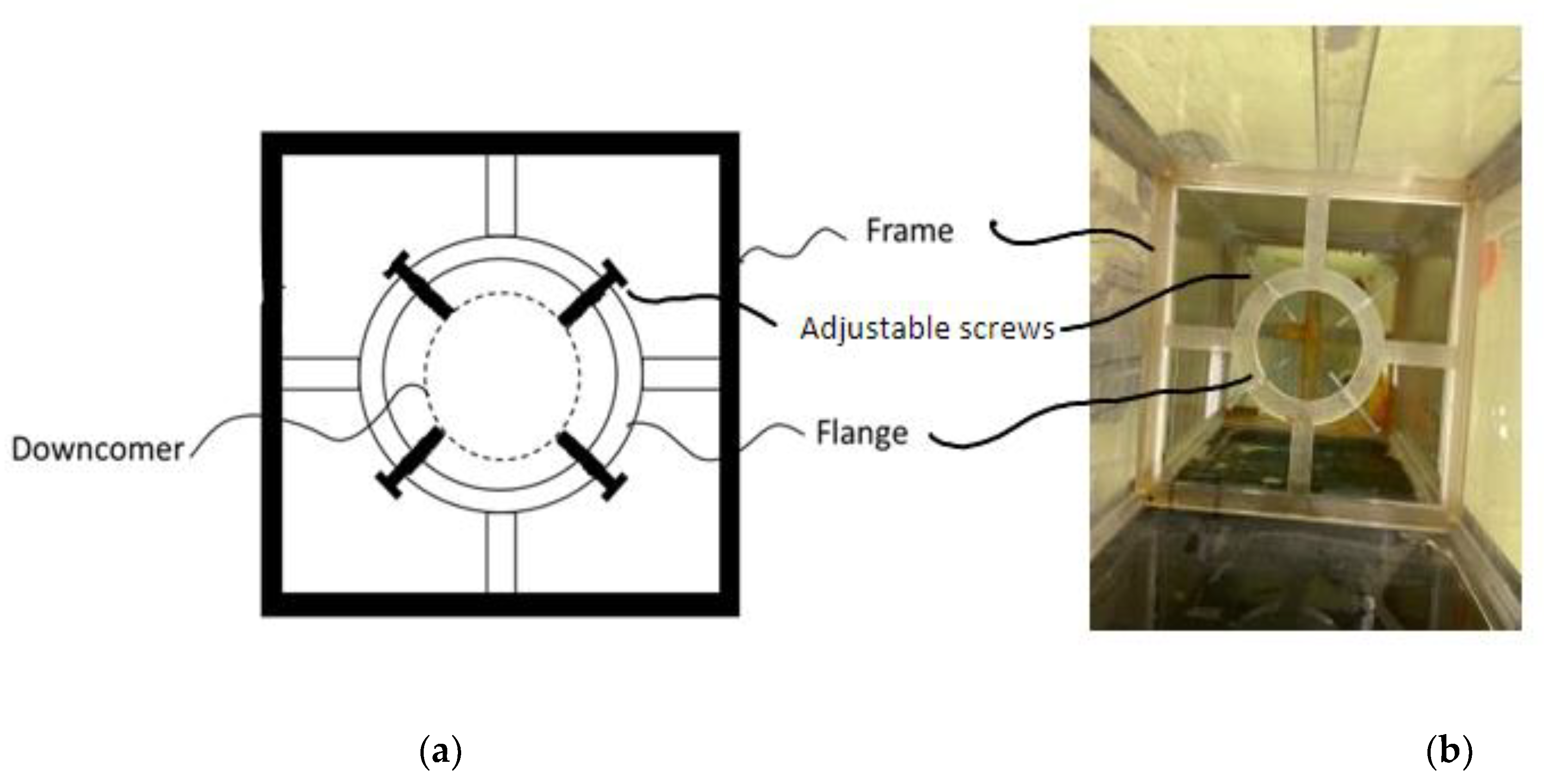
2.2. Supporting Flange/Frame

Figure 4 shows an extra external flange/frame with four adjustable screws that were spaced evenly in a radial manner. The external flange was fixed inside the tank and outside the downcomer to provide extra support for the downcomer with ADR device. The screws were adjustable; therefore, they can hold various downcomer diameters and lengths. The supporting frame/flange was rested on ledges mounted to the interior of the reservoir walls, which provided more stability to the entire system. This helped to eliminate any possible vibration and placed the nozzle concentrically inside the downcomer during the runs to ensure that the jet flowed directly into the center of the downcomer.

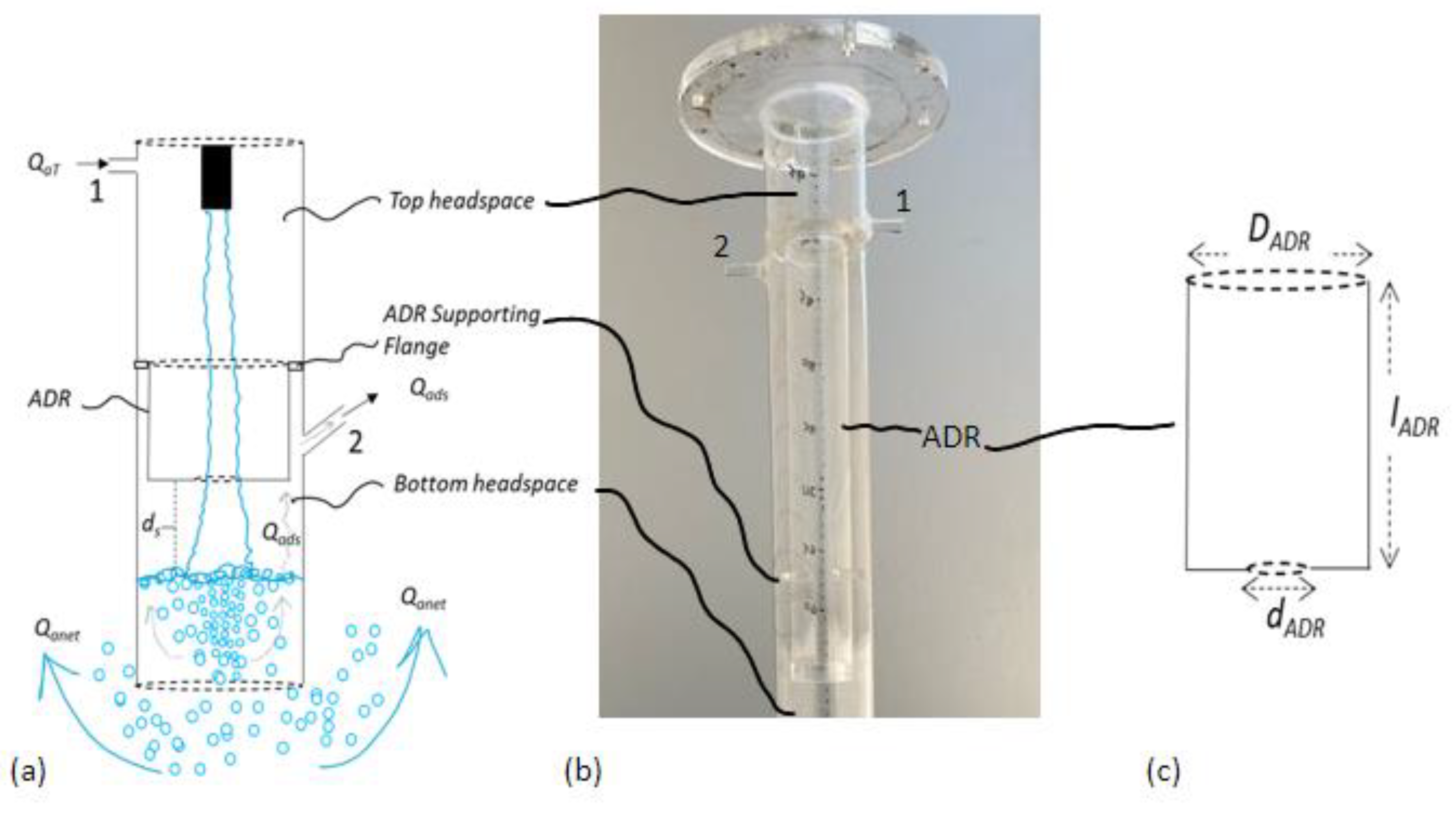
2.3. ADR Device

The other important device that was newly added to CPLJR apparatus was Al-Anzi’s disentrainment ring (ADR) device. It comprises a hollow cylindrical column with two diameters, one at each end; the larger diameter, D_ADR, is at the top, and the smaller one, d_ADR, is at the bottom. The two diameters are l_ADR apart; this figure represents the length of ADR device (Figure 5c). As shown in Figure 5b, ADR device is placed concentrically inside the CPLJR downcomer and is supported by two inner narrow ADR supporting flanges.

The purpose of the ADR device is to divide the downcomer into two headspaces (Figure 5a), top headspace above ADR and bottom headspace below ADR, which allow the initial entrained air from the top headspace to be released by the jet further down into the receiving pool below the ADR device. The released air will either leave the base of the downcomer as Q_anet or ascend inside the downcomer as Q_ads. Q_ads will be prevented from escaping back to the top headspace by ADR device, so it can be collected in the bottom headspace below the ADR. Basically, ADR prevents Q_ads from ascending back into the top headspace. Q_ads will then leave the downcomer through tapping 2 to be measured by bubble meter 2. ADR is placed at a distance d_s from the receiving water pool. Experiments were carried out with d_s lengths of 0, 1, 3, 5 and 7.5 cm, and l_ADR of 34, 35, 37, 39 and 40 cm.

2.4. Downcomer (Confining Tube)

As shown in Figure 5, the novel downcomer differs from the original one by possessing the ADR device and two tappings. The top tapping is used to allow Q_aT to enter the system, measured by bubble meter 1, and the bottom tapping is used to allow Q_ads to leave the downcomer to be measured by bubble meter 2.

The measurements of Q_aT and Q_ads (volumetric air entrainment rate) were carried out as reported by Al-Anzi [4], i.e., using a soap bubble meter. Since soap bubbles provide negligible resistance to air flow, the bubble meter was the proper device for measuring both air entrainment and disentrainment flow rates. Soap bubble meters 1 and 2 comprise, respectively, a cylindrical tube with an inner diameter of 73 mm and a length of 1000 mm, and a cylindrical tube with an inner diameter of 36 mm and a length of 500 mm, to measure total and disentrainment rates, respectively. A solution of 10% household detergent, 5% glycerin and remaining water was used to prepare the soap bubble solution.

2.5. Calculation

The following assumption was made in deriving the Q_aT formulas: all the disentrained air leaves the downcomer through tapping 2 and does not escape through ADR back into the top headspace.

The PLJR downcomers are divided into standalone (original) and novel ADR downcomers. The addition of the novel ADR changed the mass balance around the two-phase flow mixture inside the downcomer, as described below:

1. Standalone/conventional downcomer:

Figure 6a shows a schematic of the standalone downcomer, including the control volume around the two-phase flow mixture inside the downcomer. Performing a mass balance around the selected control volume produces:

$$Q_{aT}+Q_{ads}=Q_{anet}+Q_{ads}$$

(1)

Q_ads in the case of the standalone downcomer will be re-entrained by the jet, reducing Equation (1) to Equation (2):

$$Q_{aT}=Q_{anet}$$

(2)

where the initial/total air entrainment rate, Q_aT, is equal to the net entrainment rate, Q_anet (amount of the bubbles leaving the base of the downcomer).

At low jet velocities where no bubbles are leaving the base of the downcomer, Q_aT, in this case, is equal to zero, because Q_anet = 0:

$$Q_{aT}=Q_{anet}=0$$

(3)

2. Novel ADR downcomer:

The addition of the ADR device prevented Q_ads from being re-entrained by the jet (Figure 6b), and Equation (1) becomes:

$$Q_{aT}=Q_{anet}+Q_{ads}$$

(4)

At low jet velocities, Q_anet = 0, Equation (4) is simplified to:

$$Q_{aT}=Q_{ads}$$

(5)

At high jet velocities, when the bubbles leave the base of the downcomer, Equation (4) remains the same.

3. Results and Discussion

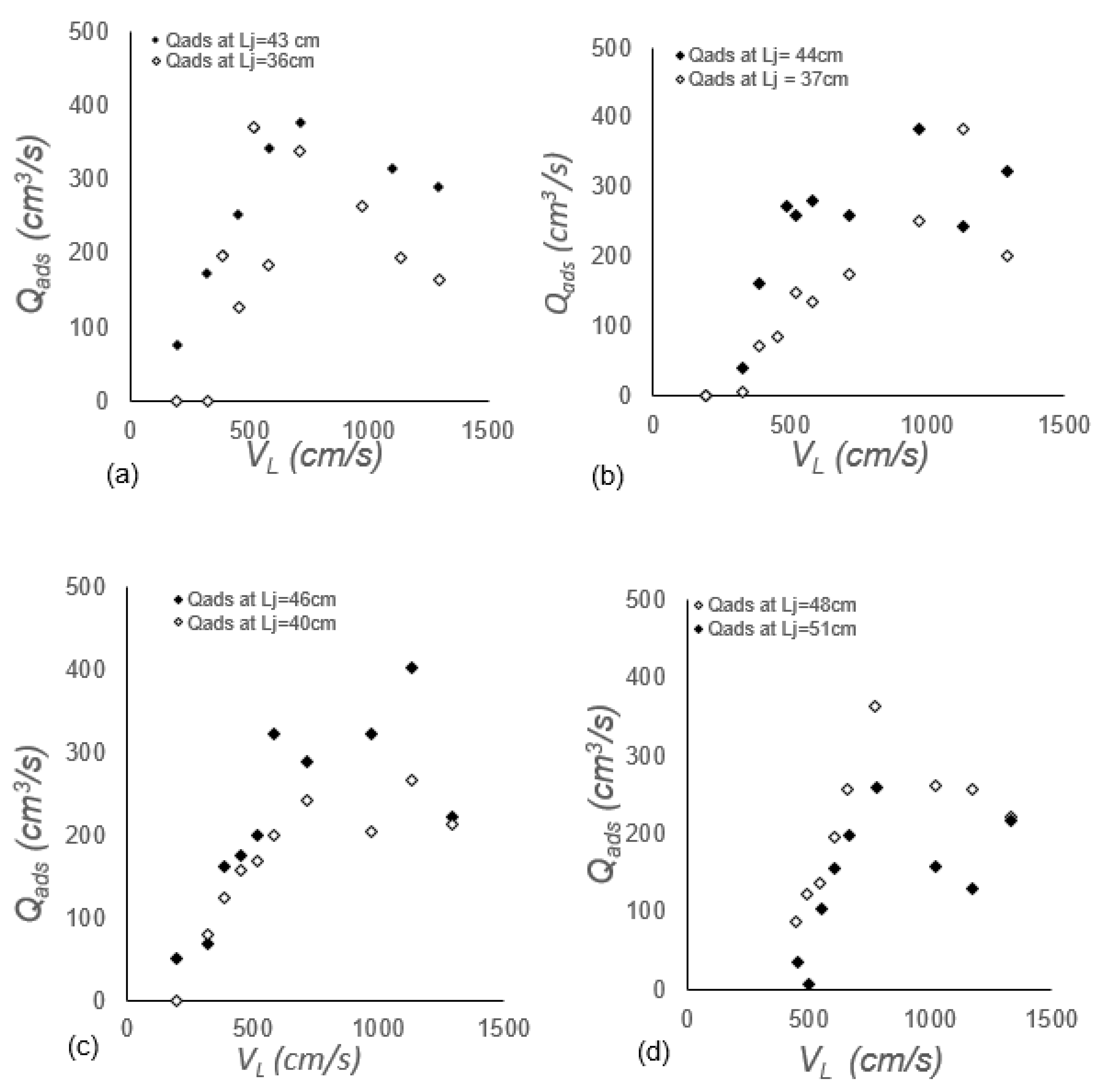
3.1. Effect of Distance between ADR Device and Receiving Pool Surface on the Air Entrainment and Disentrainment Rates

As shown in Figure 5a, d_s is the distance between the end of the ADR device and the receiving pool’s surface. Figure 7 and Figure 8 show the effect of d_s on Q_aT and Q_ads whilst keeping other main variables constant (D_c, L_j, d_ADR, H_c and l_ADR). The results obtained in this study show that Q_aT increases linearly with V_L for all d_s values with the shortest d_s = 0 measuring the highest Q_aT values for all V_L values. The high values recorded by the shortest distance, d_s, could be attributed to the ADR hindering the formation of eddies that contributed in increasing Q_ads, as reported by Al-Anzi et al. [23]. As d_s increased, another liquid jet is developed below the ADR that behaved in a similar manner to the original jet, which enhanced the Q_ads phenomenon in the second headspace (below the ADR). These results can be divided into two groups for V_L values lesser and greater than V_Lnet ≈651 cm/s, where V_Lnet is the liquid jet velocity at the impingement point that is high enough to push the first bubble/bubbles out of the base of the downcomer to record the smallest Q_anet. Group 1 represents measured Q_aT for V_L values less than V_Lnet and group 2 includes Q_aT values generated by V_L greater than V_Lnet. In group 2, the Q_aT values (shaded area) were higher than that of group 1, because Q_aT is equal to both Q_ads and Q_anet; however, this was not the case for the set of results in group 1 where Q_aT is equal to Q_ads (no Q_anet) for all of d_s values.

However, the effect of d_s on Q_ads was different from Q_anet, particularly at higher V_L (>V_Lnet), where Q_ads increased with V_L until it reached maximum, and decreased thereafter (Figure 8). This is consistent for all d_s values. The reason for the decrease of Q_ads at high V_L was that the superficial velocity of the liquid in the downcomer was strong enough to carry most of entrained bubbles downwards out of the downcomer and, hence, the net entrainment rate (Q_anet) increased at the cost of Q_ads.

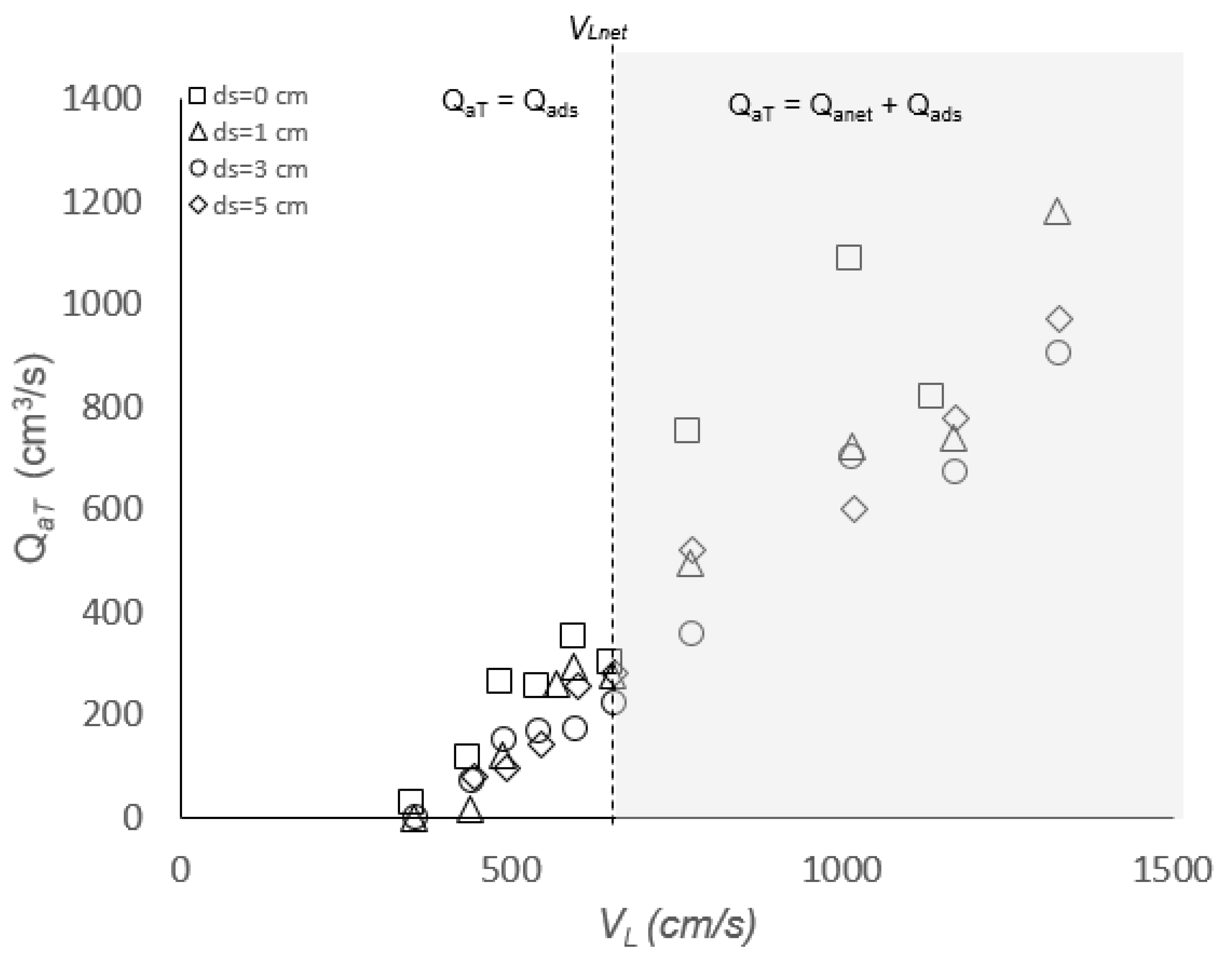
3.2. Effect of V_L on Air Disentrainment and Entrainment Rate Measurements

Figure 9 shows four representative sets of data for an 8.1 mm nozzle diameter, 36–51 cm jet length and 74 mm downcomer diameter, corresponding to H_c of 43–46 cm. All sets of results showed 2 distinct regions of Q_aT for V_L values lesser and greater than V_Lnet (651 cm/s) for all d_s values. For V_L less than V_Lnet, Q_aT = Q_ads because all of the entrained bubbles rose back inside the downcomer to be measured by disentrainment bubble meter 2. As V_L increased further beyond V_Lnet, the liquid’s superficial velocity (momentum) was sufficient to push the bubbles downward out of the base of the downcomer to increase Q_anet. This led to increased Q_aT in this region, since it is equal to the sum of both Q_ads and Q_anet. These findings are important when designing CPLJR, because they determine when the system is efficient as an aerator and mixer, thus helping in designing an efficient and feasible system.

3.3. Effect of Jet Length on Air Disentrainment and Total Entrainment Rate Measurements

L_j is one of the important variables in PLJR systems and its effect on Q_anet for a standalone downcomer has been investigated previously, by many authors, for confined and unconfined PLJR systems. Previous results in the literature showed that Q_anet increases with L_j as long as the liquid jet is coherent and less than the break-up jet under the same operating conditions. This is because longer jets tend to increase the amplitude of disturbances on the surface of the rough jet [23] and increase the value of V_j to V_L at the impingement point, since $V_L=\sqrt{V_j^2+2g{L}_j}$, which is significant at low V_j [25]. The effect of L_j on Q_ads is depicted in Figure 10a–d for d_s = 1–5 cm. All sets of data showed that Q_ads exhibited a similar trend as that described in the previous Section 3.2, with longer L_j measuring higher Q_ads, as expected. The current results confirmed the previous findings, in Section 3.2, of two distinct regions in each set of data at about V_Lnet (Q_ads increased for V_L < V_Lnet and Q_ads decreased for V_L > V_Lnet). Furthermore, the same results also showed that Q_ads is independent of d_s (almost the same range of Q_ads for all d_s).

Q_aT, however, increased linearly with L_j for all V_L values. This increase is more evident in the second region (V_L > V_Lnet), as shown by the sets of data plotted in Figure 11a–d. The effect of L_j on Q_aT in the current study is small because the difference between the two jets is small (∆Lj), with longer jets measuring slightly higher Q_aT.

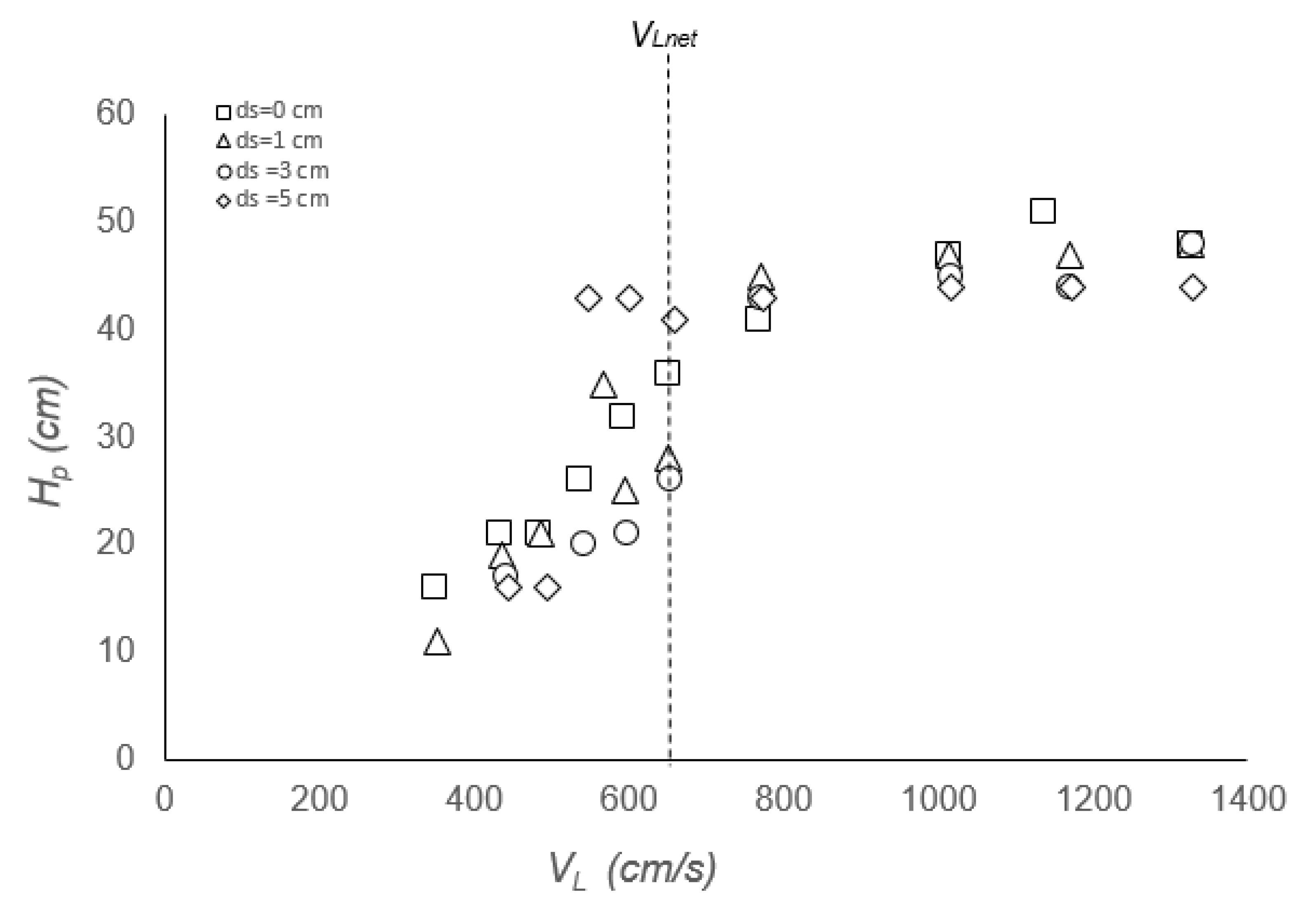
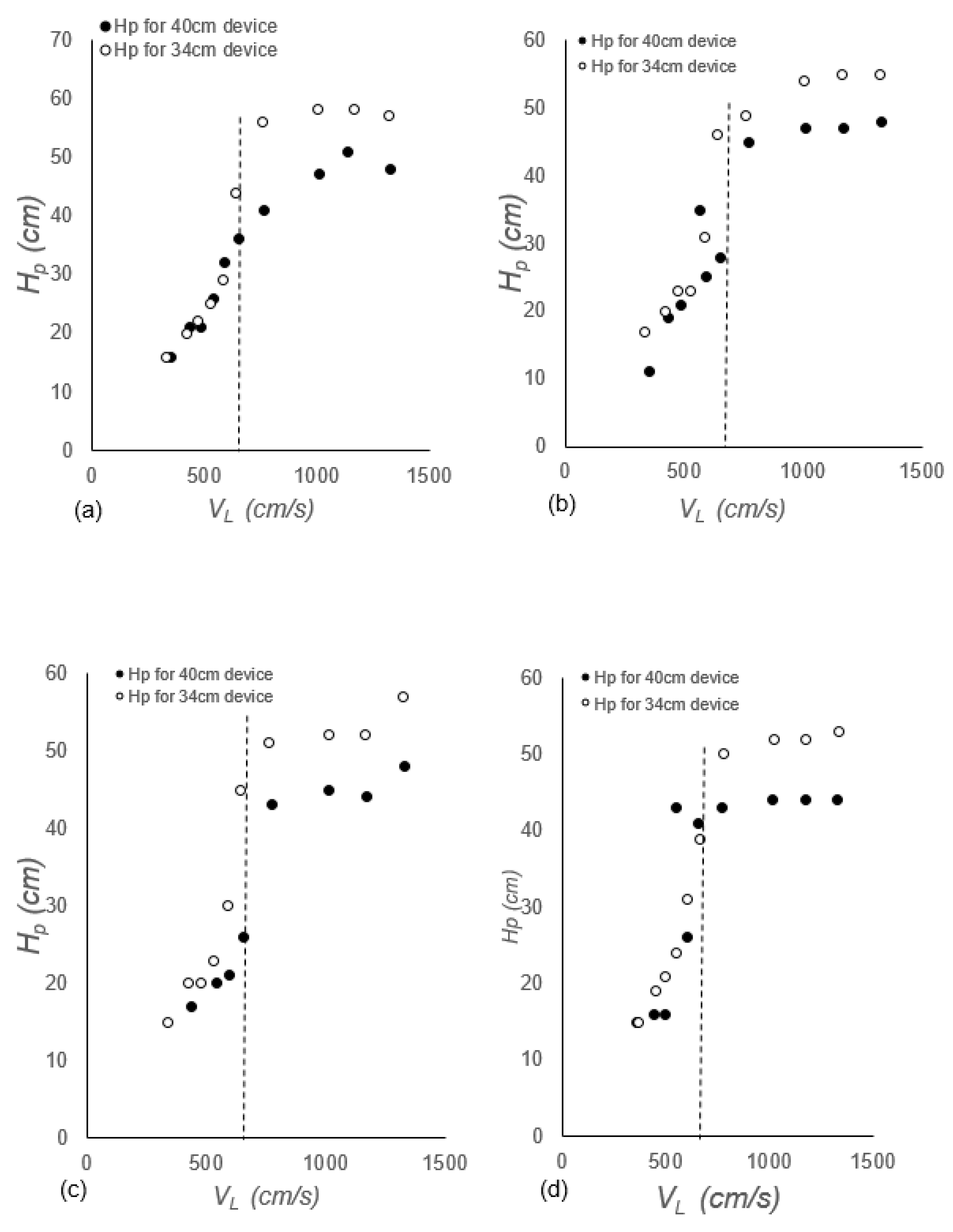
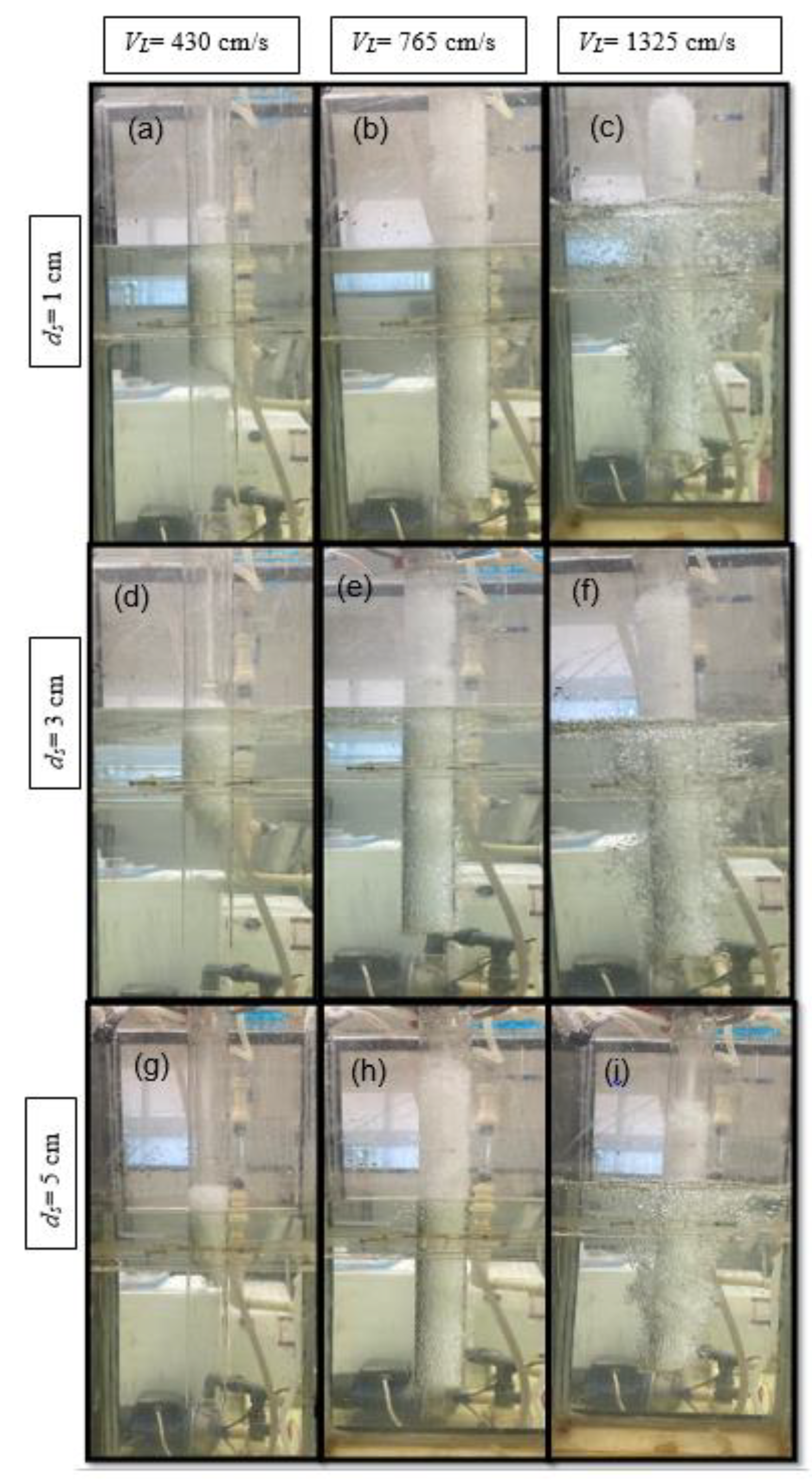
3.4. Effect of d_s and l_ADR on Bubble Penetration Depths (H_p)

One of the aims of using confined plunging jets is achieving greater bubble penetration depth to augment dissolved oxygen (DO) and provide better mixing. Figure 12 showed that H_p increased linearly with a sharp slope in region 1 (V_L < V_Lnet), whilst in region 2 (V_L > V_Lnet), the penetration depth almost remained constant for the rest of V_L values forming an “S” trend. The penetration depth, H_p, decreased with d_s. This is because short d_s hinders the formation of recirculating eddies responsible for the enhancement of Q_ads; thus, Q_ads is reduced, forcing most of the bubbles to leave the base of the downcomer as Q_anet. This, in turn, led to greater bubble penetration depths. This is under the assumption that there is no momentum loss by the jet, for all d_s values, as it passes through the ADR device.

The effect of two ADR devices (l_ADR = 34 and 40 cm) on H_p, for all d_s values, has also been investigated in this study. The results are plotted in Figure 13a–d. One can clearly see the “S” trend displayed consistently in all figures for the two l_ADR values and four d_s values (0, 1, 3 and 5 cm). This confirms the “S” shape, as discussed in the previous section (Figure 12). The effect of l_ADR is negligible at low jet velocity (region 1), particularly for short d_s values; however, at high V_L (region 2), the difference became visible, with higher H_p obtained for longer l_ADR. Again, higher l_ADR and d_s values mean shorter H_c, and, thus, low Q_ads inside the downcomer. This meant that most of the bubbles left the base of the downcomer as Q_anet and a small portion rose inside the downcomer as Q_ads, reducing the resistance of the counter current flow and achieving higher H_p.

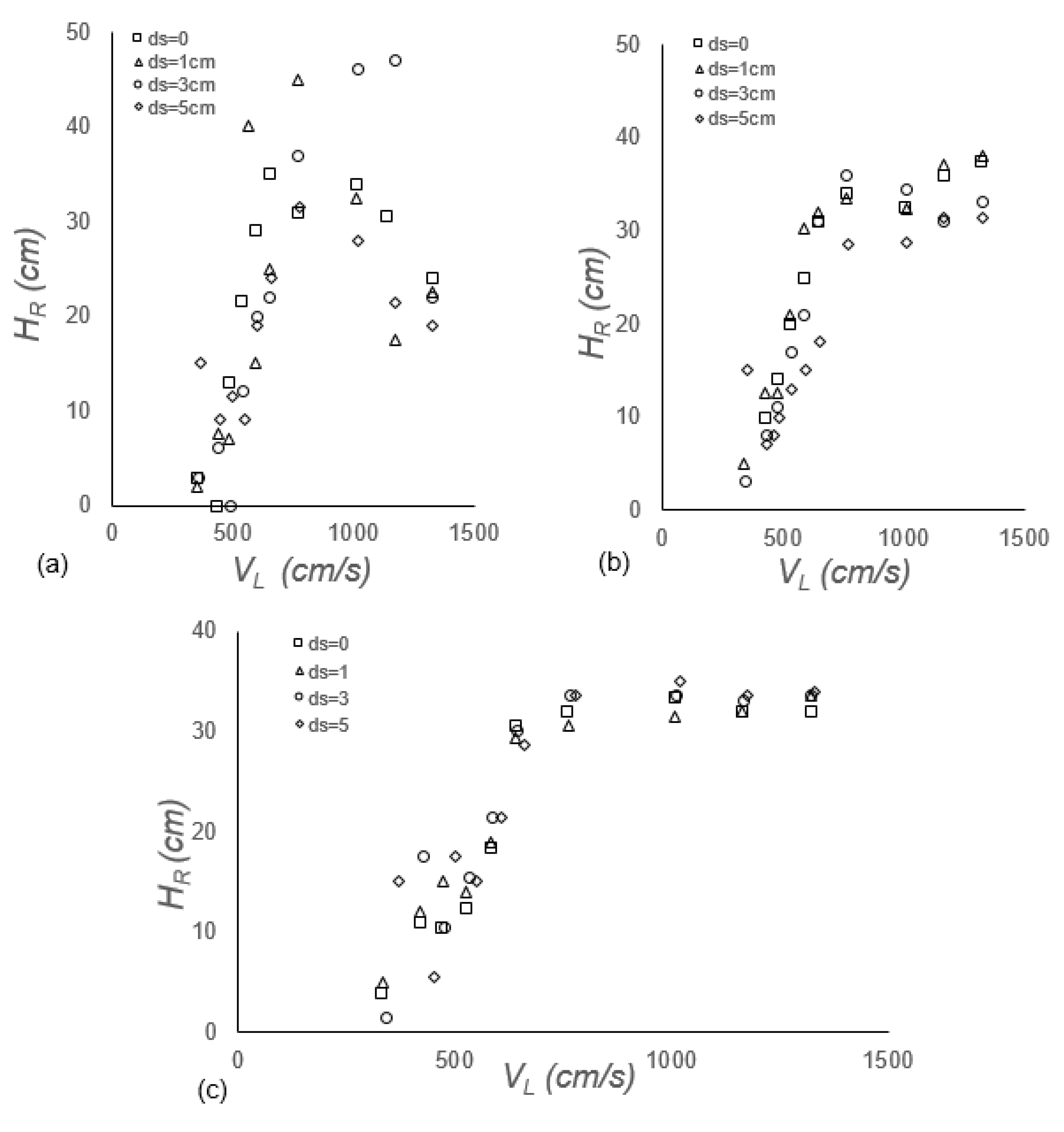
3.5. Effect of Rise Heights (H_R) for d_s Values

The H_R trends corresponded to those of H_p, except for the longest l_ADR of 40 cm, where H_R increased linearly until it reached a maximum and then decreased for all d_s values. Decreasing H_R is a good sign as it indicates that more bubbles are penetrating through the downcomer to leave as Q_anet. The effect of d_s on H_R was small for the majority of the data (Figure 14a–c). It is still premature to fully understand the reason for such phenomena, since there were several factors/variables simultaneously contributing to the results. For example, high H_R reduced the original L_j of the system which, in turn, reduced V_L and, hence, the Q_aT. This affected the physics of the entire system. Having said that, we have made significant progress in understanding PLJR as an aerator and efficient mixer. Figure 15a–i is the actual impression of the novel ADR downcomers with l_ADR = 37 cm, showing H_R and H_p levels for a range of d_s and V_L.

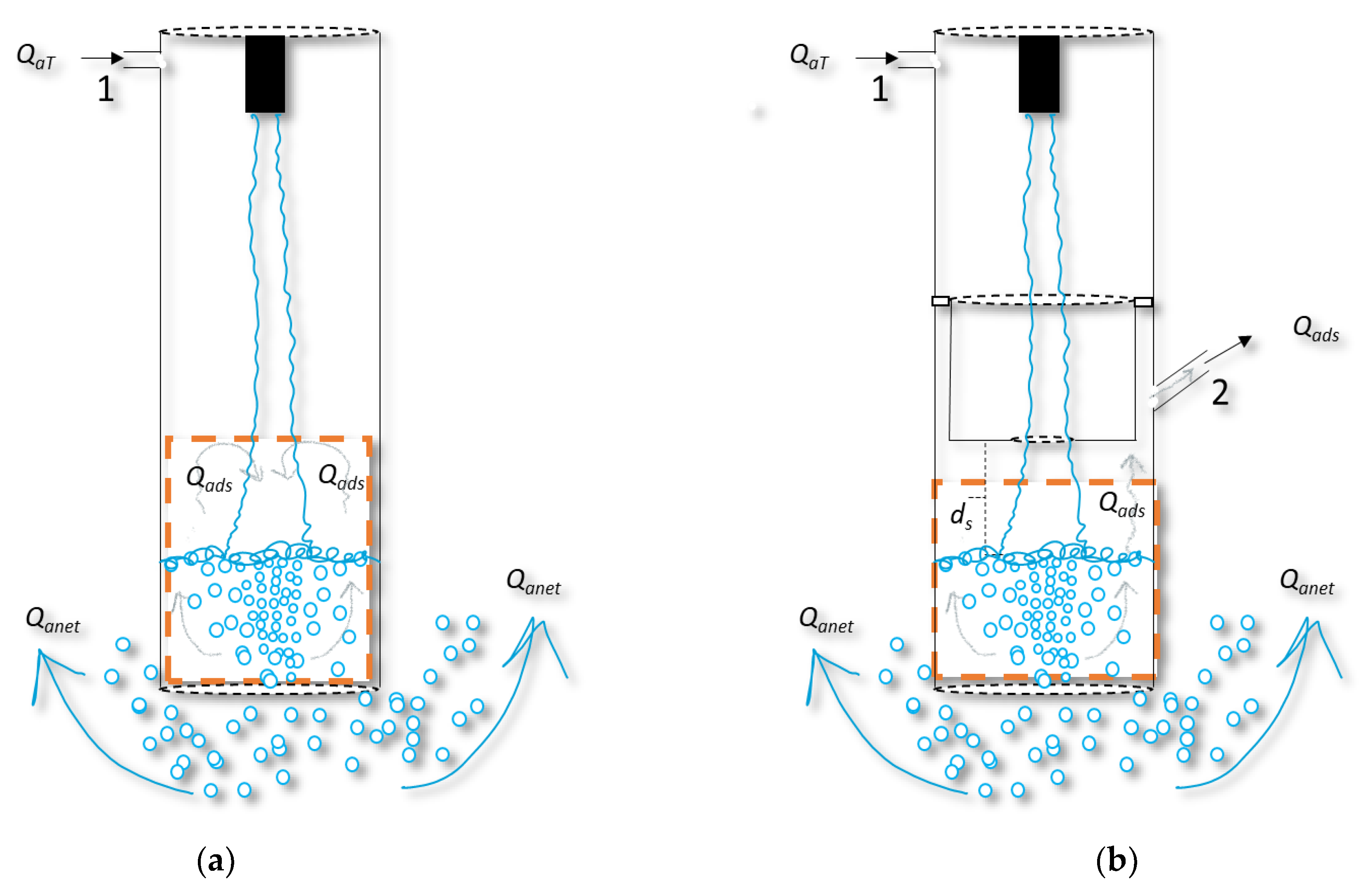
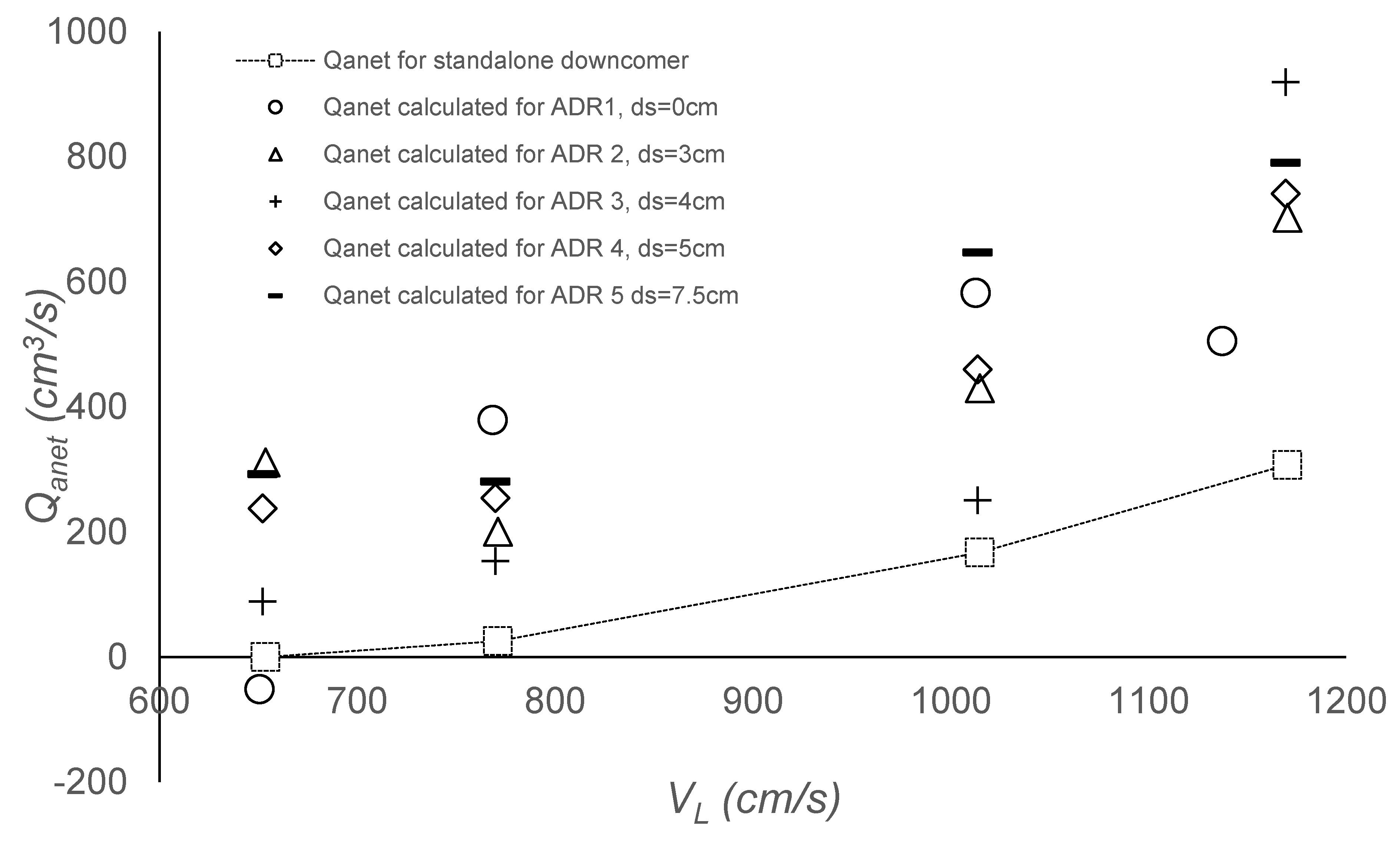
3.6. Comparison of the Effect of the Novel Downcomer (with ADR Device) and a Standalone Downcomer on Q_anet

Figure 16 is generated to show the effect of the novel ADR downcomer and standalone downcomer on the Q_anet. The same operating conditions were used for both downcomers (D_c = 7.4 cm, L_j = 45 cm, d_n = 8.1 mm, H_c = 46 cm and l_ADR = 40 cm, 39 cm, 37 cm and 35 cm) throughout the experiments. Q_anet for the standalone downcomer was measured experimentally by bubble meter 1, whereas Q_anet for the novel ADR downcomer was estimated from Equation (4) by subtracting the experimental values of Q_aT from Q_ads measured by bubble meters 1 and 2, respectively. In total, five d_s values from 0 to 7.5 cm were used for the ADR downcomer in this experiment. Both downcomers demonstrated increasing trends for Q_anet, with V_L that fit quadratic behavior: R² ranged from 0.94–1.0. The results obtained consistently showed that the ADR device enhanced Q_anet significantly for all d_s values in comparison with the results of the standalone downcomer. It is noteworthy and interesting that the ADR device actually increased the Q_anet significantly. For example, at low V_L (654 cm/s, corresponding to the V_Lnet), Q_anet increased from 0 for the standalone downcomer to a relative maximum of 477 cm³/s for the novel ADR downcomer with d_s = 4 cm. At higher V_L, Q_anet increased by 2.5-fold, with d_s = 5 cm (from 458 cm³/s for the standalone downcomer to an absolute maximum of 1143 cm³/s for the novel ADR downcomer). If it stands, this could be revolutionary in the field of CPLJR aeration. Clearly, more investigation under a wide range of operating conditions is required to confirm such important findings.

4. Conclusions

The current work is entirely novel from the perspective of idea generation, manufacturing, implementation and results. A novel ADR device with various dimensions was manufactured locally and successfully affixed inside CPLJR downcomers to measure air disentrainment rates experimentally and accurately, for the first time ever, for a range of new and old operating conditions. New variables came with the ADR, such as length of the ADR (l_ADR) and the distance (d_s) of the ADR to the receiving pool. A new jet velocity value at the impingement point (V_Lnet) was defined as the minimum jet velocity for Q_anet to be measured. The difference between Q_aT and Q_ads was negligible for V_L < V_Lnet; however, this difference became substantial for V_L > V_Lnet under the same operating conditions. Net air entrainment rate (Q_anet) increased linearly with V_L and L_j for all d_s values, with the shortest d_s (zero) measuring the highest Q_anet; however, Q_ads exhibited different behavior for V_L > V_Lnet, where it decreased after reaching an absolute maximum for all d_s values. Bubble penetration depths (H_p) and liquid rise height (H_R) increased for all V_L until they reached maximum values and leveled off for the same l_ADR. Results also showed that shorter l_ADR produced higher H_p values; however, the measured H_R values were lower under the same operating conditions.

One of the great contributions of this work is the substantial increase in Q_anet measured by the novel ADR downcomer in comparison with the conventional standalone downcomer (2.5 to 15 times increase in Q_anet), at no extra cost. This has been confirmed over a range of d_s values (0 to 7.5 cm). Despite the significant progress we made in understanding PLJR as an aerator and efficient mixer, it is still premature to fully understand the reason for such phenomena since there were several factors/variables contributing to the results simultaneously. Thus, more studies under a wide range of operating conditions are required to confirm such important findings. This, in addition to the application of new primary variables and designs pertinent to the novel ADR device, will constitute the focus of our future work.