A Review of HYDRUS 2D/3D Applications for Simulations of Water Dynamics, Root Uptake and Solute Transport in Tree Crops under Drip Irrigation
Abstract
1. Introduction
2. HYDRUS 2D/3D Software
3. Citrus Trees
3.1. HYDRUS 2D/3D Model Performance in Citrus Trees
3.1.1. Findings Regarding the Design of Drip Irrigation Systems
3.1.2. Findings Regarding Irrigation and Fertigation Strategies
3.2. Summary of HYDRUS 2D/3D Application in Citrus Trees
| Research Report | Crop | Soil Type | Irrigation System 1 | Irrigation Treatment 2 | HYDRUS 2D/3D | Simulation Processes |
|---|---|---|---|---|---|---|
| Phogat et al. [11] | orange (lysimeter) | (0–60 cm soil depth): loamy sand | DI -on a circle and fertigation | Ip vs. Ic | 2D | Water flow Root uptake Nitrate dynamics |
| (60–85 cm soil depth): sandy Loam | ||||||
| (85–110 cm soil depth): sand | ||||||
| Phogat et al. [57] | mandarin | (0–90 cm soil depth): sandy loam | DI -2 drip lines and fertigation | different schedules | 2D | Water flow Root uptake Salinity and Nitrate dynamics |
| (90–150 cm soil depth): loam | ||||||
| Panigrahi and Sharma [55] | mandarin | (0–40 cm soil depth): sandy loam | SubDI vs. DI | - | 2D | Water flow Root uptake |
| (40–100 cm soil depth): sandy clay loam | ||||||
| Phogat et al. [58] | mandarin | (0–90 cm soil depth): sandy loam | DI-2 drip lines and fertigation | - | 2D | Water flow Root uptake Salinity and Nitrate dynamics |
| (90–150 cm soil depth): loam |
4. Olive Trees
4.1. HYDRUS 2D/3D Model Performance in Olive Trees
4.1.1. Findings Regarding the Design of Drip Irrigation Systems
4.1.2. Findings Regarding Irrigation Strategies
4.2. Summary of HYDRUS 2D/3D Application in Olive Trees
| Research Report | Crop | Soil Type | Irrigation System 1 | Irrigation Treatment 2 | HYDRUS 2D/3D | Simulation Processes |
|---|---|---|---|---|---|---|
| Egea et al. [67] | olive arbequina | (soil depth 0–40): sandy loam | DI-1 vs. 2 drip lines | FI: 100% ETc | 2D | Water flow Root uptake |
| (soil depth 40–100): sandy clay | RDI: | |||||
| Autovino et al. [22] | olive nocellara del Belice | (homogenous soil depth): silty clay loam | DI-1 drip line vs. total surface irrig. | FI: 100% ETc | 2D | Water flow Root uptake |
| RDI: 50% ETc | ||||||
| Fernandes et al. [68] | olive arbequina | (soil depth 0–60): sandy | DI-1 vs. 2 drip lines | FI: 100% Etc | 2D | Water flow Root uptake |
| SDI: 45% Etc | ||||||
| RDI: 45% Etc | ||||||
| Carlos et al. [69] | olive | not listed | DI-2 drip lines | - | 2D | Water flow Root uptake |
5. Avocado Trees
5.1. HYDRUS 2D/3D Model Performance in Avocado Trees
5.1.1. Findings Regarding the Design of Drip Irrigation Systems
5.1.2. Findings Regarding Irrigation Strategies
5.2. Summary of HYDRUS 2D/3D Application in Avocado Trees
| Research Report | Crop | Soil Type | Irrigation System 1 | Irrigation Treatment 2 | HYDRUS 2D/3D | Simulation Processes |
|---|---|---|---|---|---|---|
| Assouline and Narkis [75] | avocado | (homogenous soil depth): clay | 2 drip lines; 2.0 L/h | FW | 2D | Water flow Root uptake |
| WW | ||||||
| Assouline et al. [76] | avocado | (homogenous soil depth): clay | 2 drip lines; 48 h; 3.2 L/h | FW | 2D | Water flow Root uptake |
| 2 drip lines; 48 h; 1.6 L/h | ||||||
| 2 drip lines; 48 h; 0.8 L/h | 2/3 FW—1/3 WW | |||||
| 2 drip lines; 24 h; 1.6 L/h | ||||||
| 2 drip lines; 24h; 0.8 L/h | 1/3 FW—2/3 WW | |||||
| 4 drip lines; 24 h; 0.8 L/h | ||||||
| 4 drip lines; 48 h; 1.6 L/h | WW | |||||
| 4 drip lines; 48 h; 0.8 L/h |
6. Deciduous Fruit and Nut Trees
6.1. HYDRUS 2D/3D Model Performance in Deciduous Fruit Trees and Nut Trees
6.1.1. Findings Regarding the Design of Drip Irrigation Systems
6.1.2. Findings Regarding Irrigation Strategies
6.2. Summary of HYDRUS 2D/3D Application in Deciduous Fruit Trees and Nut Trees
7. Conclusions and Future Challenges
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Correction Statement
References
- Provenzano, G.; Sinobas, L.R. Special issue on trends and challenges of sustainable irrigated agriculture. J. Irrig. Drain. Eng. 2014, 140, A2014001. [Google Scholar] [CrossRef]
- FAO. FAOSTAT: Statistical Reports of Plant Production. 2021. Available online: http://www.fao.org/faostat/en/#data/QC (accessed on 27 January 2022).
- Hamilton, A.J.; Stagnitti, F.; Xiong, X.; Kreidl, S.L.; Benke, K.K.; Maher, P. Wastewater irrigation: The state of play. Vadose Zone J. 2007, 6, 823–840. [Google Scholar] [CrossRef]
- Assouline, S.; Narkis, K. Effects of long-term irrigation with treated wastewater on the root zone environment. Vadose Zone J. 2013, 12, 1–10. [Google Scholar] [CrossRef]
- Morianou, G.; Ziogas, V.; Kourgialas, N.; Karatzas, G. Effect of irrigation practices upon yield and fruit quality of four grapefruit (Citrus paradisi Mac.) cultivars. Water Supply 2021, 21, 2735–2747. [Google Scholar] [CrossRef]
- Gärdenäs, A.; Hopmans, J.; Hanson, B.; Šimůnek, J. Two-dimensional modeling of nitrate leaching for various fertigation scenarios under micro-irrigation. Agric. Water Manag. 2005, 74, 219–242. [Google Scholar] [CrossRef]
- Dağdelen, N.; Başal, H.; Yılmaz, E.; Gürbüz, T.; Akcay, S. Different drip irrigation regimes affect cotton yield, water use efficiency and fiber quality in western Turkey. Agric. Water Manag. 2009, 96, 111–120. [Google Scholar] [CrossRef]
- Subbaiah, R. A review of models for predicting soil water dynamics during trickle irrigation. Irrig. Sci. 2013, 31, 225–258. [Google Scholar] [CrossRef]
- Phogat, V.; Mahadevan, M.; Skewes, M.; Cox, J.W. Modelling soil water and salt dynamics under pulsed and continuous surface drip irrigation of almond and implications of system design. Irrig. Sci. 2012, 30, 315–333. [Google Scholar] [CrossRef]
- Kourgialas, N.N.; Koubouris, G.C.; Dokou, Z. Optimal irrigation planning for addressing current or future water scarcity in Mediterranean tree crops. Sci. Total Environ. 2019, 654, 616–632. [Google Scholar] [CrossRef]
- Phogat, V.; Skewes, M.; Cox, J.; Alam, J.; Grigson, G.; Šimůnek, J. Evaluation of water movement and nitrate dynamics in a lysimeter planted with an orange tree. Agric. Water Manag. 2013, 127, 74–84. [Google Scholar] [CrossRef]
- Arbat, G.; Puig-Bargués, J.; Duran-Ros, M.; Barragán, J.; de Cartagena, F.R. Drip-Irriwater: Computer software to simulate soil wetting patterns under surface drip irrigation. Comput. Electron. Agric. 2013, 98, 183–192. [Google Scholar] [CrossRef]
- Communar, G.; Friedman, S.P. Relative water uptake rate as a criterion for trickle irrigation system design: I. Coupled source–sink steady water flow model. Soil Sci. Soc. Am. J. 2010, 74, 1493–1508. [Google Scholar] [CrossRef]
- Cook, F.J.; Thorburn, P.J.; Fitch, P.; Bristow, K.L. WetUp: A software tool to display approximate wetting patterns from drippers. Irrig. Sci. 2003, 22, 129–134. [Google Scholar] [CrossRef]
- Singh, D.; Rajput, T.; Sikarwar, H.; Sahoo, R.; Ahmad, T. Simulation of soil wetting pattern with subsurface drip irrigation from line source. Agric. Water Manag. 2006, 83, 130–134. [Google Scholar] [CrossRef]
- van Dam, J.C.; Groenendijk, P.; Hendriks, R.F.A.; Kroes, J.G. Advances of modeling water flow in variably saturated soils with SWAP. Vadose Zone J. 2008, 7, 640–653. [Google Scholar] [CrossRef]
- Šimůnek, J.; Van Genuchten, M.T.; Šejna, M. The HYDRUS software package for simulating two-and three-dimensional movement of water, heat, and multiple solutes in variably-saturated media. Tech. Man. Version 2006, 1, 241. [Google Scholar]
- Šimůnek, J.; Van Genuchten, M.T.; Šejna, M. Recent developments and applications of the HYDRUS computer software packages. Vadose Zone Journal 2016, 15, 1–25. [Google Scholar] [CrossRef]
- Cote, C.M.; Bristow, K.L.; Charlesworth, P.B.; Cook, F.J.; Thorburn, P.J. Analysis of soil wetting and solute transport in subsurface trickle irrigation. Irrig. Sci. 2003, 22, 143–156. [Google Scholar] [CrossRef]
- Ben-Gal, A.; Lazorovitch, N.; Shani, U. Subsurface drip irrigation in gravel-filled cavities. Vadose Zone J. 2004, 3, 1407–1413. [Google Scholar] [CrossRef]
- Kandelous, M.M.; Šimůnek, J. Comparison of numerical, analytical, and empirical models to estimate wetting patterns for surface and subsurface drip irrigation. Irrig. Sci. 2010, 28, 435–444. [Google Scholar] [CrossRef]
- Autovino, D.; Rallo, G.; Provenzano, G. Predicting soil and plant water status dynamic in olive orchards under different irrigation systems with Hydrus-2D: Model performance and scenario analysis. Agric. Water Manag. 2018, 203, 225–235. [Google Scholar] [CrossRef]
- Vrugt, J.; Hopmans, J.W.; Šimůnek, J. Calibration of a two-dimensional root water uptake model. Soil Sci. Soc. Am. J. 2001, 65, 1027–1037. [Google Scholar] [CrossRef]
- Saitta, D.; Consoli, S.; Ferlito, F.; Torrisi, B.; Allegra, M.; Longo-Minnolo, G.; Ramírez-Cuesta, J.M.; Vanella, D. Adaptation of citrus orchards to deficit irrigation strategies. Agric. Water Manag. 2021, 247, 106734. [Google Scholar] [CrossRef]
- De Silva, M.; Nachabe, M.; Šimůnek, J.; Carnahan, R. Simulating root water uptake from a heterogeneous vegetative cover. J. Irrig. Drain. Eng. 2008, 134, 167–174. [Google Scholar] [CrossRef]
- Deb, S.K.; Shukla, M.K.; Šimůnek, J.; Mexal, J.G. Evaluation of spatial and temporal root water uptake patterns of a flood-irrigated pecan tree using the HYDRUS (2D/3D) model. J. Irrig. Drain. Eng. 2013, 139, 599–611. [Google Scholar] [CrossRef]
- Han, M.; Zhao, C.; Feng, G.; Yan, Y.; Sheng, Y. Evaluating the effects of mulch and irrigation amount on soil water distribution and root zone water balance using HYDRUS-2D. Water 2015, 7, 2622–2640. [Google Scholar] [CrossRef]
- Li, J.; Zhang, J.; Rao, M. Wetting patterns and nitrogen distributions as affected by fertigation strategies from a surface point source. Agric. Water Manag. 2004, 67, 89–104. [Google Scholar] [CrossRef]
- Li, J.; Zhang, J.; Rao, M. Modeling of water flow and nitrate transport under surface drip fertigation. Trans. ASAE 2005, 48, 627–637. [Google Scholar] [CrossRef]
- Hanson, B.R.; Šimůnek, J.; Hopmans, J.W. Evaluation of urea–ammonium–nitrate fertigation with drip irrigation using numerical modeling. Agric. Water Manag. 2006, 86, 102–113. [Google Scholar] [CrossRef]
- Ajdary, K.; Singh, D.; Singh, A.K.; Khanna, M. Modelling of nitrogen leaching from experimental onion field under drip fertigation. Agric. Water Manag. 2007, 89, 15–28. [Google Scholar] [CrossRef]
- Wehrhan, A.; Kasteel, R.; Simunek, J.; Groeneweg, J.; Vereecken, H. Transport of sulfadiazine in soil columns—Experiments and modelling approaches. J. Contam. Hydrol. 2007, 89, 107–135. [Google Scholar] [CrossRef]
- Engelhardt, I.; Sittig, S.; Šimůnek, J.; Groeneweg, J.; Pütz, T.; Vereecken, H. Fate of the antibiotic sulfadiazine in natural soils: Experimental and numerical investigations. J. Contam. Hydrol. 2015, 177, 30–42. [Google Scholar] [CrossRef]
- Karandish, F.; Šimůnek, J. A comparison of the HYDRUS (2D/3D) and SALTMED models to investigate the influence of various water-saving irrigation strategies on the maize water footprint. Agric. Water Manag. 2019, 213, 809–820. [Google Scholar] [CrossRef]
- Karandish, F.; Šimůnek, J. Two-dimensional modeling of nitrogen and water dynamics for various N-managed water-saving irrigation strategies using HYDRUS. Agric. Water Manag. 2017, 193, 174–190. [Google Scholar] [CrossRef]
- García Morillo, J.; Rodríguez Díaz, J.; Camacho, E.; Montesinos, P. Drip irrigation scheduling using HYDRUS 2-D numerical model application for strawberry production in South-West Spain. Irrig. Drain. 2017, 66, 797–807. [Google Scholar] [CrossRef]
- Skaggs, T.; Trout, T.; Šimůnek, J.; Shouse, P. Comparison of HYDRUS-2D simulations of drip irrigation with experimental observations. J. Irrig. Drain. Eng. 2004, 130, 304–310. [Google Scholar] [CrossRef]
- Sharmiladevi, R.; Ravikumar, V. Simulation of nitrogen fertigation schedule for drip irrigated paddy. Agric. Water Manag. 2021, 252, 106841. [Google Scholar] [CrossRef]
- Arbat, G.; Cufí, S.; Duran-Ros, M.; Pinsach, J.; Puig-Bargués, J.; Pujol, J.; Ramírez de Cartagena, F. Modeling approaches for determining dripline depth and irrigation frequency of subsurface drip irrigated rice on different soil textures. Water 2020, 12, 1724. [Google Scholar] [CrossRef]
- Grecco, K.L.; de Miranda, J.H.; Silveira, L.K.; van Genuchten, M.T. HYDRUS-2D simulations of water and potassium movement in drip irrigated tropical soil container cultivated with sugarcane. Agric. Water Manag. 2019, 221, 334–347. [Google Scholar] [CrossRef]
- Azad, N.; Behmanesh, J.; Rezaverdinejad, V.; Abbasi, F.; Navabian, M. Developing an optimization model in drip fertigation management to consider environmental issues and supply plant requirements. Agric. Water Manag. 2018, 208, 344–356. [Google Scholar] [CrossRef]
- Phogat, V.; Šimůnek, J.; Skewes, M.; Cox, J.; McCarthy, M. Improving the estimation of evaporation by the FAO-56 dual crop coefficient approach under subsurface drip irrigation. Agric. Water Manag. 2016, 178, 189–200. [Google Scholar] [CrossRef]
- Ramos, T.; Šimůnek, J.; Gonçalves, M.; Martins, J.; Prazeres, A.; Pereira, L. Two-dimensional modeling of water and nitrogen fate from sweet sorghum irrigated with fresh and blended saline waters. Agric. Water Manag. 2012, 111, 87–104. [Google Scholar] [CrossRef]
- Lazarovitch, N.; Warrick, A.; Furman, A.; Simunek, J. Subsurface water distribution from drip irrigation described by moment analyses. Vadose Zone J. 2007, 6, 116–123. [Google Scholar] [CrossRef]
- Phogat, V.; Pitt, T.; Stevens, R.; Cox, J.; Šimůnek, J.; Petrie, P. Assessing the role of rainfall redirection techniques for arresting the land degradation under drip irrigated grapevines. J. Hydrol. 2020, 587, 125000. [Google Scholar] [CrossRef]
- Williams, C.A.; Albertson, J.D. Soil moisture controls on canopy-scale water and carbon fluxes in an African savanna. Water Resour. Res. 2004, 40, 9. [Google Scholar] [CrossRef]
- Kandelous, M.M.; Šimůnek, J. Numerical simulations of water movement in a subsurface drip irrigation system under field and laboratory conditions using HYDRUS-2D. Agric. Water Manag. 2010, 97, 1070–1076. [Google Scholar] [CrossRef]
- Šimůnek, J.; van Genuchten, M.T.; Šejna, M. The HYDRUS Software Package for Simulating Two- and Three-Dimensional Movement of Water, Heat, and Multiple Solutes in Variably-Saturated Porous Media; Technical Manual, Version 3.0; PC Progress: Prague, Czech Republic, 2018; Volume 274. [Google Scholar]
- Feddes, R.A.; Kowalik, P.J.; Zaradny, H. Simulation of Field Water Use and Crop Yield. In Simulation of Plant Growth and Crop Production; Pudoc: Wageningen, The Netherlands, 1978. [Google Scholar]
- Mualem, Y. A new model for predicting the hydraulic conductivity of unsaturated porous media. Water Resour. Res. 1976, 12, 513–522. [Google Scholar] [CrossRef]
- Van Genuchten, M.T. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Lu, P.; Zhang, Z.; Sheng, Z.; Huang, M.; Zhang, Z. Assess effectiveness of salt removal by a subsurface drainage with bundled crop straws in coastal saline soil using HYDRUS-3D. Water 2019, 11, 943. [Google Scholar] [CrossRef]
- Ziogas, V.; Tanou, G.; Morianou, G.; Kourgialas, N. Drought and salinity in citriculture: Optimal practices to alleviate salinity and water stress. Agronomy 2021, 11, 1283. [Google Scholar] [CrossRef]
- Kourgialas, N.N.; Karatzas, G.P. A modeling approach for agricultural water management in citrus orchards: Cost-effective irrigation scheduling and agrochemical transport simulation. Environ. Monit. Assess. 2015, 187, 1–21. [Google Scholar] [CrossRef]
- Panigrahi, P.; Sharma, R.K. Using hydrus-2D model for simulating soil water dynamics in drip-irrigated citrus. Agric. Eng. 2016, 1, 59–68. [Google Scholar]
- Legates, D.R.; McCabe, G.J., Jr. Evaluating the use of “goodness-of-fit” measures in hydrologic and hydroclimatic model validation. Water Resour. Res. 1999, 35, 233–241. [Google Scholar] [CrossRef]
- Phogat, V.; Skewes, M.; Cox, J.; Sanderson, G.; Alam, J.; Šimůnek, J. Seasonal simulation of water, salinity and nitrate dynamics under drip irrigated mandarin (Citrus reticulata) and assessing management options for drainage and nitrate leaching. J. Hydrol. 2014, 513, 504–516. [Google Scholar] [CrossRef]
- Phogat, V.; Skewes, M.; Cox, J.; Simunek, J. Statistical assessment of a numerical model simulating agro hydro-chemical processes in soil under drip fertigated mandarin tree. Irrig. Drain. Syst. Eng. 2016, 5, 1–9. [Google Scholar]
- Tejero, I.G.; Zuazo, V.H.D.; Bocanegra, J.A.J.; Fernández, J.L.M. Improved water-use efficiency by deficit-irrigation programmes: Implications for saving water in citrus orchards. Sci. Hortic. 2011, 128, 274–282. [Google Scholar] [CrossRef]
- Doorenbos, J.; Kassam, A.H. Yield response to water. FAO Irrig. Drain. Pap. 1979, 33, 257. [Google Scholar]
- Castel, J.R.; Buj, A. Response of Salustiana orange to high frequency deficit irrigation. Irrig. Sci. 1990, 11, 121–127. [Google Scholar] [CrossRef]
- Pérez-Pérez, J.G.; Romero, P.; Navarro, J.M.; Botía, P. Response of sweet orange cv ‘Lane late’to deficit irrigation in two rootstocks. I: Water relations, leaf gas exchange and vegetative growth. Irrig. Sci. 2008, 26, 415–425. [Google Scholar] [CrossRef]
- González-Altozano, P.; Castel, J.R. Regulated deficit irrigation in ‘Clementina de Nules’ citrus trees. I. Yield and fruit quality effects. J. Hortic. Sci. Biotech. 1999, 74, 706–713. [Google Scholar] [CrossRef]
- González-Altozano, P.; Castel, J.R. Regulated deficit irrigation in ‘Clementina de Nules’ citrus trees. II: Vegetative growth. J. Hortic. Sci. Biotech. 2000, 75, 388–392. [Google Scholar] [CrossRef]
- González-Altozano, P.; Castel, J.R. Riego deficitario controlado en ‘Clementina de Nules’. I. Efectos sobre la producciónyla calidad de la fruta. Span. J. Agric. Res. 2003, 1, 81–92. [Google Scholar]
- García-Tejera, O.; López-Bernal, Á.; Orgaz, F.; Testi, L.; Villalobos, F.J. Are olive root systems optimal for deficit irrigation? Eur. J. Agron. 2018, 99, 72–79. [Google Scholar] [CrossRef]
- Egea, G.; Diaz-Espejo, A.; Fernández, J.E. Soil moisture dynamics in a hedgerow olive orchard under well-watered and deficit irrigation regimes: Assessment, prediction and scenario analysis. Agric. Water Manag. 2016, 164, 197–211. [Google Scholar] [CrossRef]
- Fernandes, R.D.; Egea, G.; Hernandez-Santana, V.; Diaz-Espejo, A.; Fernández, J.; Perez-Martin, A.; Cuevas, M. Response of vegetative and fruit growth to the soil volume wetted by irrigation in a super-high-density olive orchard. Agric. Water Manag. 2021, 258, 107197. [Google Scholar] [CrossRef]
- Carlos, F.U.; Cristian, K.F.; Marco, G.S.; Mauricio, G.; Humberto, A.; de Miranda Jarbas, H.; Oscar, S.S. Testing the Model Efficiency of HYDRUS 2D/3D Under Desert Conditions for Water Content and Pore Electrical Conductivity: A Case Study in an Olive Orchard. J. Soil Sci. Plant Nutr. 2022, 22, 1859–1872. [Google Scholar] [CrossRef]
- CBI. The European Market Potential for Avocados. 2021. Available online: https://www.cbi.eu/market-information/fresh-fruitvegetables/avocados/market-entry (accessed on 25 July 2022).
- Schaffer, B.A.; Wolstenholme, B.N.; Whiley, A.W. The Avocado: Botany, Production and Uses; CABI: Wallingford, UK, 2013. [Google Scholar]
- Tzatzani, T.-T.; Kavroulakis, N.; Doupis, G.; Psarras, G.; Papadakis, I.E. Nutritional status of ‘Hass’ and ‘Fuerte’ avocado (Persea americana Mill.) plants subjected to high soil moisture. J. Plant Nutr. 2020, 43, 327–334. [Google Scholar] [CrossRef]
- Tzatzani, T.-T.; Psarras, G.; Scuderi, D.; Kokolakis, E.; Papadakis, I.E. Effect of Organic Fertilizers on Avocado Trees (Cvs. Fuerte, Hass, Lamb Hass) in Western Crete, a Cool Subtropical Region. Sustainability 2022, 14, 12221. [Google Scholar] [CrossRef]
- Kourgialas, N.N.; Dokou, Z. Water management and salinity adaptation approaches of Avocado trees: A review for hot-summer Mediterranean climate. Agric. Water Manag. 2021, 252, 106923. [Google Scholar] [CrossRef]
- Assouline, S.; Narkis, K. Effects of long-term irrigation with treated wastewater on the hydraulic properties of a clayey soil. Water Resour. Res. 2011, 47, 8. [Google Scholar] [CrossRef]
- Assouline, S.; Kamai, T.; Šimůnek, J.; Narkis, K.; Silber, A. Mitigating the impact of irrigation with effluent water: Mixing with freshwater and/or adjusting irrigation management and design. Water Resour. Res. 2020, 56, e2020WR027781. [Google Scholar] [CrossRef]
- Hassena, A.B.; Zouari, M.; Trabelsi, L.; Khabou, W.; Zouari, N. Physiological improvements of young olive tree (Olea europaea L. cv. Chetoui) under short term irrigation with treated wastewater. Agric. Water Manag. 2018, 207, 53–58. [Google Scholar] [CrossRef]
- Luedeling, E.; Brown, P.H. A global analysis of the comparability of winter chill models for fruit and nut trees. Int. J. Biometeorol. 2011, 55, 411–421. [Google Scholar] [CrossRef]
- Uriu, K.; Magness, J. Deciduous tree fruits and nuts. Irrig. Agric. Lands 1967, 11, 686–703. [Google Scholar]
- Browne, G.T.; Beede, R.H.; Schmidt, L.S. Irrigation water and the health of deciduous fruit and nut crops. In Biology, Detection, and Management of Plant Pathogens in Irrigation Water; Hong, C., Moorman, G., Eds.; APS Press: St. Paul, MN, USA, 2012; pp. 43–53. [Google Scholar]
- Yao, P.; Dong, X.; Hu, A. Using HYDRUS-2D simulate soil water dynamic in jujube root zone under drip irrigation. In Proceedings of the 2011 International Symposium on Water Resource and Environmental Protection, Xi’an, China, 20–22 May 2011; pp. 684–688. [Google Scholar]
- Domínguez-Niño, J.M.; Oliver-Manera, J.; Arbat, G.; Girona, J.; Casadesús, J. Analysis of the Variability in Soil Moisture Measurements by Capacitance Sensors in a Drip-Irrigated Orchard. Sensors 2020, 20, 5100. [Google Scholar] [CrossRef]
- Domínguez-Niño, J.M.; Arbat, G.; Raij-Hoffman, I.; Kisekka, I.; Girona, J.; Casadesús, J. Parameterization of soil hydraulic parameters for HYDRUS-3D simulation of soil water dynamics in a drip-irrigated orchard. Water 2020, 12, 1858. [Google Scholar] [CrossRef]
- Phogat, V.; Skewes, M.A.; Mahadevan, M.; Cox, J. Evaluation of soil plant system response to pulsed drip irrigation of an almond tree under sustained stress conditions. Agric. Water Manag. 2013, 118, 1–11. [Google Scholar] [CrossRef]
- Phogat, V.; Skewes, M.; Cox, J.; Mahadevan, M. Modelling the impact of pulsing of drip irrigation on the water and salinity dynamics in soil in relation to water uptake by an almond tree. WIT Trans. Ecol. Environ. 2012, 168, 101–113. [Google Scholar]
- Yang, T.; Li, D.; Clothier, B.; Wang, Y.; Duan, J.; Di, N.; Li, G.; Li, X.; Jia, L.; Xi, B. Where to monitor the soil-water potential for scheduling drip irrigation in Populus tomentosa plantations located on the North China Plain? For. Ecol. Manag. 2019, 437, 99–112. [Google Scholar] [CrossRef]
- Janssens, P.; Diels, J.; Vanderborght, J.; Elsen, F.; Elsen, A.; Deckers, T.; Vandendriessche, H. Numerical calculation of soil water potential in an irrigated ‘conference’ pear orchard. Agric. Water Manag. 2015, 148, 113–122. [Google Scholar] [CrossRef]
- Xi, B.; Bloomberg, M.; Watt, M.S.; Wang, Y.; Jia, L. Modeling growth response to soil water availability simulated by HYDRUS for a mature triploid Populus tomentosa plantation located on the North China Plain. Agric. Water Manag. 2016, 176, 243–254. [Google Scholar] [CrossRef]
- Nazari, E.; Besharat, S.; Zeinalzadeh, K.; Mohammadi, A. Measurement and simulation of the water flow and root uptake in soil under subsurface drip irrigation of apple tree. Agric. Water Manag. 2021, 255, 106972. [Google Scholar] [CrossRef]
- Elmaloglou, S.; Diamantopoulos, E. Simulation of soil water dynamics under subsurface drip irrigation from line sources. Agric. Water Manag. 2009, 96, 1587–1595. [Google Scholar] [CrossRef]
- Mohammad, N.; Alazba, A.; Šimůnek, J. HYDRUS simulations of the effects of dual-drip subsurface irrigation and a physical barrier on water movement and solute transport in soils. Irrig. Sci. 2014, 32, 111–125. [Google Scholar]
- Bughici, T.; Skaggs, T.H.; Corwin, D.L.; Scudiero, E. Ensemble HYDRUS-2D modeling to improve apparent electrical conductivity sensing of soil salinity under drip irrigation. Agric. Water Manag. 2022, 272, 107813. [Google Scholar] [CrossRef]
- Hardie, M.; Ridges, J.; Swarts, N.; Close, D. Drip irrigation wetting patterns and nitrate distribution: Comparison between electrical resistivity (ERI), dye tracer, and 2D soil–water modelling approaches. Irrig. Sci. 2018, 36, 97–110. [Google Scholar] [CrossRef]
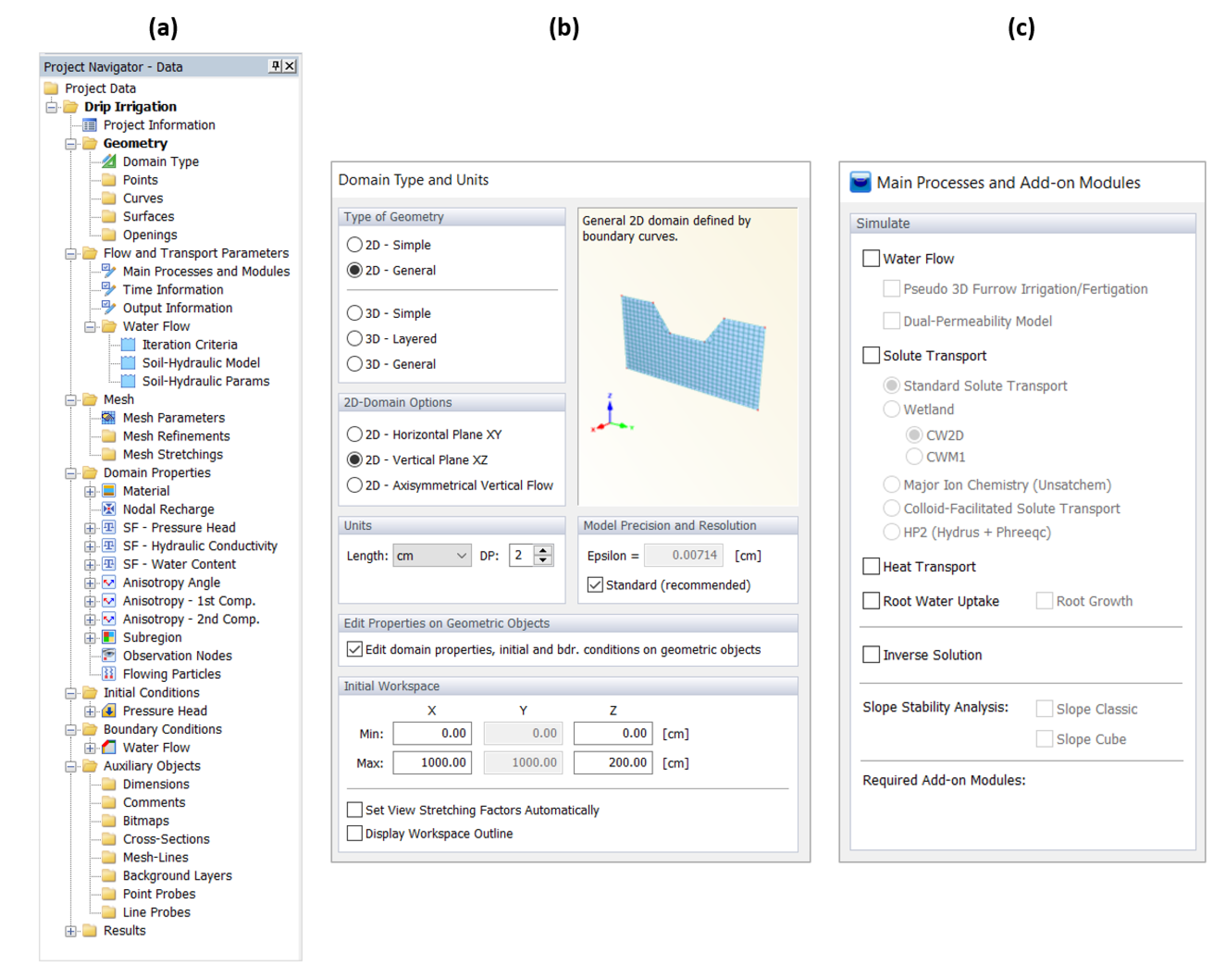
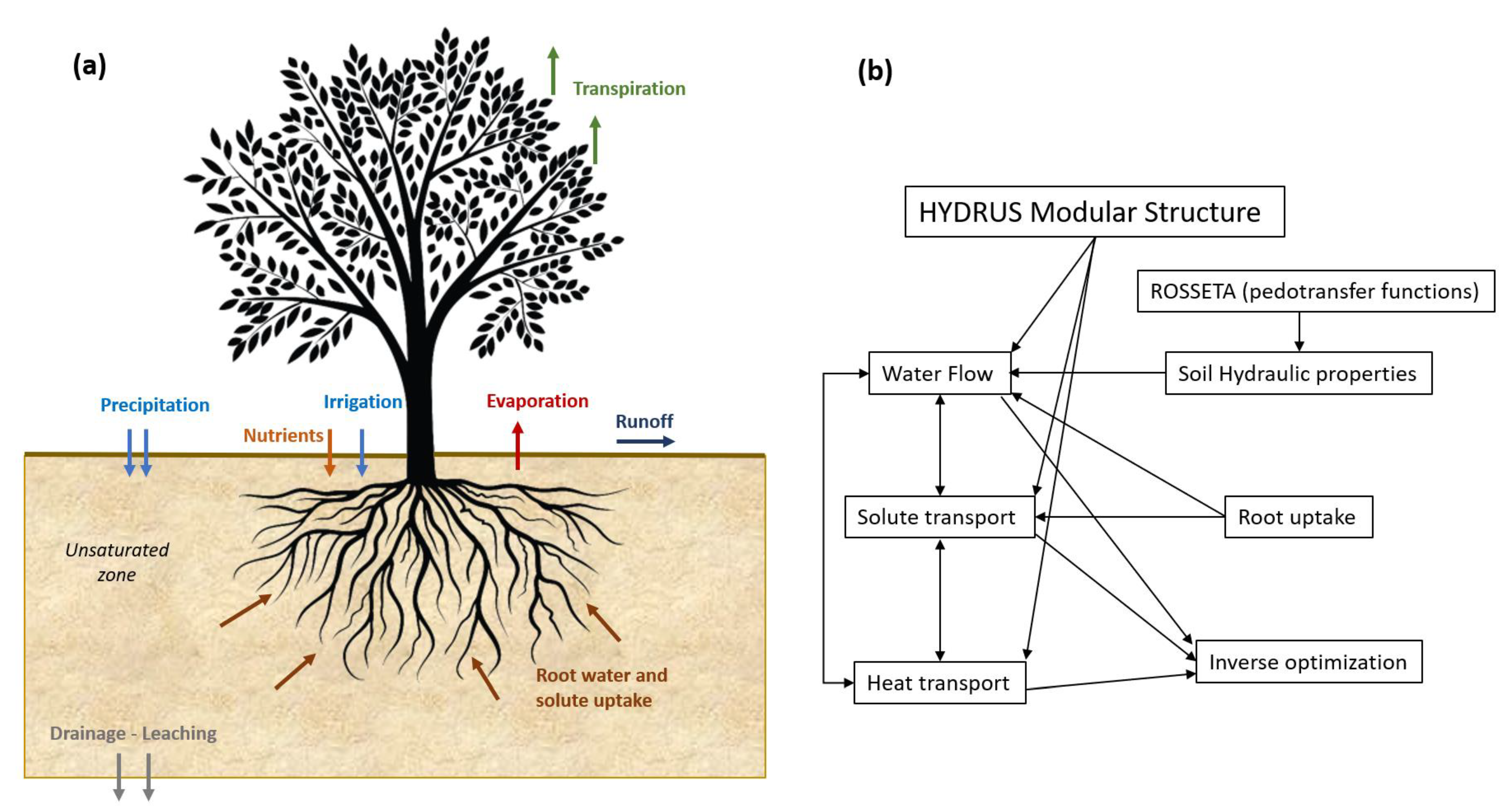
| Research Report | Crop | Soil Type | Irrigation System 1 | Irrigation Treatment 2 | HYDRUS 2D/3D | Simulation Processes |
|---|---|---|---|---|---|---|
| Yao et al. [81] | jujube | (soil depth 0–60): loam | DI-2 drip lines | - | 2D | Water flow Root uptake |
| (soil depth 60–100): clay loam | ||||||
| Phogat et al. [85] | almond | (homogenous soil depth 0–150): sand | DI-2 drip lines | Ip vs. Ic | 2D | Water flow Root uptake Salinity dynamics |
| Phogat et al. [9] | almond | (homogenous soil depth 0–150): sand | DI-2 drip lines | Ip vs. Ic | 2D | Water flow Root uptake Salinity dynamics |
| Phogat et al. [84] | almond | (homogenous soil depth 0–150): sand | DI-2 drip lines | FIp: 100% Etc | 2D | Water flow Root uptake Salinity dynamics |
| SDIp: 65% Etc | ||||||
| FIc: 100% Etc | ||||||
| Janssens et al. [87] | pear | (homogenous soil depth): silt loam | DI-1 drip line | SDI: 80% ETc | 2D | Water flow Root uptake |
| SDI: 50% ETc | ||||||
| Xi et al. [88] | P. tomentosa plantation | (soil depth 0–120): silt | SubDI-3 drip lines/tree belt | T25 | 2D | Water flow Root uptake |
| (soil depth >120): silt loam | No irrigation | 1D | ||||
| Hardie et al. [93] | apple | (soil depth 0–30): sandy loam | DI-1 drip line and fertigation | low rate | 2D | Water flow Root uptake Nitrate dynamics |
| (soil depth 30–50): sandy loam | ||||||
| (soil depth 50–70): light clay | high rate | |||||
| (soil depth >70): medium clay | ||||||
| Yang et al. [86] | P. tomentosa plantation | (soil depth 0–140): sandy loam | DI-2 drip lines | T20 | 2D | Water flow Root uptake |
| ((soil depth 140–300): silt loam | ||||||
| Domínguez-Niño et al. [82] | apple | (soil depth 0–60): loam | DI-1 drip line | FI: 100% ETc | 3D | Water flow Root uptake |
| Domínguez-Niño et al. [83] | apple | (soil depth 0–60): loam | DI-1 drip line | FI: 100% ETc | 3D | Water flow Root uptake |
| Nazari et al. [89] | apple | (soil depth 0–30): loam | SubDI-2 drip lines | different discharge and duration | 2D | Water flow Root uptake |
| (soil depth 30–120): sandy loam | ||||||
| Bughici et al. [92] | pistachio | (homogenous soil depth): clay loam | DI-1 drip line | high salinity | 2D | Water flow Root uptake Salinity dynamics |
| low salinity |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Morianou, G.; Kourgialas, N.N.; Karatzas, G.P. A Review of HYDRUS 2D/3D Applications for Simulations of Water Dynamics, Root Uptake and Solute Transport in Tree Crops under Drip Irrigation. Water 2023, 15, 741. https://doi.org/10.3390/w15040741
Morianou G, Kourgialas NN, Karatzas GP. A Review of HYDRUS 2D/3D Applications for Simulations of Water Dynamics, Root Uptake and Solute Transport in Tree Crops under Drip Irrigation. Water. 2023; 15(4):741. https://doi.org/10.3390/w15040741
Chicago/Turabian StyleMorianou, Giasemi, Nektarios N. Kourgialas, and George P. Karatzas. 2023. "A Review of HYDRUS 2D/3D Applications for Simulations of Water Dynamics, Root Uptake and Solute Transport in Tree Crops under Drip Irrigation" Water 15, no. 4: 741. https://doi.org/10.3390/w15040741
APA StyleMorianou, G., Kourgialas, N. N., & Karatzas, G. P. (2023). A Review of HYDRUS 2D/3D Applications for Simulations of Water Dynamics, Root Uptake and Solute Transport in Tree Crops under Drip Irrigation. Water, 15(4), 741. https://doi.org/10.3390/w15040741







