Experimental Analysis of 3D Flow Structures around a Floating Dike
Abstract
:1. Introduction
- (i)
- Floating dikes impose a marginal effect on sediment transport in rivers. Flow velocities are usually smaller at the riverbed than close to the water surface, while the opposite is true for sediment concentration which is high near the riverbed. In braided rivers, adopting fixed dikes such as spur dikes can change not only the discharge ratio but also the rate of sediment loading and transport. Because the influence of floating dikes on the fluvial processes is stronger in the upper part rather than the lower part of the water column, floating dikes have less impact on sediment transport than fixed dikes.
- (ii)
- Since floating dikes are located near the water surface, they are not significantly affected by bed deformation; thus, they easily adapt to diverse flow and sediment conditions and bed morphology. On the other hand, fixed dikes are usually permanent structures and difficult to remove after construction, even if they are found to modify the fluvial or sediment processes in a negative way under certain conditions.
- (iii)
- Floating dikes have little effect on the local riverbed and ecology. Construction of fixed dikes can change the riverbed substrates significantly [6] and, hence, the habitats of aquatic organisms. For example, some fixed dikes in the Yangtze River are about 100 m long, and the soft mattresses which are used to prevent local scouring are tens of thousands of square meters. Both the fixed dikes and corresponding soft mattresses lie directly on the riverbed, preventing material exchange between the riverbed and river flow, which can alter the riverbed substrates and the aquatic environment [7,8].
2. Method
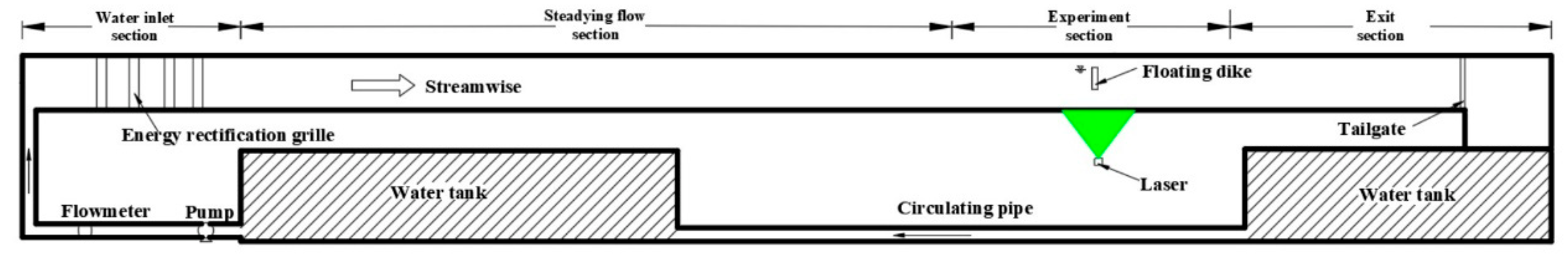
2.1. Experimental Equipment
2.2. Experiment Cases
2.3. Calculation of Hydrodynamic Characteristics
3. Results and Discussion
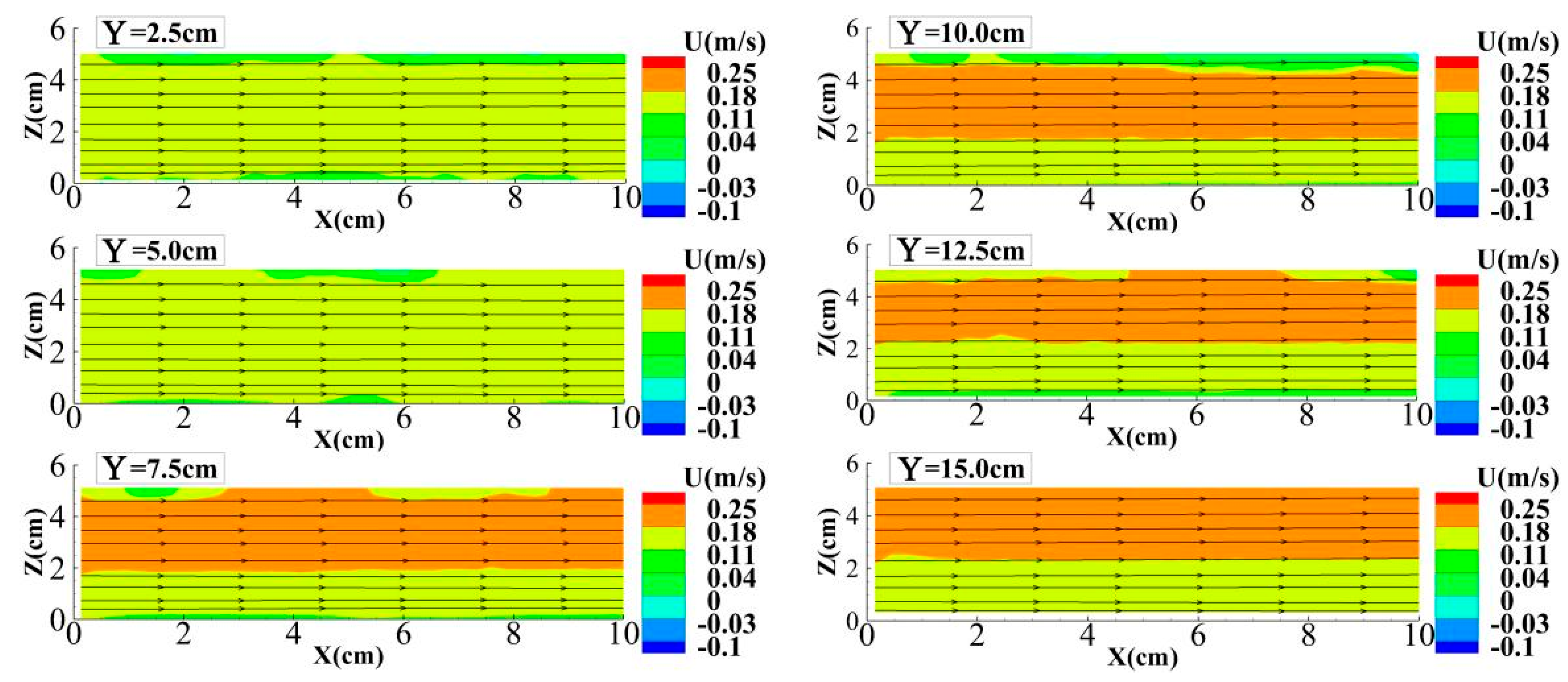
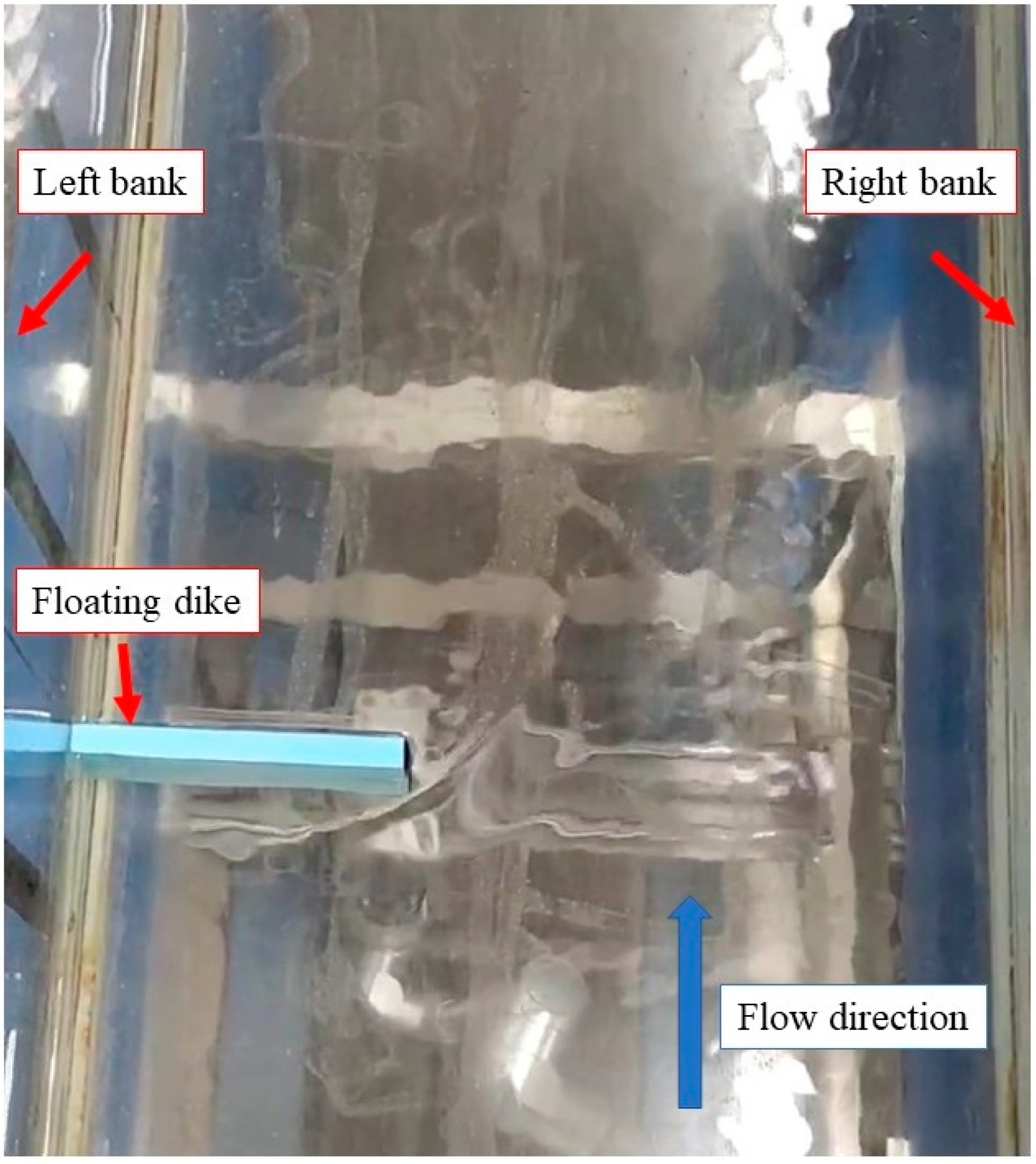
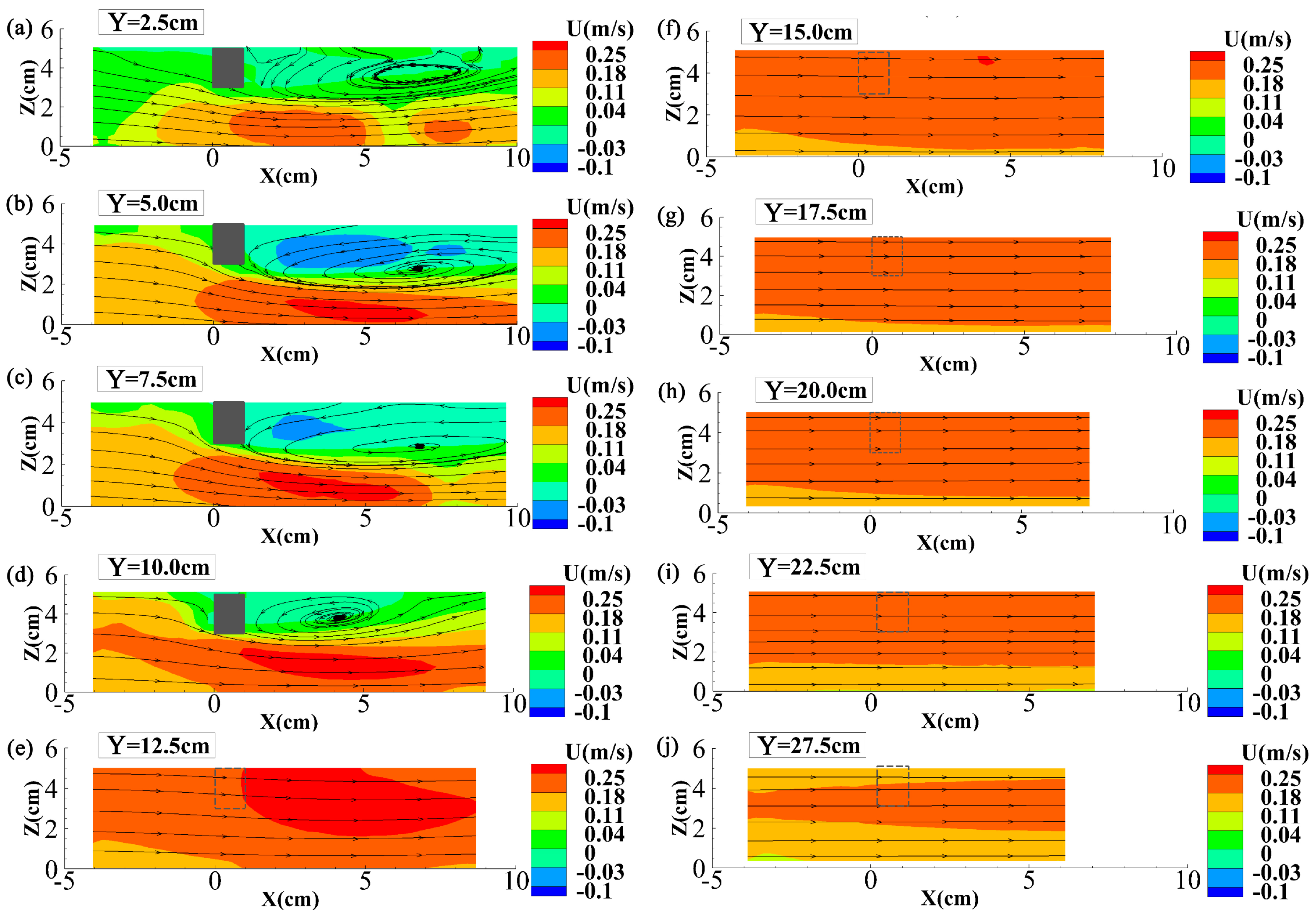
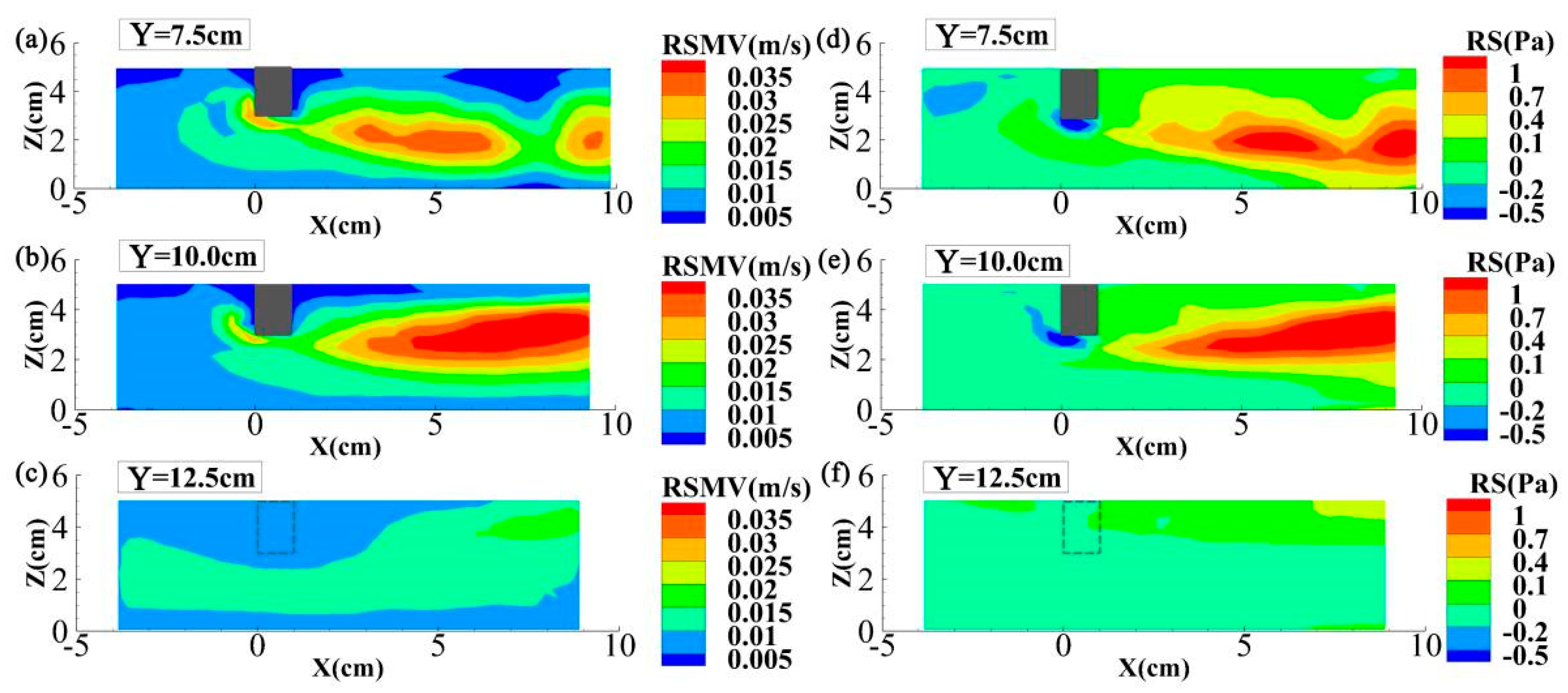
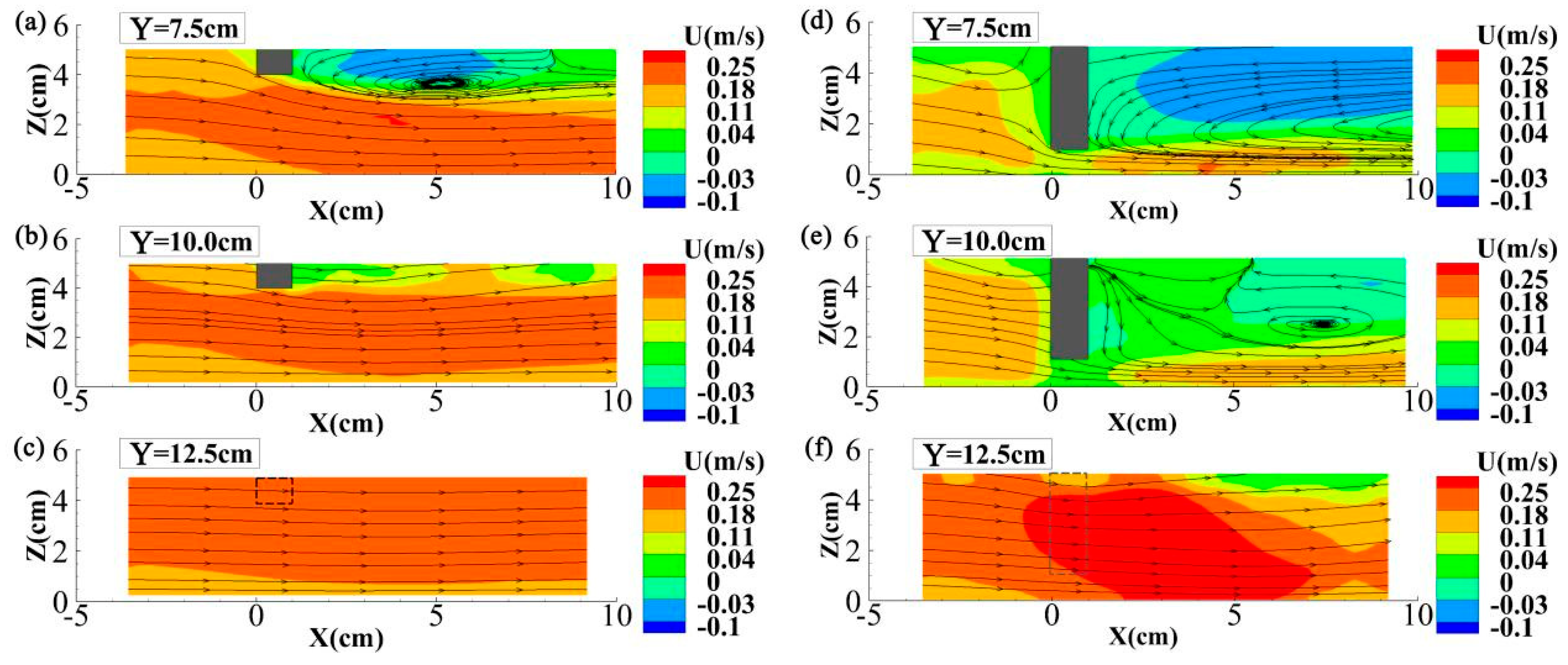
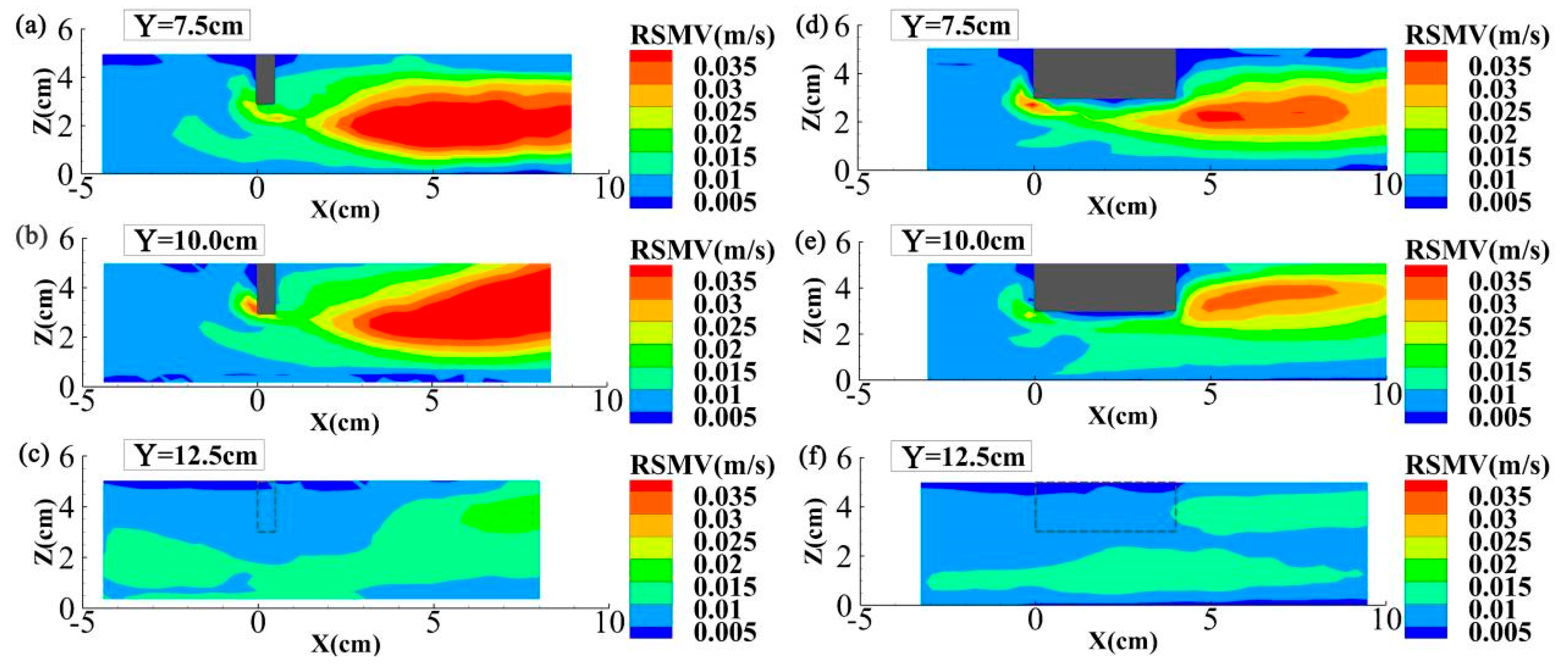
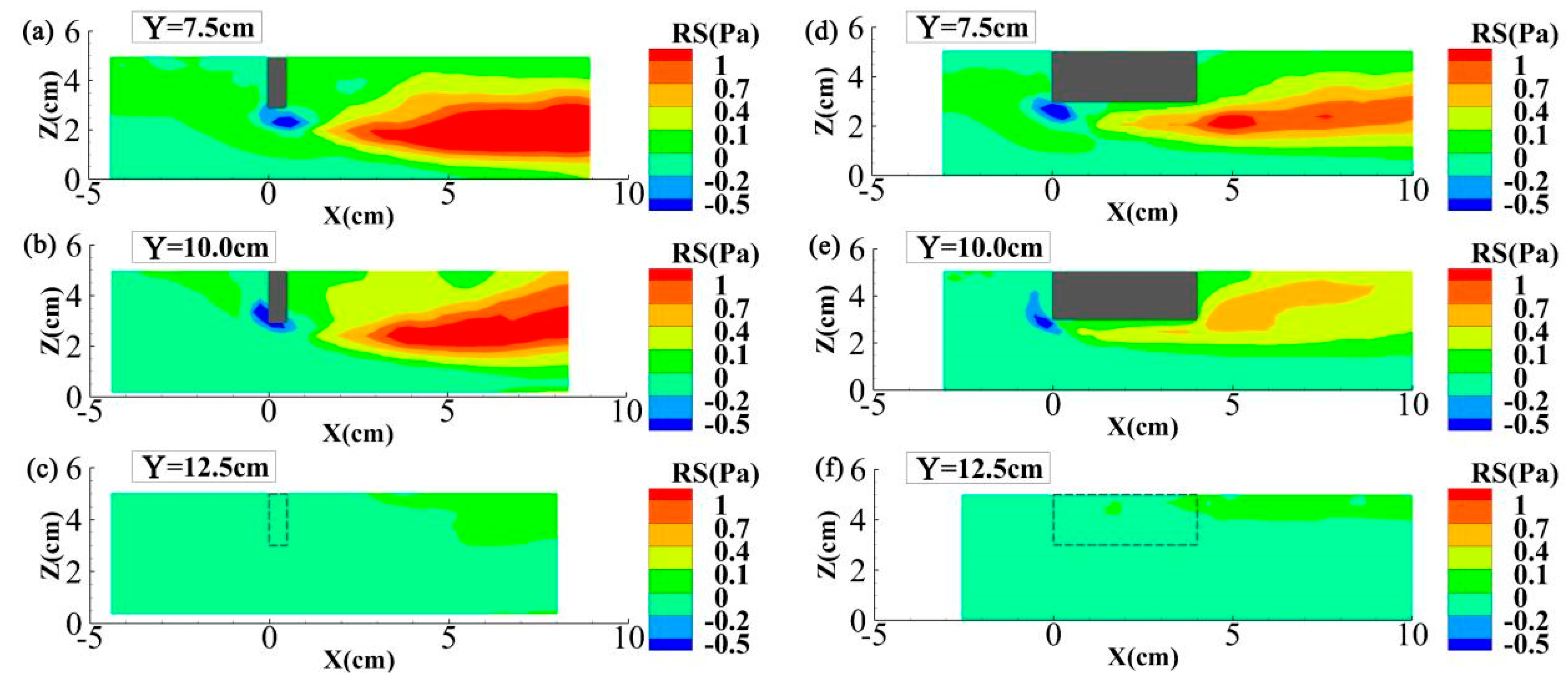
3.1. Local Flow Characteristics around a Floating Dike
3.2. Effects of Submerged Ratio of Floating Dike
3.3. Effects of Length Ratio of Floating Dike
3.4. Effects of Thickness of Floating Dike
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cai, Y.; Xie, X.; Huang, G. Research and practice of Pontoon-type floating row applied in front of Three Gorges Dam to remove floating debris. J. Yangtze River Sci. Res. Inst. 2016, 33, 63–66. (In Chinese) [Google Scholar]
- Cai, Y.; Yang, W.; Huang, G. Study and implementation of hydraulic integrated floating debris control and reservoir surface security for water control project. Water Resour. Hydropower Eng. 2017, 48, 168–173. (In Chinese) [Google Scholar] [CrossRef]
- Li, X. Design of sewage interception and cleaning for Xiaoxia Hydropower Station. Gansu Water Conserv. Hydropower Technol. 2009, 45, 33–34, 53. (In Chinese) [Google Scholar]
- Duan, W.; Huang, G.; Zhang, H.; Li, L. Experimental research on vortexes eliminating of several typical hydraulic intakes. J. Yangtze River Sci. Res. Inst. 2011, 28, 21–26. (In Chinese) [Google Scholar]
- Huang, M. Research Report on Optimization Measures to Prevent Snail Diffusion in Water Diversion Project under Different Working Conditions; Changjiang River Scientific Research Institute: Wuhan, China, 2017. (In Chinese) [Google Scholar]
- Yu, X. Flume experiment of local scour around geotextile mattress for Shuangjiansha central bar protection in Lower Reaches of Yangtze River. J. Waterw. Harb. 2014, 35, 223–226. (In Chinese) [Google Scholar]
- Duan, X. Ecological Impacts of Water and Sediment Transportation on Macroinvertebrate Community in Rivers. Ph.D. Thesis, Tsinghua University, Beijing, China, 2009. (In Chinese). [Google Scholar]
- Buss, D.F.; Baptista, D.F.; Nessimian, J.L.; Egler, M. Substrate specificity, environmental degradation and disturbance structuring macroinvertebrate assemblages in neotropical streams. Hydrobiologia 2004, 518, 179–188. [Google Scholar] [CrossRef]
- Fox, J.F.; Papanicolaou, A.N.; Kjos, L. Eddy taxonomy methodology around a submerged barb obstacle within a fixed rough bed. J. Eng. Mech. 2005, 131, 1082–1101. [Google Scholar] [CrossRef]
- Jamieson, E.C.; Rennie, C.D.; Jacobson, R.B.; Townsend, R.D. 3-D flow and scour near a submerged wing dike: ADCP measurements on the Missouri River. Water Resour. Res. 2011, 47, 1–20. [Google Scholar] [CrossRef]
- Koken, M.; Gogus, M. Effect of spur dike length on the horseshoe vortex system and the bed shear stress distribution. J. Hydraul. Res. 2015, 53, 196–206. [Google Scholar] [CrossRef]
- Nakagawa, H.; Teraguchi, H.; Kawaike, K.; Baba, Y.; Zhang, H. Analysis of Bed Variation around Bandal-like Structures. Annu. Disaster Prev. Res.Inst. Kyoto Univ. 2011, 54, 497–510. [Google Scholar]
- Papanicolaou, A.N.T.; Kjos, L.J.; Fox, J.F. Investigation of Flow and Local Scour Characteristics around a Partially Submerged Permeable WSDOT Barb; Washington State Transportation Center: Washington, DC, USA, 2004. [Google Scholar]
- Patel, H.K.; Arora, S.; Lade, A.D.; Kumar, B.; Azamathulla, H.M. Flow behavior concerning bank stability in the presence of spur dike—A review. Water Supply 2022, 23, 237–258. [Google Scholar] [CrossRef]
- Zhou, Y.; Liu, H.; He, C.; Zong, Q. Experimental study on flow character around the pillared spur dike. J. Sediment Res. 2009, 58–62. (In Chinese) [Google Scholar] [CrossRef]
- Zhou, Y.; Michiue, M.; Hinokidani, O. Study on flow characteristics around the non-submerged spur-dikes. J. Hydraul. Eng. 2004, 31–35. (In Chinese) [Google Scholar]
- Tong, Z.S.; Zhou, H.; Wu, S.Q.; Chen, W.W.; Lu, X.J. State of the art of structures guiding floating-debris in hydro-plants. Hydro-Sci. Eng. 2002, 73–78. (In Chinese) [Google Scholar] [CrossRef]

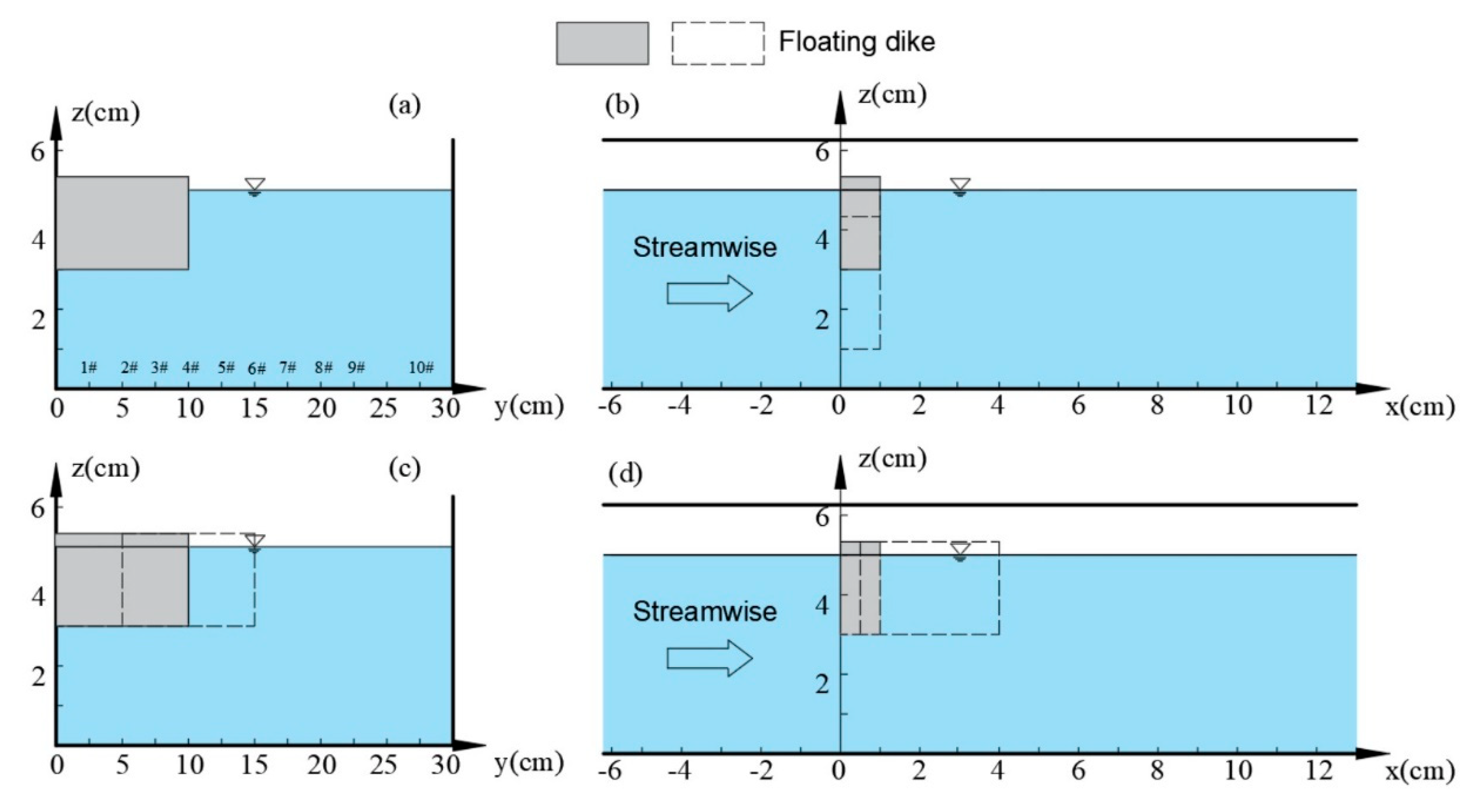






| Definition | Symbol | Dimension |
|---|---|---|
| Water depth | h | [L] |
| Channel width | W | [L] |
| Dike length | L | [L] |
| Submerged depth of the dike | d | [L] |
| Dike thickness | B | [L] |
| Length ratio | K = L/W | 1 |
| Submerged ratio | D = d/h | 1 |
| Case No. | Submerged Depth d (cm) | Submerged Ratio D = d/h | Length L (cm) | Length Ratio K = L/W | Thickness B (cm) |
|---|---|---|---|---|---|
| 1 | / | / | / | / | / |
| 2 | 2 | 2/5 | 10 | 1/3 | 1 |
| 3 | 1 | 1/5 | 10 | 1/3 | 1 |
| 4 | 4 | 4/5 | 10 | 1/3 | 1 |
| 5 | 2 | 2/5 | 5 | 1/6 | 1 |
| 6 | 2 | 2/5 | 15 | 1/2 | 1 |
| 7 | 2 | 2/5 | 10 | 1/3 | 0.5 |
| 8 | 2 | 2/5 | 10 | 1/3 | 4 |
| Case No. | In the Backflow Zone | On the Right Side of the Dike | |||
|---|---|---|---|---|---|
| U (m/s) | RSMV (m/s) | RS (Pa) | U (m/s) | ||
| Max | Min | Max | Max | Max | |
| 2 | 0.08 | −0.06 | 0.035 | 1 | 0.25 |
| 3 | 0.08 | −0.06 | 0.035 | 1 | 0.22 |
| 4 | 0.08 | −0.06 | 0.032 | 0.85 | 0.25 |
| 5 | 0.08 | −0.06 | 0.035 | 1 | 0.22 |
| 6 | 0.02 | −0.06 | 0.035 | 1 | 0.25 |
| 7 | 0.08 | −0.06 | 0.035 | 1 | 0.25 |
| 8 | 0.02 | −0.02 | 0.035 | 1 | 0.25 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, W.; Ni, Y.; Creed, M.; Yu, S. Experimental Analysis of 3D Flow Structures around a Floating Dike. Water 2023, 15, 703. https://doi.org/10.3390/w15040703
Huang W, Ni Y, Creed M, Yu S. Experimental Analysis of 3D Flow Structures around a Floating Dike. Water. 2023; 15(4):703. https://doi.org/10.3390/w15040703
Chicago/Turabian StyleHuang, Wei, Yufang Ni, Maggie Creed, and Sihan Yu. 2023. "Experimental Analysis of 3D Flow Structures around a Floating Dike" Water 15, no. 4: 703. https://doi.org/10.3390/w15040703





