Utilization of Multivariate Optimization for Preconcentration and Determination of Lead in Different Water and Food Samples Using Functionalized Activated Carbon
Abstract
:1. Introduction
2. Experiment
2.1. Apparatus
2.2. Reagents and Solutions
2.3. Synthesis of Activated Carbon
2.3.1. Preparation of Apricot-Pit-Based Activated Carbon
2.3.2. Surface Modification of Activated Carbon
2.4. Sample Collection
2.5. Microextraction Procedure
2.6. Adsorption Studies
2.7. Kinetic Study
2.8. Multivariate Experimental Design for Pb2+ Analysis
2.8.1. Plackett–Burman Design
2.8.2. Central Composite Design
3. Results and Discussion
3.1. Characterization Study of Adsorbent
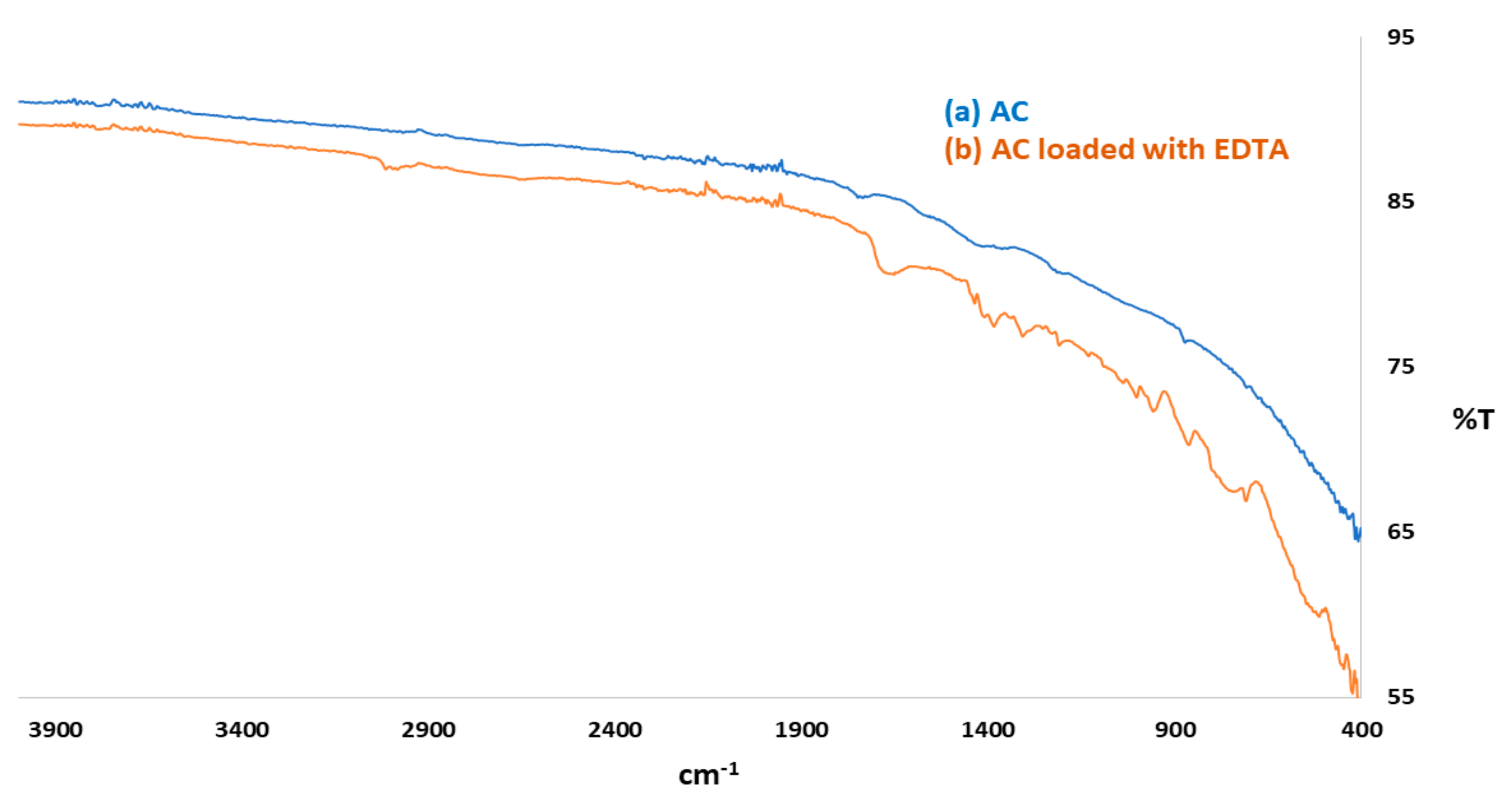
3.1.1. FTIR Analysis
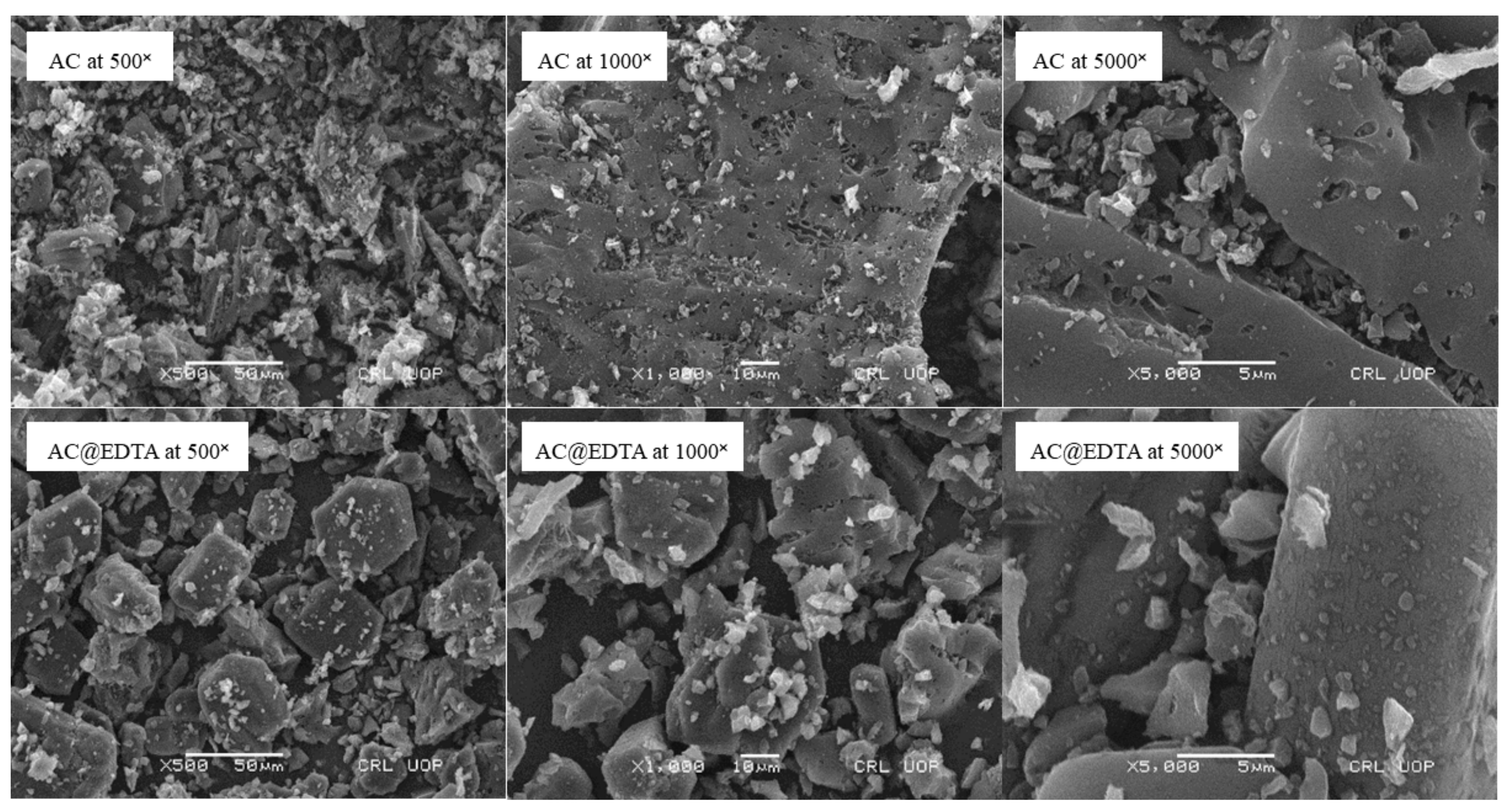
3.1.2. SEM Analysis
3.1.3. N2 Adsorption Isotherm in Activated Carbon
3.2. Optimization of Experimental Parameters
3.2.1. Design of Experiments
| Adsorbent | Adsorption Capacity (mg g−1) | Reference |
|---|---|---|
| Sawdust | 3.2 | [31] |
| Hazelnut shells | 13.0 | [32] |
| Raw rice husks | 16.5 | [33] |
| Eichhornia | 16.6 | [34] |
| Coconut shells | 17.1 | [35] |
| Peach pits | 17.5 | [35] |
| Olive stones | 18.3 | [35] |
| Calcite | 19.9 | [36] |
| Apricot kernel shells | 22.8 | [37] |
| Almond shells | 22.7 | [35] |
| Ceiba pentandra hulls | 25.5 | [38] |
| Tamarind wood | 43.0 | [39] |
| Bamboo AC | 45.4 | [40] |
| Alternanthera philoxeroides | 53.7 | [41] |
| Commercial AC | 64.2 | [42] |
| Date bead | 76.9 | [41] |
| Eucalyptus camalbulensis Dehn | 113.9 | [43] |
| Date pits AC | 115.8 | [42] |
| BAC@SiO2-EDTA | 123.4 | [40] |
| Cassava peels | 5.8 | [44] |
| Banana peels | 57.1 | [45] |
| CA@EDTA | 36.17 | Present study |
| (a) Isotherm Parameters | ||
|---|---|---|
| Type of Isotherm | Parameter | |
| Langmuir | qmax (mg/g) | 109.05 |
| KL (L/mg) | 0.03 | |
| RL | 0.39 | |
| R2 | 0.9996 | |
| Freundlich | Kf | 6.17 |
| 1/n | 0.52 | |
| R2 | 0.9202 | |
| (b) Kinetics Parameters | ||
| Order of reaction | Parameter | |
| qe,exp (mg/g) | 36.17 | |
| Pseudo-first-order | qe,cal (mg/g) | 1.36 |
| K1 (min−1) | −9.2 × 10−4 | |
| R2 | 0.53 | |
| Pseudo-second-order | qe,cal (mg/g) | 35.17 |
| K2 (g mg−1 min−1) | 0.08 | |
| R2 | 0.9998 | |
| (a) Factors and levels used in factorial design for Pb2+ analysis. | |||||||
| Factor | ID | Unit | Lower - | Higher + | Optimum a | ||
| pH | A | - | 4 | 8 | 6 | ||
| Temperature | B | °C | 30 | 60 | 46 | ||
| Sorbent amount | C | mg | 40 | 60 | 40 | ||
| Sample volume | D | mL | 10 | 20 | 17 | ||
| Sample flow rate | E | mL min−1 | 5 | 10 | 10 b | ||
| Eluent volume | F | mL | 5 | 10 | 5 b | ||
| (b) Multivariate design matrix and the result of % R (n = 6). | |||||||
| S. No | A | B | C | D | E | F | % R |
| 1 | - | + | + | - | + | - | 15.1 ± 1.9 |
| 2 | + | - | - | + | + | - | 15.8 ± 2.4 |
| 3 | - | - | + | + | - | + | 17.0 ± 3.1 |
| 4 | - | + | - | - | + | + | 16.7 ± 2.0 |
| 5 | - | + | + | - | - | + | 17.4 ± 3.4 |
| 6 | - | + | - | + | - | - | 31.9 ± 1.2 |
| 7 | - | - | - | - | + | - | 13.7 ± 0.9 |
| 8 | - | - | + | - | + | - | 9.1 ± 2.1 |
| 9 | + | + | + | + | + | - | 57.7 ± 0.9 |
| 10 | + | - | + | + | + | + | 70.0 ± 1.7 |
| 11 | + | + | - | + | - | + | 44.4 ± 3.2 |
| 12 | - | + | - | + | + | + | 25.1 ± 4.0 |
| 13 | - | - | - | - | - | - | 12.4 ± 3.1 |
| 14 | - | + | + | + | + | + | 22.7 ± 2.1 |
| 15 | + | - | + | - | + | + | 95.1 ± 2.5 |
| 16 | + | - | - | + | + | - | 99.8 ± 1.2 |
| 17 | - | - | + | + | - | - | 19.1 ± 2.4 |
| 18 | - | - | - | + | - | + | 20.8 ± 4.0 |
| 19 | + | + | + | - | - | - | 83.4 ± 3.1 |
| 20 | + | - | + | - | - | + | 90.3 ± 3.3 |
| 21 | + | - | - | - | - | + | 92.0 ± 1.8 |
| 22 | + | + | - | - | - | - | 67.1 ± 3.1 |
| 23 | + | + | - | - | + | + | 79.7 ± 2.3 |
| 24 | + | + | + | + | - | - | 87.3 ± 2.0 |
| S. No. | A | B | C | D | % R |
|---|---|---|---|---|---|
| 1 | aAo | bBo | cCo | dDo | 99.4 ± 0.7 |
| 2 | - | - | - | - | 14.1 ± 0.7 |
| 3 | + | - | - | - | 20.5 ± 2.1 |
| 4 | - | + | - | - | 60.3 ± 1.7 |
| 5 | + | + | - | - | 12.0 ± 3.1 |
| 6 | - | - | + | - | 20.1 ± 1.9 |
| 7 | + | - | + | - | 55.3 ± 2.7 |
| 8 | - | + | + | - | 56.7 ± 0.9 |
| 9 | + | + | + | - | 45.4 ± 1.1 |
| 10 | - | - | - | + | 15.1 ± 0.7 |
| 11 | + | - | - | + | 44.4 ± 1.0 |
| 12 | - | + | - | + | 33.5 ± 2.2 |
| 13 | + | + | - | + | 46.0 ± 0.9 |
| 14 | - | - | + | + | 60.8 ± 4.1 |
| 15 | + | - | + | + | 86.2 ± 2.1 |
| 16 | -aA | bB | cC | dD | 28.2 ± 2.2 |
| 17 | +aA | bB | cC | dD | 37.6 ± 0.9 |
| 18 | aA | -bB | cC | dD | 16.7 ± 4.1 |
| 19 | aA | +bB | cC | dD | 4.8 ± 3.0 |
| 20 | aA | bB | -cC | dD | 69.6 ± 1.5 |
| 21 | aA | bB | +cC | dD | 39.4 ± 2.2 |
| 22 | aA | bB | cC | -dD | 65.0 ± 1.4 |
| 23 | aA | bB | cC | +dD | 7.9 ± 0.8 |
| 24 | aAo | bBo | cCo | dDo | 99.2 ± 1.1 |
3.2.2. Response Surface Methodology
3.3. Interference Study
3.4. Analytical Figures of Merit
3.5. Validation of the Proposed Method
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Nagajyoti, P.C.; Lee, K.D.; Sreekanth, T. Heavy metals, occurrence and toxicity for plants: A review. Environ. Chem. Lett. 2010, 8, 199–216. [Google Scholar] [CrossRef]
- Sall, M.L.; Diaw, A.K.D.; Gningue-Sall, D.; Aaron, S.E.; Aaron, J.-J. Toxic heavy metals: Impact on the environment and human health, and treatment with conducting organic polymers, a review. Environ. Sci. Pollut. Res. 2020, 27, 29927–29942. [Google Scholar] [CrossRef] [PubMed]
- Ighalo, J.O.; Adeniyi, A.G.; Adeniran, J.A.; Ogunniyi, S. A systematic literature analysis of the nature and regional distribution of water pollution sources in Nigeria. J. Clean. Prod. 2021, 283, 124566. [Google Scholar] [CrossRef]
- Lu, F.; Astruc, D. Nanocatalysts and other nanomaterials for water remediation from organic pollutants. Coord. Chem. Rev. 2020, 408, 213180. [Google Scholar] [CrossRef]
- Ain, Q.-U.; Farooq, M.U.; Jalees, M.I. Application of magnetic graphene oxide for water purification: Heavy metals removal and disinfection. J. Water Process Eng. 2020, 33, 101044. [Google Scholar] [CrossRef]
- Amari, T.; Ghnaya, T.; Abdelly, C. Nickel, cadmium and lead phytotoxicity and potential of halophytic plants in heavy metal extraction. S. Afr. J. Bot. 2017, 111, 99–110. [Google Scholar] [CrossRef]
- Kam, C.S.; Leung, T.L.; Liu, F.; Djurišić, A.B.; Xie, M.H.; Chan, W.-K.; Zhou, Y.; Shih, K. Lead removal from water—Dependence on the form of carbon and surface functionalization. RSC Adv. 2018, 8, 18355–18362. [Google Scholar] [CrossRef] [PubMed]
- Xu, C.; Liu, J.; Bi, Y.; Ma, C.; Bai, J.; Hu, Z.; Zhou, M. Biomass derived worm-like nitrogen-doped-carbon framework for trace determination of toxic heavy metal lead (II). Anal. Chim. Acta 2020, 1116, 16–26. [Google Scholar] [CrossRef]
- Lin, M.; Chen, Z. A facile one-step synthesized epsilon-MnO2 nanoflowers for effective removal of lead ions from wastewater. Chemosphere 2020, 250, 126329. [Google Scholar] [CrossRef]
- Rasheed, T.; Bilal, M.; Nabeel, F.; Iqbal, H.M.; Li, C.; Zhou, Y. Fluorescent sensor based models for the detection of environmentally-related toxic heavy metals. Sci. Total Environ. 2018, 615, 476–485. [Google Scholar] [CrossRef]
- Akpomie, K.G.; Conradie, J. Advances in application of cotton-based adsorbents for heavy metals trapping, surface modifications and future perspectives. Ecotoxicol. Environ. Saf. 2020, 201, 110825. [Google Scholar] [CrossRef]
- Yang, X.; Wan, Y.; Zheng, Y.; He, F.; Yu, Z.; Huang, J.; Wang, H.; Ok, Y.S.; Jiang, Y.; Gao, B. Surface functional groups of carbon-based adsorbents and their roles in the removal of heavy metals from aqueous solutions: A critical review. Chem. Eng. J. 2019, 366, 608–621. [Google Scholar] [CrossRef]
- Duan, C.; Ma, T.; Wang, J.; Zhou, Y. Removal of heavy metals from aqueous solution using carbon-based adsorbents: A review. J. Water Process Eng. 2020, 37, 101339. [Google Scholar] [CrossRef]
- Rehman, A.; Park, M.; Park, S.-J. Current progress on the surface chemical modification of carbonaceous materials. Coatings 2019, 9, 103. [Google Scholar] [CrossRef]
- Taer, E.; Yusra, D.A.; Amri, A.; Awitdrus; Taslim, R.; Apriwandi; Agustino; Putri, A. The synthesis of activated carbon made from banana stem fibers as the supercapacitor electrodes. Mater. Today Proc. 2021, 44, 3346–3349. [Google Scholar] [CrossRef]
- Li, Z.; Chang, X.; Zou, X.; Zhu, X.; Nie, R.; Hu, Z.; Li, R. Chemically-modified activated carbon with ethylenediamine for selective solid-phase extraction and preconcentration of metal ions. Anal. Chim. Acta 2009, 632, 272–277. [Google Scholar] [CrossRef]
- Zhu, J.; Yang, J.; Deng, B. Enhanced mercury ion adsorption by amine-modified activated carbon. J. Hazard. Mater. 2009, 166, 866–872. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Zhang, J.; Liu, H. In-situ modification of activated carbon with ethylenediaminetetraacetic acid disodium salt during phosphoric acid activation for enhancement of nickel removal. Powder Technol. 2018, 325, 113–120. [Google Scholar] [CrossRef]
- Rivera-Utrilla, J.; Sánchez-Polo, M.; Gómez-Serrano, V.; Álvarez, P.; Alvim-Ferraz, M.; Dias, J. Activated carbon modifications to enhance its water treatment applications. An overview. J. Hazard. Mater. 2011, 187, 1–23. [Google Scholar] [CrossRef] [PubMed]
- Naushad, M.; Alqadami, A.A.; AlOthman, Z.A.; Alsohaimi, I.H.; Algamdi, M.S.; Aldawsari, A.M. Adsorption kinetics, isotherm and reusability studies for the removal of cationic dye from aqueous medium using arginine modified activated carbon. J. Mol. Liq. 2019, 293, 111442. [Google Scholar] [CrossRef]
- Lv, S.; Li, C.; Mi, J.; Meng, H. A functional activated carbon for efficient adsorption of phenol derived from pyrolysis of rice husk, KOH-activation and EDTA-4Na-modification. Appl. Surf. Sci. 2020, 510, 145425. [Google Scholar] [CrossRef]
- Dupont, D.; Brullot, W.; Bloemen, M.; Verbiest, T.; Binnemans, K. Selective uptake of rare earths from aqueous solutions by EDTA-functionalized magnetic and nonmagnetic nanoparticles. ACS Appl. Mater. Interfaces 2014, 6, 4980–4988. [Google Scholar] [CrossRef] [PubMed]
- Magoling, B.J.A.; Macalalad, A.A. Optimization and response surface modelling of activated carbon production from Mahogany fruit husk for removal of chromium (VI) from aqueous solution. BioResources 2017, 12, 3001–3016. [Google Scholar] [CrossRef]
- Gil, M.; Martínez, M.; García, S.; Rubiera, F.; Pis, J.; Pevida, C. Response surface methodology as an efficient tool for optimizing carbon adsorbents for CO2 capture. Fuel Process. Technol. 2013, 106, 55–61. [Google Scholar] [CrossRef]
- Jafari, M.; Rahimi, M.R.; Ghaedi, M.; Dashtian, K. ZnO nanoparticles loaded different mesh size of porous activated carbon prepared from Pinus eldarica and its effects on simultaneous removal of dyes: Multivariate optimization. Chem. Eng. Res. Des. 2017, 125, 408–421. [Google Scholar] [CrossRef]
- Arami-Niya, A.; Daud, W.M.A.W.; Mjalli, F.S.; Abnisa, F.; Shafeeyan, M.S. Production of microporous palm shell based activated carbon for methane adsorption: Modeling and optimization using response surface methodology. Chem. Eng. Res. Des. 2012, 90, 776–784. [Google Scholar] [CrossRef]
- Hidayu, A.; Mohamad, N.; Matali, S.; Sharifah, A. Characterization of activated carbon prepared from oil palm empty fruit bunch using BET and FT-IR techniques. Procedia Eng. 2013, 68, 379–384. [Google Scholar] [CrossRef]
- Reddy, K.S.K.; Al Shoaibi, A.; Srinivasakannan, C. Activated carbon from date palm seed: Process optimization using response surface methodology. Waste Biomass-Valorization 2012, 3, 149–156. [Google Scholar] [CrossRef]
- Duan, X.; Peng, J.; Srinivasakannan, C.; Zhang, L.; Xia, H.; Yang, K.; Zhang, Z. Process optimization for the preparation of activated carbon from Jatropha hull using response surface methodology. Energy Sources Part A Recover. Util. Environ. Eff. 2011, 33, 2005–2017. [Google Scholar] [CrossRef]
- Tang, Y.-B.; Liu, Q.; Chen, F.-Y. Preparation and characterization of activated carbon from waste ramulus mori. Chem. Eng. J. 2012, 203, 19–24. [Google Scholar] [CrossRef]
- Yu, B.; Zhang, Y.; Shukla, A.; Shukla, S.S.; Dorris, K.L. The removal of heavy metals from aqueous solutions by sawdust adsorption—Removal of lead and comparison of its adsorption with copper. J. Hazard. Mater. 2001, 84, 83–94. [Google Scholar] [CrossRef] [PubMed]
- Imamoglu, M.; Tekir, O. Removal of copper (II) and lead (II) ions from aqueous solutions by adsorption on activated carbon from a new precursor hazelnut husks. Desalination 2008, 228, 108–113. [Google Scholar] [CrossRef]
- Zakir, M. Adsorption of lead (II) and copper (II) ions on rice husk activated carbon under sonication 1. Int. Symp. Chem. Bioprocess Eng. 2013. [Google Scholar]
- Shekinah, P.; Kadirvelu, K.; Kanmani, P.; Senthilkumar, P.; Subburam, V. Adsorption of lead (II) from aqueous solution by activated carbon prepared from Eichhornia. J. Chem. Technol. Biotechnol.: Int. Res. Process Environ. Clean Technol. 2002, 77, 458–464. [Google Scholar]
- Caccin, M.; Giorgi, M.; Giacobbo, F.; Da Ros, M.; Besozzi, L.; Mariani, M. Removal of lead (II) from aqueous solutions by adsorption onto activated carbons prepared from coconut shell. Desalination Water Treat. 2016, 57, 4557–4575. [Google Scholar] [CrossRef]
- Yavuz, Ö.; Guzel, R.; Aydin, F.; Tegin, I.; Ziyadanogullari, R. Removal of Cadmium and Lead from Aqueous Solution by Calcite. Pol. J. Environ. Stud. 2007, 16, 467–471. [Google Scholar]
- Shaikhiev, I.; Shaykhieva, K.; Sverguzova, S.; Fomina, E.; Vinogradenko, Y.; Fediuk, R.; Amran, M.; Svintsov, A.P.; de Azevedo, A.R.G.; Gunasekaran, M. Removing Pollutants from Sewage Waters with Ground Apricot Kernel Shell Material. Materials 2022, 15, 3428. [Google Scholar] [CrossRef]
- Rao, M.M.; Rao, G.C.; Seshaiah, K.; Choudary, N.; Wang, M. Activated carbon from Ceiba pentandra hulls, an agricultural waste, as an adsorbent in the removal of lead and zinc from aqueous solutions. Waste Manag. 2008, 28, 849–858. [Google Scholar] [CrossRef]
- Acharya, J.; Sahu, J.; Mohanty, C.; Meikap, B. Removal of lead(II) from wastewater by activated carbon developed from Tamarind wood by zinc chloride activation. Chem. Eng. J. 2009, 149, 249–262. [Google Scholar] [CrossRef]
- Lv, D.; Liu, Y.; Zhou, J.; Yang, K.; Lou, Z.; Baig, S.A.; Xu, X. Application of EDTA-functionalized bamboo activated carbon (BAC) for Pb(II) and Cu(II) removal from aqueous solutions. Appl. Surf. Sci. 2018, 428, 648–658. [Google Scholar] [CrossRef]
- Mariana, M.; HPS, A.K.; Mistar, E.; Yahya, E.B.; Alfatah, T.; Danish, M.; Amayreh, M. Recent advances in activated carbon modification techniques for enhanced heavy metal adsorption. J. Water Process Eng. 2021, 43, 102221. [Google Scholar] [CrossRef]
- Krishnamoorthy, R.; Govindan, B.; Banat, F.; Sagadevan, V.; Purushothaman, M.; Show, P.L. Date pits activated carbon for divalent lead ions removal. J. Biosci. Bioeng. 2019, 128, 88–97. [Google Scholar] [CrossRef]
- Patnukao, P.; Kongsuwan, A.; Pavasant, P. Batch studies of adsorption of copper and lead on activated carbon from Eucalyptus camaldulensis Dehn. bark. J. Environ. Sci. 2008, 20, 1028–1034. [Google Scholar] [CrossRef]
- Owamah, H.I. Biosorptive removal of Pb(II) and Cu(II) from wastewater using activated carbon from cassava peels. J. Mater. Cycles Waste Manag. 2014, 16, 347–358. [Google Scholar] [CrossRef]
- Ramutshatsha-Makhwedzha, D.; Mbaya, R.; Mavhungu, M.L. Application of Activated Carbon Banana Peel Coated with Al2O3-Chitosan for the Adsorptive Removal of Lead and Cadmium from Wastewater. Materials 2022, 15, 860. [Google Scholar] [CrossRef]





| Coexisting Ions | Concentration (mg L−1) |
|---|---|
| Na+, K+, Cl− | >10,000 |
| Mg2+, Ca2+, Fe3+, | 6000 |
| Al3+, Fe2+, Co2+, Ni2+, Cd2+, Zn2+, | 5000 |
| Cr3+, PO43−, SO42− | 2000 |
| F−, CO32− | 500 |
| Analytical Parameters | |
|---|---|
| Linear range (µg L−1) | 0.84–1000 |
| Correlation coefficient | 0.996 |
| Enhancement factor | 130 |
| Extraction recovery (%) | 95 |
| Calibration equation | y = 0.002x |
| LOD (µg L−1) | 0.25 |
| LOQ (µg L−1) | 0.84 |
| RSDr (n = 6) | <3.0 |
| RSDR (n = 10) | <7.5 |
| Sample | Added Amount (mg) | Found Amount (mg) | % R † |
|---|---|---|---|
| Tap water | 0 | BDL | - |
| 0.20 | 0.19 | 99.0 ± 0.78 | |
| 0.40 | 0.39 | 97.8 ± 0.93 | |
| 0.80 | 0.79 | 98.5 ± 1.01 | |
| 1.60 | 1.54 | 96.4 ± 1.12 | |
| Wastewater | 0 | 0.01 | - |
| 0.20 | 0.20 | 96.5 ± 2.4 | |
| 0.40 | 0.41 | 99.2 ± 0.6 | |
| 0.80 | 0.79 | 98.1 ± 1.0 | |
| 1.60 | 1.48 | 91.6 ± 2.7 | |
| Milk powder | 0 | BDL | - |
| 0.20 | 0.20 | 101.0 ± 1.4 | |
| 0.40 | 0.39 | 97.2 ± 1.8 | |
| 0.80 | 0.78 | 99.9 ± 1.4 | |
| 1.60 | 1.47 | 91.6 ± 2.1 | |
| Wheat flour | 0 | BDL | - |
| 0.20 | 0.19 | 98.5 ± 0.9 | |
| 0.40 | 0.39 | 97.8 ± 1.5 | |
| 0.80 | 0.79 | 98.5 ± 1.1 | |
| 1.60 | 1.54 | 96.4 ± 3.1 | |
| Certified reference drinking water (ERM-CA011a) (µg L−1) | |||
| Certified concentration | Found concentration | % Recovery | |
| 24.5 * | 24.3 ± 0.7 | 99.2 ± 2.9 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ahmad, T.; Shah, F.; Khan, R.A.; Ahmed, A.Y. Utilization of Multivariate Optimization for Preconcentration and Determination of Lead in Different Water and Food Samples Using Functionalized Activated Carbon. Water 2023, 15, 3750. https://doi.org/10.3390/w15213750
Ahmad T, Shah F, Khan RA, Ahmed AY. Utilization of Multivariate Optimization for Preconcentration and Determination of Lead in Different Water and Food Samples Using Functionalized Activated Carbon. Water. 2023; 15(21):3750. https://doi.org/10.3390/w15213750
Chicago/Turabian StyleAhmad, Tabinda, Faheem Shah, Rafaqat Ali Khan, and Amel Y. Ahmed. 2023. "Utilization of Multivariate Optimization for Preconcentration and Determination of Lead in Different Water and Food Samples Using Functionalized Activated Carbon" Water 15, no. 21: 3750. https://doi.org/10.3390/w15213750





