Comparison of Velocity and Reynolds Stress Distributions in a Straight Rectangular Channel with Submerged and Emergent Vegetation
Abstract
1. Introduction
2. Materials and Methods
3. Results
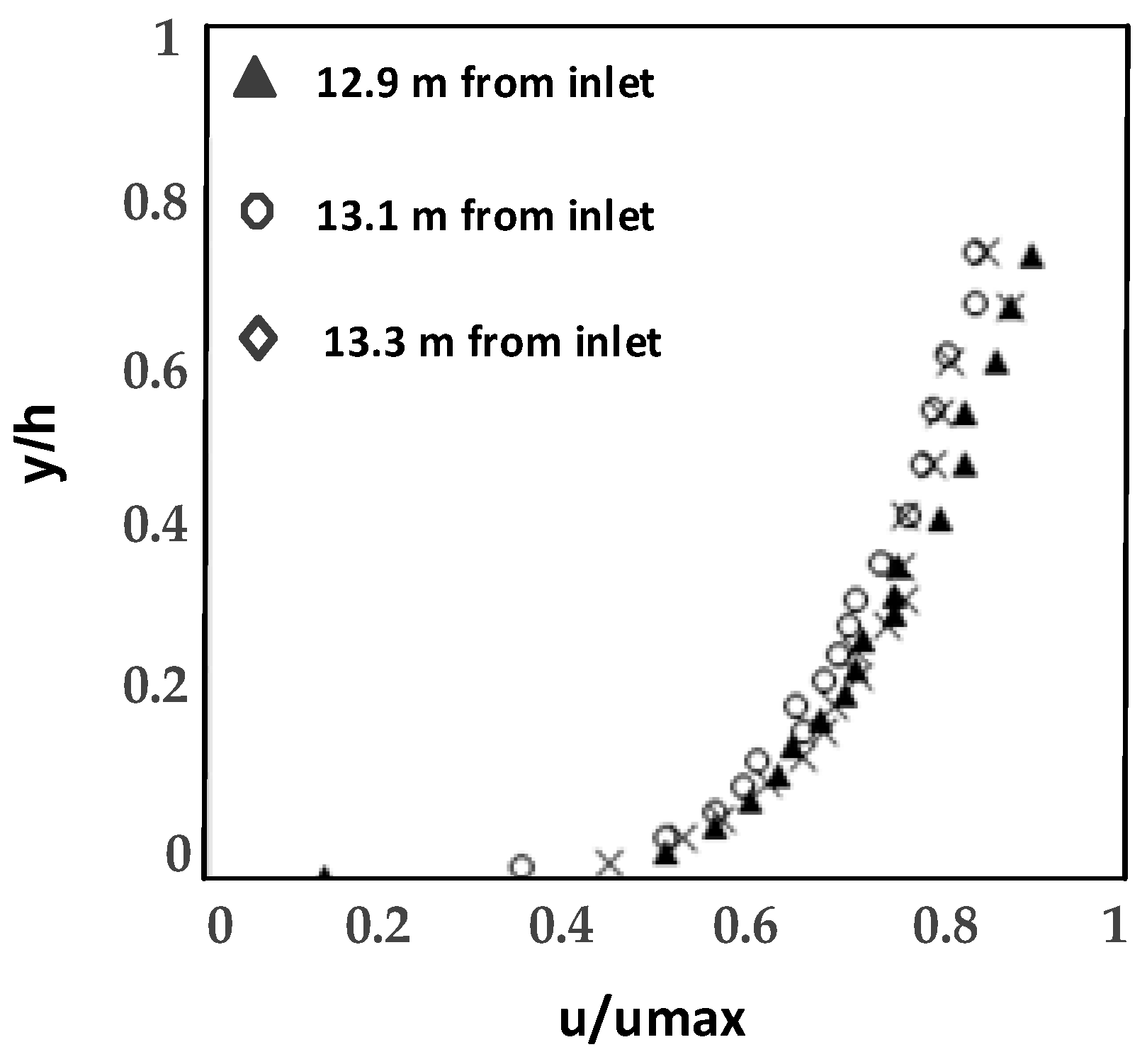
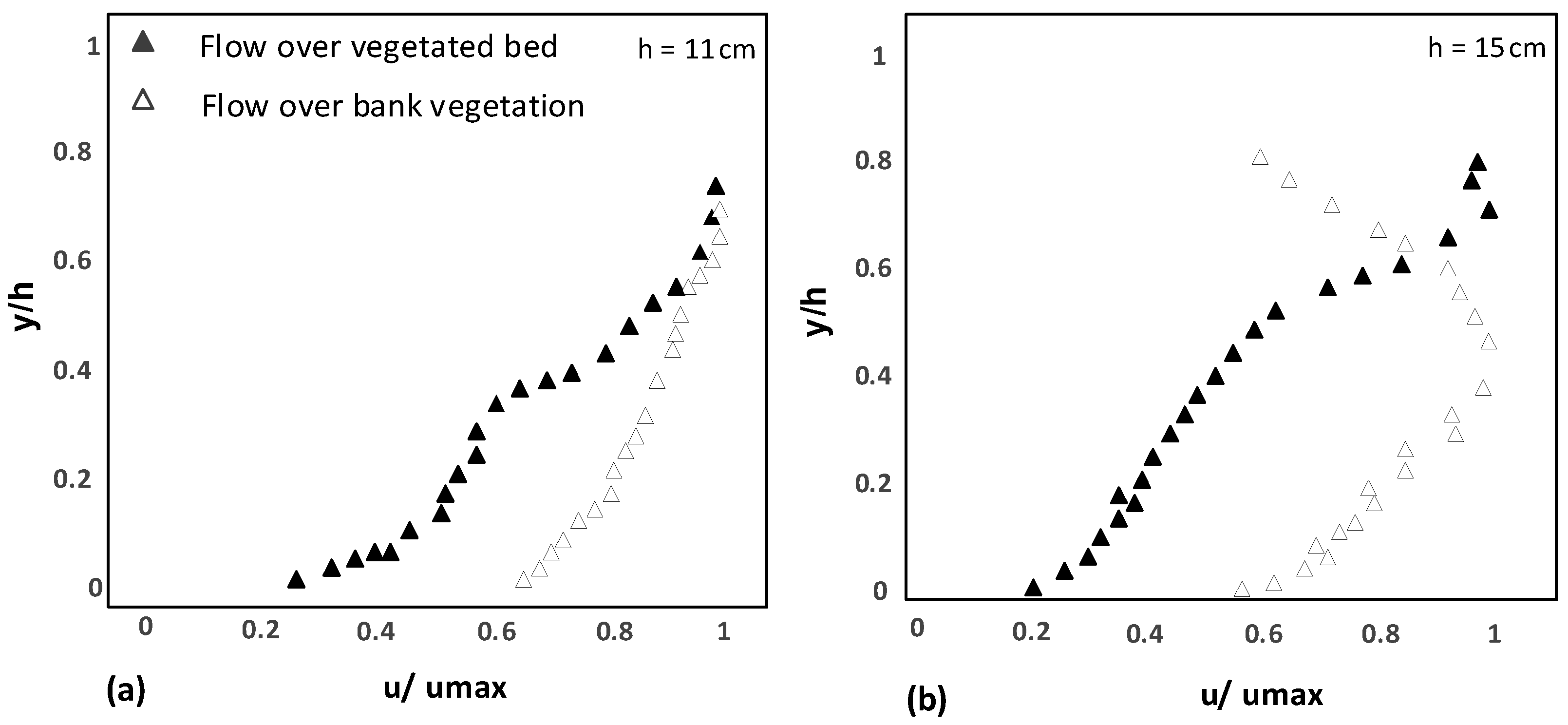
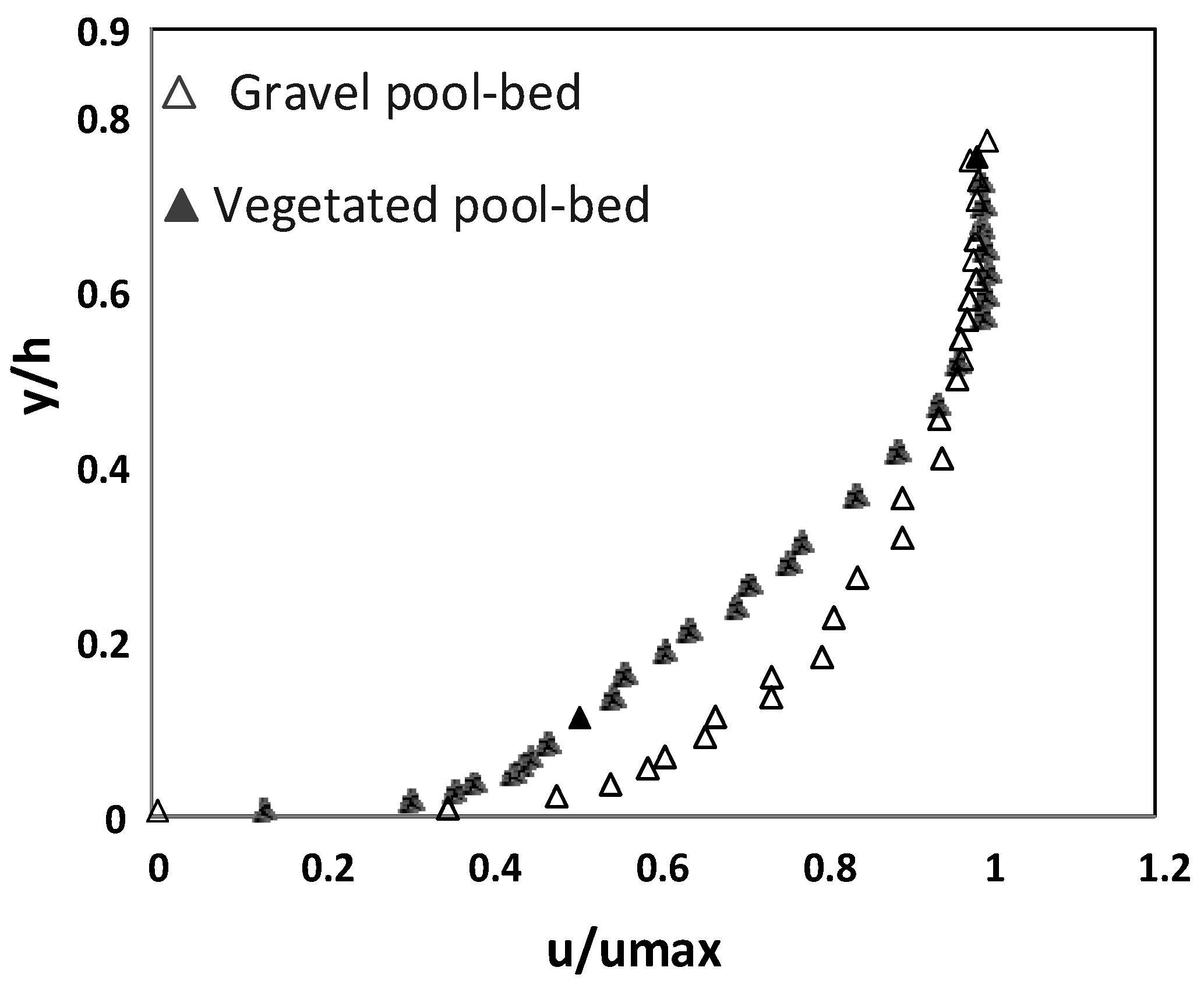
3.1. Comparison of Velocity Distributions for Submerged and Emergent Vegetation Covers
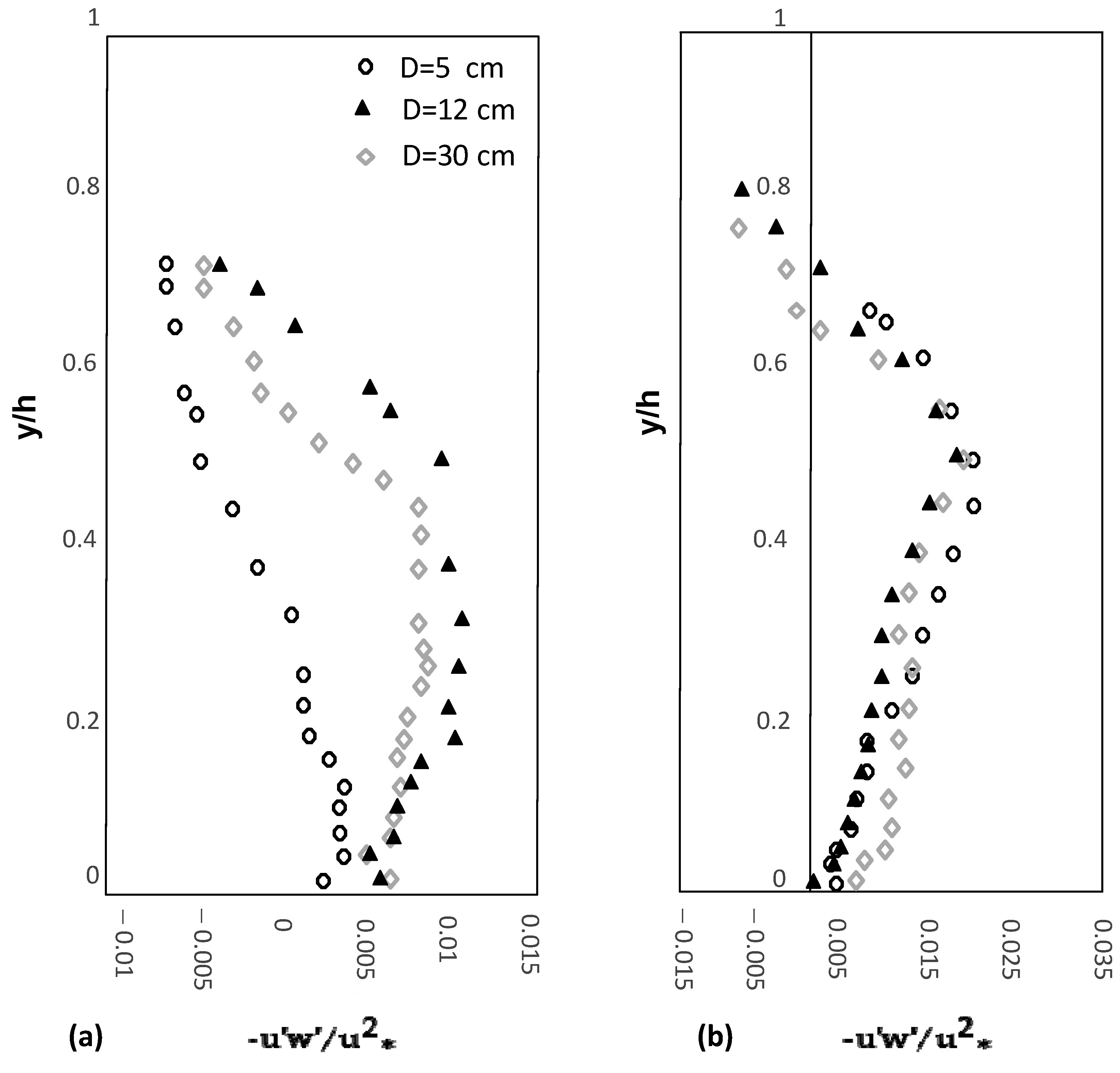
3.2. Comparison of Reynolds Shear Stress for Submerged and Emergent Vegetation
4. Discussion
5. Conclusions
- In emergent vegetation, the velocity distribution is influenced by a small aspect ratio (W/h < 5) forcing the maximum velocity to move towards the bed. However, in the submerged vegetation, the velocity distribution is less influenced by the aspect ratio, showing the location of maximum velocity far from the bed.
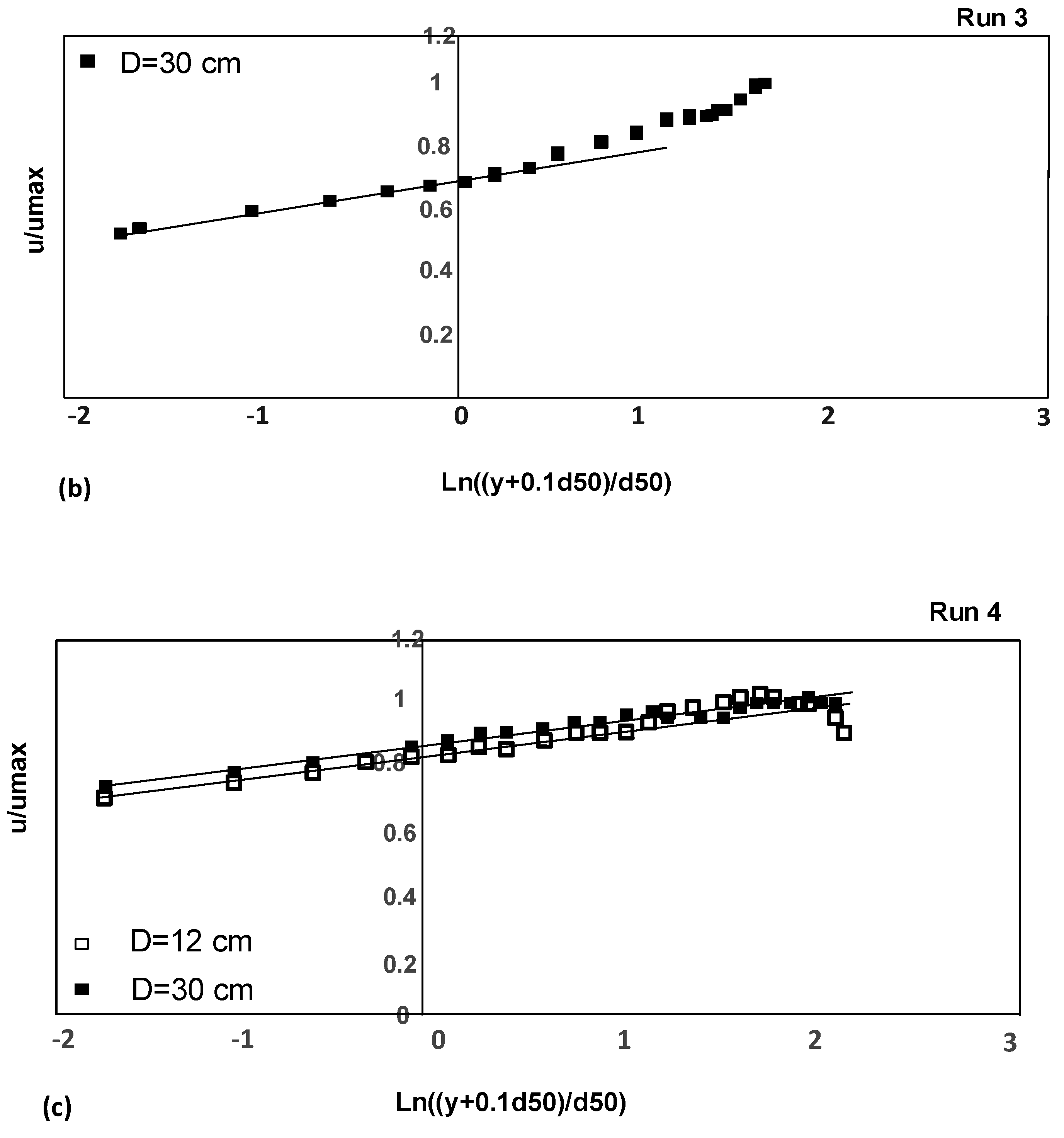
- The log law is valid up to y/h = 0.23 for the flow over submerged vegetation in different aspect ratios. However, for emergent vegetation, the validation zone depends on the distance from the vegetation bank; at small aspect ratio and the central axis of the flume, this law is valid up to y/h = 0.75.
- Reynolds stress (RS) distributions show a convex form for submerged and emergent vegetation covers at different aspect ratios. However, approaching the bank vegetation (emergent case), the power of secondary currents increases, forcing the maximum RS shifts towards the bed.
- For the aspect ratio of W/h = 4 and flow over submerged vegetation, the location of zero shear stress superposes that of maximum velocity. However, in emergent vegetation, such a superposition is not considered. Further, approaching the bank vegetation and shifting the maximum velocity towards the bed, the location of zero shear stress approaches the bed.
- The results of this research show that estimation of key parameters of fluvial projects, velocity and Reynolds stress is influenced by the vegetation arrangements, submerged or emergent. Therefore, a better estimation of these parameters influences drag coefficient and sediment determinations, improving bank stability with reasonable channel design, reducing the cost of project and modifying the input data for hydraulic models.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| d50 | Median diameter of sediment particles |
| C | Constant of the log law |
| D | Distance from the wall |
| yp | Plant height (for run 1 and 2) |
| h | Water depth above the vegetation (for run 1 and run 2) and water depth over gravel bed (for run 3 and run 4) |
| W | Flume width |
| ks | Roughness scale (equivalent sand roughness) |
| Q | Flow discharge |
| u | Mean point velocity |
| uave | Average velocity at a section; |
| umax | Maximum velocity |
| u’ | Turbulence intensity in longitudinal direction |
| w’ | Turbulence intensity in vertical direction |
| u* | Shear velocity |
| y | Distance from top of gravel or vegetation cover |
| Y | Distance from the bed |
| κ | von Karman constant |
References
- Shi, H.; Huai, W. Flow structure of open channel flow with partly submerged vegetation. In Proceedings of the 12th International Symposium on Ecohydraulics, Tokyo, Japan, 19–24 August 2018. [Google Scholar]
- Parvizi, P.; Afzalimehr, H.; Sui, J.; Raeisifar, H.R.; Eftekhari, A.R. Characteristics of Shallow Flows in a Vegetated Pool—An Experimental Study. Water 2023, 15, 205. [Google Scholar] [CrossRef]
- Barahimi, M.; Sui, J. Effects of Submerged Vegetation Arrangement Patterns and Density on Flow Structure. Water 2023, 15, 176. [Google Scholar] [CrossRef]
- Soltani, N.; Afzalimehr, H.; Shahiri Tabarestani, E.; Eftekhari, A.; Khabari, M.; Nazari-Sharabian, M.; Karakouzian, M. Turbulence Characteristics in Mild and Steep Entrance Slopes of Pool-Riffle Sequences. Water 2023, 15, 720. [Google Scholar] [CrossRef]
- Kazem, M.; Afzalimehr, H.; Sui, J. Characteristics of Turbulence in the Downstream Region of a Vegetation Patch. Water 2021, 13, 3468. [Google Scholar] [CrossRef]
- Sohrabi, S.; Afzalimehr, H.; Singh, V.P. Estimation of drag coefficient of emergent and submerged vegetation patches with various densities and arrangements in open channel flow. ISH J. Hydraul. Eng. 2022, 29, 297–307. [Google Scholar] [CrossRef]
- Hopkinson, L.; Wynn, T. Vegetation impacts on near bank flow. Ecohydrol Ecosyss Land Water Process. Interact. Ecohydrogeomorpho. 2009, 2, 404–418. [Google Scholar] [CrossRef]
- Bernhardt, E.S.; Palmer, M.A.; Allan, J.D.; Alexander, G.; Barnas, K.; Brooks, S.; Carr, J.; Clayton, S.; Dahm, C.; Follstad-Shah, J.; et al. Synthesizing U.S. river restoration efforts. Science 2005, 308, 636–637. [Google Scholar] [CrossRef] [PubMed]
- Aberle, J.; Järvelä, J. Flow resistance of emergent rigid and flexible floodplain vegetation. J. Hydraul. Res. 2013, 51, 33–45. [Google Scholar] [CrossRef]
- King, A.T.; Tinoco, R.O.; Cowen, E.A. A k–ε turbulence model based on the scales of vertical shear and stem wakes valid for emergent and submerged vegetated flows. J. Fluid Mech. 2012, 701, 1–39. [Google Scholar] [CrossRef]
- Yagci, O.; Kabdasli, M. The impact of single natural vegetation elements on flow characteristics. Hydrol. Process. 2008, 22, 4310–4321. [Google Scholar] [CrossRef]
- Järvelä, J. Effect of submerged flexible vegetation on flow structure and resistance. J. Hydrol. 2005, 307, 233–241. [Google Scholar] [CrossRef]
- Thorne., C.R. Effects of vegetation on riverbank erosion and stability. In Vegetation and Erosion; Wiley: Chichester, UK, 1990. [Google Scholar]
- Huai, W.X.; Zeng, Y.H.; Xu, Z.G.; Yang, Z.H. Three-layer model for vertical velocity distribution in open channel flow with submerged rigid vegetation. Adv. Water. Res. 2009, 32, 487–492. [Google Scholar] [CrossRef]
- Stephan, U.; Gutknecht, D. Hydraulic resistance of submerged flexible vegetation. J. Hydrol. 2002, 269, 27–43. [Google Scholar] [CrossRef]
- Kummu, M. Roughness Characteristics and Velocity Profile in Vegetated and Nonvegetated Channel. Master’s Thesis, Aalto University, Espoo, The Netherland, 2002. [Google Scholar]
- Shucksmith, J.D.; Boxall, J.B.; Guymer, I. Effects of emergent and submerged natural vegetation on longitudinal mixing in open channel flow. Water Resour. Res. 2010, 46, W04504. [Google Scholar] [CrossRef]
- Kumar, P.; Sharma, A. Experimental investigation of 3D flow properties around emergent rigid vegetation. Ecohydrology 2022, 15, e2474. [Google Scholar] [CrossRef]
- Afzalimehr, H.; Dey, S. Influence of bank vegetation and gravel bed on velocity and Reynolds stress distributions. Int. J. Sediment. Res. 2009, 24, 236–246. [Google Scholar] [CrossRef]
- Setayesh, P.; Afzalimehr, H. Effect of Reedy Emergent Side-Vegetation in Gravel-Bed Streams on Bed Shear Stress: Patch Scale Analysis. Iran. J. Sci. Technol. Trans. Civ. Eng. 2021, 46, 1375–1392. [Google Scholar] [CrossRef]
- Afzalimehr, H.; Sui, J.; Moghbel, R. Hydraulic parameters in channels with wall vegetation and gravel bed. Int. J. Sediment Res. 2010, 25, 81–90. [Google Scholar] [CrossRef]
- Nezu, I.; Nakagawa, H. Turbulent in open-channel flow. In IAHR MonographSeries; Balkema: Rotterdam, The Netherlands, 1993. [Google Scholar]
- Graf, W.H.; Altinakar, M.S. Fluvial Hydraulics: Flow and Transport Processes in Channels of Simple Geometry; Willey: New York, NY, USA, 1998. [Google Scholar]
- Hadian, S.; Afzalimehr, H.; Ahmad, S. Effects of Channel Width Variations on Turbulent Flow Structures in the Presence of Three-Dimensional Pool-Riffle. Sustainability 2023, 15, 7829. [Google Scholar] [CrossRef]
- Carollo, F.G.; Ferro, V.; Termini, D. Flow Velocity Measurements in Vegetated Channels. J. Hydraul. Eng. 2002, 128, 664–673. [Google Scholar] [CrossRef]
- Nepf, H.M.; Vivoni, E. Flow structure in depth-limited, vegetated flow. J. Geophys. Res. Oceans. 2000, 105, 28547–28557. [Google Scholar] [CrossRef]
- Velasco, D.; Bateman, A.; Redondo, J.M.; Medina, V. An Open Channel Flow Experimental and Theoretical Study of Resistance and Turbulent Characterization over Flexible Vegetated Linings. Flow Turbul. Combust. 2003, 70, 69–88. [Google Scholar] [CrossRef]
- Yang, S.-Q.; Xu, W.-L.; Yu, G.-L. Velocity distribution in a gradually accelerating free surface flow. Adv. Water Resour. 2006, 29, 1969–1980. [Google Scholar] [CrossRef]
- Nepf, H.M. Hydrodynamics of vegetated channels. J. Hydraul. Res. 2012, 50, 262–279. [Google Scholar] [CrossRef]
- Wang, J.; He, G.; Dey, S.; Fang, H. Influence of submerged flexible vegetation on turbulence in an open-channel flow. J. Fluid Mech. 2022, 947, A31. [Google Scholar] [CrossRef]
- Julien, P.Y. Erosion and Sedimentation; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Afzalimehr, H. Effect of non-uniformity of flow on velocity and turbulence intensities over a cobble-bed. Hydrol. Process. Int. J. 2009, 24, 331–341. [Google Scholar] [CrossRef]
- Simons, D.B.; Şentürk, F. Sediment Transport Technology: Water and Sediment Dynamics; Water Resources Publication: Littleton, CO, USA, 1992. [Google Scholar]
- Song, T.; Chiew, Y. Turbulence measurement in nonuniform open-channel flow using acoustic Doppler velocimeter (ADV). J. Eng. Mech. 2001, 127, 219–232. [Google Scholar] [CrossRef]
- Afzalimehr, H.; Rennie, C.D. Determination of bed shear stress in gravel-bed rivers using boundary-layer parameters. Hydrologic. Sci. J. 2009, 54, 147–159. [Google Scholar] [CrossRef]
- Afzalimehr, H.; Anctil, F. Velocity distribution and shear velocity behavior of decelerating flow over a gravel- bed. J. Can. Civ. Eng. 1999, 26, 468–475. [Google Scholar] [CrossRef]
- Wang, Y. Reynolds stress model for viscoelastic drag-reducing flow induced by polymer solution. Polymers 2019, 11, 1659. [Google Scholar] [CrossRef]
- Afzalimehr, H.; Moghbel, R.; Ghalichand, J.; Sui, J. Investigation of Turbulence characteristics in channel with dense vegetation over bed. Int. J. Sediment Res. 2011, 26, 255–268. [Google Scholar] [CrossRef]
- Yalin, M.S. Mechanics of Sediment Transport, 2nd ed.; Pergamon: New York, NY, USA, 1992. [Google Scholar]
- Vanoni, V. Sedimentation Engineering; ASCE Publications: Reston, VA, USA, 1975. [Google Scholar]


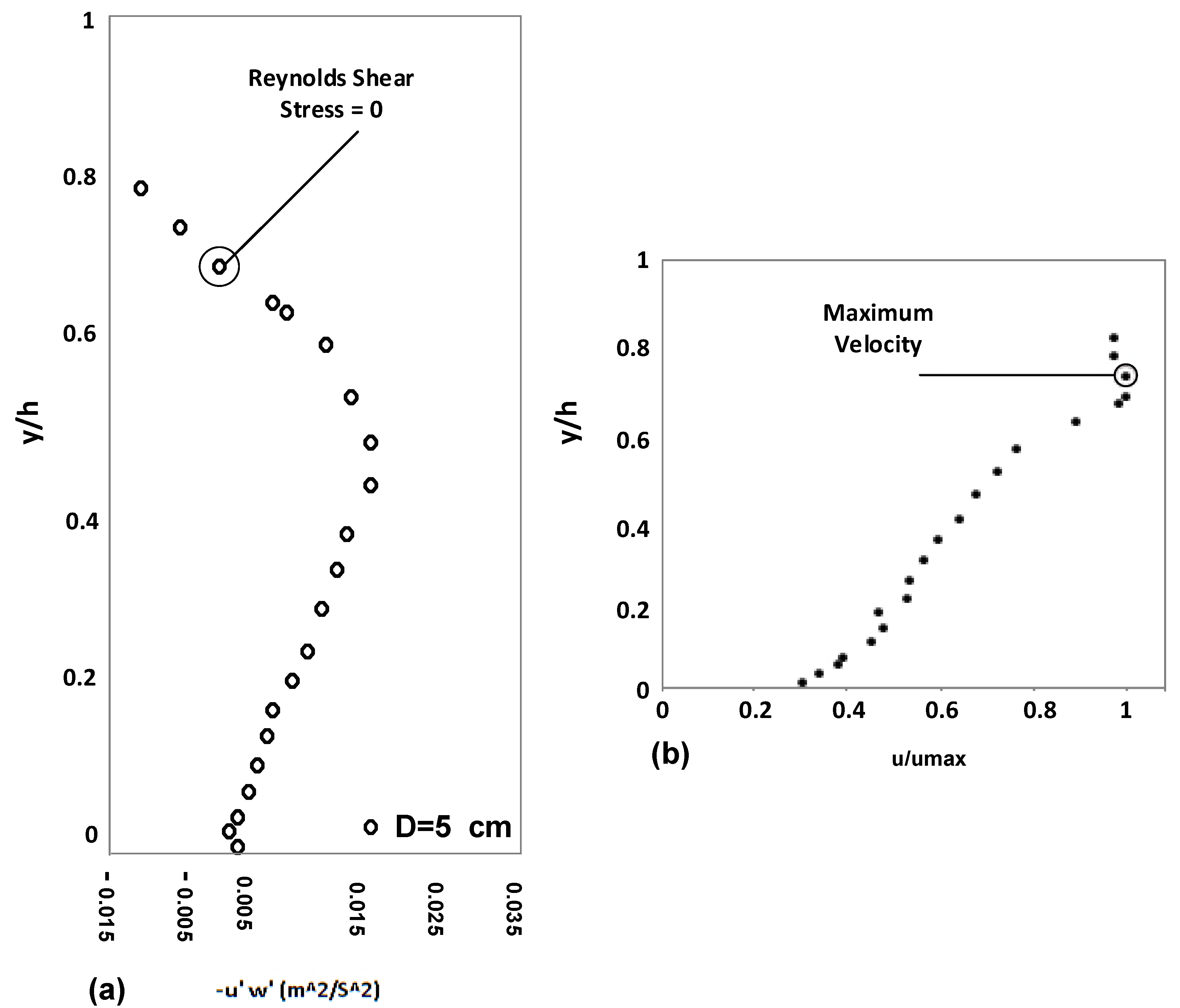

| Exp. | Q (m3/s) | h (cm) | W (cm) | Uavg (m/s) | W/h | Covered Area |
|---|---|---|---|---|---|---|
| Run 1 | 0.037 | 11 | 60 | 0.56 | 5.45 | Bed |
| Run 2 | 0.055 | 15 | 60 | 0.61 | 4 | Bed |
| Run 3 | 0.037 | 11 | 60 | 0.56 | 5.45 | Bank |
| Run 4 | 0.055 | 15 | 60 | 0.61 | 4 | Bank |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mofrad, M.R.T.; Afzalimehr, H.; Parvizi, P.; Ahmad, S. Comparison of Velocity and Reynolds Stress Distributions in a Straight Rectangular Channel with Submerged and Emergent Vegetation. Water 2023, 15, 2435. https://doi.org/10.3390/w15132435
Mofrad MRT, Afzalimehr H, Parvizi P, Ahmad S. Comparison of Velocity and Reynolds Stress Distributions in a Straight Rectangular Channel with Submerged and Emergent Vegetation. Water. 2023; 15(13):2435. https://doi.org/10.3390/w15132435
Chicago/Turabian StyleMofrad, Mohammad Reza Tabesh, Hossein Afzalimehr, Parsa Parvizi, and Sajjad Ahmad. 2023. "Comparison of Velocity and Reynolds Stress Distributions in a Straight Rectangular Channel with Submerged and Emergent Vegetation" Water 15, no. 13: 2435. https://doi.org/10.3390/w15132435
APA StyleMofrad, M. R. T., Afzalimehr, H., Parvizi, P., & Ahmad, S. (2023). Comparison of Velocity and Reynolds Stress Distributions in a Straight Rectangular Channel with Submerged and Emergent Vegetation. Water, 15(13), 2435. https://doi.org/10.3390/w15132435







