Collaborative Ecological Flow Decision Making under the Bengbu Sluice Based on Ecological-Economic Objectives
Abstract
:1. Introduction
2. Materials and Methods
2.1. Habitat Simulation Method
- (1)
- Selection of indicator fish and mapping of habitat suitability curves for fish. The habitat suitability index (HSI) was associated with habitat parameters, including water depth, flow rate, water temperature, and substrate, of a typical fish species in the study area. An HSI value of 1 indicated that the fish species was suitable for survival, whereas a value of 0 indicated that the fish species was unsuitable for survival.
- (2)
- Hydrodynamic model. Water depth and flow velocity distributions of the studied river sections were simulated using the MIKE21 model. For more details about the model, please refer to [20].
- (3)
- Habitat simulation. The habitat simulation method emphasizes estimating the weighted usable area (WUA) of a habitat. The equation of WUA is expressed as follows [21]:
2.2. Hydrological Method
- (1)
- Improved Tennant method. The minimal, optimal, and maximal ecological flows for each month were determined to be 10%, 60%, and 200% of the average monthly flow over several years, respectively.
- (2)
- Improved monthly frequency calculation method. With the improved Tennant method as a reference, the guaranteed rates for the three periods of abundance, flatness, and depletion were computed using a trial-and-error approach to obtain the guaranteed rates that best fit the study area and determine the optimal ecological flow in this area. The maximum and minimum ecological flows in the study area were determined using the same algorithm.
- (3)
- Improved monthly minimum method. To protect the safety of aquatic ecosystems, the sub-minimum and sub-maximum monthly runoff values were selected as the minimum and maximum ecological flows, respectively.
2.3. Multi-Objective Collaborative Decision Model
2.3.1. Computation of the Synergy Contribution Function
- (1)
- is the objective function for which a high index value is preferable;
- (2)
- is the objective function for which a small value of the index is preferable;
2.3.2. Computation of the Synergistic Contribution Replacement Rate
2.3.3. Computation of the System Coordination Degree Function
2.3.4. Computation of Ecological Objectives
2.3.5. Computation of Economic Objectives
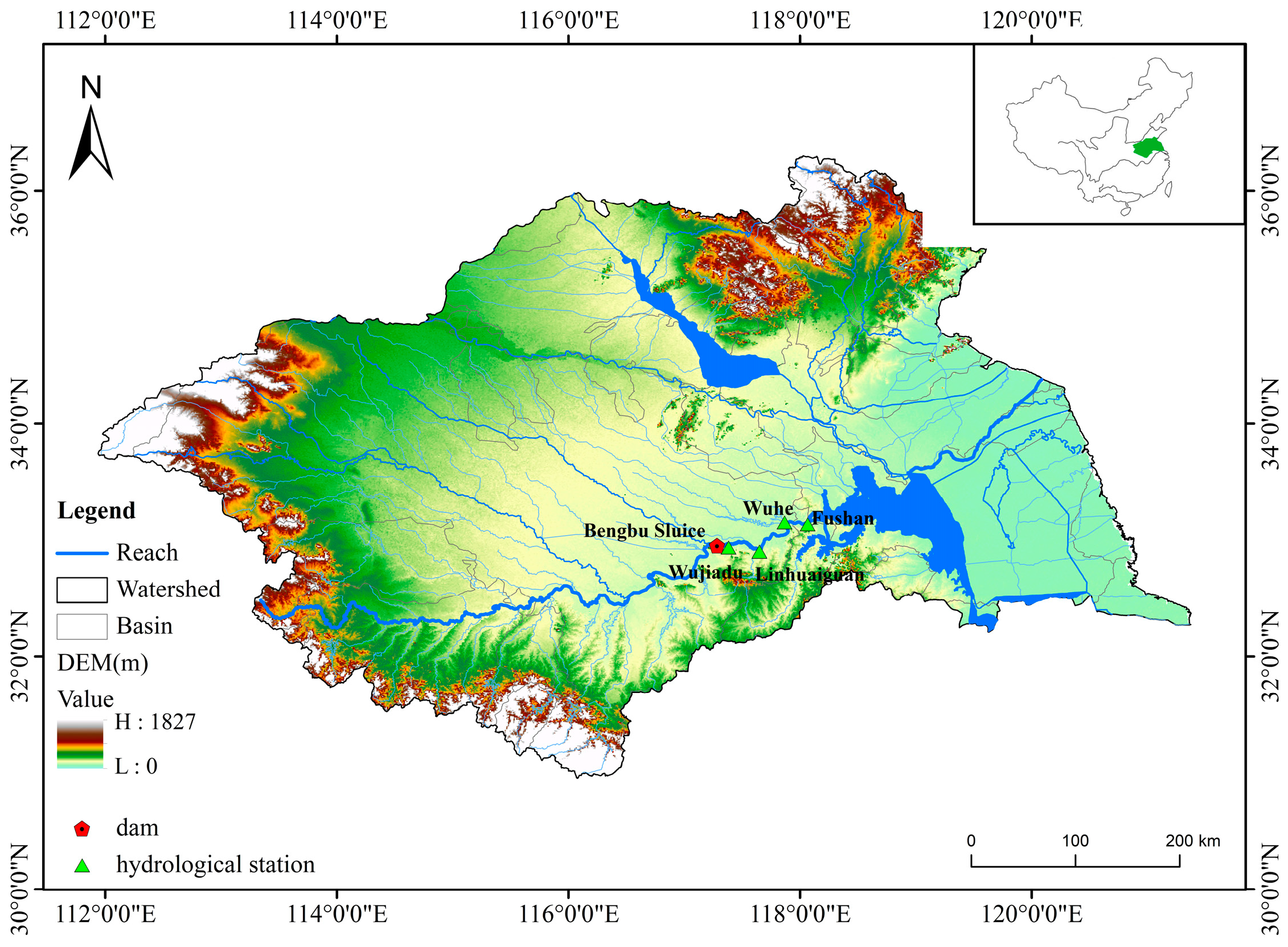
2.4. Study Area
3. Results and Discussion
3.1. Computation Results of Habitat Simulation Method
3.2. Comparison of Computation Results of Habitat Simulation and Hydrological Methods and Selection of Optimal Solution
3.3. Multi-Objective Analysis of Ecological Flow Control Based on Synergy Theory
3.4. Evaluation of Ecological Operation
- (1)
- Optimized scheduling to achieve an ecological flow rate guarantee. According to the incoming water from upstream, the river channel storage capacity, and the future weather conditions predicted by relevant software, the opening and closing of the barrage gates should be optimized to ensure downstream biological flow.
- (2)
- Implementation of a policy for monitoring and early warning. By monitoring the flow in the Wujiadu section, the ecological health of the river may be maintained when the flow value exceeds 120% of the downstream ecological flow value. When the observed flow value is less than the predetermined ecological flow value with a continuous downward trend, an early warning is required to enhance the downstream flow. When the flow in the monitoring section exceeds the established ecological flow value and does not decrease within three days, the alert can be discontinued.
- (3)
- Development of non-engineering measures. (i) Optimize the discharge process and regulate water consumption by collecting data on precipitation, flow rates, and water consumption to optimize the discharge process and water allocation. (ii) Develop a water withdrawal restriction plan to regulate upstream water consumption when downstream water volume exceeds the maximum warning. (iii) Establish an emergency response system when faced with unforeseen water conditions or an abnormally dry year. A plan for emergency upstream and downstream dispatching should be developed and implemented.
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhao, Y.J.; Ding, A.Z.; Sheng, F.X.; Wei, K.M. On theory of river function. J. Beijing Norm. Univ. Nat. Sci. 2013, 49, 68–74. Available online: https://kns.cnki.net/kcms/detail/detail.aspx?FileName=BSDZ201301015&DbName=CJFQ2013 (accessed on 15 December 2013).
- Li, H.L.; Chen, J.; Jin, Q.; Chen, L.F.; Xu, Y.F. Study on influence of sluice size on hydrodynamic and water environment of river channels. Yangtze River 2019, 50, 181–185+196. [Google Scholar] [CrossRef]
- Zuo, Q.T.; Chen, H.; Zhang, Q.Y. Impact factors and health assessment of aquatic ecosystem in Upper and Middle Huai River Basin. J. Hydraul. Eng. 2015, 46, 1019–1027. [Google Scholar] [CrossRef]
- Yao, W.W.; Chen, Y.S.; Zhong, Y.; Zhang, W.Y.; Fan, H.Y. Habitat models for assessing river ecosystems and their application to the development of river restoration strategies. J. Freshw. Ecol. 2017, 32, 601–617. [Google Scholar] [CrossRef] [Green Version]
- Damiani, M.; Núñez, M.; Roux, P.; Loiseau, E.; Rosenbaum, R.K. Addressing water needs of freshwater ecosystems in life cycle impact assessment of water consumption: State of the art and applicability of eco-hydrological approaches to ecosystem quality characterization. Int. J. Life Cycle Assess. 2018, 23, 2071–2208. [Google Scholar] [CrossRef]
- Tharme, R.E. A global perspective on environmental flow assessment: Emerging trends in the development and application of environmental flow methodologies for rivers. River Res. Appl. 2013, 19, 397–441. [Google Scholar] [CrossRef]
- Chen, Q.; Wang, Q.; Li, Z.; Li, R. Uncertainty analyses on the calculation of water environmental capacity by an innovative holistic method and its application to the Dongjiang River. J. Environ. Sci. 2014, 6, 1783–1790. [Google Scholar] [CrossRef]
- Karimi, S.; Salarijazi, M.; Ghorbani, K.; Heydari, M. Comparative assessment of environmental flow using hydrological methods of low flow indexes, Smakhtin, Tennant and flow duration curve. Acta Geophys. 2021, 69, 285–293. [Google Scholar] [CrossRef]
- Theiling, C.H.; Nestler, J.M. River stage response to alteration of Upper Mississippi River channels, floodplains, and watersheds. Hydrobiologia 2010, 640, 17–47. [Google Scholar] [CrossRef]
- Sedighkia, M.; Datta, B.; Abdoli, A. Utilizing classic evolutionary algorithms to assess the Brown trout (Salmo trutta) habitats by ANFIS-based physical habitat model. Model. Earth Syst. Environ. 2022, 8, 857–869. [Google Scholar] [CrossRef]
- Wang, Y.; Xia, Z.Q.; Wang, D. Assessing the effect of Separation Levee Project on Chinese sturgeon (Acipensor sinensis) spawning habitat suitability in Yangtze River, China. Aquat. Ecol. 2011, 45, 255–266. [Google Scholar] [CrossRef]
- Cheng, K.; Fu, Q.; Chen, X.; Li, T.X.; Jiang, Q.X.; Ma, X.S.; Zhao, K. Adaptive Allocation Modeling for a Complex System of Regional Water and Land Resources Based on Information Entropy and its Application. Water Resour. Manag. 2015, 29, 4977–4993. [Google Scholar] [CrossRef]
- Williams, K. Management Models and Industrial Applications of Linear Programming. J. Oper. Res. Soc. 1962, 13, 274–275. [Google Scholar] [CrossRef]
- Ye, Y. Approximating quadratic programming with bound and quadratic constraints. Math. Program. 1999, 84, 219–226. [Google Scholar] [CrossRef]
- Zomorodian, M.; Lai, S.H.; Homayounfar, M. Development and application of coupled system dynamics and game theory: A dynamic water conflict resolution method. PLoS ONE 2017, 12, e0188489. [Google Scholar] [CrossRef] [Green Version]
- Atef, S.S.; Sadeqinazhad, F.; Farjaad, F. Water conflict management and cooperation between Afghanistan and Pakistan. J. Hydrol. 2019, 570, 875–892. [Google Scholar] [CrossRef]
- Almazán-Gómez, M.Á.; Sánchez-Chóliz, J.; Sarasa, C. Environmental flow management: An analysis applied to the Ebro River Basin. J. Clean. Prod. 2018, 182, 838–851. [Google Scholar] [CrossRef] [Green Version]
- Bhatti, E.; Khan, M.M.; Shah, S.A.R.; Raza, S.S.; Shoaib, M.; Adnan, M. Dynamics of water quality: Impact assessment process for water resource management. Processes 2019, 7, 102. [Google Scholar] [CrossRef] [Green Version]
- Cheng, K.; Yao, J.P.; Ren, Y.T. Evaluation of the coordinated development of regional water resource systems based on a dynamic coupling coordination model. Water Supply 2019, 19, 565–573. [Google Scholar] [CrossRef]
- Ahn, J.; Na, Y.; Park, S.W. Development of Two-Dimensional Inundation Modelling Process using MIKE21 Model. KSCE J. Civ. Eng. 2019, 23, 3968–3977. [Google Scholar] [CrossRef]
- Yi, Y.J.; Wang, Z.Y.; Yang, Z.F. Impact of the Gezhouba and Three Gorges Dams on habitat suitability of carps in the Yangtze River. J. Hydrol. 2010, 387, 283–291. [Google Scholar] [CrossRef]
- Liao, S.H.; Cheng, X.S.; Shi, Y.; Ma, Z.Z.; Zhao, J.S.; Wang, Z.J. Study on ecological water use of Huaihe River with multi-level analysis platform and multi-objective optimization model. J. Hydroelectr. Eng. 2010, 29, 14–19+27. [Google Scholar]
- Wang, L. Method for Estimating Ecological Hydrograph of Plain River with Series of Dams and Gates. Ph.D. Thesis, Hohai University, Nanjing, China, 2017. [Google Scholar]
- Zhang, H.; Zhang, S.M.; Cui, X.S.; Yang, S.L.; Hua, C.J.; Ma, H.Y. Spatio-temporal dynamics in the location of the fishing grounds and catch per unit effort (CPUE) for Chilean jack mackerel (Trachurus murphyi Nichols, 1920) from Chinese trawl fleets on the high seas of the Southeast Pacific Ocean, 2001–2010. J. Appl. Ichthyol. 2015, 31, 646–656. [Google Scholar] [CrossRef]









| Level | Value | Evaluation | Significance |
|---|---|---|---|
| I | 0–0.25 | Unsuitable | Habitat ecology was damaged and unsuitable for habitat |
| II | 0.25–0.50 | Less suitable | Habitat was usually destroyed and unable to maintain essential functions post-disturbance |
| III | 0.50–0.75 | Suitable | Habitat ecological functions were relatively well developed and could be restored post-disturbance |
| IV | 0.75–1.00 | Optimal | The habitat ecological function was perfect and had strong restoration ability |
| Station | CC 1 | NSE 2 | MRE 3 |
|---|---|---|---|
| Linhuaiguan | 0.999 | 0.991 | 1.303‰ |
| Wuhe | 0.999 | 0.996 | 2.824‰ |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pei, Y.; Lu, B.; Song, Y.; Yang, Y.; Feng, X.; Shen, W. Collaborative Ecological Flow Decision Making under the Bengbu Sluice Based on Ecological-Economic Objectives. Water 2022, 14, 4133. https://doi.org/10.3390/w14244133
Pei Y, Lu B, Song Y, Yang Y, Feng X, Shen W. Collaborative Ecological Flow Decision Making under the Bengbu Sluice Based on Ecological-Economic Objectives. Water. 2022; 14(24):4133. https://doi.org/10.3390/w14244133
Chicago/Turabian StylePei, Ying, Baohong Lu, Yang Song, Yan Yang, Xinyue Feng, and Wenlong Shen. 2022. "Collaborative Ecological Flow Decision Making under the Bengbu Sluice Based on Ecological-Economic Objectives" Water 14, no. 24: 4133. https://doi.org/10.3390/w14244133







