A Comparative Study on Water and Gas Permeability of Pervious Concrete
Abstract
:1. Introduction
2. Experiments
2.1. Sample Preparation
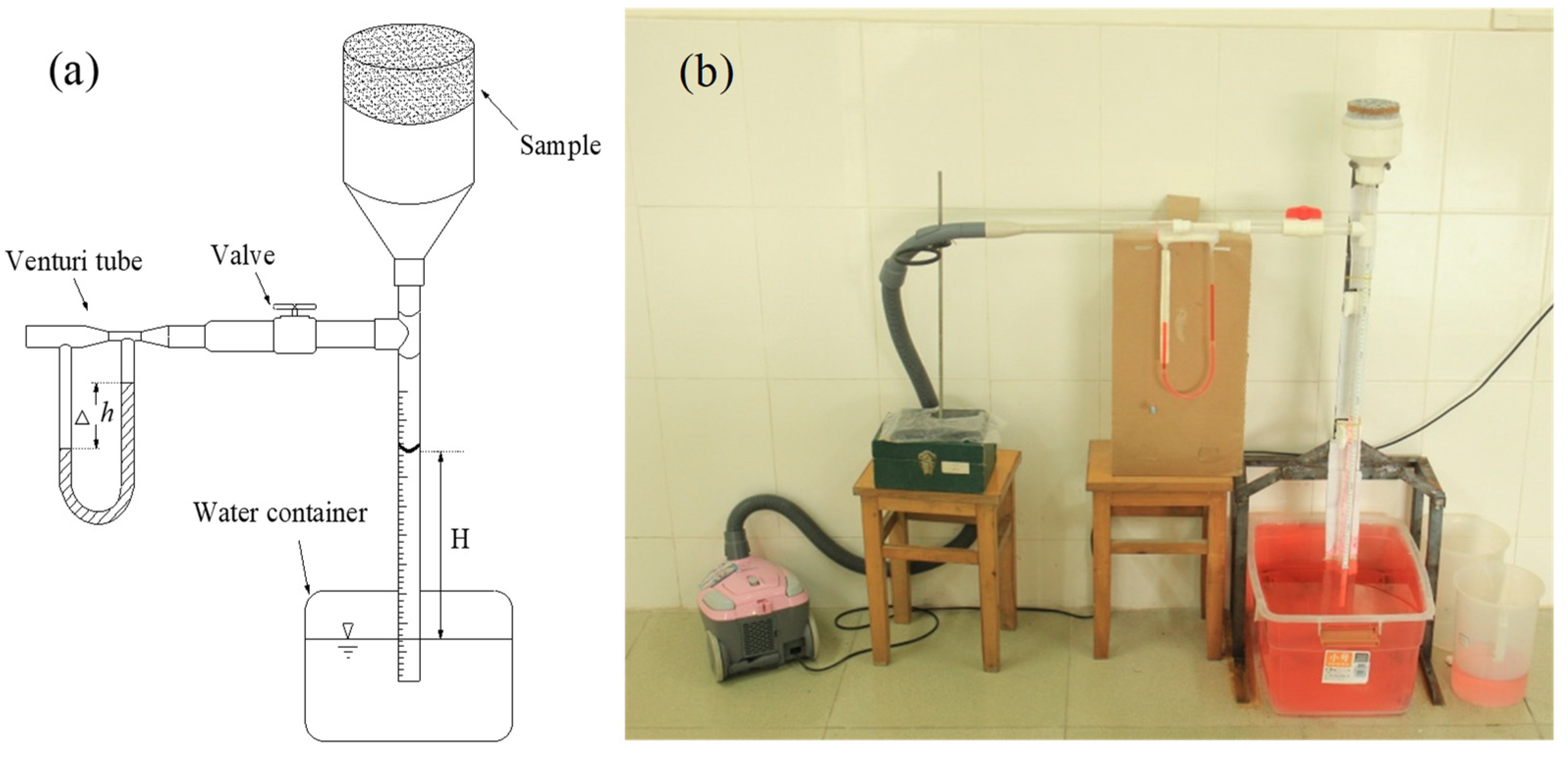
2.2. Water Permeability Test
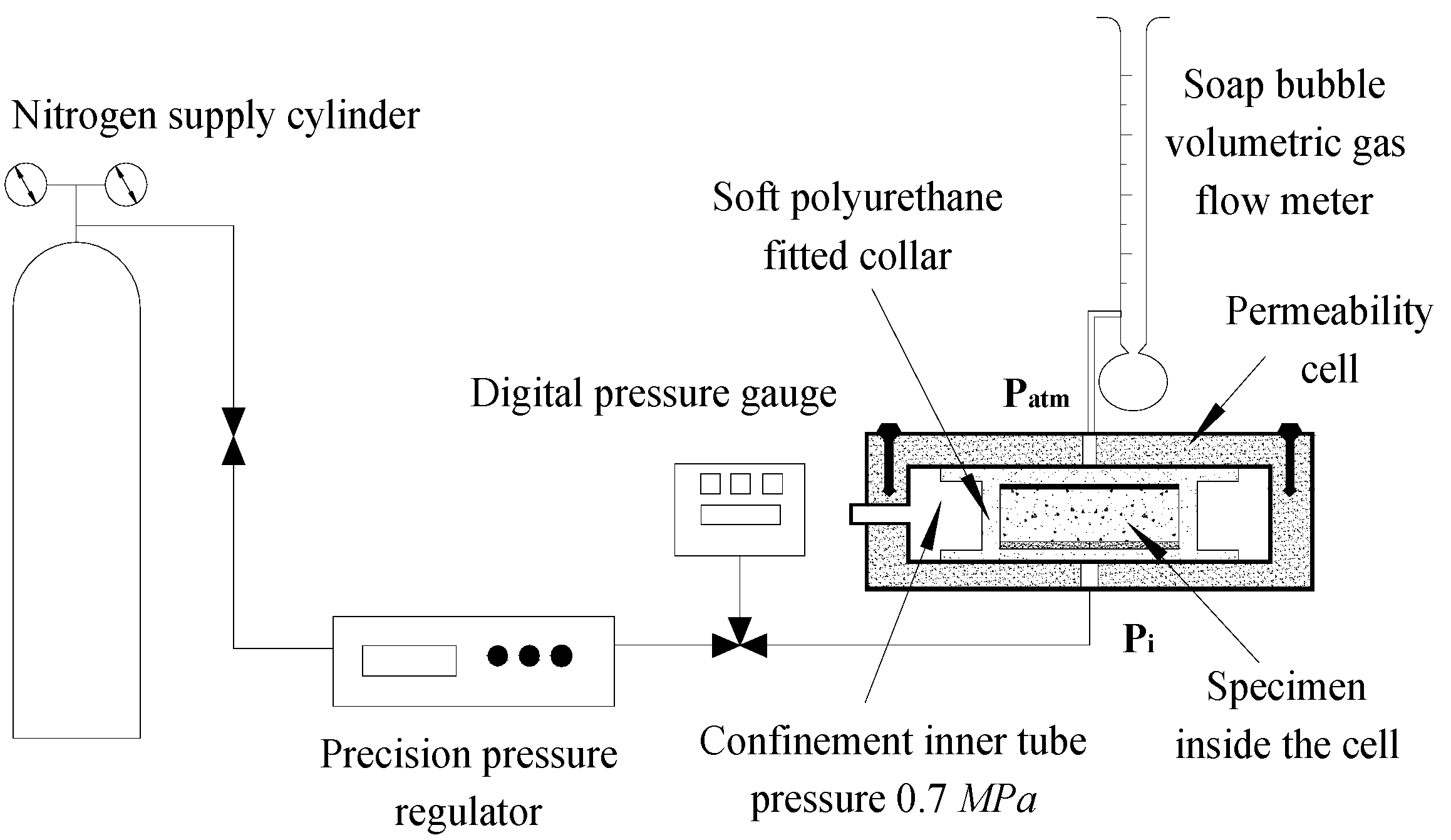
2.3. Gas Permeability Test
2.3.1. Gas Permeameter for Normal Concrete
2.3.2. A Novel Device for Testing the Gas Permeability of Pervious Concrete
2.4. Testing Information
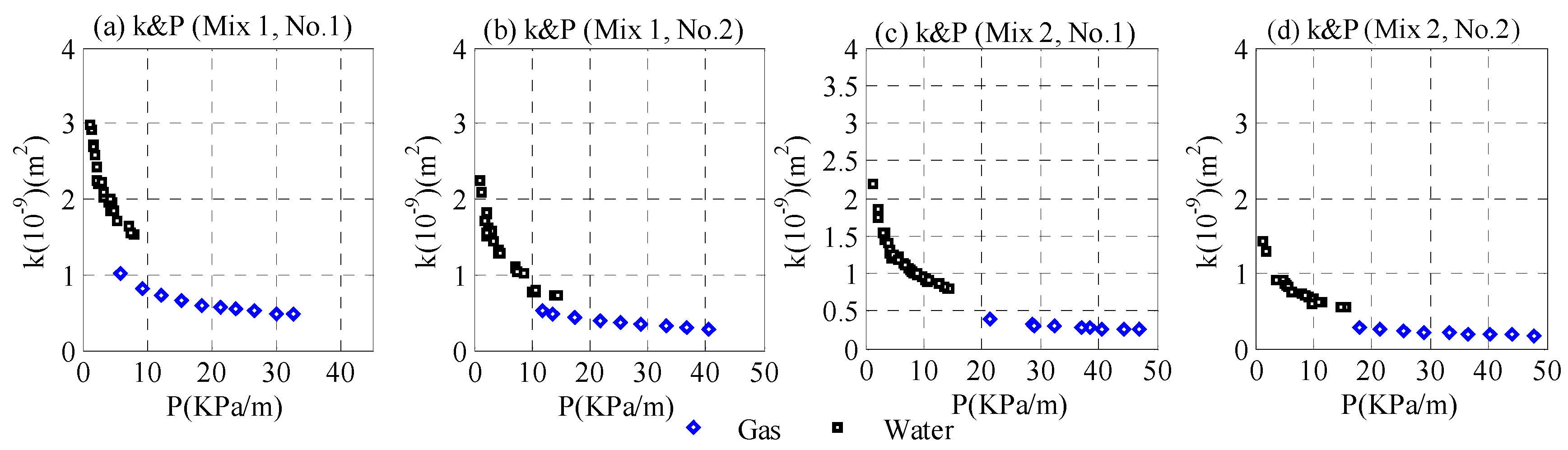
3. Results
4. Discussion
4.1. Difference between Water and Gas Permeability
4.2. Standardizing the Permeability Test for Pervious Concrete
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Tan, K.; Qin, Y.; Du, T.; Li, L.; Zhang, L.; Wang, J. Biochar from waste biomass as hygroscopic filler for pervious concrete to improve evaporative cooling performance. Constr. Build. Mater. 2021, 287, 123078. [Google Scholar] [CrossRef]
- Wang, J.; Meng, Q.; Zou, Y.; Qi, Q.; Tan, K.; Santamouris, M.; He, B.J. Performance synergism of pervious pavement on stormwater management and urban heat island mitigation: A review of its benefits, key parameters, and co-benefits approach. Water Res. 2022, 221, 118755. [Google Scholar] [CrossRef] [PubMed]
- Tan, K.; Qin, Y.; Wang, J. Evaluation of the properties and carbon sequestration potential of biochar-modified pervious concrete. Constr. Build. Mater. 2022, 314, 125648. [Google Scholar] [CrossRef]
- Wang, J.; Meng, Q.; Tan, K.; Santamouris, M. Evaporative cooling performance estimation of pervious pavement based on evaporation resistance. Build. Environ. 2022, 217, 109083. [Google Scholar] [CrossRef]
- ASTM C1701/C1701M-17a; Standard Test Method for Infiltration Rate of In Place Pervious Concrete. ASTM International: West Conshohocken, PA, USA, 2017. Available online: https://www.astm.org/c1701_c1701m-09.html (accessed on 30 March 2017).
- ASTM-D2434-68; Standard Test Method for Permeability of Granular Soils (Constant Head). ASTM International: West Conshohocken, PA, USA, 2019. Available online: https://www.astm.org/d2434-22.html (accessed on 1 October 2019).
- ASTM-D5084-03; Standard Test Method for Measurement of Hydraulic Conductivity of Saturated Porous Materials Using a Flexible Wall Permeameter. ASTM International: West Conshohocken, PA, USA, 2003. Available online: https://www.astm.org/d5084-16a.html (accessed on 1 November 2003).
- Park, S.B.; Lee, B.J.; Lee, J.; Jang, Y.I. A study on the seawater purification characteristics of water-permeable concrete using recycled aggregate. Resour. Conserv. Recycl. 2010, 54, 658–665. [Google Scholar] [CrossRef]
- Tho-In, T.; Sata, V.; Chindaprasirt, P.; Jaturapitakkul, C. Pervious high-calsium fly ash geopolymer concrete. Constr. Build. Mater. 2012, 30, 366–371. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, H.; Abdelhady, A.; Yang, J. Comparative laboratory measurement of pervious concrete permeability using constant-head and falling-head permeameter methods. Constr. Build. Mater. 2020, 263, 120614. [Google Scholar] [CrossRef]
- Qin, Y.; Yang, H.; Deng, Z.; He, J. Water permeability of pervious concrete is dependent on the applied pressure and testing methods. Adv. Mater. Sci. Eng. 2015, 404136. [Google Scholar] [CrossRef]
- Qin, Y.; Liang, J.; Yang, H.; Deng, Z. Gas permeability of pervious concrete and its implications on the application of pervious pavements. Measurement 2016, 78, 104–110. [Google Scholar] [CrossRef]
- Innocentini, M.D.M.; Pandolfelli, V.C. Permeability of porous ceramics considering the Klinkenberg and Inertial Effects. J. Am. Ceram. Soc. 2001, 84, 941–944. [Google Scholar] [CrossRef]
- Loosveldt, H.; Lafhaj, Z.; Skoczylas, F. Experimental study of gas and liquid permeability of a mortar. Cement Concrete Res. 2002, 32, 1357–1363. [Google Scholar] [CrossRef]
- Klinkenberg, L.J. The permeability of porous media to liquid and gases. In Drilling and Production Practice; American Petroleum Institute: New York, NY, USA, 1941. [Google Scholar] [CrossRef]
- Qin, Y.; Zhang, M.; Mei, G. A new simplified method for measuring the permeability characteristics of highly porous media. J. Hydrol. 2018, 562, 725–732. [Google Scholar] [CrossRef]
- Ministry of Housing and Urban-Ural Construction of the People’s Republic of China. CJJ/T 135: TechnicalSpecification for Pervious Cement Concrete Pavement; Ministry of Housing and Urban-Ural Construction of the People’s Republic of China: Beijing, China, 2009. Available online: https://ishare.iask.sina.com.cn/f/11867439.html (accessed on 29 August 2022). (In Chinese)
- Lafhaj, Z.; Richard, G.; Kaczmarek, M.; Skoczylas, F. Experimental determination of intrinsic permeability of limestone and concrete: Comparison between in situ and laboratory results. Build. Environ. 2007, 42, 3042–3050. [Google Scholar] [CrossRef]
- Dhir, R.K.; Hewlett, P.C.; Chan, Y.N. Near-surface characteristics of concrete: Assessment and development of in situ test methods. Mag. Concrete Res. 1987, 39, 183–195. [Google Scholar] [CrossRef]
- Cather, R.; Figg, J.W.; Marsden, A.F.; O’Brien, T.P. Improvements to the Figg method for determining the air permeability of concrete. Mag. Concrete Res. 1984, 36, 241–245. [Google Scholar] [CrossRef]
- Picandet, V.; Khelidj, A.; Bastian, G. Effect of axial compressive damage on gas permeability of ordinary and high-performance concrete. Cement Concrete Res. 2001, 31, 1525–1532. [Google Scholar] [CrossRef]
- Tsivilis, S.; Tsantilas, J.; Kakali, G.; Chaniotakis, E.; Sakellariou, A. The permeability of Portland limestone cement concrete. Cement Concrete Res. 2003, 33, 1465–1471. [Google Scholar] [CrossRef]
- Katz, J. Introductory Fluid Mechanics; Cambridge University Press: Cambridge, UK, 2010; 432p. [Google Scholar]
- Ward, J.C. Turbulent flow in porous media. ASCE J. Hydraulic Div. 1964, 90, 1–12. [Google Scholar] [CrossRef]
- Montes, F.; Vlavala, S.; Haselbach, L.M. A New Test Method for Porosity Measurements of Portland Cement Pervious Concrete. J. ASTM Int. 2005, 2, 1–13. [Google Scholar]
- Kevern, J.; Schaefer, V.R.; Wang, K.; Suleiman, M. Pervious Concrete Mixture Proportions for Improved Freeze-Thaw Durability. J. ASTM Int. 2008, 5, 1–12. [Google Scholar]
- Schaefer, V.R.; Wang, K. Mix Design Development for Pervious Concrete in Cold Weather Climates. Iowa Department of Transportation. Highway Division, 2006. Available online: www.perviouspavement.org/downloads/Iowa.pdf (accessed on 29 August 2022).
- Edvardsen, C. Water permeability and autogeneous healing of cracks in concrete. ACI Mater. J. 1999, 96, 448–454. [Google Scholar]
- Hearn, N. Self-sealing, autogeneous healing and continued hydration: What is the difference? Mater. Struct. 1998, 31, 563–567. [Google Scholar] [CrossRef]
- Hearn, N.; Morley, C.T. Self-sealing property of concrete--experimental evidence. Mater. Struct. 1997, 30, 404–411. [Google Scholar] [CrossRef]
- Yang, J.; Jiang, G. Experimental study on properties of pervious concrete pavement materials. Cem. Concr. Res. 2003, 33, 381–386. [Google Scholar] [CrossRef]
- Deo, O.; Sumanasooriya, M.; Neithalath, N. Permeability Reduction in Pervious Concretes due to Clogging: Experiments and Modeling. J. Mater. Civ. Eng. 2010, 22, 741–751. [Google Scholar] [CrossRef]
- Alarcon-Ruiz, L.; Brocato, M.; Dal Pont, S.; Feraille, A. Size Effect in Concrete Intrinsic Permeability Measurements. Transp. Porous Med. 2010, 85, 541–564. [Google Scholar] [CrossRef]
- Montes, F.; Haselbach, L. Measuring hydraulic conductivity in pervious concrete. Environ. Eng. Sci. 2006, 23, 960–969. [Google Scholar] [CrossRef]




| Sample | Cement | Water | Aggregate | Water Reducer |
|---|---|---|---|---|
| w/c = 0.25 | 523.16 | 130.79 | 1509.00 | 1.40 |
| w/c = 0.28 | 486.35 | 136.18 | 1509.00 | 1.40 |
| Sample | kv (m2) | b (KPa) | R2 | Porosity | Density | ||||
|---|---|---|---|---|---|---|---|---|---|
| Water | Gas | Water | Gas | Water | Gas | (%) | (kg/m3) | ||
| Mix1 | No.1 | 12.43 | 3.77 | 0.22 | 1.08 | 0.931 | 0.986 | 31.59 | 1731 |
| No.2 | 8.06 | 2.00 | 0.25 | 1.98 | 0.916 | 0.997 | 28.19 | 1767 | |
| Mix2 | No.1 | 7.56 | 1.74 | 0.31 | 2.56 | 0.930 | 0.956 | 26.59 | 1802 |
| No.2 | 4.96 | 1.09 | 0.35 | 3.01 | 0.929 | 0.998 | 25.64 | 1828 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, G.; Tan, K.; Liang, T.; Qin, Y. A Comparative Study on Water and Gas Permeability of Pervious Concrete. Water 2022, 14, 2846. https://doi.org/10.3390/w14182846
Wei G, Tan K, Liang T, Qin Y. A Comparative Study on Water and Gas Permeability of Pervious Concrete. Water. 2022; 14(18):2846. https://doi.org/10.3390/w14182846
Chicago/Turabian StyleWei, Gang, Kanghao Tan, Tenglong Liang, and Yinghong Qin. 2022. "A Comparative Study on Water and Gas Permeability of Pervious Concrete" Water 14, no. 18: 2846. https://doi.org/10.3390/w14182846
APA StyleWei, G., Tan, K., Liang, T., & Qin, Y. (2022). A Comparative Study on Water and Gas Permeability of Pervious Concrete. Water, 14(18), 2846. https://doi.org/10.3390/w14182846








