Assessment of Annual Erosion and Sediment Yield Using Empirical Methods and Validating with Field Measurements—A Case Study
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Area
2.2. MPSIAC Method
2.2.1. Surface Geology Factor (f1)
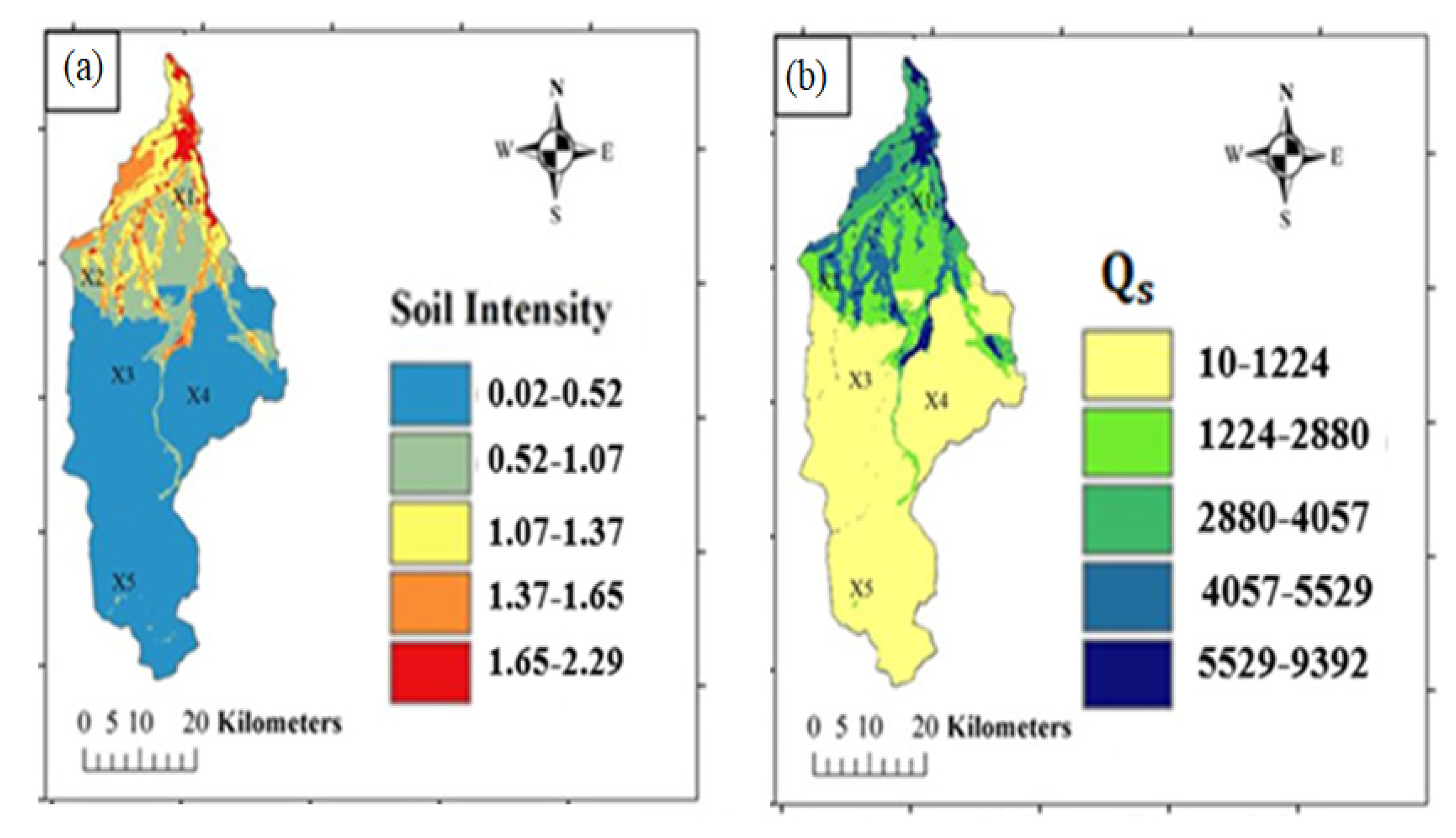
2.2.2. Soil Factor (f2)
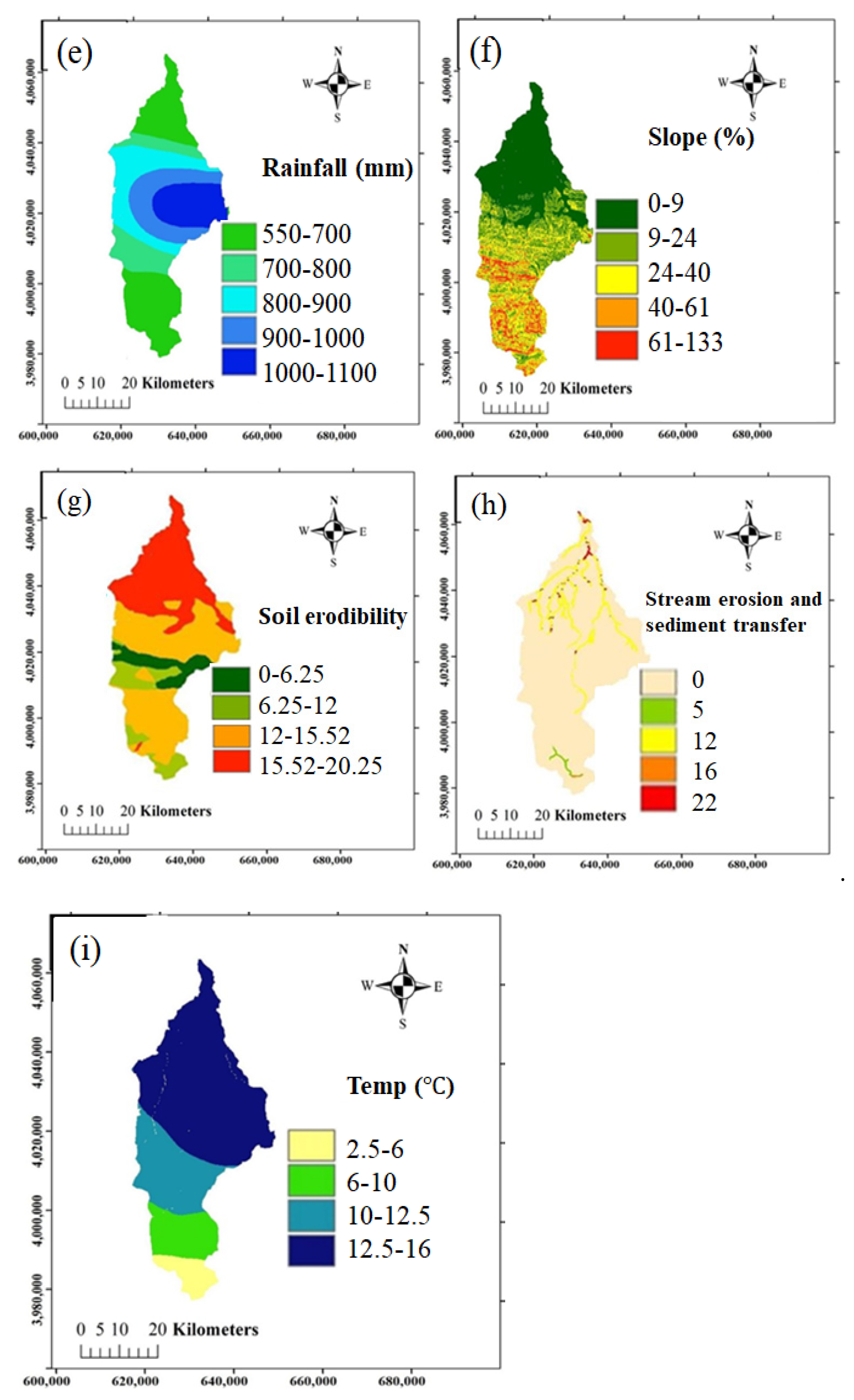
2.2.3. Climate Factor (f3)
2.2.4. Runoff Factor (f4)
2.2.5. Topography (f5)
2.2.6. Ground Cover (f6)
2.2.7. Land Use (f7)
2.2.8. Upland Erosion (f8)
2.2.9. Channel Erosion (f9)
2.2.10. Sediment Flux
2.3. EPM Method
2.4. Fournier Method
2.5. Research Data
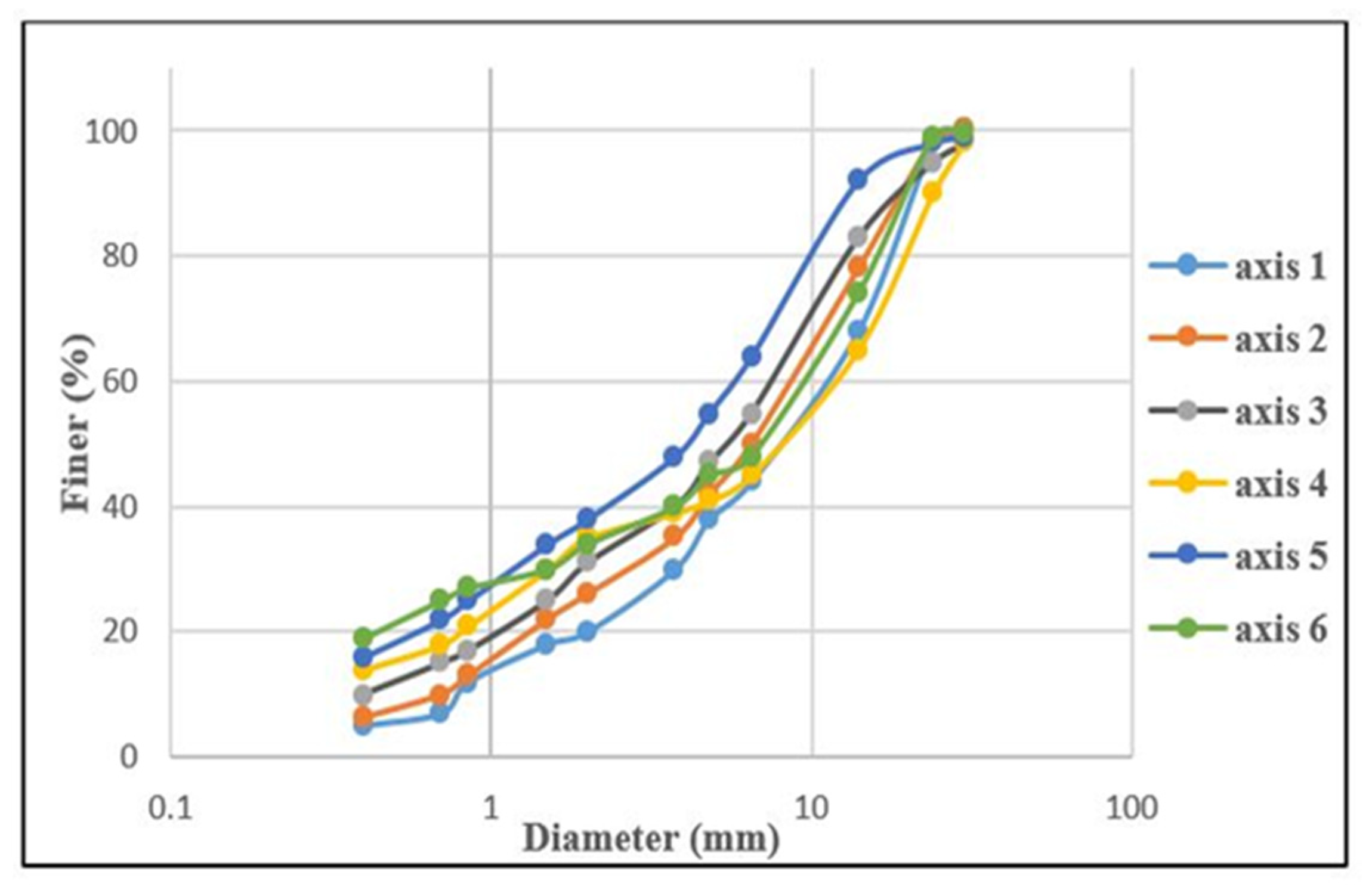
2.6. Field Data
3. Results
3.1. Determination of Sediment Production and Erosion Class using the MPSIAC Method
3.2. Calculation of Erosion Intensity Coefficient and Annual Sediment Yield by the EPM Method
3.3. Calculation of Erosion Intensity Coefficient and Annual Rate of Sediment Yield Using the Fournier Method
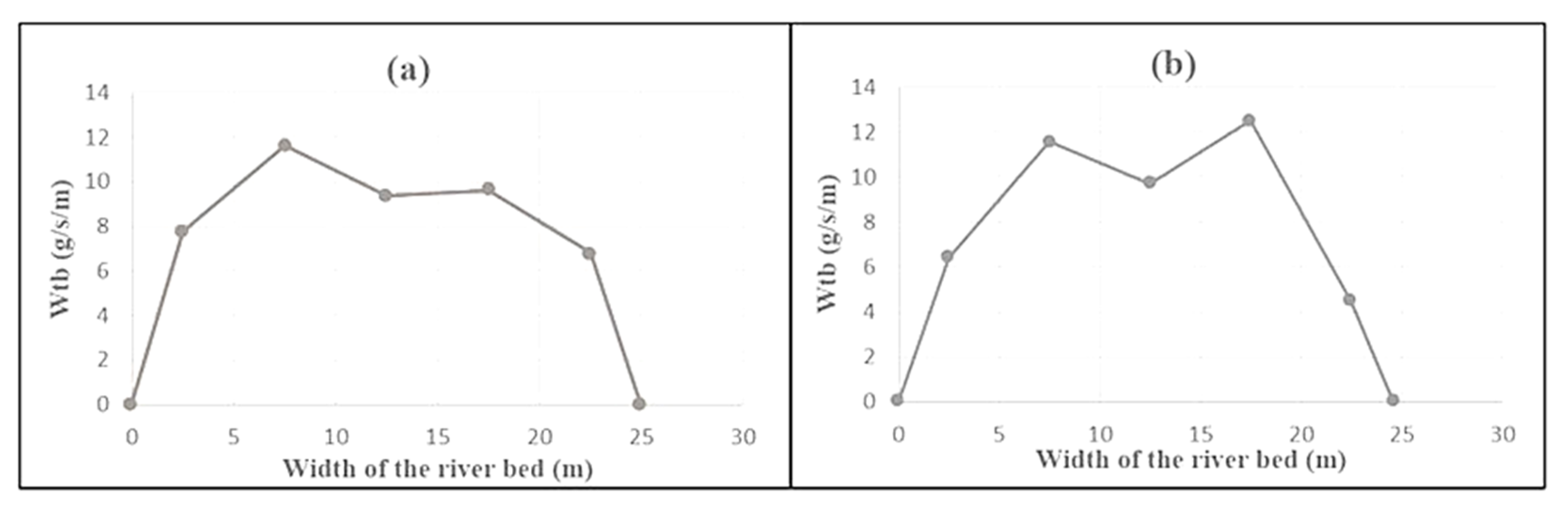
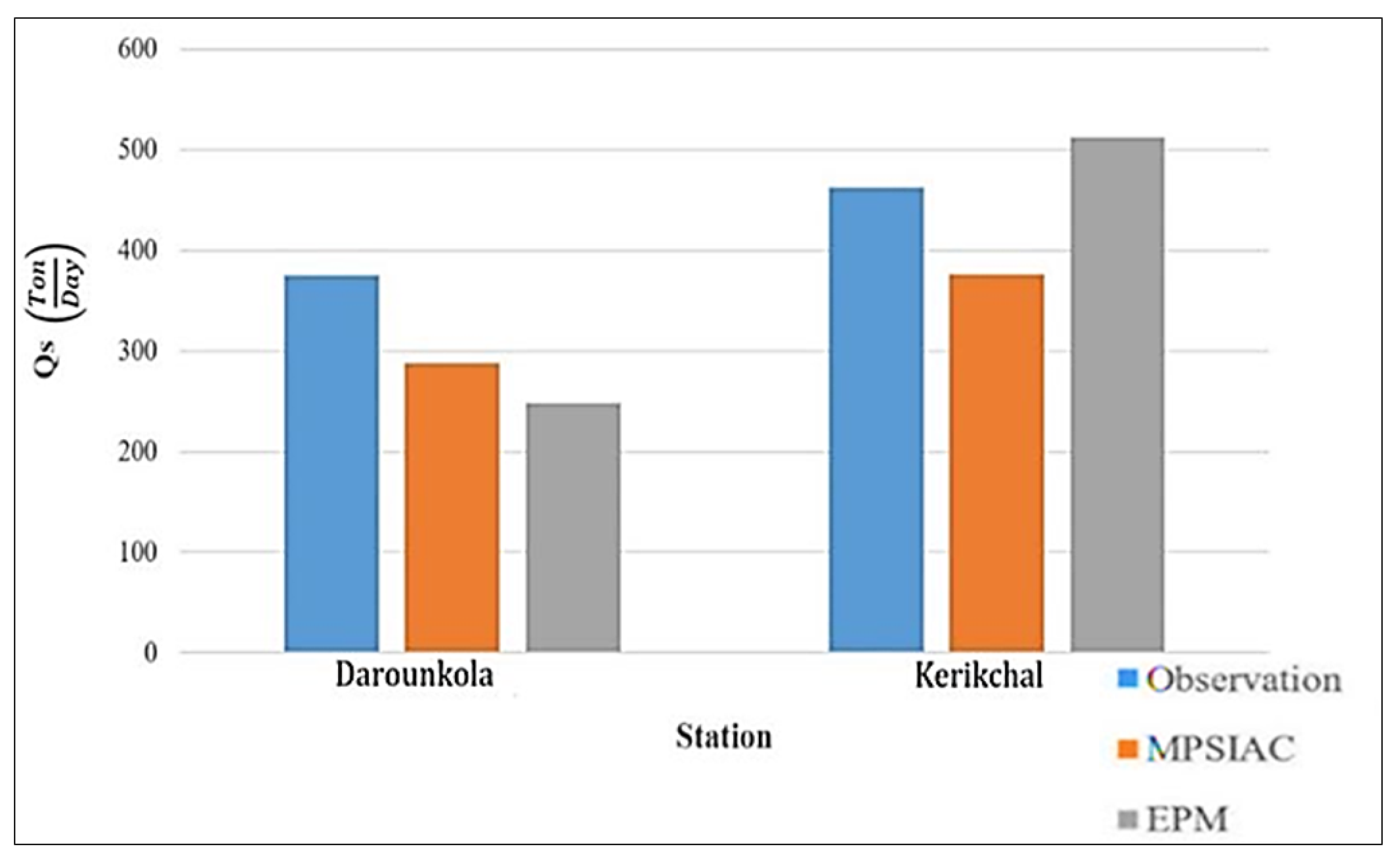
3.4. Verification of Model with Field Measurements
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Refahi, H.G. Water Erosion and Conservatio; University of Tehran Publication: Tehran, Iran, 2015. [Google Scholar]
- Wang, G.; Gertner, G.; Fang, S.; Anderson, A.B. Mapping Multiple Variables for Predicting Soil Loss by Geostatistical Methods with TM Images and a Slope Map. Photogramm. Eng. Remote Sens. 2003, 69, 889–898. [Google Scholar] [CrossRef]
- Alizadeh, A. Soil Erosion and Conservation; Astan Qods Razavi Publication: Mashhad, Iran, 1990. [Google Scholar]
- Wischmeier, W.H.; Smith, D.D. Predicting rainfall erosion losses. In USDA Agricultural Research Services Handbook; USDA: Washington, DC, USA, 1978. [Google Scholar]
- Jain, M.K.; Das, D. Estimation of sediment yield and areas of soil erosion and deposition for watershed prioritization using GIS and remote sensing. Water Resour. Manag. 2010, 24, 2091–2112. [Google Scholar] [CrossRef]
- Jemai, S.; Kallel, A.; Agoubi, B.; Abida, H. Soil Erosion Estimation in Arid Area by USLE Model Applying GIS and RS: Case of Oued El Hamma Catchment, South-Eastern Tunisia. J. Indian Soc. Remote Sens. 2021, 49, 1293–1305. [Google Scholar] [CrossRef]
- Renard, K.G.; Foster, G.R.; Weesies, G.A.; Porter, J.P. RUSLE: Revised universal soil loss equation. J. Soil Water Conserv. 1991, 46, 30–33. [Google Scholar]
- Williams, J.R. Sediment routing for agricultural watersheds. JAWRA J. Am. Water Resour. Assoc. 1975, 11, 965–974. [Google Scholar] [CrossRef]
- Bagherzadeh, A.; Daneshvar, M.R.M. Sediment yield assessment by EPM and PSIAC models using GIS data in semi-arid region. Front. Earth Sci. 2011, 5, 207–216. [Google Scholar] [CrossRef]
- Noori, H.; Siadatmousavi, S.M.; Mojaradi, B. Assessment of sediment yield using RS and GIS at two sub-basins of Dez Watershed, Iran. Int. Soil Water Conserv. Res. 2016, 4, 199–206. [Google Scholar] [CrossRef]
- Ganasri, B.P.; Ramesh, H. Assessment of soil erosion by RUSLE model using remote sensing and GIS—A case study of Nethravathi Basin. Geosci. Front. 2016, 7, 953–961. [Google Scholar] [CrossRef]
- Singh, G.; Panda, R.K. Grid-cell based assessment of soil erosion potential for identification of critical erosion prone areas using USLE, GIS and remote sensing: A case study in the Kapgari watershed, India. Int. Soil Water Conserv. Res. 2017, 5, 202–211. [Google Scholar] [CrossRef]
- Pourkarimi, M.; Mahmoudi, S.; Masihabadi, M.; Pazira, E.; Moeini, A. Use of MPSIAC and EPM to estimate sediment yield and erosion-a case study of a watershed of the second urban phase, Mashhad, Khorasan Province. Agric. For. 2017, 63, 201–213. [Google Scholar] [CrossRef][Green Version]
- Batista, P.V.G.; Silva, M.L.N.; Silva, B.P.C.; Curi, N.; Bueno, I.T.; Acérbi Júnior, F.W.; Davies, J.; Quinton, J. Modelling spatially distributed soil losses and sediment yield in the upper Grande River Basin—Brazil. Catena 2017, 157, 139–150. [Google Scholar] [CrossRef]
- Mirakhorlo, M.S.; Rahimzadegan, M. Application of sediment rating curves to evaluate efficiency of EPM and MPSIAC using RS and GIS. Environ. Earth Sci. 2018, 77, 723. [Google Scholar] [CrossRef]
- Kidane, M.; Bezie, A.; Kesete, N.; Tolessa, T. The impact of land use and land cover (LULC) dynamics on soil erosion and sediment yield in Ethiopia. Heliyon 2019, 5, e02981. [Google Scholar] [CrossRef] [PubMed]
- Rajbanshi, J.; Bhattacharya, S. Assessment of soil erosion, sediment yield and basin specific controlling factors using RUSLE-SDR and PLSR approach in Konar river basin, India. J. Hydrol. 2020, 587, 124935. [Google Scholar] [CrossRef]
- Pijl, A.; Reuter, L.E.H.; Quarella, E.; Vogel, T.A.; Tarolli, P. GIS-based soil erosion modelling under various steep-slope vineyard practices. Catena 2020, 193, 104604. [Google Scholar] [CrossRef]
- Mezosi, G.; Mucsi, L. Soil erosion assessment with the help of remote sensing methods. In Proceedings of the International Symposium of Operationalization of Remote Sensing, Enschede, The Netherlands, 19–23 April 1993; pp. 19–23. [Google Scholar]
- Tangestani, M.H. Integrating geographic information systems in erosion and sediment yield applications using the erosion potential method (EPM). In Proceedings of the GIS Research UK, Ninth Annual Conference, Glamorgan, Wales, 18–20 April 2001; pp. 18–20. [Google Scholar]
- Lin, C.Y.; Lin, W.T.; Chou, W.C. Soil erosion prediction and sediment yield estimation: The Taiwan experience. Soil Tillage Res. 2002, 68, 143–152. [Google Scholar] [CrossRef]
- Shrimali, S.S.; Aggarwal, S.P.; Samra, J.S. Prioritizing erosion-prone areas in hills using remote sensing and GIS—A case study of the Sukhna Lake catchment, Northern India. Int. J. Appl. Earth Obs. Geoinf. 2001, 3, 54–60. [Google Scholar] [CrossRef]
- Shahiri Tabarestani, E.; Afzalimehr, H. Artificial neural network and multi-criteria decision-making models for flood simulation in GIS: Mazandaran Province, Iran. Stoch. Environ. Res. Risk Assess. 2021, 35, 2439–2457. [Google Scholar] [CrossRef]
- Hadian, S.; Tabarestani, E.S.; Pham, Q.B. Multi attributive ideal-real comparative analysis (MAIRCA) method for evaluating flood susceptibility in a temperate Mediterranean climate. Hydrol. Sci. J. 2022, 67, 401–418. [Google Scholar] [CrossRef]
- Yang, C.T. Sediment Transport: Theory and Practice; McGraw-Hill: New York, NY, USA, 1996; ISBN 0070723109. [Google Scholar]
- Haddadchi, A.; Mohammad, H.O.; Amir, A.D.; Haddadchi, A.; Mohammad, H.O.; Amir, A.D. Assessment of Bed-Load Predictors Based on Sampling in a Gravel Bed River. J. Hydrodyn. 2012, 24, 145–151. [Google Scholar] [CrossRef]
- López, R.; Vericat, D.; Batalla, R.J. Evaluation of bed load transport formulae in a large regulated gravel bed river: The lower Ebro (NE Iberian Peninsula). J. Hydrol. 2014, 510, 164–181. [Google Scholar] [CrossRef]
- Shahiri Tabarestani, E.; Afzalimehr, H.; Pham, Q.B. Flow structure investigation over a pool-riffle sequence in a variable width river. Acta Geophys. 2022, 1, 713–727. [Google Scholar] [CrossRef]
- Yu, B.Y.; Wu, P.; Sui, J.; Ni, J.; Whitcombe, T. Variation of runoff and sediment transport in the huai river—A case study. J. Environ. Inform. 2020, 35, 138–147. [Google Scholar] [CrossRef]
- Jhonson, C.W.; Gembhart, A.C. Predicting sediment yield from sagerbrush range lands. Agric. Rev. Man. 1982, 26, 145–156. [Google Scholar]
- Gavrilovic, Z. The Use of an Empirical Method for Calculating Sediment Production and Transport in Unsuited or Torrential Streams. Open J. Geol. 1988, 6, 411–422. [Google Scholar]
- Costea, M. Using the Fournier indexes in estimating rainfall erosivity. Case study-the Secasul Mare Basin. Aerul Si Apa. Compon. ale Mediu. 2012, 2012, 313–320. Available online: http://aerapa.conference.ubbcluj.ro/ (accessed on 25 March 2022).
- Song, T.; Chiew, Y.M.; Chin, C.O. Effect of Bed-Load Movement on Flow Friction Factor. J. Hydraul. Eng. 1998, 124, 165–175. [Google Scholar] [CrossRef]
- Sui, J.; He, Y.; Liu, C. Changes in sediment transport in the Kuye River in the Loess Plateau in China. Int. J. Sediment Res. 2009, 24, 201–213. [Google Scholar] [CrossRef]
- Liu, C.; Sui, J.; Wang, Z.Y. Changes in runoff and sediment yield along the Yellow River during the period from 1950 to 2006. J. Environ. Inform. 2008, 12, 129–139. [Google Scholar] [CrossRef]
- Liu, C.; Sui, J.; Wang, Z.Y. Sediment load reduction in Chinese rivers. Int. J. Sediment Res. 2008, 23, 44–55. [Google Scholar] [CrossRef]
- Kavian, A.; Safari, A. Determining the appropriate model for estimating sedimentation using statistical methods. J. Appl. Res. Geogr. Sci. 2013, 30, 111–130. [Google Scholar]
- Najm, Z.; Keyhani, N.; Rezaei, K.; Nezamabad, A.N.; Vaziri, S.H. Sediment yield and soil erosion assessment by using an empirical model of MPSIAC for Afjeh & Lavarak sub-watersheds, Iran. Earth 2013, 2, 14–22. [Google Scholar]
- Daneshvar, M.; Bagherzadeh, A. Evaluation of sediment yield in PSIAC and MPSIAC models by using GIS at Toroq Watershed, Northeast of Iran. Front. Earth Sci. 2012, 6, 83–94. [Google Scholar] [CrossRef]
- Núñez-González, F.; Rovira, A.; Ibàñez, C. Bed load transport and incipient motion below a large gravel bed river bend. Adv. Water Resour. 2018, 120, 83–97. [Google Scholar] [CrossRef]
- Afzalimehr, H.; Hadian, S.; Shahiri Tabarestani, E.; Mohammadi, M. Influence of Suspended Sediment Load on Roughness Coefficient and Intensity of Flow Turbulence (Case study: Haraz, Rostamabad and Beheshtabad Rivers). Environ. Water Eng. 2020, 6, 459–472. [Google Scholar] [CrossRef]
- Shahiri Tabarestani, E.; Afzalimehr, H.; Pham, Q.B. Validation of double averaged velocity method in a variable width river. Earth Sci. Inform. 2021, 14, 2265–2278. [Google Scholar] [CrossRef]
- Yang, C.T.; Huang, C. Applicability of sediment transport formulas. Int. J. Sediment Res. 2001, 16, 335–353. [Google Scholar]
- Bravo-Espinosa, M.; Osterkamp, W.R.; Lopes, V.L. Bedload Transport in Alluvial Channels. J. Hydraul. Eng. 2003, 129, 783–795. [Google Scholar] [CrossRef]
- Yu, B.Y.; Wu, P.; Sui, J.; Yang, X.; Ni, J. Fluvial geomorphology of the Middle Reach of the Huai River. Int. J. Sediment Res. 2014, 29, 24–33. [Google Scholar] [CrossRef]
- Sui, J.; He, Y.; Karney, B.W. Flow and high sediment yield from the Huangfuchuan watershed. Int. J. Environ. Sci. Tech 2008, 5, 149–160. [Google Scholar] [CrossRef]




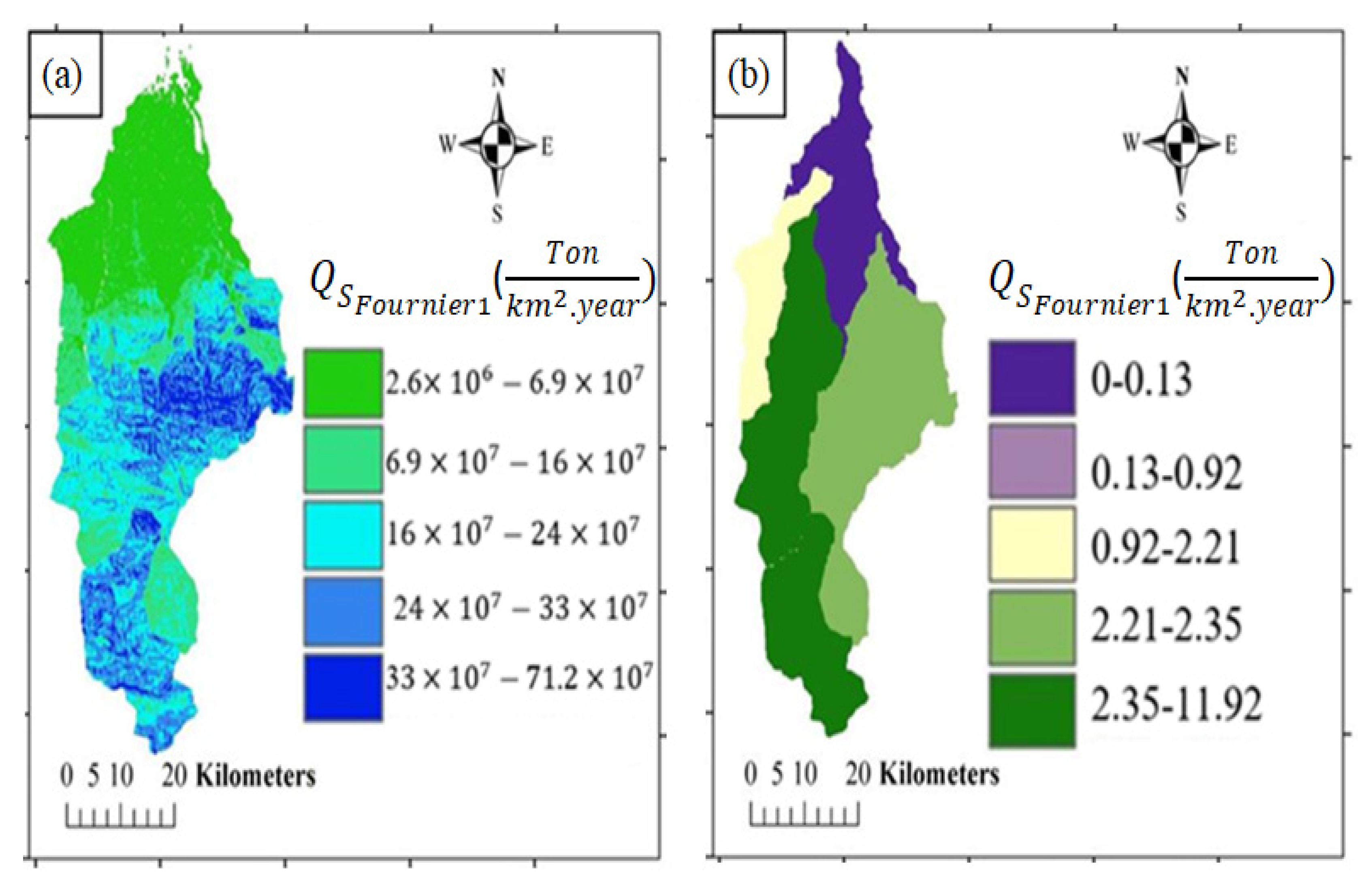
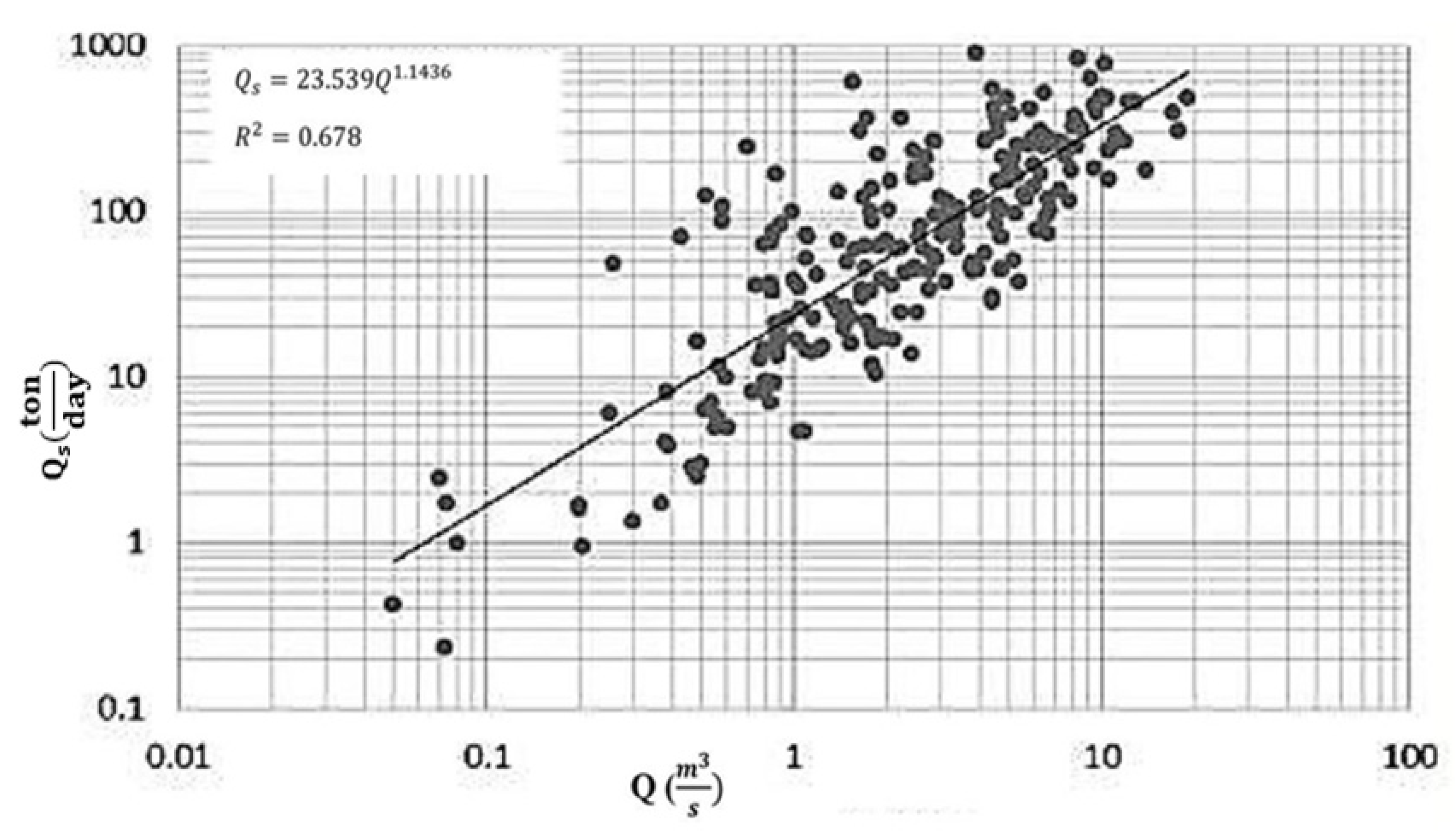
| Geounit | Description | f1 |
|---|---|---|
| Qm | Swamp and marsh | 2 |
| Pel | Medium- to thick-bedded limestone | 6 |
| Mm,s,l | Marl, calcareous sandstone, sandy limestone, and minor conglomerate | 5 |
| TRJs | Dark-gray shale and sandstone | 9 |
| K2l2 | Thick-bedded to massive limestone | 5 |
| Plc | Polymictic conglomerate and sandstone | 5 |
| TRe | Bedded dolomite and dolomitic limestone | 3 |
| Ktzl | Thick-bedded to massive, white to pinkish orbitolina-bearing limestone | 6 |
| Jl | Light-gray, thin-bedded to massive limestone | 5 |
| Kbvt | Basaltic volcanic tuff | 5 |
| Qft2 | Low-level piedmont fan and valley terrace deposits | 5 |
| Type of Soil | f2 | k |
|---|---|---|
| Mollisols | 6 | 0.36 |
| Rock Outcrops/Entisols | 3 | 0.18 |
| Alfisols | 7.1 | 0.43 |
| Inceptisols | 8 | 0.48 |
| Mollisols | 6 | 0.36 |
| Inceptisols | 8 | 0.48 |
| Alfisols | 7.1 | 0.43 |
| Sediment Production m3/(km2.year) | Erosion Intensity | Erosion Classification |
|---|---|---|
| >1429 | Very high | V |
| 476–1429 | High | IV |
| 238–476 | Moderate | III |
| 95–238 | Low | II |
| <95 | Very low | I |
| Ranges | Erosion Intensity | Erosion Classification |
|---|---|---|
| Z > 1 | Very high | V |
| 0.71 < Z < 1 | High | IV |
| 0.41 < Z < 0.71 | Moderate | III |
| 0.2 < Z < 0.71 | Low | II |
| Z < 0.2 | Very low | I |
| Dataset | Source | Data Type | Scale of Source Data | Derived Factors |
|---|---|---|---|---|
| Digital elevation model (DEM) | United States Geological Survey (USGS) site | Raster | 1:25,000 | Elevation, slope |
| Rainfall | 10-year meteorological data (2009–2019), Iran | Vector | 1:25,000 | Rainfall map |
| Geological map | Mazandaran Regional Water Authority, Iran | Vector | 1:100,000 | Geology, soil type |
| Land cover | Mazandaran Regional Water Authority, Iran | Vector | 1:100,000 | Land use |
| Region | R | Area (km2) | Class | ||
|---|---|---|---|---|---|
| X1 | 51.9 | 238.44 | 166 | 39,582.19 | III |
| X2 | 45.167 | 188.38 | 94 | 17,708.26 | II |
| X3 | 36.327 | 138.25 | 226 | 31,245.38 | II |
| X4 | 35.562 | 134.60 | 147 | 19,786.38 | II |
| X5 | 40.053 | 157.51 | 329 | 51,821.45 | II |
| basin | 41.27 | 166.469 | 962 | 160,143.17 | II |
| Herbaceous Plants–Groves | Urban Areas–Beaches | Forest Land–Agriculture | Fruit Trees–Agricultural Lands | Dense Forest–Mountainous Lands |
|---|---|---|---|---|
| 0.4 | 1 | 0.3 | 0.7 | 0.2 |
| Geo-unit | Description | Y |
|---|---|---|
| Qm | Swamp and marsh | 2 |
| Pel | Medium- to thick-bedded limestone | 1 |
| Mm,s,l | Marl, calcareous sandstone, sandy limestone, and minor conglomerate | 1 |
| TRJs | Dark-gray shale and sandstone | 1 |
| K2l2 | Thick-bedded to massive limestone | 1 |
| Plc | Polymictic conglomerate and sandstone | 1.2 |
| TRe | bedded dolomite and dolomitic limestone | 1 |
| Ktzl | Thick-bedded to massive, white to pinkish orbitolina-bearing limestone | 1 |
| Jl | Light-gray, thin-bedded to massive limestone | 1 |
| Kbvt | Basaltic volcanic tuff | 1 |
| Qft2 | Low-level piedmont fan and valley terrace deposits | 2 |
| Urban Areas | Floodplain | Lowlands | Alluvial Plain | Hillside | Plateau | Crop Coverage | Forest Cover |
|---|---|---|---|---|---|---|---|
| 0.3 | 1 | 0.6 | 0.8 | 0.5 | 0.2 | 0.15 | 0.1 |
| Region | Z | Ru | Area (km2) | Class | ||
|---|---|---|---|---|---|---|
| X1 | 1.2 | 0.31 | 1057.36 | 166 | 175,521.76 | V |
| X2 | 0.81 | 0.32 | 738.13 | 94 | 69,384.22 | IV |
| X3 | 0.45 | 0.57 | 688.35 | 226 | 15,5567.1 | III |
| X4 | 0.32 | 0.74 | 578.87 | 147 | 85,093.89 | II |
| X5 | 0.23 | 1.25 | 236.06 | 329 | 77,663.74 | II |
| Basin | 0.54 | 0.79 | 585.47 | 962 | 563,230.71 | III |
| Region | Area (km2) | ||
|---|---|---|---|
| X1 | 166 | 1.3 × 109 | 23.24 |
| X2 | 94 | 4.2 × 109 | 88.36 |
| X3 | 226 | 3.5× 1010 | 537.88 |
| X4 | 147 | 3.3 × 1010 | 327.81 |
| X5 | 329 | 8.6 × 1010 | 3911.81 |
| Basin | 962 | 6.7 × 1011 | 4889.1 |
| Cross-Section | Slope S (m/m) | Width W (m) | Hydraulic Depth h (m) | Mean Flow Velocity Ueq (m/s) | Bed Load Transport Rate qb (ton/day) | Discharge q (m2/s) |
|---|---|---|---|---|---|---|
| D1 | 0.0071 | 23.3 | 0.395 | 0.989 | 0.634 | 0.391 |
| D2 | 0.0077 | 25 | 0.391 | 1.094 | 0.717 | 0.428 |
| D3 | 0.0056 | 24.7 | 0.432 | 0.965 | 0.702 | 0.417 |
| K1 | 0.0009 | 28 | 0.385 | 1.093 | 0.580 | 0.421 |
| K2 | 0.0007 | 25.2 | 0.521 | 0.95 | 0.762 | 0.496 |
| K3 | 0.0058 | 24.6 | 0.570 | 0.926 | 0.736 | 0.528 |
| K4 | 0.0078 | 25.4 | 0.561 | 0.862 | 0.612 | 0.484 |
| Cross-Section | Q (m3/s) | Calculated | Measured |
|---|---|---|---|
| D1 | 9.1 | 294.136 | 14.772 |
| D2 | 10.7 | 353.990 | 17.925 |
| D3 | 10.3 | 338.897 | 17.339 |
| K1 | 11.8 | 395.906 | 16.240 |
| K2 | 12.5 | 422.877 | 19.202 |
| K3 | 13 | 442.276 | 18.106 |
| K4 | 12.3 | 415.148 | 15.545 |
| Field Measurements | MPSIAC | EPM | ||||
|---|---|---|---|---|---|---|
| Station | Q | |||||
| Darounkola | 10.7 | 353.99 | 9.86 | 371.915 | 287.38 | 248.272 |
| Kerikchal | 13 | 442.276 | 18.106 | 460.382 | 376.24 | 520.72 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tabarestani, E.S.; Afzalimehr, H.; Sui, J. Assessment of Annual Erosion and Sediment Yield Using Empirical Methods and Validating with Field Measurements—A Case Study. Water 2022, 14, 1602. https://doi.org/10.3390/w14101602
Tabarestani ES, Afzalimehr H, Sui J. Assessment of Annual Erosion and Sediment Yield Using Empirical Methods and Validating with Field Measurements—A Case Study. Water. 2022; 14(10):1602. https://doi.org/10.3390/w14101602
Chicago/Turabian StyleTabarestani, Ehsan Shahiri, Hossein Afzalimehr, and Jueyi Sui. 2022. "Assessment of Annual Erosion and Sediment Yield Using Empirical Methods and Validating with Field Measurements—A Case Study" Water 14, no. 10: 1602. https://doi.org/10.3390/w14101602
APA StyleTabarestani, E. S., Afzalimehr, H., & Sui, J. (2022). Assessment of Annual Erosion and Sediment Yield Using Empirical Methods and Validating with Field Measurements—A Case Study. Water, 14(10), 1602. https://doi.org/10.3390/w14101602





