Probability Analysis and Control of River Runoff–sediment Characteristics based on Pair-Copula Functions: The Case of the Weihe River and Jinghe River
Abstract
1. Introduction
2. Materials and Methods
2.1. Research Area and Data
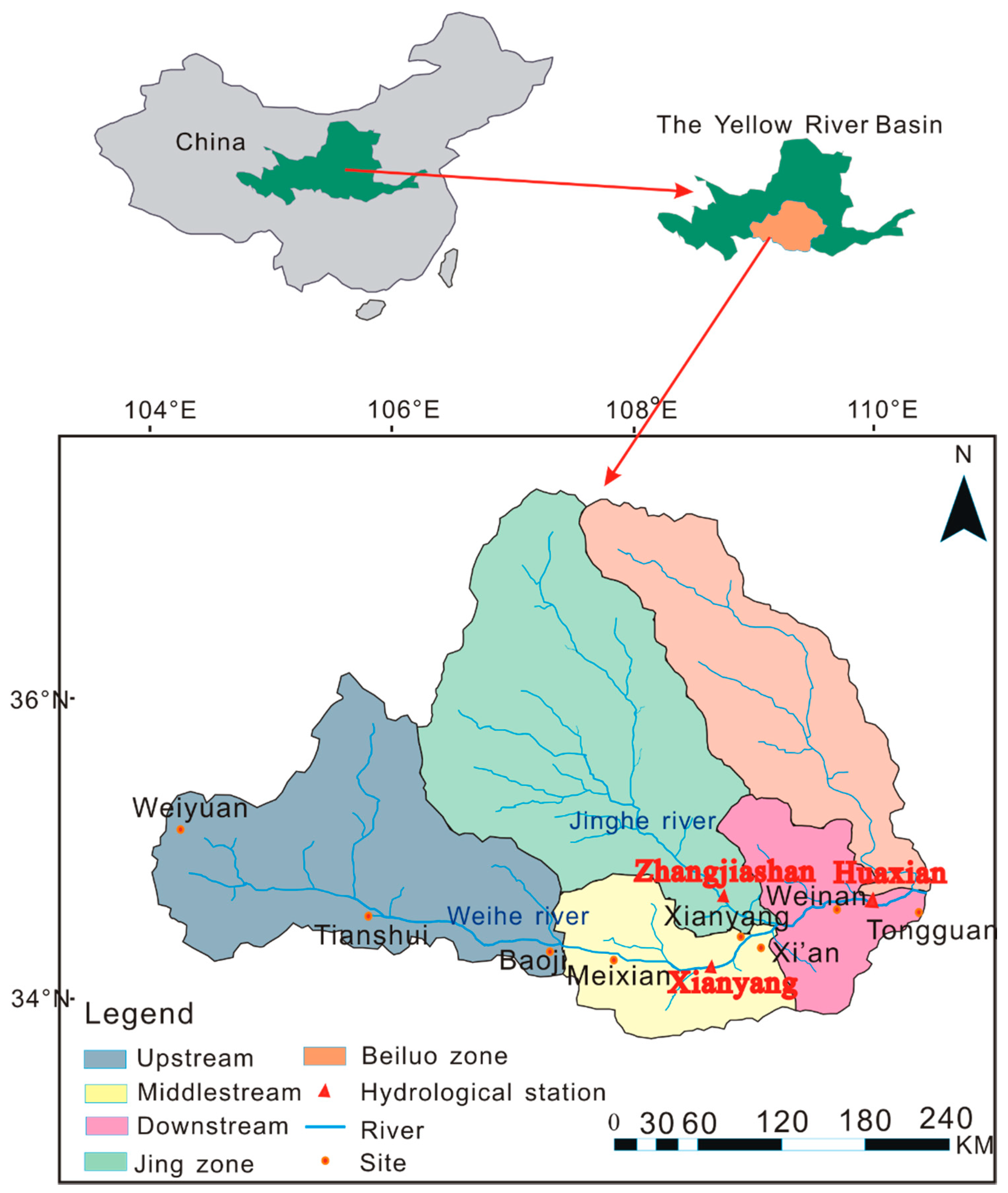
2.1.1. Brief Introduction to the Research Area
2.1.2. Data
2.2. Research Methods
2.2.1. Rescaled Adjusted Partial Sums (RAPS) Method
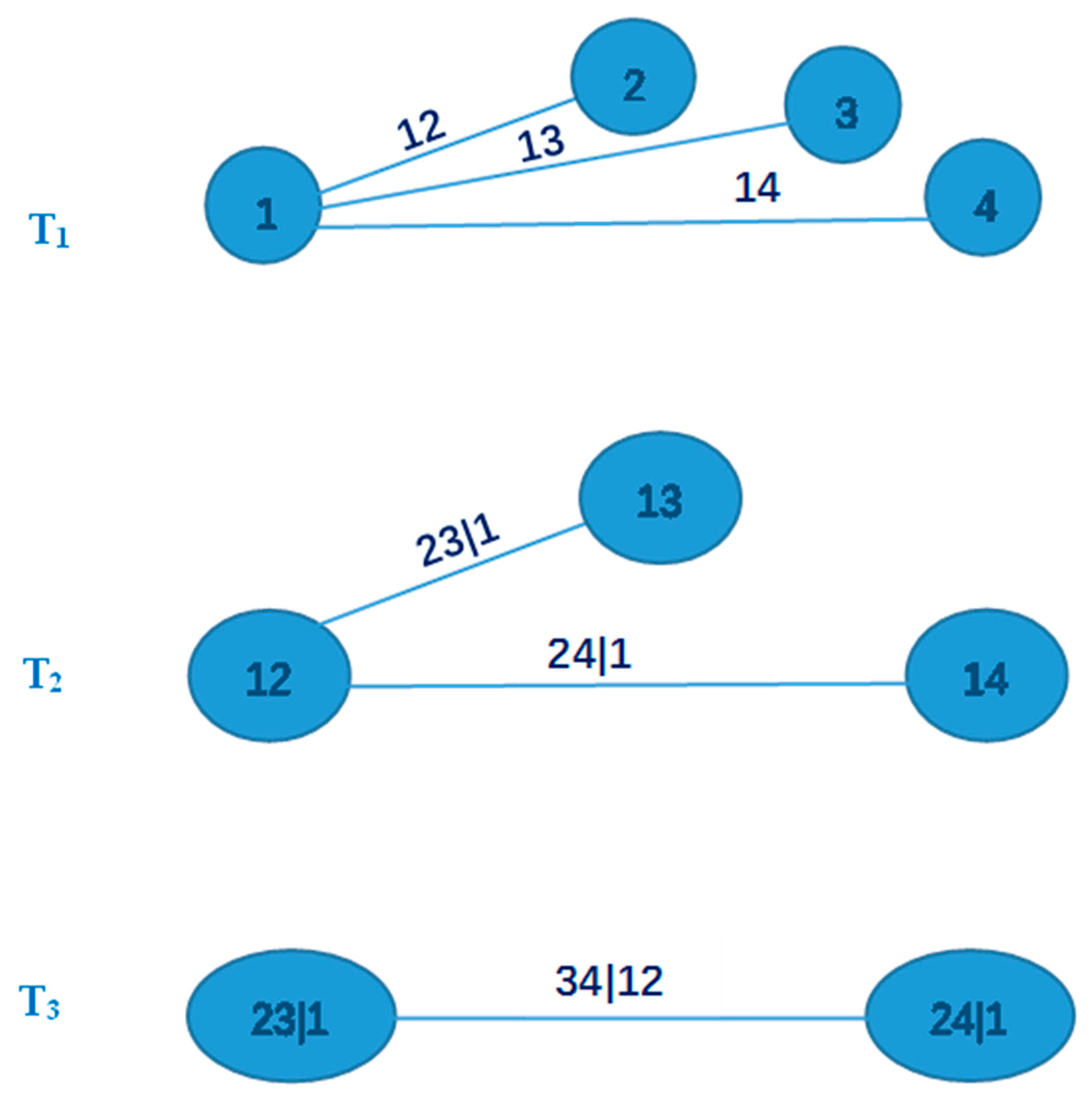
2.2.2. Pair-Copula Function
2.2.3. Three-Dimensional C-Vine Pair-Copula Function Probability Calculation
2.2.4. Calculation of the Synchronous and Asynchronous Probability of High–Low Runoff–Sediment
3. Results and Discussion
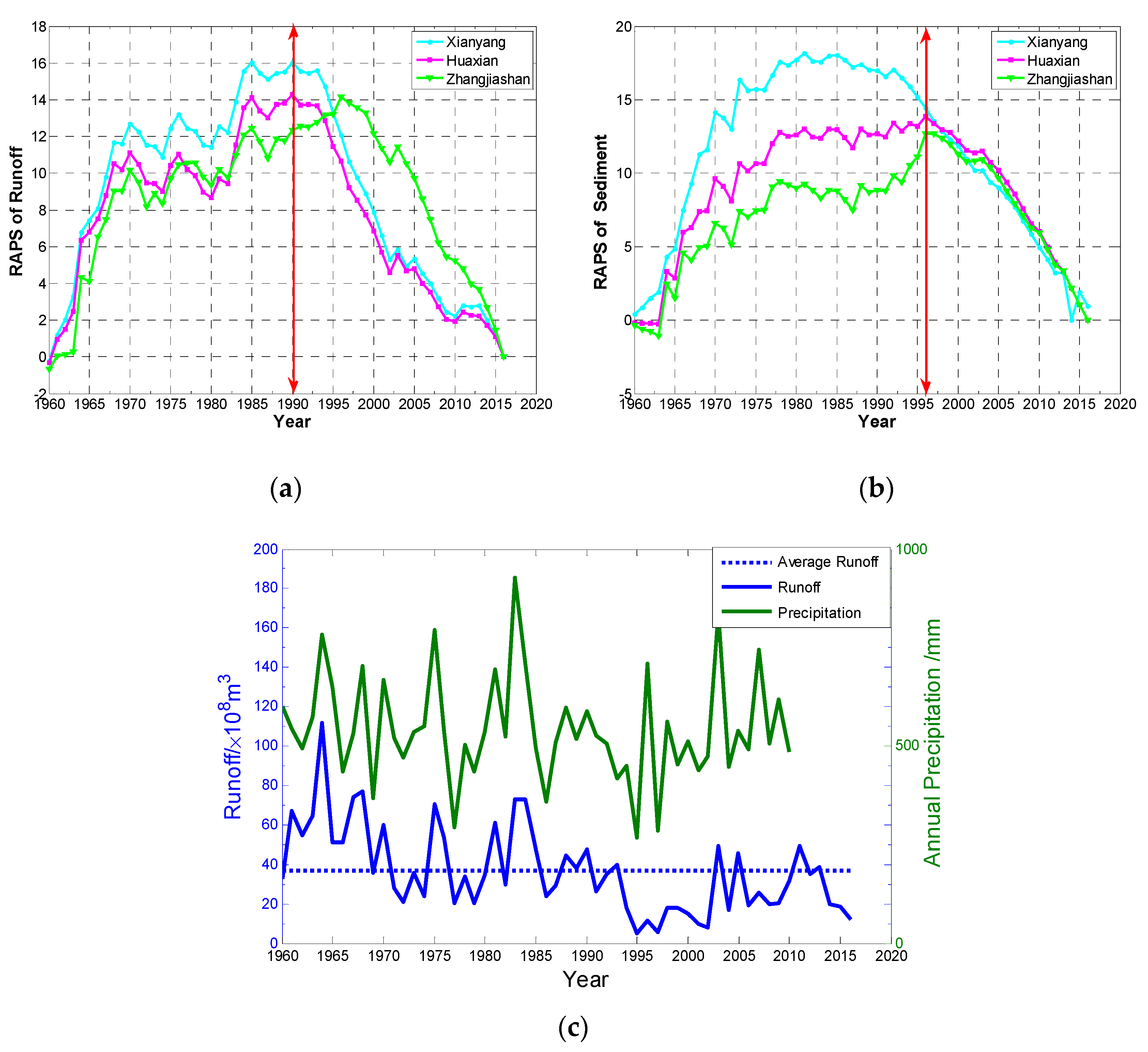
3.1. Division of the Study Intervals
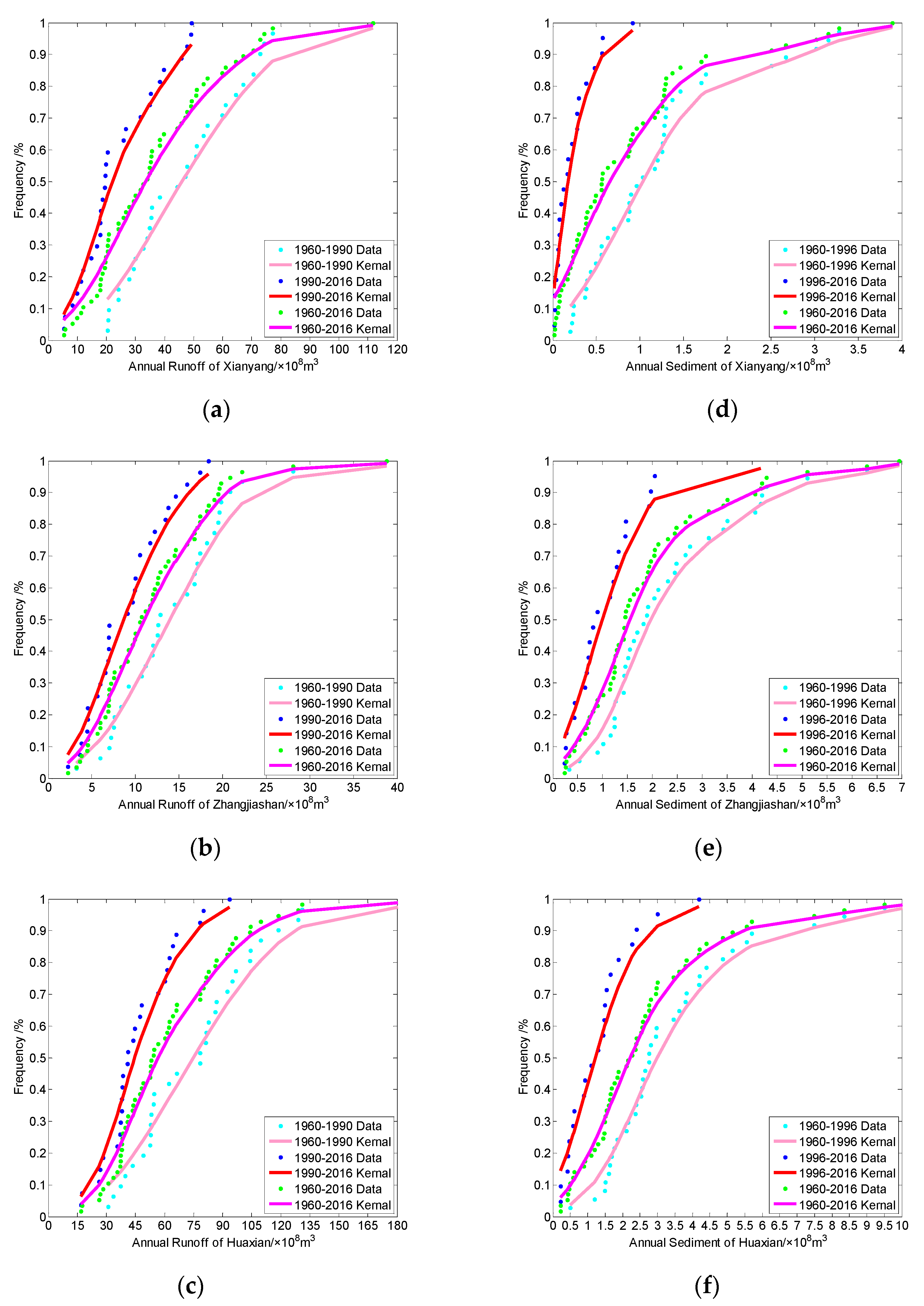
3.2. Establishment of the Marginal Distribution Function
Establishment of the 2D Joint Distribution Functions
3.3. Analysis on the Runoff–Sediment Relationships between the Three Stations through Joint Distribution Functions
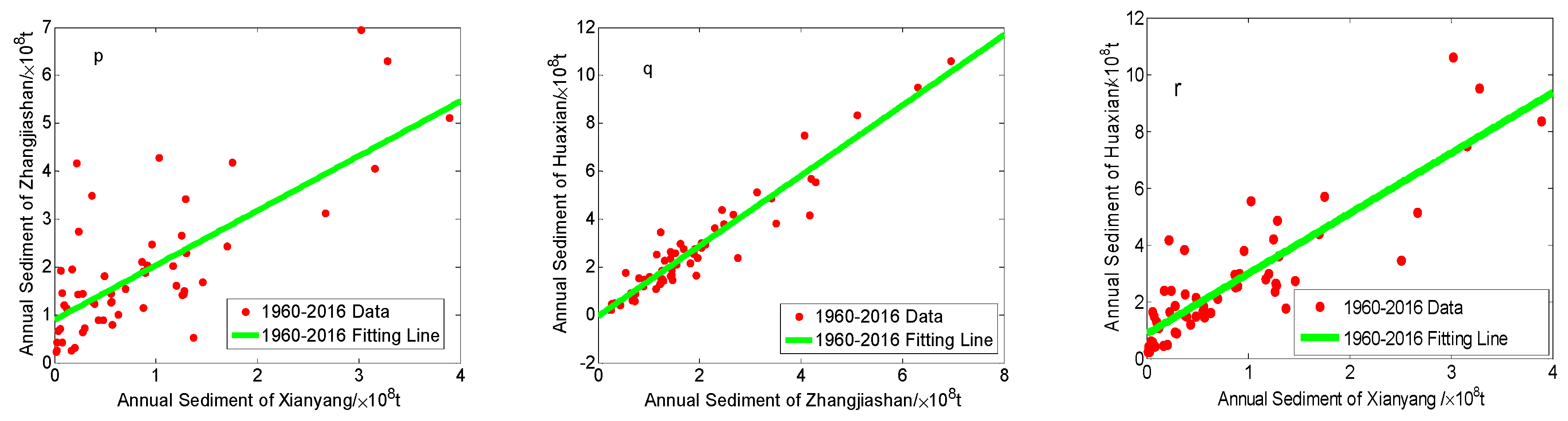
3.3.1. Analysis on Runoff–Sediment Probability of the Three Stations through Pair-Copula Function
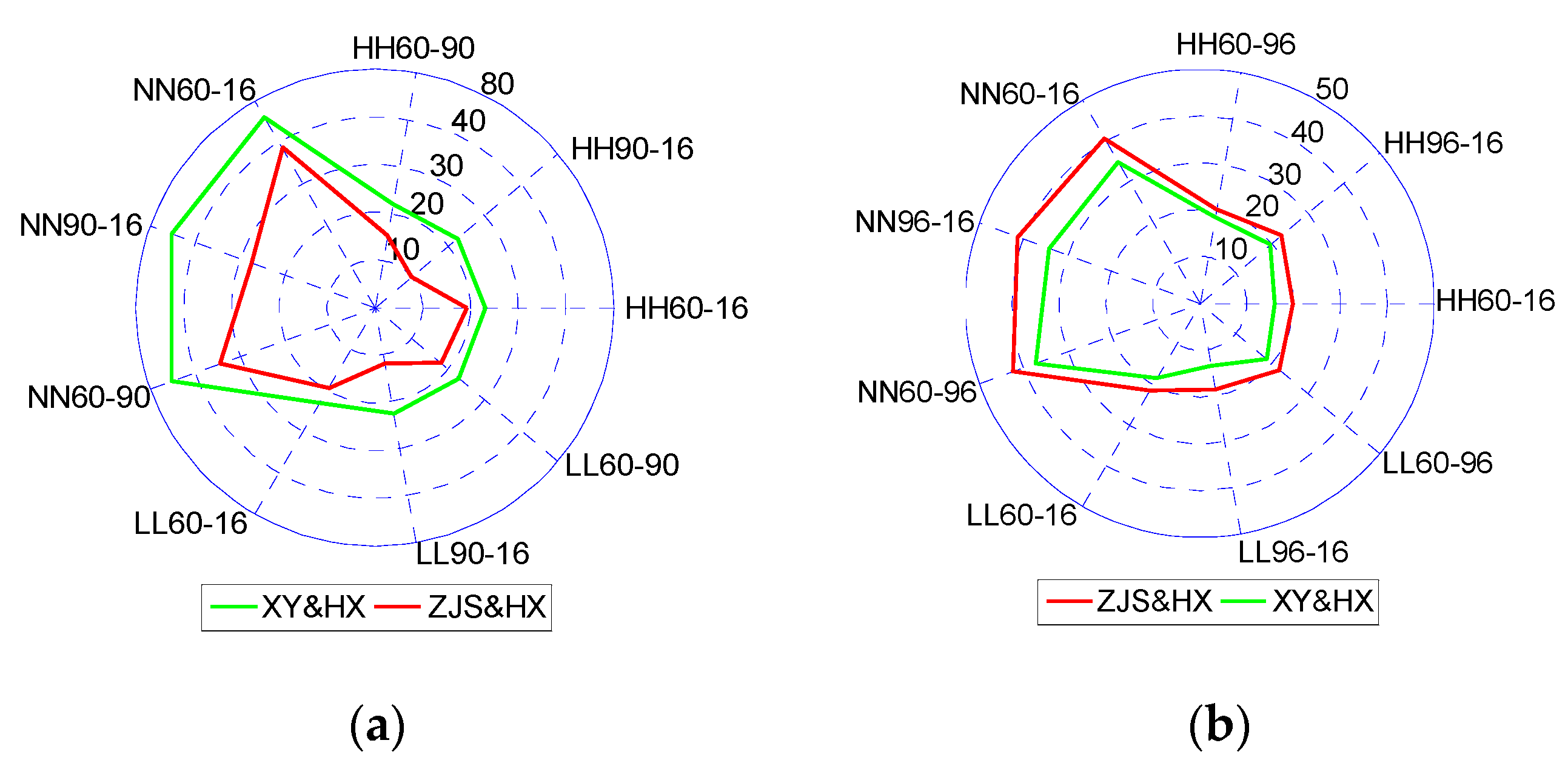
3.3.2. Probability Analysis on High–Low runoff and Sediment Yield of the Three Stations
3.3.3. Probability Control of Runoff and Sediment Yield of the Three Stations
4. Conclusions
- (1)
- The pair-copula function is flexible in adoption and simple in parameter solution. It has obvious advantages, in terms of building multidimensional joint distribution functions. Kernel distribution theory and C-vine pair-copula functions were used to obtain the annual runoff and sediment marginal distribution functions and the runoff–sediment joint distribution functions of the three stations of the Weihe River, in different time intervals, accurately.
- (2)
- The synchronous and asynchronous encounter probabilities of high–low runoff between the three stations, and between each pair of stations, were accurately calculated. Meanwhile, through conditional probability formulas, the probabilities that the high, normal, and low status of runoff and sediment yield of downstream hydrologic stations were caused by different statuses of different upstream stations were also calculated.
- (3)
- The designed runoff–sediment yields of upstream stations were estimated, in order to guarantee the runoff–sediment yields of downstream stations being in a certain range, in high and low runoff years, respectively.
Author Contributions
Funding
Conflicts of Interest
References
- Guo, S.L.; Yan, B.W.; Xiao, Y.; Fang, B.; Zhang, N. Multivariate Hydrological Analysis and Estimation. J. China Hydrol. 2008, 28, 1–7. [Google Scholar]
- Correia, F.N. Multivariate partial duration series in flood risk analysis. In Hydrologic Frequency Modeling; Springer: Dordrecht, The Netherlands, 1987; pp. 541–554. [Google Scholar]
- Kotz, S.; Balakrishnan, N.; Johnson, N.L. Continuous Multivariate Distributions; Wiley: New York, NY, USA, 2000; pp. 31–60. [Google Scholar]
- Lall, U.; Bosworth, K. Multivariate kernel estimation of functions of space and time. In Stochastic and Statistical Methods in Hydrology and Environmental Engineering; Springer: Dordrecht, The Netherlands, 1994; pp. 56–89. [Google Scholar]
- De Michele, C.; Salvadori, G. A generalized Pareto intensity-duration model of storm rainfall exploiting 2-Copulas. J. Geophy. Res. Atmos. 2003, 108, 4067. [Google Scholar] [CrossRef]
- De Michele, C.; Salvadori, G.; Canossi, M.; Petaccia, A.; Rosso, R. Bivariate Statistical Approach to Check Adequacy of Dam Spillway. J. Hydrol. Eng. 2005, 10, 50–57. [Google Scholar] [CrossRef]
- Salvadori, G.; De Michele, C. Statistical characterization of temporal structure of storms. Adv. Water Resour. 2006, 29, 827–842. [Google Scholar] [CrossRef]
- Salvadori, G.; De Michele, C.; De Michele, C. Multivariate return period calculation via survival functions. Water Resour. Res. 2013, 49, 2308–2311. [Google Scholar] [CrossRef]
- Fan, L.; Wang, H.; Liu, Z.; Li, N. Quantifying the relationship between drought and water scarcity using Copulas: Case study of Beijing–Tianjin–Hebei metropolitan areas in China. Water 2018, 10, 1622. [Google Scholar] [CrossRef]
- Razmkhah, H.; AkhoundAli, A.M.; Radmanesh, F.; Saghafian, B. Evaluation of rainfall spatial correlation effect on rainfall-runoff modeling uncertainty, considering 2-copula. Arab. J. Geosci. 2016, 9, 125–138. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, Y.; Hao, Y.; Wang, T.; Yeh, T.C.; Fan, Y.; Zhang, Q. Probabilistic analysis of extreme discharges and precipitations with a nonparametric Copula Model. Water 2018, 10, 823. [Google Scholar] [CrossRef]
- Liu, C.L.; Zhou, Y.W.; Sui, J.; Gao, L. Research of methodology of multivariate analysis of design storm based on 3-copula function. J. Harbin Inst. Technol. 2015, 47, 87–92. [Google Scholar]
- Pham, M.T.; Vernieuwe, H.; De Baets, B.; Willems, P.; Verhoest, N.E.C. Stochastic simulation of precipitation-consistent daily reference evapotranspiration using vine copulas. Stoch. Environ. Res. Risk Assess. 2016, 30, 2197–2214. [Google Scholar] [CrossRef]
- Gyasi-Agyei, Y.; Melching, C.S. Modelling the dependence and internal structure of storm events for continuous rainfall simulation. J. Hydrol. 2012, 464, 249–261. [Google Scholar] [CrossRef]
- Ridolfi, E.; Montesarchio, V.; Rianna, M.; Sebastianelli, S.; Russo, F.; Napolitano, F. Evaluation of Rainfall Thresholds Entropy: Influence of Bivariate Distribution Selection. Irrig. Drain. 2013, 62, 50–60. [Google Scholar] [CrossRef]
- Gräler, B.; van den Berg, M.; Vandenberghe, S.; Petroselli, A.; Grimaldi, S.; De Baets, B.; Verhoest, N. Multivariate return periods in hydrology: A critical and practical review focusing on synthetic design hydrograph estimation. Hydrol. Earth Syst. Sci. 2013, 17, 1281–1296. [Google Scholar] [CrossRef]
- Wahl, T.; Mudersbach, C.; Jensen, J. Assessing the hydrodynamic boundary conditions for risk analyses in coastal areas: A multivariate statistical approach based on Copula functions. Nat. Hazards Earth Syst. Sci. 2012, 12, 495–510. [Google Scholar] [CrossRef]
- Chen, L.; Singh, V.P.; Guo, S.L.; Hao, Z.C.; Li, T.Y. Flood Coincidence Risk Analysis Using Multivariate Copula Functions. J. Hydrol. Eng. 2012, 17, 742–755. [Google Scholar] [CrossRef]
- Guo, A.J.; Chang, J.X.; Wang, Y.M.; Huang, Q. Variations in the Runoff–sediment Relationship of the Weihe River Basin Based on the Copula Function. Water 2016, 8, 223. [Google Scholar] [CrossRef]
- Wang, M.L.; Xia, C.Y.; Lu, C.S.; Huang, J. Analysis of variation trend of water and sediment in jinsha river basin. Yangtze River 2008, 39, 15–22. [Google Scholar]
- Wang, P.; Fang, G.; Guo, Y.; Wen, X. Reseach on water resources optimal scheduling based on improved Quantum Genetic algorithm. J. China Three Gorges Univ. (Nat. Sci.) 2016, 38, 7–13. [Google Scholar]
- Fu, Y. Inflow Analysis and Optimal Dispatching of Jinpen Reservoir in Shaanxi Province; Xi’an University of Technology: Xi’an, China, 2004; pp. 36–41. [Google Scholar]
- Huang, C.; Wang, Z.; Li, S.; Chen, S. A multi-reservoir operation optimization model and application in the upper Yangtze River Basin I. Principle and solution of the model. J. Hydrol. Eng. 2014, 45, 1009–1019. [Google Scholar]
- Guan, S.; Lin, Y.; Zha, X.; Ding, B.; Tao, N.; Liu, Z. Copula Function-Based Flood Coincidence Probability Analysis for Mainstream and Tributary of the Hanjiang River Basin. Acta Sci. Nat. Univ. Sunyatseni 2015, 54, 130–137. [Google Scholar]
- Huaxiong, L.; Yu, K.; Gottschalk, L. Estimation of the distribution of annual runoff from climatic variables using copulas. Water Resour. Res. 2014, 50, 7134–7152. [Google Scholar]
- Vernieuwe, H.; Vandenberghe, S.; De Baets, B.; Verhoest, N.E.C. A continuous rainfall model based on vine copulas. Hydrol. Earth Syst. Sci. 2015, 19, 2685–2699. [Google Scholar] [CrossRef]
- Poduje, A.C.C.; Haberlandt, U. Spatio-Temporal Synthesis of Continuous Precipitation Series Using Vine Copulas. Water 2018, 10, 862. [Google Scholar] [CrossRef]
- Shafaei, M.; Fakheri-Fard, A.; Dinpashoh, Y.; Mirabbasi, R.; De Michele, C. Modeling flood event characteristics using D-vine structures. Theor. Appl. Climatol. 2017, 130, 713–724. [Google Scholar] [CrossRef]
- Huang, S.; Liu, D.; Huang, Q.; Chen, Y. Contributions of climate variability and human activities to the variation of runoff in the Wei River Basin, China. Hydrol. Sci. J. 2016, 61, 1026–1039. [Google Scholar] [CrossRef]
- Zhu, H.Y.; Han, C.B.; Jia, Z.F.; Liu, Y.; Li, P.C. Analysis on characteristics of runoff and sediment of Zhangjiashan hydrological station and case study for Jing he River. Trans. CSAE 2012, 28, 48–55. [Google Scholar]
- Gao, P.; Geissen, V.; Ritsema, C.J.; Mu, X.M.; Wang, F. Impact of climate change and anthropogenic activities on stream flow and sediment discharge in the Wei River basin, China. Hydrol. Earth Syst. Sci. 2013, 17, 961–972. [Google Scholar] [CrossRef]
- Zhang, J.P.; Ding, Z.H.; Guo, B.T. Study on evolution trends and encounter risk of runoff and sediment in Jinghe river. Hydrol. Eng. 2015, 34, 11–16. (In Chinese) [Google Scholar]
- Chang, J.X.; Wang, Y.M.; Istanbulluoglu, E.; Bai, T.; Huang, Q.; Yang, D.W.; Huang, S.Z. Impact of climate change and human activities on runoff in the Weihe River Basin, China. Quat. Int. 2015, 380, 169–179. [Google Scholar] [CrossRef]
- Tadic, L.; Bonacci, O.; Dadic, T. Analysis of the Drava and Danube rivers floods in Osijek (Croatia) and possibility of their coincidence. Environ. Earth Sci. 2016, 9, 1238. [Google Scholar] [CrossRef]
- Sklar, A. Fonction de répartition à n dimensions et leurs marges. Publ. Inst. Stat. Univ. Paris 1959, 8, 229–231. [Google Scholar]
- Joe, H. Families of m-variate distributions with given margins and m(m−1)/2 bivariate dependence parameters. Distributions with Fixed Marginals and Related Topics. Lect. Notes-Monogr. Ser. 1996, 28, 120–141. [Google Scholar]
- Aas, K.; Czado, C.; Frigessi, A.; Bakken, H. Pair-copula constructions of multiple dependence. Insur. Math. Econ. 2009, 44, 182–198. [Google Scholar] [CrossRef]
- Rosenblatt, M. Remarks on a Multivariate Transformation. Ann. Math. Stat. 1952, 23, 470–472. [Google Scholar] [CrossRef]
- Genest, C.; Rémillard, B.; Beaudoin, D. Goodness-of-fit tests for copulas: A review and a power study. Insur. Math. Econ. 2009, 44, 199–213. [Google Scholar] [CrossRef]
- Zeng, Z. Application of Pair-Copulas Function in Drought Characteristics Analysis; Northwest A&F University: Yangling, China, 2012; pp. 24–27. [Google Scholar]
- You, Q.Y.; Liu, Y.; Liu, Z. Probability analysis of the water table and driving factors using a multidimensional Copula Function. Water 2018, 10, 472. [Google Scholar] [CrossRef]
- R Copula Package: Program R. R Version 3.5.0. Available online: https://cran.r-project.org/package=copula (accessed on 23 April 2018).
- R CDVine Package: Program R. R Version 3.5.0. Available online: https://cran.r-project.org/package=CDVine (accessed on 29 October 2015).
- Chen, F. Analysis of water-sand evolution characteristics in the lower reaches of weihe river. Shaanxi Water Resour. 2010, 1, 29–32. [Google Scholar]
- Tu, X. The Wei River Hydrology; Shaanxi Science and Technology Press: Xi’an, China, 2012; ISBN 9787536956865. [Google Scholar]

| Runoff | Year | 1960–1990 | 1990–2016 | 1960–2016 | |||
| D | p-Value | D | p-Value | D | p-Value | ||
| Xianyang | 0.0972 | 0.2903 | 0.1266 | 0.0610 | 0.0652 | 0.5564 | |
| Zhangjiashan | 0.0888 | 0.4695 | 0.1062 | 0.2348 | 0.0507 | 0.9680 | |
| Huaxian | 0.0933 | 0.3636 | 0.1018 | 0.3157 | 0.0663 | 0.5824 | |
| Sediment | Year | 1960–1996 | 1996–2016 | 1960–2016 | |||
| D | p-Value | D | p-Value | D | p-Value | ||
| Xianyang | 0.0982 | 0.1818 | 0.1171 | 0.3157 | 0.1156 | 0.0718 | |
| Zhangjiashan | 0.0681 | 0.9161 | 0.0995 | 0.6723 | 0.0646 | 0.6923 | |
| Huaxian | 0.0986 | 0.1858 | 0.1062 | 0.4715 | 0.0642 | 0.6703 | |
| C-Vine | Year | Variables | Copula | θ | p-Value |
|---|---|---|---|---|---|
| Runoff | 1960–1990 | 3, 2 | Frank | 11.83 | 0.42 |
| 3, 1 | Frank | 36.22 | |||
| 1, 2; 3 | Frank | −0.69 | |||
| 1990–2016 | 3, 2 | Gumbel | 1.35 | 0.53 | |
| 3, 1 | Frank | 29.21 | |||
| 1, 2; 3 | Frank | −0.91 | |||
| 1960–2016 | 3, 2 | Gumbel | 2.39 | 0.31 | |
| 3, 1 | Gumbel | 8.14 | |||
| 1, 2; 3 | Frank | −0.03 | |||
| Sediment | 1960–1996 | 3, 1 | Gumbel | 2.50 | 0.07 |
| 3, 2 | Gumbel | 4.59 | |||
| 2, 1; 3 | Frank | −7.73 | |||
| 1996–2016 | 3, 1 | Clayton | 2.82 | 0.40 | |
| 3, 2 | Clayton | 7.18 | |||
| 2, 1; 3 | Frank | −4.96 | |||
| 1960–2016 | 3, 1 | Frank | 10.27 | 0.31 | |
| 3, 2 | Frank | 16.35 | |||
| 1, 2; 3 | Frank | −4.44 |
| Year | LLL | NNN | HHH | Synchronous | Asynchronous | |
|---|---|---|---|---|---|---|
| Runoff | 1960–1990 | 0.1815 | 0.3626 | 0.1815 | 0.7256 | 0.2744 |
| 1990–2016 | 0.0886 | 0.2477 | 0.1082 | 0.4445 | 0.5555 | |
| 1960–2016 | 0.1427 | 0.3146 | 0.1720 | 0.6293 | 0.3707 | |
| Sediment | 1960–1996 | 0.1154 | 0.2833 | 0.1541 | 0.5528 | 0.4472 |
| 1996–2016 | 0.1778 | 0.2723 | 0.0879 | 0.5320 | 0.4680 | |
| 1960–2016 | 0.1485 | 0.3075 | 0.1596 | 0.6156 | 0.3844 |
| Year | P(Hxy|Hhx) | P(Hzjs|Hhx) | P(Nxy|Hhx) | P(Nzjs|Hhx) | P(Lxy|Hhx) | P(Lzjs|Hhx) | |
|---|---|---|---|---|---|---|---|
| Runoff | 1960–1990 | 0.9236 | 0.7744 | 0.0764 | 0.2248 | 0 | 0.0008 |
| 1990–2016 | 0.9052 | 0.4732 | 0.0948 | 0.4128 | 0 | 0.1140 | |
| 1960–2016 | 0.9244 | 0.7232 | 0.0756 | 0.2636 | 0 | 0.0132 | |
| Sediment | 1960–1996 | 0.7364 | 0.8624 | 0.2528 | 0.1372 | 0.0108 | 0.0004 |
| 1996–2016 | 0.5648 | 0.7488 | 0.4264 | 0.2512 | 0.0088 | 0 | |
| 1960–2016 | 0.7452 | 0.8784 | 0.2528 | 0.1216 | 0.0020 | 0 |
| Year | P(Hxy|Lhx) | P(Hzjs|Lhx) | P(Nxy|Lhx) | P(Nzjs|Lhx) | P(Lxy|Lhx) | P(Lzjs|Lhx) | |
|---|---|---|---|---|---|---|---|
| Runoff | 1960–1990 | 0 | 0.0008 | 0.0764 | 0.2248 | 0.9236 | 0.7744 |
| 1990–2016 | 0 | 0.1140 | 0.0948 | 0.4916 | 0.9052 | 0.3944 | |
| 1960–2016 | 0 | 0.0132 | 0.1160 | 0.3596 | 0.8840 | 0.6272 | |
| Sediment | 1960–1996 | 0.0108 | 0.0004 | 0.3472 | 0.2020 | 0.642 | 0.7976 |
| 1996–2016 | 0.0088 | 0 | 0.2064 | 0.0920 | 0.7848 | 0.9080 | |
| 1960–2016 | 0.0020 | 0 | 0.2416 | 0.1808 | 0.7564 | 0.8192 |
| Typical Conditional Probability | |
|---|---|
| Runoff | P(40.82 < Runoffhx < 48.10|26.50 > Runoffxy > 18.70, 10.10 > Runoffzjs > 7.00) = 0.7780 |
| P(32.50 < Runoffhx < 17.80|14.50 > Runoffxy > 5.80, 5.60 > Runoffzjs > 2.30) = 0.7055 | |
| Sediment | P(1.49 > Sedihx > 0.92|0.22 > Sedixy > 0.12, 1.21 > Sedizjs > 0.80) = 0.8156 |
| P(0.57 > Sedihx > 0.41|0.074 > Sedixy > 0.06, 0.61 > Sedizjs > 0.22) = 0.9517 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
You, Q.; Jiang, H.; Liu, Y.; Liu, Z.; Guan, Z. Probability Analysis and Control of River Runoff–sediment Characteristics based on Pair-Copula Functions: The Case of the Weihe River and Jinghe River. Water 2019, 11, 510. https://doi.org/10.3390/w11030510
You Q, Jiang H, Liu Y, Liu Z, Guan Z. Probability Analysis and Control of River Runoff–sediment Characteristics based on Pair-Copula Functions: The Case of the Weihe River and Jinghe River. Water. 2019; 11(3):510. https://doi.org/10.3390/w11030510
Chicago/Turabian StyleYou, Qiying, Hao Jiang, Yan Liu, Zhao Liu, and Zilong Guan. 2019. "Probability Analysis and Control of River Runoff–sediment Characteristics based on Pair-Copula Functions: The Case of the Weihe River and Jinghe River" Water 11, no. 3: 510. https://doi.org/10.3390/w11030510
APA StyleYou, Q., Jiang, H., Liu, Y., Liu, Z., & Guan, Z. (2019). Probability Analysis and Control of River Runoff–sediment Characteristics based on Pair-Copula Functions: The Case of the Weihe River and Jinghe River. Water, 11(3), 510. https://doi.org/10.3390/w11030510




