An Improved Grid-Xinanjiang Model and Its Application in the Jinshajiang Basin, China
Abstract
1. Introduction
2. Improved GXAJ Model Formulation
2.1. Two-Source Potential Evapotranspiration (TSPE) Model
2.2. Infiltration and Saturation Excess Runoff Model (ISER)
2.3. Nonlinear Muskingum–Cunge Routing Method
2.4. Schematic Diagram of the Integration of the ANNs with Conceptual Models
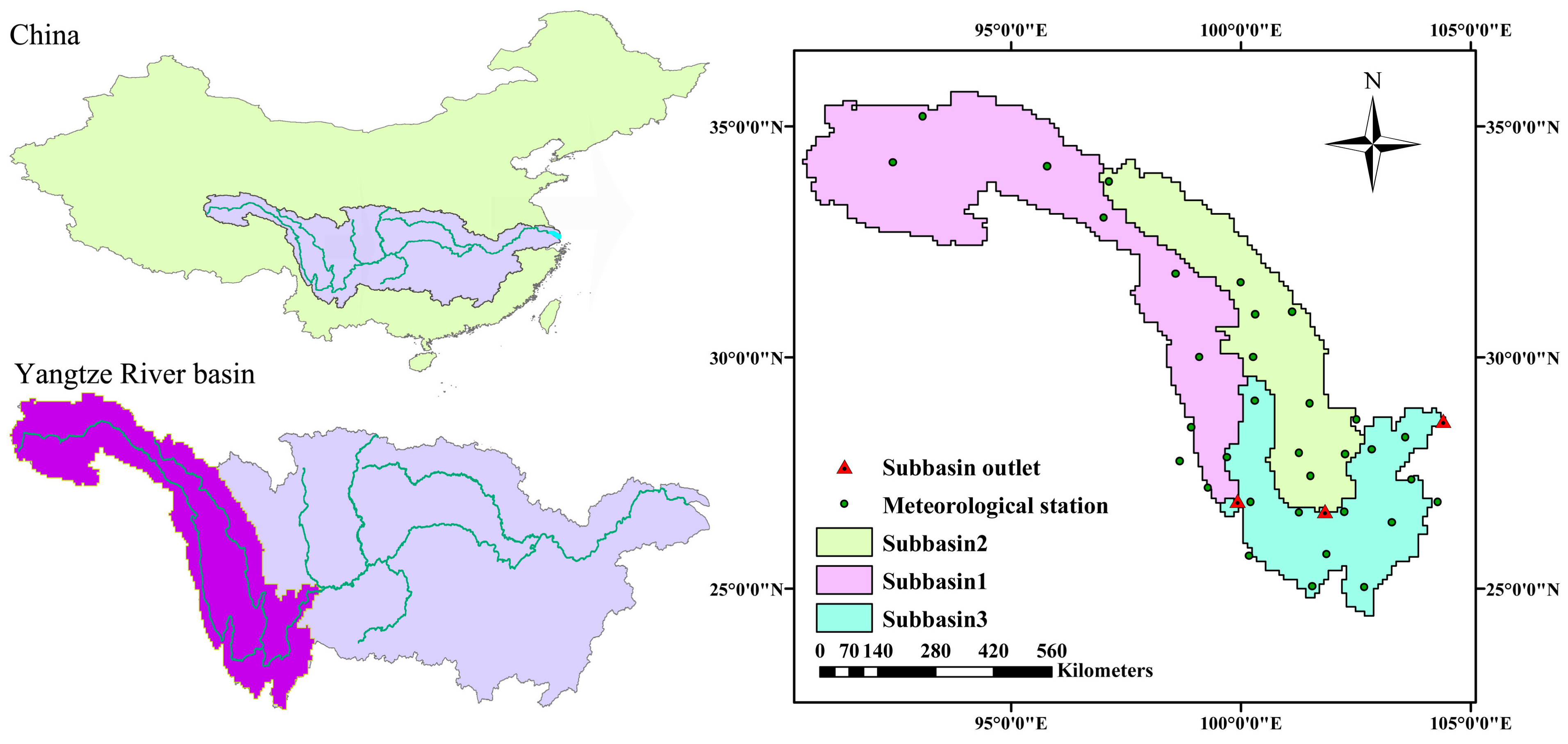
3. A Brief Description of the Study Area
4. Materials and Methods
4.1. Topographical Land Cover Data
4.2. Modis LAI
4.3. Meteorological Data
4.4. A Prior Parameter Estimation
5. Results and Discussion
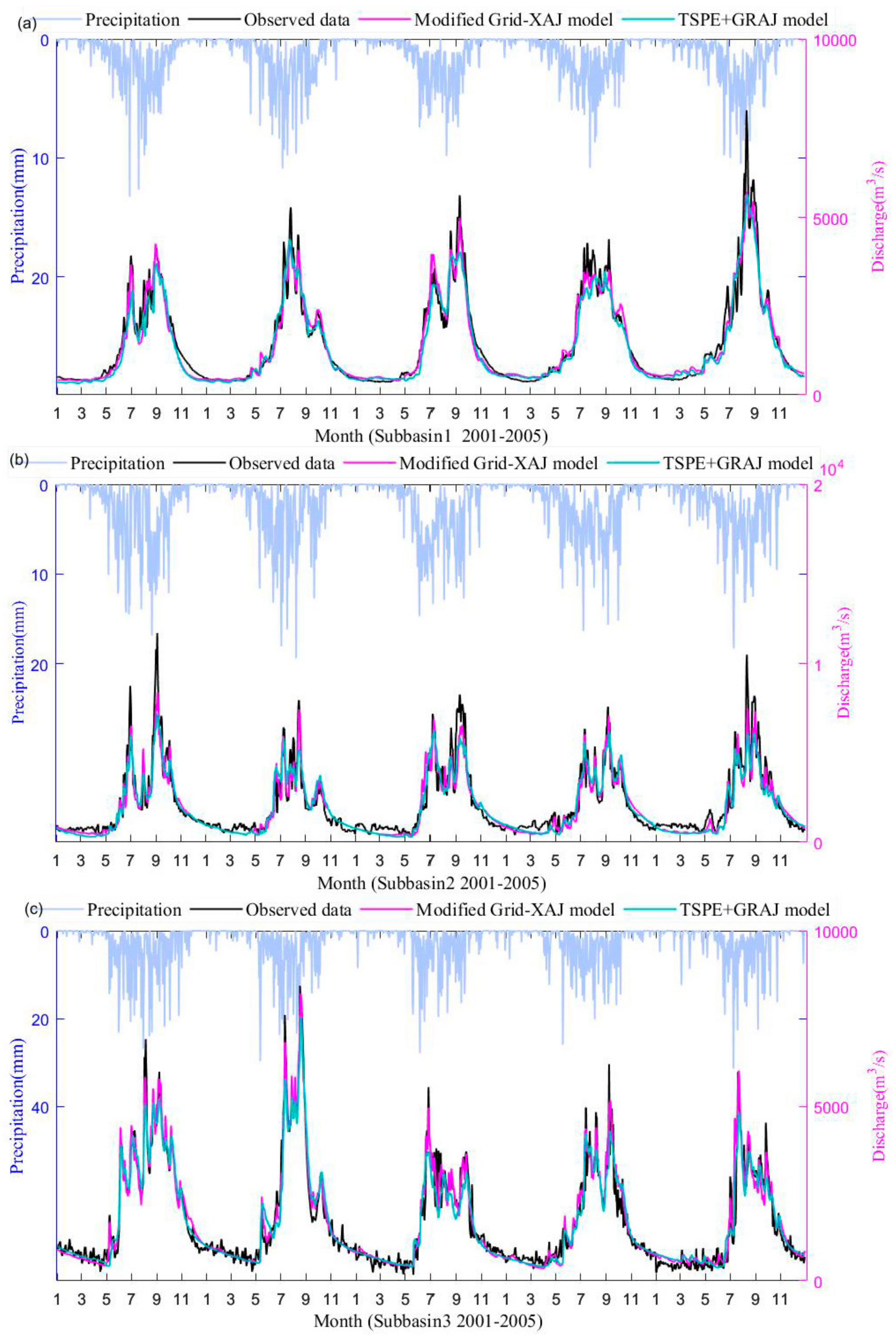
5.1. Evaluation of the Simulated Streamflow
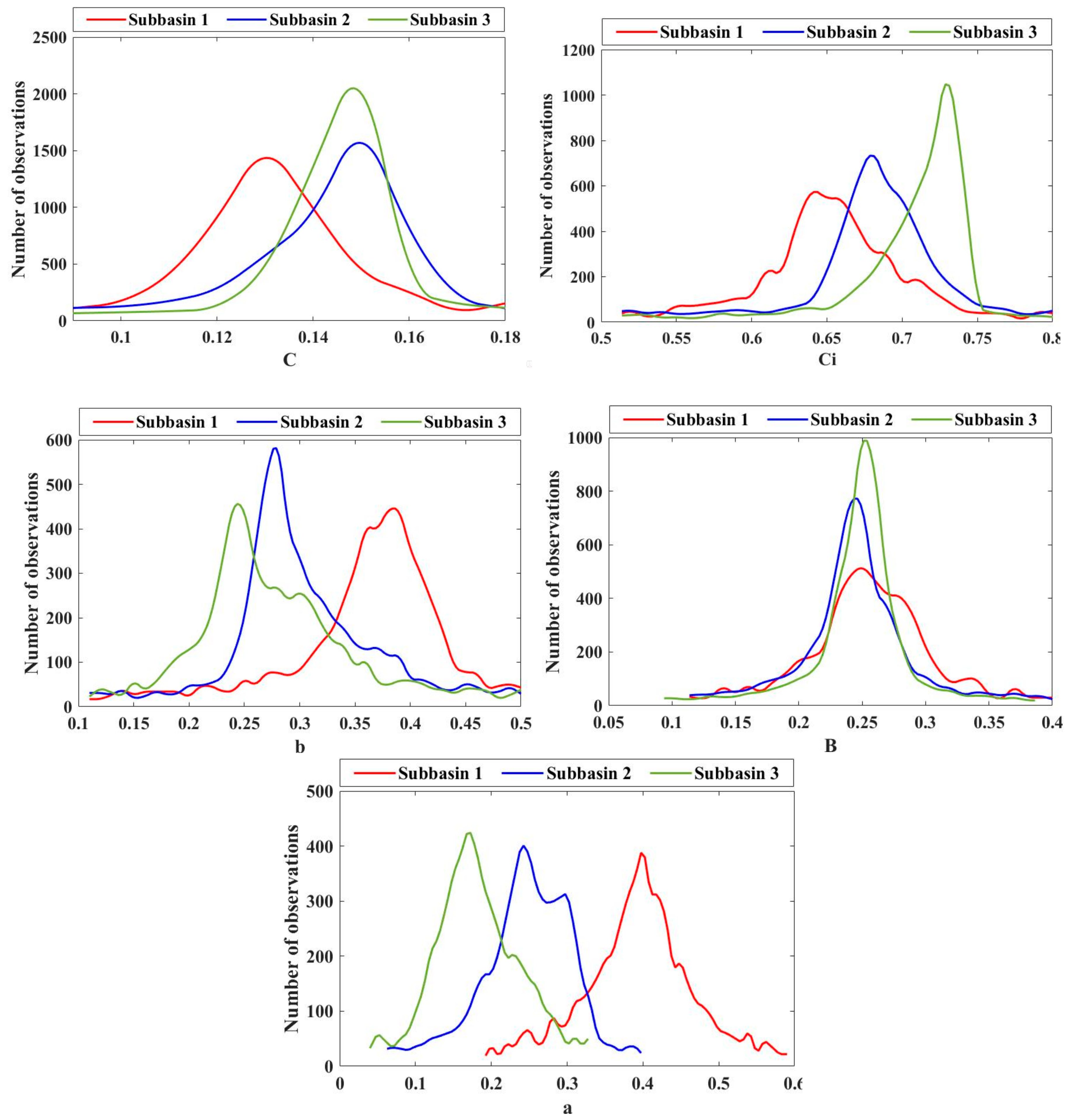
5.2. Uncertainty Analysis
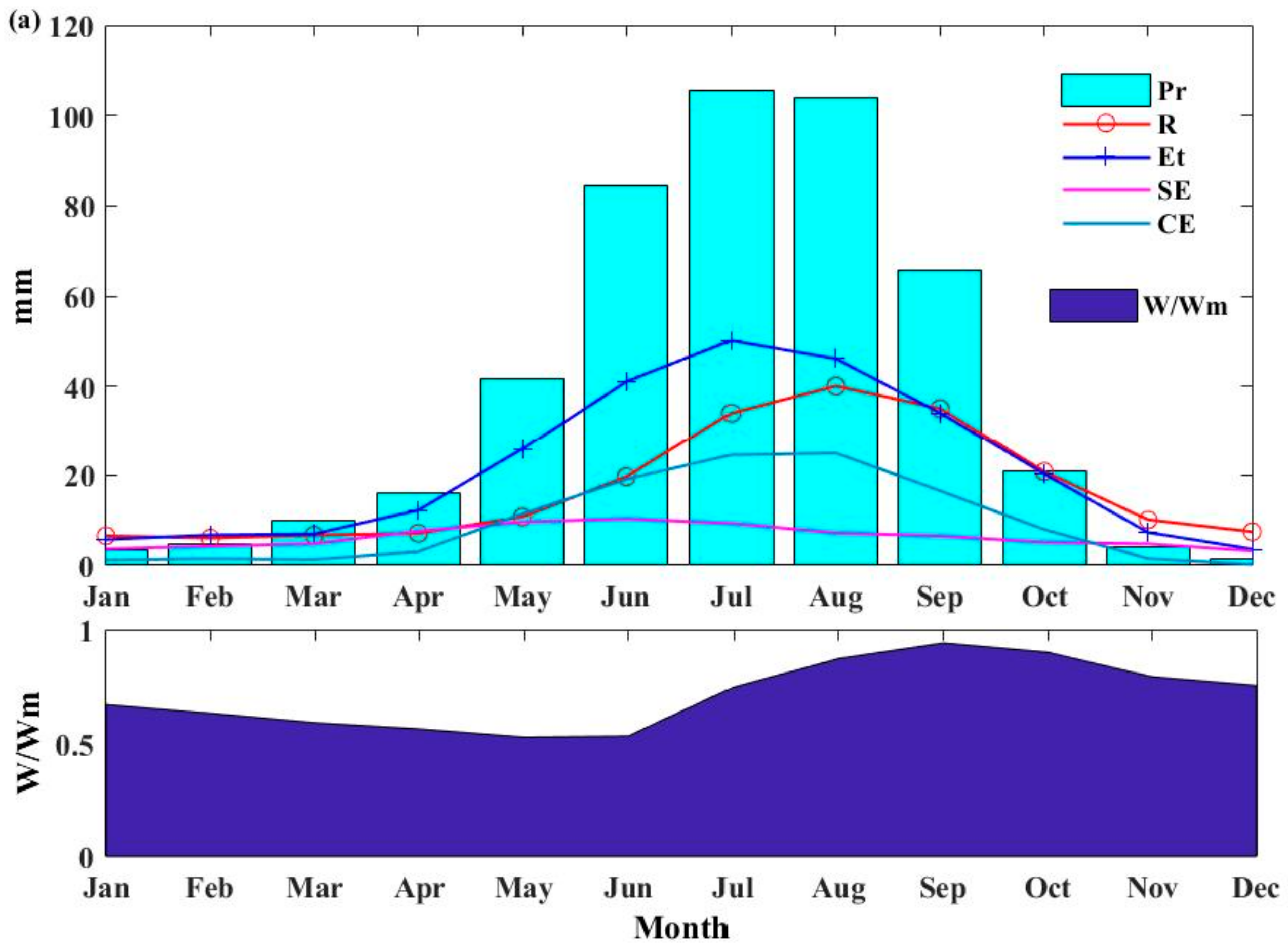
5.3. Annual Water Budget Simulated by the Modified Xinanjiang Model
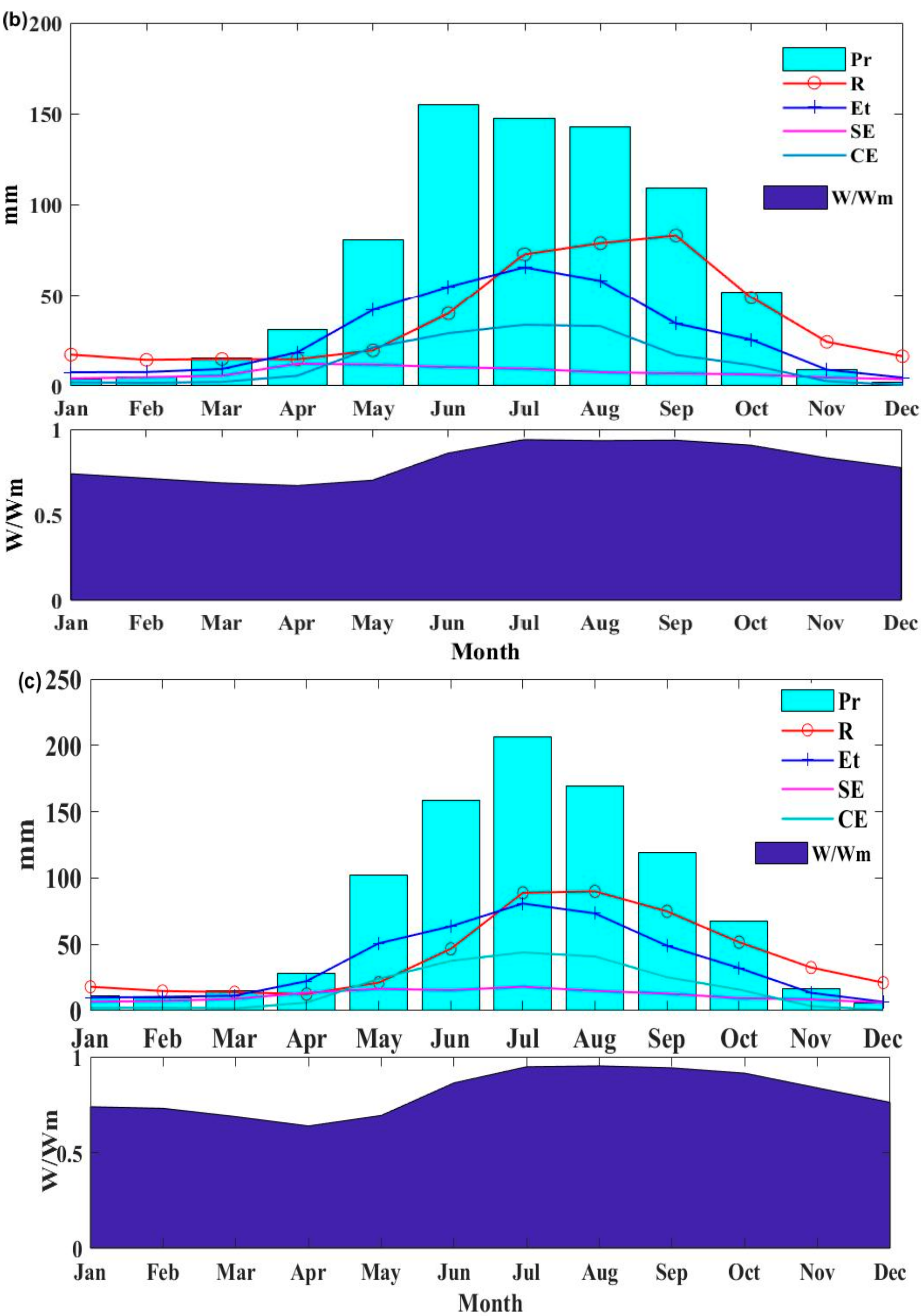
5.4. Seasonal Variation of the Evapotranspiration and the Soil Moisture
5.5. Spatial Patterns of the Annual Precipitation and Evapotranspiration
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| GXAJ | Grid-Xinanjiang model |
| TSPE | two-source potential evapotranspiration model |
| CT | canopy transpiration |
| IE | interception evaporation |
| SE | soil evaporation |
| PE | potential evapotranspiration |
| DEM | digital elevation model |
| MODIS | Moderate Resolution Imaging Spectroradiometer |
| LAI | leaf area index |
| BNU | Beijing Normal University |
| XAJ | Xinanjiang |
| IER | infiltration excess runoff |
| SER | saturation excess runoff |
| AE | actual evapotranspiration |
| NMC | nonlinear Muskingum–Cunge |
| SR | surface runoff |
| TWC | tension water capacity |
| SIC | soil infiltration capacity |
| ISER | Infiltration and saturation excess runoff model |
| FWC | free water capacity |
| SRTM | shuttle radar topography mission |
| MMAP | measured mean annual precipitation |
| GR | groundwater runoff |
References
- Hu, C.; Guo, S.; Xiong, L.; Peng, D. A modified Xinanjiang model and its application in northern China. Nord. Hydrol. 2005, 36, 175–192. [Google Scholar] [CrossRef]
- Zhao, R.; Liu, X. The Xinanjiang model. In Computer Models of Watershed Hydrology; Singh, V.P., Ed.; Water Resources Publications: Littleton, CO, USA, 1995. [Google Scholar]
- Gan, T.Y.; Dlamini, E.M.; Biftu, G.F. Effects of model complexity and structure, data quality, and objective functions on hydrologic modeling. J. Hydrol. 1997, 192, 81–103. [Google Scholar] [CrossRef]
- Jayawardena, A.; Zhou, M. A modified spatial soil moisture storage capacity distribution curve for the Xinanjiang model. J. Hydrol. 2000, 227, 93–113. [Google Scholar] [CrossRef]
- Zhang, D.; Zhang, L.; Guan, Y.; Chen, X.; Chen, X. Sensitivity analysis of Xinanjiang rainfall–runoff model parameters: a case study in Lianghui, Zhejiang province, China. Hydrol. Res. 2012, 43, 123–134. [Google Scholar] [CrossRef]
- Xu, H.; Xu, C.; Chen, H.; Zhang, Z. Assessing the influence of rain gauge density and distribution on hydrological model performance in a humid region of China. J. Hydrol. 2013, 505, 1–12. [Google Scholar] [CrossRef]
- Yao, C.; Li, Z.; Bao, H.; Yu, Z. Application of a developed Grid-Xinanjiang model to Chinese watersheds for flood forecasting purpose. J. Hydrol. Eng. 2009, 14, 923–934. [Google Scholar] [CrossRef]
- Yao, C.; Li, Z.; Yu, Z.; Zhang, K. A priori parameter estimates for a distributed, grid-based Xinanjiang model using geographically based information. J. Hydrol. 2012, 468, 47–62. [Google Scholar] [CrossRef]
- Ren, G.; Guo, J. Change in pan evaporation and influential factors over China:1956–2000. J. Nat. Resour. 2006, 21, 31–44. (In Chinese) [Google Scholar]
- Wang, Y.; Liu, B.; Zhai, J.; Su, B.; Luo, Y.; Zhang, Z. Relationship between potential and actual evaporation in Yangtze River Basin. Adv. Clim. Chang. Res. 2011, 6, 393–399. (In Chinese) [Google Scholar]
- Penman, H.L. Natural evaporation from open water, hare soil and grass. Proc. R. Soc. Lond. Ser. A 1948, 193, 120–145. [Google Scholar] [CrossRef]
- Monteith, J.L. Evaporation and the Environment, the State and Movement of Water in Living Organisms; Cambridge University Press: London, UK, 1965; pp. 205–234. [Google Scholar]
- Allen, R.G.; Pereira, L.S.; Raes, D.; Smith, M. Crop evapotranspiration. In Food and Agriculture Organisation Irrigation and Drainage Paper 56; Food and Agriculture Organization: Rome, Italy, 1988. [Google Scholar]
- Choudhury, B.J.; Monteith, J.L. A four-layer model for the heat budget of homogeneous land surfaces. Q. J. R. Meteorolog. Soc. 1988, 114, 373–398. [Google Scholar] [CrossRef]
- Yuan, F.; Ren, L.; Yu, Z.; Xu, J. Potential evapotranspiration computation using a two-source method for the Xin’anjiang hydrological model. J. Hydrol. Eng. 2008, 13, 305–316. [Google Scholar] [CrossRef]
- Holtan, H.N. A Concept for Infiltration Estimates in Watershed Engineering. Agric. Res. 1961, 150, B16–B25. [Google Scholar]
- Getirana, A.C.V.; Boone, A.; Peugeot, C. Evaluating LSM-based water budgets over a west African basin assisted with a river routing scheme. J. Hydrometeorol. 2014, 15, 2331–2346. [Google Scholar] [CrossRef]
- Gangopadhyay, M.; Uryvaev, V.A.; Oman, M.H.; Nordenson, T.J.; Harbeck, G.E. Measurement and Estimation of Evaporation and Evapotranspiration; WMO: Geneva, Switzerland, 1966. [Google Scholar]
- Liu, X.; Ren, L.; Yuan, F.; Singh, V.P.; Fang, X.; Yu, Z.; Zhang, W. Quantifying the effect of land use and land cover changes on green water and blue water in northern part of China. Hydrol. Earth Syst. Sci. 2009, 13, 735–747. [Google Scholar] [CrossRef]
- MO, X.; LIN, Z.; LIU, S. An improvement of the dual-source model based on Penman-Monteith formula. J. Hydrol. Eng. 2000, 5, 6–11. [Google Scholar]
- Xu, J.; Ren, L.; Ruan, X.; Liu, X.; Yuan, F. Development of a physically based PDSI and its application for assessing the vegetation response to drought in northern China. J. Geophys. Res. Atmos. 2012, 117, D8. [Google Scholar] [CrossRef]
- Jinshajiang River Basin in China. Data Center for Resources and Environmental Sciences, Chinese Academy of Sciences (RESDC). Available online: http://www.resdc.cn/data.aspx?DATAID=141 (accessed on 12 May 2016).
- Changjiang & Southwest Rivers Water Resources Bulletin, Changjiang Water Resources Commission of the Ministry of Water Resources. Available online: http://www.cjw.gov.cn/zwzc/bmgb/ (accessed on 12 May 2016). (In Chinese)
- Fu, B.; Lu, Q. Atlas of Microclimate over Shanxi Basin; Nanjing University Press: Nanjing, China, 1990. (In Chinese) [Google Scholar]
- Song, X.; Kong, F.; Zhan, C.; Han, J.; Zhang, X. Parameter identification and global sensitivity analysis of Xin’anjiang model using meta-modeling approach. Water Sci. Eng. 2013, 6, 1–17. [Google Scholar]
- Zhao, R.; Wang, P. Parameter analysis for Xinanjiang model. J. China Hydrol. 1988, 6, 2–9. Available online: http://www.cnki.com.cn/Article/CJFDTotal-SWZZ198806000.htm (accessed on 12 May 2016). (In Chinese).
- Zhao, R.; Wang, P.; Hu, F. Relations between parameter values and corresponding natural conditions of Xinanjiang model. J. Hohai Univ. 1992, 20, 52–59. (In Chinese) [Google Scholar]
- Shu, C.; Liu, S.; Mo, X.; Liang, Z.; Dai, D. Uncertainty analysis of Xinanjiang model parameter. Geogr. Res. 2008, 27, 343–352. (In Chinese) [Google Scholar]
- Anderson, R.; Koren, V.; Reed, S. Using SSURGO data to improve Sacramento Model a priori parameter estimates. J. Hydrol. 2006, 320, 103–116. [Google Scholar] [CrossRef]
- Koren, V.; Smith, M.; Wang, D.; Zhang, Z. Use of Soil Property Data in the Derivation of Conceptual Rainfall–Runoff Model Parameters, 2000. Available online: http://www.nws.noaa.gov/oh/hrl/modelcalibration/3.%20%20A%20priori%20model%20parameters/ams_2000_koren_soils_parameters.pdf (accessed on 12 May 2016).
- Duan, Q.Y.; Gupta, V.K.; Sorooshian, S. Shuffled complex evolution approach for effective and efficient global minimization. J. Optim. Theory Appl. 1993, 76, 501–521. [Google Scholar] [CrossRef]
- Duan, Q.; Sorooshian, S.; Gupta, V.K. Optimal use of the SCE-UA global optimization method for calibrating watershed models. J. Hydrol. 1994, 158, 265–284. [Google Scholar] [CrossRef]
- Nash, J.E.; Sutcliffe, J.V. River flow forecasting through conceptual models, Part-I: A discussion of principles. J. Hydrol. 1970, 10, 282–290. [Google Scholar] [CrossRef]
- Walega, A.; Ksiazek, L. Influence of rainfall data on the uncertainty of flood simulation. Soil Water Res. 2016, 11. [Google Scholar] [CrossRef]
- Blasone, R.S. Parameter Estimation and Uncertainty Assessment in Hydrological Modelling. Ph.D. Thesis, Technical University of Denmark, Copenhagen, Denmark, August 2007. [Google Scholar]
- Jin, X.; Xu, C.; Zhang, Q.; Singh, V.P. Parameter and modeling uncertainty simulated by GLUE and a formal Bayesian method for a conceptual hydrological model. J. Hydrol. 2010, 383, 147–155. [Google Scholar] [CrossRef]
- Xiong, L.; Wan, M.; Wei, X. Indices for assessing the prediction bounds of hydrological models and application by generalized likelihood uncertainty estimation. Hydrol. Sci. J. 2009, 54, 852–871. [Google Scholar] [CrossRef]




| Watershed | Area (km2) | Evaporation (mm) | PE (mm) | Temperature (°C) | Runoff (mm) | Runoff Coeff | Climate Zone |
|---|---|---|---|---|---|---|---|
| Sub-basin 1 | 214,184 | 461.5 | 1351.54 | 0.44 | 201.90 | 0.44 | Semi-arid, semi-humid |
| Sub-basin 2 | 136,000 | 753.16 | 1264.57 | 7.43 | 446.16 | 0.59 | Semi-humid |
| Sub-basin 3 | 108,616 | 909.76 | 1267.21 | 13.52 | 479.53 | 0.53 | Humid |
| Module | Parameter | Description | Acquisition Method |
|---|---|---|---|
| Two-source potential evapotranspiration | rmin | minimum stamatal resistance(sm−1) | based on land data assimilation system (LDAS) |
| ac | albedo | based on land data assimilation system (LDAS) | |
| h | height of vegetation(m) | based on land data assimilation system (LDAS) | |
| Wmax | maximum leaf width | based on land data assimilation system (LDAS) | |
| d0 | monthly zero-plane displacement | based on land data assimilation system (LDAS) | |
| z0 | monthly roughness length | based on land data assimilation system (LDAS) | |
| LAI | Leaf area index | from the BNU MODIS LAI dataset | |
| Actual evaporation | Wum | tension water capacity (TWC) of upper layer (mm) | Based on WM |
| Wlm | TWC of lower layer (mm) | Based on WM | |
| C | evapotranspiration coefficient of deeper layer | determined by optimization algorithm | |
| Runoff generation | Wm | TWC (mm) | using θfc, θwp, and aeration zone thickness |
| θs | saturated moisture content | from literature | |
| θfc | field capacity | from literature | |
| θwp | wilting point | from literature | |
| Sm | free water capacity (FWC; mm) | using θs, θfc, and humus layer thickness | |
| Ki | outflow coefficient of FWS to interflow | on the basis of soil properties | |
| Kg | outflow coefficient of FWS to groundwater | on the basis of soil properties | |
| Ci | recession coefficient of interflow water | determined by optimization algorithm | |
| Cg | recession coefficient of groundwater water | outflow of dry season at time t+1, t | |
| Nonlinear Muskingum–Cunge routing method | n | Manning roughness coefficient | from literature |
| q0 | water discharge of reference(m3s−1) | using outflow and inflow of each grid | |
| S0 | river bed slope | derived from the shuttle radar topography mission (SRTM) digital elevation model (DEM) | |
| w | width of a river reach (m) | derived from the drainage area | |
| Dx′ | length of a river reach(m) | using total river length within each grid | |
| Infiltration and saturation excess runoff | b | exponent of TWC capacity distribution curve | determined by optimization algorithm |
| B | exponent of soil infiltration capacity distribution curve | determined by optimization algorithm | |
| a | average basal area of plant stems | determined by optimization algorithm |
| Parameter | Category | Calibration | Validation | ||||
|---|---|---|---|---|---|---|---|
| Sub-Basin 1 | Sub-Basin 2 | Sub-Basin 3 | Sub-Basin 1 | Sub-Basin 2 | Sub-Basin 3 | ||
| Modified GRAJ Model | |||||||
| NSE (%) | mean annual flow | 95.39 | 88.97 | 94.80 | 94.81 | 88.35 | 95.63 |
| rainy season | 91.26 | 83.54 | 92.19 | 91.58 | 85.03 | 93.86 | |
| dry season | 81.68 | 61.06 | 82.82 | 76.94 | 58.49 | 87.70 | |
| RE | mean annual flow | −2.60 | −6.51 | 1.41 | 4.26 | −6.88 | 1.20 |
| rainy season | −3.65 | −5.97 | 1.11 | 3.46 | −4.53 | 2.97 | |
| dry season | 4.95 | −11.10 | 2.43 | 8.27 | −12.77 | −3.97 | |
| PEP | rainy season | −3.80 | −9.80 | −4.40 | −4.80 | −8.60 | −3.40 |
| PEV | rainy season | −6.50 | −11.50 | 2.60 | 6.13 | −8.65 | 5.35 |
| ISER + GRAJ Model | |||||||
| NSE | mean annual flow | 94.22 | 86.68 | 93.34 | 93.59 | 85.87 | 94.66 |
| rainy season | 89.29 | 81.87 | 90.05 | 90.02 | 83.10 | 93.00 | |
| dry season | 80.34 | 62.18 | 80.95 | 74.34 | 56.67 | 85.37 | |
| RE | mean annual flow | −3.70 | −6.88 | −2.10 | 4.65 | −6.30 | -2.50 |
| rainy season | −4.50 | −6.60 | −1.82 | 4.12 | −5.70 | −2.90 | |
| dry season | 6.20 | −12.00 | −4.20 | 8.67 | −11.90 | 3.50 | |
| PEP | rainy season | −4.60 | −10.10 | −5.10 | −5.30 | 9.50 | −4.10 |
| PEV | rainy season | −7.10 | −13.20 | −4.30 | 7.32 | −12.46 | −5.12 |
| TSPE + GRAJ Model | |||||||
| NSE | mean annual flow | 92.33 | 81.90 | 91.16 | 92.59 | 76.98 | 90.66 |
| rainy season | 87.17 | 80.44 | 89.15 | 87.66 | 80.30 | 92.32 | |
| dry season | 75.60 | 60.66 | 76.90 | 69.38 | 56.99 | 82.46 | |
| RE | mean annual flow | −8.03 | −7.83 | −2.01 | −5.12 | −8.94 | −3.31 |
| rainy season | −7.90 | −7.37 | −2.53 | −4.17 | −7.24 | −2.90 | |
| dry season | −8.23 | −12.00 | 5.62 | −9.46 | −15.30 | −7.42 | |
| PEP | rainy season | −9.70 | −12.40 | −7.70 | −9.20 | −11.70 | −6.20 |
| PEV | rainy season | −14.50 | −16.30 | −7.05 | −8.47 | −14.87 | −5.31 |
| Uncertainty Parameter | Catchment | ||
|---|---|---|---|
| Sub-Basin 1 | Sub-Basin 2 | Sub-Basin 3 | |
| average relative length (ARIL) | 0.265 | 0.513 | 0.267 |
| average asymmetry degree (AAD) | 0.300 | 0.324 | 0.298 |
| average deviation amplitude (ADA) | 285.878 | 406.498 | 108.610 |
| Watershed | Year | Precipitation | Storage Change | Modeled Annual Actual Evaporation | Measured Discharge |
|---|---|---|---|---|---|
| Sub-basin 1 | mean annual (mm) | 461.50 | −1.08 | 258.63 | 201.90 |
| σ/Mean | 0.23 | - | 0.09 | 0.38 | |
| correlation with Precipitation | 1.00 | 0.85 | 0.41 | 0.81 | |
| Sub-basin 2 | mean annual (mm) | 753.16 | −1.40 | 338.12 | 446.16 |
| σ/Mean | 0.20 | - | 0.07 | 0.40 | |
| correlation with Precipitation | 1.00 | 0.66 | 0.64 | 0.95 | |
| Sub-basin 3 | mean annual (mm) | 909.76 | 1.62 | 422.21 | 479.53 |
| σ/Mean | 0.27 | - | 0.07 | 0.46 | |
| correlation with Precipitation | 1.00 | 0.62 | 0.59 | 0.94 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meng, C.; Zhou, J.; Zhong, D.; Wang, C.; Guo, J. An Improved Grid-Xinanjiang Model and Its Application in the Jinshajiang Basin, China. Water 2018, 10, 1265. https://doi.org/10.3390/w10091265
Meng C, Zhou J, Zhong D, Wang C, Guo J. An Improved Grid-Xinanjiang Model and Its Application in the Jinshajiang Basin, China. Water. 2018; 10(9):1265. https://doi.org/10.3390/w10091265
Chicago/Turabian StyleMeng, Changqing, Jianzhong Zhou, Deyu Zhong, Chao Wang, and Jun Guo. 2018. "An Improved Grid-Xinanjiang Model and Its Application in the Jinshajiang Basin, China" Water 10, no. 9: 1265. https://doi.org/10.3390/w10091265
APA StyleMeng, C., Zhou, J., Zhong, D., Wang, C., & Guo, J. (2018). An Improved Grid-Xinanjiang Model and Its Application in the Jinshajiang Basin, China. Water, 10(9), 1265. https://doi.org/10.3390/w10091265




