Estimating Erodibility Parameters for Streambanks with Cohesive Soils Using the Mini Jet Test Device: A Comparison of Field and Computational Methods
Abstract
:1. Introduction
2. Background for Jet Test Data Analysis
3. Materials and Methods
3.1. Study Design
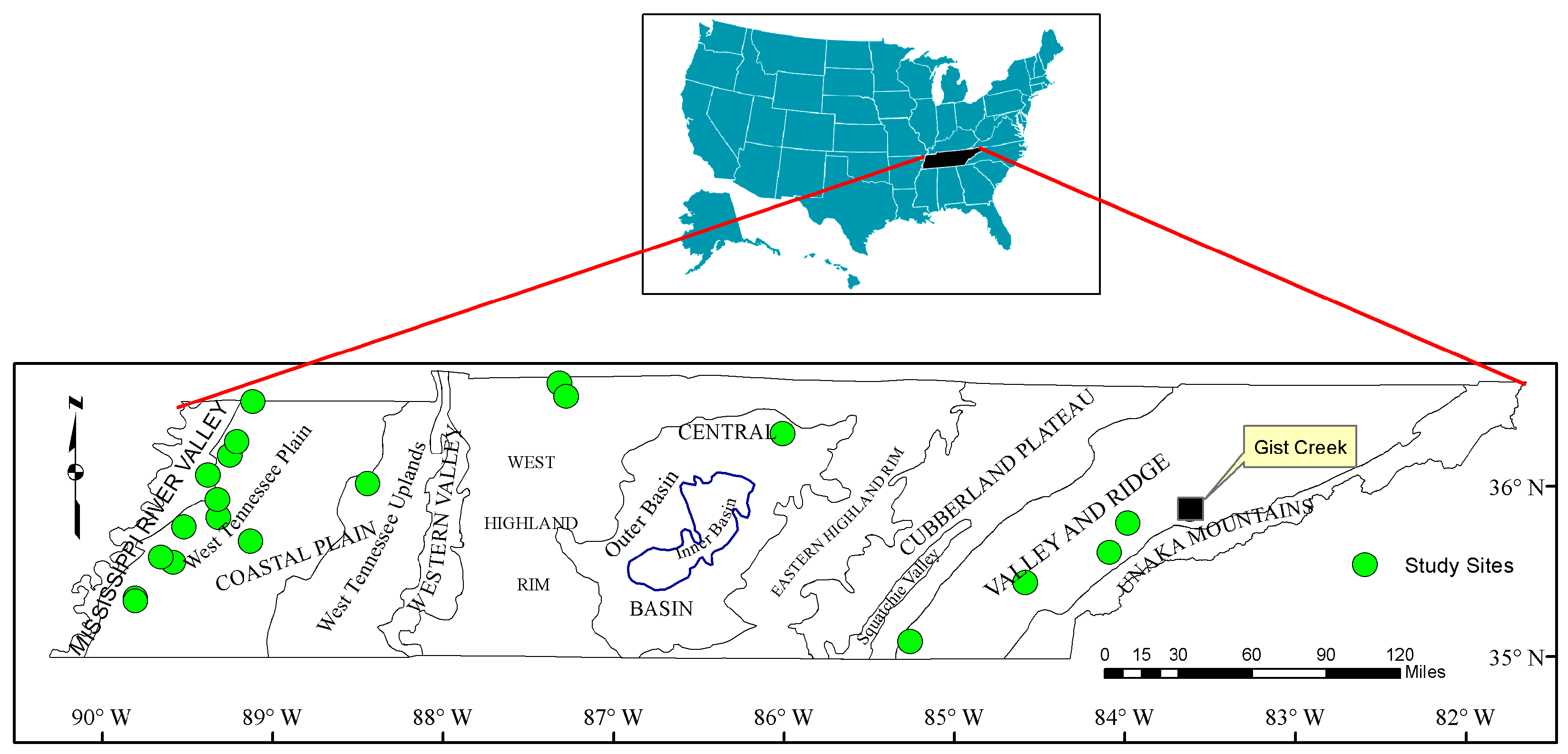
3.2. Study Area
3.3. Field Data Collection and Computational Procedures
4. Results and Discussion
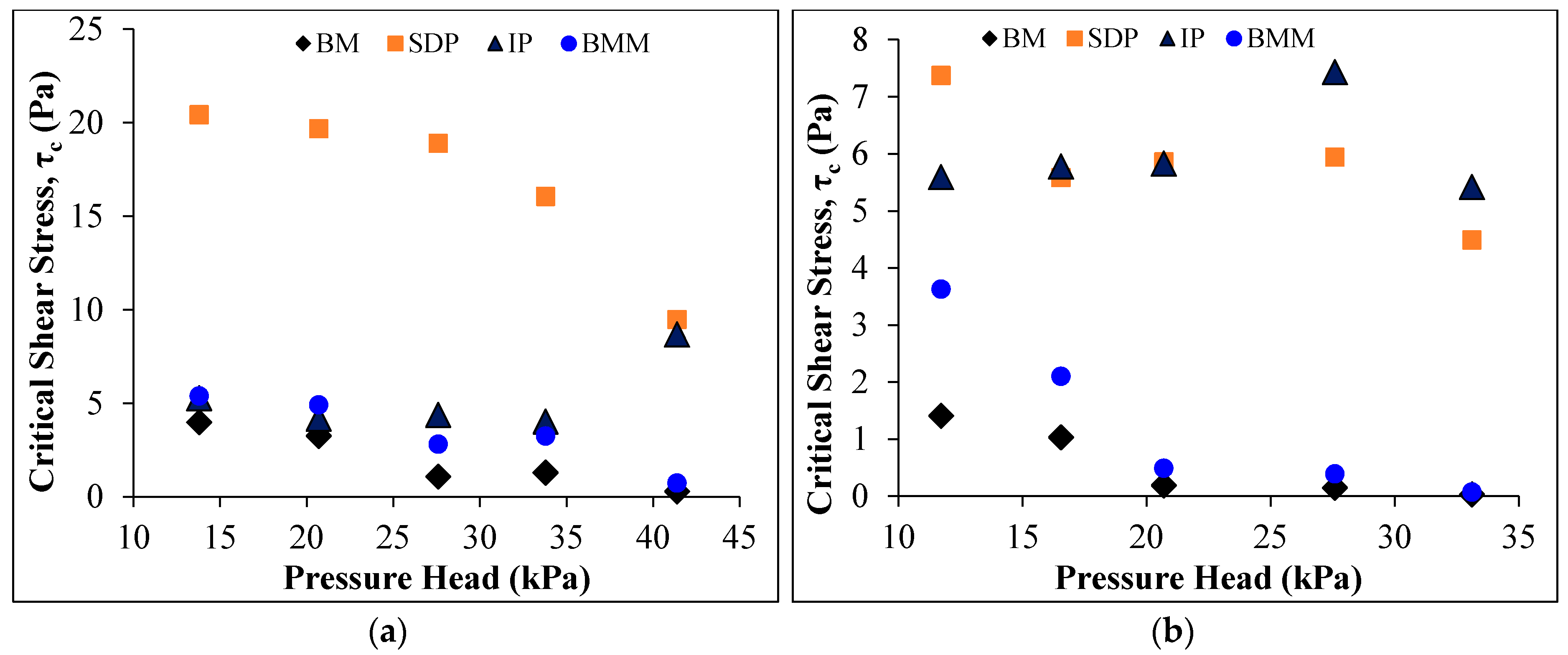
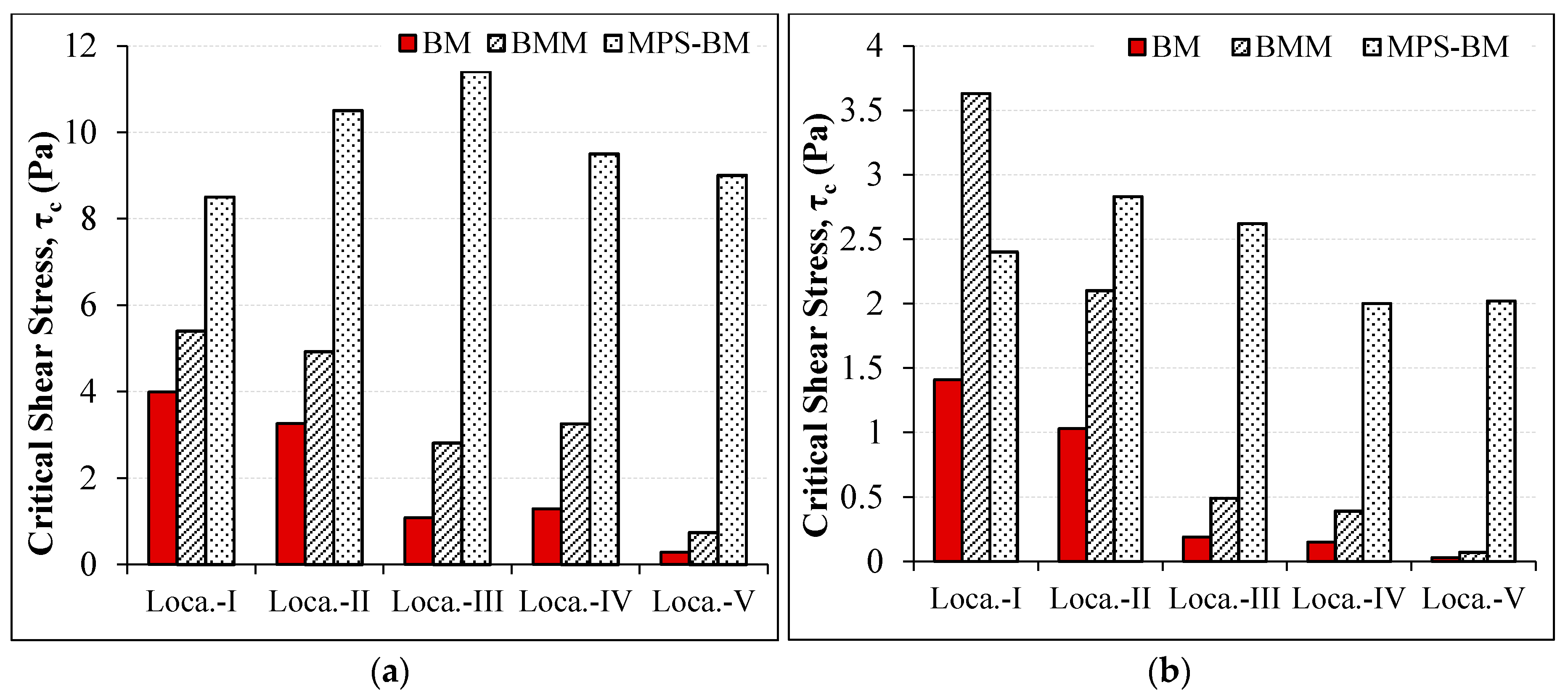
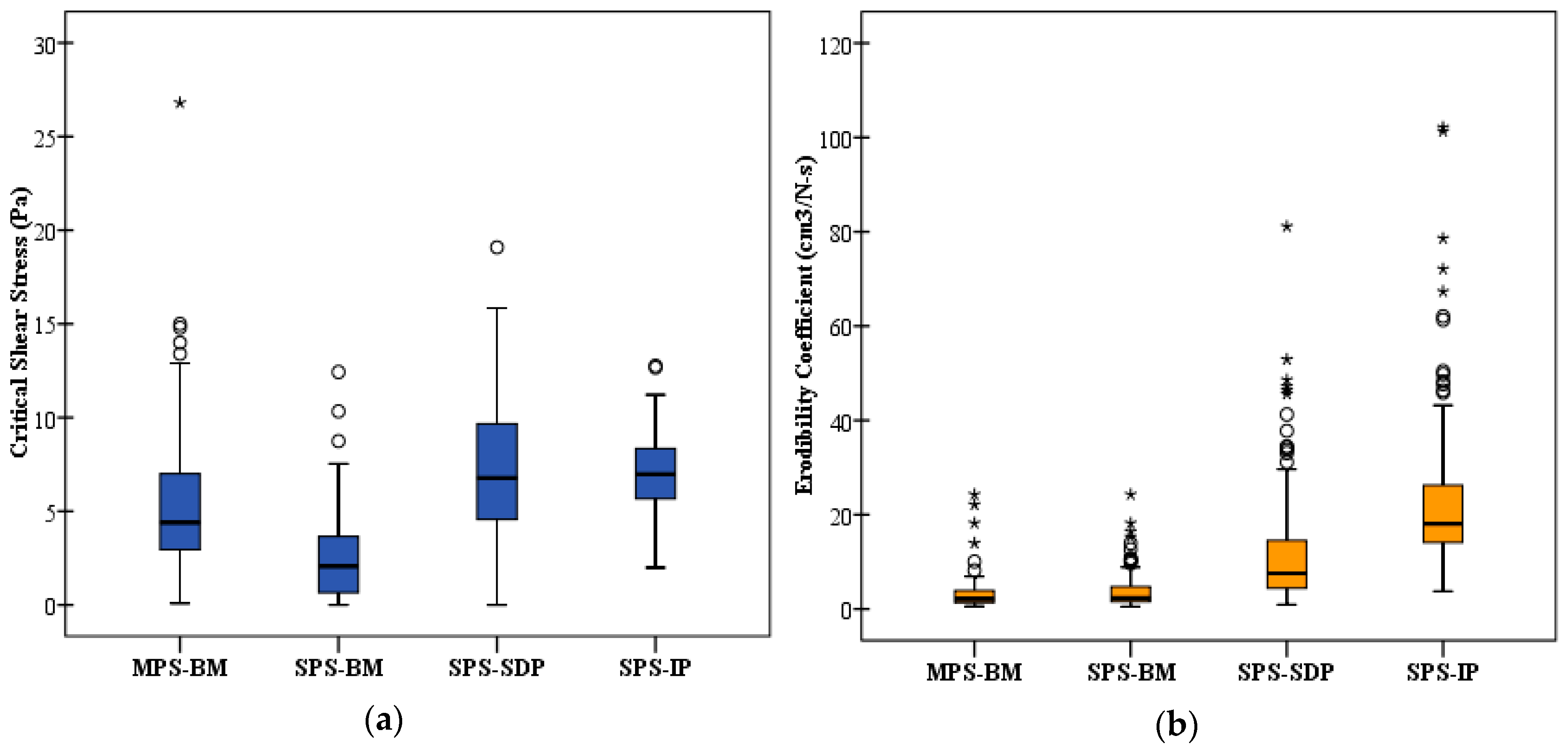
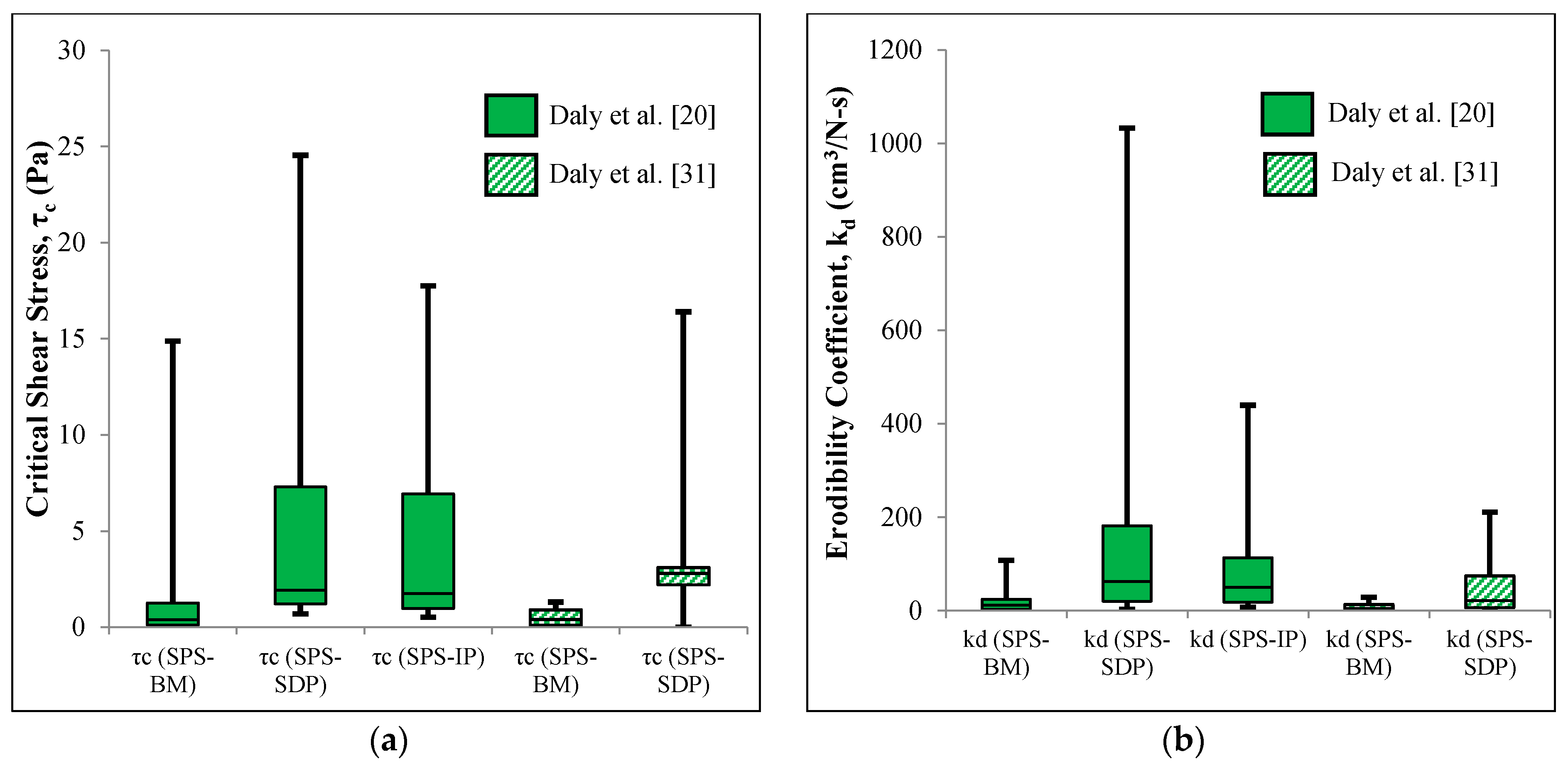
4.1. Comparison of SPS and MPS Methods for Estimating Critical Shear Stress
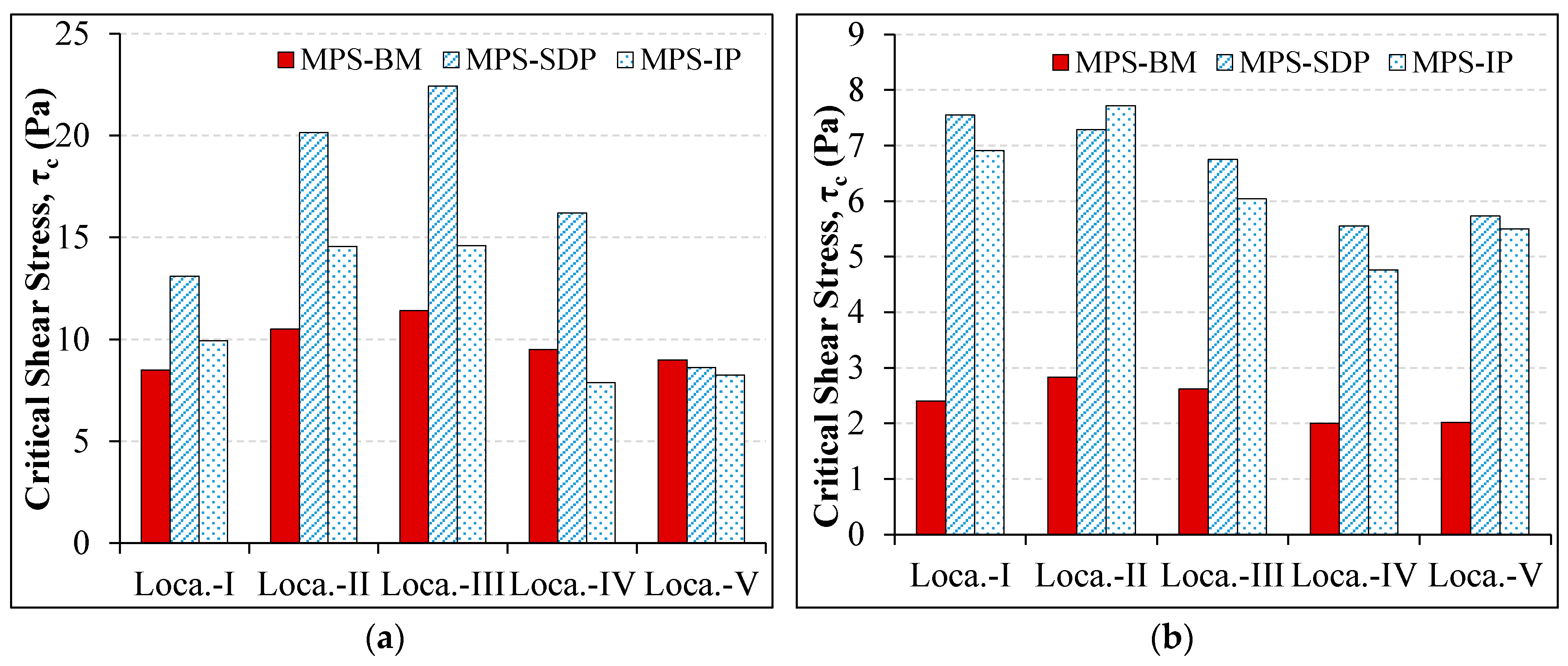
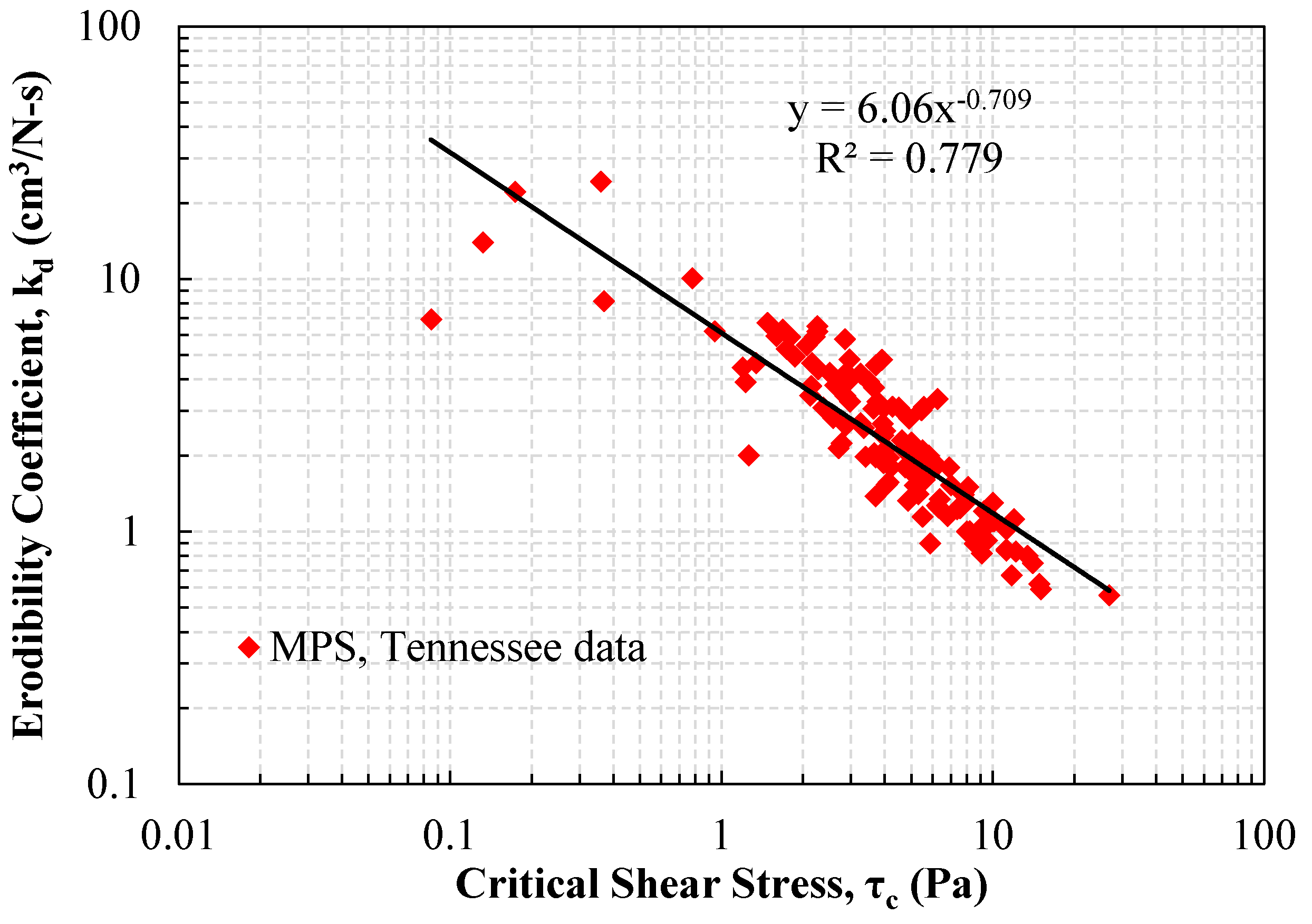
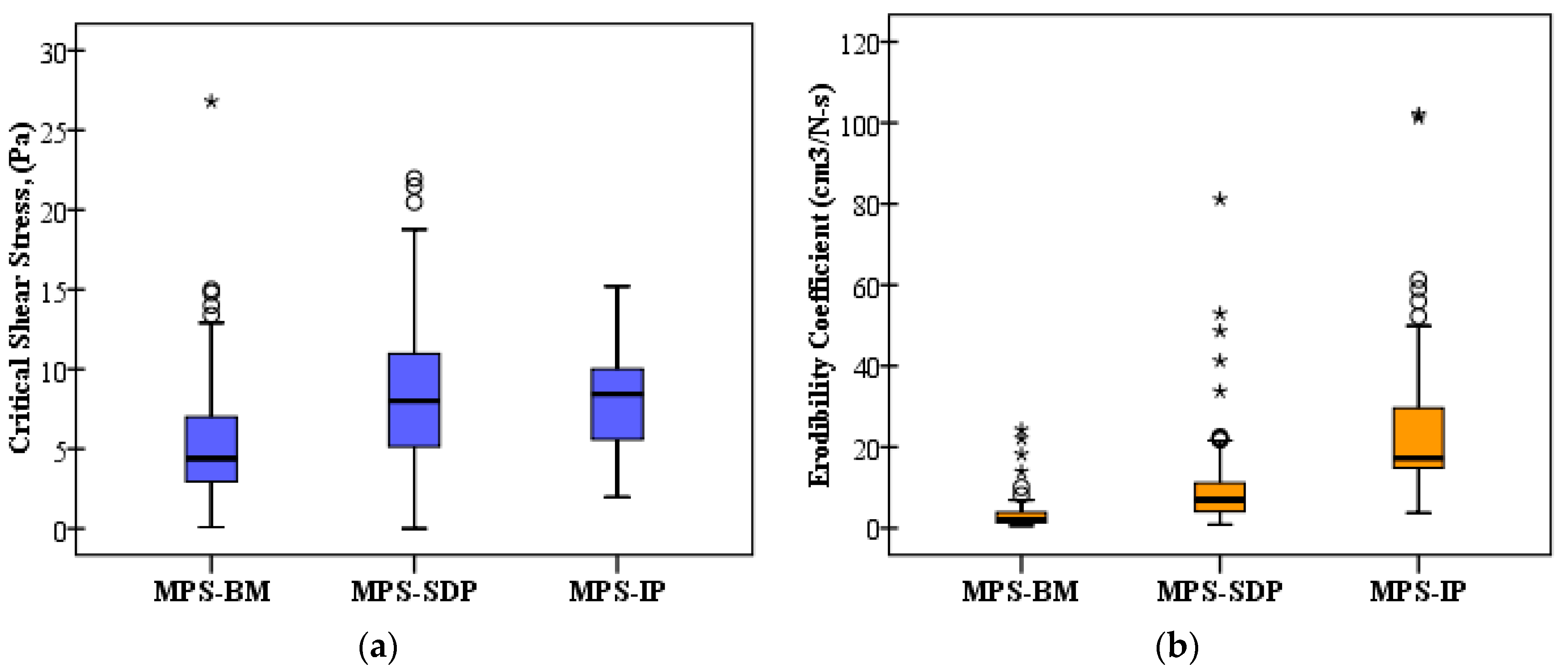
4.2. Comparison of MPS Method and Computational Procedures for Erodibility Parameters
4.3. Qualitative Observations in Scour-Hole Development and Morphology
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Dunn, I.S. Tractive Resistance of Cohesive Channels. J. Soil Mech. Found. 1959, 85, 1–24. [Google Scholar]
- Arulanandan, K.; Gillogley, E.; Tully, R. Development of a Quantitative Method to Predict Critical Shear Stress and Rate of Erosion of Natural Undisturbed Cohesive Soils; Waterways Experiment Station Technical Report, GL-80-5; USACE: Vicksburg, MS, USA, 1980. [Google Scholar]
- Hanson, G.J. Surface Erodibility of Earthen Channels at High Stresses. Part I-Open Channel Testing. Trans. ASAE 1990, 33, 127–131. [Google Scholar] [CrossRef]
- Hanson, G.J.; Simon, A. Erodibility of Cohesive Streambeds in the Loess Area of the Midwestern USA. Hydrol. Process 2001, 15, 23–28. [Google Scholar] [CrossRef]
- Sanford, L.P.; Maa, J.P.Y. A Unified Erosion Formulation for Fine Sediments. Mar. Geol. 2001, 179, 9–23. [Google Scholar] [CrossRef]
- Hanson, G.J.; Cook, K.R. Development of Excess Shear Stress Parameters for Circular Jet Testing. In ASAE Paper No. 97-2227; ASABE: St. Joseph, MI, USA, 1997. [Google Scholar]
- Hanson, G.J.; Cook, K.R. Apparatus, Test Procedures, and Analytical Methods to Measure Soil Erodibility in-situ. Appl. Eng. Agric. 2004, 20, 455–462. [Google Scholar] [CrossRef]
- Simon, A.; Thomas, R.E.; Klimetz, L. Comparison and Experiences with Field Techniques to Measure Critical Shear Stress and Erodibility of Cohesive Deposits. In Proceedings of the 2nd Joint Federal Interagency Conference on Sedimentation and Hydrologic Modeling, Las Vegas, NV, USA, 27 June–1 July 2010. [Google Scholar]
- Al-Madhhachi, A.T.; Hanson, G.J.; Fox, G.A.; Tyagi, A.K.; Bulut, R. Measuring Soil Erodibility Using a Laboratory “mini” JET. Trans. ASABE 2013, 56, 901–910. [Google Scholar]
- Khanal, A.; Fox, G.A.; Al-Madhhachi, A.T. Variability of Erodibility Parameters from Laboratory mini Jet Erosion Tests. J. Hydrol. Eng. 2016, 21. [Google Scholar] [CrossRef]
- Briaud, J.; Ting, F.; Chen, H.C.; Gudavilli, R.; Kwak, K.; Philogene, B.; Han, S.; Perugu, S.; Wei, G.; Nurtjahyo, P.; et al. SRICOS: Prediction of Scour Rate at Bridge Piers; Report 2937-1; Texas Transportation Institute, The Texas A&M University: College Station, TX, USA, 2001. [Google Scholar]
- Julian, J.; Torres, R. Hydraulic Erosion of Cohesive Riverbanks. Geomorphology 2006, 76, 193–206. [Google Scholar] [CrossRef]
- Wynn, T.M.; Mostaghimi, S. The Effects of Vegetation and Soil Type on Streambank Erosion, Southwestern Virginia, USA. J. Am. Water Resour. Assoc. AWARA 2006, 42, 69–82. [Google Scholar] [CrossRef]
- Thoman, R.W.; Nieggoda, S.L. Determining Erodibility, Critical Shear Stress, and Allowable Discharge Estimates for Cohesive Channels; Case Study in the Powder River Basin of Wyoming. J. Hydraul. Eng. 2008, 134, 1677–1687. [Google Scholar] [CrossRef]
- Grabowski, R.C.; Droppo, I.G.; Wharton, G. Erodibility of Cohesive Sediment: The Importance of Sediment Properties. Earth Sci. Rev. 2011, 105, 101–120. [Google Scholar] [CrossRef]
- Mahalder, B.; Schwartz, J.S.; Palomino, A.M.; Zirkle, J. Relationships between physical-geochemical soil properties and erodibility of streambanks among different physiographic provinces of Tennessee, USA. Earth Surf. Process. Landf. 2017. [Google Scholar] [CrossRef]
- Lick, W.; McNeil, J.; Xu, Y.J.; Taylor, C. Measurements of the Resuspension and Erosion of Sediments in Rivers; Department of Mechanical and Environmental Engineering, University of California: Santa Barbara, CA, USA, 1994. [Google Scholar]
- Zreik, D.A.; Krishnappan, B.G.; Germaine, J.T.; Madsen, O.S.; Ladd, C.C. Erosional and Mechanical Strengths of Deposited Cohesive Sediments. J. Hydraul. Eng. 1998, 124, 1076–1085. [Google Scholar] [CrossRef]
- Midgley, T.L.; Fox, G.A.; Wilson, G.V.; Heeren, D.M.; Langendoen, E.; Simon, A. Streambank Erosion and Instability Induced by Seepage: In-situ Constant-head Experiments. J. Hydrol. Eng. 2013, 18, 1200–1210. [Google Scholar] [CrossRef]
- Daly, E.R.; Fox, G.A.; Enlow, H.K.; Storm, D.E.; Hunt, S.L. Site-scale Variability of Streambank Fluvial Erodibility Parameters as Measured with a Jet Erosion Test. J. Hydrol. Process. 2015, 29, 5451–5464. [Google Scholar] [CrossRef]
- Sutarto, T.; Papanicolaou, T.A.N.; Wilson, C.G.; Langendoen, E.J. Stability Analysis of Semicohesive Streambanks with CONCEPTS: Coupling Field and Laboratory Investigations to Quantify the Onset of Fluvial Erosion and Mass Failure. J. Hydraul. Eng. 2014, 140. [Google Scholar] [CrossRef]
- Daly, E.R.; Miller, R.B.; Fox, G.A. Modelling Streambank Erosion and Failure along Protected and Unprotected Composite Streambanks. Adv. Water Resour. 2015, 81, 114–127. [Google Scholar] [CrossRef]
- Daly, E.R.; Fox, G.A.; Fox, A.K. Correlating Site-scale Erodibility Parameters from Jet Erosion Tests to Soil Physical Properties. Trans. ASABE 2016, 59, 115–128. [Google Scholar] [CrossRef]
- McNeil, J.; Taylor, C.; Lick, W. Measurements of Erosion of Undisturbed Bottom Sediments with Depth. J. Hydraul. Eng. 1996, 122, 316–324. [Google Scholar] [CrossRef]
- Taylor, C.; Lick, W. Erosion Properties of Great Lakes Sediments; Department of Mechanical and Environmental Engineering, University of California: Santa Barbara, CA, USA, 1996. [Google Scholar]
- Jepsen, R.; Roberts, J.; Lick, W. Effects of Bulk Density on Sediment Erosion Rates. Water Air Soil Pollut. 1997, 99, 21–31. [Google Scholar] [CrossRef]
- Roberts, J.; Jepsen, R.; Gotthard, D.; Lick, W. Effects of Particle Size and Bulk Density on Erosion of Quartz Particles. J. Hydraul. Eng. 1998, 124, 1261–1267. [Google Scholar] [CrossRef]
- Perkins, R.G.; Honeywill, C.; Consalvey, M.; Austin, H.A.; Tolhurst, T.J.; Paterson, D.M. Changes in Microphytobenthic Chlorophyll a and EPS Resulting from Sediment Compaction Due to de-watering: Opposing Patterns in Concentration and Content. Cont. Shelf Res. 2003, 23, 575–586. [Google Scholar] [CrossRef]
- Perkins, R.G.; Sun, H.; Watson, J.; Player, M.A.; Paterson, D.M. In-line Laser Holography and Video Analysis of Eroded Flocs from Engineered and Estuarine Sediments. Environ. Sci. Technol. 2004, 38, 4640–4648. [Google Scholar] [CrossRef] [PubMed]
- Lick, W.; McNeil, J. Effects of Sediment Bulk Properties on Erosion Rates. Sci. Total Environ. 2001, 266, 41–48. [Google Scholar] [CrossRef]
- Daly, E.R.; Fox, G.A.; Al-Madhhachi, A.T.; Miller, R.B. A Scour Depth Approach for Deriving Erodibility Parameters from Jet Erosion Tests. Trans. ASABE 2013, 56, 1343–1351. [Google Scholar] [CrossRef]
- Karamigolbaghi, M.; Ghaneeizad, S.M.; Atkinson, J.F.; Bennett, S.J.; Wells, R.R. Critical Assessment of Jet Erosion Test Methodologies for Cohesive soil and Sediment. Geomorphology 2017, 295, 529–536. [Google Scholar] [CrossRef]
- Mahalder, B.; Schwartz, J.S.; Papanicolaou, A.N.T.; Palomino, A.M.; Zirkle, J. Comparison of erodibility parameters for cohesive streambank soils between an in-situ jet test device and laboratory conduit flume. J. Hydraul. Eng. 2018. (submitted). [Google Scholar]
- Craft, T.; Graham, L.; Launder, B. Impinging Jet Studies for Turbulence Model Assessment—II. An Examination of the Performance of Four Turbulence Models. Int. J. Heat Mass Transf. 1993, 36, 2685–2697. [Google Scholar] [CrossRef]
- Gibson, M.M.; Launder, B.E. Ground Effects on Pressure Fluctuations in the Atmospheric Boundary Layer. J. Fluid Mech. 1978, 86, 491–511. [Google Scholar] [CrossRef]
- Mercier, F.; Bonelli, S.; Pinettes, P.; Golay, F.; Anselmet, F.; Philippe, P. Comparison of CFD simulations with experimental Jet Erosion Tests results. J. Hydraul. Eng. 2014, 140. [Google Scholar] [CrossRef]
- Clark, L.A.; Wynn, T.M. Methods for Determining Streambank Critical Shear Stress and Soil Erodibility: Implications for Erosion Rate Predictions. Trans. ASABE 2007, 50, 95–106. [Google Scholar] [CrossRef]
- Bonelli, S.; Brivois, O. The Scaling Law in the Hole Erosion Test with a Constant Pressure Drop. Int. J. Numer. Anal. Methods Geomech. 2008, 32, 1573–1595. [Google Scholar] [CrossRef]
- Bonelli, S.; Golay, F.; Mercier, F. On the Modelling of Interface Erosion; Erosion of Geomaterials; Wiley/ISTE: Hoboken, NJ, USA, 2012; Chapter 6; pp. 187–222. [Google Scholar]
- Golay, F.; Lachouette, D.; Bonelli, S.; Seppecher, P. Numerical Modelling of Interfacial Soil Erosion with Viscous Incompressible Flows. Comput. Methods Appl. Mech. Eng. 2011, 200, 383–391. [Google Scholar] [CrossRef]
- Stein, O.R.; Nett, D.D. Impinging jet calibration of excess shear sediment detachment parameters. Trans. ASAE 1997, 40, 1573–1580. [Google Scholar] [CrossRef]
- Blaisdell, F.W.; Clayton, L.A.; Hebaus, C.G. Ultimate Dimension of Local Scour. J. Hydraul. Div. 1981, 107, 327–337. [Google Scholar]
- Miller, R.A. The Geologic History of Tennessee; Tennessee Division of Geology: Nashville, TN, USA, 1974; Volume 74. [Google Scholar]
- Hanson, G.J.; Robinson, K.M.; Cook, K.R. Scour below an overfall: Part II. Prediction. Trans. ASAE 2002, 45, 957–964. [Google Scholar] [CrossRef]
- Raudkivi, A.J. Loose Boundary Hydraulics, 3rd ed.; Pergamon Press: New York, NY, USA, 1990. [Google Scholar]
- Mitchell, J.K.; Soga, K. Fundamentals of Soil Behavior, 3rd ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2005. [Google Scholar]

| Procedures | Description/Working Principles |
|---|---|
| Field Data Collection Procedures | |
| Single Pressure Setting (SPS) | A single pressure setting is chosen in which scour depth readings are collected at pre-defined time intervals. Finally, equilibrium scour depth is assumed based on different computational methods (BM, SDP, and IP) and used in τc and kd calculation. |
| Multiple Pressure Settings (MPS) | Incrementally five different pressure heads are used at a test location starting from lower to higher. At each pressure head, test duration is about 12–20 min, where three different intervals are used for recording the scour depth readings. |
| Computational Procedures | |
| Blaisdell Method (BM) | The Blaisdell et al. [42] approach is used for equilibrium scour depth calculation and subsequently τc and kd are calculated [44]. |
| Modified Blaisdell Method (BMM) | The Blaisdell et al. [42] approach is used for equilibrium scour depth calculation and subsequently τc and kd are calculated [44], however a head loss coefficient of 0.39 was applied as per Karamigolbaghi et al. [32] where 0.16 is typically used. The adjusted coefficient addresses the jet confinement in the mini-jet’s submergence (tank) test chamber. |
| Scour Depth Principle (SDP) | Solved for τc and kd iteratively by plotting the original scour depth versus predicted scour depth using excess shear stress equation. In this simultaneous solution method, by minimizing the sum of squared errors between the measured scour data and the predicted scour depth data τc and kd values are estimated. Single pressure head is used for jet operation [31]. |
| Iterative Principle (IP) | In the IP approach, τc and kd values are estimated from Blaisdell solution approach based on T* and J* values. Initial τc and kd values are estimated from Blaisdell method and simultaneously solved for erosion parameters iteratively by minimizing root-mean-square error between the measured and predicted time. In this method an upper limit is employed for the iteration of τc values and similar to the other two methods, the jet device is operated using a single pressure head [8]. |
| Computational Procedures for Erodibility Parameters Estimation | |
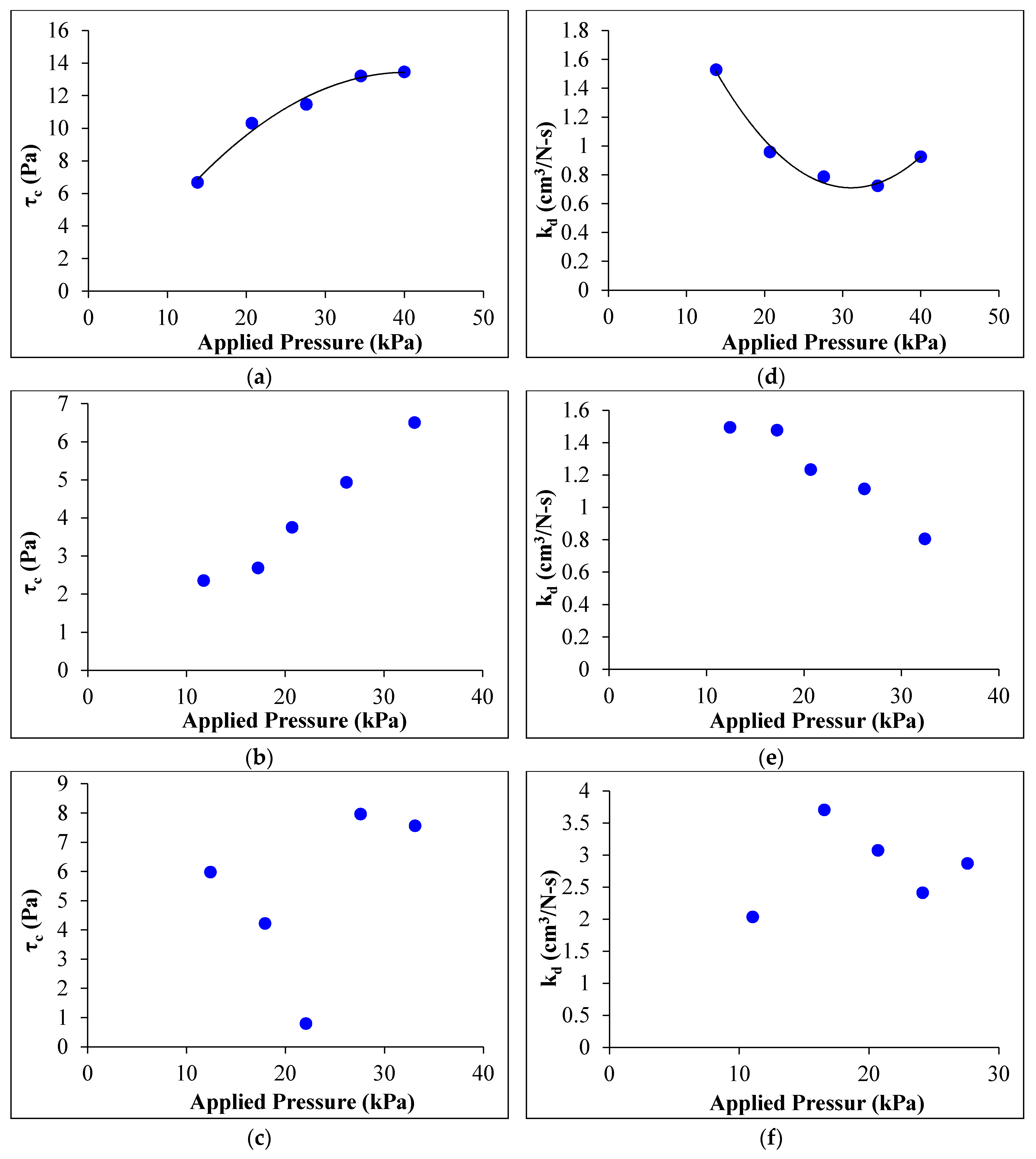
| Multiple Pressure Settings using the BM (MPS-BM) | Solution approach follows the BM method for equilibrium scour depth prediction. However, MPS field data are used for the final τc and kd values estimation, where a spreadsheet is run separately using the scour depth readings for each applied pressure head. The estimated τc and kd values obtained from each pressure head and the corresponding scour depths data are then plotted on a normal graph against the corresponding pressure head. Finally, erodibility parameters at a test location are estimated based on the shape of plots (see Figure 2). |
| Multiple Pressure Settings using the SDP (MPS-SDP) | Solution approach follows the SDP method for equilibrium scour depth prediction using the MPS field procedures and data. A similar approach is followed for the final τc and kd values estimation as the MPS-BM method. |
| Multiple Pressure Settings using the IP (MPS-IP) | Solution approach follows the IP method for equilibrium scour depth prediction using the MPS field procedures and data. A similar approach is followed for the final τc and kd values estimation as the MPS-BM method. |
| Bank Position | Test Identifier | Test Location | Selected Pressure Head (kPa) | Test Duration (min) | Water Content (%) | Bulk Density (gm/cm3) |
|---|---|---|---|---|---|---|
| Upper Bank | SPS-1 | I | 11.72 | 46 | 17.73 | 1.77 |
| MPS-1 | 11.72–33.09 | 80 | 18.58 | 1.77 | ||
| SPS-2 | II | 16.55 | 46 | 21.20 | 1.79 | |
| MPS-2 | 11.72–33.09 | 78 | 20.89 | 1.78 | ||
| SPS-3 | III | 20.68 | 46 | 19.42 | 1.78 | |
| MPS-3 | 11.72–33.09 | 100 | 20.89 | 1.79 | ||
| SPS-4 | IV | 27.58 | 46 | 20.89 | 1.76 | |
| MPS-4 | 11.72–33.09 | 100 | 21.12 | 1.78 | ||
| SPS-5 | V | 33.09 | 46 | 20.80 | 1.79 | |
| MPS-5 | 11.72–33.09 | 100 | 21.85 | 1.78 | ||
| Lower Bank | SPS-1 | I | 13.79 | 46 | 30.83 | 1.92 |
| MPS-1 | 13.79–41.37 | 80 | 31.83 | 1.90 | ||
| SPS-2 | II | 20.68 | 46 | 31.45 | 1.90 | |
| MPS-2 | 13.79–41.37 | 80 | 32.14 | 1.90 | ||
| SPS-3 | III | 27.58 | 46 | 30.14 | 1.90 | |
| MPS-3 | 13.79–41.37 | 90 | 29.54 | 1.90 | ||
| SPS-4 | IV | 33.78 | 46 | 29.51 | 1.92 | |
| MPS-4 | 13.79–41.37 | 100 | 29.96 | 1.91 | ||
| SPS-5 | V | 41.37 | 46 | 31.28 | 1.90 | |
| MPS-5 | 13.79–41.37 | 100 | 31.27 | 1.91 |
| Methods | Critical Shear Stress, τc (Pa) | Erodibility Coefficient, kd (cm3/N·s) | ||||||
|---|---|---|---|---|---|---|---|---|
| Min | Max | Mean | Std. Dev | Min | Max | Mean | Std. Dev | |
| Single Pressure Setting (SPS-BM) | 0.00 | 12.43 | 2.48 | 2.25 | 0.53 | 24.28 | 3.84 | 3.84 |
| Multiple Pressure Settings (MPS-BM) | 0.09 | 26.80 | 5.13 | 3.82 | 0.56 | 24.28 | 3.26 | 3.54 |
| Scour Depth Principle (SPS-SDP) | 0.00 | 19.09 | 6.88 | 3.78 | 0.93 | 81.13 | 12.28 | 13.02 |
| MPS using SDP method (MPS-SDP) | 0.00 | 21.97 | 8.51 | 4.32 | 0.89 | 81.13 | 9.44 | 10.48 |
| Iterative Principle (SPS-IP) | 1.99 | 12.76 | 7.07 | 2.09 | 3.73 | 102.12 | 23.92 | 16.77 |
| MPS using IP method (MPS-IP) | 1.99 | 15.20 | 8.12 | 2.61 | 3.73 | 102.12 | 24.27 | 15.98 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mahalder, B.; Schwartz, J.S.; Palomino, A.M.; Zirkle, J. Estimating Erodibility Parameters for Streambanks with Cohesive Soils Using the Mini Jet Test Device: A Comparison of Field and Computational Methods. Water 2018, 10, 304. https://doi.org/10.3390/w10030304
Mahalder B, Schwartz JS, Palomino AM, Zirkle J. Estimating Erodibility Parameters for Streambanks with Cohesive Soils Using the Mini Jet Test Device: A Comparison of Field and Computational Methods. Water. 2018; 10(3):304. https://doi.org/10.3390/w10030304
Chicago/Turabian StyleMahalder, Badal, John S. Schwartz, Angelica M. Palomino, and Jon Zirkle. 2018. "Estimating Erodibility Parameters for Streambanks with Cohesive Soils Using the Mini Jet Test Device: A Comparison of Field and Computational Methods" Water 10, no. 3: 304. https://doi.org/10.3390/w10030304
APA StyleMahalder, B., Schwartz, J. S., Palomino, A. M., & Zirkle, J. (2018). Estimating Erodibility Parameters for Streambanks with Cohesive Soils Using the Mini Jet Test Device: A Comparison of Field and Computational Methods. Water, 10(3), 304. https://doi.org/10.3390/w10030304




