Global Sensitivity Analysis of a Water Quality Model in the Three Gorges Reservoir
Abstract
:1. Introduction
2. Study Area
3. Materials and Methods
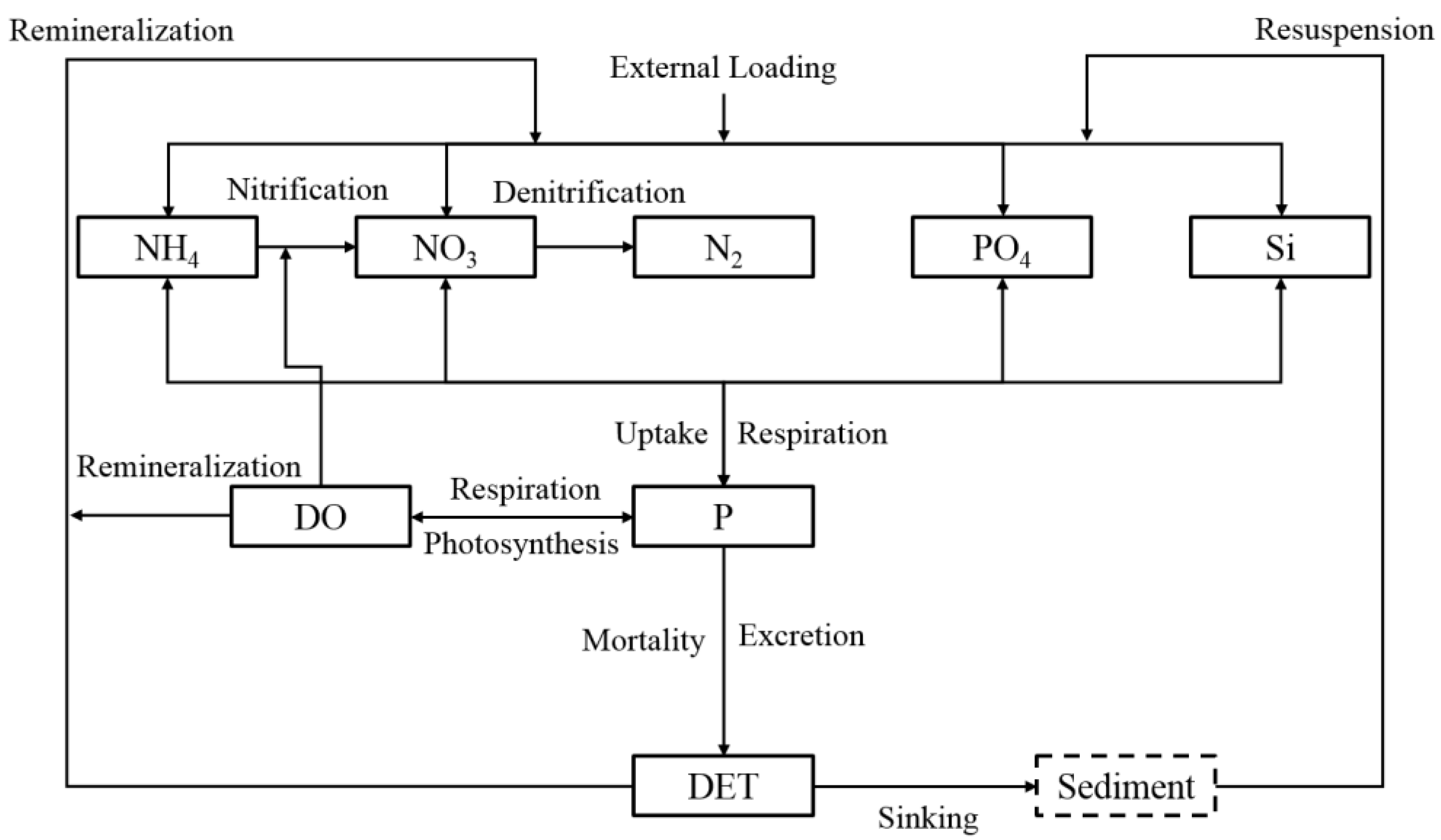
3.1. Tributary Bay Water Quality Model
3.2. Global Sensitivity Analysis
3.3. Design of Numerical Experiments
3.4. Initial Conditions
4. Results
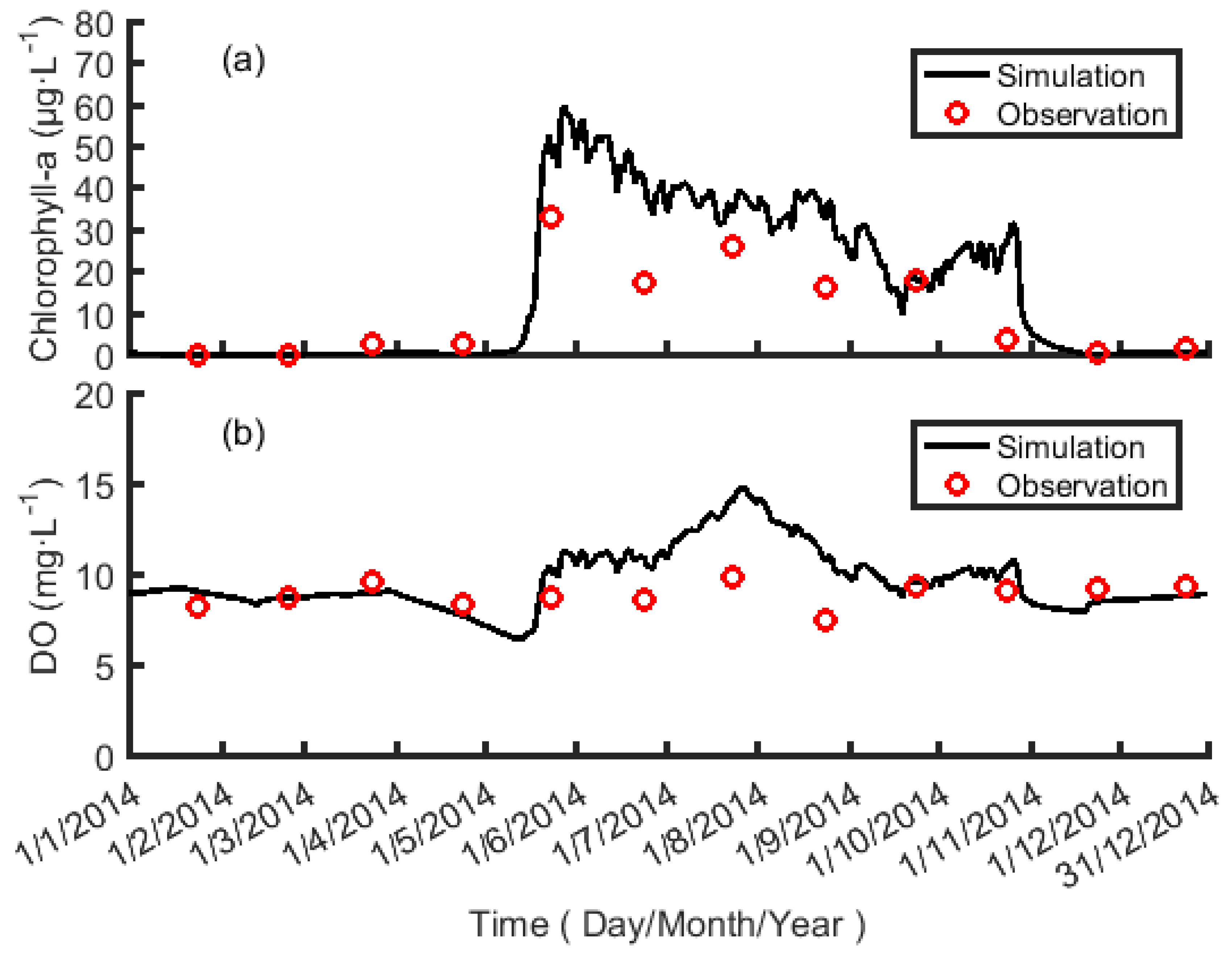
4.1. Simulation Result for the Water Quality Model
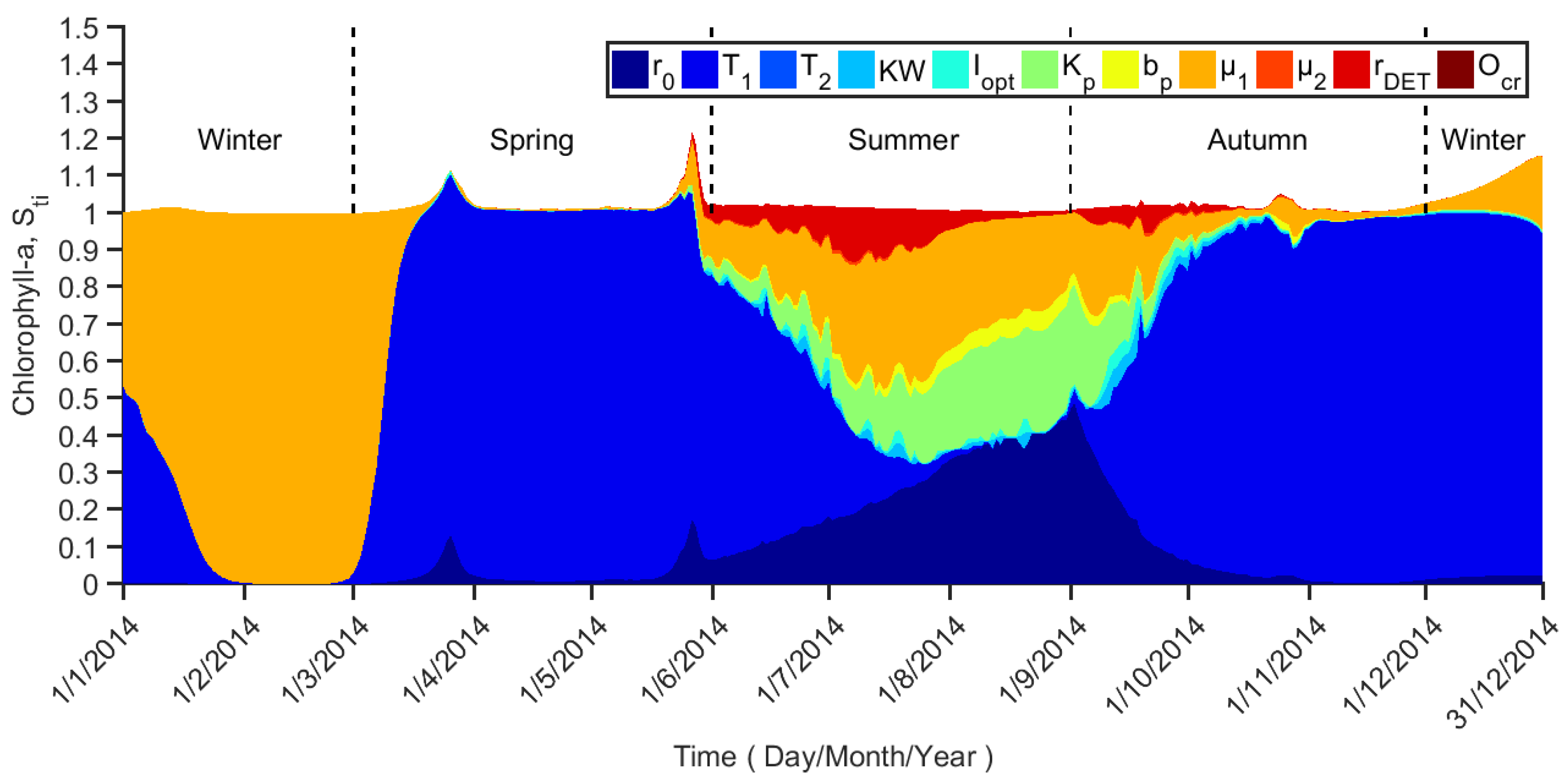
4.2. Parameter Sensitivity Temporal Variation for Chlorophyll-a
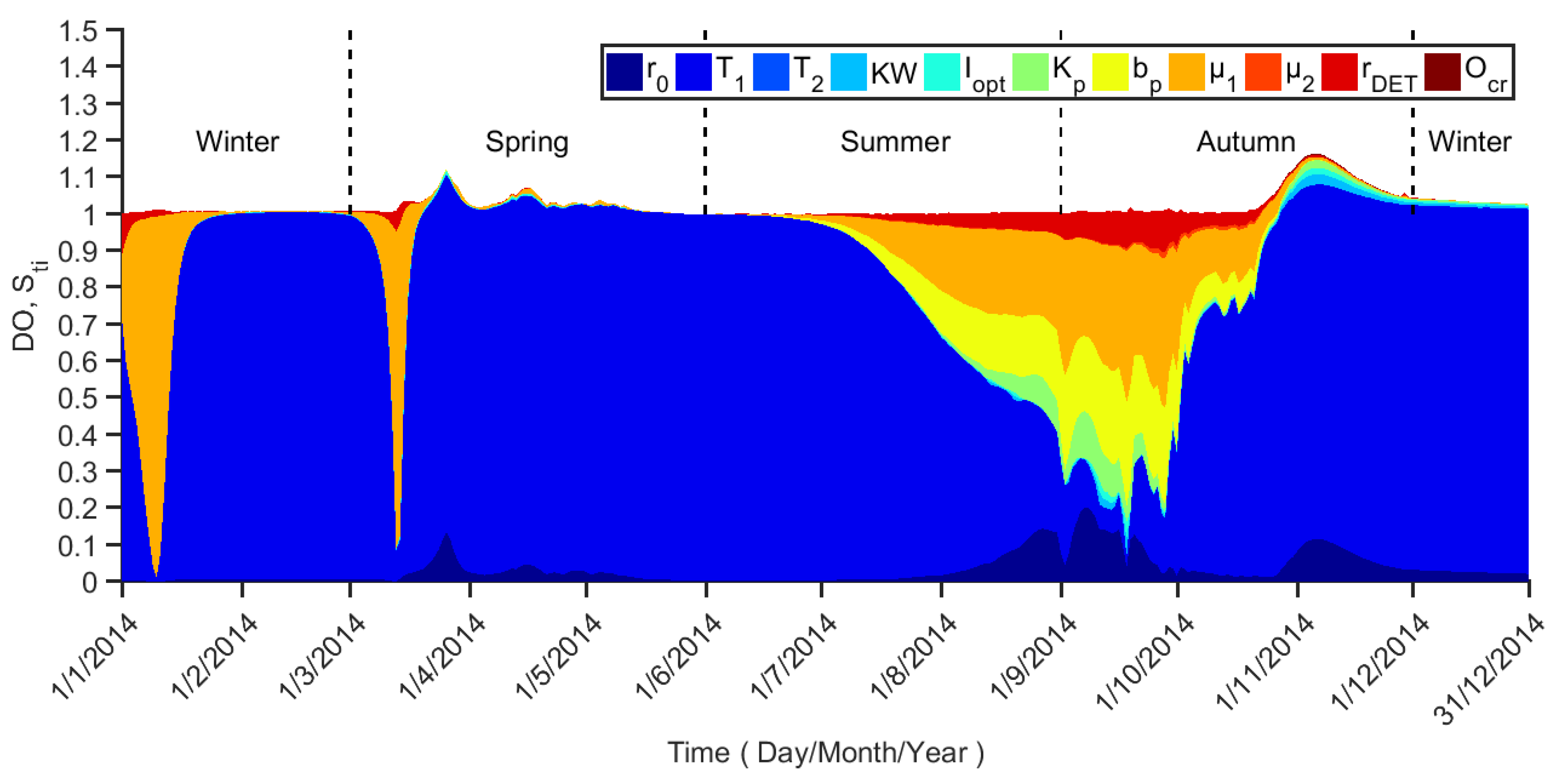
4.3. Parameter Sensitivity Temporal Variation for DO
5. Discussion
5.1. Ecological Implication from Parameter Sensitivity for Chlorophyll-a
5.2. Ecological Implication from Parameter Sensitivity for DO
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A
References
- Chen, C.; Ji, R.; Schwab, D.J.; Beletsky, D.; Fahnenstiel, G.L.; Jiang, M.; Johengen, T.H.; Vanderploeg, H.; Eadie, B.; Budd, J.W. A model study of the coupled biological and physical dynamics in Lake Michigan. Ecol. Model. 2002, 152, 145–168. [Google Scholar] [CrossRef]
- Park, K.; Jung, H.S.; Kim, H.S.; Ahn, S.M. Three-dimensional hydrodynamic-eutrophication model (HEM-3D): Application to Kwang-Yang Bay, Korea. Mar. Environ. Res. 2005, 60, 171–193. [Google Scholar] [CrossRef] [PubMed]
- Jin, K.R.; Ji, Z.G.; James, R.T. Three-dimensional water quality and SAV modeling of a large shallow lake. J. Great Lakes Res. 2007, 33, 28–45. [Google Scholar] [CrossRef]
- Wan, Y.; Ji, Z.G.; Shen, J.; Hu, G.; Sun, D. Three dimensional water quality modeling of a shallow subtropical estuary. Mar. Environ. Res. 2012, 82, 76–86. [Google Scholar] [CrossRef] [PubMed]
- Leon, L.F.; Smith, R.E.H.; Hipsey, M.R.; Bocaniov, S.A.; Higgins, S.N.; Hecky, R.E.; Antenucci, J.P.; Imberger, J.A.; Guildford, S.J. Application of a 3D hydrodynamic-biological model for seasonal and spatial dynamics of water quality and phytoplankton in lake Erie. J. Great Lakes Res. 2011, 37, 41–53. [Google Scholar] [CrossRef]
- Warren, I.R.; Bach, H.K. Mike 21: A modelling system for estuaries, coastal waters and seas. Environ. Softw. 1992, 7, 229–240. [Google Scholar] [CrossRef]
- Los, F.J.; Villars, M.T.; Van der Tol, M.W.M. A 3-dimensional primary production model (BLOOM/GEM) and its applications to the (southern) North Sea (coupled physical–chemical–ecological model). J. Mar. Syst. 2008, 74, 259–294. [Google Scholar] [CrossRef]
- Saltelli, A.; Tarantola, S.; Campolongo, F.; Ratto, M. Sensitivity Analysis in Practice: A Guide to Assessing Scientific Models; John Wiley & Sons: Hoboken, NJ, USA, 2004. [Google Scholar]
- Griensven, A.V.; Meixner, T.; Grunwald, S.; Bishop, T.; Diluzio, M.; Srinivasan, R. A global sensitivity analysis tool for the parameters of multi-variable catchment models. J. Hydrol. 2006, 324, 10–23. [Google Scholar] [CrossRef]
- Hill, M.C.; Østerby, O. Determining extreme parameter correlation in ground water models. Groundwater 2003, 41, 420–430. [Google Scholar] [CrossRef]
- Tian, W. A review of sensitivity analysis methods in building energy analysis. Renew. Sustain. Energy Rev. 2013, 20, 411–419. [Google Scholar] [CrossRef]
- Oakley, J.E.; O’Hagan, A. Probabilistic sensitivity analysis of complex models: A Bayesian approach. J. R. Stat. Soc. 2004, 66, 751–769. [Google Scholar] [CrossRef]
- Saltelli, A.; Chan, K.; Scott, E.M. Sensitivity Analysis; Wiley: New York, NY, USA, 2000. [Google Scholar]
- Saltelli, A.; Ratto, M.; Tarantola, S.; Campolongo, F. Sensitivity analysis practices: Strategies for model-based inference. Reliab. Eng. Syst. Saf. 2006, 91, 1109–1125. [Google Scholar] [CrossRef]
- Soboĺ, I.M. Quasi-monte carlo methods. Prog. Nucl. Energy 1990, 24, 55–61. [Google Scholar] [CrossRef]
- Saltelli, A.; Ratto, M.; Andres, T.; Campolongo, F.; Cariboni, J.; Gatelli, D.; Saisana, M.; Tarantola, S. Global Sensitivity Analysis: The Primer; Wiley: New York, NY, USA, 2008. [Google Scholar]
- Morris, M.D. Factorial sampling plans for preliminary computational experiments. Technometrics 1991, 33, 161–174. [Google Scholar] [CrossRef]
- Makler-Pick, V.; Gal, G.; Gorfine, M.; Hipsey, M.R.; Carmel, Y. Sensitivity analysis for complex ecological models—A new approach. Environ. Model. Softw. 2011, 26, 124–134. [Google Scholar] [CrossRef]
- Ciric, C.; Ciffroy, P.; Charles, S. Use of sensitivity analysis to identify influential and non-influential parameters within an aquatic ecosystem model. Ecol. Model. 2012, 246, 119–130. [Google Scholar] [CrossRef]
- Zheng, W.; Shi, H.; Fang, G.; Hu, L.; Peng, S.; Zhu, M. Global sensitivity analysis of a marine ecosystem dynamic model of the Sanggou Bay. Ecol. Model. 2012, 247, 83–94. [Google Scholar] [CrossRef]
- Stone, R. Three gorges dam: Into the unknown. Science 2008, 321, 628–632. [Google Scholar] [CrossRef] [PubMed]
- Ye, L.; Xu, Y.; Han, X.; Cai, Q. Daily dynamics of nutrients and chlorophyll a during a spring phytoplankton bloom in Xiangxi Bay of the three gorges reservoir. J. Freshw. Ecol. 2006, 21, 315–321. [Google Scholar] [CrossRef]
- Zhang, S.; Li, C.M.; Fu, Y.C.; Zhang, Y.; Zheng, J. Trophic states and nutrient output of tributaries bay in three gorges reservoir after impoundment. Environ. Sci. 2008, 29, 7–12. [Google Scholar]
- Liu, L.; Liu, D.; Johnson, D.M.; Yi, Z.; Huang, Y. Effects of vertical mixing on phytoplankton blooms in Xiangxi Bay of three gorges reservoir: Implications for management. Water Res. 2012, 46, 2121–2130. [Google Scholar] [CrossRef] [PubMed]
- Mao, J.; Jiang, D.; Dai, H. Spatial-temporal hydrodynamic and algal bloom modelling analysis of areservoir tributary embayment. J. Hydro-Environ. Res. 2015, 9, 200–215. [Google Scholar] [CrossRef]
- Ma, J.; Liu, D.; Wells, S.A.; Tang, H.; Ji, D.; Yang, Z. Modeling density currents in a typical tributary of the three gorges reservoir, China. Ecol. Model. 2015, 296, 113–125. [Google Scholar] [CrossRef]
- Chen, C.; Li, J.; Shen, H.; Wang, Z. Yangtze River of China: Historical analysis of discharge variability and sediment flux. Geomorphology 2001, 41, 77–91. [Google Scholar] [CrossRef]
- Nilsson, C.; Reidy, C.A.; Dynesius, M.; Revenga, C. Fragmentation and flow regulation of the world’s large river systems. Science 2005, 308, 405–408. [Google Scholar] [CrossRef] [PubMed]
- Yang, S.L.; Zhang, J.; Dai, S.B.; Li, M.; Xu, X.J. Effect of deposition and erosion within the main river channel and large lakes on sediment delivery to the estuary of the Yangtze River. J. Geophys. Res. Earth Surf. 2007, 112, 111–119. [Google Scholar] [CrossRef]
- Gordon, D.C., Jr.; Boudreau, P.R.; Mann, K.H.; Ong, J.E.; Silvert, W.L.; Smith, S.V.; Wattayakorn, G.; Wulff, F.; Yanagi, T. LOICZ Biogeochemical Modelling Guidelines; Netherlands Institute for Sea Research: Den Burg, The Netherlands, 1996. [Google Scholar]
- Bowie, G.L.; Mills, W.B.; Porcella, D.B.; Campbell, C.L.; Pagenkopf, J.R.; Rupp, G.L.; Johnson, K.M.; Chan, P.W.H.; Gherini, S.A. Rates, Constants, and Kinetics Formulations, Surface Water Quality Modeling; Environmental Protection Agency: Washington, DC, USA, 1985.
- Jørgensen, S.E.; Nielsen, S.N.; Jørgensen, L.A. Handbook of Ecological Parameters and Ecotoxicology; Elsevier: Amsterdam, The Netherlands, 1991; Volume 29, p. 791. [Google Scholar]
- Chen, C.; Ji, R.; Zheng, L.; Zhu, M.; Rawson, M. Influences of physical processes on the ecosystem in Jiaozhou Bay: A coupled physical and biological model experiment. J. Geophys. Res. Oceans 1999, 104, 29925–29930. [Google Scholar] [CrossRef]
- Cannavó, F. Sensitivity analysis for volcanic source modeling quality assessment and model selection. Comput. Geosci. 2012, 44, 52–59. [Google Scholar] [CrossRef]
- Baklouti, M.; Faure, V.; Pawlowski, L.; Sciandra, A. Investigation and sensitivity analysis of a mechanistic phytoplankton model implemented in a new modular numerical tool (Eco3M) dedicated to biogeochemical modelling. Prog. Oceanogr. 2006, 71, 34–58. [Google Scholar] [CrossRef]
- Bierman, V.J.; James, R.T. A preliminary modeling analysis of water quality in Lake Okeechobee, Florida: Diagnostic and sensitivity analyses. Water Res. 1995, 29, 2767–2775. [Google Scholar] [CrossRef]
- Omlin, M.; Reichert, P.; Forster, R. Biogeochemical model of Lake Zürich: Model equations and results. Ecol. Model. 2001, 141, 77–103. [Google Scholar] [CrossRef]
- Chu, P.C.; Ivanov, L.M.; Margolina, T.M. On non-linear sensitivity of marine biological models to parameter variations. Ecol. Model. 2007, 206, 369–382. [Google Scholar] [CrossRef]
- Fragoso, J.C.R.; Marques, D.M.L.M.; Collischonn, W.; Tucci, C.E.M.; Nes, E.H.V. Modelling spatial heterogeneity of phytoplankton in Lake Mangueira, a large shallow subtropical lake in South Brazil. Ecol. Model. 2008, 219, 125–137. [Google Scholar] [CrossRef]
- Arhonditsis, G.B.; Brett, M.T. Eutrophication model for Lake Washington (USA): Part I. Model description and sensitivity analysis. Ecol. Model. 2005, 187, 140–178. [Google Scholar] [CrossRef]
- Guven, B.; Howard, A. Identifying the critical parameters of a cyanobacterial growth and movement model by using generalised sensitivity analysis. Ecol. Model. 2007, 207, 11–21. [Google Scholar] [CrossRef]
- Yi, X.; Zou, R.; Guo, H. Global sensitivity analysis of a three-dimensional nutrients-algae dynamic model for a large shallow lake. Ecol. Model. 2016, 327, 74–84. [Google Scholar] [CrossRef]
- Estrada, V.; Diaz, M.S. Global sensitivity analysis in the development of first principle-based eutrophication models. Environ. Model. Softw. 2010, 25, 1539–1551. [Google Scholar] [CrossRef]


| Parameter | Description | Value | Unit | Selected to GSA (Y/N) |
|---|---|---|---|---|
| Maximum phytoplankton growth rate | 3.039 | day−1 | Y | |
| Lower optimum temperature for algal growth | 24 | °C | Y | |
| Upper optimum temperature for algal growth | 29 | °C | Y | |
| Light extinction coefficient for all absorption components (except algae) | 1 | m−1 | Y | |
| Factor for light extinction coefficient for algae | 0.01 | m−1 mmolC−1 | N | |
| Optimum light intensity | 80.0 | W m−2 | Y | |
| Nitrate half saturation constant for algae | 0.040 | mmolN m−3 | N | |
| Ammonia half saturation constant for algae | 0.030 | mmolN m−3 | N | |
| Phosphate half saturation constant for algae | 0.285 | mmolP m−3 | Y | |
| Silica half saturation constant for algae | 1.16 | mmolSi m−3 | N | |
| Phytoplankton linear mortality rate | 0.335 | day−1 | Y | |
| Phytoplankton second order mortality rate | 0.001 | mmolC day−1 | Y | |
| Phytoplankton excretion rate | 0.15 | day−1 | N | |
| Phytoplankton basal respiration rate | 0.2 | day−1 | Y | |
| Phytoplankton active respiration rate | 0.1 | day−1 | N | |
| Oxygen critical concentration for nitrification | 11.161 | mmolO2 m−3 | Y | |
| Oxygen confinement factor for nitrification | 6.0 | -- | N | |
| Detritus remineralization rate | 0.127 | day−1 | Y | |
| Temperature confinement factor for remineralization | 20.0 | -- | N | |
| Reference temperature for remineralization | 13.0 | °C | N | |
| Nitrification rate | 0.045 | day−1 | N | |
| Denitrification rate | 0.01 | mmolN m−3 day−1 | N | |
| Detritus half saturation constant | 6.625 | mmolC m−3 | N | |
| Denitrification ratio of detritus | 1.25 | -- | N | |
| Ammonia release ratio for denitrification | 0.189 | -- | N | |
| Phosphate release ratio for denitrification | 0.012 | -- | N | |
| Silica release ratio for denitrification | 0.259 | -- | N | |
| Redfield ratio P:C | 1:106 | -- | N | |
| Redfield ratio N:C | 16:106 | -- | N | |
| Redfield ratio Si:C | 22:106 | -- | N | |
| Stoichiometric number of carbon to oxygen | 1 | mmolO2 mmolC−1 | N | |
| Stoichiometric number of nitrogen to oxygen | 2 | mmolO2 mmolN−1 | N |
| Symbol | Description |
|---|---|
| N | Sample size |
| k | Number of factors |
| Xi | Generic factor |
| X | N × k matrix of input factors |
| N × (k − 1) matrix of all factors but Xi | |
| , | Variance or mean of argument (·) taken over Xi |
| , | Variance or mean of argument (·) taken over all factors but Xi |
| Condition | Description |
|---|---|
| 0.8 ≤ Sti ≤ 1 | Very important |
| 0.5 ≤ Sti < 0.8 | Important |
| 0.3 ≤ Sti < 0.5 | Unimportant |
| 0 ≤ Sti < 0.3 | Irrelevant |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cheng, Y.; Li, Y.; Ji, F.; Wang, Y. Global Sensitivity Analysis of a Water Quality Model in the Three Gorges Reservoir. Water 2018, 10, 153. https://doi.org/10.3390/w10020153
Cheng Y, Li Y, Ji F, Wang Y. Global Sensitivity Analysis of a Water Quality Model in the Three Gorges Reservoir. Water. 2018; 10(2):153. https://doi.org/10.3390/w10020153
Chicago/Turabian StyleCheng, Yao, Yajun Li, Fei Ji, and Yuchun Wang. 2018. "Global Sensitivity Analysis of a Water Quality Model in the Three Gorges Reservoir" Water 10, no. 2: 153. https://doi.org/10.3390/w10020153
APA StyleCheng, Y., Li, Y., Ji, F., & Wang, Y. (2018). Global Sensitivity Analysis of a Water Quality Model in the Three Gorges Reservoir. Water, 10(2), 153. https://doi.org/10.3390/w10020153






